Received 16 January 2015; accepted 8 February 2015; published 13 February 2015

ABSTRACT

In the paper, the homoclinic (hateroclinic) breather limit method (HBLM) is applied to seek rogue wave solution of the Benjamin Ono equation. We find that the rational breather wave solution is just a rogue wave solution. This result shows that rogue wave can come from the extreme behavior of the breather solitary wave for (1+1)-dimensional nonlinear wave fields.

Keywords:

Benjamin Ono Equation, Extended Homoclinic Test Method, Homoclinic (Hateroclinic) Breather Limit Method, Rogue Wave Solution

1. Introduction

As is well known that solitary wave solutions of nonlinear evolution equations play an important role in nonlinear science fields, especially in nonlinear physical science, since they can provide much physical information and more insight into the physical aspects of the problem and thus lead to further applications [1] . In this paper, we will consider the Benjamin Ono (BO) equation

where 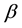and 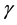are non-zero constants. The BO equation is one of the important nonlinear model in physics [2] [3] . By means of traveling wave method, the exact solutions of the BO equation were obtained. Using the F-expansion method and the Jacobi elliptic function expansion method to the BO equation, a series of periodic wave solutions were got [4] . Based on an improved projective Riccat equation method, the traveling wave solutions of single variable were found [5] . Applying the bilinear method and extended homoclinic test approach [6] -[10] , periodic solitary wave and doubly periodic solutions for the BO equation were obtained [11] .

In recent years, rogue waves, as a special type of nonlinear waves and also known as freak waves, monster waves, killer waves, extreme waves, abnormal waves [12] , have triggered much interest in various physical branches. Rouge wave is a kind of wave that seems abnormal which is first served in the deep ocean. It always has two to three times amplitude higher than its surrounding waves and generally forms in a short time for which people think that it comes from nowhere. Rouge waves have been the subject of intensive research in oceanography [13] [14] , optical fibers [15] -[17] , superfluids [18] , Bose-Einstein condensates, financial markets and other related fields [19] -[22] . In this work, we will apply the homoclinic (hateroclinic) breather limit method (HBLM) [23] , to seek rogue wave solution of the BO equation. We take the following four steps:

Step 1

By Painleve analysis, a transformation is made for some new and unknown function f.

Step 2

By using the transformation in step 1, original equation can be converted into Hirota’s bilinear form , where the D-operator [24] is defined by

Step 3

Solve the above equation to get homoclinic (heteroclinic) breather wave solution by using extended homoclinic test approach (EHTA) [25] .

Step 4

Let the period of periodic wave go to infinite in homoclinic (heteroclinic) breather wave solution, we can Obtain a rational homoclinic (heteroclinic) wave and this wave is just a rouge wave.

2. Rational Breather Wave (Rogue Wave)

The BO equation,

(1)

By Painleve analysis, let

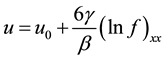(2)

where 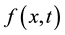is unknown real function, and is the small perturbation parameter. Substituting (2) into (1) will get the following equation:

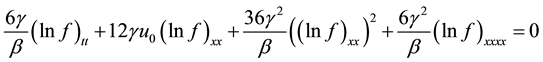(3)

By means of the hirota bilinear operator, which is defined by

(4)

we will get

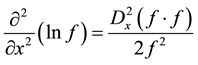(5)

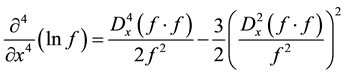(6)

Putting (5) (6) into (3) implies the following bilinear equation:

(7)

In this case we choose extended homoclinic test function

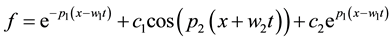(8)

where p₁, p₂, w₁, w₂, c₁ and c₂ are real constants to be determined.

Substituting Equation (8) into (7), collecting coefficients of the terms, , , and the constant, and let coefficients of these terms to zero, we get an algebraic equation

(9)

Solving Equation (9), then taking, we have

(10)

where w₁, w₂, c₂ are some free real constants. Choosing and, we get from(10).

Substituting (10) into (8), we get

(11)

where, ,. Substituting (11) into (2) yields the solutions of (1) as follows, respectively

(12)

(13)

The solution (or) shows a new family of two-wave, breather solitary wave, which is a solitary wave and also is a periodic wave.

Substituting into the solution, it can be rewritten as follows

(14)

where (see Figure 1).

Now we consider a limit behavior of as the period of periodic wave goes to infinite, i.e.. By computing, we get the following result

(15)

where, and and as (see Figure 2).

Especially, if let, we will get, so the two breather wave solution can not be obtained, meanwhile, the rational breather wave solution (rogue wave solution) can’t also be find. The small perturbation parameter plays a huge part in finding rouge wave solution.

Equation (15) is a rational solution of Equation (1), and it is also a breather-type solution. for fixed t as. So, the solution is a rogue wave solution which has two to three times amplitude higher than its surrounding waves and forms in a short time. One may think that whether the energy collection and superposition of breather solitary wave in many periods lead to a rogue wave or not.

3. Conclusion

In the paper, we apply the homoclinic (hateroclinic) breather limit method (HBLM) to find the BO equation’s breather solitary solution and rational breather solution. Meanwhile, rational breather solution obtained here is just a rogue wave solution of the BO equation. Furthermore, the small perturbation parameter u₀ plays an important role in seeking rouge wave solution too. Next, we will try to use some methods to look for multi-rogue waves, such as the two-order wronskian determinant, Darboux transformation and so on.

Acknowledgements

The authors are grateful to the referee for a number of helpful suggestions to improve the paper.

References