World Journal of Condensed Matter Physics
Vol.06 No.02(2016), Article ID:66472,8 pages
10.4236/wjcmp.2016.62012
Application of Electrodynamic Theory on Quantum Hall Effect
Saleem Iqbal1, Muhammad Zafar2, Farhana Sarwar1,3, Syed Mohsin Raza4, Muhammad Afzal Rana2
1Department of Mathematics, University of Balochistan, Quetta, Pakistan
2Department of Basic Sciences, Riphah International University, Islamabad, Pakistan
3Department of Mathematics, F. G. Girls Degree College, Quetta, Pakistan
4Department of Physics, University of Balochistan, Quetta, Pakistan

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 9 February 2016; accepted 13 May 2016; published 16 May 2016
ABSTRACT
The quantum electrodynamic (QED) behaviour is studied for quantum Hall effect (QHE). Quantum theory with conjecture of fractional charge quantization (quantum dipole moment), eigenfunctions for fractional charge quantization at the surface of a twisted and twigged electron quanta and above its surface, fractional Fourier transform and Hermite function for fractional charge quantization is developed. With energy eigen value equation for QHE and with energy operator on an eigenfunction of a twisted and twigged electron quanta, the corresponding eigenfunctions are normalized with Schrodinger’s quantum wave mechanical equation for electric scalar and magnetic potentials, respectively (QED behavior). The fractional electric and magnetic fields with their corresponding potentials for the quantized fractional states in semiconducting hereto structures are theoretically calculated. Such mathematical expressions are in good agreement with experimental results of Nobel Prize winning scientists Klitzing, Haroche, Peter and Gruebber. Our results can also explain the hybridized states of orbits with emphasis on sigma and pi bonding and their corresponding antibonding orbitals as a manifestation of electrophilic and nucleophilic chemical reactions.
Keywords:
Fractional Charge Quantization, Fractional Fourier Transform, Quantum Hall Effect, Quantum Electrodynamics, Electron Quanta String, Twisted and Twigged Electron Quanta

1. Introduction
Von Klitzing in the year 1987 won the Nobel Prize for physics on deciphering quantum Hall effect in heteoro- structures (sandwich like) semi conductors [1] . With this discovery, Van Wees et al. [2] reported “quantum conductance” in a two dimensional electron gas of GaAs-AlGaAs heteorostructure. Serge Haroche and David J. Wineland produced exciting experimental results on “individual quantum systems”, without destroying the particle or quanta which led for them the Nobel prize winning award in physics 2012 “for ground-breaking experimental methods that enable measuring and manipulation of individual quantum systems” [3] [4] . To our understanding, the cavity quantum electrodynamics is a manifestation of fractional charge quantization or quantum dipole or quantum multi-pole moments [5] - [7] . We recently proposed with our conjuncture of fractional charge quantization, the measuring techniques of quantum behaviour for electric resistivity, dielectricity, giant magneto resistances (GMR) [8] , Hall effect and conductance [9] .
A new theory describing how charge being a constant physical entity on an electron in the momentum space is fractionally quantized, is presented [10] [11] . The eigen functions for a free electron quanta, twisting and twigging effects, at the surface, above the surface and inside the surface for the resuting electron quanta sring and twigs (sub-quanta) are determined [12] . With theory of fractional charge quantization for a free electron quanta above the surface, the shape of eigen functions, the energy eigen values and the quantum scattering for absorption in the matter are studied [13] . We conjuncture that the twisted and twigged electron quanta which appears in the form of an electron quant string with twigs (sub-quanta) on its lateral surface will be fractionally quantized in heterostructures semi conductors, as a consequence of which, quantum Hall effect due to fractional electric fields is observed with a gap of quantum Hall resistance, i.e., 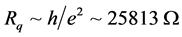 [9] . This is why quantum electrodynamic behaviour of fractionally quantized single or many electrons in the semiconducting heteorostructures is crucial especially for learning the behaviour of electric and magnetic fields, and their corresponding potentials.
[9] . This is why quantum electrodynamic behaviour of fractionally quantized single or many electrons in the semiconducting heteorostructures is crucial especially for learning the behaviour of electric and magnetic fields, and their corresponding potentials.
The magnetoresistance in quantum Hall effect (QHE) is of two types, one is longitudinal and the other is transverse. The longitudinal magnetoresistance is associated with magnetic field parallel to the current. The excitonic quantized Hall state becomes maximum at the total Landau level with vanishing longitudinal component and overwhelming transverse magnetoresistance [14] . This shows that the giant magnetoresistance (GMR) in quantum Hall effect (QHE) is due to transverse component of magnetic fields. There is no electron- electron interaction in quantum Hall effect (QHE). We conjuncture that (GMR) is due to fractionalized electric fields and a manifestation of gyroscopic constant, 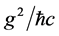 , i.e.,
, i.e., 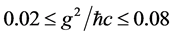 [7] . The QHE is discussed in detail by Gievin and McDonald [15] . The preliminary equations of quantum electrodynamics (QED) in our calculations are considered [16] [17] .
[7] . The QHE is discussed in detail by Gievin and McDonald [15] . The preliminary equations of quantum electrodynamics (QED) in our calculations are considered [16] [17] .
2. Results and Discussions
Saleem Iqbal et al. [9] obtain the eigen functions for a twisted and twigged electron quanta on its surface by using the fractional Fourier ransform (FRFT) of order 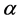 (rotational angle) [18] ,
(rotational angle) [18] ,
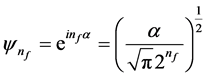 . (1)
. (1)
Equation (1) represents plane wave for a rotation vector 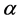 in radians, on the surface of a twisted and twigged electron quanta (almost a quanta electron string), with fractional quantum numbers, i.e.,
in radians, on the surface of a twisted and twigged electron quanta (almost a quanta electron string), with fractional quantum numbers, i.e.,
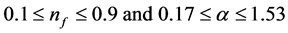 . (2)
. (2)
The Hermite function for the fractional quantum states [9] is
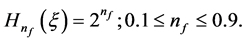 (3)
(3)
The normalized eigenfunction for a twisted and twigged electron quanta above its surface [12] is given below:
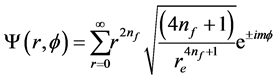 . (4)
. (4)
Equation (4) yields theoretical eigenvalues
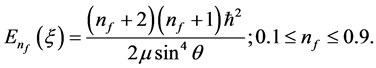 (5)
(5)
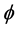 , the azimuthal angle in Equation (4) is related to Gyroscopic constant, where as Equation (5) refers to fractional quantum scattering of energy profiles for reduced mass
, the azimuthal angle in Equation (4) is related to Gyroscopic constant, where as Equation (5) refers to fractional quantum scattering of energy profiles for reduced mass 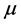 of an electron. The enegy profile at the surface of the twisted and twigged electron quanta is fractionally quantized while the energy above its surface is consumed for quantizing the fractional states and appeared in the form of partial waves. This is experimentally evidenced [5] . For a twisted and twigged electron quanta in semiconductor heteorostructures one would like to know the quantum electrodynamic behaviour, especially in this study for QHE.
of an electron. The enegy profile at the surface of the twisted and twigged electron quanta is fractionally quantized while the energy above its surface is consumed for quantizing the fractional states and appeared in the form of partial waves. This is experimentally evidenced [5] . For a twisted and twigged electron quanta in semiconductor heteorostructures one would like to know the quantum electrodynamic behaviour, especially in this study for QHE.
Rewriting the equation of QHE [9] , we have
 (6a)
(6a)
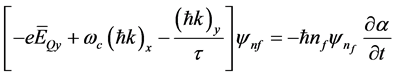 (6b)
(6b)
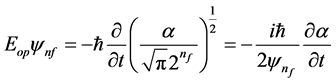 (6c)
(6c)
where 






we know the values of x and y and change the crystal momentum with their corresponding momentum operators. With Hermitian Hamiltonian operator, energy eigen values can be determined. The QED behaviour of a quantized twisted and twigged electron quanta in the semi conductor heteorostructurescan be envisaged with electric fields (fractionally quantized in QHE). Writing the electric filed, 

where 



where 

where 
matter, i.e., 

Equation(8) for dipole radiation yields

The last term in Equation (10) is oscillatory with natural frequency
which is neglected in our calculations. The part of some energy above the surface of the twisted and twigged electron quanta with its equivalent frequency coincides with natural frequency of the sample (resonance or damped oscillation), as a consequence of which, the energy associated with resonance is dissipated in free space where as the remaining energy is used to quantize the twisted and twigged electron quanta [Equation (5) is applicable with Equation (4)]. Equation (10) becomes

In our case, we have fractional electric fields appearing due to fractional charge quantization on twigs (sub- quanta) which are at the lateral surface of an electron string quanta.

Changing the x- and y-components of crystal momentum, 




On comparison of Equations (13) and (14), we have

Equation (15) on rearrangement for 




Equation (17) on simplification yields

Subtracting Equation (16) from itself for both LHS and RHS, we have

Putting Equation (19) in Equation (18) for determining the electric scalar potential due to a quantized twisted and twigged electron quanta in semiconductor heteorostructures, we get on simplification

Equation (20) shows that the scalar electric filed potential is zero for fractional charge quantization, i.e., for QHE
Adding Equation (16) from its self for both LHS and RHS, we get on simplification

which is similar to Equation (19). Thus, the validity of 

On comparison of RHS of Equations (13) and (14), we can put the equality of RHS to the equality of the above expression:


Using Parseval’ formulas,. i.e.,
on Equation (22) where 


The 

Integrating this expression with respect to time

For oscillatory effect in semi conductor hetreostructure the energy is dissipated, i.e.,
becomes negligible. Equation (25) becomes

Equation (26) shows that the magnetic vector potential for fractional quantized states depends on twisting angle 




where
[from Equation (26)]

Equation (27) shows the quantization of fractional electric field for 




where 




Putting Equation (27) in Equation (29), we have

The negative sign shows induced magnetic fields due to twisting and corresponding phase changes (energy changes) on twigs. Neglecting the negative sign, we have

Equation (30) shows that
follows the helical pattern for dipole radiations due to twisted magnetic fields for each of the twigs on the lateral surface of an electron string quanta in semiconducting hetreostructures for QHE. 


3. Conclusion
With conjecture of fractional charge quantization, eigenfunctions for a bounded, stretched, twisted and twigged electron quanta are obtained. The fractional electric and magnetic fields at each of the twigs of a bounded, stretched, and twisted electron quanta, and the corresponding scalar electric and magnetic vector potentials for the quantized fractional states in semiconducting heterostructures are theoretically calculated and explained with obtained mathematical relations. Our results can also explain the hybridized states of orbits with emphasis on sigma and pi bonding and their corresponding antibonding orbitals as a manifestation of electrophilic and nucleophilic chemical reactions.
Cite this paper
Saleem Iqbal,Muhammad Zafar,Farhana Sarwar,Syed Mohsin Raza,Muhammad Afzal Rana,1 1, (2016) Application of Electrodynamic Theory on Quantum Hall Effect. World Journal of Condensed Matter Physics,06,87-94. doi: 10.4236/wjcmp.2016.62012
References
- 1. Klitzing, V. (1987) Quantum Hall Effect in Heterostructure Semiconductors. American Physical Society News Letter.
- 2. Van Wees, B.J., Van Houten, H., et al. (1988) Quantized Conductance of Point Contacts in a Two-Dimensional Electron Gas. Physical Review Letters, 60, 848-850.
http://dx.doi.org/10.1103/PhysRevLett.60.848 - 3. Haroche, S. and Kleppner, D. (1989) Cavity Quantum Electodynamics. Physics Today, 42, 24-30.
http://dx.doi.org/10.1063/1.881201 - 4. Haroche, S. (1995) Manipulating Quantum Fields with a Single Atom in a Cavity. The 7th International Symposium: Resonance Ionization Spectroscopy, 329, 30-35.
http://dx.doi.org/10.1063/1.47571 - 5. Yousaf, S., Raza, S.M., et al. (2008) Absorption of Radiant Energy in Water: A New Conjecture and Theory of Charge Quantization in Chromotized Water Samples. Science International Lahore. Pakistan, 20, 189-195.
- 6. Rehman, F., Raza, S.M., et al. (2009) Quantum Theory of Dielectric and Its Applications to Dolomite of Balochistan, Pakistan. Science International Lahore. Pakistan, 21, 29-32.
- 7. Jabeen, S., Raza, S.M., et al. (2012) Quantum Mechanical Analysis on Faujasite-Type Molecular Sieves by Using Fermi Dirac Statistics and Quantum Theory of Dielectricity. Journal of the Chemical Society of Pakistan, 28, 251-255.
- 8. Fert, A. and Grnberg, P. (2007) Giant Magnetoresistances. APS News Letter, 16, No. 10.
- 9. Iqbal, S., Sarwar, F., et al. (2016) Applications of Quantum Physics on Resistivity, Dielectricity, Giant Magneto resistance, Hall Effect and Conductance. WJCMP, 6.
- 10. Iqbal, S., Sarwar, F., Raza, S.M. and Rehman, A. (2015) How Fractional Charge on an Electron in the Momentum Space is Quantized? ASRJETS, 14, 265-272.
- 11. Iqbal, S. (2012) Analysis and Applications of Fractional Fourier Transform. Unpublished PhD Thesis, University of Balochistan, Quetta, Pakistan.
- 12. Iqbal, S., Sarwar, F. amd Raza, S.M. (2016) Eigenfunctions for a Quantum Wire on a Single Electron at Its Surface and in the Quantum Well with Beaded Fractional Quantized States for the Fractional Charges. WJAMP, 4, 320-327.
- 13. Yousaf, S., Raza, S.M. and Ahmed, M.A. (2008) Newly Developed Recursive Relationship for Fractional Quantum States and Associated Energy Eigen Values. Science International (Lahore), 20, 255-260.
- 14. Kellogg, M., Spielman, I.B., et al. (2002) Observation of Quantized Hall Drag in a Strong Correlated Bilayer Electron System. Physical Review Letter, 88, 126801-126804.
http://dx.doi.org/10.1103/PhysRevLett.88.126804 - 15. Gievin, S.M. and Macdonald, A.H. (1997) Prospective in Quntum Hall Effects. Wiley, New York.
- 16. Gaseoroweiz, S. (2003) Quantum Mechanics. 3rd Edition, Wiley, New York.
- 17. Griffth, D.J. (2005) Introduction to Quantum Mechanics. 2nd Edition, Pearson Education.
- 18. Almeida, L.B. (1994) The Fractional Fourier Transform and Time-Frquency Representations. IEEE Transactions on Signal Processing, 42, 3084-3091.
http://dx.doi.org/10.1109/78.330368
























