Open Journal of Fluid Dynamics
Vol.06 No.04(2016), Article ID:72586,18 pages
10.4236/ojfd.2016.64023
Effect of Magnetic Field on the Flow and Heat Transfer in a Casson Thin Film on an Unsteady Stretching Surface in the Presence of Viscous and Internal Heating
Nalleboyina Vijaya, Kata Sreelakshmi, Ganganapalli Sarojamma
Department of Applied Mathematics, Sri Padmavati Mahila Visvavidyalayam, Tirupati, India

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: November 4, 2016; Accepted: December 4, 2016; Published: December 7, 2016
ABSTRACT
The aim of this investigation is to analyze the effectiveness of Lorentz force, viscous dissipation and internal heating on the heat and flow characteristics of a non-Newt- onian Casson fluid thin film resting on a stretching surface under the influence of a magnetic field. Employing suitable similarity variables and shooting technique and integrating scheme numerical solutions for velocity and temperature are obtained. The results of this analysis are compared with the published work and are found to be in good agreement. The thickness of the thin film is evaluated and is observed that Lorentz force and the non-Newtonian nature of the fluid have a thinning influence on the film. Velocity and temperature distributions in the thin film are discussed for various flow parameters.
Keywords:
Unsteady Stretching Sheet, Casson Thin Film, Viscous Dissipation, Internal Heating

1. Introduction
During the recent years, study of liquid film flows has received significant attention from researchers due to its possible practical applications in several branches of science and technology. The characteristics of flow and heat transfer in a thin film are extremely useful to understand the coating process, design of different heat exchangers and chemical processing equipments. Similar situations prevail in wire and fiber coating, transpiration cooling, food stuff processing, reactor fluidization, manufacture of plastic and rubber sheets. Primary objective of all applications of extrusion is to maintain the surface quality of the final extrudate as best. It is reported that quality of the product depends on rate of heat transfer at the stretching surface. Hence, study of heat transfer under different thermal conditions shall be helpful to industry. Rassoulinejad-Mousavi and Abbasbandy [1] made an analytical study of forced convection in a circular tube filled with a Darcy-Brinkman-Forchheimer porous medium using spectral Homotopy analysis method. They studied the effect of high values of Forchheimer non-linear term on velocity, temperature and Nusselt number. Rassoulinejad-Mousavi et al. [2] examined the temperature and Nusselt number employing non-thermal equilibrium model in a PPC with permeable walls. Shahabeddin et al. [3] studied the effect of embedding aluminium porous metal foam inside the flow channels of an air-cooled lithium-ion battery to improve its thermal management. Several studies on boundary layer flows in a thin film in Newtonian fluids are available. Andersson et al. [4] explored the flow characteristics of an axisymmetric flow in a Newtonian film. A similar investigation in a liquid film on an unsteady stretching sheet was examined by Usha and Sridharan [5] . Wang [6] investigated the flow characteristics in a Newtonian thin liquid film on an unsteady stretching surface. This study was extended by Dandapat et al. [7] to include heat transfer analysis. Dandapat and Ray [8] [9] explored the flow characteristics in a thin liquid film on a rotating disk taking the effect of cooling and thermocapillarity into account. Study of electrically conducting fluid with heat transfer under the influence of a magnetic field has significant applications in several engineering problems such as re-entry of missiles, space crafts, MHD generators, devices in petroleum industry, nuclear reactors, astro physical flows and the boundary layer control. Gailitis and Lielausis [10] mentioned the effects of Lorentz force on controlling flow of an electrically conducting fluids flowing over a flat plate utilizing an external electromagnetic field with ordering electrodes and permanent magnates with alternating polarity and magnetization. Shirazpour et al. [11] investigated the fully developed flow of a viscous fluid in a porous saturated channel under the influence of Lorentz force using the Darcy-Brinkman model. Analytical solutions are obtained employing Homotopy perturbation method. Rassoulinejad-Mousavi et al. [12] made a theoretical study of the temperature and Nusselt number of a conducting Maxwell fluid through a porous saturated parallel plates channel with different boundary conditions using non-thermal equilibrium model. Lin et al. [13] explored the flow and heat transfer of MHD pseudo-plastic nanofluid in a finite film over a stretching surface with internal heating effects.
The effect of viscous dissipation in heat transfer problems is of importance as it plays significant role as an energy source. The presence of viscous dissipation affects both the temperature and heat transfer rates. Rassoulinejad-Mousavi and Hessameddin [14] explored the effect of quadratic drag on viscous dissipation in a fluid saturated porous medium through a channel bounded by two isothermal or isoflux walls. They observed that the Nusselt number is sensitive to the Forchheimer number. The study of viscous heating in thin film flows has considerable interest. For example, in polymer processing flows like injection modelling or extrusion at high rates and aerodynamic heating in the thin layer around a high speed aircraft, the temperature is observed to increase prominently. Vajravelu et al. [15] carried out a mathematical analysis of the effects of thermo physical properties on the thin film flow of an Ostwald-de Waele liquid over a stretching surface in the presence of viscous dissipation. Abel et al. [16] examined the effect of viscous dissipation on the MHD flow and heat transfer in a liquid film due to a stretching surface. Krishna Jyothi et al. [17] analysed the effect of viscous dissipation on the unsteady boundary layer flow of an incompressible, viscous electrically conducting fluid over a sheet that stretches exponentially in the presence of temperature dependent heat source and thermophoresis with chemical reaction. Sarojamma et al. [18] presented a mathematical model to study the effect of viscous dissipation on the time dependent flow of a Casson fluid due to a stretching sheet embedded in a rotating fluid subject to a uniform magnetic field, with thermal radiation and chemical reaction of nth order.
In industry free surface flows of non-Newtonian fluids in thin films are frequently encountered in industry especially in polymer and plastic fabrication and in coating equipment. It is well known that the process of protective coatings on an extrudate use paints or fluids that are generally non-Newtonian in nature. Studies on film flow of power-law fluids have been examined by several researchers [19] [20] [21] [22] .
However, studies on flows in Casson fluid film are very limited in literature. Casson fluid is a non-Newtonian fluid that can be described as a shear thinning fluid having an infinite viscosity at zero shear rates and a yield stress with no flow below this critical yield value. The empirical model was suggested by Casson [23] while analyzing the flow curves of suspensions of pigments in lithographic varnishes. It is reported that Casson’s constitutive equation described accurately the silicon suspensions [24] , suspensions of bentonite in water [25] and many other industrial polymers. It is well established that when blood flows through small vessels its behavior is satisfactorily explained by modeling it as a Casson fluid. It is reported that blood flow affects the thermal response of living tissues. Craciunescu and Clegg [26] studied the oscillatory flow of heat transfer in blood flow considering the vessels of blood as rigid. Weinbaun et al. [27] and Jiji et al. [28] studied the heat transfer under the influence of vascular microstructure on surface tissue. Cavaliere [29] examined the heat supply to tumors in human beings in the extremities by local perfusion with warm blood. Sarojamma et al. [30] explored the characteristics of heat and mass transfer on the MHD boundary layer flow of a Casson fluid over stretching surface under the influence of a magnetic field. Megahed [31] examined the impact of variable heat flux, viscous heating and velocity slip flow on the heat transfer of a Casson fluid in thin film on a stretching sheet.
In this paper, influence of magnetic field on the thin film flow and heat transfer of a Casson fluid over an unsteady stretching sheet is investigated. The effects of viscous dissipation and internal heating are taken into consideration. Application of suitable similarity variables reduced the governing PDEs into a set of coupled non-linear ODEs for which numerical solutions have been obtained. To the best of knowledge of the authors, influence of transverse magnetic field, viscous dissipation and internal heating on the flow and heat transfer of a Casson fluid film over an unsteady stretching has not yet been studied in literature.
2. Mathematical Formulation
Consider a conducting incompressible non-Newtonian Casson thin film over a heated elastic sheet that emerges from a narrow slit at the origin of the Cartesian coordinate system for investigations as shown schematically in Figure 1. A thin film of uniform thickness 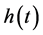 lies on the horizontal stretching sheet. Liquid motion within the film is caused by stretching sheet.
lies on the horizontal stretching sheet. Liquid motion within the film is caused by stretching sheet.
The continuous sheet is parallel to x-axis and moves in its own plane with a velocity
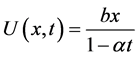 (1)
(1)
where  and
and  are positive constants with dimension per time. The elastic sheet’s temperature
are positive constants with dimension per time. The elastic sheet’s temperature 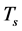 is assumed to vary with the distance x from the slit as
is assumed to vary with the distance x from the slit as
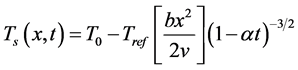 (2)
(2)
where 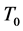 is the temperature at the slit,
is the temperature at the slit, 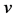 is the kinematic viscocity,
is the kinematic viscocity,  is the con-
is the con-
stant reference temperature such that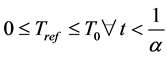 . A transverse magnetic field
. A transverse magnetic field
 is applied to the thin liquid film.
is applied to the thin liquid film.
The constitutive of the Casson fluid can be written as [32]
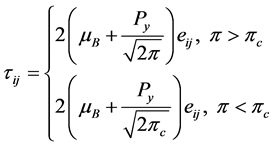 (3)
(3)
where 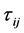 is the
is the 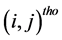 component of the stress tensor,
component of the stress tensor, 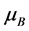 is the plastic dynamic viscosity of the non-Newtonian fluid,
is the plastic dynamic viscosity of the non-Newtonian fluid, 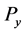 is the yield stress of the fluid,
is the yield stress of the fluid, 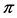 is the product of the component of deformation rate with itself, namely,
is the product of the component of deformation rate with itself, namely, 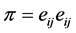 , and
, and 



Figure 1. Physical model and coordinate system.
on non-Newtonian model.
Under these assumptions, the motion of liquid film due to stretching is governed by



where 








The term Q is heat generation (>0) or absorption (<0) per unit volume which is modeled as [33]

where 
The boundary conditions on the stretching sheet are no slip, no penetration and imposed sheet temperature distribution and they are represented respectively as


The following similarity transformation are introduced


Also, 

where prime denotes differentiation with respect to


Subject to the boundary conditions


where 






Since 

Thus, the kinematic constraint at 
The parameters of engineering interest in heat transfer problems are Skin friction coefficient 



where 
The coupled ordinary differential Equations (13) and (14) are non linear and exact analytical solutions are not possible. Equations (13) and (14) with the pertinent boundary conditions (15) and (16) are solved numerically by the most efficient numerical shooting technique with fourth order Runge-Kutta algorithm. First we convert these equations into a set of first order equations as follows:


Corresponding boundary conditions take the form,


Here 






value of 





3. Results and Discussion
The coupled non-linear differential Equations (13) and (14) along with the appropriate boundary conditions (15) and (16) are numerically solved employing the BVP4C technique. In order to have a physical insight of the flow in the thin film, numerical computations of flow variables for various sets of governing parameters have been carried out and graphically presented.
Variation of film thickness versus magnetic field parameter for different values of the unsteadiness parameter is plotted in Figure 2. It may be concluded that film becomes thinner for increasing values of
Behavior of free surface velocity 
Table 1. Comparison of 



unsteadiness parameter is highlighted in Figure 3. It is evident from this figure that free surface velocity remains almost uniform for all values of the magnetic field. As unsteadiness parameter 

Figure 2. Variation of film thickness 
Figure 3. Variation of free surface velocity 
Figure 4 illustrates the influence of magnetic field and unsteadiness on free surface temperature. It is clear that free surface temperature increases as unsteadiness parameter increases. When 

Figure 5 and Figure 6 are the plots of velocity and temperature respectively for a
Figure 4. Variation of free surface temperature 
Figure 5. Velocity profiles for different values of S.
Figure 6. Temperature profiles for different values of S.
variation of unsteadiness parameter S. It is observed that velocity distribution in the film decreases monotonically for small values of unsteadiness parameter. As the unsteadiness parameter takes higher values fluids gets accelerated and hence higher velocities occur. Films become thinner for increasing values of unsteadiness parameter. When unsteadiness parameter S = 1.4, film thickness is reduced by two and half times than that of the film corresponding to S = 0.8. Fluid in the film gets cooled as unsteadiness parameter increases. However, reduction in the temperature is small.
Figure 7 and Figure 8 highlight the impact of strength of magnetic field on the velocity and temperature distributions in the film. In the absence of magnetic field, velocity steadily decreases in the film. Presence of magnetic field causes a rapid reduction of velocity in the vicinity of the boundary due to the action of Lorentz force which resists the fluid flow. Temperature shows an opposite behavior with a gradual enhancement in temperature for increasing values of the magnetic parameter M. For all values of M, temperature assumes its minimum value at the free surface.
Figure 9 and Figure 10 illustrate the influence of non-Newtonian nature of the fluid through the Casson parameter on velocity. It is observed that velocity in the vicinity of the boundary becomes a constant function of the Casson parameter. However, a significant reduction in the velocity in the film is observed away from the boundary for increasing values of


Figure 11 presents the plots of temperature for a variation in Prandtl number. It is revealed that temperature drops from its higher value on the wall to its minimum value
Figure 7. Velocity profiles for different values of M.
Figure 8. Temperature profiles for different values of M.
on the surface. For higher values of Pr, temperature falls rapidly near the boundary. When 


Figure 12 illustrates the impact of Eckert number on temperature. Temperature plots
Figure 9. Velocity profiles for different values of
Figure 10. Temperature profiles for different values of
indicate that increasing values of 


Figure 11. Temperature profiles for different values of Pr.
Figure 12. Temperature profiles fordifferent values of Ec.
in the presence of heat sink 

Surface drag coefficient and Nusselt number on the surface for different variations of
Figure 13. Temperature profiles fordifferent values of
the flow parameters are tabulated in Table 2. Skin friction coefficient is found to enhance with elapse of time. Rate of heat transfer at the boundary is seen to decrease with increase in the unsteadiness parameter which is in conformity with the behavior of the temperature with unsteadiness parameter. Increasing values of Casson parameter reduce surface drag marginally due to smaller velocities. Rate of heat transfer is found to be small for variation in Casson parameter. Due to stronger magnetic fields the surface drag coefficient is significantly reduced. The Lorentz force is observed to favour the heat transfer. Table 3 presents the Nusselt number for different values of Pr, Ec and
4. Conclusions
In this investigation, effect of viscous dissipation and velocity slip on the characteristics of flow and heat transfer in a thin Casson liquid film resting on an unsteady stretching sheet under the influence of a uniform transverse magnetic field and thermal radiation is studied. The partial differential equations governing the flow and energy are transformed into a set of ordinary differential equations by employing appropriate similarity transformations. These ODEs are later solved numerically using shooting technique along with the efficient Runge-Kutta method. Results of the present analysis are validated with available data in literature and are found to be in excellent agreement. The following are some of the salient features of the investigation:
Ø Film thickness is found to decrease as unsteady parameter increases. Qualitatively
Table 2. Variation of 


Table 3. Variation of 

similar behavior is noticed for increasing values of magnetic field and Casson parameters.
Ø Free surface velocity is found to be a decreasing function of the magnetic field parameter.
Ø Temperature distribution in the thin film is enhanced with increase in magnetic field strength and non-Newtonian nature of fluid.
Ø Viscous heating enhances rate of heat transfer.
Acknowledgements
The authors express their sincere thanks to the editor and referee for their valuable comment and suggestions, which lead to a significant improvement of the paper.
Cite this paper
Vijaya, N., Sreelakshmi, K. and Sarojamma, G. (2016) Effect of Magnetic Field on the Flow and Heat Transfer in a Casson Thin Film on an Unsteady Stretching Surface in the Presence of Viscous and Internal Heating. Open Journal of Fluid Dynamics, 6, 303-320. http://dx.doi.org/10.4236/ojfd.2016.64023
References
- 1. Rassoulinejad-Mousavi, S.M. and Abbasbandy, S. (2011) Analysis of Forced Convection in a Circular Tube Filled with a Darcy-Brinkman-Forchheimer Porous Medium Using Spectral Homotopy Analysis Method. Journal of Fluids Engineering, 133, 101207.
https://doi.org/10.1115/1.4004998 - 2. Rassoulinejad-Mousavi, S.M., Hamid, R.S. and Abbasbandy, S. (2013) Heat Transfer through a Porous Saturated Channel with Permeable Walls Using Two-Equation Energy Model. Journal of Porous Media, 16, 241-254. https://doi.org/10.1615/JPorMedia.v16.i3.60
- 3. Shahabeddin, K.M., Rassoulinejad-Mousavi, S.M. and Zhang, Y. (2015) Thermal Management Improvement of an Air-Cooled High-Power Lithium-Ion Battery by Embedding Metal Foam. Journal of Power Sources, 296, 305-313. https://doi.org/10.1016/j.jpowsour.2015.07.056
- 4. Andersson, H.I., Aarseth, J.B., Braud, N. and Dandapat, B.S. (1996) Flow of a Power-Law Fluid Film on an Unsteady Stretching Surface. Journal of Non-Newtonian Fluid Mechanics, 62, 1-8.
https://doi.org/10.1016/0377-0257(95)01392-X - 5. Usha, R. and Sridharan, R. (1993) On the Motion of a Liquid Film on an Unsteady Stretching Surface. ASME Fluids Engineering, 150, 43-48.
- 6. Wang, C.Y. (1990) Liquid Film on an Unsteady Stretching Surface. Quarterly of Applied Mathematics, 48, 601-610.
- 7. Dandapat, B.S., Andersson, H.I. and Aarseth, J.B. (2000) Heat Transfer in a Liquid Film on an Unsteady Stretching Surface. International Journal of Heat and Mass Transfer, 43, 69- 74.
https://doi.org/10.1016/S0017-9310(99)00123-4 - 8. Dandapat, B.S. and Ray, P.C. (1990) Film Cooling on a Rotating Disk. International Journal of Non-Linear Mechanics, 25, 569-582. https://doi.org/10.1016/0020-7462(90)90019-6
- 9. Dandapat, B.S. and Ray, P.C. (1994) The Effect of Thermocapillarity on the Flow of a Thin Film on a Rotating Disc. Journal of Physics D: Applied Physics, 27, 2041-2045.
https://doi.org/10.1088/0022-3727/27/10/009 - 10. Gailitis, A. and Lielausis, O. (1961) On a Possibility to Reduce the Hydrodynamic Resistance of a Plate in Aelectro-Lyte. Applied Magnetohydrodynamics, 12, 143-146.
- 11. Shirazpour, A., Rassoulinejad-Mousavi, S.M. and Hamid, R.S. (2011) HPM Solution of Momentum Equation for Darcy-Brinkman Model in a Parallel Plates Channel Subjected to Lorentz Force. Int. J. Mechanical, Aerospace, Mechatronic and Manufacturing Engineering, 5, 258-262.
- 12. Rassoulinejad-Mousavi, S.M., Abbasbandy, S. and Alsulami, H.H. (2014) Analytical Flow of a Conducting Maxwell Fluid through a Porous Saturated Channel at Various Wall Boundary Conditions. European Physical Journal Plus, 129, 181.
https://doi.org/10.1140/epjp/i2014-14181-4 - 13. Lin, Y., Zheng, L., Zhang, X., Ma, L. and Chen, G. (2015) MHD Pseudo-Plastic Nanofluid Unsteady Flow and Heat Transfer in a Finite Thin Film over Stretching Surface with Internal Heat Generation. International Journal of Heat and Mass Transfer, 84, 903-911.
https://doi.org/10.1016/j.ijheatmasstransfer.2015.01.099 - 14. Rassoulinejad-Mousavi, S.M. and Hessameddin, Y. (2014) Effect of Non-Linear Drag Term on Viscous Dissipation in a Fluid Saturated Porous Medium Channel with Various Boundary Conditions at Walls. Arabian Journal for Science and Engineering, 39, 1231-1240.
https://doi.org/10.1007/s13369-013-0676-0 - 15. Vajravelu, K., Prasad K.V. and Raju, B.T. (2013) Effects of Variable Fluid Properties on the Thin Film Flow of Ostwald-de Waele Fluid over a Stretching Surface. Journal of Hydrodynamics, 25, 10-19. https://doi.org/10.1016/S1001-6058(13)60333-9
- 16. Abel, M.S., Mahesha, N. and Tawade, J. (2009) Heat Transfer in a Liquid Film over an Unsteady Stretching Surface with Viscous Dissipation in Presence of External Magnetic Field. Applied Mathematical Modelling, 33, 3430-3441. https://doi.org/10.1016/j.apm.2008.11.021
- 17. Krishna Jyothi, P., Sreelakshmi, K., Nagendramma, V. and Sarojamma, G. (2015) Study of Thermophoresis on the MHD Flow Due to an Exponentially Stretching Sheet in the Presence of Viscous Dissipation. Procedia Engineering, 127, 340-346.
https://doi.org/10.1016/j.proeng.2015.11.379 - 18. Sarojamma, G., Sreelakshmi, K. and Vasundhara, B. (2016) Mathematical Model of MHD Unsteady Flow Induced by a Stretching Surface Embedded in a Rotating Casson Fluid with Thermal Radiation. 978-9-3805-4421-2/16/$31.00_c 2016 IEEE, 1590-1595.
- 19. Andersson, H.I. and Irgens, F. (1988) Gravity-Driven Laminar Film Flow of Power-Law Fluids along Vertical Walls. Journal of Non-Newtonian Fluid Mechanics, 27, 153-172.
https://doi.org/10.1016/0377-0257(88)85011-0 - 20. Wang, C. and Pop, I. (2006) Analysis of the Flow of a Power-Law Fluid Film on an Unsteady Stretching Surface by Means of Homotopy Analysis Method. Journal of Non-Newtonian Fluid Mechanics, 138, 161-172. https://doi.org/10.1016/j.jnnfm.2006.05.011
- 21. Mahmoud, M.A.A. and Megahed, A.M. (2009) MHD Flow and Heat Transfer in a Non- Newtonian Liquid Film over an Unsteady Stretching Sheet with Variable Fluid Properties. Canadian Journal of Physics, 87, 1065-1071. https://doi.org/10.1139/P09-066
- 22. Chen, C.H. (2006) Effect of Viscous Dissipation on Heat Transfer in a Non-Newtonian Liquid Film over an Unsteady Stretching Sheet. Journal of Non-Newtonian Fluid Mechanics, 135, 128-135.
https://doi.org/10.1016/j.jnnfm.2006.01.009 - 23. Casson, N. (1959) A Flow Equation for Pigment Oil Suspensions of Printing Ink Type. In: Mil, C.C., Ed., Rheology of Dispersed System, Pergamon Press, Oxford, 84-102.
- 24. Walawander, W.P., Chen, T.Y. and Cala, D.F. (1975) An Approximate Casson Fluid Model for Tube Flow of Blood. Biorheology, 12, 111-119.
- 25. Tamamashi, B. (1968) Consideration of Certain Haemorheological Phenomenon from the Stand-Point of Surface Chemistry. In: Copley, A.L., Ed., Haemorheology, Pergamon Press, London, 89.
- 26. Craciunescu, O.I. and Clegg, S.T. (2001) Pulsatile Blood Flow Effects on Temperature Distribution and Heat Transfer in Rigid Vessels. Journal of Biomechanical Engineering, 123, 500-505. https://doi.org/10.1115/1.1392318
- 27. Weinbaun, S., Jiji, L.M. and Lemons, D.E. (1984) Theory and Experiment for the Effect of Vascular Microstructure on Surface Tissue Heat Transfer—Part I: Anatomical Foundation and Model Conceptualization. Journal of Biomechanical Engineering, 106, 321-330.
https://doi.org/10.1115/1.3138501 - 28. Jiji, L.M., Weinbaun, S. and Lemons, D.E. (1984) Theory and Experiment for the Effect of Vascular Microstructure on Surface Tissue Heat Transfer—Part II: Model Formulation and Solution. Journal of Biomechanical Engineering, 106, 331-341.
https://doi.org/10.1115/1.3138502 - 29. Cavaliere, R., Ciocatto, E.C., Giovannela, B.C., Heidelberger, C., Hojnson, R.O., Martotini, M., Rmondovi, B., Moricca, G. and Rossi-Fanelli, A. (1967) Selective Heat Sensitivity of Cancer Cells: Biochemical and Clinical Studies. Cancer, 20, 1351-1381.
https://doi.org/10.1002/1097-0142(196709)20:9<1351::AID-CNCR2820200902>3.0.CO;2-# - 30. Sarojamma, G., Ramana, B. and Vendabai, K. (2014) Heat and Mass Transfer on MHD Boundary Layer Flow of a Chemically Reacting Non-Newtonian Fluid over a Stretching Sheet with Suction. International Journal of Engineering Sciences & Research Technology, 3, 197-205.
- 31. Megahed, A.M. (2015) Effect of Slip Velocity on Casson Thin Film Flow and Heat Transfer Due to Unsteady Stretching Sheet in Presence of Variable Heat Flux and Viscous Dissipation. Applied Mathematics and Mechanics, 36, 1273-1284. https://doi.org/10.1007/s10483-015-1983-9
- 32. Eldabe, N.T.M. and Salwa, M.G.E. (1995) Heat Transfer of MHD Non-Newtonian Casson Fluid Flow between Two Rotating Cylinders. Journal of the Physical Society of Japan, 64, 41-64.
- 33. Aziz, R.C., Hashim, I. and Alomari, A.K. (2011) Thin Film Flow and Heat Transfer on an Unsteady Stretching Sheet with Internal Heating. Meccanica, 46, 349-357.
https://doi.org/10.1007/s11012-010-9313-0 - 34. Abel, M.S., Tawade, J. and Nandeppanavar, M.M. (2009) Effect of Non-Uniform Heat Source on MHD Heat Transfer in a Liquid Film over an Unsteady Stretching Sheet. International Journal of Non-Linear Mechanics, 44, 990-998. https://doi.org/10.1016/j.ijnonlinmec.2009.07.004















