Open Journal of Discrete Mathematics
Vol.06 No.03(2016), Article ID:68338,6 pages
10.4236/ojdm.2016.63015
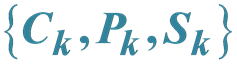 -Decompositions of Balanced Complete Bipartite Multigraphs
-Decompositions of Balanced Complete Bipartite Multigraphs
Jenq-Jong Lin1, Min-Jen Jou2
1Department of Finance, Ling Tung University, Taiwan
2Department of Information Technology, Ling Tung University, Taiwan

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 16 June 2016; accepted 11 July 2016; published 14 July 2016
ABSTRACT
Let  be a family of subgraphs of a graph G. An L-decomposition of G is an edge- disjoint decomposition of G into positive integer
be a family of subgraphs of a graph G. An L-decomposition of G is an edge- disjoint decomposition of G into positive integer 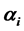 copies of
copies of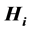 , where
, where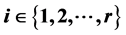 . Let
. Let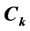 ,
, 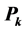 and
and 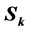 denote a cycle, a path and a star with k edges, respectively. For an integer
denote a cycle, a path and a star with k edges, respectively. For an integer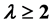 , we prove that a balanced complete bipartite multigraph
, we prove that a balanced complete bipartite multigraph  has a
has a 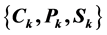 -decomposition if and only if k is even,
-decomposition if and only if k is even, 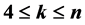 and
and .
.
Keywords:
Balanced Complete Bipartite Multigraph, Cycle, Path, Star, Decomposition

1. Introduction
Let F, G and H be graphs. A G-decomposition of F is a partition of the edge set of F into copies of G. If F has a G-decomposition, we say that F is G-decomposable. Let 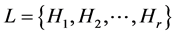 be a family of subgraphs of a graph G. An L-decomposition of G is an edge-disjoint decomposition of G into positive integer
be a family of subgraphs of a graph G. An L-decomposition of G is an edge-disjoint decomposition of G into positive integer 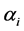 copies of
copies of![]() , where
, where![]() . If G has an L-decomposition, we say that G is L-decomposable.
. If G has an L-decomposition, we say that G is L-decomposable.
For positive integers m and n, ![]() denotes the complete bipartite graph with parts of sizes m and n. A complete bipartite graph is balanced if
denotes the complete bipartite graph with parts of sizes m and n. A complete bipartite graph is balanced if![]() . A k-cycle, denoted by
. A k-cycle, denoted by![]() , is a cycle of length k. A k-star, denoted by
, is a cycle of length k. A k-star, denoted by![]() , is the complete bipartite graph
, is the complete bipartite graph![]() . A k-path, denoted by
. A k-path, denoted by![]() , is a path with k edges. For a graph G and an integer
, is a path with k edges. For a graph G and an integer![]() , we use
, we use ![]() to denote the multigraph obtained from G by replacing each edge e by
to denote the multigraph obtained from G by replacing each edge e by ![]() edges each of which has the same ends as e.
edges each of which has the same ends as e.
Decompositions of graphs into k-stars have also attracted a fair share of interest (see [1] - [3] ). Articles of ![]() - decompositions of interest include [4] [5] . Decompositions of some families of graphs into k-cycles have been a popular topic of research in graph theory (see [6] [7] for surveys of this topic). The study of
- decompositions of interest include [4] [5] . Decompositions of some families of graphs into k-cycles have been a popular topic of research in graph theory (see [6] [7] for surveys of this topic). The study of ![]() -decom- position was introduced by Abueida and Daven in [8] . Abueida and Daven [9] investigated the problem of
-decom- position was introduced by Abueida and Daven in [8] . Abueida and Daven [9] investigated the problem of ![]() -decomposition of the complete graph
-decomposition of the complete graph![]() . Abueida and O’Neil [10] settled the existence problem for
. Abueida and O’Neil [10] settled the existence problem for ![]() -decomposition of the complete multigraph
-decomposition of the complete multigraph ![]() for
for![]() . In [11] , Priyadharsini and Muth- usamy gave necessary and sufficient conditions for the existence of a
. In [11] , Priyadharsini and Muth- usamy gave necessary and sufficient conditions for the existence of a ![]() -factorization of
-factorization of ![]() where
where![]() . Furthermore, Shyu [12] investigated the problem of decomposing
. Furthermore, Shyu [12] investigated the problem of decomposing ![]() into paths and stars with k edges, giving a necessary and sufficient condition for
into paths and stars with k edges, giving a necessary and sufficient condition for![]() . In [13] , Shyu considered the existence of a decomposition of
. In [13] , Shyu considered the existence of a decomposition of ![]() into paths and cycles with k edges, giving a necessary and sufficient condition for
into paths and cycles with k edges, giving a necessary and sufficient condition for![]() . Shyu [14] investigated the problem of decomposing
. Shyu [14] investigated the problem of decomposing ![]() into cycles and stars with k edges, settling the case
into cycles and stars with k edges, settling the case![]() . Recently, Lee [15] [16] established necessary and sufficient conditions for the existence of a
. Recently, Lee [15] [16] established necessary and sufficient conditions for the existence of a ![]() -decomposition of a complete bipartite graph and
-decomposition of a complete bipartite graph and ![]() -decomposition of a balanced complete bi- partite graph. Lin and Jou [17] investigated the problems of the
-decomposition of a balanced complete bi- partite graph. Lin and Jou [17] investigated the problems of the ![]() -decomposition of the balanced complete bipartite graph
-decomposition of the balanced complete bipartite graph![]() . It is natural to consider the problem of the
. It is natural to consider the problem of the ![]() -decomposition of the balanced complete bipartite multigraph
-decomposition of the balanced complete bipartite multigraph ![]() for
for![]() . In this paper, the necessary and sufficient conditions for the existence of such decomposition are given.
. In this paper, the necessary and sufficient conditions for the existence of such decomposition are given.
2. Preliminaries
Let G be a graph. The degree of a vertex x of G, denoted by![]() , is the number of edges incident with x. The vertex of degree k in
, is the number of edges incident with x. The vertex of degree k in ![]() is the center of
is the center of![]() . For
. For ![]() and
and![]() , we use
, we use ![]() and
and ![]() to denote the subgraph of G induced by A and the subgraph of G obtained by deleting B, respectively.
to denote the subgraph of G induced by A and the subgraph of G obtained by deleting B, respectively.
When ![]() are graphs, not necessarily disjoint, we write
are graphs, not necessarily disjoint, we write ![]() or
or ![]() for the graph with vertex set
for the graph with vertex set ![]() and edge set
and edge set![]() . When the edge sets are disjoint,
. When the edge sets are disjoint, ![]()
expresses the decomposition of G into![]() .
. ![]() is the short notation for the union of n copies of disjoint graphs isomorphic to G. Let H be a subgraph of
is the short notation for the union of n copies of disjoint graphs isomorphic to G. Let H be a subgraph of ![]() with vertex set
with vertex set ![]() and edge set
and edge set![]() , and let r be a nonnegative integer. We use
, and let r be a nonnegative integer. We use ![]() to denote the graph with vertex set
to denote the graph with vertex set ![]() and edge set
and edge set ![]() where the subscripts of b are taken modulo n. For any vertex x of a digraph G, the outdegree
where the subscripts of b are taken modulo n. For any vertex x of a digraph G, the outdegree ![]() (respectively, indegree
(respectively, indegree![]() ) of x is the number of arcs incident from (respectively, to) x. A multistar is a star with multiple edges allowed. We use
) of x is the number of arcs incident from (respectively, to) x. A multistar is a star with multiple edges allowed. We use ![]() to denote a multistar with k edges. Let G be a multigraph. The edge-multiplicity of an edge in G is the number of edges joining the vertices of the edge. The multiplicity of G, denoted by
to denote a multistar with k edges. Let G be a multigraph. The edge-multiplicity of an edge in G is the number of edges joining the vertices of the edge. The multiplicity of G, denoted by![]() , is the maximum edge- multiplicity of G.
, is the maximum edge- multiplicity of G.
Lemma 1. ( [3] ) For integers m and n with![]() , the graph
, the graph ![]() has an
has an ![]() -decomposition if and only if
-decomposition if and only if ![]() and
and
![]()
Lemma 2. ( [18] ) Suppose that![]() . Then
. Then ![]() is
is ![]() -decomposable.
-decomposable.
Let ![]() denote the edge ab in the s-th copy
denote the edge ab in the s-th copy ![]() of
of ![]() for
for![]() .
.
Lemma 3. If k is an even integer with![]() , then there exist
, then there exist ![]() edge-disjoint 2k-cycles in
edge-disjoint 2k-cycles in![]() .
.
Proof. A decomposition of ![]() into 2k-cycles is given by the following
into 2k-cycles is given by the following ![]() cycles:
cycles:![]() , where
, where![]() ,
, ![]() and
and![]() . □
. □
Note that ![]() can be decomposed into two copies of k-paths:
can be decomposed into two copies of k-paths:
![]() and
and![]() , that
, that
is, ![]() can be decomposed into
can be decomposed into ![]() copies of k-paths.
copies of k-paths.
Lemma 4. ( [4] ) There exists a ![]() -decomposition of
-decomposition of ![]() if and only if
if and only if![]() , and one of the following (see Table 1) cases occurs.
, and one of the following (see Table 1) cases occurs.
Lemma 5. ( [19] ) For positive integers m, n and k, the graph ![]() has a
has a ![]() -decomposition if and only if
-decomposition if and only if ![]() and k are even,
and k are even, ![]() ,
, ![]() , and
, and![]() .
.
![]()
Table 1. The conditions of a ![]() -decomposition of
-decomposition of![]() .
.
3. Main Results
With the results ( [17] ) of the ![]() -decomposition of the balanced complete bipartite graph
-decomposition of the balanced complete bipartite graph![]() , it is assumed that
, it is assumed that ![]() in the sequel. In this section, we will prove the following result.
in the sequel. In this section, we will prove the following result.
Main Theorem. Let k and n be positive integers. The graph ![]() has a
has a ![]() -decomposition if and only if k is even,
-decomposition if and only if k is even, ![]() and
and![]() .
.
We first give necessary conditions for a ![]() -decomposition of
-decomposition of![]() .
.
Lemma 6. If ![]() has a
has a ![]() -decomposition, then k is even,
-decomposition, then k is even, ![]() and
and![]() .
.
Proof. Since bipartite graphs contain no odd cycle, k is even. In addition, the minimum length of a cycle and the maximum size of a star in ![]() are 4 and n, respectively, we have
are 4 and n, respectively, we have![]() . Finally, the size of each member in the decomposition is k and
. Finally, the size of each member in the decomposition is k and![]() ; thus
; thus![]() . □
. □
Throughout this paper, let ![]() denote the bipartition of
denote the bipartition of![]() , where
, where ![]() and
and![]() . We now show that the necessary conditions are also sufficient. The proof is divided into cases
. We now show that the necessary conditions are also sufficient. The proof is divided into cases![]() ,
, ![]() , and
, and![]() , which are treated in Lemmas 7, 8, and 9, respectively.
, which are treated in Lemmas 7, 8, and 9, respectively.
Lemma 7. For an even integer![]() , then
, then ![]() has a
has a ![]() -decomposition.
-decomposition.
Proof. Note that![]() . By Lemmas 1 and 4,
. By Lemmas 1 and 4, ![]() has a
has a ![]() -decomposition and a
-decomposition and a ![]() -decomposition. In addition, by Lemma 5,
-decomposition. In addition, by Lemma 5, ![]() has a
has a ![]() -decomposition. Hence
-decomposition. Hence ![]() has a
has a ![]() -decomposition. □
-decomposition. □
Lemma 8. Let k be a positive even integer and let n be a positive integer with![]() . If
. If ![]() is divisible by k, then
is divisible by k, then ![]() has a
has a ![]() -decomposition.
-decomposition.
Proof. Let![]() . From the assumption
. From the assumption![]() , we have
, we have![]() . Let
. Let![]() . Since
. Since![]() , we have
, we have![]() , which implies that t is a positive integer. The proof is divided into two cases according to the values of t.
, which implies that t is a positive integer. The proof is divided into two cases according to the values of t.
Case 1.![]() .
.
Let![]() ,
, ![]() ,
,
![]() and
and![]() .
.
Clearly![]() . Note that G is isomorphic to
. Note that G is isomorphic to![]() ,
, ![]() is isomorphic to
is isomorphic to![]() ,
, ![]() is isomorphic to
is isomorphic to ![]() and F is isomorphic to
and F is isomorphic to![]() , which can be decomposed into
, which can be decomposed into ![]() copies of
copies of ![]() by Lemmas 1 and 2. In the following, we will show that
by Lemmas 1 and 2. In the following, we will show that ![]() can be decomposed into
can be decomposed into ![]() copies of
copies of![]() , one copy of
, one copy of ![]() and
and ![]() copies of
copies of![]() .
.
Let![]() , where
, where ![]() and
and![]() . Define a subgraph W of G as follows:
. Define a subgraph W of G as follows:
![]()
and the subscripts of b are taken modulo k. Note that ![]() for t is even, and
for t is even, and
![]() for t is odd, this assures us that there are enough edges for W. Note that a
for t is odd, this assures us that there are enough edges for W. Note that a ![]() can be decomposed into 2 copies of
can be decomposed into 2 copies of![]() . In addition,
. In addition, ![]() for t is even as well as
for t is even as well as ![]() for t is odd, it follows that W can be decomposed into t copies of
for t is odd, it follows that W can be decomposed into t copies of![]() . Since
. Since![]() , we
, we
interchange two edges ![]() in
in ![]() and
and ![]() in
in![]() , then we obtain a new cycle
, then we obtain a new cycle
![]() . Hence
. Hence ![]() can be decomposed into
can be decomposed into ![]()
copies of ![]() and one copy of
and one copy of![]() .
.
Let ![]() be the graph obtained from G by deleting the edges in W. For the case of t is even, we have that
be the graph obtained from G by deleting the edges in W. For the case of t is even, we have that
![]()
The other case of t is odd, we have that
![]()
Let ![]() for
for![]() . Then for t is even
. Then for t is even![]() , and for t is odd
, and for t is odd
![]()
with the center at![]() .
.
In the following, we will show that ![]() can be decomposed into r copies of
can be decomposed into r copies of ![]() with centers in
with centers in![]() , and into k copies of
, and into k copies of ![]() with centers in
with centers in ![]() for t is even as well as k/2 copies
for t is even as well as k/2 copies
of ![]() with centers in
with centers in ![]() and
and ![]() copies of
copies of ![]() with centers in
with centers in ![]() for t
for t
is odd, that is, there exists an orientation of ![]() such that
such that
![]() (1)
(1)
where![]() , and for t is even
, and for t is even
![]() (2)
(2)
where![]() , and for t is odd
, and for t is odd
![]() (3)
(3)
We first consider the edges oriented outward from![]() . If t is even, then the edges
. If t is even, then the edges
![]() are all oriented outward from
are all oriented outward from ![]() where
where![]() . If t is odd, for
. If t is odd, for
![]() , the edges
, the edges ![]() and
and
![]() are all oriented outward from
are all oriented outward from![]() , where the subscripts of b are taken modulo r in the set
, where the subscripts of b are taken modulo r in the set![]() . In both of the cases the subscripts of b are taken modulo r in the set of numbers
. In both of the cases the subscripts of b are taken modulo r in the set of numbers![]() . Since
. Since ![]() for t is even, and
for t is even, and ![]() for t is odd, this assures us that there are enough edges for the above orientation. Finally, the edges which are not oriented yet are all oriented from
for t is odd, this assures us that there are enough edges for the above orientation. Finally, the edges which are not oriented yet are all oriented from ![]() to
to![]() .
.
From the construction of the orientation, it is easy to see that (2) and (3) are satisfied, and for all![]() , we have
, we have
![]() (4)
(4)
So, we only need to check (1).
Since ![]() for
for![]() , it follows from (4) that
, it follows from (4) that ![]()
for![]() . Note that t is even,
. Note that t is even, ![]() , and t is odd,
, and t is odd,
![]()
Thus,
![]()
Therefore ![]() for
for![]() . This proves (1). Hence, there exists the required decomposition
. This proves (1). Hence, there exists the required decomposition ![]() of
of![]() . Let
. Let ![]() be the star with center at
be the star with center at ![]() in
in ![]() for
for![]() . Then
. Then ![]() is an
is an![]() . Since
. Since![]() , by Lemma 2, we obtain that
, by Lemma 2, we obtain that ![]() can be decomposed into
can be decomposed into ![]() copies of
copies of ![]() for
for![]() .
.
Let ![]() be the
be the ![]() -multistar with center at
-multistar with center at ![]() in
in ![]() for
for![]() . Let
. Let
![]() for
for![]() . Then
. Then ![]() is decomposed into
is decomposed into![]() ,
, ![]() ,
,
and each![]() . It follows that
. It follows that![]() . Since
. Since![]() , by Lemma 2, we obtain that
, by Lemma 2, we obtain that ![]() can be decomposed into
can be decomposed into ![]() copies of
copies of ![]() for
for![]() . Recall that
. Recall that ![]() , we have that
, we have that ![]() is
is ![]() -decomposable.
-decomposable.
Case 2.![]() .
.
Let![]() ,
, ![]() ,
,
![]() and
and![]() .
.
Then![]() . By similar arguments as in the proof of Case 1, we have that
. By similar arguments as in the proof of Case 1, we have that ![]() can be decomposed into one copy of
can be decomposed into one copy of ![]() and
and ![]() copies of
copies of![]() . On the other hand, by Lemma 5,
. On the other hand, by Lemma 5, ![]() has a
has a ![]() -decomposition. Hence
-decomposition. Hence ![]() has a
has a ![]() -decomposition. □
-decomposition. □
Lemma 9. Let k be a positive even integer and let n be a positive integer with![]() . If
. If ![]() is divisible by k, then
is divisible by k, then ![]() has a
has a ![]() -decomposition.
-decomposition.
Proof. Let ![]() where q and r are integers with
where q and r are integers with![]() . From the assumption of
. From the assumption of![]() , we have
, we have![]() . Note that
. Note that
![]()
Trivially, ![]() ,
, ![]() and
and ![]() are multiples of k. Thus
are multiples of k. Thus
![]() from the assumption that
from the assumption that ![]() is divisible by k. By Lemmas 1 and 2,
is divisible by k. By Lemmas 1 and 2, ![]() ,
, ![]() and
and ![]() have
have ![]() -decomposition.
-decomposition.
In the case of![]() , by Lemma 7, we obtain that
, by Lemma 7, we obtain that ![]() has a
has a ![]() -decomposition. In addition, by Lemma 8,
-decomposition. In addition, by Lemma 8, ![]() has a
has a ![]() -decomposition for
-decomposition for![]() . Hence there exists a
. Hence there exists a ![]() -de composition of
-de composition of![]() . □
. □
Now we are ready for the main result. It is obtained by combining Lemmas 6, 7, 8 and 9.
Theorem 1. Let k and n be positive integers. The graph ![]() has a
has a ![]() -decomposition if and only if k is even,
-decomposition if and only if k is even, ![]() and
and![]() .
.
Remark. Let m and n be positwe integers with![]() . Since bipartite graphs contain no odd cycle, k is even. In addition, the minimum length of a cycle and the maximum size of a star in
. Since bipartite graphs contain no odd cycle, k is even. In addition, the minimum length of a cycle and the maximum size of a star in ![]() are 4 and m, respectively, we have
are 4 and m, respectively, we have![]() . Moreover, each k-cycle in
. Moreover, each k-cycle in ![]() uses
uses ![]() vertices of each partite set, which implies
vertices of each partite set, which implies
that![]() . Finally, the size of each member in the decomposition is k and
. Finally, the size of each member in the decomposition is k and![]() , thus
, thus
![]() . Hence the obvious necessary conditions for the graph
. Hence the obvious necessary conditions for the graph ![]() to have a
to have a ![]() -de composition are: 1) k is even, 2)
-de composition are: 1) k is even, 2)![]() , and 3)
, and 3)![]() . It is natural to ask whether they are sufficient.
. It is natural to ask whether they are sufficient.
Acknowledgements
The authors are grateful to the referees for the helpful comments.
Cite this paper
Jenq-Jong Lin,Min-Jen Jou, (2016) {Ck, Pk, Sk} -Decompositions of Balanced Complete Bipartite Multigraphs. Open Journal of Discrete Mathematics,06,174-179. doi: 10.4236/ojdm.2016.63015
References
- 1. Tazawa, S. (1985) Decomposition of a Complete Multipartite Graph into Isomorphic Claws. SIAM Journal on Algebraic Discrete Methods, 6, 413-417.
http://dx.doi.org/10.1137/0606043
- 2. Sotteau, D. (1981) Decomposition of Km,n (K*m,n) into Cycles (Circuits) of Length . Journal of Combinatorial Theory, Series B, 30, 75-81.
- 3. Lin, C., Lin, J.J. and Shyu, T.W. (1999) Isomorphic Star Decomposition of Multicrowns and the Power of Cycles. Ars Combinatoria, 53, 249-256.
- 4. Lin, J.J. and Jou, M.J. (2016) {Ck, Pk, Sk}-Decompositions of Balanced Complete Bipartite Graphs. (Submitted)
- 5. Lee, H.C. and Chu, Y.-P. (2013) Multidecompositions of the Balanced Complete Bipartite Graph into Paths and Stars. ISRN Combinatorics, 2013, Article ID: 398473.
http://dx.doi.org/10.1155/2013/398473
- 6. Lee, H.C. (2013) Multidecompositions of Complete Bipartite Graphs into Cycles and Stars. Ars Combinatoria, 108, 355-364.
- 7. Shyu, T.W. (2013) Decomposition of Complete Graphs into Cycles and Stars. Graphs and Combinatorics, 29, 30-313.
http://dx.doi.org/10.1007/s00373-011-1105-3
- 8. Shyu, T.W. (2010) Decompositions of Complete Graphs into Paths and Cycles. Ars Combinatoria, 97, 257-270.
- 9. Shyu, T.W. (2010) Decomposition of Complete Graphs into Paths and Stars. Discrete Mathematics, 310, 2164-2169.
http://dx.doi.org/10.1016/j.disc.2010.04.009
- 10. Priyadharsini, H.M. and Muthusamy, A. (2009) -Multifactorization of . Journal of Combinatorial Mathematics and Combinatorial Computing, 69, 145-150.
- 11. Abueida, A. and O’Neil, T. (2007) Multidecomposition of λKm into Small Cycles and Claws. Bulletin of the Institute of Combinatorics and Its Applications, 49, 32-40.
- 12. Abueida, A. and Daven, M. (2004) Mutidecompositons of the Complete Graph. Ars Combinatoria, 72, 17-22.
- 13. Abueida, A. and Daven, M. (2003) Multidesigns for Graph-Pairs of Order 4 and 5. Graphs and Combinatorics, 19, 433-447.
http://dx.doi.org/10.1007/s00373-003-0530-3
- 14. Lindner, C.C. and Rodger, C.A. (1992) Decomposition in Cycles II: Cycle Systems, In: Dinitz, J.H. and Stinson, D.R., Eds., Contemporary Design Theory: A Collection of Surveys, Wiley, New York, 325-369.
- 15. Bryant, D. and Rodger, C.A. (2007) Cycle Decompositions. In: Colbourn, C.J. and Dinitz, J.H., Eds., The CRC Handbook of Combinatorial Designs, 2nd Edition, CRC Press, Boca Raton, 373-382.
- 16. Shyu, T.W. (2007) Path Decompositions of λKn,n. Ars Combinatoria, 85, 211-219.
- 17. Parker, C.A. (1998) Complete Bipartite Graph Path Decompositions. PhD Dissertation, Auburn University, Auburn.
- 18. Yamamoto, S., Ikeda, H., Shige-Ede, S., Ushio, K. and Hamada, N. (1975) On Claw Decomposition of Complete Graphs and Complete Bipartie Graphs. Hiroshima Mathematical Journal, 5, 33-42.
- 19. Ushio, K., Tazawa, S. and Yamamoto, S. (1978) On Claw-Decomposition of Complete Multipartite Graphs. Hiroshima Mathematical Journal, 8, 207-210.
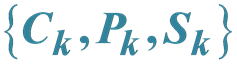 -Decompositions of Balanced Complete Bipartite Multigraphs
-Decompositions of Balanced Complete Bipartite Multigraphs



 be a family of subgraphs of a graph G. An L-decomposition of G is an edge- disjoint decomposition of G into positive integer
be a family of subgraphs of a graph G. An L-decomposition of G is an edge- disjoint decomposition of G into positive integer 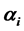 copies of
copies of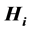 , where
, where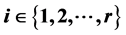 . Let
. Let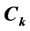 ,
, 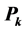 and
and 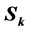 denote a cycle, a path and a star with k edges, respectively. For an integer
denote a cycle, a path and a star with k edges, respectively. For an integer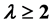 , we prove that a balanced complete bipartite multigraph
, we prove that a balanced complete bipartite multigraph  has a
has a 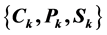 -decomposition if and only if k is even,
-decomposition if and only if k is even, 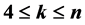 and
and .
.
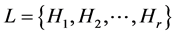 be a family of subgraphs of a graph G. An L-decomposition of G is an edge-disjoint decomposition of G into positive integer
be a family of subgraphs of a graph G. An L-decomposition of G is an edge-disjoint decomposition of G into positive integer 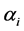 copies of
copies of

































































































































































































































































































































