Paper Menu >>
Journal Menu >>
 Applied Mathematics, 2011, 2, 155-164 doi:10.4236/am.2011.22018 Published Online February 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM Formulation of the Post-Newtonian Equations of Motion of the Restricted Three Body Problem Fawzy A. Abd El-Salam1, Sobhy Abd El-Bar2 1Department of Astronomy, Faculty of Science, Cairo University, Cairo, Egypt 2Department of Mathematics, Faculty of Science, Tanta University, Tanta, Egypt E-mail: f.a.abdelsalam@gmail.com, fawzy_zaher@yahoo.co.uk Received May 15, 201 0; revised November 10, 2010; accepted November 14, 2010 Abstract In the present work the geodesic equation represents the equations of motion of the particles along the geo- desics is derived. The deviation of the curved space-time metric tensor from that of the Minkowski tensor is considered as a perturbation. The quantities is expanded in powers of 2 c . The equations of motion of the relativistic three body problem in the PN formalism are obtained. Keywords: Post Newtonian Approximation, Geodesic Equation, Restricted Three Body Problem 1. Introduction The Three body problem concerns with the motion of a small particle of negligible mass moving under the gravi- tational influence of two massive objects 1 m and 2 m. It’s history began with Euler and Lagrange continues with Jacobi [1], Hill [2], Poincaré [3], and Birkhoff [4]. In 1772, Euler [5] first introduced a synodic (rotating) coordinate system, the use of which led to an integral of the equations of motion, known today as the Jacobian integral. Euler himself did not discover the Jacobia in- tegral which was first given by Jacobi [1] who, as Wint- ner remarks, “rediscovered” the synodic system. The ac- tual situation is somewhat complex since Jacobia pub- lished his integral in a sideral (fixed) system in which its significance is definitely less than in the synodic system. Many authors hope to investigate the relativistic effe- cts in this problem. But unfortunately, the Einstein field equations are nonlinear, and therefore cannot in general be solved exactly. By imposing the symmetry require- ments of time independence and spatial isotropy we are able to find one useful exact solution, the Schwarzsch ild metric, but we cannot actually make use of the full con- tent of this solution, because in fact the solar system is not static and isotropic. Indeed, the Newtonian effects of the planet’s gravita- tional fields are an order of magnitude greater than the first corrections due to general relativity, and completely swamp the higher corrections that are in principle pro- vided by the exact Schwarzschild solution. It is worth noting to hig hlight some important articles in this field. Krefetz [6] computed the post-Newtonian deviations of the triangular Lagrangian points from their classical positions in a fixed frame of reference for the first time, but without explicitly stating the equations of motion. Contopoulos [7] treated the relativistic (RTBP) in rotat- ing coordinates. He derived the Lagrangian of the system and the deviations of the triangular points as well. Wein- berg [8] calculated the components of the metric tensor by using the post-Newtonian approximation in order to obtain the (RTBP) problem equations of motion. Soffel [9] obtained The angular frequency ω of the rotating frame for the relativistic two-body problem. Brumberg [10,11] studied the problem in more details and collected most of the important results on relativistic celestial me- chanics. He did not obtain only the equations of motion for the general problem of three bodies but also deduced the equations of motion for the restricted problem of three bodies. Bhatnagar and Hallan [12] studied the exis- tence stability of the triangular points 4,5 L in the relati- vistic (RTBP), they concluded that 4,5 L are always unst- able in the whole range 00.5 in contrast to the previous results of the classical restricted three-body problem where they are stable for 0 0 where is the mass ratio and 00.03852 . Lucas [13] found that the difference between Newtonian and post-Newto- nian trajectories for the restricted three-body problem is greater for chaotic trajectories than it is for trajectories that are not chaotic. Finally, the possibility of using this Chaotic Amplification Effect as a novel test of general  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 156 relativity is discussed. 2. Expansion Dimensionless Parameter In the presence of weak gravitational fields and small ve- locities, the physics of general relativity should become Newtonian. This suggest an expansion of the metric, Christoffel symbols, other tensors and the field equations in powers of small parameter, similar to the perturbation expansions of classical and quantum mechanics. The nature and value of the expansion parameter is de- termined by the system at hand. But in general we can characterize a self-gravitating system by a characteristic mass M, characteristic length L and a characteristic time T. The mass could be the mass of the principal body in the system. The length could be its physical radius, and the time could be the time it takes an object to traverse the length scale. Each of these quantities set a scale for its particular dimension, and from them we can establish the dimensionless quantities. In a bound system where motion is periodic, the Virial theorem says (for a nonrelativistic velocities) that 2 11 22 mv V or, for a Newtonian gravitational potential GmM r 2GM vr Dividing both sides by the 2 c, where c is the velocity of light yields 2 22 vGM ccr This very simple equation enables us to identify the expansion parameter as 2 2 22 LGM cT cL In the following we will set G = c = L = 1, and have 12 TM As an example, we can compute the parameter for the Sun, taking its radius as the distance scale. 33 8 1.477 101.4586 10 6.9598 10 What we need then is not to find more exact solutions, but rather to develop some systematic approximation me- thod that will not rely on any assumed symmetry proper- ties of the system. There are two such methods that have been particular- ly useful they are called the Post-Newtonian approxima- tion (PN) and the weak field approximation. The first is adapted to a system of slowly moving particles bound together by gravitational forces. The second method treats the fields in a lower order of approximation but does not assume that the matter moves non-relativisti- cally. A test particle in a circular o rbit of radius r about a central mass M will have velocity v given in Newtonian mechanics by the exact formula 2 vGMr. The PN approximation may be described as a method for obtaining the motions of the system to one higher power of the small parameters GMr and 2 v than given by Newtonian mechanics. It is sometimes referred to as an expansion in inverse powers of the speed of light. We prefer to say that our expansion parameter is 2 c , note that geo metric units will not b e used, so that 1G , and 1c . We now proceed to find the equations of mo- tion of the relativistic three body problem in the PN for- malism, or more precisely the equation of the RTBP. 3. The Geodesic Equation According to the theory of general relativity a particle moving under the influence of gravity follows a Geodes- ic in a four dimensional space called the space-time ma- nifold. The path that it follows is called a geodesic. Let the coordinate of this manifold be as follows: 0, x ct 12 , x xx y and 3. x z Consider a particle moving along a geodesic x s in the space-time manifold, where s is the arc length. A straight line is defined as any path in which its tangent vectors all points in the same direction. Let the tangen t to the curve x (s) be defined dx s Ads (1) The magnitude of this tangent vector is obtained from the tensor algebra as; 1 2 AgAA (2) where g is the metric tensor, which can be obtained from 2 dsgdx dx (3) substituting into Equation (2) yields 1A which means that the tangent vectors all have a constant length, usually called the unit tangent to the curve. This property leads to (when differentiating) 0 dA ds (4)  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 157 If a unit tangent A is parallel displaced from a point p to a point pone can easily get A Adx (5) where is the affine connection, to be defined later. , A using the elementary mathematics, can be written as pp A A AA ds s (6) substituting from Equation (5) into Equation (6) yields 0 dA dx cA ds ds (7) this equation merely represents a division of dA by ds and not to be confused with Equation (4). Substituting from Equation (1) into Equation (7) yields directly the geodesic equation. In fact that the geodesic equation represents the equations of motion of the particles along the geodesics 2 20 d xdxdx ds ds ds (8) Since the proper time is proportional to the arc- length these equations can be written as 2 20 d xdxdx dd d (9) where the affine connection is given by 1 2 pp p p ggg gxxx (10) Now the accelerations can be computed using Equation (9) as 2 2 21 2 2 22 2 2 22 2 2 2 2 2 ii ii ii ii i dxd dxd dt ddt dt dxddx ddt dtddt d d dx ddxdtd dt dtdddt d d d xdtdxdtdddt ddd ddtd d dx dtd d d 322 22 0 ii i i xdtddx dd dt dt dx dxdxdx dx dt dtdt dtdt (11) where we made use the geodesic Equation (9) for the inboxed terms. Remark: We now perform the sums over the dummy indices, namely , and to separate the time and space indexed term, 2 00 0 2 0 00 0 2 2 ijki ii i jk j jk i ii jjk d xdxdxdxdx dtdtdt dt dt dxdx dxdx dtdt dtdt In the Newtonian limit we treat all velocities as vani- shingly small, and so have to zeroth Newtonian order 2 00 2 00 2 2 22 11 1 22 i i ii dx dt gGM x xcr GM Oc cr 4. The Metric Tensor Since a body of a mass b m tends to curve space-time, the metric of the space-time will deviate from that of the Minkowski tensor which represents the flat space. But assuming, without loss of the accuracy required, that the mass of the body is so small, so that the departure from the Minkowski tensor will be in powers of 1,c or in other words the effect on the space-time can be consi- dered as a perturbation to the metric of the flat space, i.e. isso smallghh (12) where , the metric of the flat space-time, is given, in matrix representation, by 1000 0100 0010 0001 (13) Using the fact that the velocity of a test particle of mass p m relative to the body is ,vc and assuming 0, pb mm so that the effects on space-time originating from the particle are negligible, the metric tensor of the perturbed flat space-time can generally be described by 00 00 00 1 ijij ij ii gh g h gh (14) We see that the metric tensor is no longer diagonal. We would like to find the corrections to the metric ten-  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 158 sor, induced by the theory o f general relativity, that is, to determine 00 h to order 6 Oc and 0 j h to order 5 Oc , by solving the Einstein field equations for 00 h and 0. j h Our objective in using the PN approximation will be to compute 22i dxdt to order 4 c . Since the ij g are expected to include powers of GMr , the partial derivatives of the ij g are significantly smaller than ij g themselves. The components of the metric tensor are assumed ex- pandable as 24 00 00 00 24 35 000 1 ij ijij ij iii ggg ggg ggg The symbol N g denoting the term in g of order 1 N c. Odd powers of 1 cc occur in 0i g because 0i g must change sign under the time-reversal transforma- tiontt . The inverse of the metric tensor is defined by the equ- ations 0 0000 0000 000 00 0, 1, . iiij j i oi iiik j jjkij gggg gg gggg gg gggg gg (15) We expect that 24 0000 00 24 35 000 1 ijij ij ij iii ggg ggg gg g (16) Using these expansions into (15) we find 22 00 00 22 33 00 , , ij ij i i gg gg gg (17) The affine connection may now be obtained from the familiar formula 1 2 pp p p ggg gxxx (18) Using (15), (16) and (17) we find that 00 , ii j k and 0 o i have the expansions 24 (19) While the components 0 000 , i j and 0 ij have the ex- pansions 33 (20) The symbol N , denoting the non vanishing terms in of order 1 N c, are given by 2 200 00 43 2 42 00 000 00 23 3 30 0 0 22 2 2 2 3000 00 2 2000 0 1 2 11 22 1 2 1 2 1 2 1 2 i i ii ij ij ij j ii jji ij jk iik jk kji ii g x gg g g t x x gg g t xx gg g xx x g t g x (21) 5. Einstein Field Equations We are now ready to compute the metric tensor compo- nents ij g up to the different orders appeared in Equa- tions (15) and (16). To do that, the Einstein field equa- tions will be used in the following form. 4 81 2 G RTgT c (22) where R is the Ricci tensor and T is the stress- energy-momentum tensor. Note that Greek indices range from 0 to 3 while roman indices range from 1 to 3. 6. The Ricci Tensor R The Ricci tensor is defined by Rxx (23) we find that the components of R have the expansions, 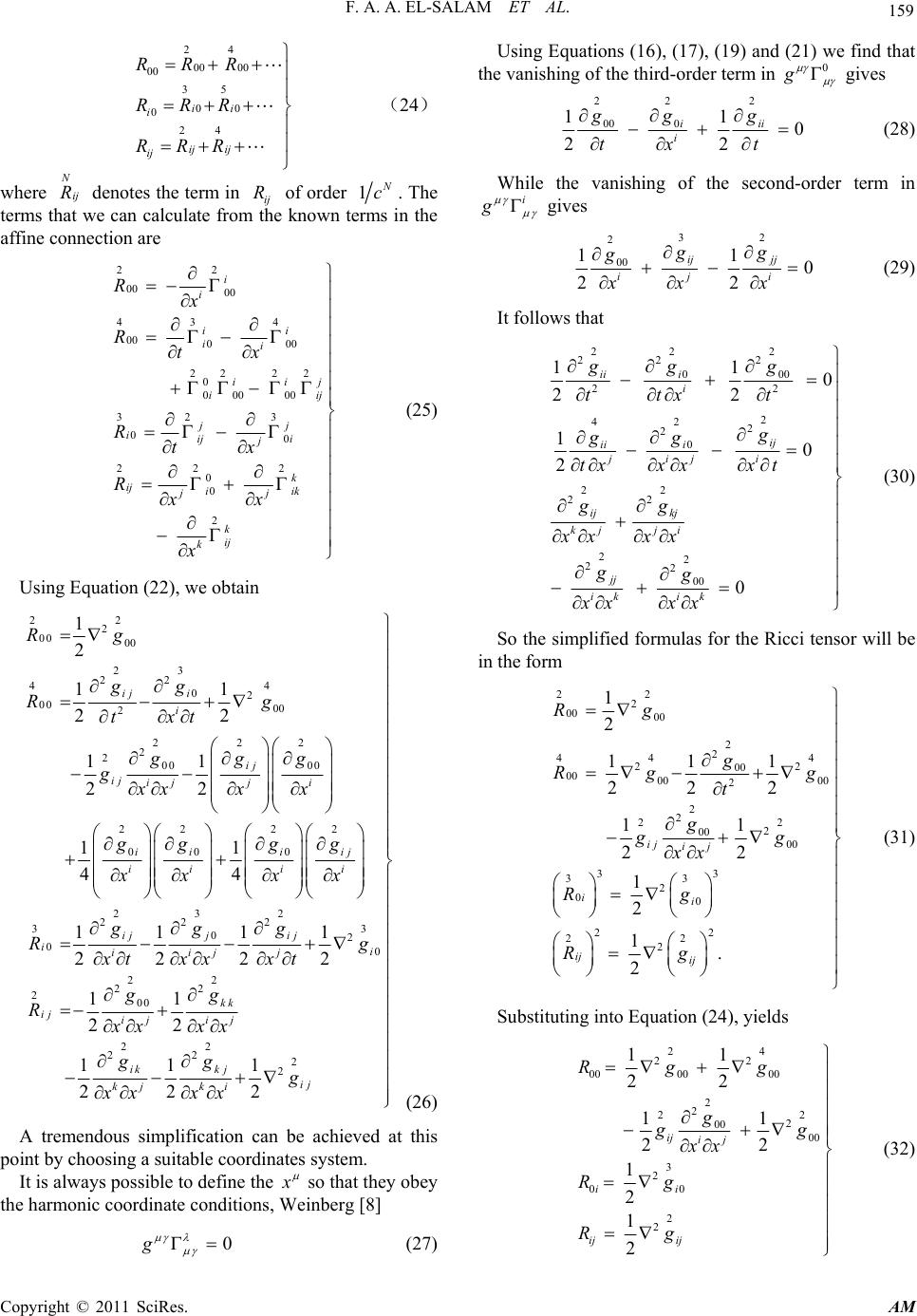 F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 159 24 00 00 00 35 00 0 24 ii i ij ij ij RRR RRR RRR (24) where N ij R d enotes the term in ij R of order 1 N c. The terms that we can calculate from the known terms in the affine connection are 22 00 00 43 4 00 000 22 22 0 00000 32 3 00 22 2 00 2 i i ii ii iij iij jj iij i j k ij iik jj k ij k Rx Rtx Rtx Rxx x (25) Using Equation (22), we obtain 22 2 00 00 23 22 44 02 00 00 2 222 2 200 00 22 22 00 0 2 2 3 0 1 2 11 22 11 22 11 44 1 2 iji i ij ij ijj i iii ij ii ii ij i Rg gg Rg txt ggg gxxxx gg gg xx xx g R 32 22 3 020 22 22 200 22 22 2 2 111 222 11 22 111 222 jij i iijj kk ij ij ij ikk j ij kj ki gg g xtxxx t gg Rxx xx ggg xxxx (26) A tremendous simplification can be achieved at this point by choosing a suitable coordinates system. It is always possible to define the x so that they obey the harmonic coordinate conditions, Weinberg [8] 0g (27) Using Equations (16), (17), (19) and (21) we find that the vanishing of the third-order term in 0 g gives 22 2 00 0 11 0 22 iii i gg g tt x (28) While the vanishing of the second-order term in i g gives 32 2 00 11 0 22 ij jj ij i gg g xx x (29) It follows that 22 2 22 2 000 22 2 42 2 20 22 22 22 2200 11 0 22 10 2 0 ii i i ij ii i jij i ij kj kj ji jj ik ik ggg ttxt g gg txxxxt gg xx xx gg xx xx (30) So the simplified formulas for the Ricci tensor will be in the form 22 2 00 00 2 2 44 4 22 00 00 00 00 2 2 2 22 2 00 00 33 33 2 00 22 22 2 1 2 111 222 11 22 1 2 1. 2 ij ij ii ij ij Rg g Rg g t g gg xx Rg Rg (31) Substituting into Equation (24), yields 24 22 00 00 00 2 2 22 2 00 00 3 2 00 2 2 11 22 11 22 1 2 1 2 ij ij ii ij ij Rgg g gg xx Rg Rg (32)  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 160 7. The Energy-Momentum Tensor T It is assumed that, it is possib le to describe the matter of a system as a perfect fluid, i.e. an isotropic fluid with a diagonal energy-momentum tensor (no shear stress), in the rest frame of the system. This energy-momentum ten- sor which is required in the field equation can be written as 1 23 1 1nn n nn n dx dxd TM xx dt dtdt g (33) where g is the determinant of the metric tensor and , nn M x and n are the mass, space-time position, and proper time for the two massive objects. The expression is simplified by calculating the energy-momentum tensor in the rotating frame, in which the velocity of th e prima- ries is zero. The nonvanishing components of the energy- momentum tensor are 2 00 3 11 1 3 22 2 cdt TM xx d g dt Mxx d (34) We expect that 000 ,i TT and ij T will have the expan- sions 02 000000 13 000 24 iii ijij ij TTT TTT TTT (35) where N T denotes the term in T of order 1 N c. In particular 000 T is the density of rest-mass, while 200 T is the non-relativistic part o f the energy density. What we need now is 1 2 ST gT (36) So that Equation (35) gives 02 00 00 00 13 00 0 02 ii i ij ij ij SSS SSS SSS (37) where N S denotes the term in S of order 1 N c In particular 00 00 00 02202 00 00 00 00 11 0 0 00 00 1 2 12 2 1 2 ij i i ij ij ST STgTT ST ST (38) Using Equations (31) and (37) in the field Equation (22), we find that the field equations in harmonic coordi- nates are indeed consistent with the expansions we have been using, and give 20 200 00 4 2 22 22 4 200 00 00 220 00 00 00 4 21 20 0 20 200 4 8 82 16 8 ij ij i l i i ij ij l G gT c gg gg xx x GTgT c gGT G gT c (39) From the first equation in (39) we find as expected 2 00 2g (40) where is the Newtonian potential defined by Poisson’s equation 0 200 4 8GT c (41) Also 2 00 g must vanish at infinity, so the solution is 0003 4 , ,Txt G x tdx xx c (42) From the fourth equation in (39) we find that the solu- tion for 2 ij g that vanishes at infinity is 22ij ii g (43) On the other hand, 3 0 i g is a new vector potential 3 0i i g (44) and the solution of the third equation in (39) that vanish- es at infinity is  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 161 103 4 , 4 , i i Txt G x tdx xx c (45) Finally, we may simplify the second equation in (39) by using (41), (42) and the id entity 22 2 1 2 ii xx (46) the result is 400 2 22g (47) where is a second potential 22 200 24 4GT tc (48) Again, 4 00 g must vanish at infinity, so the solution is 200 3 4 , ,Txt G x tdx xx c (49) To evaluate these potentials we need the proper time derivatives which can be obtained from the static Schwarzschild line element (in isotropic coordinates) for an observer at the position of 1 M or 2 M . 22 00 nn dgdt (50) Using Misner, et al. [14] yields 22 00 22 11 22 nn n nn GM GM gcr cr (51) To the order of the required accuracy we find 2 2 1 2 1GM dt dcR (52) 1 2 2 2 1GM dt dcR (53) The determinant of the metric tensor is given b y 24 00 00 114ggg (54) 112 g (55) Substituting these all into the second potential yields 212 412 211 ,GMM xt rr cR (56) Returning to the metric tensor components yields 2 12 12 00 22 22 12 12 212 412 12 0 22 12 22 22 1 211 22 1,0,otherwise ii i GMGMGM GM gcr crcr cr GMM rr cR GM GM gg cr cr (57) 8. The Equations of Motion of Restricted Three-Body Problem The standard form of Euler-Lagrange equations read 0 dL L dxx (58) It can be shown that the geodesic equations of motion can be obtained from the Euler-Lagrange equations by defining the Lagrangian L as follows (see Foster, and Nightingale, [15]) 1, ,0,1,2,3 2 Lgxx (59) where the dot denotes the derivatives with respect to pro- per time , and g are the components of the covariant metric tensor. Using the components of the metric tensor the Lagrangian, after some lengthy computations, can be constructed. Then evaluating the derivatives in Euler- Lagrange equations results in the equations of motion (61) and (62) in the following section. 9. The Restricted Three-Body Problem Notations The well known Restricted Three-Body Problem, e.g. the Earth-Moon system, (from now on, RTBP) models the motion of an infinitesimal particle P under the gravita- tional attraction of two massive bodies, usually called primaries of masses 12 1, ,MM under the following assumptions: 1) The particle is of infinitesimal mass that it does not affect the motion of the primaries, 2) The primaries are point masses that revolve in cir- cular orbits around their common centre of mass. It is usual to take a rotating reference frame with the origin at the centre of mass, and such that the two mas- sive bodies are kept fixed on the axis, the , plane is the plane of motion of the primaries, and the axis is orthogonal to the , plane. These coordinates are sometimes called synodical. In the restricted three-body problem this must be transformed from the inertial ,, x yz to the rotating  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 162 coordinate system ,, by using the transformation cossin 0 sincos0 001 nt nt x nt nt y z (60) where n is the angular frequency of the rotating frame The primary coordinates on the x -axis (−µ, 0), (1 − µ, 0) are kept fixed and the origin at the center of mass. Now denoting ,1,2,3x for ,, in the Euler- Lagrangian Equation (58), we obtain 22 33 12 233 12 . 22 2 3 1212 12 11 2 11 2 11 11 2 ntn t ntGtrr Gnt cr r ntntn tGt rrrr rr 3 333 3 121 2 222222 111 11 222 2 rrr r nt nt (61) 22 33 12 233 12 22 2 33 1212 12 1 2 11 2 1 111 21 2 ntn t ntGtrr Gnt cr r ntntn tGt rrrr rr 33 12 222222 33 12 11 12 22 rr nt nt rr (62) where t, the coordinate time and K, the constant of motion are given by 222 12 24 12 em MM K tKNGn rr cc (63) and 22 22222 2 2 12 12 22 2 22 224 24 4 12 1212 2 21 21 11 21 21 2111 112 2 1 GG Kn rr rr cc G GG ncc rr rrrr cc c G c 22 2 24 4 12 1212 1 2 22 212 21 121 11 12 21 1 G G rr rrrr cc c G rr c (64)  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 163 The coordinate time can be eliminated from the equa- tions of motion by using Equation (57), the transforma- tion (60) and the general relativistic line element 22 2 222 2 11 cddsg dx cdtgdxdy which leads to 22 22 222 2 22 dxdynd ddtdd nddtnd dt Thus the line element in th e ro tatin g coord inate system is 2222222 2 00 00 22 22 cdcg dtgndddt ddnddtn ddt Dividing by 2 d allows an expression for . t to be obtained. This can then be used to eliminate . tfrom Eq- uations (61) and (62) and after some algebraic manipula- tion, we get 2 2 Ud U ndt Ud U ndt (65) where U is the potential–lik e function of the relativistic restricted three-body problem, which can be written as composed of two components, namely the potential of the classical restricted three-body problem c Uand the relativistic correction ; r U cr UU U (66) where c Uand r U are given by 2 12 1 2 c r Urr (67) and 22 22 2 2 212 2 2 2 212 2112 2 33 12 1 13 28 31 2 11 2 1111 137 8 2 1 r r Ucc rr c rr c rrr c rr (68) Figure 1. Inertial and rotating frames. The rotating coordi- nate system with coordinates and moves counter- clockwise with unit angular velocity relative to the inertial frame with coordinates X and Y. with 2 22 22 1 22 2 1 113, 2 , , 1. nc r r r where the parameters 1,2 (, )rrr are best illustrated in Figure 1. 10. Conclusions An explicit form of the potential-like function of the rela- tivistic restricted three-body problem is derived. Equa- tions (65) to (68) represent the equati ons of motion of the relativistic three body problem in the PN formalism. These equations will be used in the subsequent works to evaluate the locations of the Lagrangian points, and therefore to investig ate their linear stability. 11. References [1] C. G. Jacobi, “Sur le Movement d’un Point et sur un cas Particulier du Probleme des trios Corps,” Compte Rendus de l’Académie des Sciences, Vol. 3, 1836, p. 59. [2] G. W. Hill, “Researches in the Lunar Theory,” American Journal of Mathematics, Vol. 1, No. 1, 1878, pp. 5-26. doi:10.2307/2369430 [3] H. Poincaré, “Les Methodes Mouvelles de la Mecanique Celeste,” Vol. 3, Gauthier-Villars, Paris, 1892. [4] G. D. Birkhoff, “The Restricted Problem of Three Bo- dies,” Rendiconti del Circolo Matematico di Palermo,  F. A. A. EL-SALAM ET AL. Copyright © 2011 SciRes. AM 164 Vol. 39, 1915, pp. 265-334. doi:10.1007/BF03015982 [5] L. Euler, “Theoria Motuum Lumae,” Typis Academiae Imperialis Scientiarum, Petropoli, 1772. [6] E. Krefetz, “Restricted Three-Body Problem in the Post- Newtonian Approximation,” Astronomical Journal, Vol. 72, 1967, p. 471. doi:10.1086/110252 [7] G. Contopoulos, “In Memoriam D. Eginitis,” D. Kotsa- kis, Ed., Athens, 1976, p. 159. [8] S. Weinberg, “Gravitation and Cosmology Principles and Applications of the General Theory of Relativity,” Chap- ter 9, John Wiley & Sons, New York, 1972. [9] M. H. Soffel, “Relativity in Astrometry, Celestial Me- chanics and Geodesy,” Springer-Verlag, Berlin, 1989, p. 173. [10] V. A. Brumberg, “Relativistic Celestial Mechanics,” Nauka Press (Science), Moscow, 1972. [11] V. A. Brumberg, “Essential Relativistic Celestial Me- chanics,” Adam Hilger, Ltd., New York, 1991. [12] K. B. Bhatnagar and P. P. Hallan, “Existence and Stabili- ty of L4,5 in the Relativistic Restricted Three Body Prob- lem,” Celestial Mechanics, Vol. 69, No. 3, 1998, pp. 271- 281. [13] F. W. Lucas, “Chaotic Amplification in the Relativistic Restricted Three-Body Problem,” Verlag der Zeitschrift für Naturforschung, Tübingen, Vol. 58a, No. 1, 2003, pp. 13-22. [14] C. W. Misner, K. S. Thorne and J. A. Wheeler, “Gravita- tion,” Freeman and Company, San Francisco, 1973, p. 840. [15] J. Foster and J. D. Nightingale, “A Short Course in Gen- eral Relativity,” Springer, New York, 1995, p. 147. |

