 Journal of Applied Mathematics and Physics, 2013, 1, 121-127 Published Online November 2013 (http://www.scirp.org/journal/jamp) http://dx.doi.org/10.4236/jamp.2013.15018 Open Access JAMP Advection Dispersion Equation and BMO Space Kan Zhang1,2*, Tieliang Wang1#, Xue Feng2 1Postdoctoral of Agricultural Engineering, Shenyang Agricultural University, Shenyang, China 2College of Sciences, Shenyang Agricultural University, Shenyang, China Email: kanzhang2004@163.com, #wzhangpaper@163.com, xfeng2000@163.com Received October 15, 2013; revised November 10, 2013; accepted November 14, 2013 Copyright © 2013 Kan Zhang et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT In this paper, we prov ide a new way of characterizing th e upper and lower bound for the concentration and the gradient of concentration in advection dispersion equation under the condition that source term, concentration and stirring term belong to BMO space. Keywords: Advection Dispersion Equation; BMO; Concentration 1. Introduction Throughout the paper we fix a positive in teger n and let n CC C denote the n-dimensional complex Euclidean space. For and 12 ,,, n zzz z 12 ,,, n in we write n C 22 1 n i i zz and 11 ,, nn zz z where i is the complex conjugate of i . Let n denote the unit ball in and let be the Lebesgue volume measure on . For Bn Cv n B1 , we denote by v the measure on defined by n B 2 d1dvz czvz , where 1 !1 n cn is a normalizing constant such that 1. n vB For 1p, we write , for the norm on ,d pn LBv ,d , pp nn n BLBvHB where n BB denote the space of all holomorphic functions in n. Reproducing kernels w and normal- ized reproducing kernels w k in n 2 B are given by, respectively, 1 1 1, wn Kz zw and 12 2 1 1 1, n wn w kz zw for ,n zw B . For every we have 2n hAB ,ww hK h for all . The orthogonal pro- n wB jection P of 2,d n LB v onto 2n B is given by 1 1 ,d 1, n wn B PgwgKg zvz wz for 2,d n LB v and . When n wB1n , we write for . D1 B and , for the inner product on 2,d n LB vFor 1,d n LB v , we define the Berezin transform of to be the function , that is . The weighted Bergman space pn B consists of holomorphic functions in ,d n LBv p , that is, 2d. nz B zfwkwvw If is bounded, then is a bounded function on n. Since the normalized reproducing kernels B k con- verge weakly to zero as tends , we have that if zn B *The fir st author is partly supported by NSFC, It em Number: 11371182. #Corresponding author.  K. ZHANG ET AL. 122 is compact, then as . The con- verse (in both case) is not necessarily true. 0fz n zB The traditional advection dispersion equation is a standard model for contaminant transport. The advection dispersion equation is the basis of many physical and chemical phenomena, and its use has also spread into economics, financial forecasting and other fields. In gen- eral, the numerical solution of advection dispersion equa- tions has been dominated by either finite difference, fi- nite element or boundary element methods. These meth- ods are derived from local interpolation schemes and require a mesh to support the application. It is well known that the numerical solution of advection disper- sion equation is a difficult task. Scholars try to find the new way to obtain the solution of advection dispersion equation. For the passive scalar, complicated behavior is often observed even for laminar velocity fields. This is the well-known effect of ch aotic advection in [1,2 ]. Thus we can choose the source and stirring term of the advec- tion dispersion equation to be any divergence-free, pos- sibly time-dependent flow field. The mixing efficiency depends on specific properties of the stirring and source term. Schumacher, Sreenivasan and Yeung have obtained bounds on high-order derivative moments of a passive scalar for large values of the Schmidt number in [3]. Thiffeault, Doer ing and G ibbon hav e obtained bound s on mixing efficiency for the passive scalar under the influ- ence of advection and diffusion with a body source in [4]. The advection dispersion equation for the concentra- tion , t of a passive scalar is .uk t s (1) where is the dispersion coefficient, k , xt is a source term, and is the stirring term. It is clear that an exciting mixing configuration would have small concentration for a given source term and stirring term, indicating a steady state with low density of concentra- tion. We use the fluctuations in the concentration as a useful measure of the degree of well-mixedness, as has long been the practice in [5,6]. ,uxt In this paper we apply some recent developments in the analysis of the BMO space to the advection disper- sion equation. We further provide a new way of giving the upper and lower bound for the concentration and the gradient of concentration in the advection dispersion equation by using BMO theory. The bounds on mixing efficiency in this paper mainly depend on the stirring field and the source distribution, which is very important for allowing comparison of the relative effectiveness of various source term for specified stirring scenarios. Throughout the paper, we will use the letter to de- note a generic positiv e constant that can change its value at each occurrence. c 2. Some Lemmas and Basic Definitions For n zB , let be the automorphism of such n B that 0 zz and 1 z , which is described in [7]. It has the real Jacobian equal to 1 2 2 22 1, 1, n zn z wzw for ,n zw B . Thus we have the change-of-variable formula 2dd nn zz BB hwkwvw hwvw , (2) for every 1,d n hLB v . By a well-known theorem of John-Nirenberg in [8,9], the classical BMO of the unit circle is independent of the L norm used to define it. It is also well known that a function on the circle is in BMO if and only if the Hankel operators with symbol and ff are both bounded on the Hardy space of the circle. A new type of BMO, Denoted BMO , is introduced in [10,11] for any bounded domain in the complex space . In this paper we define the BMO space in the Bergman metric by the n C L norm. Let ,d n fLBv 1 and , we say that 1p pn MOB fB whenever , sup . p n z BMO p zB fffz Note that MO does not distinguish constants, while ,0 p BMO p ff f is a norm in pn BMO B . By the Theorem 5 in [12], we know the fact that pn O B BM is equivalent to r BMO in [12]. For ,n zw B , let 1 1 ,log 21 z z w zw w n zB denote the Bergman metric on . For any and , let n B0r , n Dzw Bzwr be the Bergman metric ball with center and radius z r. Let Dzv Dz , which is equivalent to 1 2n z 1 (see Lemma 1.24 of [13]). For 1,d n LBv , the average of over Dz is defined by Open Access JAMP  K. ZHANG ET AL. 123 1 ˆd. Dz zfwv Dz w Using the properties of Bergman metric, it follows that 1ˆ sup d n p Dz zB fwfzv w Dz if and only if p BMO f . Thus, functions in pn BMO B have bounded mean oscillation in the Bergman metric. Since pn BMO B functions are locally in ,d pn LBv , it is not hard to see that ,d ,1, pp nnn LB BMOBLBvp 1,1 . qp nnn BMO BBMOBBMO Bp q Since the space is the largest among the for , fro m now on we will be mainly interested in functions belonging to this class. The study of BMO spaces plays an important role on modern analysis and applied scien ce in [14,15]. For simplicity we will write 1n BMO B 1p pn BMO B BMO instead of . pn BMO B VMO consists of functions in BMO such that ,0 zp ffz as 1z . Lemma 2.1 Suppose 1 BMO , then the following quantities are equivalent: (1) ˆ is bounded on ; n B (2) is bounded on ; n B (3) is bounded on ; n B (4) is bounded on . n B Proof 12: Since 1 2 ˆ 1d 1d d d , n n Dz Dz z B z B BMO fz fz fz fv Dz fz fv Dz cfzf kv cfzf v cf then ˆ is bounded on if and only if n B is bounde d o n . n B 3 2 : Since 2 2 1 d d d , n n z B z B z Bn BMO fz fz ffzk v fz fkv fz fv f then is bounded on if and only if n B is bounde d o n . n B 43: The proof is trivial. Lemma 2.1 implies the fact that we may regard the average function and Berezin transform as positive func- tion, which is very important for us to research the pa- rameters in advection dispersion equation by using BMO theory. 3. Main Results In this section, we further obtain the upper and lower bound for the concentration and the gradient of concen- tration in the advection dispersion equation. We give the reasons why the source term, stirring term and concen- tration in the advection dispersion equation belong to BMO space. In fact, BMO space extends the mean- variance theory. According to the definition of average function and norm of BMO space, it is clear that the av- erage function extends the mean theory and the norm of BMO space extends the variance theory. The parameters in advection dispersion equation are uniformly bounded in time, which is true under the physical assumption that ,1 is uniformly bounded in time. In addition, Lemma 2.1 provides the reasons for regarding the concentration, source term and stirring term as BMO function. The formula ,d ,1, pp nnn LB BMOBLBvp also prov ides t he r e a s on for 1 ,,us BMO . In [4], the advection dispersion operator is defined by .Luk t It is well known that the space is Banach space instead of Hilbert space. So it is difficult to obtain the adjoint of the advection dispersion operator. Then we have to find new way of characterizing the upper and lower bound for the concentration and the gradient of concentration in the advection dispersion equation by using BMO theory. 1,d n LB v Next, we will obtain the lower bound for ,1 and ,1 in the advection dispersion equation. Open Access JAMP 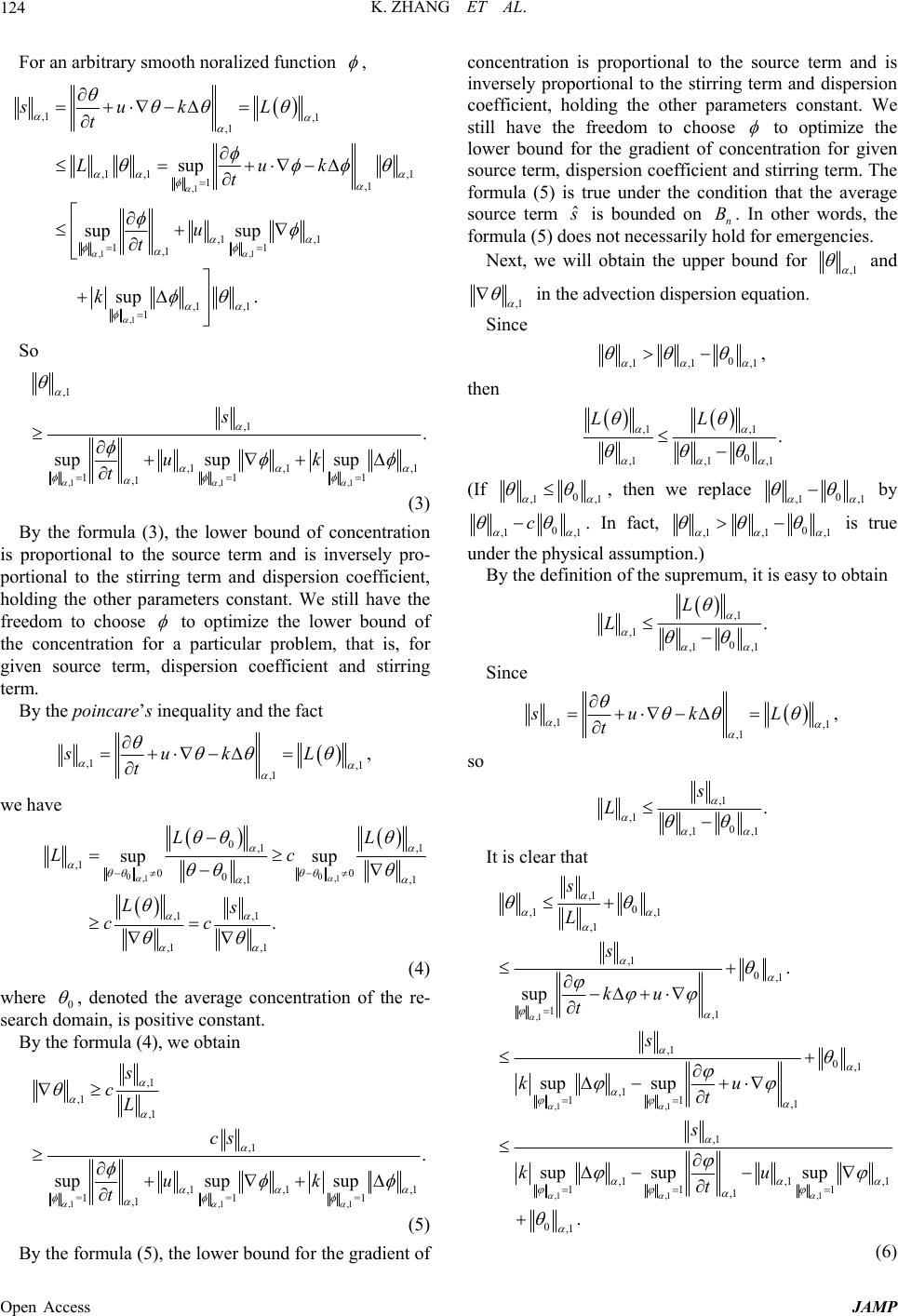 K. ZHANG ET AL. 124 For an arbitrary smooth noralized function , ,1 ,1 ,1 ,1 ,1 ,1 ,1 ,1 ,1,1 1,1 ,1 ,1 11 ,1 ,1 ,1 1 sup sup sup . sup sukL t Luk t u t k So ,1,1 ,1 ,1 ,1 ,1 ,1,1 11 ,1 . supsup sup s uk t 1 (3) By the formula (3), the lower bound of concentration is proportional to the source term and is inversely pro- portional to the stirring term and dispersion coefficient, holding the other parameters constant. We still have the freedom to choose to optimize the lower bound of the concentration for a particular problem, that is, for given source term, dispersion coefficient and stirring term. By the poincare ’s inequality and the fact ,1 ,1 ,1 ,sukL t we have 00 ,1 ,1 0,1 ,1 ,1 00 0,1 ,1 ,1 ,1 ,1 ,1 sup sup . LL Lc Ls cc (4) where 0 , denoted the average concentration of the re- search domain, is positive constant. By the formula (4), we obtain ,1,1,1 ,1 ,1 ,1 ,1 ,1 ,1,1 =1=1 =1 ,1 . supsup sup s cL cs uk t (5) By the formula (5), the lower bound for the gradient of concentration is proportional to the source term and is inversely proportional to the stirring term and dispersion coefficient, holding the other parameters constant. We still have the freedom to choose to optimize the lower bound for the gradient of concentration for given source term, dispersion coefficient and stirring term. The formula (5) is true under the condition that the average source term ˆ is bounded on n. In other words, the formula (5) does not necessarily hold for emergencies. B Next, we will obtain the upper bound for ,1 and ,1 in the advection dispersion equation. Since 0 ,1 ,1,1, then ,1 ,1 0 ,1,1 ,1 . LL (If 0 ,1 ,1 , then we replace 0 ,1 ,1 by 0 ,1 ,1 c . In fact, 0 ,1 ,1,1 is true under the physical assumption.) By the definition of the supremum, it is easy to obtain ,1 ,1 0 ,1 ,1 . L L Since ,1 ,1 ,1 ,sukL t so ,1 ,1 0 ,1 ,1 . s L It is clear that ,1 ,1 ,1 ,1 ,1,1 ,1 0 ,1 ,1 ,1 ,1 0,1 1,1 ,1 0,1 ,1 11,1 ,1 ,1,1 ,1 11 1 ,1 0,1 . sup sup sup sup supsup . s L s ku t s ku t s ku t (6) Open Access JAMP  K. ZHANG ET AL. Open Access JAMP 125 per and lower bound of the gradient of concentration. Compared with the formula (3), the formula (6) gives the error between concentration and average concentra- tion, which has important significance in practice. ,1,1 ,1 11 ,1 ,1 ,1 ,1 ,1,1 111 ,1 1 1,1 ,1 ,1 00 11 ,1 ,1 ,1 ,1 supsup sup sup sup . cs uk t t ks cuI (9) Let ,, , n cc c , so ,1,1 Ic . By the formula (1), it is easy to obtain .uIIk s t Since ,1 ,1 ,1 ,1 ,1 ,1 ,1 ,1 ,1 , cuI cuI uI I uks t ks t An efficient mixing configuration would have small concentration ,1 for a given source term and stirring term, indicating a steady state with small variations in the concentration. In general we expect that increasing source term at fixed stirring term should augment con- centration. For the concentration, we focus on the formula (8). As we increase the source amplitude, holding the other pa- rameters constant, the concentration ,1 must even- then 11 ,1 ,1 1 1,1 ,1 ,1 00 11 ,1 ,1 ,1 ,1 sup sup . t k cuI s (7) tually increase. However large concentration does not necessarily imply large source term, as the difference can be made up by the dispersion coefficient or stirring term. This is what makes enhanced mixing possible. An k increase of ,1 implies that the scalar is more poorly mixed. Formula (8) reflects that we can postpone the increasing of lower bound of concentration by raising the stirring term. The formula (5) and the formula (7) make trouble for us to research the factors on influencing the upper and lower bound for the gradient of concentration in the ad- vection dispersion equation. As we increase the disper- sion coefficient, holding the other parameters constant, the lower bound for the gradient of concentration must decrease and the upper bou nd for the gradient of concen- tration must increase. One of the reasons for this phe- nomenon is that the gradient of concentration can be af- fected by several environmental factors such as tempera- ture, PH, salinity, etc. (see, [16-20]). For the gradient of concentration, we focus on the formula (9). As we increase the source amplitude, hold- ing the other parameters constant, the gradient of con- centration ,1 must eventually increase. However large gradient of concentration does not necessarily im- ply large source term, as the difference can be made up by other factors. Formula (9) also reflects that we can postpone the increasing of the upper and lower bound of gradient of concentration by raising the stirring term. By formula (3) and formula (6), we obtain the upper and lower bound for the concentration in the advection dispersion Equation (8). By the formula (8) and (9), the concentration ,1 and the gradient of concentration ,1 seem to have the same lower bound, which does not imply that the By the formula (5) and formula (7), we obtain the up- ,1,1 ,1 ,1 ,1,1 ,1 ,1 ,1 ,1,1 111 ,1 ,1 0,1 ,1,1 ,1 11 1 ,1 supsup sup . sup supsup s uk t s ku t (8)  K. ZHANG ET AL. 126 formula (8) and formula (9) are wrong. By the poincare’s inequality, the concentration ,1 and the gradient of concentration ,1 must have the same form of the lower bound. Although our results are established on the unit ball in , our results are obviously correct for any bounded domain in . Since , so the results in this paper are correct for any bounded domain in . By the formula (8), if n Bn Cn Cn RC 1 n n R VMO and the aver- age function tends zero, then the lower bound of concentration in advection dispersion equation must eventually tend zero and at the same time the upper bound of concentration tends the average concentration of the whole research domain. By the formula (9), if s ˆ 1 VMO and the average function tends zero, then the lower bound for the gradient of concentration in ad- vection dispersion equation must eventually tend zero and at the same time the upper bound for the gradient of concentration does not necessarily tend zero. s ˆ By the formulas (8) and (9), we have freedom to choo se and calculate its integral for optimizing the upper and lower bound for the concentration and the gradient of concentration. There is a long way to go before we have satisfactory results. 1,d n LB v If and are time-dependent and still satisfy the formula (8), then we obtain the following result, namely, ,1 ,1 ,1 ,1 ,1 ,1 ,1 ,1,1 11 ,1 0,1 ,1 ,1,1 11 sup sup . sup sup s uk s ku If 1 and are time-dependent and still satisfy the formula (9), then we obtain the following result, namely, ,1 ,1 1,1 ,1 ,1 ,1 ,1,1 11 1,1 ,1 01,1 ,1 sup sup sup . cs uk ks cuI 4. Conclusions It is encouraging that we may obtain the upper and lower bound for the dispersion coefficient k and ,1 using the same method under the conditions that concen- tration, source term and stirring term are the control pa- rameters. As a physically meaningful measure of mixing 2 20,1 2k 2 ,1 , eq k where efficiency, we introduce the equivalent diffusivity in [21-23], namely, eq k 0 is the solution of the advection dispersion equation with the same source but no stirring term. By the upp er and lower bound of dispersion coefficient, it is easy to obtain the upper and lower of the equivalent diffusivity. The effec- tive diffusivity is defined in terms of a large-scale gradi- ent of the concentration, whereas here we use the ampli- tude of the source term, which makes more sense in the present context. In closing we note that all of our analysis, as well as the general result that the equivalent diffusivity depends on the source distribution being smooth enough to have a finite variance. Point sources, for example where ~ z , may be of interest in applications but do not e variance. In this situation we may still define the mixing efficiency and an equivalent diffusivity have finit via 0,1 0,1 eq kk . Although we provide a new way to illuminate the quantitative relation among the concentra- s left for future w REFERENCES [1] H. Aref, “Stiction,” Journal of tion, dispersion coefficient and gradient of concentration by using BMO theory, it is clear that the concentration, dispersion coefficient and gradient of concentration in advection dispersion equation can be affected by several environment factors(for example [24-29]). So how to fully consider the influence factors on the concentration, disper sion co effic ien t and gr adie nt of con cen tration is th e key for proceeding the subsequent job. The investigation of these works i ork. rring by Chaotic Adve Fluid Mechanics, Vol. 143, No. 1, 1984, pp. 1-21. http://dx.doi.org/10.1017/S0022112084001233 [2] J. M. Ottino, “The Kinematics of Mi xin g: Stretchi n g, C ha os , umacher, K. R. Sreenivasan and P. K. Yeung, and Transprot,” Cambridge University Press, Cambridge, 1989. [3] J. Sch “Schmidt Number Dependence of Derivative Moments for Quasi-Static Straining Motion,” Journal of Fluid Me- chanics, Vol. 479, No. 1, 2003, pp. 221-230. http://dx.doi.org/10.1017/S0022112003003756 [4] J. L. Thiffeault, C. R. Doering and J. D. Gibbon, “A Bound on Mixing Efficiency for the Advection-Diffusion Equa- tion,” Journal of Fluid Mechanics, Vol. 521, No. 1, 2004, pp. 105-114. http://dx.doi.org/10.1017/S0022112004001739 [5] P. V. Danckwerts, “The Definition and Measurement of rac- teristics of Fluorescein Dye and Temperature Fluctuations Some Characteristics of Mixtures,” Applied Scientific Re- search, Section A, Vol. 3, No. 4, 1952, pp. 279-296. [6] H. Rehab, R. A. Antonia, L. Djenidi and J. Mi, “Cha Open Access JAMP  K. ZHANG ET AL. 127 in a Turbulent Near-Wake,” Experiments in Fluids, Vol. 28, No. 5, 2000, pp. 462-470. http://dx.doi.org/10.1007/s003480050406 [7] W. Rudin, “Function Theory in the Unit Ball of Springer-Verlage, New York, 1980. C,” n http://dx.doi.org/10.1007/978-1-4613-8098-6 [8] J. Garnett, “Bounded Analytic Functions,” Aca New York, 1981. demic Pre s s unications on Pure and Applied Ma- , [9] F. John and L. Nirenberg, “On Functions of Bounded Mean Oscillation,” Comm thematics, Vol. 14, No. 3, 1961, pp. 415-426. http://dx.doi.org/10.1002/cpa.3160140317 [10] D. Békollé, C. A. Berger, L. A. Coburn and K “BMO in the Bergman Metric on Bound. H. Zhu ed Symmetric , Domains,” Journal of Functional Analysis, Vol. 93, No. 2, 1990, pp. 310-350. http://dx.doi.org/10.1016/0022-1236(90)90131-4 [11] C. A. Berger, L. A. Coburn and K. H. Zhu, “BMO Bergman Spaces of the Classical Domains,” Bu lletin o on the f the American Mathematical Society, Vol. 17, No. 1, 1987, pp. 133-136. http://dx.doi.org/10.1090/S0273-0979-1987-15539-X [12] K. H. Zhu, “BMO and Hankel Operators on Bergman Spaces,” Pacific Journal of Mathematics, Vol. 155, No. 2, 1992, pp. 377-395. http://dx.doi.org/10.2140/pjm.1992.155.377 [13] K. H. Zhu, “Spaces of Holomorphic Functions Ball,” Springer-Verlage, New York, 2004. in the man Space of Unit [14] K. Zhang, C. M. Liu and Y. F. Lu, “Toeplitz Operators with BMO Symbols on the Weighted Berg the Unit Ball,” Acta Mathematica Sinica, English Series, Vol. 27, No. 6, 2011, pp. 2129-2142. http://dx.doi.org/10.1007/s10114-011-0038-3 [15] K. E. Petersen, “Brownian Motion, Hardy Bounded Mean Oscillation,” Cambridge Unive Spaces and rsity Press, Cambridge, 1977. http://dx.doi.org/10.1017/CBO9780511662386 [16] M. Rosso, J. F. Gouyet and B. Sapoval, “Dete of Percolation Probability from the Use of a Crmination oncentr a- tion Gradient,” Physical Review B, Condensed Matter, Vol. 32, No. 9, 1985, pp. 6053-6054. http://dx.doi.org/10.1103/PhysRevB.32.6053 [17] V. Markin, T. Tsong, R. Astumian and B. R “Energy Transduction between a Concentratiobertson, on Gradient and an Alternating Electric Field,” The Journal of Chemi- cal Physics, Vol. 93, No. 7, 1990, pp. 5062-5066. http://dx.doi.org/10.1063/1.458644 [18] F. Stümpel and K. Jungermann, “Sensing by Intrahepatic Muscarinic Nerves of a Portal-Arte rial Glucose Concen- Presence of a Con- ulation of the 2432-1 tration Gradient as a Signal for Insulin-Dependent Glu- cose Uptake in the Perfused Rat Liver,” FEBS Letters, Vol. 406, No. 1, 1997, pp. 119-122. [19] A. Lasia, “Porous Electrodes in the centration Gradient,” Journal of Electroanalytical Chem- istry, Vol. 428, No. 1, 1997, pp. 155-164. [20] M. Higa, A. Tanioka and K. Miyasaka, “Sim Transport of Ions against Their Concentration Gradient across Charged Membranes,” Journal of Membrane Sci- ence, Vol. 37, No. 3, 1988, pp. 251-266. http://dx.doi.org/10.1016/S0376-7388(00)8 raphy, [21] M. B. Isichenko, “Percolation, Statistical Topog and Transport in Random Media,” Reviews of Modern Physics, Vol. 64, No. 4, 1992, pp. 961-1043. http://dx.doi.org/10.1103/RevModPhys.64.961 [22] S. B. Pope, “Turbulent Flow,” Cambridge University BO9780511840531 Press, Cambridge, 2000. http://dx.doi.org/10.1017/C Thiffeault [23] N. J. Balmforth, W. R. Young, J. Fields, J. L. and C. Pasquero, “Stirring and Mixing: 1999 Program of Summer Study in Geophysical Fluid Dynamics,” Woods Hole Oceanographic Institution, 2000. http://dx.doi.org/10.1575/1912/94 [24] B. Gaylord and S. D. Gaines, “Temperature or Transport Range Limits in Marine Species Mediated Solely by Fl o w , ” The American Naturalist, Vol. 155, No. 6, 2000, pp. 769-789. http://dx.doi.org/10.1086/303357 [25] N. Margvelashvily, V. Maderich and M. Zheleznyak, 128 “THREETOX—A Computer Code to Simulate Three-Di- mensional Dispersion of Radionuclides in Stratified Wa- ter Bodies,” Radiation Protection Dosimetry, Vol. 73, No. 1-4, 1997, pp. 177-180. http://dx.doi.org/10.1093/oxfordjournals.rpd.a032 f [26] D. T. Ho, P. Schlo sser and T. Caplow , “Determinat ion o Longitudinal Dispersion Coefficient and Net Advection in the Tidal Hudson River with a Large-Scale, High Reso- lution SF6 Tracer Release Experiment,” Environmental Science and Technology, Vol. 36, No. 15, 2002, pp. 3234- 3241. http://dx.doi.org/10.1021/es015814+ [27] G. H. O. Essink, “Salt Water Intrusion in a Three-Dimen- 3/A:1010625913251 sional Groundwater System in the Netherlands: A Nu- merical Study,” Transport in Porous Media, Vol. 43, No. 1, 2001, pp. 137-158. http://dx.doi.org/10.102 . Garcia, C. [28] E. Sierra, F. G. Acien, J. M. Fernandez, J. L Gonzalez and E. Molina, “Characterization of a Flat Plate Photobioreactor for the Production of Microalgae,” Che- mical Engineering Journal, Vol. 138, No. 1, 2008, pp. 136-147. http://dx.doi.org/10.1016/j.cej.2007.06.004 [29] L. Y. Chang and W. C. Chen, “Data Mining of Tree- Based Models to Analyze Freeway Accident Frequency,” Journal of Safety Research, Vol. 36, No. 4, 2005, pp. 365- 375. http://dx.doi.org/10.1016/j.jsr.2005.06.013 Open Access JAMP
|