 Journal of Applied Mathematics and Physics, 2013, 1, 110-120 Published Online November 2013 (http://www.scirp.org/journal/jamp) http://dx.doi.org/10.4236/jamp.2013.15017 Open Access JAMP Fractional Order Two Temperatur e Thermo-Elastic Behavior of Piezoelectric Materials Essam Bassiouny1,2, Refaat Sabry1,3,4 1Department of Mathematics, Faculty of Science and Humanitarian Studies, Salman Bin AbdulAziz University, Al Kharj, KSA 2Department of Mathematics, Faculty of Science, Fayoum University, Fayoum, Egypt 3Theoretical Physics Group, Department of Physics, Faculty of Science, Mansoura University, Damietta Branch, New Damietta, Egypt 4International Centre for Advanced Studies in Physical Sciences, Faculty of Physics and Astronomy, Ruhr University Bochum, Bochum, Germany Email: esambassiouny@yahoo.com, Sabry_nonlinear@yahoo.com Received July 13, 2013; revised August 21, 2013; accepted August 27, 2013 Copyright © 2013 Essam Bassiouny, Refaat Sabry. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT A new mathematical model of time fractional order heat equation and fractional order boundary condition have been constructed in the context of the generalized theory of thermo piezoelasticity. The governing equations have been ap- plied to a semi infinite piezoelectric slab. The Laplace transform technique is used to remove the time-dependent terms in the governing differential equations and the boundary condition. The solution of the problem is first obtained in the Laplace transform domain. Furthermore, a complex inversion formula of the transform based on a Fourier expansion is used to get the numerical solutions of the field equations which are represented graphically. Keywords: Fractional Order; Two Temperature; Generalized Thermoelectricity; Weak Diffusion; Thermal Loading; Piezoelectric Materials; Ceramics 1. Introduction Chen and Gurtin [1-3] have formulated a theory of heat conduction in deformable bodies, which depends upon two distinct temperatures, the conductive temperature and the dynamical temperature T. Regarding time inde- pendent situations, the difference between these two temperatures is proportional to the heat supply. However, in the absence of any heat supply, the two temperatures are identical [1,2]. On the other hand in time dependent problems, particularly for wave propagation problems, the two temperatures are generally different regardless of the presence of heat supply. The two temperatures T, and the strain are found to have representations in the form of a traveling wave plus a response, which occur instantaneously throughout the body [4]. Warren and Chen [5] investigated the wave propagation in the two- temperature theory of thermoelasticity, but Youssef [7] investigated this theory in the context of generalized thermoelasticity. Because the non-local property of fractional order of differential equations (FODE), FODE becomes prominent and extensively used in many applications in fluid me- chanics, physics, engineering, viscoelasticity and many other fields. The presence of the fractional order operator in the differential equations affects the history of the system, which means that the next states of the system will depend, on the current state and also upon all of its previous states, making it more realistic: Caputo [7], Mainardi [8] and Podlubny [9]. FODE has been used successfully in modeling of various physical phenomena and in many applications such as chemistry, biology, electronic, wave propagation and viscoelasticity Hilfer [10], Caputo and Mainardi [11], Caputo [12], Bagley and Torvik [13], Koeller [14] and Rossikhin and Shitikova [15]. In the second half of the 19th century, both of the the- ory of fractional derivatives and integrals were estab- lished. The first application of fractional derivatives was applied by Abel to solve an integral equation that arises in the formulation of the Tautochrone problem [16,17]. Various definitions and approaches of fractional de- rivatives have become the main purpose of many studies  E. BASSIOUNY, R. SABRY 111 [18,19]. Kimmich [19] study time—fractional diffusion—wave equation and use the Riemann-Liouville fractional inte- gral as follows: 1 0 1d,0 2, , t tf Ift ft 0 (1) where is the Gamma function. Fujita [20,21] considered a fractional order heat wave equation for the case 12 obtained from the non local constitutive equation for the heat flux components in the form i q 1, 12 ii qIT (2) Povestenko [22,23] used the heat Equation (2) to study the theories of thermal stresses based on space-time frac- tional telegraph equations. To eliminate the paradox of the instantaneous propa- gation of heat, Cattaneo [24] introduced a law of heat conduction to replace the classical Fourier law of heat conduction. The propagation of discontinuities of solu- tions in this theory was investigated by Ezzatt and Kara- many [25]. Ezzatt and Karamany [25-28] established a new model of fractional heat equation based on a Taylor expansion of time-fractional order. They studied the non-homoge- neous anisotropic elastic solid using two new models and they considered the uniqueness theorem in linear frac- tional two temperatures thermoelasticity and the theory of a perfect conducting thermoelastic medium. Also, they constructed a new model of electro-thermoelasticity in the context of a new consideration of heat conduction with fractional order. Sherief et al. [16] used the following form of the heat conduction law , 01 i io qT qt t 1 (3) and derived the governing equations of the fractional order theory of thermoelasticity using Caputo [7] defini- tion of fractional derivatives of order 0 of ab- solutely continuous function t given by: 1 d d tIft t (4) 1 0 d tts ftfs s (5) where I is the fractional integral of the function t of order defined by [19]. In the limit as 1 , Equation (3) can be reduced to Cattaneo law [24]. A new formula of heat conduction has been considered in the context of fractional integral operator defined by Youssef [29] who introduces the following form of heat conduction law 1, 02 ioi i qq IT (6) Taking into consideration the works of Fujita [20,21] and Povestenko [22,23], Youssef proved the uniqueness of the solutions in this case. In the present work a model for generalized ther- mopiezoelasticity has been constructed in the context of the fractional heat equation where 02 0 to describe different types of diffusion where 1 corresponds to weak diffusion, 1 corresponds to normal diffu- sion, 12 corresponds to strong diffusion and 2 corresponds to ballistic diffusion. This is used to investigate the propagation of thermal wave through a semi infinite slab subjected to thermal loading of frac- tional order of exponential type applied for finite period of time. 2. Governing Equations In the absence of body force, free charge and inner heat sources, we consider generalized thermo-piezoelectric governing differential equations Youssef [29] and Yous- sef and Bassiouny [30] follows: Equations of motion: 2 ,2 i ij j u t (7) Equation of entropy increment (in the absence of inner heat source): ,, ii o qT t (8) Stress-strain-temperature: , ijijkl klkijkij ce hD (9) Gauss equation and electric field relation: ,0 ii D (10) , i Ev i (11) iikl klikki Ehe Dd (12) Equation of entropy density: ij ijii edDcT (13) Strain-displacement relations: ,, 1 2 ijijj i euu (14) The heat conduction 2 1 2 iioE o CT tt e (15) Open Access JAMP  E. BASSIOUNY, R. SABRY 112 where 1 0 1d, 02, , 0 t tf Ift ft (16) The thermodynamical temperature relates with the conductive temperature by the relation , ii a (17) in which is the two-temperature parameter. 0a In the above equations, a comma followed by a suffix denotes material derivatives and a superposed dot de- notes the derivatives with respect to time. 3. One Dimension Formulation Consider a semi-infinite piezoelectric rod occupying the region . At the near end a uniform flow of heat is supplied to the rod during a finite period of time. All the state functions field will depend only on the dimension x and the time t. We assume the following form for the displacement component: 0x ,, 0 xy uuxtuu z (18) We consider the following forms of the linearized ba- sic equations in one-dimensional formulation: 22 2 2u x2 u t (19) 2uhD x (20) 22 1 22 oEo CT t xt e (21) 2 2 a (22) ,uxt e (23) 0 D x (24) , v E (25) where 32, t t is the coefficient of the linear thermal expansion, is the coefficient of ther- mal conductivity and is the coordinate taken along the rod. It is convenient now to introduce the following dimen- sionless variables: 2 22 2 , , , , 2 , , , , 2 , , , 2 o oo o o o o oo o o E o oo TT ucutct T T tctxcx cT C qh qDD c kc Tk (26) From Gauss’s law, since there is no free charge inside the piezoelectric rod we have 0, D x (27) which gives constD (28) Substituting from Equation (26) into Equations (19)- (25) and dropping the primes for convenience, we obtain the following set of non-dimensional equations Youssef [29] and Youssef and Bassiouny [30] follows: 22 22 e 2 2 e xt (29) eD (30) 22 1 22 o e t xt (31) and the following relation between the conductive tem- perature and the thermodynamical one: 2 2 (32) where 2 22 2,,and o E ac C x (33) The boundary conditions are: 1 0, t o e tt (34) ,0,0, ,0,0 ,0,0,0,0 tetett ttt 2 (35) where 0 , while the initial conditions are as- sumed to be: ,0 0,,0 0,,0 0,0xex xx (36) Applying the Laplace transform defined by: 0 d st Lftfse ft t (37) to both sides of Equations (29)-(32), we obtain: Open Access JAMP  E. BASSIOUNY, R. SABRY 113 22 2 22 dd dd e e xx (38) D e (39) 2 2 12 1d , doo 2 sss sx e (40) 2 2 d. d (41) Using Equations (29) and (30) with the definitions (23) and (37) we can obtain 2d d us (42) where s denotes the complex argument related to the Laplace transform. The transformed boundary conditions take the forms 1 0, t o e Fs t (43) where 1 1 o Fsss (44) while the Equations (35) become ,0, 0,,0 ,0, 0,0, seses ss , (45) and the corresponding transformed initial conditions of the Equations (36) assume the form: ,0,0,00, 0xex xx (46) Eliminating between Equations (40) and (41), we get: 2 DLLe (47) where 22 2 2 12 d and d o o ss LLs D sss Substituting from Equation (47) into Equation (41) we obtain 1LLe (48) Using Equation (47) we can easily eliminate be- tween Equation (38) and (48) to obtain 2 De MNe (49) where 2 1 1 1 and 1 LL MMs L LL NNs L (50) Solving Equations (47) and (49) together we get the following fourth order equation 42 0kakb (51) where 21 111 o o ss ass (52) 22 11 11 1 o o ss s bss (53) It is worth mentioning here that the roots of equation (51) are functions of s and assume the forms: 22 12 44 , 22 aa baa b kk (54) Thus the solutions of the Equations (47) and (49) sat- isfying the boundary conditions at infinity are: 1 12 2 k AeA e xk (55) 1 12 2 k eBe Be xk (56) where 121 ,, AB and 2 are coefficients depending on s to be determined using the boundary conditions (43) and (45). B 2 12 22 12 1o Fs ANks Ns kk s (57) 2 21 22 12 1o Fs ANks Ns kk s (58) While the constants 12 are related to the con- stants ,BB 12 , A according to the following relations: 211, 1 i iioo o A Bksss s 1,2 i (59) Substituting from Equations (57) and (58) into the Equations (55) and (56) the heat conduction and the strain field in the Laplace domain take the forms: 12 22 12 22 21 , 11 xk xk oo Fs xs Nskk ssNkessNke (60) 1 2 2 11 2 22 1 ,1 11 11 o xk oo xk oo exs s Ak ssse Ak ssse (61) Using the expressions of and of e from Equa- tions (60) and (61) to find the thermodynamical function Open Access JAMP 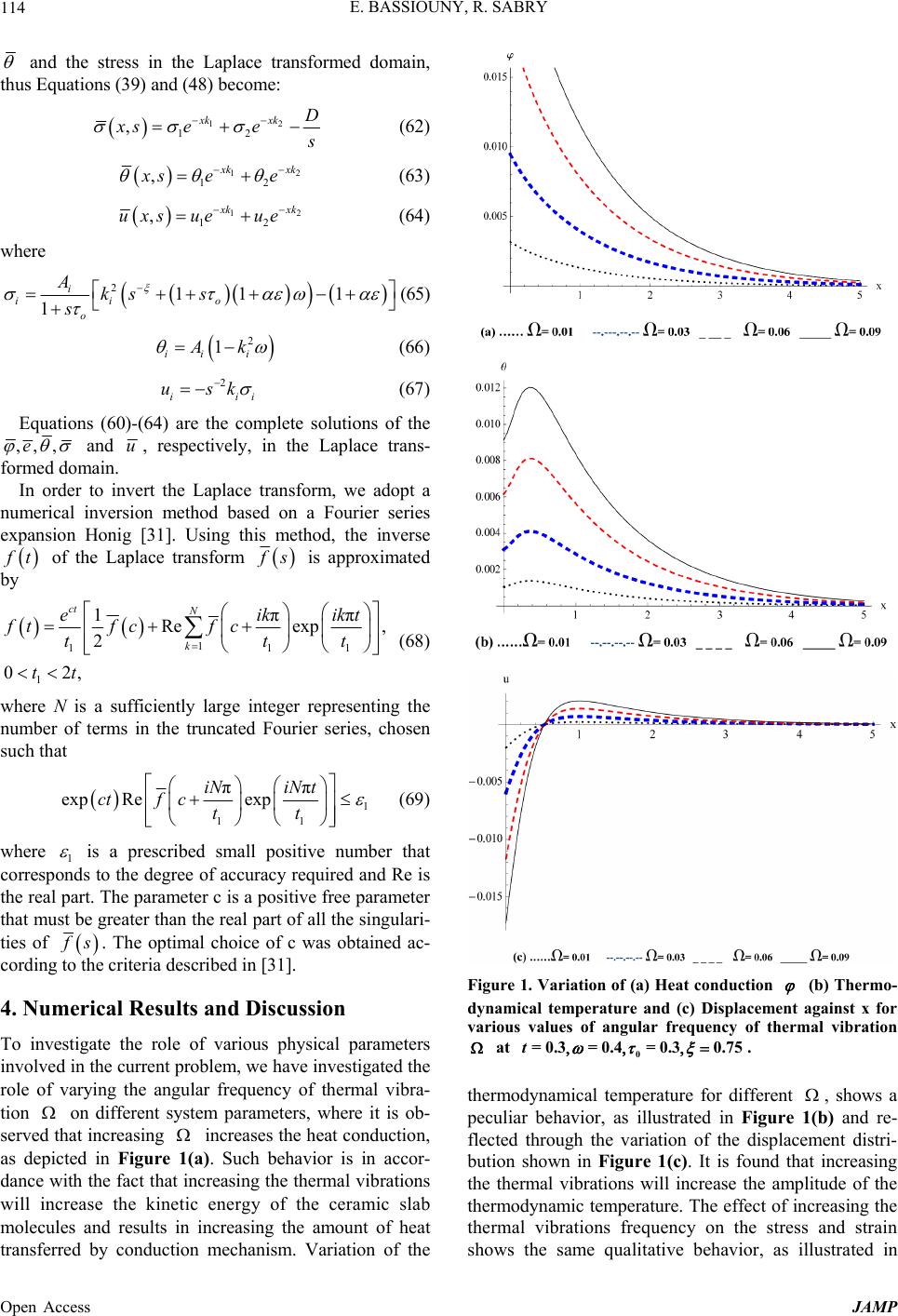 E. BASSIOUNY, R. SABRY 114 and the stress in the Laplace transformed domain, thus Equations (39) and (48) become: 12 12 ,xkxk D xs ee (62) 1 12 ,2 kxk se e (63) 1 12 ,2 k uxs ueue xk (64) where 2111 1 i ii o o Aks s s (65) 2 1 ii i Ak (66) 2 i usk i i (67) Equations (60)-(64) are the complete solutions of the ,, ,e and u, respectively, in the Laplace trans- formed domain. In order to invert the Laplace transform, we adopt a numerical inversion method based on a Fourier series expansion Honig [31]. Using this method, the inverse t of the Laplace transform s is approximated by 1 11 1 1ππ Reexp, 2 02, ct N k eik ftfcf c tt tt 1 ikt t (68) where N is a sufficiently large integer representing the number of terms in the truncated Fourier series, chosen such that 1 11 ππ exp Reexp iNiN t ctf ctt (69) where 1 is a prescribed small positive number that corresponds to the degree of accuracy required and Re is the real part. The parameter c is a positive free parameter that must be greater than the real part of all the singulari- ties of s. The optimal choice of c was obtained ac- cording to the criteria described in [31]. 4. Numerical Results and Discussion To investigate the role of various physical parameters involved in the current problem, we have investigated the role of varying the angular frequency of thermal vibra- tion on different system parameters, where it is ob- served that increasing increases the heat conduction, as depicted in Figure 1(a). Such behavior is in accor- dance with the fact that increasing the thermal vibrations will increase the kinetic energy of the ceramic slab molecules and results in increasing the amount of heat transferred by conduction mechanism. Variation of the Figure 1. Variation of (a) Heat conduc tion (b) Thermo- dynamical temperature and (c) Displacement against x for various values of angular frequency of thermal vibration at 0 =0.3 =0.4=0.30.75t . thermodynamical temperature for different , shows a peculiar behavior, as illustrated in Figure 1(b) and re- flected through the variation of the displacement distri- bution shown in Figure 1(c). It is found that increasing the thermal vibrations will increase the amplitude of the thermodynamic temperature. The effect of increasing the thermal vibrations frequency on the stress and strain shows the same qualitative behavior, as illustrated in Open Access JAMP 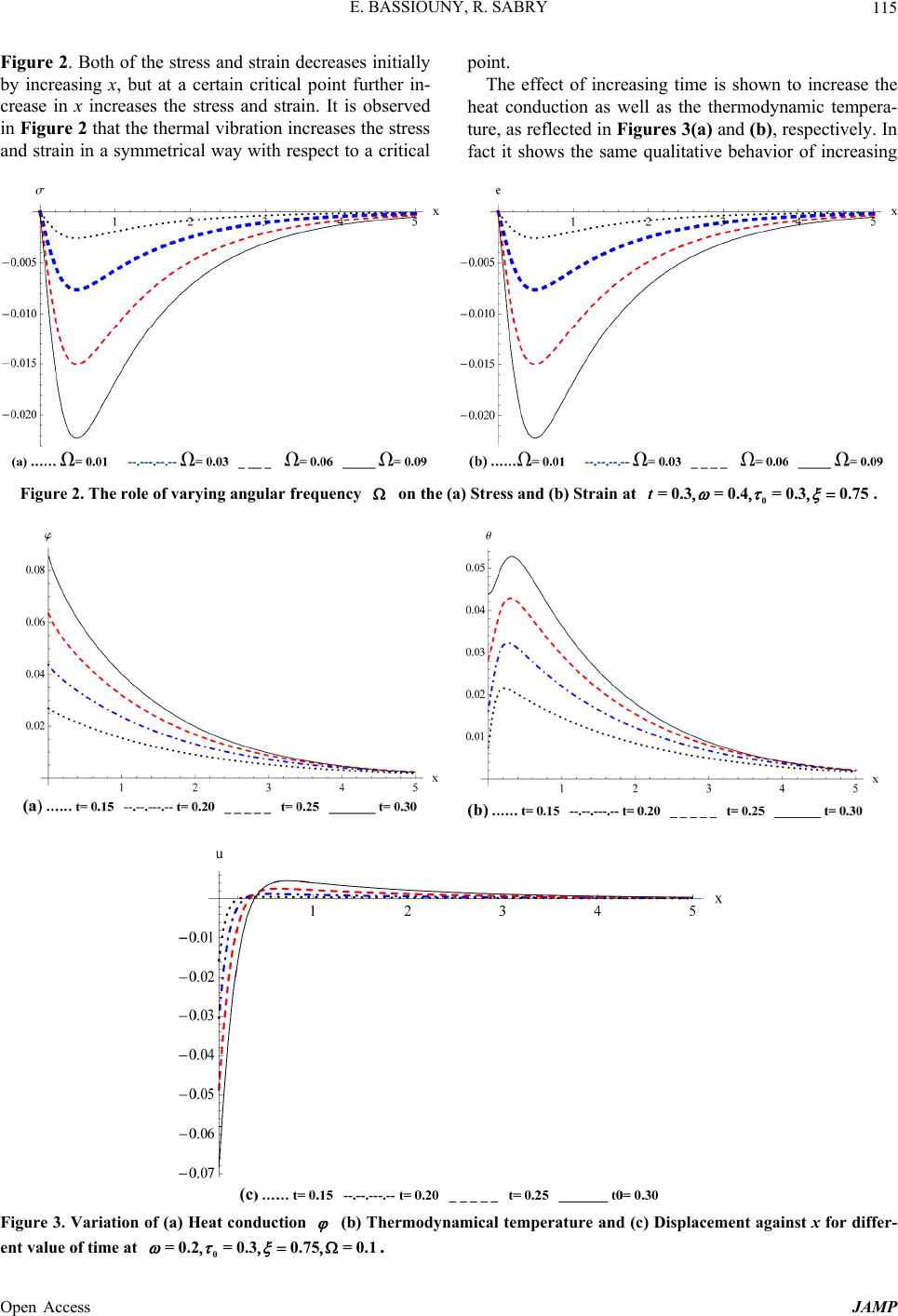 E. BASSIOUNY, R. SABRY Open Access JAMP 115 Figure 2. Both of the stress and strain decreases initially by increasing x, but at a certain critical point further in- crease in x increases the stress and strain. It is observed in Figure 2 that the thermal vibration increases the stress and strain in a symmetrical way with respect to a critical point. The effect of increasing time is shown to increase the heat conduction as well as the thermodynamic tempera- ture, as reflected in Figures 3(a) and (b), respectively. In fact it shows the same qualitative behavior of increasing Figure 2. The role of varying angular frequency on the (a) Stress and (b) Strain at 0 =0.3 =0.4=0.30.75t . Figure 3. Variation of (a) Heat conduction (b) Thermodynamical temperature and (c) Displacement against x for differ- nt value of time at 0 =0.2 =0.30.=0.175 . e 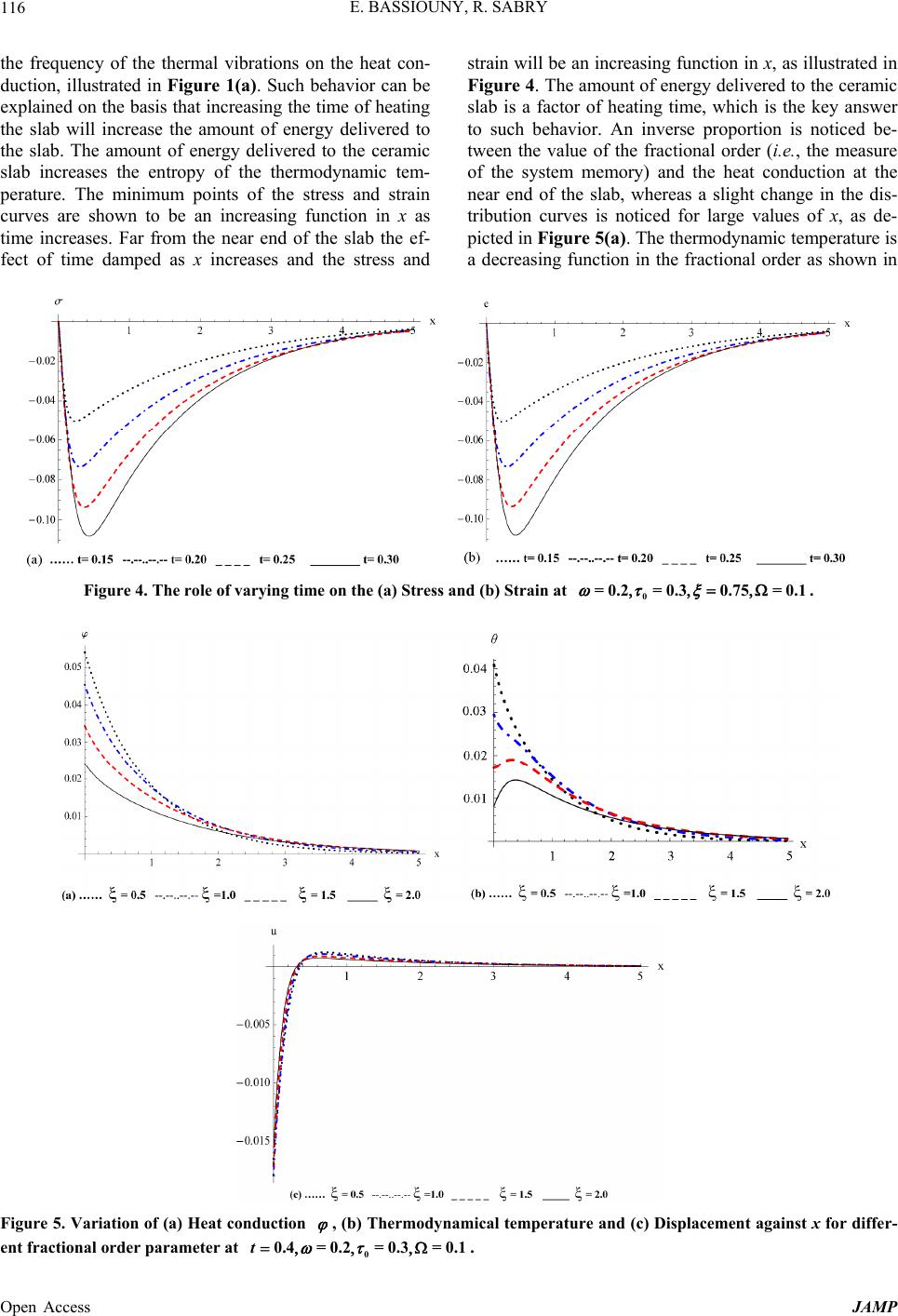 E. BASSIOUNY, R. SABRY 116 the frequency of the thermal vibrations on the heat con- duction, illustrated in Figure 1(a). Such behavior can be strain will be an increasing function in x, as illustrated in Figure 4. The amount of energy delivered to the ceramic explained on the basis that increasing the time of heating the slab will increase the amount of energy delivered to the slab. The amount of energy delivered to the ceramic slab increases the entropy of the thermodynamic tem- perature. The minimum points of the stress and strain curves are shown to be an increasing function in x as time increases. Far from the near end of the slab the ef- fect of time damped as x increases and the stress and slab is a factor of heating time, which is the key answer to such behavior. An inverse proportion is noticed be- tween the value of the fractional order (i.e., the measure of the system memory) and the heat conduction at the near end of the slab, whereas a slight change in the dis- tribution curves is noticed for large values of x, as de- picted in Figure 5(a). The thermodynamic temperature is a decreasing function in the fractional order as shown in Figure 4. The role of varying time on the (a) Stress and (b) Strain at 0 =0.2 =0.30.75 =0.1 . Figure 5. Variation of (a) Heat c onduction , (b) Thermodynamical temperature and (c) Displacement against x for differ- ent fractional order parameter at 0 0.40.2=0.3= 0.1t = . Open Access JAMP 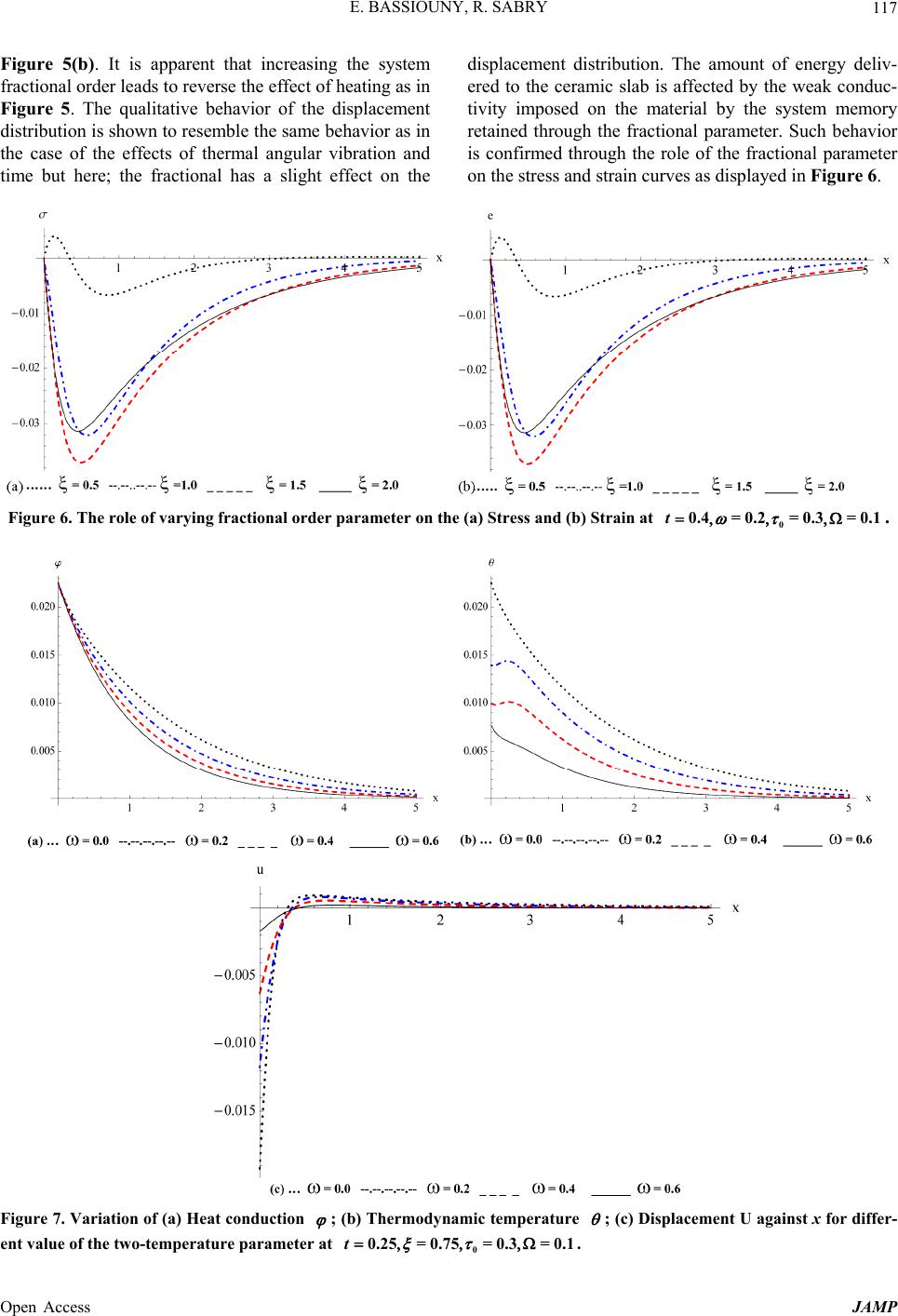 E. BASSIOUNY, R. SABRY 117 Figure 5(b). It is apparent that increasing the system ractional order leads to reverse t displacement distribution. The amount of energy deliv- er he effect of heating as in ed to the ceramic slab is affected by the weak conduc- f Figure 5. The qualitative behavior of the displacement distribution is shown to resemble the same behavior as in the case of the effects of thermal angular vibration and time but here; the fractional has a slight effect on the tivity imposed on the material by the system memory retained through the fractional parameter. Such behavior is confirmed through the role of the fractional parameter on the stress and strain curves as displayed in Figure 6. 0 0.4= 0.2= 0.3= 0.1t .Figure 6. The role of varying fractional order parameter on the (a) Stress and (b) Strain at Figure 7. Variation of (a) Heat c onduction ; (b) Thermodynamic temperature ; (c) Displacement U against x for differ- ent value of the two-temperature parameter at 0 0.25= 0.75= 0.3= 0.1t . Open Access JAMP  E. BASSIOUNY, R. SABRY 118 Figure 8. The role of varying the two-temperature parameter on the (a) Stress and (b) Strain at 0.25t 0 = 0.75= 0.3= 0.1 . pThe two temperaturearameter ω, which depends on o distinct temperatures, the conductive temperature an tw d the thermodynamic temperature where the difference between these two temperatures is proportional to the heat supply, is found to increase the heat conduction as shown in Figure 7(a). The thermodynamic temperature increases by increasing ω as illustrated in Figure 7(b). The displacement is found to have a critical behavior at 0.4x, as depicted in Figure 7(c) where the displace- ment distribution curves for all values of ω pass by this point and the distribution becomes positive after it. The strain distribution confirms similar qualitative be- havior as the stress distribution as illustrated in Figure 8. Both distributions of the stress and strain are decreased by increasing ω for 0.4 critical , as shown in Figure 8. However, for 0.4 the stress and strain distributions remain decreasing funcn the two temperature pa- rameter ω. tions i REFERENCES [1] P. J. Chen andy of Heat Con- duction InvolZeitschrift M. E. Gurtin, “On a Theor ving Two Temperatures,” für Angewandte Mathematik und Physik ZAMP, Vol. 19, No. 4, 1968, pp. 614-627. http://dx.doi.org/10.1007/BF01594969 [2] P. J. Chen, M. E. Gurtin Non-Simple Heat Conduction,” Zeits and W. O. Williams, “A Note on chrift für Ange- wandte Mathematik und Physik ZAMP, Vol. 19, No. 6, 1968, pp. 969-970. http://dx.doi.org/10.1007/BF01602278 [3] P. J. Chen, M. E. Gurtin and W. O. Williams, “On the Thermodynamics of Non-Simple Elastic Materials with Two Temperatures,” Zeitschrift für Angewandte Mathe- matik und Physik ZAMP, Vol. 20, No. 1, 1969, pp. 107- 112. http://dx.doi.org/10.1007/BF01591120 [4] B. A. Boley and I. S. Tolins, “Transient Coupled Ther- moelastic Boundary Value Problems in the Half-Space,” Journal of Applied Mechanics, Vol. 29, No. 4, 1962, pp. 637-646. http://dx.doi.org/10.1115/1.3640647 [5] W. E. Warren and P. J. Chen, “Wave Propagation in the Two-Temperature Theory of Thermoelasticity,” Acta Me- chanica, Vol. 16, No. 1-2, 1973, pp. 21-33. http://dx.doi.org/10.1007/BF01177123 [6] H. M. Youssef, “Theory of Two-Temperature General- ized Thermoelasticity,” IMA Journal of Applied Mathe- matics, Vol. 71, No. 3, 2006, pp. 383-390. http://dx.doi.org/10.1093/imamat/hxh101 [7] M. Caputo, “Linear Models of Dissipation Whose Q Is almost Frequently Independent II,” Geophysical Journal International, Vol. 13, No. 5, 1967, pp. 529-539. http://dx.doi.org/10.1111/j.1365-246X.1967.tb02303.x [8] F. Mainardi, “Fractional Calculus: Some Basic Problems ainardi, “Linear Model of Dissipation in Continuum and Statistical Mechanics,” In: A. Carpin- teri and F. Mainardi, Eds., Fractals and Fractional Cal- culus in Continuum Mechanics, Springer, New York, 1997, pp. 291-348. [9] I. Podlubny, “Fractional Differential Equations,” Aca- demic Press, New York, 1999. [10] R. Hilfer, “Application of Fraction Calculus in Physics,” World Scientific, Singapore, 2000. [11] M. Caputo and F. M in Inelastic Solids,” Rivis Ta El Nuovo Cimento, Vol. 1, No. 2, 1971, pp. 161-198. http://dx.doi.org/10.1007/BF02820620 [12] M. Caputo, “Vibrations of an Infinite Viscoelastic Layer with a Dissipative Memory,” The Journal of the Acousti- cal Society of America, Vol. 56, 1974, pp. 897-904. http://dx.doi.org/10.1121/1.1903344 [13] R. L. Bagley and P. J. Torvik, “A Theoretical Basis for the Application of Fractional Calculus to Viscoelasticity,” Journal of Rheology, Vol. 27, 1983, pp. 201-210. http://dx.doi.org/10.1122/1.549724 [14] R. C. Koeller, “Applications of Fractional Calculus to the Theory of Viscoelasticity,” Journal of Applied Mechanics, Vol. 51, No. 2, 1984, pp. 299-307. http://dx.doi.org/10.1115/1.3167616 [15] Yu. A. Rossikhin and M. V. Shitikova, “Applications of Fractional Calculus to Dynamic Problems of Linear and Open Access JAMP  E. BASSIOUNY, R. SABRY 119 Nonlinear Heredity Mechanics of Solids,” Applied Me- chanics Reviews, Vol. 50, No. 1, 1997, pp. 15-67. http://dx.doi.org/10.1115/1.3101682 [16] H. H. Sherief, A. El-Said and A. Abd El-Latief, “Frac- tional Order Theory of Thermoelasticity,” International Journal of Solids and Structures, Vol. 47, No. 2, 2010, pp. 269-275. http://dx.doi.org/10.1016/j.ijsolstr.2009.09.034 [17] M. A. Ezzat and A. S. El-Karamany, “On the Frac Thermo-Elasticity,” Mathematics and tional , Mechanics of Solid 2011. [18] I. Podlubny, “Fractional Differential Equations,” Aca- demic Press, New York, 1999. [19] R. Kimmich, “Strange Kinetics, Porous Media, and NMR,” Journal of Chemical Physics, Vol. 284, 2002, pp. 243-285. [20] Y. Fujita, “Integrodifferential Equation which Interpo- lates the Heat Equation and Wave Equation (II),” Osaka Journal of Mathematics, Vol. 27, 1990, pp. 797-804. [21] Y. Fujita, “Integrodifferential Equation which Interpo- lates the Heat Equation and Wave Equation (I),” Osaka Journal of Mathematics, Vol. 27, 1990, pp. 309-321. [22] Y. Z. Povstenko, “Fractional Heat Conductive and Asso- ciated Thermal Stress,” Journal of Thermal Stresses, Vol. 28, No. 1, 2004, pp. 83-102. http://dx.doi.org/10.1080/014957390523741 [23] Y. Z. Povstenko, “Theories of Thermal Stresses Based on Space-Time-Fractional Telegraph Equations,” Computers wa.2012.01.066 and Mathematics with Applications, Vol. 64, No. 10, 2012, pp. 3321-3328. http://dx.doi.org/10.1016/j.cam e la Chaleur Stresses, Vol. 27, No. 12, 2004, pp. [24] C. Cattaneo, “Sur une Forme de I’equation d Eliminant le Paradoxe d’une Propagation Instantanee’,” Comptes Rendus de l’Académie des Sciences, Vol. 247, 1958, pp. 431-433. [25] M. A. Ezzat and A. S. El-Karamany, “Discontinuities in Generalized Thermoviscoelasticity under Four Theories,” Journal of Thermal 1187-1212. http://dx.doi.org/10.1080/014957390523598 [26] M. A. Ezzat and A. S. El-Karamany, “Fractional Order Theory of a Prefect Conducting Thermoelastic Medium,” Canadian Journal of Physics, Vol. 89, No. 3, 2011, pp. 311-318. http://dx.doi.org/10.1139/P11-022 [27] M. A. Ezzat and A. S. El-Karamany, “Theory of Frac- tional Order in Electro-Thermoelasticity,” European Jour- nal of Mechanics A/Solids, Vol. 30, No. 4, 2011, pp. 491- 500. http://dx.doi.org/10.1016/j.euromechsol.2011.02.004 [28] A. S. El-Karamany and M. A. Ezzat, “Convolutional Va- riational Principle, Reciprocal and Uniqueness Theorems in Linear Fractional Two-Temperature Thermoelasticity,” Journal of Thermal Stresses, Vol. 34, No. 3, 2011, pp. 264-284. http://dx.doi.org/10.1080/01495739.2010.545741 [29] H. Youssef, “Theory of Fractional Order Generalized Ther- moelasticity, 2010, pp. 1-7. ” Journal of Heat Transfer, Vol. 132, No. 6, http://dx.doi.org/10.1115/1.4000705 thods l and Applied Mathematics, Vol. 10, No. 1, 1984, pp. [30] H. M. Youssef and E. Bassiouny, “Two-Temperature Gen- eralized Thermopiezoelasticity for One Dimensional Pro- blems—State Space Approach,” Computational Me in Science and Technology, Vol. 14, No. 1, 2008, pp. 55- 64. [31] G. Honig and U. Hirdes, “A Method of the Numerical Inversion of Laplace Transform,” Journal of Computa- tiona 113-132. http://dx.doi.org/10.1016/0377-0427(84)90075-X Open Access JAMP  E. BASSIOUNY, R. SABRY 120 Nomenclatures : The components of relaxation time. ture parameter. : Coefficient of linear thermal expansion. ij A a: The two-tempera C: Specific heat at constant strain. ijkl : The elastic constants. c 2 o c : Longitudinal wave sp eed. : The components of electric displacement. : The pyroe nsor. conductivity. vector. ment vector. i D i dlectric constants. i E: The components of electric field vector. ijk el: The components of strain te ijk h: The piezoelectric coefficients. ij k: The components of thermal. i q: The components of the heat flux T: Absolute temperature. o T: Reference temperature. Time. t: i u: Components of displace ectric potential function. i: The elv 2 o T : Dimensionless thermoelasti T : The components of dielectric tensor. ik ij : The thermal modulus. 32 T . c coupling con- stant. : The angular frequency of thermal vibration. : Kronecker delta function. ij C nstant : Dimensionless mechanical coupling co : The entropy density. C k : The thermal viscosity. o C CT o TT that : The dynamical temperature increment such 1 o o TT T . , : Lamé’s constants. : Mass density. ij : Components of stress tensor. x : The principal stress component. o : One relaxation time parameter. Open Access JAMP
|