Engineering, 2013, 5, 856-864
Published Online November 2013 (http://www.scirp.org/journal/eng)
http://dx.doi.org/10.4236/eng.2013.511104
Open Access ENG
Load-Measuring Pot Bearing with Built-In Load Cell
—Part I: Design and Performance
Jeong-Rae Cho, Young Jin Kim*, Jong-Won Kwark, Sung Yong Park,
Won Jong Chin, Byung-Suk Kim
Structural Engineering Research Division, Korea Institute of Construction Technology, Goyang-Si, Korea
Received September 4, 2013; revised October 4, 2013; accepted October 11, 2013
Copyright © 2013 Jeong-Rae Cho et al. This is an open access article distributed under the Creative Commons Attribution License,
which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
ABSTRACT
This paper presents the underlying principle and the results of various performance evaluations for a load-measuring pot
bearing with built-in load cell. The pot bearing composed of a pot made of steel in which an elastomer disk is inserted is
a bearing supporting larger loads than the elastomeric bearing and accommodating rotational movement. Owing to a
Poisson’s ratio close to 0.5, elastomer withstands hydrostatic pressure when confined in a rigid body. Accounting for
this principle, the vertical load applied on the pot bearing can be obtained by converting the pressure acting on the elas-
tomer. Therefore, a load-measuring pot bearing is developed in this study by embedding a load cell exhibiting remark-
able durability in the base plate of the bearing. The details for the insertion of the load cell in the base plate of the pot
were improved through finite element analysis to secure sufficient measurement accuracy. The evaluation of the static
performance of the pot bearing applying these improved details verified that the bearing exhibited sufficient accuracy
for the intended measurement purpose. The dynamic performance evaluation results indicated that accurate measure-
ment of the dynamic load was also achieved without time lag.
Keywords: Bridge Bearing; Pot Bearing; Load-Measuring; Load Cell
1. Introduction
Bridge bearings are elements transmitting the loads from
the superstructure to the substructure and allowing the
designer to make the bridge behave as intended by re-
straining or enabling movements of the superstructure.
Recently, efforts are being undertaken to supplement
such basic functions of the bearing with additional meas-
uring functions to help the erection and maintenance of
the bridge. Especially, the possibility to measure vertical
loads will enable to monitor or identify factors that may
affect the structural health of the bridge such as differen-
tial settlement of the foundations, eventual unbalanced
force caused by inexact installation of the superstructure,
severe change of the structural system during the service
life or overloaded vehicles. Moreover, the bridges of the
recently rapidly constructed high-speed railway are fea-
tured by the higher importance of the dynamic train loads
compared to the permanent loads and the large magnifi-
cation of the dynamic response occurring when the trains
run at speed close to the resonant speed. Accordingly, the
dynamic vertical load measured continuously by the
bridge bearing can be exploited to improve the service-
ability of the high-speed railway bridge like the riding
comfort of the passengers and the stability of the track.
The company Maurer [1] commercializes bearings
measuring the vertical load by inserting pressure sensors
in pot bearings and spherical bearings that have been
applied in real bridge sites. Agrawal et al. [2] developed
a smart bridge bearing system in which pressure sensor,
accelerometer, displacement sensor and thermocouple
are applied in an elastomeric bearing. Choo et al. [3]
proposed a multi-functional bridge bearing enabling to
measure loads and harvest energy using a piezocompo-
site electricity generating element (PCGE). Udd et al. [4]
and Chang et al. [5] developed load-measuring bearings
using FBG sensors.
The pot bearing composed of a pot made of steel in
which an elastomer disk is inserted is a bearing support-
ing larger loads than the elastomeric bearing and ac
commodating rotational movement. Owing to a Poisson’s
ratio close to 0.5, the elastomer is subjected to hydro-
*Corresponding author.

J.-R. CHO ET AL. 857
static pressure when confined in a rigid body. Consider-
ing the large stiffness of the steel pot composing the pot
bearing, the elastomer in the pot is also in a state close to
hydrostatic pressure. If this principle is used, the pressure
acting on the elastomer can be converted into the vertical
load applied on the pot bearing.
This paper presents the results obtained for a load-
measuring bearing equipped with a small size load cell
inserted to the bottom steel plate of the pot bearing. The
details for the insertion of the load cell in the base plate
of the pot were improved through finite element analysis
to secure sufficient measurement accuracy. The evalua-
tion of the static performance of the pot bearing applying
these improved details verified that the bearing exhibited
sufficient accuracy for the intended measurement pur-
pose. The dynamic performance evaluation results indi-
cated that accurate measurement of the dynamic load was
achieved without time lag.
2. Basic Principle
The elastomer confined in the pot bearing is in a state
close to hydrostatic pressure when loading is applied on
the pot bearing. In such case, the measurement of the
pressure can provide the total load applied on the bearing.
The load-measuring pot bearing with built-in load cell
proposed in this study has been conceived as shown in
Figure 1 by inserting a small size load cell equipped
with a load detection button on the bottom steel plate of
the pot bearing so that pressure is applied on the load
detection button [6-9]. Assuming hydrostatic pressure for
the elastomer, the linear relation between the total load F
and the load f transmitted to the load cell can be ex-
pressed as follows.
fSF  , (1)
where F = pA, f = pa, S = A/a. A is the area of the elas-
tomer disk; a is the area of the detection button of the
load cell; and, S is the area ratio of A to a. In Equation
(1), F and f are linearly proportional with a slope corre-
sponding to the area ratio S.
In order to secure the safety and accuracy of a meas-
uring device like the load cell, the appropriate capacity of
the device should be selected. The capacity of the built-in
load cell can be determined as follows from Equation (1).
Figure 1. Conceptual scheme of the load-measuring pot
bearing with built-in load cell.
ap
S
F
f max
max
max , (2)
where fmax is the capacity of the load cell; Fmax is the
maximum load applied on the bearing; and, pmax = Fmax
/A is the pressure in the elastomer disk occurring under
application of the maximum load. Assuming that the er-
ror of the whole load-measuring bearing is caused by the
error of the load cell, the corresponding theoretical error
can be expressed as follows.
e
SF
f
e
F
F
E


max
max
max
, (3)
where E and e are respectively the error rates of the
bearing and load cell; and, ΔF is the minimum measure-
ment unit of the load-measuring bearing that is the error.
If fmax is set as Fmax/S = pmax /a in Equations (2) and (3), E
= e and the error of the bearing is minimized regardless
of S, fmax and Fmax. In other words, selecting fmax to ap-
proach the right-hand side of Equation (2) is advanta-
geous for the accuracy.
The KS standards [10] prescribe a value of 40 MPa for
the allowable pressure of the elastomer disk in the pot
bearing, and the manufacturers apply values ranging be-
tween 25 MPa and 40 MPa. The allowable pressure of
the elastomer disk is a design factor determining the di-
ameter D of the elastomer disk. Accordingly, the capac-
ity of the load cell can be determined according to the
applied allowable stress from Equation (2). Table 1 ar-
ranges the capacity of the load cell according to the al-
lowable stress when applying a diameter of 10 mm for
the detection button of the load cell. In general, since
identical allowable pressure of the elastomer disk is ap-
plied by the same manufacturer, the load cell to be em-
bedded is decided regardless of the total capacity of the
bearing. For example, in the case of a manufacturer ap-
plying an allowable pressure of 30 MPa, a load cell with
capacity of about 2400 N can be adopted.
3. Insertion Details of Load Cell
In the load cell equipped with a load detection button, the
condition to achieve exact measurement is that the verti-
cal load must be transmitted through the button as shown
Table 1. Optimal capacity of the load cell according to the
allowable pressure of the elastomer disk (diameter of 10
mm for the detection button of the load cell).
Allowable pressure of
elastomer plate, pa (MPa) Optimal load cell capacity (N)
25 1963.5
30 2356.2
40 3141.6
Open Access ENG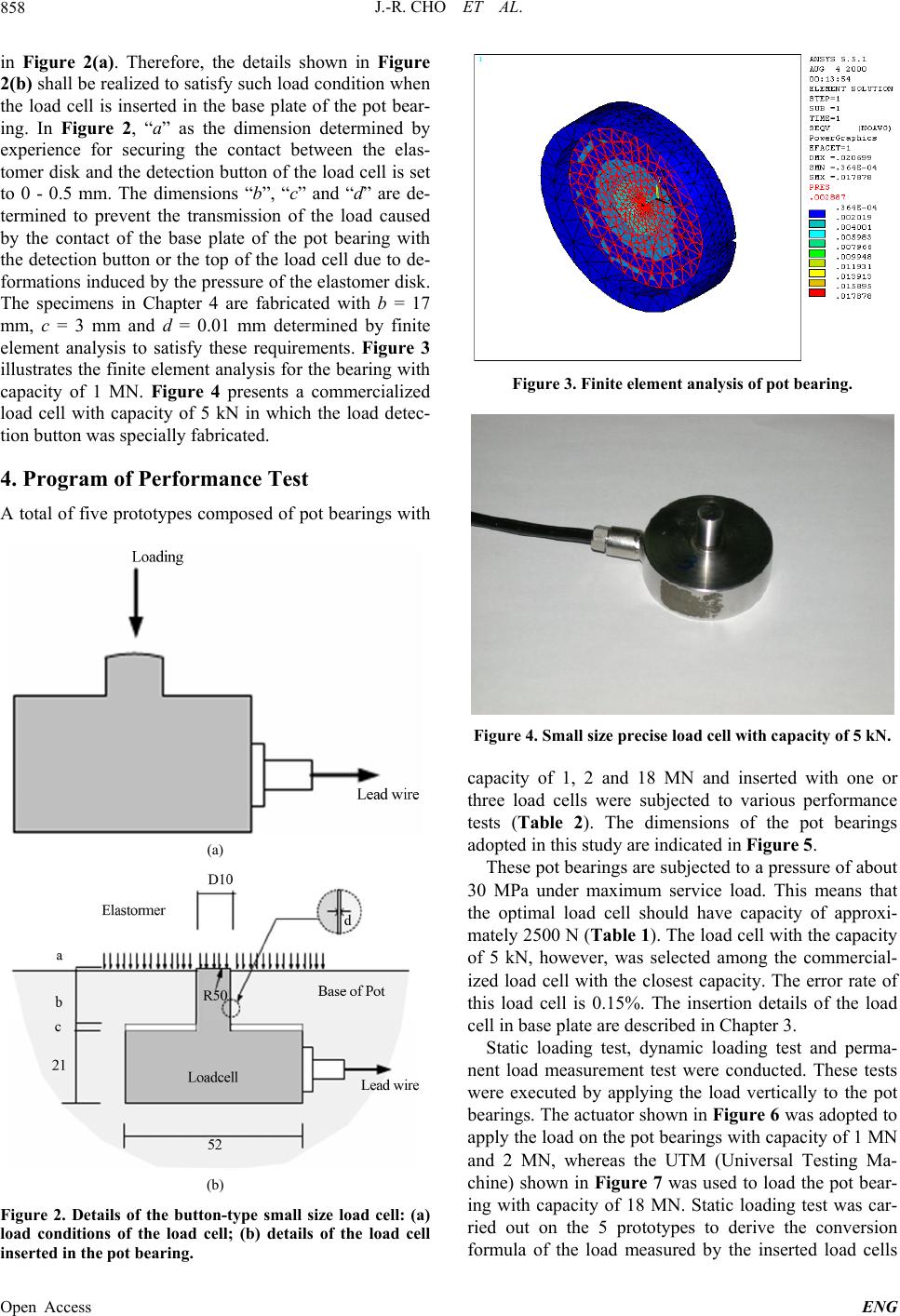

J.-R. CHO ET AL. 858
in Figure 2(a). Therefore, the details shown in Figure
2(b) shall be realized to satisfy such load condition when
the load cell is inserted in the base plate of the pot bear-
ing. In Figure 2, “a” as the dimension determined by
experience for securing the contact between the elas-
tomer disk and the detection button of the load cell is set
to 0 - 0.5 mm. The dimensions “b”, “c” and “d” are de-
termined to prevent the transmission of the load caused
by the contact of the base plate of the pot bearing with
the detection button or the top of the load cell due to de-
formations induced by the pressure of the elastomer disk.
The specimens in Chapter 4 are fabricated with b = 17
mm, c = 3 mm and d = 0.01 mm determined by finite
element analysis to satisfy these requirements. Figure 3
illustrates the finite element analysis for the bearing with
capacity of 1 MN. Figure 4 presents a commercialized
load cell with capacity of 5 kN in which the load detec-
tion button was specially fabricated.
4. Program of Performance Test
A total of five prototypes composed of pot bearings with
(a)
(b)
Figure 2. Details of the button-type small size load cell: (a)
load conditions of the load cell; (b) details of the load cell
inserted in the pot bearing.
Figure 3. Finite element analysis of pot bearing.
Figure 4. Small size precise load cell with capacity of 5 kN.
capacity of 1, 2 and 18 MN and inserted with one or
three load cells were subjected to various performance
tests (Table 2). The dimensions of the pot bearings
adopted in this study are indicated in Figure 5.
These pot bearings are subjected to a pressure of about
30 MPa under maximum service load. This means that
the optimal load cell should have capacity of approxi-
mately 2500 N (Table 1). The load cell with the capacity
of 5 kN, however, was selected among the commercial-
ized load cell with the closest capacity. The error rate of
this load cell is 0.15%. The insertion details of the load
cell in base plate are described in Chapter 3.
Static loading test, dynamic loading test and perma-
nent load measurement test were conducted. These tests
were executed by applying the load vertically to the pot
bearings. The actuator shown in Figure 6 was adopted to
apply the load on the pot bearings with capacity of 1 MN
and 2 MN, whereas the UTM (Universal Testing Ma-
chine) shown in Figure 7 was used to load the pot bear-
ing with capacity of 18 MN. Static loading test was car-
ried out on the 5 prototypes to derive the conversion
ormula of the load measured by the inserted load cells f
Open Access ENG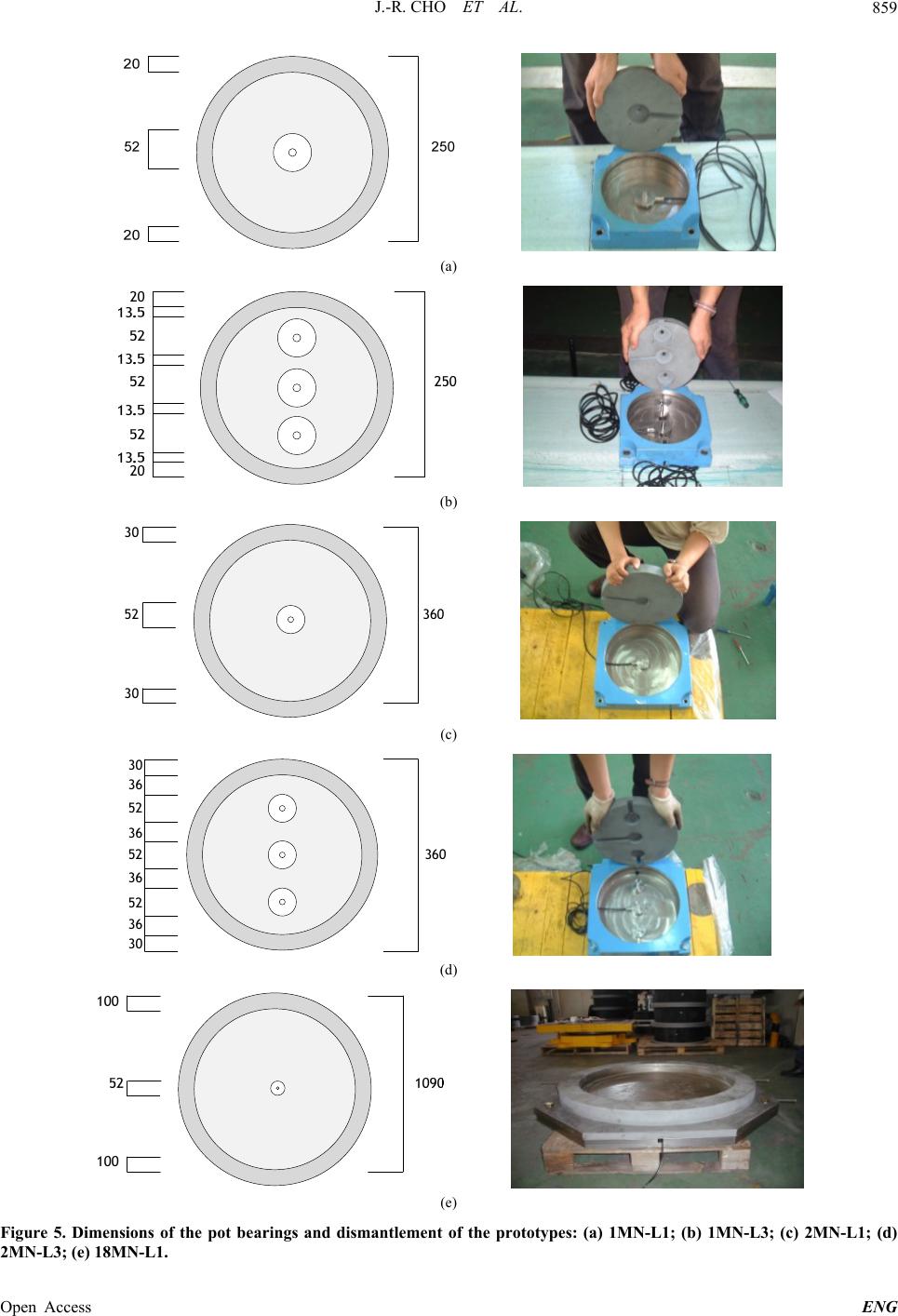

J.-R. CHO ET AL.
Open Access ENG
859
(a)
(b)
30
30
52 360
(c)
30
30
52
52
52
36
36
36
36
360
(d)
(e)
Figure 5. Dimensions of the pot bearings and dismantlement of the prototypes: (a) 1MN-L1; (b) 1MN-L3; (c) 2MN-L1; (d)
MN-L3; (e) 18MN-L1. 2

J.-R. CHO ET AL. 860
Figure 6. Test of specimen 1MN-L1.
Figure 7. Test of specimen 18MN-L1.
Table 2. Description of speci me ns.
Designation
of specimens
Capacity of
pot bearing
Diameter of
elastomer disk, D
No. of inserted
load cells
1 MN-L1 1 MN 210 mm 1
1 MN-L3 1 MN 210 mm 3
2 MN-L1 2 MN 300 mm 1
2 MN-L3 2 MN 300 mm 3
18 MN-L1 18 MN 890 mm 1
into the statically applied load and compute the accuracy.
Loading was applied up to 850 kN for the pot bearing
with capacity of 1 MN considering the capacity of the
actuator while loading was applied up to full capacity for
the pot bearings with the capacity of 2 MN and 18 MN.
Dynamic loading test was executed by applying a
permanent load with definite level from which the am-
plitude of the sinusoidal load was varied with a fre-
quency of 3 Hz. Dynamic loading test was performed on
specimen 1MN-L1 to verify the possibility to measure
the dynamic load caused by the vehicles traveling above
the pot bearing with built-in load cell.
Permanent load measurement test was conducted to
examine the measurement performance of the change in
the static load caused by the change in the bridge struc-
tural system. This series of test was carried out on
specimen 1MN-L1 by increasing the load from 600 kN to
700 kN by steps of 10 kN corresponding to 1% of the
capacity of the bearing and maintaining the increased
load during 1 minute at each step. Figure 8 describes the
loading histories applied in each performance test.
5. Test Results and Discussion
5.1. Static Loading Test
Static loading test was performed to derive the conver-
sion formula of the load measured by the inserted load
cells into the load applied statically on the pot bearing
and compute the measurement accuracy. Figure 9 plots
the static loading test results of each specimen where the
(a)
(b)
(c)
Figure 8. Loading histories of the performance tests: (a)
Static test; (b) Dynamic test; (c) Permanent load measure-
ent test. m
Open Access ENG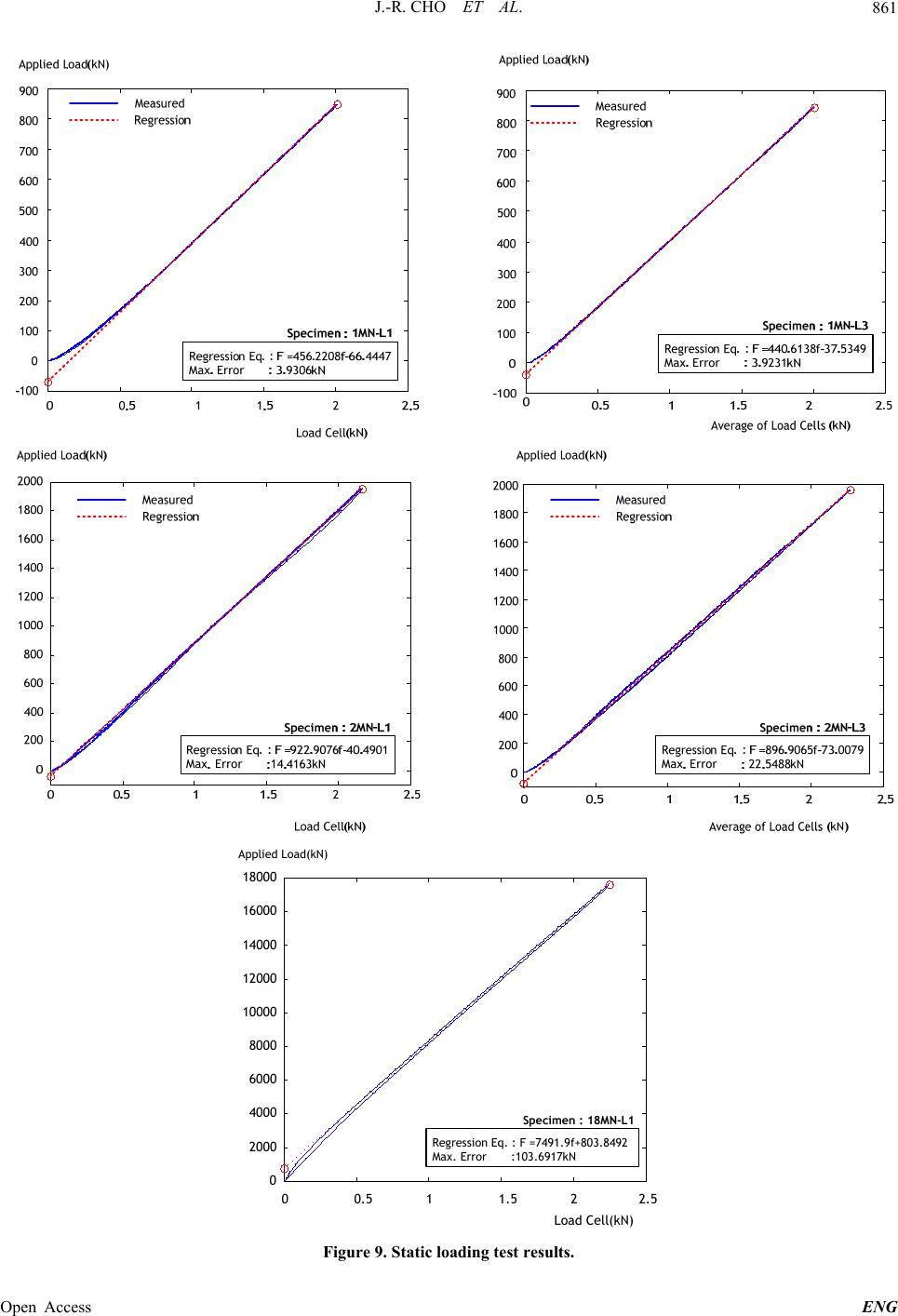

J.-R. CHO ET AL. 861
00.5 11.5 22.5
0
2000
4000
6000
8000
10000
12000
14000
16000
18000
Applied Load(kN)
Load Cell(kN)
Regression Eq. : F =7491.9f+803.8492
Max. Error :103.6917kN
Specimen : 18MN-L1
Figure 9. Static loading test results.
Open Access ENG

J.-R. CHO ET AL. 862
Y-axis is the applied vertical load and the X-axis is the
load measured by the load cell. The values in the case in
which three load cells are inserted correspond to the av-
erage of the three measurements.
All of the four specimens exhibit linear relation be-
tween the applied load and the load measured by the load
cell after a short nonlinear section. The nonlinear section
at the beginning appears during the period in which the
elastomer disk adheres to the load detection button of the
load cell due to the effect of the value of “a” in Figure 2.
It can be observed experimentally that this period occurs
at 30% of the capacity. Bridge bearings supporting the
superstructure (permanent loading state) are basically
loaded up to 40% - 60% of their capacity and experience
additional varying loads due to traffic. Therefore, the
load corresponding to 30% of the capacity in the nonlin-
ear section is in fact not applied to the bearings. Accord-
ingly, the section below 30% of the capacity is excluded
three load cells. Even if not indicated in Figure 9, the
central load cell in the specimens with three load cells
gave uniform results whereas the other two load cells
exhibited strong nonlinearity according to the increase or
decrease of the load. This nonlinearity can be attributed
to the eccentricity and shows that the use of one load cell
at the center is advantageous for measuring the vertical
load applied on the bearing.
Table 3 compares the conversion formulae calculated
from the test results and the theoretical conversion for-
mulae as well as the corresponding errors. The theoretic-
cal conversion formulae and errors are those obtained
when applying Equations (1) and (3) and correspond to
the cases where the elastomer disk is assumed to be con-
fined ideally in a rigid body. The results in Table 3 show
that the error ranges between 0.39% and 0.72% when one
1oad cell is inserted and is extremely close to the theo-
ng between 0.30% and 0.34%.
with built-in load cell. The test was executed by applying
a permanent load with definite level from which the am-
plitude of the sinusoidal load was varied with a fre-
quency of 3 Hz. Figure 10 plots the dynamic loading test
results for specimen 1MN-L1. In the figure, “Applied
Load” stands for the actually applied load and “Measured
Load” means the load obtained by the conversion for-
mula using the load measured by the built-in load cell.
The results show the possibility to measure the dynamic
load caused by traffic and without time lag.
5.3. Permanent Load Measurement Test
Permanent load measurement test was conducted to
examine the measurement performance of the change in
the static load caused by the change in the bridge
structural system. This series of test was carried out on
lae and errors per specimen.
Theoretical
ear regression
retical error rangi
from the analysis. In other words, lin
analysis is performed for the 1 MN, 2 MN and 18 MN
bearings considering the sections larger than 300 kN, 600
kN, and 5400 kN, respectively. For example, in the case
of specimen 1MN-L1, the computed regression equation
is F = 456.2208 f – 66.4447 where f stands for the load
(kN) measured by the load cell and F is the vertical load
(kN) on the bearing. This equation expresses thus the
conversion formula of the measured load into the applied
load on the bearing (in kN). The only load measurable in
the constructed bearing is the load measured by the load
cell. Therefore, the load applied on the bearing can be
obtained by applying this regression equation determined
in advance through static loading test.
The maximum error indicated in Figure 9 is the dif-
ference between the load computed by the regression
equation and the actual load applied on the bearing in the
sections with loads larger than 300 kN, 600 kN and 5400
kN. In view of the results, the maximum errors for the 1
MN bearings are similar regardless of the number of load
cells but the error appears to be smaller for the 2 MN
bearing with one load cell than the 2 MN bearing with
Table 3. Conversion formu
Experimental
5.2. Dynamic Test
Dynamic loading test was performed on specimen 1MN-
L1 to verify the possibility to measure the dynamic load
caused by the vehicles traveling above the pot bearing
Specimen
Conversion formula (kN) Error Conversion formula (kN)* Error**
1MN-L1 F = 456.2208 f – 66.4447 0.39%
1MN-L3 F = 440.6138 f – 37.5349
2MN-L1 F = 922.9076 f – 40.4901
0.39%
F = 441 f 0.33%
0.72%
1.10%
F = 900 f 0.34%
0.58% F = 7921 f 0.33%
2MN-L3 F = 896.9065 f – 73.0079
18MN-L1 F = 7491.900 f – 803.8492
*Theoretical formula obtained by applying (1); **Theoretical error obtained by applying Equation (3).
Open Access ENG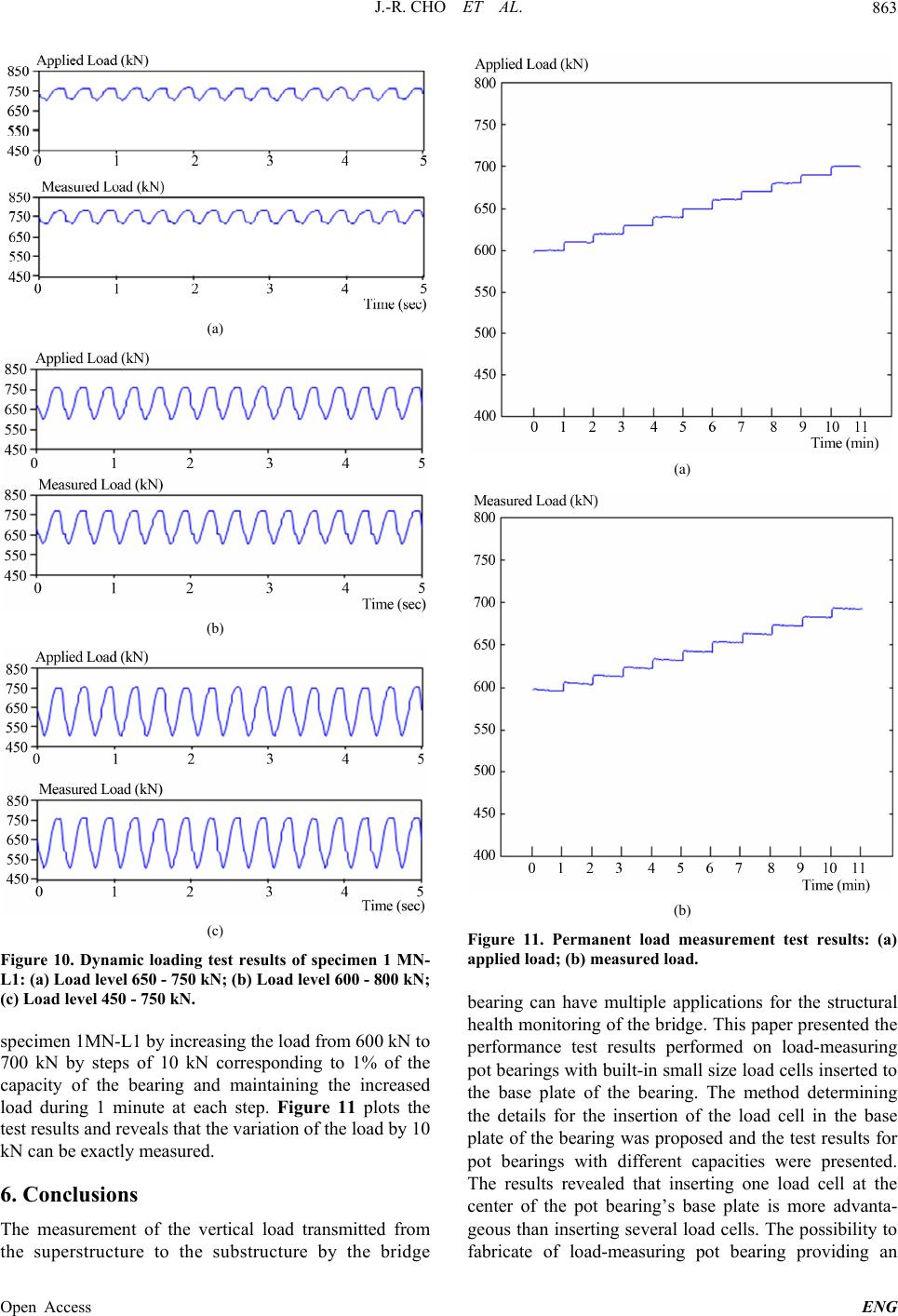

J.-R. CHO ET AL. 863
(a)
(b)
(c)
Figure 10. Dynamic loading test results of specimen 1 MN-
L1: (a) Load level 650 - 750 kN; (b) Load level 600 - 800 kN;
(c) Load level 450 - 750 kN.
specimen 1MN-L1 by increasing the load from 600 kN to
700 kN by steps of 10 kN corresponding to 1% of th
capacity of the bearing amaintaining the increased
load during 1 minute at each step. Figure 11 plots the
test results and reveals that the variation of the load by 10
kN can be exactly measured.
6. Conclusions
The measurement of the vertical load transmitted from
the superstructure to the substructure by the bridge
e
nd
(a)
(b)
Figure 11. Permanent load measurement test results: (a)
applied load; (b) measured load.
bearing can have multiple applications for the structural
health monitoring of the bridge. This paper presented the
performance test results performed on load-measuring
pot bearings with built-in small size load cells inserted to
the base plate of the beari The method determining
the details for the insertion of the load cell in the base
plate of the bearing was proposed and the test results for
pot bearings with different capacities were presented.
The results revealed that inserting one load cell at the
center of the pot bearing’s base plate is more advanta-
geous than inserting several load cells. The possibility to
fabricate of load-measuring pot bearing providing an
ng.
Open Access ENG

J.-R. CHO ET AL. 864
error close to the theoretical error corresponding to the
ideal case where the elastomer disk is confined in a rigid
body was verified experimentally. Moreover, measure-
ment of dynamic loading due to traffic was seen to be
possible without time lag.
The accuracy of the load-measuring pot bearing with
built-in load cell appeared to be extremely sensitive to
the details of the load cell inserted in the base plate of the
bearing and the selection of the appropriate load cell.
Besides, the effect of the temperature occurring in actual
bridge sites and the problem of durability caused by the
traffic loads should be investigated additionally for fur-
ther field application of theposed load-measuring pot
NCES
Bearings with Load Measuring Capabil-
/www.maurer-soehne.com
Study,” City University of New York, New York, 2005.
[3] J. F. Choo, D. H. Ha, N. S. Goo and W. S. Jang, “Pre-
liminary Tests for a Multi-Functional Bridge Bearing
with Built-in Piezoelectric Material,” Advanced Science
Letters, Vol. 19, No. 1, 2013, pp. 37-41.
http://dx.doi.org/10.1166/asl.2013.4714
[4] E. Udd, W. L. Schulz, J. M. Seim, K. Corona-Bittick, J.
Dorr, K. T. Slattery, H. M. Laylor and G. E. McGill, “Fi-
ber Optic Smart Bearing Load Structure,” In: S. B. Chase,
Ed., Nondestructive Evaluation of Bridges and Highways
III, 1999, pp. 40-48. http://dx.doi.org/10.1117/12.339933
[5] S. J. Chang, N. S. Kim and J. H. Baek, “Development of
Smart Seismic Device Using FBG Sensor for Measuring
Vertical Load,” Transactions of the Korean Society for
Noise and Vibration Engineering, Vol. 22, No. 11, 2012,
pp. 1089-1098, in Korean.
http://dx.doi.org/10.5050/KSNVE.2012.22.11.1089
pro
bearing.
7. Acknowledgements
This research was supported by a grant from a Strategic
Research Project (Development of smart prestressing
system for prestressed concrete bridges) funded by the
Korea Institute of Construction Technology.
REFERE
[1] Maurer, “Bridge
ity,” 2013. http:/
[2] A. K. Agrawal, K. Subramaniam and Y. Pan, “Develop-
ment of Smart Bridge Bearings System: A Feasibility
[6] J.-R. Cho, Y. J. Kim, S. Y. Park, B.-S. Kim and J. I. Lee,
“Development of High Performance Bearing for Bridge I:
Load Measurable Bearing,” Proceedings of the 2002
Conference of the Korean Society of Civil Engineers,
2002, pp. 221-224, in Korean.
[7] Korea Institute of Construction Technology, “R&D on
the Safety of High-Speeilway Structures,” R&D Re-
the Safety of High-Speed Railway Structures,” R&D Re-
d Ra
port, 2001, in Korean.
[8] Korea Institute of Construction Technology, “R&D on
port, 2002, in Korean.
[9] Korea Institute of Construction Technology, “Develop-
ment of Stabilization Techniques for Track Construction
System of the High-speed Railway,” R&D Report, 2003,
in Korean.
[10] Korean Standards Association, KS F 4424, “Pot Bearings
for Bridges,” 1996, in Korean.
Open Access ENG