Paper Menu >>
Journal Menu >>
 J ournal o f A pp http://dx.doi.or g Copyright © 2 0 Coll e ABSTRA C The numbers respectively. W p roduct states Keywords: G 1. Introdu c Graph states a associated to tions in quan t tum compute r measures ha v states, such a s [8], the Relat i Geometric M ment measur e known that t h Thus these en state. The ge o to its closest entanglement b een calculat e [13]. We wil l of 9 to 11 q u paper. Anoth e the Schmidt m 2. Prelimi n The geometr i ψ , is define d where Pro i (;)GV i s of edges spe c an nn sy m entries and 0 ab oth e p lied Mathemat i g /10.4236/jamp . 0 13 SciRes. Enta n G e ge of Informat i C T of local com p W e calculate t for all these g G raph State; E n c tion a re certain pu r graphs [1-5] t um error corr e r [7]. A vari e v e been propo s , the (Global ) i ve Entropy o f M easure [11]. e s are all equa l h e graph state tanglement m e o metric meas u product state of graph stat e e d and the cl o l consider the u bits and their e r closely rel a m easure [4,14] . n ar y i c measure o f d as () m g E i s the set o s composed o f c ified by the a m metric mat r 1 ab if ver t e rwise. The gr a i cs and Ph y sics , .2013.14010 P u n gleme n G raph Cui f i on and Electro n p limentary in e the entangle m g raph states. N n tanglemen t ; C r e multipartite and have im p e ction [6] and e ty of differe n sed for multi p ) Robustness o f Entanglemen t Fortunately, l for stabilize r is a subset o f e asures are all u re is the dist a in terms of t h e s of 1 to 8 q u o sest product s entanglemen t closest prod u a ted entangle m . f entanglemen t 2 Pr log m in o o f product s t f a set V of n v djacency mat r r ix with van i t ices ,ab ar e a ph state rela t , 2013, 1, 51-5 5 u blished Online O n t and C States w f eng Wang, L n ic Engineering , Email: w c Recei v e quivalent gra p m ent, the lowe r ew patterns o f C losest Produc t quantum stat e p ortant applic one-way qua n nt entangleme n p artite quantu m o f Entangleme n t [9,10], and t h these entangl r state [12]. It f stabilizer sta t equal for gra p a nce of the st a h e fidelity. T h u bit systems h a s tates are fou n of graph stat e u ct states in t h m ent measure t for pure st a 2 . ( t ates. A gra p ertices and a s r ix , which i shing diago n e connected a n t ed to graph G 5 O ctobe r 2013 ( h C losest w ith 9 t L izhen Jian g , Zhejiang, Gon g c f_0815@126. c v e d August 201 3 p h states for 9 r and upper b o f closest produ t State e s a- n - n t m nt h e e- is t e. p h a te h e a s nd e s h is is a te 1) p h et is n al n d G is defi n where vector s G c a where p ure s t graph s only L C concer n inequi v numbe r 146, th and th e noted w The ation a L OCC E b er of fectly b b iparti t “matc h For a have E determ i h ttp://www.scir p Produ c t o 11 Q u g , Lei Wang g shang Univers i c o m 3 9 , 10 and 11 q o unds of the e ct states are a n n ed as G 0 = (0, 0,…, s of length n. a n be written a ()EG ( j pj t ate. All loc a s tates have th e C inequivale n n ing with the v alent graph s r of LC inequ i e entangleme n e closest prod u w ith .1No t o entanglement a nd classica l 2 lognN , w graph basis s t b y LOCC [1 3 t e entangleme n h ing” bound E E a large numbe r bi LOGG E E, t h i ned. While fo p .org/journal/ ja m c t State u bits i ty, Hangzhou, 3 q ubit systems e ntanglement n alyzed. 1 1 2 0 1(1) 2n 0) and 1 = ( The entangle m a s () m g EG 01 pj pj e a l complime n e same amoun n t graph states entanglemen t tates increase i valent graph s n t is calculate d u ct states are f o .146No . is upper bou n l communica w here N is t t ates that can 3 ,15], and lo w n t deduced fro m bi E [16]. Thus () bi E EG r of graph stat e h e entangle m fo r graph stat e m p ) s of 3 10018, China are 440, 313 2 and obtain th e 1 2 T z 1, 1,…, 1) a r m ent of a gr a 2 log m in G 1) j i e is the n tary (LC) e q ts of entangle m should be c o t . The numb e s with n rapi d s tates up to 8 d with iterativ e f ound [13] an d n ded by the lo c tion (LOCC ) t he the maxi m be discrimin a w er bounded b m the state, t h LOGG E e s considered m ent can be e e s with unequ JAMP 2 , 40457, e closest (2) r e binary a ph state 2 (3) product q uivalent m ent, so o nsidered e r of LC d ly. The qubits is e method d are de- c al oper- ) boun d m al nu m - a ted per- b y some h at is, the (4) later, we e asily be u al lower 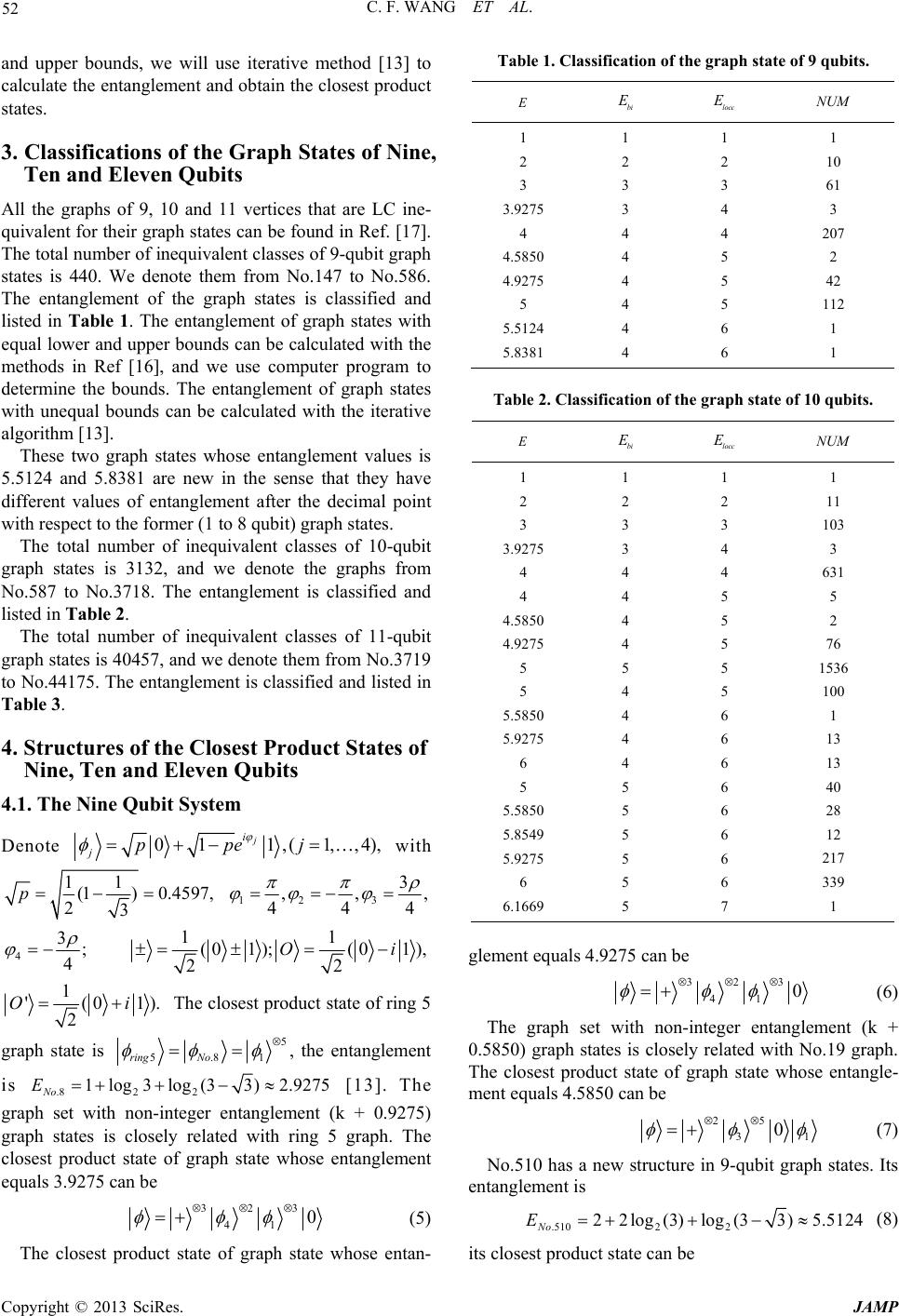 C. F. WANG ET AL. Copyright © 2013 SciRes. JAMP 52 and upper bounds, we will use iterative method [13] to calculate the entanglement and obtain the closest product states. 3. Classifications of the Graph States of Nine, Ten and Eleven Qubits All the graphs of 9, 10 and 11 vertices that are LC ine- quivalent for their graph states can be found in Ref. [17]. The total number of inequivalent classes of 9-qubit graph states is 440. We denote them from No.147 to No.586. The entanglement of the graph states is classified and listed in Table 1. The entanglement of graph states with equal lower and upper bounds can be calculated with the methods in Ref [16], and we use computer program to determine the bounds. The entanglement of graph states with unequal bounds can be calculated with the iterative algorithm [13]. These two graph states whose entanglement values is 5.5124 and 5.8381 are new in the sense that they have different values of entanglement after the decimal point with respect to the former (1 to 8 qubit) graph states. The total number of inequivalent classes of 10-qubit graph states is 3132, and we denote the graphs from No.587 to No.3718. The entanglement is classified and listed in Table 2. The total number of inequivalent classes of 11-qubit graph states is 40457, and we denote them from No.3719 to No.44175. The entanglement is classified and listed in Table 3. 4. Structures of the Closest Product States of Nine, Ten and Eleven Qubits 4.1. The Nine Qubit System Denote 01 1,(1,,4), j i jppej with 11 (1) 0.4597, 23 p12 3 3 ,,, 444 4 3; 4 11 (0 1);(01), 22 Oi 1 '(01). 2 Oi The closest product state of ring 5 graph state is 5 5.81ring No , the entanglement is .822 1log3log(33)2.9275 No E [13]. The graph set with non-integer entanglement (k + 0.9275) graph states is closely related with ring 5 graph. The closest product state of graph state whose entanglement equals 3.9275 can be 323 41 0 (5) The closest product state of graph state whose entan- Table 1. Classification of the graph state of 9 qubits. E bi E locc E NUM 1 2 3 3.9275 4 4.5850 4.9275 5 5.5124 5.8381 1 2 3 3 4 4 4 4 4 4 1 2 3 4 4 5 5 5 6 6 1 10 61 3 207 2 42 112 1 1 Table 2. Classification of the graph state of 10 qubits. E bi E locc E NUM 1 2 3 3.9275 4 4 4.5850 4.9275 5 5 5.5850 5.9275 6 5 5.5850 5.8549 5.9275 6 6.1669 1 2 3 3 4 4 4 4 5 4 4 4 4 5 5 5 5 5 5 1 2 3 4 4 5 5 5 5 5 6 6 6 6 6 6 6 6 7 1 11 103 3 631 5 2 76 1536 100 1 13 13 40 28 12 217 339 1 glement equals 4.9275 can be 323 41 0 (6) The graph set with non-integer entanglement (k + 0.5850) graph states is closely related with No.19 graph. The closest product state of graph state whose entangle- ment equals 4.5850 can be 5 2 31 0 (7) No.510 has a new structure in 9-qubit graph states. Its entanglement is .5102 2 22log (3)log (33)5.5124 No E (8) its closest product state can be 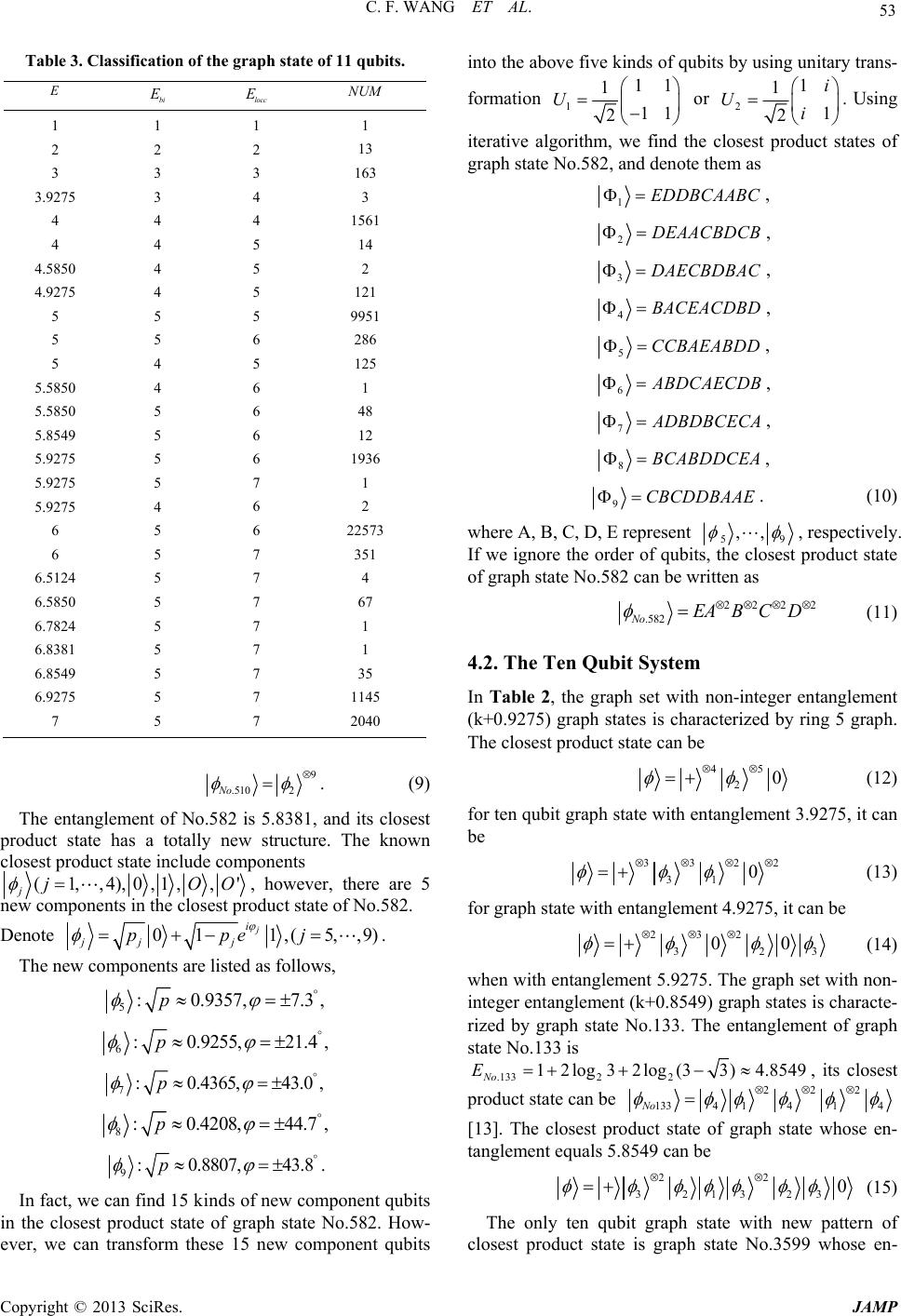 C. F. WANG ET AL. Copyright © 2013 SciRes. JAMP 53 Table 3. Classification of the graph state of 11 qubits. E bi E locc E NUM 1 2 3 3.9275 4 4 4.5850 4.9275 5 5 5 5.5850 5.5850 5.8549 5.9275 5.9275 5.9275 6 6 6.5124 6.5850 6.7824 6.8381 6.8549 6.9275 7 1 2 3 3 4 4 4 4 5 5 4 4 5 5 5 5 4 5 5 5 5 5 5 5 5 5 1 2 3 4 4 5 5 5 5 6 5 6 6 6 6 7 6 6 7 7 7 7 7 7 7 7 1 13 163 3 1561 14 2 121 9951 286 125 1 48 12 1936 1 2 22573 351 4 67 1 1 35 1145 2040 9 .510 2No . (9) The entanglement of No.582 is 5.8381, and its closest product state has a totally new structure. The known closest product state include components (1,,4),0,1,,' jjOO , however, there are 5 new components in the closest product state of No.582. Denote 01 1,(5,,9) j i jj j ppej . The new components are listed as follows, 5:0.9357,7.3 ,p 6:0.9255,21.4 ,p 7:0.4365,43.0 ,p 8:0.4208,44.7,p 9:0.8807, 43.8p . In fact, we can find 15 kinds of new component qubits in the closest product state of graph state No.582. How- ever, we can transform these 15 new component qubits into the above five kinds of qubits by using unitary trans- formation 1 11 1 11 2 U or 2 1 1 1 2 i Ui . Using iterative algorithm, we find the closest product states of graph state No.582, and denote them as 1EDDBCAABC , 2DEAACBDCB , 3DAECBDBAC , 4BACEACDBD, 5CCBAEABDD, 6ABDCAECDB , 7ADBDBCECA , 8BCABDDCEA , 9CBCDDBAAE . (10) where A, B, C, D, E represent 59 ,, , respectively. If we ignore the order of qubits, the closest product state of graph state No.582 can be written as 222 2 .582 No EA B CD (11) 4.2. The Ten Qubit System In Table 2, the graph set with non-integer entanglement (k+0.9275) graph states is characterized by ring 5 graph. The closest product state can be 45 20 (12) for ten qubit graph state with entanglement 3.9275, it can be 3 322 31 0 (13) for graph state with entanglement 4.9275, it can be 3 22 323 00 (14) when with entanglement 5.9275. The graph set with non- integer entanglement (k+0.8549) graph states is characte- rized by graph state No.133. The entanglement of graph state No.133 is .1332 2 12 log32 log(33)4.8549 No E , its closest product state can be 222 13341 4 1 4 No [13]. The closest product state of graph state whose en- tanglement equals 5.8549 can be 22 3 21323 0 (15) The only ten qubit graph state with new pattern of closest product state is graph state No.3599 whose en-  C. F. WANG ET AL. Copyright © 2013 SciRes. JAMP 54 tanglement is .3599 2 32log 36.1699 No E (16) the closest product state can be 5 22 .35993 431 No (17) 4.3. The Eleven Qubit System For eleven qubit graph states show in Table 3, a detail comparison of computed closest product states of No.3724, No.3765 shows that all these closest states have a substructure of the closest product state of ring 5 graph. Ring 5 graph is essential to all these graph states with entanglement k + 0.9275 (integer k). The closest product states can be 4 5 .37242 30 No (18) 422 .37652 42 10 No (19) The graph set (No.3764, No.3936) with non-integer entanglement (k + 0.5850) graph states is characterized by graph No.19. The entanglement of graph state No.19 is .19 2 2log 33.5850 No E Typically, the closest product state is 33 .193 4 No [13]. The closest state for No.3764 and No.3936 graph state can be 2 4 .3764232 31 0 No (20) 32 .39363 2 442 00 No (21) respectively. The graph set (No.4113, No.30597) with non-integer entanglement (k + 0.8549) graph states is characterized by graph state No.133. The closest state for No.4113 and No.30597 graph state can be 23 .41131 3 1 4 1 40 No , 222 .305971111 4 00 No . The entanglement of No.30505 is a new type in 11-qubit graph states. Its entanglement is .30505 2 23log(33)6.7824 No E (22) its closest product state can be 42 .305051414141 No (23) The entanglement of No.23813 is 6.8381, the closest product state of No.23813 can be .23813 1 No BEDB DCAAC (24) It contains the closest product of graph state No.582 .582No as its substructure. Note that, we have just found one of the many closest product states for each LC inequivalent graph state. Ac- tually, there are many local equivalent closest product states for the same graph state. 5. Conclusions We calculate the entanglement of all local complimenta- ry inequivalent graph states with nine, ten and eleven qubits. The total number of the graph states we treated is 44029, and we list the numbers of them in Tables 1-3 according to their entanglement. Further calculation for twelve qubits is quite difficult since there are more than 1.27 million of local complimentary inequivalent graph states. Four new types of non-integer entanglement val- ues appear in 9, 10 and 11 qubit graph states. The detail results and some special characters of the closest product states are as follows: (1) The graph sets with non-integer entanglement (k + 0.9275, k + 0.5850 and k + 0.8549) graph states are specified by ring 5 graph, No.19 graph and No.133 graph, respectively. Their closest product states contain five, six and eight (1,,4) jj ; (2) The closest product states of graph states with non-in- teger entanglement (5.5124 for nine qubits, 6.1669 for ten qubits, 6.7824 for eleven qubits) contains 9, 10 and 11 (1,,4) jj , respectively; (3) The graph states with non-integer entanglement (k + 0.8381) have a new structure in their closest product states. We find 5 new components (5,,9) jj in the closest product states. (4). The closest product state for graph state with integer entanglement does not contain (5,,9) jj . 6. Acknowledgements Funding by the National Natural Science Foundation of China (Grant No. 60972071), Natural Science Founda- tion of Zhejiang Province (Grant No. Y6100421), Zhe- jiang Province Science and Technology Project (Grant No. 2009C31060) are gratefully acknowledged. REFERENCES [1] R. Raussendorf, D. E. Browne and H. J. Briegel, “Mea- surement-Based Quantum Computation on Cluster States,” Physical Review A, Vol. 68, No. 2, 2003, Article ID: 022312. http://dx.doi.org/10.1103/PhysRevA.68.022312 [2] D.-M. Schlingemann, Quant. Inf. Comp., Vol. 2, 2002, p. 307. [3] D.-M. Schlingemann, Quant. Inf. Comp., Vol. 4, 2002, p. 287. [4] M. Hein, J. Eisert and H. J. Briegel, “Multiparty Entan- glement in Graph States,” Physical Review A, Vol. 69, No. 6, 2004, Article ID: 062311. http://dx.doi.org/10.1103/PhysRevA.69.062311 [5] M. Hein, W. Dur, J. Eisert, R. Raussendorf, M. Van den Nest and H. J. Briegel, In G. Casati, D. L. Shepelyansky, P. Zoller and G. Benenti, Eds., Quantum Computers, Al- gorithms and Chaos, IOS Press, Amsterdam, 2006. [6] M. Grassl, A. Klappenecker and M. Rotteler, “Graphs,  C. F. WANG ET AL. Copyright © 2013 SciRes. JAMP 55 Quadratic Forms, and Quantum Codes,” Proceedings of 2002 IEEE International Symposium on Information Theory, Lausanne, Switzerland, p. 45. http://dx.doi.org/10.1109/ISIT.2002.1023317 [7] R. Raussendorf and H. J. Briegel, “A One-Way Quantum Computer,” Physical Review Letters, Vol. 86, No. 22, 2001, pp. 5188-5191. http://dx.doi.org/10.1103/PhysRevLett.86.5188 [8] G. Vidal and R. Tarrach, “Robustness of Entanglement,” Physical Review A, Vol. 59, No. 1, 1999, pp. 141-150. http://dx.doi.org/10.1103/PhysRevA.59.141 [9] V. Vedral, M. B. Plenio, M. A. Rippin and P. L. Knight, “Quantifying Entanglement,” Physical Review Letters, Vol. 78, No. 12, 1997, pp. 2275-2279. http://dx.doi.org/10.1103/PhysRevLett.78.2275 [10] V. Vedral and M. B. Plenio, “Entanglement Measures and Purification Procedures,” Physical Review A, Vol. 57, No. 3, 1998, pp. 1619-1633. http://dx.doi.org/10.1103/PhysRevA.57.1619 [11] T.-C. Wei and P. M. Goldbart, “Geometric Measure of Entanglement and Applications to Bipartite and Multipar- tite Quantum States,” Physical Review A, Vol. 68, No. 4, 2003, Article ID: 042307. http://dx.doi.org/10.1103/PhysRevA.68.042307 [12] M. Hayashi, D. Markham, M. Murao, M. Owari and S. Virmani, “Entanglement of Multiparty-Stabilizer, Sym- metric, and Antisymmetric States,” Physical Review A, Vol. 77, No. 1, 2008, Article ID: 012104. http://dx.doi.org/10.1103/PhysRevA.77.012104 [13] X. Y. Chen, “Entanglement of Graph States up to Eight Qubits,” Journal of Physics B, Vol. 43, No. 8, 2010, Ar- ticle ID: 085507. http://dx.doi.org/10.1088/0953-4075/43/8/085507 [14] A. Cabello, A. J. Lopez-Tarrida, P. Moreno and J. R. Portillo, “Entanglement in Eight-Qubit Graph States,” Physics Letters A, Vol. 373, No. 26, 2009, pp. 2219-2225. http://dx.doi.org/10.1016/j.physleta.2009.04.055 [15] M. Hayashi, D. Markham, M. Murao, M. Owari and S. Virmani, “Bounds on Multipartite Entangled Orthogonal State Discrimination Using Local Operations and Clas- sical Communication,” Physical Review Letters, Vol. 96, No. 4, 2006, Article ID: 040501. http://dx.doi.org/10.1103/PhysRevLett.96.040501 [16] D. Markham, A. Miyake and S. Virmani, “Entanglement and Local Information Access for Graph States,” New Journal of Physics, Vol. 9, 2007, pp. 194. http://dx.doi.org/10.1088/1367-2630/9/6/194 [17] L. E. Danielsen, “Database of Self-Dual Quantum Codes.” http://www.ii.uib.no/larsed/vncorbits/ |

