 Applied Mathematics, 2013, 4, 1590-1594 Published Online November 2013 (http://www.scirp.org/journal/am) http://dx.doi.org/10.4236/am.2013.411214 Open Access AM On a Generalized Integral Operator Irina Dorca1, Daniel Breaz2, Mugur Acu3 1Department of Mathematics, University of Pitesti, Argeş, România 2Department of Mathematics, University “1st December 1918” of Alba Iulia, Alba Iulia, România 3Department of Mathematics, “Lucian Blaga” University of Sibiu, Sibiu, România Email: irina.dorca@gmail.com Received April 10, 2013; revised May 10, 2013; accepted May 17, 2013 Copyright © 2013 Irina Dorca et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT We have considered several integral operators from literature and we have made a generalization of them. It can be eas- ily seen that their properties are also preserved. Therefore, we use known results concerning the starlike functions (see [1,2]) and we unify some known integral operators (see [3]) into one single integral operator, called I1(z), in Section 3 of this paper. Keywords: Univalent Function; Integral Operator; Generalization; Particular Cases 1. Introduction Let H(U) be the set of functions which are regular in the unit disc U, :0 010fUf f and :is univalent in.Sf fU Prof. G. S. Sălăgean has introduced the subfamily T of S consisting of functions f of the form 2 ,0,2,3,, j jj j zz azajzU (1) in 1999. Thus, we have the subfamily S-T consisting of func- tions f of the form 2 ,0,2,3,, j jj j zz azajzU (2) The purpose of this paper is to give a generalization with respect to several integral operators that exist in literature. In order to do so, we have firstly considered the univalent function f and the family S of analytic and univalent functions, notions that we use further. 2. Preliminary Results Remark 2.1. In [1], we have introduced the follo wing operator concerning the functions of Form (1): 1 :; 11 j jn DA ADfz zjaz (3) Definition 2.1. [2] Let β, λ R, β ≥ 0, λ ≥ 0 and 2 j j zz az . We denote by D the linear operator defined by 01 1 ;, nn Df zfxDf zDf zzfz Df zDDf z (4) Professor Breaz ([3]) has introduced the following in- tegral operators on univalent function spaces: 1 1 1 0 d p z nn p Jf tztf ,t (5) 1 1 1 1 0 d p z p zHtftftt, (6) 1 1 0 d, p z p ft ft Ftt zt (7) 1 1 1 0 d p z p ft ft t tt Gz , (8)  I. DORCA ET AL. 1591 1 1 1 1 1 1 , 0 d p z p ft ft tt tt z (9) and 1 11 11 ,1 0 11d , zp pp Gz pgtgtt (10) where ,,, 1,,0,0 ,1 . iipp n Let be the Sălăgean differential operator, , defined as: n D :D, nn 01 1 ,, nn Df zfzDf zDf zzfz Df zDDf z (11) and ,: ,0 kk DD k of form: 0 1 2 ,, . kk k n n Df zfz Df zDDfzznaz n , F (12) The following integral operator is studied in [4], where are considered to be of Form (2): ,1,,, i fi nn Definition 2.2. [4] We define the general integral operator by ,,, : kn n I ,,, 1 ,, , kn n Iff (13) 1 11 0 d n z nn kDf tDft Fz t tt where 10 1 ,0,,, ,, , n in n n fi n , and . 0 Lemma 2.1. [5] (Schwarz Lemma) Let the function f(z) be regular in U, with f(0) = 0. If k 1 zzU , then zz for all z U, where the equality holds only if ,1fz KzK . Some integral operators and the related properties of them are also studied in [6,7]. The neighborhoods of the class of functions defined using the operator (3) is studied in [8]. Remark 2.2. In [9], we have introduced and studied the following operator of the functions f S, : :SfAfisunivalentin U 12 , ,1 1 1 22 11 1, 11 n k k k Dfz hfz kc zC kc k nkaz (14) where 1 1 1 ,; 1 k k n Cnk k n is the Pochammer symbol; k ≥ 2, c ≥ 0 and Re{c} ≥ 0; z U. In [10], Prof. G. S. Sălăgean has introduced the sub- family T of S consisting of functions f of the Form (1); see Section 1. Remark 2.3. If we denote by k the Pochammer symbol, we define it as follows: 10 12 10 k x forkx xxxxkforkandx ,0 . Following, we introduce a new general integral opera- tor in Theorem 3.1, 1 z, giving also several examples which prove its relevance. We derive it as well in order to show its applicability. Considering the univalent functions with negative co- efficients (see [11]), we develop, 2 z from Corollary 3.1. 3. Main Results Theorem 3.1. Let 12 ,,, ,Re0a and 12 12 , ,12 , , ,,,0, 1, ; ,, n j nn j Dfzj p pDfz of Form (14). If 12 12 , , , , 1 nn j nn j Dfz n Dfz and 12 12 , , , , 1,,1 nn j nn j Dfz zUjp n Dfz , , (15) 12 12 1 12 12 1 212 1 1 212 1 p jj j p jj j (16) and 12 12 1 1 2 212 1 22 , 2 p jj j na nana n (17) Open Access AM 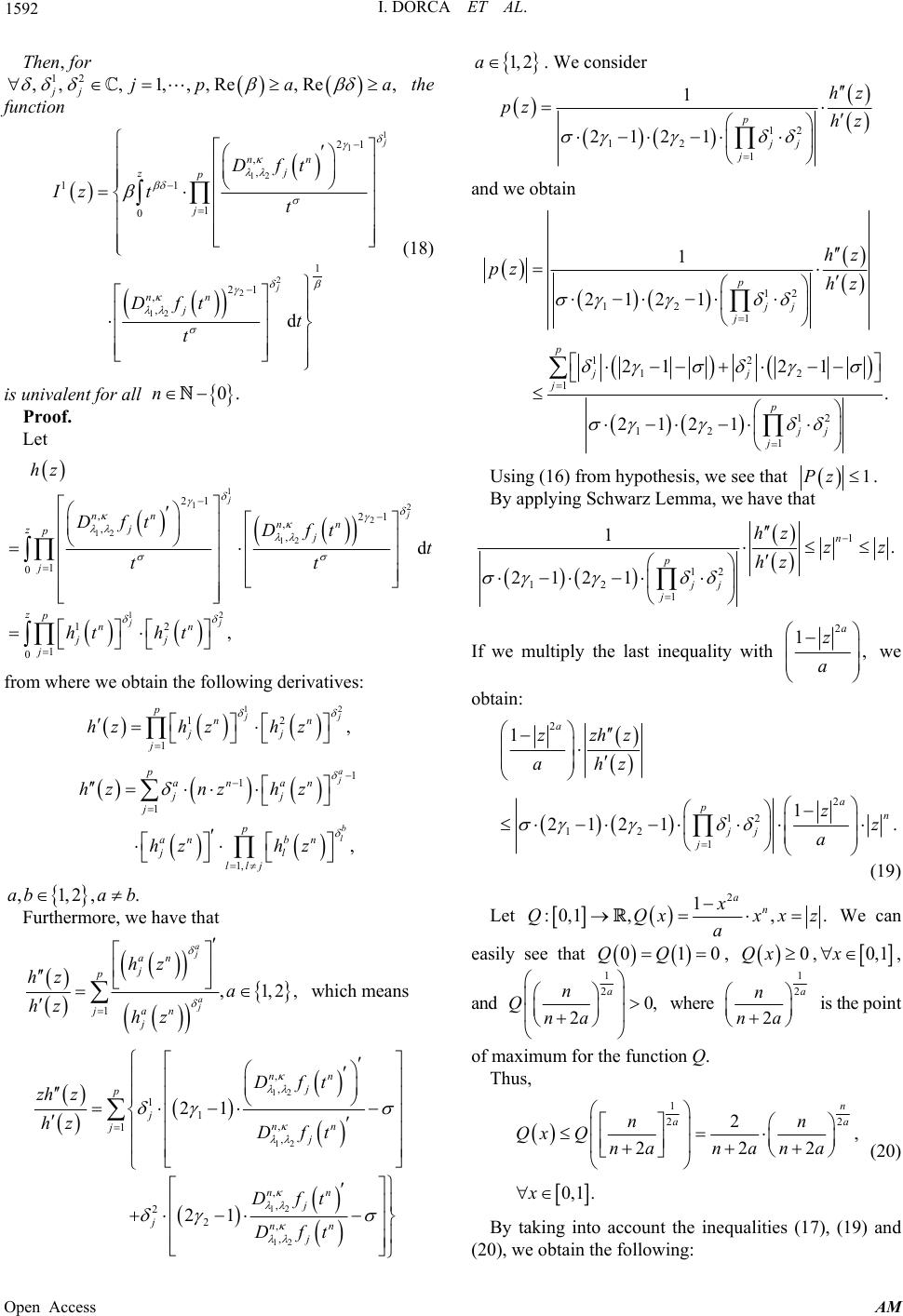 I. DORCA ET AL. 1592 Then, for 12 ,,,1,,,Re,Re, jj jp a a the function 1 1 12 2 2 12 21 , , 11 1 0 1 21 , ,d j nn j zp j nn j Dft Iz tt Dft t t (18) is univalent for all 0.n Proof. Let 1 12 2 12 12 12 21 ,21 , ,, 1 0 12 1 0 d , j j jj nn nn j zpj j zp nn jj j hz Dft Dft t tt ht ht from where we obtain the following derivatives: 12 12 1 , j p nn jj j hz hhzz 1 1 1 1, , a j b l p anan jj j p an bn jl llj hznzh z hz hz ,1,2,abab. Furthermore, we have that 1 ,1,2 a j a j an j p jan j hz hz a hz hz , which means 12 12 12 12 , , 1 1 , 1 , , , 2 2, , 21 21 nn j p j nn j j nn j jnn j Dft zh z hz Dft Dft Dft 1, 2a. We consider 12 12 1 1 212 1 p jj j hz pz hz and we obtain 12 12 1 1 12 2 1 1 1 2 1 2 1 2121 . 2121 212 1 p jj j p jj p j j j j hz pz hz Using (16) from hypothesis, we see that 1Pz . By applying Schwarz Lemma, we have that 1 12 12 1 1. 212 1 n p jj j hz zz hz If we multiply the last inequality with 2 1, a z a we obtain: 1 2 2 2 12 1 212 1 1 1. p a jj j a n zz a z a z z h hz (19) Let 2 1 :0,1 ,,. a n x QQxxx a z We can easily see that 010QQ , 0Qx , 0,1x , and 1 20, 2 a n a Qn where 1 2 2 a n na is the point of maximum for the function Q. Thus, 1 22 2, 222 0,1 . n aa nn QxQ na nana x (20) By taking into account the inequalities (17), (19) and (20), we obtain the following: Open Access AM  I. DORCA ET AL. 1593 2 11, a zz a hz hz which means that the function 1 z of Form (18) is univalent for all 0n. Remark 3.1. The mapping properties with respect to integral operator 1 z of Form (18) are studied in [7]. If we consider the operator Dz of Form (3) we obtain the following Corollary. It can be proved in a similar way as the Theorem 3.1 is. Corolla ry 3 .1. Let 12 ,,, ,Re0a 0, and , ,0, j Dfz Dfz of Form (3). If 1 and , ,1,, nn jj n nj j Df zDf z nn Df z Df z zUj p 1 12 12 1 12 12 11 212 2 1 121 p jj p j j j j and 12 12 1 2 1 22 , 1 2 221 p jj j na nana n then for all 12 ,,,1,,,Re,Re, jj jp a a the function 1 1 1 2 2 21 21 1 0 21 d j n j zp j n j Df t Iz tt Df t t t is univalent for all . 0n Remark 3.2. The Corollary is true also fo r Dz of Form (3), 1, ,.pjp N Next we give few examples of particular cases which can be found in literature. Let β = 0 in Dz of Form (3) or (4). So we have that 0,Df zfz 0 12 . We will use this form of the integral operator, where the function f is of Form (2) with respect to the operator (21). For further simplifica- tion, we consider that 1 and δ = 1 (except of Example 3.5). For the first four examples we consider 101 0,1 jn ,,jpp . Example 3.1. If σ = 1, χ = 1 and we use the notation 2,1,,jpp 0 jj we obtain the operator F(z) of Form (7). Example 3.2. If σ = 1 we obtain the operator G(z) of Form (8) for 20jpp 1, 1,, jj Example 3.3. If σ = 1 and we use the notation . 21, 1,,0 jj jpp we obtain the operator , z of Form (9). Example 3.4. If σ = 0 we obtain the operator ,p Gz of Form (10) fo r 11p and 21 1, j . For the next two examples we consider 20,1, ,0 jjpp and σ = 0. Example 3.5. a) If χ = 1, δ = 1, we obtain a particular case of the function J(z) of Form (5), in which 1, 0n . b) If 1 1,,1, 0, jj jpp we obtain the operator J(z) of Form (5). Example 3.6. If n = 1 we obtain the operator H(z) of Form (6) for 1,1,, 0 jj jpp . Remark 3.3. It can be easily proved that the examples above are also true for f(z) of Form (1). 4. Conclusions The integral operator which is introduced and studied in Theorem 3.1 of this paper, called I1(z), is a generalization of several integral operators that are taken from literature (for e.g., see [3]). Looking to the examples from above, we can easily see that our integral operator can be used further without any concern, because it preserves the ini- tial state of the already known integral operators. There- fore, the integral operator I1(z) covers the integral opera- tors from [3] without loss of generality. Open Problem 3.1. It would be interesting to prove that the integral operator (18) or (21) covers also the integral operator from [5]. 5. Acknowledgements This work was partially supported by the strategic project POSDRU 107/1.5/S/77265, inside POSDRU Romania 2007-2013 co-financed by the European Social Fund- Investing in People. Open Access AM  I. DORCA ET AL. Open Access AM 1594 REFERENCES [1] M. Acu, I. Dorca and S. Owa, “On Some Starlike Func- tions with Negative Coefficients,” Proceedings of the In- ternational Conference on Theory and Applications of Mathematics and Informatics, Alba Iulia, 21-24 July 2011, pp. 101-112. [2] M. Acu and S. Owa, “Note on a Class of Starlike Func- tions,” Proceeding of the International Short Joint Work on Study on Calculus Operators in Univalent Function Theory, Kyoto, 2006, pp. 1-10. [3] D. Breaz, “Integral Operators on Univalent Function Spaces,” Academiei Române, Bucureşti, 2004. [4] D. Breaz, H. O. Güney and G. Ş. Sălăgean, “A New Gen- eral Integral Operator,” Tamsui Oxford Journal of Mathe- matical Sciences, Vol. 25, No. 4, 2009, pp. 407-414. [5] M. Darus and R. W. Ibrahim, “Generalized Cesáro Inte- gral Operator,” International Journal of Pure and Applied Mathematics, Vol. 69, No. 4, 2011, pp. 421-427. [6] I. Rahovean (Dorca) and A. I. Rahovean, “New Integral Operators—Properties,” LAP Publishing, Saarbrücken, 2013. [7] I. Dorca, D. Breaz and M. Acu, “Mapping Properties of Some Classes of Analytic Functions under Generalized Integral Operators,” Advances in Mathematics: Scientific Journal, Vol. 1, No. 1, 2012, pp. 51-57. [8] I. Dorca, M. Acu and D. Breaz, “Note on Neighborhoods of Some Classes of Analytic Functions with Negative Coefficients,” ISRN Mathematical Analysis, 2011, Article ID: 610549. [9] I. Dorca, D. Breaz and M. Acu, “Subordonation of Cer- tain Subclass of Convex Function,” Studia Universitatis Babeş-Bolyai, Vol. 57, No. 2, 2012, pp. 181-187. [10] G. S. Sălăgean, “Geometria Planului Complex,” Prome- dia Plus, Cluj-Napoca, 1999. [11] H. Silverman, “Univalent Functions with Negative Coef- ficients,” Proceedings of the American Mathematical So- ciety, Vol. 5, 1975, pp. 109-116. http://dx.doi.org/10.1090/S0002-9939-1975-0369678-0
|