Assessment of debris flow magnitude in small catchments of the lombardy alps: the val gola case study

doi:10.4236/as.2011.21002

Paper Menu >>

Journal Menu >>

Vol.2, No.1, 9-15 (2011) Agricultural Sciences

doi:10.4236/as.2011.21002

Assessment of debris flow magnitude in small

catchments of the lombardy alps: the val gola case

study

Daniele de Wrachien1, Stefano Mambretti2*

1Department of Agricultural Hydraulics, State University of Milan, Milano, Italy;

2 DIIAR, Politecnico di Milano, Piazza Leonardo da Vinci, Milano, I taly; *Corresponding Author: stefano.mambretti@polimi.it

Received 12 December 2010; revised 30 December 2010; accepted 6 January 2011

ABSTRACT

Debris flows are among the most destructive of

all water-related disasters. They mainly affect

mountain areas in a wide range of morpho-

climatic environments. Therefore, accurate pre-

diction of their run out distances, magnitudes

and velocities plays a role of paramount impor-

tance, in order to plan and design appropriate

structural and non-structural defence measures.

In this context, a number of Authors have de-

veloped methods feasible to evaluate the ten-

dency of a catchment to generate debris flow,

without giving an estimation of the magnitude.

Other empirical procedures are based on the

analysis of historical series of debris flow, oc-

curred in similar environments, to assess the

relationship between the catchment character-

istics and the maximum movable debris vol-

umes. In this paper, and with reference to Val

Gola—a small catchment in the North-East Lom-

bardy where debris flows frequently occur—a

number of methods, belonging to each of the

above mentioned categories, have been briefly

reviewed and applied in order to evaluate their

effectiveness a nd c ons istency.

Keywords: Val Gola Catchment; Debris Flow;

Magnitude Assessment; Frequency Analysis

1. INTRODUCTION

Sediment transport in steep, small (<10 km2) catch-

ments of the Alps is often characterised by both newto-

nian (flood waves of clear water) and non newtonian

(debris and mud flows) behaviour. The availability of

long-term series of data (e.g. volumes, magnitude, ve-

locity and frequency) is crucial in order to provide sta-

tistically significant analyses and predictions, thus

making experimental measuring stations highly valu-

able for the scientific community as well as for the lo-

cal agencies dealing with structural protection meas-

ures and land use planning.

In Italy, like the other European countries, there is a

lack of such experimental data, with a few exceptions

[1-3].

In this context, a number of authors developed me-

thods feasible to evaluate the tendency of a catchment

to generate debris flow, without giving an estimation of

the magnitude [4,5]. Other empirical (statistical and

semi-quantitative) procedures are based on the analysis

of historical series of debris flow, occurred in similar

environments, to assess the relationship between the

catchment characteristics and the maximum movable

debris volume.

In this paper, and with reference to Val Gola—a

small catchment in the North-East Lombardy where

debris flow frequently occur—a number of procedures,

belonging to each of the above mentioned categories,

have been briefly reviewed and applied in order to

evaluate their effectiveness and consistency.

2. EMPIRICAL RELATIONSHIPS

At present, there are no rigorous methods feasible to

assess the exact probability of debris flow occurrence.

Semi-quantitative methods which allow to estimate the

likelihood of debris flow occurrence in a particular tor-

rent basin have been proposed by different authors [6].

As an alternative, numerical simulation models can be

used to assess the flow properties and the deposition

process [7].

2.1. Debris Flow Characteristics

From the point of view of the evaluation of a potential

hazard, the volume

and the peak discharge

Q of

a debris flow represent the most important parameters.

In general, a spectrum of possible debris flow volumes

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

and peak discharges can be expected to occur with dif-

ferent probabilities.

a) Many attempts have been made to estimate a

maximum debris flow volume for a given torrent catch-

ment. These empirical equations are usually based on the

most important morphometric characteristics of a catch-

ment [8,9]. It was found that these equations may over-

estimate the actual debris flow volume by up to a factor

of 100 [2]. To overcome these uncertainties, D’Agostino

[10-12] introduced a Geologic Index, which takes into

account the different lithologic units of the catchment.

Others followed the same path [13]. On the other hand,

simplest method relies on fewer parameters, as the area

[14].

b) Knowledge of the peak discharge and the associ-

ated flow velocity play a role of paramount importance

when evaluating the conveyance capacity of a stream

channel reaches or critical cross sections, as, for example,

under bridges. It has been shown that empirical rela-

tionships can be established between the peak discharge,

Qp, of a debris flow and the debris flow volume [15].

c) Other parameters like mean flow velocity, flow

cross-section, travel distance and runout distance on fan

play important roles in the assessment of a debris flow

potential hazard [2].

Further characteristics, observed in the field, may also

be used to estimate the probability of debris flow occu r-

rence and feature the triggering, mobilisation and stop-

ping processes [4 ,5 ,1 6,17].

2.2. Probabilistic Analysis of Historical Data

Most empirical and statistical procedures for the esti-

mation of debris flow magnitude compute “maximum”

or “extreme” possible volumes. Less information is usu-

ally available about magnitude-frequency relations,

which can usefully contribute to the definition of de-

fence measures [18].

On the whole, empirical and probabilistic predictive

relationships provide an approximate assessment of de-

bris flow characteristics and their use should be re-

stricted to the environmental context (geological, geo-

morphological, climatic) where they have been devel-

oped. It has been pointed out [19] that these procedures

produce very different results where employed in other

geographical areas, without a previous check of their

applicability in a given region.

3. THE VAL GOLA CATCHMENT

3.1. Geological and Geographical

Framework

The Val Gola catchment (3.5 km2), located in the

eastern Italian Alps (Val Camonica, Lombardy Region),

belongs to the Olio river basin. The catchment shows an

elliptical form, with the main axes oriented in NW-SE

direction (Figure 1).

Geologically, the catchment, of great importance from

both economic and turistic points of view, is character-

ised by different lithologies, essentially represented by

Figure 1. Location map of the study area.

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

evaporite and carbonate sedimentary units (Angolo,

Camorelli, and Esimo limestones) chronologically dated

between low and medium Triassic. Quaternary fans are,

mostly, present in the lower part of th e valley. According

to their genesis, these deposits can be classified as

gravitative fans or screes, deposits due to the fluvial

processes and glacial deposits (moraines). Upper Qua-

ternary fans, outcropping in different areas and having

different stratigraphical positions are present, mainly, in

the southern part of the catchment (Fermata Castello).

3.2. Field Surveys

Field surveys have been carried out to directly collect

data and to verify those indirectly collected by air-photo

interpretation and historical sources (flood frequencies,

date and magnitude of past events, damages, landsliding

and areal extension of flooded areas). Based on these

observations an estimatio n of the main debris flow char-

acteristics (magnitude, mixture and water discharges etc.)

has been carrie d out .

From the morphology of the fans, the knowledge of the

occurred past events, the characteristics of the drainage

basin and the prevalent transport mechanism associated

with individual fans were determined. The main outcomes

of these investigations are summarized in the following

chapters.

4. DATA ANALYSIS

The analysis of morphometric and hydrologic pa-

rameters used to describe the catchment characteristics

and behaviour was based on the most frequently adopted

approaches for the assessment of the type and magnitude

of dominant alluvial fan activity.

The main aims of the analysis performed in this study

can be summarized as follow:

 to put in evidence any significant statistical rela-

tionship among the parameters;

 to analyse the relationships between the group of

morphometric parameters and the type of activity;

 to analyse the relationships between the mor-

phometric parameters and the feasible maximum inten-

sity of the different events.

4.1. Empirical Methods

Figure 2 shows the catchment’s tendency to debris

flow generation according to Melton and Aulitzky’s in-

dexes, modified by Ceriani et al. [20]. The Aulitzky’s

index was evaluated equal to 15, featuring the bent of

the basin for debris flow triggering as medium-high.

Table 1 summarizes the results of the first nine em-

pirical relationships. It is quite evident that this formulae

give only approximate estimations of the possible maxi-

mum intensity of debris flow events. Such relationships

0.00

0.05

0.10

0.15

0.20

0.25

0.30

0.35

00.1 0.2 0.3 0.4 0.5 0.6 0.70.8 0.91

Melton Fan Index

Slope Fan

Bed load fan

Debris flood fan

Debris flow fan

Figure 2. Catchment’s tendency to the debris flow genera-

tion according to Melton index.

Table 1. Comparison of debris flow magnitude assessed by

empirical relationships based on morphometric parameters

Applied method Magnitude [m3]

Marchi and Tecca [9] 20440

Bottino, Crivellari and Mandrone [14] 28674

Rickenmann [2] 150000

Kronfellner—Kraus [16] 186150

Hampel [8] 296150

M1 = 51257

D'Agostino et al. [10,11] M2 = 58250

D'Agostino et al. [12] 64482

Bianco and Franzi [13] 37547

h = 1 m 42395

h = 1.5 m 63592

Tropeano and Turconi [17]

h = 2 m 84790

can probably be improved if factors controlling sediment

supply are also taken into account.

At present it appears that a geomorphologic assess-

ment in the field of material likely to be mobilized may

be the best approach to arrive at a more precise estimate

of a possible debris flo w v ol u m e .

It seems, therefore, necessary to test the reliability of

these relationships by means of physically based meth-

ods.

Beside the above mentioned empirical formulae, the

following relationship can be used, suitable to assess the

rate between the debris flow T

Q and water W

Q dis-

charges of the catchment [15]:





*b b*

QcS Scc





 

(1)

where *

c is the packing concentration of the solid

phase (usually equal to 0.65), c is the debris flow con-

centration and b

S the degree of saturation of the river

bed before the debris flow passage. Assuming b

S = 1

[21] the expected debris flow discharge is equal to 2-3

times the liquid discharge. The trend of the function (1)

is shown in Figure 3.

The simplicity of this procedure does not prevent the

assessment of the debris flow magnitude as function of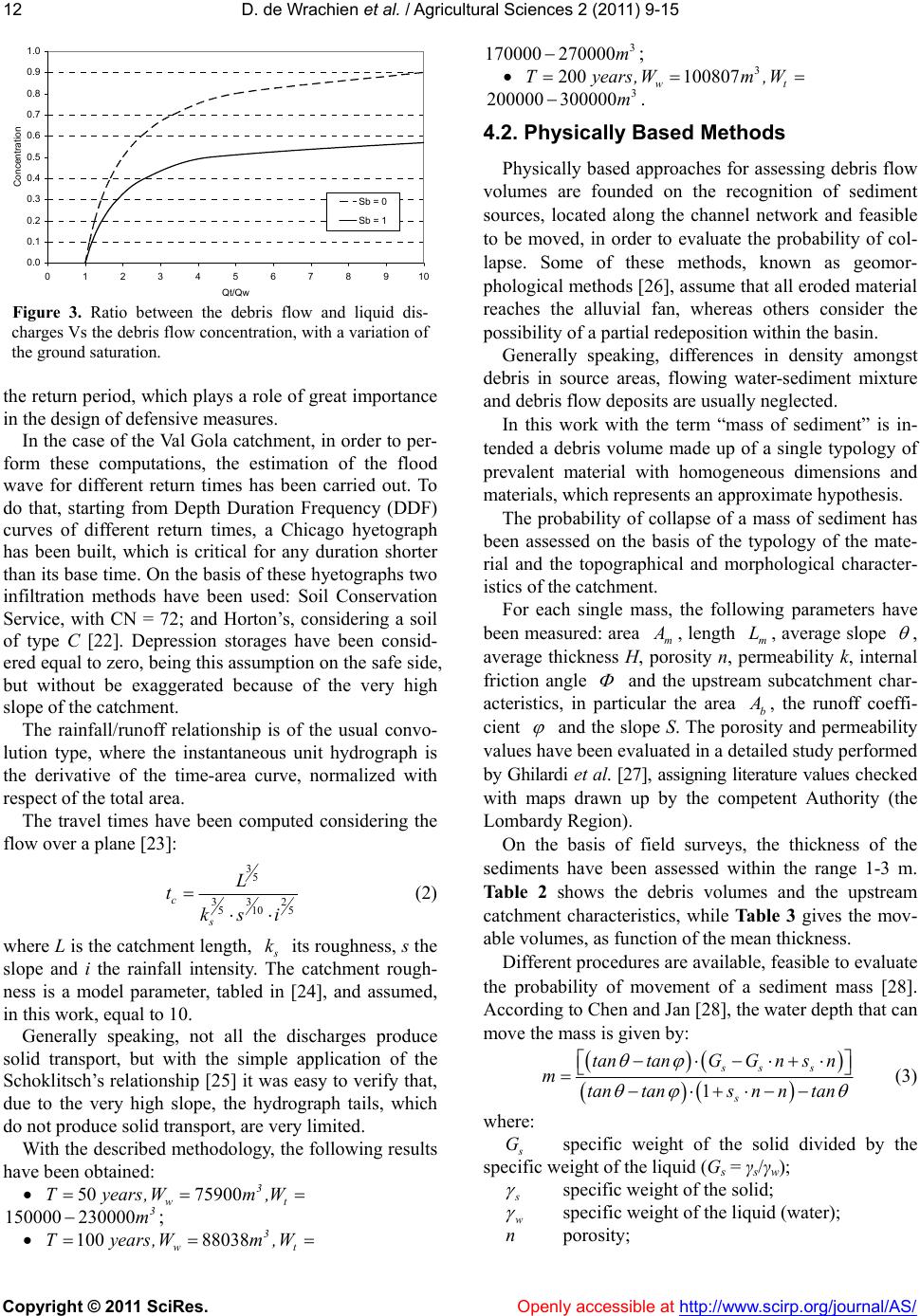

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

0.0

0.1

0.2

0.3

0.4

0.5

0.6

0.7

0.8

0.9

1.0

012345678910

Qt/Qw

Concentration

Sb = 0

Sb = 1

Figure 3. Ratio between the debris flow and liquid dis-

charges Vs the debris flow concentration, with a variation of

the ground saturation.

the return period, which plays a role of great importance

in the design of defensive measures.

In the case of the Val Gola catchment, in order to per-

form these computations, the estimation of the flood

wave for different return times has been carried out. To

do that, starting from Depth Duration Frequency (DDF)

curves of different return times, a Chicago hyetograph

has been built, which is critical for any duration shorter

than its base time. On the basis of these hyetographs two

infiltration methods have been used: Soil Conservation

Service, with CN = 72; and Horton’s, considering a soil

of type C [22]. Depression storages have been consid-

ered equal to zero, being this assumption on the safe side,

but without be exaggerated because of the very high

slope of the catchment.

The rainfall/runoff relationship is of the usual convo-

lution type, where the instantaneous unit hydrograph is

the derivative of the time-area curve, normalized with

respect of the total area.

The travel times have been computed considering the

flow over a plane [23]:

332

5105

ksi





 (2)

where L is the catchment length,

k its roughness, s the

slope and i the rainfall intensity. The catchment rough-

ness is a model parameter, tabled in [24], and assumed,

in this work, equa l to 10.

Generally speaking, not all the discharges produce

solid transport, but with the simple application of the

Schoklitsch’s relationship [25] it was easy to verify that,

due to the very high slope, the hydrograph tails, which

do not produce solid transport, are very limited.

With the described methodology, the following results

have been obtained:

 5075900 3

T years,Wm,W

150000230000 3

m;

 10088038 3

T years,Wm,W

170000 270000m;

 3

200 100807

T years,Wm,W





200000 300000m.

4.2. Physically Based Methods

Physically based approaches for assessing debris flow

volumes are founded on the recognition of sediment

sources, located along the channel network and feasible

to be moved, in order to evaluate the probability of col-

lapse. Some of these methods, known as geomor-

phological methods [26], assume that all eroded material

reaches the alluvial fan, whereas others consider the

possibility of a partial redeposition within the basin.

Generally speaking, differences in density amongst

debris in source areas, flowing water-sediment mixture

and debris flow deposits are usually neglected.

In this work with the term “mass of sediment” is in-

tended a debris volume made up of a single typology of

prevalent material with homogeneous dimensions and

materials, which represents an approximate hypothesis.

The probability of collapse of a mass of sediment has

been assessed on the basis of the typology of the mate-

rial and the topographical and morphological character-

istics of the catchment.

For each single mass, the following parameters have

been measured: area m

, length m

L, average slope



average thickness H, porosity n, permeability k, internal

friction angle



and the upstream subcatchment char-

acteristics, in particular the area b

, the runoff coeffi-

cient



and the slope S. The porosity and permeability

values have been evaluated in a detailed study performed

by Ghilardi et al. [27], assigning literature values checked

with maps drawn up by the competent Authority (the

Lombardy Region).

On the basis of field surveys, the thickness of the

sediments have been assessed within the range 1-3 m.

Table 2 shows the debris volumes and the upstream

catchment characteristics, while Ta b l e 3 gives the mov-

able volumes, as function of the mean thickness.

Different p roc edur es a re av ailab le , fe asib le to ev alu ate

the probability of movement of a sediment mass [28].

According to Chen and Jan [28], the water depth that can

move the mass is given by:







ss s

tantanGG nsn

mtantans n ntan

















 (3)

where:

G specific weight of the solid divided by the

specific weight of the liquid (Gs = γs/γw);



specific weight of the solid;



specific weight of the liquid (water);

n porosity;

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

Table 2. Debris volumes and upstream catchment characteristics.

AM1 AM2 AM3

Area Am [km2] 0.266 0.237 0.132

Maximum elevation Hmax [m asl] 1400 1300 1150

Minimum elevation Hmin [m asl] 900 890 944.74

Length Lm [km] 0.861 0.666 0.519

Mean slope θ [° ] 30.14 31.4 21.55

Mean thickness H [m] 1-3

Porosity n [-] 0.5

Permeability k [m/s] 0.1

MASS CHARACTERIS-

TICS

Int. friction angle Φ [°] 33

Area Ab [km2] 0.158 0.113 0.976

Maximum elevation hmax [m asl] 1600 1400 1718.32

Minimum elevation hmin [m asl] 1400 1300 1150

Length Lb [km] 0.252 0.156 0.903

Mean slope S [°] 38.31 32.62 32.61

UPSTREAM CATCHMENT

CHARACTERISTICS

Runoff coefficient φ [-] 0.7 0.7 0.7

Table 3. Debris movable volumes vs mean thickness.

Potentially movable volume [m3]

H = 1.0 m H = 1.5 m H = 2.0 m H = 2.5 m H = 3.0 m

AM1 266000 399000 532000 665000 798000

AM2 237000 355500 474000 592500 711000

AM3 132000 198000 264000 330000 396000

saturation of the solid;



slope;



stratification angle of the solid.

Known m and H, the critical water depth h to generate

a slide is given by:

hmH (4)

while the discharge Q through a vertical section of a

sediment mass with height H can be assessed as follows:

Qkhnl (5)

where m

l is the mean width of the debris mass, defined

as the ratio between its area and length along the direc-

tion of the maximum slope:

 (6)

The critical intensity of the rainfall to generate Q is

given by:

028b

i,A



 (7)

with b

the area of the subcatchment upstream the

debris mass.

For the Val Gola catchment 07.



 has been se-

lected on the basis of accurate hydrologic investigations

[27].

On the basis of these parameters, further characteris-

tics have been evaluated. Ta bl e 4 gives the return peri-

ods needed to move masses of debris flows of different

thickness.

For masses so large, the hypothesis that they can col-

lapse entirely at the same time cannot be accepted. So,

these debris masses have been divided in “sub-masses”,

generated by different subcatchments.

Table 5 gives the return periods of the rainfalls that

triggered the collapse of the debris flow sub-masses.

To achieve a more reliable assessment of the catch-

ment instability, the well-known Shalstab code [29] was

applied. To this end, the basin area has been divided with

DEM cells of 20 m × 20 m (Figure 4). The Figure 4

shows that about 65% of the area results to be uncondi-

tionally unstable (indep endently of the rainfall intensity)

and for about 30% the stability depends on the rate be-

tween the rainfall intensity and the soil p ermeability. The

remaining 5% of the catchment results unconditionally

stable.

5. CONCLUDING REMARKS

With respect to the hazard assessment of a small tor-

rent catchment of the Alps (Val Gola) different empirical

methods have been applied with the aim of determining

whether debris flow are likely to occur or not.

Each of the considered methods shows both advan-

tages and shortcomings. The wise application and a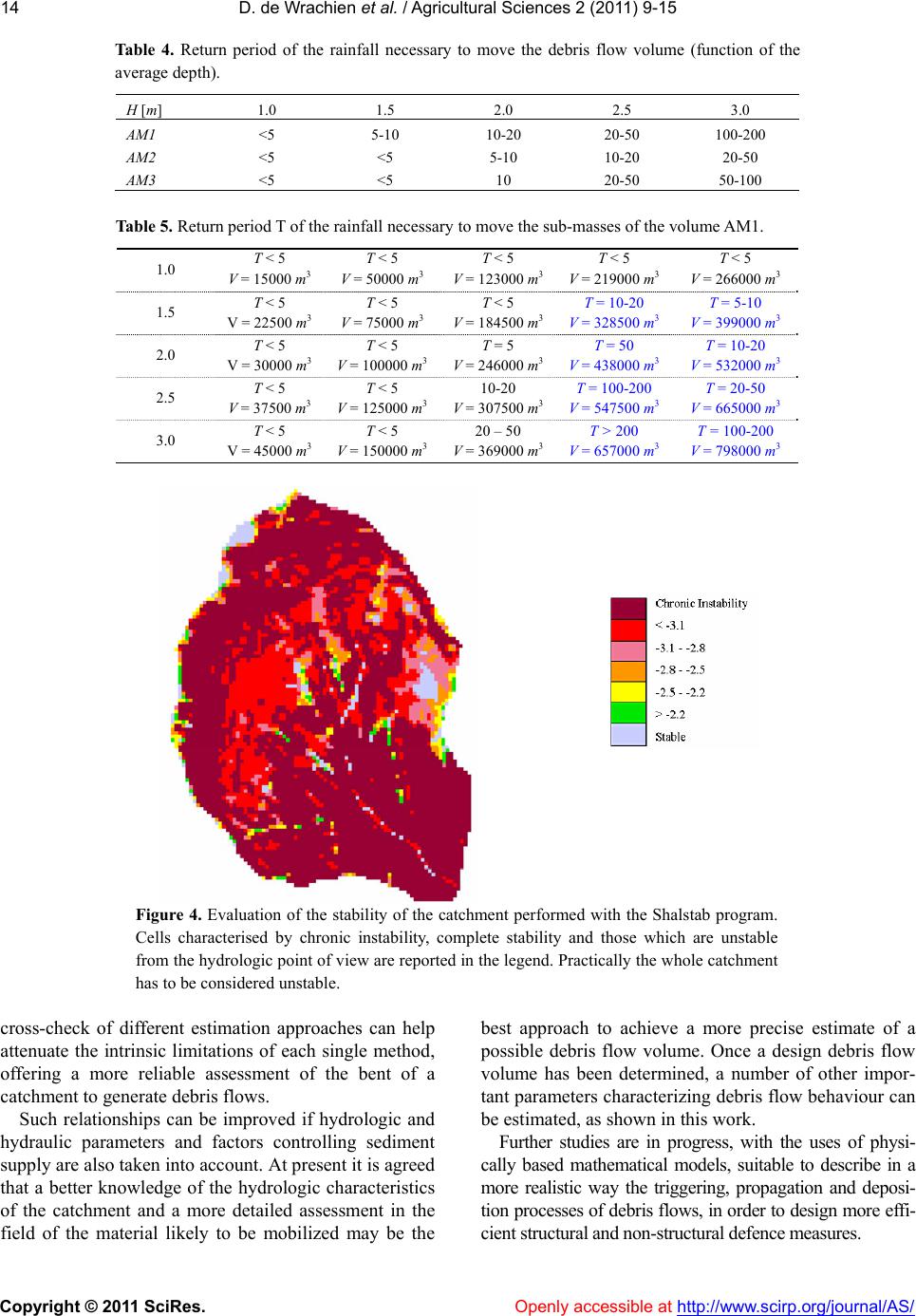

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

Table 4. Return period of the rainfall necessary to move the debris flow volume (function of the

average depth).

H [m] 1.0 1.5 2.0 2.5 3.0

AM1 <5 5-10 10-20 20-50 100-200

AM2 <5 <5 5-10 10-20 20-50

AM3 <5 <5 10 20-50 50-100

Table 5. Return period T of the rainfall necessary to move the sub-masses of the volume AM1.

1.0 T < 5

V = 15000 m3

T < 5

V = 50000 m3

T < 5

V = 123000 m3

T < 5

V = 219000 m3

T < 5

V = 266000 m3

1.5 T < 5

V = 22500 m3

T < 5

V = 75000 m3

T < 5

V = 184500 m3

T = 10-20

V = 328500 m3

T = 5-10

V = 399000 m3

2.0 T < 5

V = 30000 m3

T < 5

V = 100000 m3

T = 5

V = 246000 m3

T = 50

V = 438000 m3

T = 10-20

V = 532000 m3

2.5 T < 5

V = 37500 m3

T < 5

V = 125000 m310-20

V = 307500 m3

T = 100-200

V = 547500 m3

T = 20-50

V = 665000 m3

3.0 T < 5

V = 45000 m3

T < 5

V = 150000 m320 – 50

V = 369000 m3

T > 200

V = 657000 m3

T = 100-200

V = 798000 m3

Figure 4. Evaluation of the stability of the catchment performed with the Shalstab program.

Cells characterised by chronic instability, complete stability and those which are unstable

from the hydrologic point of view are reported in the legend. Practically the whole catchment

has to be considered unstable.

cross-check of different estimation approaches can help

attenuate the intrinsic limitations of each single method,

offering a more reliable assessment of the bent of a

catchment to generate debris flows.

Such relationships can be improved if hydrologic and

hydraulic parameters and factors controlling sediment

supply are also taken into accoun t. At present it is agreed

that a better knowledge of the hydrologic characteristics

of the catchment and a more detailed assessment in the

field of the material likely to be mobilized may be the

best approach to achieve a more precise estimate of a

possible debris flow volume. Once a design debris flow

volume has been determined, a number of other impor-

tant parameters characterizing debris flow behaviour can

be estimated, as shown in this work.

Further studies are in progress, with the uses of physi-

cally based mathematical models, suitable to describe in a

more realistic way the triggering, propagation and deposi-

tion processes of debris flows, in order to design more effi-

cient structural and non-structural defence measures.

D. de Wrachien et al. / Agricultural Sci ences 2 (2011) 9-15

REFERENCES

[1] Zimmerman, M., Mani, P. and Romang, H. (1997)

Magnitude-frequency aspects of alpine debris flow.

Eclogae Geologicae Helvetiae, 90, 415-420.

[2] Rickenmann, D. (1999) Empirical relationships for

debris flows. Natural Hazards, 19, 47-77.

doi:10.1023/A:1008064220727

[3] Marchi, L., Arattano, M. and Deganutti, A.M. (2002)

Ten years of debris-flow monitoring in the Moscardo

Torrent (Italian Alps). Geomorphology, 46, 1-17.

doi:10.1016/S0169-555X(01)00162-3

[4] Melton, M.A. (1965) The geomorphic and paleoclimatic

significance of alluvial deposits in Southern Arizona.

Journal of Geology, 73, 1-38. doi:10.1086/627044

[5] Aulitzky, H. (1980) Preliminary two—fold classification

of torrents. Proceedings of International Symposium,

Austria, 285-310.

[6] Nakamura, J. (1980) Investigation manual on prediction

of occurence od Dosekiryu [debris flow] delineation of

sangerous zone affected by Dosekiryu and arrangement

of warning and evacuation system in mountain torrents in

Japan. Proceedings of International Symposium, Austria,

41-81.

[7] Hungr, O. (1995) A model for the runout analysis of

rapid flow slides. Canadian Geotechnical Journal, 32,

610-623. doi:10.1139/t95-063

[8] Hampel, R. (1977) Geschiebewirtschaft in Wildbächen

[sediment budget of torrents]. Wildbach-und Lawinen-

verbau, 41, 3-34 (in German).

[9] Marchi, L. and Tecca, P.R. (1996) Magnitudo delle

colate detritiche nelle Alpi Orientali. Italiane Geoin-

gegneria Ambientale e Mineraria, 33, 79-86. (in Italian).

[10] D’Agostino, V. (1996) Analisi quantitativa del trasporto

solido torrentizio nei bacini montani del Trentino

Orientale in “Scritti dedicati a Giovanni Tournon”.

Associazione italiana di Ingegneria agraria—Associa-

zione Idrotecnica Italiana, Novara (Italy), 111-123 (in

Italian).

[11] D’Agostino, V., Cerato, M. and Coali, R. (1996) Extreme

events of sediment transport in the eastern Trentino

torrents. Atti del Convegno Interpraevent, 1, 377-386.

[12] D’Agostino, V. and Marchi, L. (2004) Estimation of

debris-flow magnitude in the Eastern Italian Alps. Earth

Surface Processes and Landforms, 29, 207-220.

doi:10.1002/esp.1027

[13] Bianco, G. and Franzi, L. (2000) Estimation of debris

flow volumes from storm events. Proceedings of Debris

Flow Mitigation: Mechanics, Prediction and Assessment,

Taipei, Taiwan, 441-448.

[14] Bottino, G., Crivellari, R. and Mandrone, G. (1996)

Eventi pluviometrici critici e dissesti: Individuazione

delle soglie d’innesco di colate detritiche nell’anfiteatro

morenico di Ivrea. Proceedings of La prevenzione delle

catastrofi idrogeologiche: il contributo alla ricerca scien-

tifica, Alba (Torino), 201-210.

[15] Takahashi, T., Sawada, T., Suwa, H., Mizuyama, T.,

Mizuhara, K., Wu, J., Tang, B., Kang, Z., Zhou, B. (1994)

Japan-China joint research on the prevention from debris

flow hazards. Research Report, Japanese Ministry of

Education, Science and Culture, International Scientific

Research Program, No. 03044085.

[16] Kronfellner-Kraus, G. (1984) Extreme feststofffrachten

und grabenbildungen von wildbächen [extreme sediment

loads and erosion of torrents]. Proceedings of

International Symposium Interpraevent, Villach, Austria,

2, 109-118 (in German).

[17] Tropeano, D. and Turconi, L. (1999) Valutazione del

potenzial e d e tr iti co in p icc oli b a cini delle Alpi Occidentali e

Centrali. CNR-IRPI/GNDCI, Pubbl. n. 2058 Linea 1,

151.

[18] Johnson, P.A., McCuen, R.H. and Hromadka, T.V. (1990)

Magnitude and frequency of debris flow. Journal of

Hydrology, 123, 69-82.

doi:10.1016/0022-1694(91)90069-T

[19] Meunier, M., Rickenmann, D. and Rahuel, J.L. (2000)

Workshop 3—torrential hazard—general in “risques

naturel en montagne”. In: Gillet, F. and Zanolini, F., Eds.,

Cemagref Editions, France, 329-333.

[20] Ceriani, M., Fossati, D. and Quattrini, S. (1998)

Valutazione della pericolosità idrogeologica sulle conoidi

alpine; esempio della metodologia di Aulitzky applicata

alla conoide del torrente Re di Gianico-Valcamonica (Bs)

—Alpi Centrali. Atti del XXVI Convegno di Idraulica e

Costruzioni Idrauliche, Catania (in Italian).

[21] Armanini, A. (1996) Colate di detrito. Rapporti di lavoro

dell’Istituto Geologico della Repubblica Italiana e del

Cantone del Ticino (in Italian).

[22] Soil Conservation Service Hydrology National Eng i n ee r in g

Handbook (1956) Section 4, Washington D.C., U.S.A.

[23] Wooding, R.A. (1965) A hydraulic model for the

catchment stream problem. Kinematic wave theory.

Journal of Hydrology, 3, 254-267.

doi:10.1016/0022-1694(65)90084-3

[24] Mignosa, P. (1989) Sui problemi di dimensionamento

delle reti di drenaggio urbano. Dissertazione per il

conseguimento del titolo di Dottore di Ricerca, Istituto di

Idraulica, Politecnico di Milano (in Italian).

[25] Schoklitsch, A. (1962) Handbuch des Wasserbaues,

Springer, Wien (in German).

[26] Marchi, L. and D’Agostino, V. (2004) Estimation of

debris flow magnitude in the eastern Italian Alps. Earth

Surface Processes and Landforms, 29, 207-220.

doi:10.1002/esp.1027

[27] Ghilardi, F., Ghilardi, S. and Mambretti, S. (2006) Studio

di dettaglio della conoide della Val Gola in comune di

Costa Volpino Relazione Tecnica (in Italian).

[28] Chen, J.C. and Jan, C.D. (2000) Debris flow occurrence

probability on hillslopes. Proceedings of Debris Flow

Mitigation: Mechanics, Prediction and Assessment, Balkema,

Rotterdam.

[29] Montgomery, D.R and Dietrich, W.E. (1994) A physically

based model for the topographic control on shallow

landsliding. Water Resources Research, 30, 1153-1171.

doi:10.1029/93WR02979