Engineering, 2013, 5, 30-36
http://dx.doi.org/10.4236/eng.2013.59B006 Published Online September 2013 (http://www.scirp.org/journal/eng)
The Reliability Evaluation Method Study of Power System
Communication Networks in Case of Ice Storm
Jianghua Yang1,2, Huan Teng1,2, Chonggu Yao1,2, Nian Liu1, Bin Sun3,
Hanyun Yuan3, Ming Liu3, Jialin Bai3
1School of Electrical Engineering & Information, Sichuan University, Chengdu, China
2Sichuan Smart Grid Key Laboratory, Chengdu, China
3Guizhou Electric Power Grid Dispatching and Control Center, Guiyang, China
Received July 2013
ABSTRACT
This paper is divided into two cases to study the communication trans mission equipment reliability in the state of the ice
storm, according to the huge losses of power system communication caused by the ice storm. For the nodes or links
which are not affected by the ice storm, we use the calculation w ith “the mean time between failures (MTBF)” and “the
mean time to repair” (MTTR) to put forward the calculation methods; for the OPGW cable which influenced greater in
ice storm, we use the fiber excess length and the elongation of fiber optic cable. It obtains all the paths of the network
through improved adjacency matrix method, and then it uses binary decision diagram to obtain the overall reliability of
the network. By testing the network nodes and links using “N-1” inspection, the key nodes and key links can be ob-
tained. Finally, considering the importance degree of network transmission business, the reliability evaluation method
of power system communication network based on the risk theory in the case of the ice storm has been put forward, and
the example to verify that the method can provide the basis for the reliability assessment of the power system commu-
nication in the case of the ice storm has been given.
Keywords: Ice Storm; Pow er Communication; Reliability; Risk Theory; A djacency Matrix Method; Binary Decision
Diagram
1. Introduction
Power communication network serves grid automation
control commercial operation and modern management
[1], which is the basement of the power system security
and stability control system, therefore, the safety and
reliability of the electric power communic ations network
impact seriously on the security of the power system
production [2,3]. Ice storm causing huge damage to the
electric power communication network in recent years
that affected the delivery of the electricity business se-
riously [4-6] arouses the scholars to research in-depth
and proposes new requirements on the reliability of the
power system communication network. Therefore, the
study of the reliability of the power communication net-
work is necessary.
The reliability of electric power communication re-
search has two problems: 1) There are some similarities
between electric power communication network and
public network, and many studies directly draw on the
public network reliability results did not fully consider
the special needs of the reliability of electric power
communication network; 2) Existing research depth is
not enough, with few high level research achievements.
This article draws on some of the results of the public
network combined with the special needs of the electric
power communication network, and puts forward the
suitable reliability evaluation method of the power com-
munication network in case of the ice storm.
2. Power Communication Reliability
Overview
Power communication network is a significant part of the
secondary system of the gr id, and serves for the commu-
nication network of the power system specialized, pro-
viding indispensable services for power scheduling, pro-
duction, operation and management. Currently, power
system communication transmission network is mainly
constituted by the power of optical fiber communication
network [7], using synchronization sequence (Synchron-
ous Digital Hierarchy, SDH) transmission network as the
core of the electric power optical fiber communication
network, which is the example of this article to assess the
reliability of the electric power communication network.
The reliability of the communication network is not yet

J. H. YANG ET AL.
31
clearly defined, but the contents of the various definitions
are nearly the same that is the ability to complete the
required communication functions of the communication
network in a specific environment and within the speci-
fied time under the damaging effects of man-made or
natural [7,8]. The reliability study of the power commu-
nication network can be classified as graph theory basic,
probability theory basic, and the model basic methods [2];
according to the measurement, it can be divided into the
study of survivability [4], survival [9] and availability [7].
The essay [10] analyzed that the availability is based on
the survivability, considerin g the business performance
of the communication network, which can better describe
the basic functions of the communicati on network, and for
this reason that this article will utilize the availability
measure based reliability assessment.
Power communication network can be seen as a col-
lection of nodes and links, each path a collection of
nodes and links as well, whose reliability di rectly affects
the reliability of the pa th and further aff e cts the re liability
of the communication network. Therefore, assessing the
communication network reliability in the ice storm cir-
cumstances of nodes and links in the actual operation of
the electricity production is important.
3. Reliability in the Ice Storm
By analyzing all communication failure in Guizhou
Province in 2011, the main fault occurs in the case of the
ice storm is cable fault. The impact of ice cover is not the
same with the different types of fiber optic cable, in
which fiber composite overhead ground (OPGW) af-
fected by ice cover greater due to its internal structure is
fiber external and overhea d ground wi re on t he outside [11].
Consequently, we can simply study OPGW cable in the
case of ice cover and calculate the reliability using fiber
length and fiber optic cable elongation, while other types
of fiber optic cable have no effect under ice cover, whose
reliability can be calculate d using the mean time to repair
(MTTR) and mean time between failures (MTBF) [10,12].
3.1. The Reliability of Node & Non-OPGW
Cable
MTBF (Mean Time Between Failure, MTBF) is defined
as the time average of faulty work from the beginning to
the equipment in the specified operating environment
conditions. For a system with constant failure rate λ, the
relationship between MTBF and λ is:
1
=MTBF
λ
(1)
Detecting a transmission unit within a predetermined
time period T, to statistics the number of fault occur d, then:
=T MTBF d
(2)
MTTR (Mean Time to Repair, MTTR) is defined as
the expected value of the recovery time of a random va-
riable, including the time required for confirmation fail-
ure occurs and maintenance. When the repair rate con-
stant is μ, the relationship between MTTR and μ can be
expressed as:
1
=MTTR
µ
(3)
Detecting a transmission unit within a predetermined
time period T, to statistics the number of fault occur d,
recording each fault repair time as
1
t
,
2
t
,…
d
t
respec-
tively, then:
1
d
i
i
t
MTTR d
=
=
∑
(4)
The communication network is repairable system that
in the work—failure—repair repeat process. In this con-
dition, available is defined as the probability of the sys-
tem or apparatus in the active state in a given random
time, thus, the steady-state expression is:
MTBF
AMTBF MTTR
µ
λµ
= =
++
(5)
3.2. The Reliability of OPGW Fiber Optic Cable
The OPGW cable suspension model diagram is shown in
Figure 1:
The catenary equation of Figure 1 is [13]:
1
x
y ch αα

= −


(6)
In this equation , σ is the horizontal stresses and α is th e
introduction of the parameters which is the ratio of the
cable horizontal tension and the unit weight. Parameter σ
changes, and α will change as well.
Integrating the curve of Equitation (6) will obtain the
length of the suspension cable:
=( )
BA
ll
L shsh ααα +
(7)
y
x
A
B
h
( )
,
AA
ly−
( )
,
BB
ly
B
l
A
l−
l
σ
0
Figure 1. The model of the suspension cable.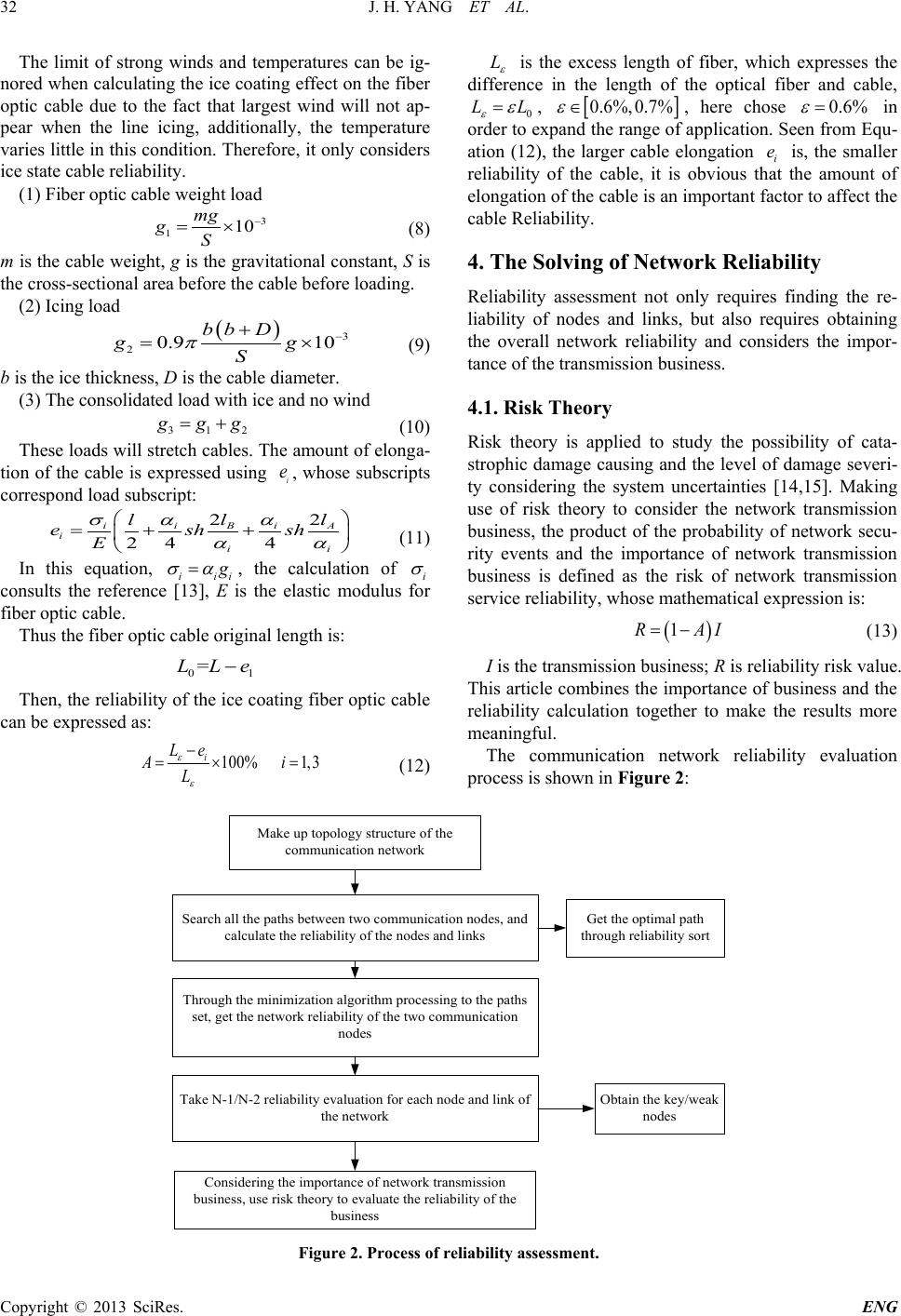

J. H. YANG ET AL.
32
The limit of strong winds and temperatures can be ig-
nored when calculating the ice coating effect on the fiber
optic cable due to the fact that largest wind will not ap-
pear when the line icing, additionally, the temperature
varies little in this condition. Therefore, it only considers
ice state cable reliability.
(1) Fiber optic cable weight load
3
110
mg
gS
−
= ×
(8)
m is the cable weight, g is the gravitational constant, S is
the cross-sectional area before the cable before loading.
(2) Icing loa d
( )
3
2
0.9 10
bb D
gg
S
π
−
+
= ×
(9)
b is the ice thickness, D is the cable diameter.
(3) The consolidated load with ice and no wind
312
g gg= +
(10)
These loads will stretch cables. The amount of elonga-
tion of the cable is expressed using
i
e
, whose subscripts
correspond load subscript:
22
24 4
ii i BA
i
ii
ll l
esh sh
E
σα α
αα

=++


(11)
In this equation,
i ii
g σα =
, the calculation of
i
σ
consults the reference [13], E is the elastic modulus for
fiber optic cable.
Thus the fiber optic cable original length is:
01
=LLe−
Then, the reliability of the ice coating fiber optic cable
can be expressed as:
100% 1,3
i
Le
Ai
L
ε
ε
−
=×=
(12)
L
ε
is the excess length of fiber, which expresses the
difference in the length of the optical fiber and cable,
0
LL
εε
=
,
[ ]
0.6%,0.7%
ε
∈
, here chose
0.6% ε =
in
order to expand the range of application. Seen from Equ-
ation (12), the larger cable elongation
i
e
is, the smaller
reliability of the cable, it is obvious that the amount of
elongation of the cable is an important factor to affect the
cable Reliability.
4. The Solving of Network Reliability
Reliability assessment not only requires finding the re-
liability of nodes and links, but also requires obtaining
the overall network reliability and considers the impor-
tance of the transmission business.
4.1. Risk Theory
Risk theory is applied to study the possibility of cata-
strophic damage causing and the level of damage severi-
ty considering the system uncertainties [14,15]. Making
use of risk theory to consider the network transmission
business, the product of the probability of network secu-
rity events and the importance of network transmission
business is defined as the risk of network transmission
service reliability, whose mathematical expression is:
( )
1R AI= −
(13)
I is the transmission business; R is reliability r isk valu e.
This article combines the importance of business and th e
reliability calculation together to make the results more
meaningful.
The communication network reliability evaluation
process is shown in Fi gure 2 :
Search all the paths between two communication nodes, and
calculate the reliability of the nodes and linksGet the optimal path
through reliability sort
Through the minimization algorithm processing to the paths
set, get the network reliability of the two communication
nodes
Take N-1/N-2 reliability evaluation for each node and link of
the network Obtain the key/weak nodes
Considering the importance of network transmission
business, use risk theory to evaluate the reliability of the
business
Make up topology structure of the
communication network
Figure 2. Process of reliability assessment.

J. H. YANG ET AL.
33
First calculate the reliability of the nodes and links in
the ice storm, obtaining all the paths and the reliability
between two nodes requiring communication through
improved adjacency matrix method, using binary deci-
sion diagram obtains the overall reliability between two
nodes after disjoint, and then using Equitation (13) com-
bining with the degree of the transport business impor-
tance to get the reliable transmission of business risk
value.
4.2. Improved Adjacency Matrix Method
Seeking all network paths there are adjacency matrix
method, Boolean determinant method and node traversal
method [16,17]. Node traversal method needs judge too
many times that prone to error; it is much more complex
to expand the matrix to the summation of Boolean prod-
uct using Boolean determinant method when more nodes;
the idea of adjacency matrix method is simple and easy
to implement on a computer through the cycle matrix
multiplication, which is a suitable way to solve the com-
plex network paths collection [ 18 ].
Adjacency matrix method algorithm steps are as fol-
lows:
(1) The structure of the associated matrix
Suppose there is an n node, m bar link communication
network, the associated matrix can be expressed as:
=
ij
CC
nn



×
Among them,
1
0
inf
ij
C ij



= =


when it has direct links butween the node i and node j
when it hasn't direct links butween the node i and node j
(2) Using matrix multiplication to calculate all paths
()()( )
11
1
=
n
rr
ijik kjkjkj
k
cccc c
−
=
=∑，
where in
( )
r
ij
c
signify all paths from node i to node j in
length
r
.
Let:
( )
1
1
nr
ij
r
Lc
−
=
=
Thus, L is the collection of all the required paths from
node I to node j in the network.
In order to illustrate the adjacency matrix method
clearly, a typical power optical fiber communication
configuration diagram is putted as an example
The traditional method used the reliability of two links
to replace 1 in the correlation matrix, but this method
only considered the reliability of the link. Owing to the
node may also be in a failure, it must consider the relia-
bility of the node. The way to improve is to let the r elia-
bility of the node equivalent to the reliability of the link,
thus all the reliability of links becomes to the reliability
of the original reliability multiplied by the square root of
two nodes connected thereto, the reliability of which with
the start node and the end node is connected directly
multiplied the reliability of the start node and the end
node in addition. For example, after equivalent the relia-
bility of each link is:
101 102
=A AAA
,
122 125
=AAA A
The same way to equivalent links in other route. The
correction of this equivalent method has been verified
through testing all paths, which will not affect the relia-
bility of the calculated results. Assuming that the relia-
bility of all the nodes and links are obtained using the
method shown above :
A1 = 1.0, A2 = 0.997, A3 = 0.995, A4 = 0.988, A5 =
0.995, A6 = 0.989, A7 = 0.989, A8 = 1.0, A9 = 0.997, A10
=.991, A11 = 0.993, A12 = 0.999, A13 = 0.988, A14 = 0.996,
A15 = 0.987, A16 = 0.995, A17 = 0.999,
Substituting the parameters into1 - 8 path:
Compared with the four paths from node 1 to node 8,
the reliability of path 4 is the highest, which is the op-
timal path. Actually, the reliability of each path is not
very different, due to the path 4 passes the least nodes
and links, the reliability is the highes t obv iously.
According to improve the adjacency matrix method,
considering the reliability of both nodes and links, ap-
plying the method of nodes reliability equivalent to the
link reliability, which makes the algorithm easy to un-
derstand and greatly reduces the calculated items.
Therefore, it is conducive to the computer calculation
and store.
4.3. Binary Decision Diagram
The reliability of each of the paths obtained from the
upper section need for the disjoint processing because
intersecting may happen between each path. The short-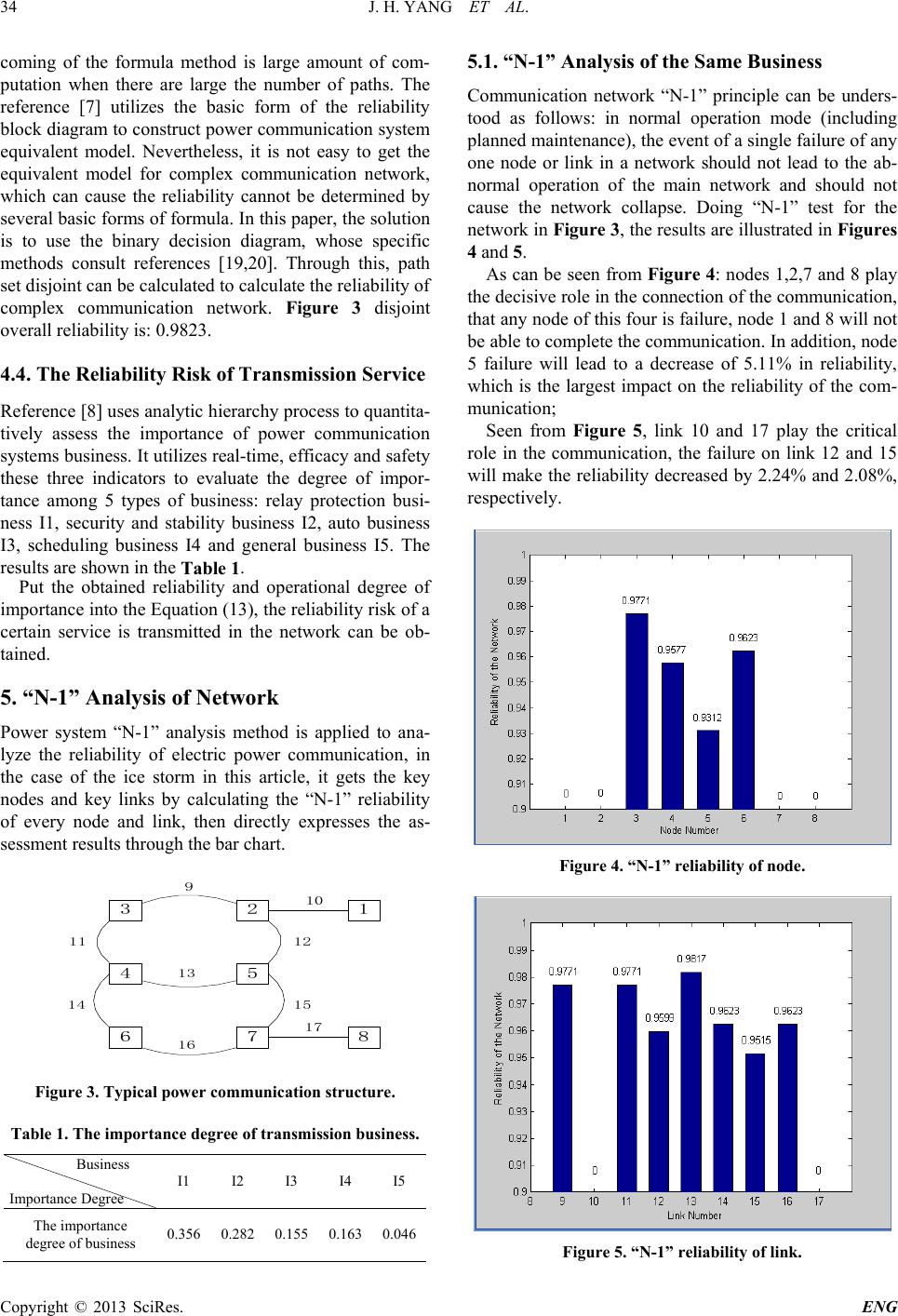

J. H. YANG ET AL.
34
coming of the formula method is large amount of com-
putation when there are large the number of paths. The
reference [7] utilizes the basic form of the reliability
block diagram to construct power communication system
equivalent model. Nevertheless, it is not easy to get the
equivalent model for complex communication network,
which can cause the reliability cannot be determined by
several basic forms of formula. In this paper, the solution
is to use the binary decision diagram, whose specific
methods consult references [19,20]. Through this, path
set disjoint can be calcu lated to calcu late the reliability of
complex communication network. Figure 3 disjoint
overall reliability is: 0. 982 3.
4.4. The Reliability Risk of Transmission Service
Reference [8] uses analytic hierarchy process to quantita-
tively assess the importance of power communication
systems business. It utilizes real-time, efficacy and safety
these three indicators to evaluate the degree of impor-
tance among 5 types of business: relay protection busi-
ness I1, security and stability business I2, auto business
I3, scheduling business I4 and general business I5. The
results are shown in the Table 1.
Put the obtained reliability and operational degree of
importance into the Equation (13), the reliability risk of a
certain service is transmitted in the network can be ob-
tained.
5. “N-1” Analysis of Network
Power system “N-1” analysis method is applied to ana-
lyze the reliability of electric power communication, in
the case of the ice storm in this article, it gets the key
nodes and key links by calculating the “N-1” reliability
of every node and link, then directly expresses the as-
sessment results through the bar chart.
32 1
4 5
67 8
9
10
11 12
13
14 15
16
17
Figure 3. Typical power communication structure.
Table 1. The importance degree of transmission business.
Business
Importance Degree
I1 I2 I3 I4 I5
The importance
degree of business 0.356 0.282 0.155 0.163 0.046
5.1. “N-1” Analysis of the Same Business
Communication network “N-1” principle can be unders-
tood as follows: in normal operation mode (including
planned maintenance), the event of a single failure of any
one node or link in a network should not lead to the ab-
normal operation of the main network and should not
cause the network collapse. Doing “N-1” test for the
network in Figure 3, the results are illustrated in Figu res
4 and 5.
As can be seen from Fi gure 4: nodes 1,2,7 and 8 play
the decisive role in the connection of the communication,
that any node of this four is failure, node 1 and 8 will not
be able to complete the communication. In addition, node
5 failure will lead to a decrease of 5.11% in reliability,
which is the largest impact on the reliability of the com-
munication;
Seen from Figure 5, link 10 and 17 play the critical
role in the communication, the failure on link 12 and 15
will make the reliability decreased by 2.24% and 2.08%,
respectively.
Figure 4. “N-1” reliability of node.
Figure 5. “N-1” reliability of link.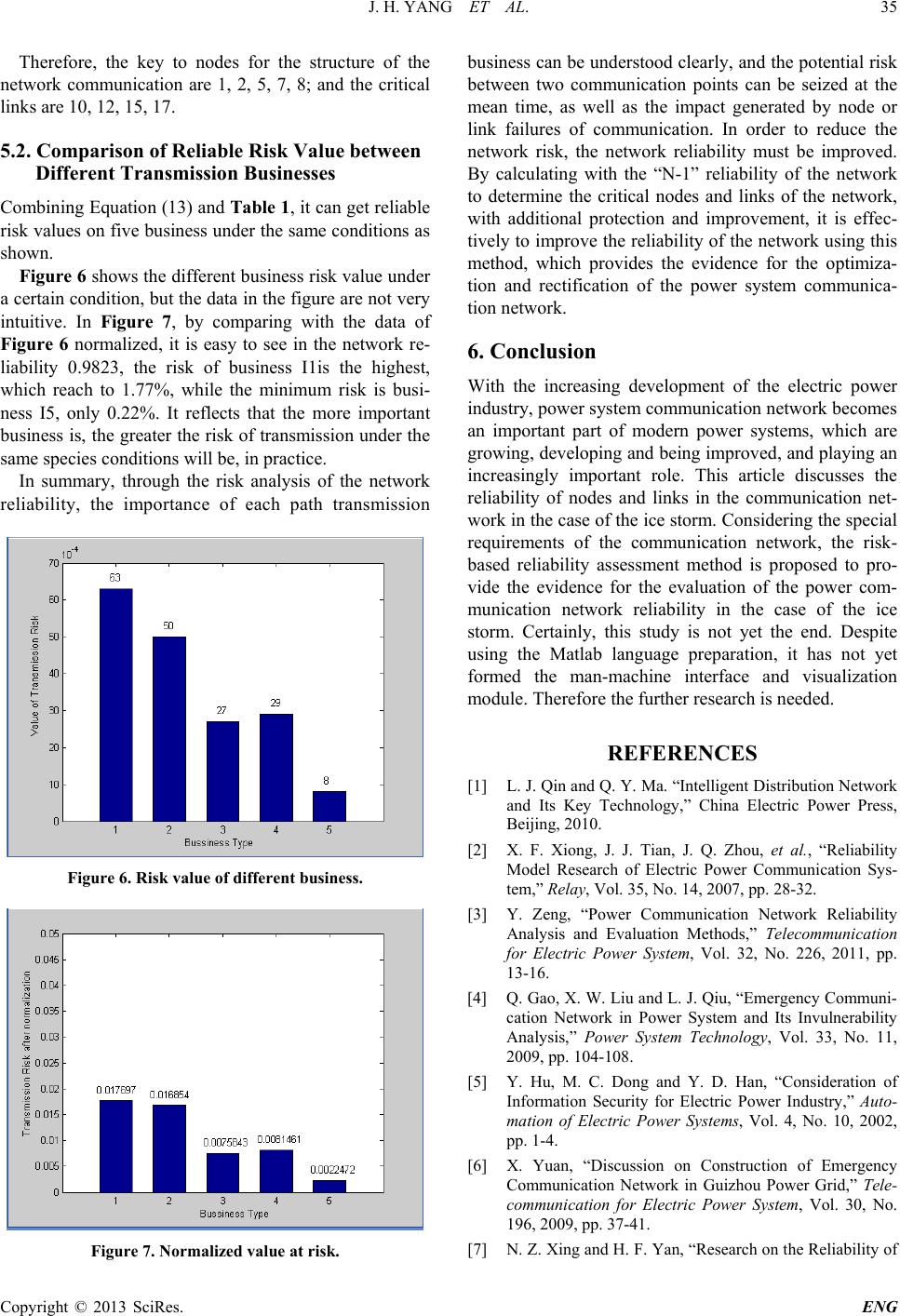

J. H. YANG ET AL.
35
Therefore, the key to nodes for the structure of the
network communication are 1, 2, 5, 7, 8; and the critical
links are 10 , 12, 15, 17.
5.2. Comparison of Reliable Risk Value between
Different Transmission Businesses
Combining Equation (13) and Table 1, it can get reliable
risk values on five business under the same conditions as
shown.
Figu re 6 shows the different business risk value under
a certain condition, but the data in the figure are not very
intuitive. In Figure 7, by comparing with the data of
Figure 6 normalized, it is easy to see in the network re-
liability 0.9823, the risk of business I1is the highest,
which reach to 1.77%, while the minimum risk is busi-
ness I5, only 0.22%. It reflects that the more important
business is, the greater the risk of transmission under the
same species conditions will be, in practice.
In summary, through the risk analysis of the network
reliability, the importance of each path transmission
Figure 6. Risk value of different business.
Figure 7. Normalized value at risk.
business can be understood clearly, and the potential risk
between two communication points can be seized at the
mean time, as well as the impact generated by node or
link failures of communication. In order to reduce the
network risk, the network reliability must be improved.
By calculating with the “N-1” reliability of the network
to determine the critical nodes and links of the network,
with additional protection and improvement, it is effec-
tively to improve the reliability of the network using this
method, which provides the evidence for the optimiza-
tion and rectification of the power system communica-
tion network.
6. Conclusion
With the increasing development of the electric power
industry, power system communication network becomes
an important part of modern power systems, which are
growing, developing and being improved, and p laying an
increasingly important role. This article discusses the
reliability of nodes and links in the communication net-
work in the case of the ice storm. Considering the special
requirements of the communication network, the risk-
based reliability assessment method is proposed to pro-
vide the evidence for the evaluation of the power com-
munication network reliability in the case of the ice
storm. Certainly, this study is not yet the end. Despite
using the Matlab language preparation, it has not yet
formed the man-machine interface and visualization
module. Therefore the further research is needed.
REFERENCES
[1] L. J. Qin and Q. Y. Ma. “Intelligent Distribution Network
and Its Key Technology,” China Electric Power Press,
Beijing, 2010.
[2] X. F. Xiong, J. J. Tian, J. Q. Zhou, et al., “Reliability
Model Research of Electric Power Communication Sys-
tem,” Relay, Vol. 35, No. 14, 2007, pp. 28-32.
[3] Y. Zeng, “Power Communication Network Reliability
Analysis and Evaluation Methods,” Telecommunication
for Electric Power System, Vol. 32, No. 226, 2011, pp.
13-16.
[4] Q. Gao, X. W. Liu and L. J. Qiu, “Emergency Communi-
cation Network in Power System and Its Invulnerability
Analysis,” Power System Technology, Vol. 33, No. 11,
2009, pp. 104-108.
[5] Y. Hu, M. C. Dong and Y. D. Han, “Consideration of
Information Security for Electric Power Industry,” Auto-
mation of Electric Power Systems, Vol. 4, No. 10, 2002,
pp. 1-4.
[6] X. Yuan, “Discussion on Construction of Emergency
Communication Network in Guizhou Power Grid,” Tele-
communication for Electric Power System, Vol. 30, No.
196, 2009, pp. 37-41.
[7] N. Z. Xing and H. F. Yan, “Research on the Reliability of

J. H. YANG ET AL.
36
Electric Power Telecommunication System,” Telecom-
munication for Electric Power System, Vol. 28, No. 176,
2007, pp. 26-30.
[8] A. H. Mohamed, K. Nicholas and H. Sayed, “Towards a
Standardized Terminology for Network Performance,”
IEEE Transactions on Reliability, Vol. 57, No. 2, 2008,
pp. 267-271. http://dx.doi.org/10.1109/TR.2008.920800
[9] P. Deng, X. Peng, L. F. Tian, et al ., “Survivability Analy-
sis of Power System Information Network Bases on
Connectivity,” Engineering Journal of Wuhan University,
Vol. 43, No. 6, 2010, pp. 792-795 .
[10] X. X. Li, W. Zheng and Q. Liu, “Program Development
on Index Evaluation for Inherent Reliability,” Electronic
Communications and Control (ICECC), 2011, pp. 3844-
3847.
[11] X. H. Chen, J. H. Huang, J. M. Zhang, et al. , Telecommu-
nication for Electric Power System, Vol. 29, No. 191,
2008, pp. 8-13.
[12] J. A. Dzekevich and J. Garretson, “A Design Margin
Index for MTBF and Ao,” Reliability and Maintainability
Symposium, Vol. 22, No. 25, 2007, pp. 288-291.
[13] W. A. Chisholm, J. P. Levine and P. Chowdhuri,
“Lightning Arc Damage to Optical Fiber Ground Wires
(OPGW): Parameters and Test Methods,” Power Engi-
neering Society Summer Meeting, Vol. 1, No. 1, 2001, pp.
88-93.
[14] R. X. Liu, J. H. Zhang and D. Wu, “Research on Static
Security Index of Distribution Network Based on Risk
Theory,” Power System Protection and Control, Vol. 39,
No. 15, 2011, pp. 89-95.
[15] W. H. Chen, Q. Y. Jiang and Y. J. Cao, “Risk Assessment
of Power System Cascading Failure Considering Hidden
Failures of Protective Relayings,” Power System Tech-
nology, Vol. 30, No. 13, 2006, pp. 14-19.
[16] Y. Zhou, B. X. Zhou and Y. Xing, “Graphical Power
Network Topology Analysis Based on Adjacengcy Ma-
trix,” Power System Protection and Control, Vol. 37, No.
17, 2009, pp. 49-52.
[17] F. S. Dai, “Objective Evaluation Method of Significance
of Nodes Links in Communication Network,” Journal of
Nanjing University of Science and Technology, Vol. 30,
No. 6, 2006, pp. 748-753.
[18] Y. F. Shi, N. Lu and H. M. Li, “An Algorithm of Network
System Reliability Based on an Improved Disjointed Mi-
nimal Path Set,” Computer Engineering and Science, Vol.
33, No. 1, 2011, pp. 30-35.
[19] D. K. Li, “New Algorithm for Computing Network Sys-
tem Reliability,” Communications Technology, Vol. 42,
No. 11, 2009, pp. 149-151.
[20] M. Q. Wang, H. B. Yu and H. Wang, “Overview on the
Research of Binary Decision Diagrams Optimization,”
Information and Control, Vol. 33, No. 5, 2004, pp.
567-572.