Creative Education
2013. Vol.4, No.10, 663-672
Published Online October 2013 in SciRes (http://www.scirp.org/journal/ce) http://dx.doi.org/10.4236/ce.2013.410095
Exploring Teaching Performance and Students’ Learning
Effects by Two Elementary Indigenous Teachers
Implementing Culture-Based Mathematics Instruction
Wei-Min Hsu1, Chih-Lung Lin2*, Huey-Lien Kao1
1Graduate Institute of Mathematics and Science Education, National Pingtung University of Education, Pingtung,
Taiwan, China
2Department of Computer Science, National Pingtung University of Education, Pingtung, Taiwan, China
Received August 2nd, 2013; revised September 2nd, 2013; accepted September 9th, 2013
Copyright © 2013 Wei-Min Hsu et al. This is an open access article distributed under the Creative Commons
Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the
original work is properly cited.
This study aims to probe into the teaching performance and the effects of implementation of culture-based
mathematics instruction by two indigenous teachers. By case study, this study treats two Paiwan elemen-
tary school teachers as the subjects and collects data by the design of teaching plans, instructional obser-
vations, video recordings, and mathematical cognitive tests. The researcher thus explores their cul-
ture-based curriculum design, instructional implementation, and the effect on Grade 5 and Grade 6 Pai-
wan students’ learning performance of mathematics. The findings demonstrate that prior to implementa-
tion of culture-based mathematics instruction, mathematics learning performance of the students of the
two teachers was behind those of other counties, cities, and schools. The two teachers adopt three types of
instructional design, namely, Paiwan culture and festivals, stories and traditional art, and practice mathe-
matics questions upon cultural situations by teacher demonstration, individual problem-solving, and group
discussion. After the teachers practice 23 and 31 units of culture-based mathematics instruction, the re-
searcher finds that the gap of learning performance between Paiwan students and those in other cities,
counties, and schools is reduced, which demonstrates that culture-based mathematics instruction can en-
hance Paiwan students’ learning performance of mathematics.
Keywords: Culture-Based; Indigenous Students; Mathematics Instruction; Paiwan
Research Background and Purpose
Minority students’ mathematics learning is the focus of the
governments and related researchers in many countries. They
not only suggest that “all students” should be successful in
mathematics learning (Artzt & Armour-Thomas, 2002; Na-
tional Council of Teachers of Mathematics (NCTM), 2000;
Rodriguez, 2005), but also determine better instructional strate-
gies in mathematics for minority students (Boaler & Staples,
2008; Gutstein, 2003; Huang, 2006) in order to accomplish
educational equity. Mathematics knowledge means more than
the students’ subject learned in schools; it also plays the role as
a critical filter and is the most important indicator of individual
success (Ernest, 1998). Moreover, mathematics is one of the
most difficult subjects for minority students (Ensign, 2005;
Rodriguez, 2005). In Taiwan, although many researches have
investigated indigenous people’s mathematics learning, most
focused on “the comprehension” of indigenous students’ learn-
ing performance of mathematics, and attempted to interpret
indigenous students’ inferior learning performance of mathe-
matics per individual, level of school education, and environ-
ment (Chi, 2001; Hsu & Yang, 2009; Li, 2006; Tan & Lin,
2002), but rarely focused on the effects of mathematics cur-
riculum and instruction of students’ mathematics learning.
However, teachers’ mathematics instruction is the key that
directly influences students’ mathematics learning (Gutstein,
2003; Henningsen & Stein, 1997; Hiebert & Grouws, 2007;
Hsu, 2011; Stein, Remillard, & Smith, 2007).
In societies of multiple cultures, two principal objectives of
mathematics instruction are “teaching for understanding” and
“teaching for diversity” (Rodriguez, 2005). In order to fulfill
the instructional goals of “teaching for understanding”, it is
necessary to connect the students’ new learning content with
previous experience when designing curriculum (Wiske, Franz,
& Breit, 2005). Thus, the students will have meaningful learn-
ing and be able to comprehend mathematics concepts; in order
to accomplish “teaching for diversity”, it is important to value
the students’ language and culture in curriculum planning, as
knowledge is part of culture and each student is a unique indi-
vidual with different cultural backgrounds (Barnes, M. B. &
Barnes, L. W., 2005). In other words, in order to fulfill the goals
of mathematics instruction under multiple cultures, curriculum
must include the students’ life experience and culture in cur-
riculum design in order to match the equity emphasized in
mathematics instruction. This view meets many suggestions for
minority learning. For instance, Cummins (1986) suggested that
including the students’ language and culture in curriculum design
*Corresponding author.

W.-M. HSU ET AL.
can enhance their learning performance; Leonard and Dantley
(2005) proposed “culturally relevant teaching”, and indicated
that students should be empowered through instruction, and
their culture and life experience could be included in curricu-
lum design in order to lead to the success of mathematics
learning for minority students; according to Ensign (2005), for
all students, when culture both in and out of schools is closely
connected, they were more likely to achieve successful mathe-
matics learning; by planning and implementation of mathemat-
ics curriculum, based upon life experience, and Gutstein (2003)
effectively enhanced minority students’ learning performance
of mathematics.
The objectives of mathematics instruction in multiple cul-
tures, and the views related to minority students’ mathematics
learning, demonstrate the importance of “culture-based” in-
struction for the students’ mathematics learning. In Taiwan,
although there exists research on indigenous students’ mathe-
matics learning, as well as the factors of their learning per-
formance in mathematics (Chi, 2001; Hsu & Yang, 2009), few
studies investigated the influence of culture-based mathematics
instruction on indigenous students’ mathematics learning. Al-
though Paiwan is one of the main tribes in southern Taiwan,
almost no research has focused on the effect of Paiwan culture
based instruction on Paiwan students’ mathematics learning.
Thus, this study treats two Paiwan elementary school teachers
as the subjects, and by case study, probes into the teachers’
implementation of culture-based mathematics instruction and
effect of culture-based instruction on Paiwan students’ mathe-
matics learning in order to enrich our comprehension of mathe-
matics instruction and learning of indigenous students.
Literature Review
Relationship between Culture and Mathematics
Learning
Mathematics has been regarded as cultural neutral or value
neutral knowledge system. For instance, the “angle sum of a
triangle is 180 degrees”, is a universal principle (Bishop, 1988);
however, anthropologists’ research gradually overthrew this
idea, and they suggested that mathematics was the product of
human cultural development. They studied the development of
mathematics knowledge from the perspective of culture, and
proposed the concept “ethno-mathematics” (D’Ambrosio, 1985).
In studies on the importance of mathematics knowledge and its
contribution to life, suggested that mathematics knowledge was
part of the human culture (Barton, 2009; Orton & Frobisher,
1996). In many countries, mathematics history has been gradu-
ally included in textbooks (Fauvel & Van Maanen, 2000), which
allows students to recognize the evolution of mathematics con-
cepts, and it suggests, “mathematics is a human cultural prod-
uct”, which changes previous views of mathematics knowledge.
Bishop (1988) elaborated on the relationship between mathe-
matics and culture and suggested that different nations develop
their own mathematics knowledge through six common activi-
ties of humans, including counting, locating, measuring, de-
signing, playing, and explaining. Through such activities, they
can create many important concepts related to mathematics
knowledge. Anthropologists suggest that mathematics knowl-
edge is produced through “exploration, invention, use of proper
symbols, symbol normalization, transmission, and sharing
within the culture” (Gerdes, 1996). Barton (2009) suggested
that mathematics knowledge is the knowledge system of hu-
mans, which aims at understanding numbers, relationships, and
spaces encountered in daily life, thus, mathematics knowledge
is closely related to social culture.
In addition, many empirical studies demonstrate that, in Tai-
wan, the reason for indigenous students’ inferior mathematics
learning performance can be attributed to “cultural differences”.
For instance, in traditional indigenous cultures, the people have
language instead of words, thus, they cannot develop logic and
reasoning by words. When encountering complicated mathe-
matical problems, they will not be able to comprehend the
meanings of the questions and solve the problems (Chien,
1998); indigenous tribes emphasize sharing rather than compe-
tition (Chien, 1998), and they use “approximation” rather than
requiring precise numbers (Huang, 2002), which is different
from severely competitive study environments and precision of
mathematics. Thus, Chi (2001) suggested that, due to cultural
differences, there was a gap between indigenous students’
self-concepts constructed in daily life and the science concept,
as instructed in schools.
Mathematics Curriculum and Instruction Suitable for
the Minority
According to the relationship between mathematics and cul-
ture, as well as related research findings, effective instruction
must allow students to learn mathematics based on familiar
culture and life experience. Thus, students can acquire the
mathematics concepts from their original knowledge and ex-
perience. During instruction, teachers should reduce the gap
between the students’ original reasoning model and important
mathematics concepts (Anderson, 2003), as “cultural” differ-
ence might result in indigenous students’ inferior learning per-
formance of mathematics. Based on past research and literature,
this study attempts of determine mathematics curriculum and
instruction suitable for minority students.
Requirements of Culture-Based and Life Experience
Curriculum
Rodriguez (2005) proposed two major goals of mathematics
instruction for multiple cultures: “teaching for understanding”
and “teaching for diversity”, which match “student-centered”
and “understanding-based” purposes in the current reform of
mathematics instruction (Hudson & Miller, 2006; Kilpatrick &
Silver, 2000). In order to accomplish “teaching for understand-
ing”, the design of curriculum based upon students’ life ex-
periences (Anderson, 2003), connect mathematics concepts
with students’ previous experiences and allow students to en-
gage in active exploration and open discussion. Thus, students
can become involved in meaningful learning and gain an un-
derstanding of course content (Wiske et al., 2005). “Teaching
for diversity” should include students’ language and culture
content in courses and curriculum design (Barton, 2009; Cum-
mins, 1986), as this combination can lead to minority students’
successful mathematics learning (Ensign, 2005). Thus, includ-
ing students’ life experiences and culture in mathematics cur-
riculum is the first step to enhance minority students’ learning
performance of mathematics.
Instruction upon Empower ment and Exploration
After the inclusion of culture and life experience in mathe-
matics curriculum, how to implement instruction that effec-
tively enhances minority students’ mathematics learning per-
664

W.-M. HSU ET AL.
formance and accomplishes the goals of mathematics instruc-
tion becomes the issue of focus. Cummins (1986) suggested
that instruction should be based upon interaction and collabora-
tive learning. Thus, minority students are provided with oppor-
tunities in active participation, thinking, exploration, discussion,
and opinion sharing. In class, students change from passive
listening and receiving to active thinking and problem-solving
in order to be successful in learning. Wiske et al. (2005) sug-
gested that, besides the students’ life experience, instruction
should allow students to comprehend concepts by multiple
representations, such as objects, images, and symbols. In addi-
tion, through exploration, students can comprehend concepts by
continuous construction and feedback during interactions with
others. Through the design of interesting open-ended questions,
students can participate in discussions from different perspec-
tives, thus, increasing learning. According to M. B. Barnes and
L. W. Barnes (2005), teachers should allow students to explore
and help them to recognize the gap between the world they
perceive and the world of science through the construction of
bridges. In the process, the teachers should be sensitive and
continuously review students’ comprehension and modify the
instruction in order to provide the students with different assis-
tance and guidance. The above statements emphasize empow-
erment and exploration oriented mathematics instruction. Thus,
students can explore the relationships of mathematics concepts,
and through cooperation and dialogue, their mathematics
learning will be enhanced (Feldman, 2003; Fuson et al., 2000).
Statements related to minority students’ mathematics instruc-
tion reflect the current trends of mathematics instruction, plac-
ing emphasize on active participation, exploration, and con-
struction through comprehension (Artzt & Armour-Thomas,
2002; Hudson & Miller, 2006; NCTM, 2000).
Inspiration from Related Studies : Ef fec tiv eness of
Culture-Based Mathematics Instruction
Mathematics concepts are originated from the daily life ex-
perience model (White & Mitchelmore, 2010), which is a
knowledge system developed to solve the problems related to
numbers, relationships, and spaces in daily life (Barton, 2009).
Therefore, design of a mathematics curriculum and instruction
must include the students’ cultural experiences in order to ac-
complish the goal of equity in mathematics learning.
From the perspective of a mathematics curriculum, Gutstein
(2003) probed into the effect on students’ mathematics learning
by treating the students of immigrants from Latin America,
low-income work class, and studies in urban schools as the
subjects. By curriculum and instructional design, he intended to
allow students to learn the world through mathematics, culti-
vate mathematics ability, and change students’ attitudes toward
mathematics. In order to connect mathematics learning and
students’ life experiences, Gutstein not only selected the text-
book “Mathematics in Context”, but also designed mathematics
questions matching reality. After implementation for two years,
students could demonstrate their problem solving abilities by
varied methods, and with effective communication.
Boaler and Staples (2008) treated three high schools, with
students of different backgrounds as the subjects, and con-
ducted a five-year study. They found that the students of the
Railside high school were consisted of varied ethic groups,
were mostly from low-income families, and their mathematics
performance was significantly lower than two high schools.
Through cooperation and interaction, the teachers conducted
instruction by open-ended questions regarding concepts with
high cognitive demands (in the other two high schools, the
teachers adopted more traditional and closed-ended questions).
Testing after implementation for one year demonstrated that
mathematics performance of the students in Railside was the
same as those in the other two high schools. After implementa-
tion for two years, the mathematics performance of the students
in Railside was significantly higher than those in the other two
high schools. Moreover, the gap of mathematics performance
among the students in different ethic groups in Railside was the
least among the three schools, most students intended to study
more advanced mathematics courses, and they were more posi-
tive toward mathematics learning.
The findings of above two researches not only match the
theoretical suggestion of minority students’ mathematics cur-
riculum and instruction, but also show the effects of enhance-
ment of minority students’ mathematics learning performance
(including cognition and affection). In Taiwan, although there
are 14 different indigenous tribes, few studies probe into the
effects of culture-based curriculum or instruction on the stu-
dents’ mathematics learning. The research of Hsu and Yang
(2009) demonstrated that when indigenous students were used
to solving mathematics questions with regular processes and
teachers’ direct demonstrations, the design of culture-based
mathematics questions and group discussions would be resisted
by students, and thus, would influence the effectiveness of in-
structional implementation. The findings of Hsu and Yang
(2009) did not totally match the research results of Gutstein
(2003), or Boaler and Staples (2008). This not only demon-
strates the complicated factors of indigenous students’ mathe-
matics learning, but also suggests that it is worthy to probe into
culture-based mathematics instruction in Taiwan.
Research Method, Subjects, and Field
Research Method
In Taiwan, there exist only primary studies on the enhancement
of indigenous students’ learning performance of mathematics
by culture-based mathematics instruction. In addition, teachers’
mathematics instructions are a highly personalized result with
varied factors (Artzt & Armour-Thomas, 2002; Hsu, 2011).
Case study is suitable for both primary study and complicated
issues for exploring the process and effectiveness of the teach-
ers’ implementation of culture-based mathematics instruction.
Research Subjects
Two teachers, Wei and Ling (pseudonym), who participate in
this study, are Paiwan people. Currently they teach Grades 5
and 6 in the Happy Elementary School (pseudonym, HES) of
the Paiwan tribe in Pingtung County, Taiwan. They have taught
for 3 and 5 years, respectively. Before participating in the re-
search project, Wei and Ling tended to conduct traditional lec-
tures in mathematics instruction. After an explanation, they
allowed the students to practice with questions. When solving
the problems, Wei usually cooperated with the students through
questions and responses. The questions were mostly close-
ended and with fixed answers. Ling asked the students to the
stage only when encountering special questions. After integrat-
ing culture-based instruction, both teachers decided to change
their teaching methods from the traditional means. Wei and
Ling asked students to discuss and cooperate in class rather

W.-M. HSU ET AL.
than the traditional means, lecturing. They provided more op-
portunities for students to discuss their thinking and ideas on
problem solving. Therefore, more student interaction and em-
powerment were found in their classrooms.
Research Field
HES is located in the indigenous tribe of Pingtung County,
and is a small school, with one class for each grade. There are
more than 60 students in the school, and they are Paiwan people.
The school is at a distance of about 20 minutes drive from other
towns in the neighborhood. Most of the students are nurtured
by grandparents, and young adults mostly work out of town.
Although the tribe is an independent community, it is not iso-
lated from the external world. There are two internet cafes and
most of the students have cable TV at home. Common student
hobbies after school are attending the internet cafes or watching
TV. On holidays, the tribe hosts activities, thus, the students in
HES approach both traditional culture and external information.
Data Collection
Instructional Observations and Video Recordings
In order to probe into the two teachers’ implementations of
culture-based mathematics instruction, the researcher collects
the data of one semester by instructional observations and video
recordings (From March to June 2011). Wei and Ling recorded
23 and 31 lessons in one semester. The focuses of observation
and video recording were based on the suggestions of Stein et
al. (2007) and Hsu (2011), and were the content and imple-
mentation of mathematics questions.
Interviews and Documents
Interviews were conducted immediately following the two
teachers’ instruction, and lasted 10 - 20 minutes. The purpose
was to learn the teachers’ aims to design the teaching plans,
thoughts after implementation, students’ reactions, and future
improvements. Throughout the interviews, the researcher in-
tended to probe into the teachers’ instructional implementation
and determine the effects of the students’ reactions on teachers’
instructional implementation, and learn the teachers’ feelings
regarding the implementation and objectives of mathematics
instruction.
Cognitive Test of Mathematics
According to the specifications of the national curriculum
outline of mathematics in 2003 (Ministry of Education, 2003),
the researcher plans cognitive testing of mathematics. Specifi-
cations in Taiwan not only emphasize mathematics concept, but
also indicate the cultivation of mathematics abilities. Thus,
mathematics concepts and mathematics abilities become two
critical dimensions in the design of cognitive testing. Regarding
mathematics concepts, this study analyzes the concepts of
mathematics learning required by students upon reaching the
first semesters of Grades 5, 6, and 7 as the criteria to design
tests. Mathematics abilities include “conceptual comprehen-
sion”, “calculation fluency”, and “problem solving” based on
the ability indicators of the curriculum outline.
Data Analysis
Qualitative Data
Qualitative data includes instructional observations and video
recordings, as well as teachers’ interview records and docu-
ments. Regarding video recordings of instructions, the re-
searcher transcribes the instructional processes, treats the
mathematics questions as units, and analyzes the content and
implementation of mathematics questions in order to learn the
teachers’ implementation of culture-based mathematics instruc-
tion. Regarding the content of mathematics questions, the re-
searcher reviews the suggestion of Stein et al. (2007) and Hsu
(2011) on the analysis of mathematics instruction, as well as
past literature related to minority’s mathematics instruction
(Barton, 2009; Cummins, 1986). The researcher analyzes the
sources and situations of mathematics questions. The sources
include the total matches between the questions and the text-
books, partial revisions, and self-design (Llody, 2008). Ac-
cording to the sources, the researcher can recognize the teach-
ers’ roles and the role of textbooks in culture-based mathemat-
ics instruction. The situations of the questions, according to the
relationship with Paiwan culture, are divided into cultural situa-
tions, common situations, and pure calculation. The analysis of
the situations shows the culture-based degree and focus of the
teachers’ instruction. Interviews and records will be transcribed
and encoded. The encoding is based on “date-category” and the
date is shown by “month-day”.
Quantitative Data
Analysis of quantitative data refers to the analysis of cogni-
tive testing. After designing mathematics cognitive tests, the
researcher invites two experienced mathematics education re-
searchers to conduct expert testing and review the formation of
the two-way specification table, classification of tests, and con-
tents of tests in order to share opinions regarding the revision of
the tests. After expert review and revision of the tests, the re-
searcher examines the quality of tests by the pretest. Regarding
“from Grades 4 to 5”, there are 177 participants, in 7 classes, in
4 schools, in the pretest. Average difficulty is .58 and average
discrimination is .54. Internal consistency reliability α is .89.
Regarding “Grades 5 to 6”, there are 295 participants, from 12
classes, in 6 schools, in the pretest. Average difficulty is .62,
average discrimination is .49, and internal consistency reliabil-
ity α is .86. Regarding “Grades 6 to 7”, there are 191 partici-
pants, in 7 classrooms, in 4 schools, in the pretest. Average
difficulty is .53, average discrimination is .49, and internal
consistency reliability α is .86. The pretest was conducted in
October 2010. In pretesting, according to the percentages of the
schools, through purposive stratified sampling, the researcher
conducted pretesting on schools of different scales in Pingtung
County and Kaohsiung City. By the end of June 2011, the
post-testing was randomly conducted in the schools from pre-
testing.
Reliability and Validity of Data Analysis
Regarding qualitative data, the classifications (situations and
implementations of mathematics questions) are not only based
on the suggestions of Stein et al. (2007) and Hsu (2011), but
also rely on three researchers to construct score reliability. First,
the researchers explained the definitions of the categories to
three researchers, and tested the analysis by the recordings of
one class period. The results showed that, regarding the classi-
fication of situations and implementations of the questions, the
three researchers’ analytical results are consistent. The sources
of questions are compared with mathematics questions in text-
books in order to allocate them into “entirely the same as the
666

W.-M. HSU ET AL.
textbooks”, “partially the same as the textbooks”, or “entirely
different from the textbooks”. Regarding quantitative data,
according to the specifications and objectives of national cur-
riculum, each cognitive test of mathematics is turned into a
two-way specification table. After editing the tests, the re-
searcher invited two experienced mathematics education re-
searchers to revise the tests, and then modify the pretest, the
items, and construct proper difficulty, discrimination, and in-
ternal consistency reliability.
Research Results
Mathematics Learning Performance of the Students
in HES before Culture-Based Mathematics
Instruction
There are 2 large schools (at least 24 classes) in Pingtung
County (PC), with 3 classes of participants in each school; 4
medium schools (12 - 23 classes), with 2 classes of participants
in each school; and 6 small schools (under 11 classes) (includ-
ing HES), with one class of participants in each school. There
are 12 schools, 20 classes, 466 Grade 5, and 475 Grade 6 stu-
dents in the pretest. Regarding Kaohsiung City (KC), there are
4 large schools, with 3 classes of participants in each school;
and 2 medium schools with 2 classes of participants in each
school. A total of 16 classes, 459 Grade 5 and 477 Grade 6
students participated in the pretest. The number of selected
schools is based on the percentages of the schools in different
counties and cities.
Regarding the locations of the schools (Table 1), in the pre-
test, Grades 5 and 6 students in the HES demonstrate an aver-
age number of items with correct responses, lower than those in
PC and KC. Since the numbers of the samples in the pretest are
extremely different, the researcher conducted the analysis by
the methods of Post hoc Comparison. Grade 5 students are
analyzed by the Post hoc Comparison of Scheffe and LSD. It
demonstrates that only KC and PC reveal significant differ-
ences (p < .001); Grade 6 students are analyzed by the Scheffe
method, which shows that KC and PC reveal significant differ-
ences (p = .046). Through LSD, KC & PC (p < .001) and KC &
HES (p = .023) show significant differences.
Regarding the school scale (Table 2), Grade 5 students are
analyzed by the Post hoc Comparison of the Scheffe and LSD
method. The researcher demonstrates that there are significant
differences between large and medium schools (p < .001), and
between large and small schools (p < .001). Moreover, through
LSD, large schools and HES reveal no significant differences (p
= .06). Grade 6 students are analyzed by the Scheffe and LSD
method, which shows that there are significant differences be-
tween large and small schools (p < .001), and between medium
and small schools (p < .001). And through LSD, the researcher
realizes that there are also significant differences between large
schools and the HES (p = .049).
Teachers’ Implementation of Culture-Based
Mathematics Instruction
Instructional Design upon Cultural Situations and
Conceptual Frameworks of the Textbooks
Regarding lesson plan design before the instruction, the two
teachers focused on the content in the textbooks (regarding the
concern of national curriculum), and the design of each unit is
based on one cultural theme or story. In the design of four units,
Table 1.
Mean and standard deviation of students’ pretest performance from
different school locations.
GradeLocation# of SubjectsMean SD Df F Sig.
HES 9 22.56 2.10
PC 457 23.63 .40 Grade 5
KC 459 26.14 .32
2 12.90<.001***
HES 10 19.80 2.24
PC 465 22.00 .28 Grade 6
KC 477 24.10 .26
2 16.40.003**
Note: **p < .01, ***p < .001.
Table 2.
Mean and standard deviation of students’ pretest performance from
different school scale.
GradeSchool Scale# of Subjects Mean SD Df F Sig.
HES 9 22.56 2.10
Large 571 27.09 .25
Medium 253 21.14 .59
Grade
5
Small 92 21.51 .76
3 48.18<.001***
HES 10 19.80 2.24
Large 578 23.51 .24
Medium 269 23.24 .36
Grade
6
Small 95 19.86 .62
3 11.46<.001***
Note: ***p < .001.
Wei used one type of culture and festivals (including a Paiwan
wedding and a harvest ritual). In six units, Ling adopted three
methods: culture and festivals (hunting season and wedding),
stories (legend of the origin of Paiwan and Kua’s and Tai’s
participation in harvest ritual), and traditional art (Paiwan
handicraft and traditional art fair). Using line symmetry units
(Paiwan handicrafts) designed by Ling as an example, the unit
includes three important concepts: introduction of figures of
line symmetry, introduction of symmetrical points, symmetrical
edges, and symmetrical angles and drawing of line symmetry
figures. Regarding each concept, Ling used related traditional
handicraft figures as the teaching materials. For instance, in the
introduction of line symmetry, Ling allows the students to ob-
serve line symmetry in the Figure 1.
In culture and festivals, or stories in the design of teaching
plans, in the first section of the unit, the teacher describes the
situations related to the festivals or mythology, and designs
mathematics questions for students’ problem-solving according
to the situations.
Before culture-based instruction, the two teachers would
analyze the contents of the textbook, and extract the main con-
cepts of mathematics in the culture-based unit. Then they would
consider cultural customs, festivals, stories, or traditional art as
the base of the unit. Thus, in cultural situations, the students
can learn the mathematics concepts in the textbook.

W.-M. HSU ET AL.
Figure 1.
The figures of Paiwan traditional handicrafts.
Mathematics Questions Are Mostly from the Textbooks,
However, They Are Revised According to the Situations of
Paiwan Cul ture
1) Sources of Mathematics Questions
When designing culture-based lesson plans, the two teachers
followed the conceptual framework of the textbooks, and
adopted and designed the units according to related cultural
situations. Thus, the sources of mathematics questions are
mostly from the textbook. In 23 lessons, Wei used 105 mathe-
matics questions, for an average of 4.6 questions adopted in
each lesson. In 31 lessons, Ling used 271 mathematics ques-
tions, for an average of 8.7 questions adopted in each lesson.
The researcher compared mathematics questions adopted by
the two teachers with the questions in the textbook, and realized
that, from among the 105 mathematics questions of Wei, only 3
are entirely the same as those in the textbooks. The teacher
describes the definitions and relationships according to the
questions in the textbooks: 1) question is used in the unit “equa-
tion and problem-solving”. The question introduces the equa-
tion of the circumference of a rectangle; 2) questions are used
in “problem solving”, and one describes the meanings of base
and comparison. One question describes the definition of “price
= cost × (1 + profit)”. The remaining 102 questions are “par-
tially the same” as the textbooks. Some questions are adopted
into the situations of Paiwan culture, some add situations re-
garding students’ levels (non-Paiwan culture), and some are the
questions of pure calculation. However, the types of the above
three types of questions are the same as the textbooks. Among
271 mathematics questions, Ling used 130 questions entirely
the same as the textbooks, 139 questions that are partially the
same and 2 questions entirely different. Ling used many ques-
tions from the textbooks, as she adopts the practices in the
textbooks. In addition, after finishing culture-based instruction,
she mostly reviewed the questions in the textbooks with the
students (she reviewed 27 out of 31 lessons). Thus, she used
more mathematics questions than Wei. Ling suggests that there
are key points in the questions or activities designed in the
textbooks, and the editors have rich knowledge and experience.
In order to allow the students to have complete learning and
attempt to determine if the students learn the related mathemat-
ics concepts in culture-based situations, the teacher reviewed
the questions in the textbooks, with the focus on the practices
(0503 interviews). Regarding the questions that are partially the
same, the types are the same as those in the textbooks; however,
the numbers or situations are adopted. Regarding the 2 ques-
tions that are entirely different, they are the questions of review
for Ling before implementing the units. For example, in “deci-
mal fraction multiplication and estimation”, the teacher first
reviews addition and subtraction of decimal fraction (0315
observation); in “time calculation”, before the multiplication
and division of time, the teacher first reviews addition (0411
observation). The sources of the questions used by the two
teachers in the instruction are as shown in Table 3.
2) Situations of Questions
Upon the situations of the questions, the researcher analyzes
the types of mathematics questions adopted by the two teachers,
and demonstrates that the types can be allocated into three
categories: Paiwan culture situations, common situations, and
pure calculation. Wei used culture-based questions the most,
while Ling used three types of questions. The statistics of the
types of mathematics questions used by the two teachers are
shown in Table 4. Chi-square testing on the difference of the
types of questions used by the teachers shows that Wei signifi-
cantly focused on Paiwan culture, while Ling does not demon-
strate significant differences (χ2 values of Wei and Ling are
55.6 and .49, respectively, and p value is .78).
Implementation of the Questions is Based on Individual
Problem Solving, and Problem-Solving Relies on Teacher
Demonstrations
The researcher studied two teachers’ implementation through
mathematics questions as the units, and demonstrates that the
two teachers both adopt three methods: teachers’ demonstration,
the students’ individual problem solving, and group discussion.
Teachers’ demonstration means after introducing mathematics
questions, the two teachers directly explain the meanings of the
questions and demonstrate the methods and results, or directly
inform the students of the problem-solving method and proc-
esses for their calculations and do not allow them to have the
opportunity to think, discuss, or share opinions. The students’
individual problem solving and group discussion mean after
introducing the questions, the teachers ask the students to solve
the problems individually or by group discussion. After the
students complete the work, individually or in groups, the two
teachers enact three types of actions: including providing the
answers without description and explanation (the students can
compare their answers with the correct ones), direct demonstra-
tion (sometimes they invite the students to write their solution
on the board and the teachers directly explain the solution), and
asking students to share their solutions on the board. Wei di-
rectly demonstrates 20 mathematics questions (19%), the stu-
dents’ solve 44 questions individually (41.9%), and solve 41
questions in group discussion (39.1%); Ling directly demon-
strates 29 questions (10.7%), the students solve 161 questions
individually (59.4%), and solve 81 questions in group discus-
sion (29.9%). The two teachers mostly allow the students to
solve the problems individually and rarely demonstrate the
solution. However, Wei, in comparison to Ling, adopted group
discussions more frequently. Statistics of the implementation of
the two teachers’ mathematics questions are as shown in Table 5 .
668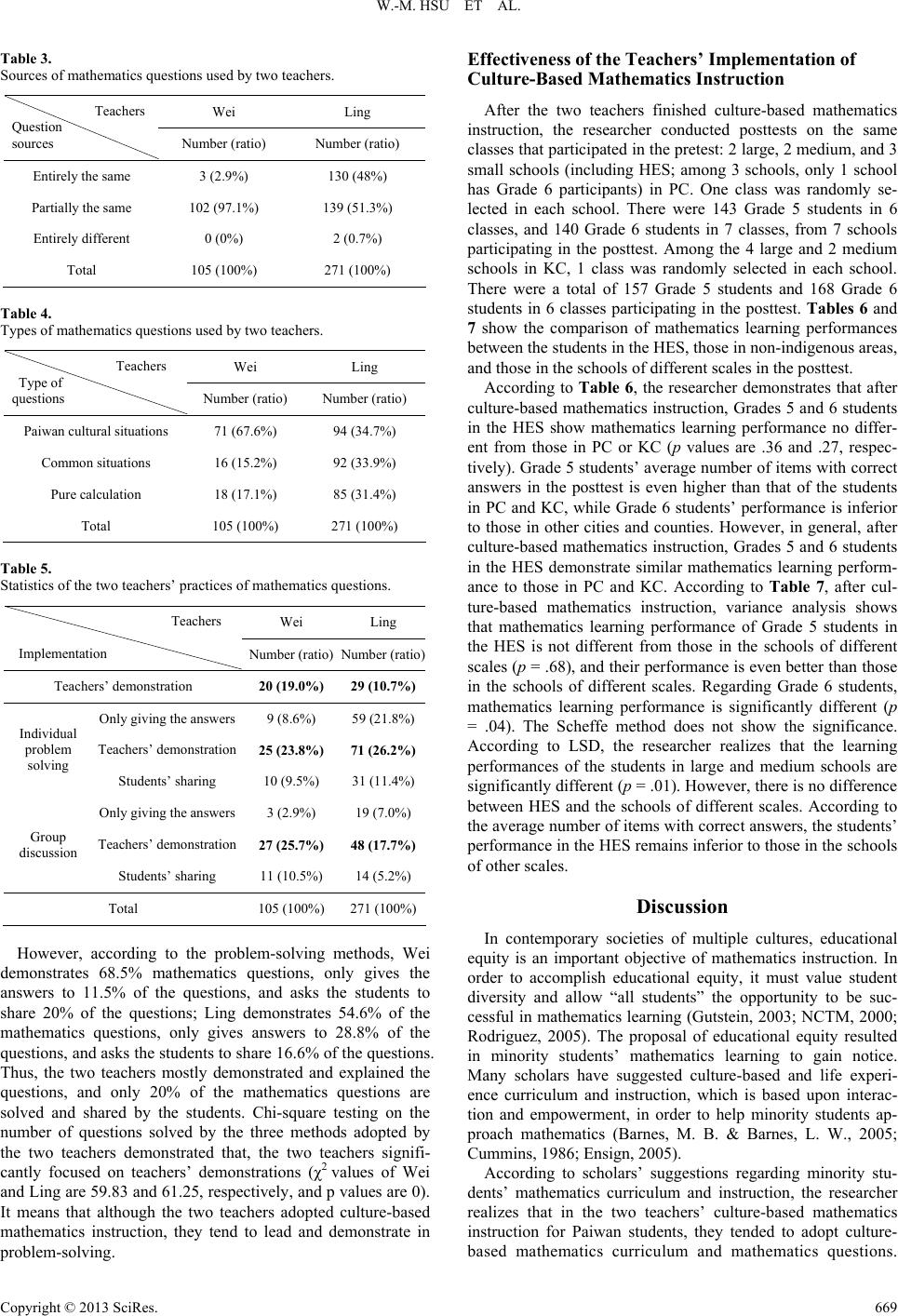

W.-M. HSU ET AL.
Table 3.
Sources of mathematics questions used by two teachers.
Wei Ling
Teachers
Question
sources Number (ratio) Number (ratio)
Entirely the same 3 (2.9%) 130 (48%)
Partially the same 102 (97.1%) 139 (51.3%)
Entirely different 0 (0%) 2 (0.7%)
Total 105 (100%) 271 (100%)
Table 4.
Types of mathematics questions used by two teachers.
Wei Ling
Teachers
Type of
questions Number (ratio) Number (ratio)
Paiwan cultural situations 71 (67.6%) 94 (34.7%)
Common situations 16 (15.2%) 92 (33.9%)
Pure calculation 18 (17.1%) 85 (31.4%)
Total 105 (100%) 271 (100%)
Table 5.
Statistics of the two teachers’ practices of mathematics questions.
Wei Ling Teachers
Implementation Number (ratio) Number (ratio)
Teachers’ demonstration 20 (19.0%) 29 (10.7%)
Only giving the answers9 (8.6%) 59 (21.8%)
Teachers’ demonstration25 (23.8%) 71 (26.2%)
Individual
problem
solving
Students’ sharing 10 (9.5%) 31 (11.4%)
Only giving the answers3 (2.9%) 19 (7.0%)
Teachers’ demonstration27 (25.7%) 48 (17.7%)
Group
discussion
Students’ sharing 11 (10.5%) 14 (5.2%)
Total 105 (100%) 271 (100%)
However, according to the problem-solving methods, Wei
demonstrates 68.5% mathematics questions, only gives the
answers to 11.5% of the questions, and asks the students to
share 20% of the questions; Ling demonstrates 54.6% of the
mathematics questions, only gives answers to 28.8% of the
questions, and asks the students to share 16.6% of the questions.
Thus, the two teachers mostly demonstrated and explained the
questions, and only 20% of the mathematics questions are
solved and shared by the students. Chi-square testing on the
number of questions solved by the three methods adopted by
the two teachers demonstrated that, the two teachers signifi-
cantly focused on teachers’ demonstrations (χ2 values of Wei
and Ling are 59.83 and 61.25, respectively, and p values are 0).
It means that although the two teachers adopted culture-based
mathematics instruction, they tend to lead and demonstrate in
problem-solving.
Effectiveness of the Teachers’ Implementation of
Culture-Based Mathematics Instruction
After the two teachers finished culture-based mathematics
instruction, the researcher conducted posttests on the same
classes that participated in the pretest: 2 large, 2 medium, and 3
small schools (including HES; among 3 schools, only 1 school
has Grade 6 participants) in PC. One class was randomly se-
lected in each school. There were 143 Grade 5 students in 6
classes, and 140 Grade 6 students in 7 classes, from 7 schools
participating in the posttest. Among the 4 large and 2 medium
schools in KC, 1 class was randomly selected in each school.
There were a total of 157 Grade 5 students and 168 Grade 6
students in 6 classes participating in the posttest. Tables 6 and
7 show the comparison of mathematics learning performances
between the students in the HES, those in non-indigenous areas,
and those in the schools of different scales in the posttest.
According to Table 6, the researcher demonstrates that after
culture-based mathematics instruction, Grades 5 and 6 students
in the HES show mathematics learning performance no differ-
ent from those in PC or KC (p values are .36 and .27, respec-
tively). Grade 5 students’ average number of items with correct
answers in the posttest is even higher than that of the students
in PC and KC, while Grade 6 students’ performance is inferior
to those in other cities and counties. However, in general, after
culture-based mathematics instruction, Grades 5 and 6 students
in the HES demonstrate similar mathematics learning perform-
ance to those in PC and KC. According to Table 7, after cul-
ture-based mathematics instruction, variance analysis shows
that mathematics learning performance of Grade 5 students in
the HES is not different from those in the schools of different
scales (p = .68), and their performance is even better than those
in the schools of different scales. Regarding Grade 6 students,
mathematics learning performance is significantly different (p
= .04). The Scheffe method does not show the significance.
According to LSD, the researcher realizes that the learning
performances of the students in large and medium schools are
significantly different (p = .01). However, there is no difference
between HES and the schools of different scales. According to
the average number of items with correct answers, the students’
performance in the HES remains inferior to those in the schools
of other scales.
Discussion
In contemporary societies of multiple cultures, educational
equity is an important objective of mathematics instruction. In
order to accomplish educational equity, it must value student
diversity and allow “all students” the opportunity to be suc-
cessful in mathematics learning (Gutstein, 2003; NCTM, 2000;
Rodriguez, 2005). The proposal of educational equity resulted
in minority students’ mathematics learning to gain notice.
Many scholars have suggested culture-based and life experi-
ence curriculum and instruction, which is based upon interac-
tion and empowerment, in order to help minority students ap-
proach mathematics (Barnes, M. B. & Barnes, L. W., 2005;
Cummins, 1986; Ensign, 2005).
According to scholars’ suggestions regarding minority stu-
dents’ mathematics curriculum and instruction, the researcher
realizes that in the two teachers’ culture-based mathematics
instruction for Paiwan students, they tended to adopt culture-
based mathematics curriculum and mathematics questions.

W.-M. HSU ET AL.
Table 6.
Mean and standard deviation of students’ posttest performance from
different school locations.
Grade School scale # of subjects Mean SD Df F Sig.
HES 9 23.78 1.51
PC 134 22.18 .54
Grade 5
KC 157 23.12 .47
2 1.03.36
HES 10 17.70 1.69
PC 130 20.14 .58 Grade 6
KC 168 20.89 .54
2 1.32.27
Table 7.
Mean and standard deviation of students’ posttest performance from
different school scale.
Grade School scale # of subjects Mean SD Df F Sig.
HES 9 23.78 1.51
Large 182 22.99 .43
Medium 92 22.18 .65
Grade 5
Small 17 22.18 1.80
3 .5.68
HES 10 17.70 1.69
Large 158 21.51 .52
Medium 117 19.38 .64
Grade 6
Small 23 20.00 1.49
3 2.91.04*
Note: *p < .05.
Without changing the original conceptual framework and order
of the textbooks, the teachers adopted units and questions into
Paiwan culture related situations, including customs, festivals,
legends, and traditional art, in order to trigger students’ learning
motivation, where students can learn mathematics from the
cultural situations. According to Paiwan students’ performance
in pretests and posttests, in comparison to students of other
schools, culture-based instruction and implementation should
enhance Paiwan students’ mathematics learning performance.
However, regarding instruction methods, although the two
teachers adopted teacher demonstrations, individual problem
solving, and group discussions, they tended to lead the prob-
lem-solving processes and results, and failed to adopt interac-
tion, exploration, and empowerment, as suggested by other
scholars. The main reason is that the two teachers were adopt-
ing culture-based instruction for the first time; however, it is
difficult to change one’s original teaching habits or patterns,
particularly when theory suggests that the teachers’ past in-
struction or learning experiences are different (Rodriguez, 2005;
Stuart & Thurlow, 2000). Therefore, it is understandable that
the two teachers mostly demonstrated the lessons; however, if
the two teachers were to adopt interaction and empowerment
oriented instruction, as suggested by theory, would they effec-
tively enhance Paiwan students’ mathematics learning? The
answer to this question will rely on further study.
Both the teachers used the design and implementation of
culture-based mathematics instruction. However, why does it
seem that Grade 5 students’ learning effectiveness is more sig-
nificant than Grade 6 students? The reason can be that before
the instruction, Grade 5 students’ learning performance is not
significantly different from those in other areas. In addition, it
can be because Ling was more involved and provided more
opportunities of mathematics question practice. Regarding the
design of unit plans, 6 culture-based unit plans are designed by
Ling. Among the unit plans designed, Wei designed only one 1
independently (Mayi’s harvest ritual). Mayi’s wedding is the
adoption of a unit plan designed by Ling. Teachers’ personal
commitment and involvement are the keys to students’ learning
effectiveness, as demonstrated in the research of Boaler and
Staples (2008), and Gutstein (2003). However, how do the
teachers’ involvement and offerings of practice opportunities
influence indigenous students’ mathematics learning? This is an
issue for further exploration by future research.
Conclusions and Suggestions
Conclusions
This study treats two Paiwan teachers as subjects and probes
into their implementation of culture-based mathematics instruc-
tion, and the possible effects on Grades 5 and 6 indigenous
students’ mathematics learning. Regarding the implementation
of culture-based mathematics instruction, this study demon-
strates that when two teachers design culture-based mathemat-
ics instruction, they rely on the conceptual framework in text-
books, and consider situations that include Paiwan culture, such
as culture and festivals, stories, and traditional art of Paiwan;
although most of the mathematics questions in the instruction
are from the textbooks, they turn the situations in the questions
into those related to Paiwan culture, while the types and struc-
tures remain the same. Ling uses more mathematics questions
that are entirely the same as in the textbooks. The reason is that
after implementing culture-based mathematics instruction, she
was concerned about the representative nature of the content in
the textbooks and reviewed the students’ learning effectiveness.
Thus, she guides the students to review mathematics questions
in the textbooks, which is why she used more mathematics
questions than Wei (by almost two times). Regarding the situa-
tions of mathematics questions, Wei significantly used ques-
tions with cultural situations, whereas Ling focused on ques-
tions related to cultural situations, common situations, and pure
calculation. Regarding the implementation of mathematics
questions, although the two teachers use demonstrations, indi-
vidual problem solving, and group discussions, according to the
problem solving methods and results, the two teachers signifi-
cantly relied on demonstrations, and rarely allowed the students
to think and discuss. Although the two teachers’ culture-based
instructional implementation is different from the views of
theoretical argument in the literatures, particularly the empow-
erment of students’ learning, after their implementation of cul-
ture-based mathematics instruction, the gap of mathematics
learning performance between the students in the school and
those in other regions, and with medium and large scales, is
reduced. Grade 5 students’ performance is similar to those in
the schools of Pingtung County and Kaohsiung City; moreover,
their average number of items with correct responses is higher
than those in schools of Pingtung County, Kaohsiung City, and
of different scales. In addition to culture-based mathematics
instruction, success can be related to the active involvement of
Ling, and more practices with mathematics questions. However,
the effect of these two factors should be further studied.
670

W.-M. HSU ET AL.
Suggestions
Regarding the findings, this study proposes the suggestions
below for future researchers or teachers. First, regarding the
design of culture-based mathematics instruction, this study
demonstrates that the teachers’ design is based on the concep-
tual framework in the textbooks and situations of Paiwan cul-
ture. Can we include the unique learning style of the indigenous
students (particularly Paiwan students) in the design? How to
combine them? What about effectiveness after implementation?
Such questions will rely on further study to enhance the sig-
nificance of culture-based instruction. In addition, regarding
implementation of instruction, Wei used more questions of cul-
tural situations, whereas Ling used questions of cultural situa-
tions, common situations, and pure calculation. How do we
distribute different types of questions in order to enhance in-
digenous students’ mathematics learning? Are there different
kinds of implementation for different types of questions (for
instance, questions of cultural situations are based on group
discussion)? These issues require further study. Finally, upon
the findings in this study, future researchers can treat students
of other indigenous tribes in Taiwan as the subjects to probe
into the design and implementation of culture-based instruction
or determine the effects of mathematics instruction upon inter-
actions and empowerment on indigenous students’ mathematics
learning in order to develop mathematics learning model suit-
able for indigenous students’ in Taiwan and accomplish educa-
tional equity.
REFERENCES
Anderson, C. W. (2003). How can schools support teaching for under-
standing in mathematics and science? In G. Adam et al. (Eds.),
Transforming: How schools and districts can support change (pp.
1-21). New York: Teacher College.
Artzt, A., & Armour-Thomas, E. (2002). Becoming a reflective mathe-
matics teacher: A guide for observations and self-assessment. Mah-
wah, NJ: Lawrence Erlbaum.
Barnes, M. B., & Barnes, L. W. (2005). Using inquiry processes to
investigate knowledge, skills, and perceptions of diverse learners: An
approach to working with prospective and current science teachers.
In A. J. Rodriguez, & R. S. Kitchen (Eds.), Preparing mathematics
and science teachers for diverse classrooms: Promising strategies
for transformative pedagogy (pp. 61-86). Mahwah, NJ: Laurence
Erlbaum.
Barton, B. (2009). The language of mathematics: Telling mathematical
tales. New York: Springer.
Bishop, A. J. (1988). Mathematics education in its cultural context.
Educational Studies in Mathematics, 19, 179-191.
http://dx.doi.org/10.1007/BF00751231
Boaler, J., & Staples, M. (2008). Creating mathematical futures through
an equitable teaching approach: The case of Railside school. Teacher
College Record, 110, 608-645.
Chi, H. Y. (2001). The Ethnography of a Mathematics Classroom in an
Atayal Native Elementary School. Unpublished Doctoral Dissertation,
Taipei: National Taiwan Normal University.
Chien, S. C. (1998). Cultural features and math learning: A case of
Yami people in the orchid island. Journal of National Taitung
Teachers College, 9, 283-306.
Cummins, J. (1986). Empowering minority students: A framework for
intervention. Harva rd Educational R eview, 56, 18-36.
D’Ambrosio, U. (1985). Ethnomathematics and its place in the history
and pedagogy of mathematics. For the Learning of Mathematics, 5,
44-48.
Ensign, J. (2005). Helping teachers use students’ home cultures in
mathematics lessons: Developmental stages of becoming effective
teachers of diverse students. In A. J. Rodriguez, & R. S. Kitchen
(Eds.), Preparing mathematics and Science Teachers for diverse
classrooms: Promising strategies for transformative pedagogy (pp.
225-242). Mahwah, NJ: Laurence Erlbaum.
Ernest, P. (1998). A postmodern perspective on research in mathemat-
ics education. In A. Sierpinska, & J. Kilpatrick (Ed.), Mathematics
education as a research domain: A search for identify (pp. 71-85).
Dordrecht: Kluwer Academic.
Fauvel, J., & Van Maanen, J. (2000). History in mathematical educa-
tion: The ICMI study . Dordrecht: Kluwer Academic.
Feldman, A. (2003). Mathematics instruction: Cognitive, affective, and
existential perspectives. In J. M. Royer (Ed.), Mathematical cogni-
tion. Greenwich, CT: Information Age.
Fuson, K. C. et al. (2000). Blending the best of the twentieth century to
achieve a mathematics equity pedagogy in the twenty-first century.
In M. J. Burke, & F. R. Curcio (Eds.), Learning mathematics for a
new century (pp. 197-212). Reston, VA: NCTM.
Gerdes, P. (1996). Ethnomathematics and mathematics education. In A.
J. Bishop, et al. (Eds.), International handbook of mathematics edu-
cation (pp. 909-943). Dordrecht: Kluwer Academic.
http://dx.doi.org/10.1007/978-94-009-1465-0_28
Gutstein, E. (2003). Teaching and learning mathematics for social
justice in an urban Latino school. Journal for Research in Mathe-
matics Education, 34, 37-73. http://dx.doi.org/10.2307/30034699
Henningsen, M., & Stein, M. K. (1997). Mathematical tasks and student
cognition: Classroom-based factors that support and inhabit high-
level mathematical thinking and reasoning. Journal for Research in
Mathematics Education, 2 8 , 524-549.
http://dx.doi.org/10.2307/749690
Hiebert, J., & Grouws, D. A. (2007). The effects of classroom mathe-
matics teaching on students’ learning. In F. K. Lester Jr. (Ed.), Sec-
ond handbook of research on mathematics teaching and learning (pp.
371-404). Charlotte, NC: Information Age.
Hsu, W. M., & Yang, Y. C. (2009). What factors influence aboriginal
students’ mathematics learning: An action research in a tribal ele-
mentary school of Pingtung County. Journal of National Taichung
University: Education, 23, 129-152.
Hsu, W. M. (2011). A case study: How did an experienced teacher
implement mathematics curriculum in her classroom? Chinese Jour-
nal of Science Education, 19, 101-122.
Huang, C. H. (2006). Investigating the mathematical teaching module
of Taiwanese Tayal junior high students: The dialectic and practice
of activity theory. Unpublished Doctoral Dissertation, Taipei: Na-
tional Taiwan Normal University.
Huang, C. W. (2002). Impact of multiple cultures on mathematics cur-
riculum: Deprivation and return of ethno-mathematics. The Educator
Monthly, 415, 38-41.
Hudson, P., & Miller, S. P. (2006). Designing and implementing
mathematics instruction for students with diverse learning need.
Boston, MA: Allyn and Bacon.
Leonard, J., & Dantley, S. J. (2005). Breaking through the ice: Dealing
with issues of diversity in mathematic and science education course.
In A. J. Rodriguez, & R. S. Kitchen (Eds.), Preparing mathematics
and science teachers for diverse classrooms: Promising strategies
for transformative pedagogy (pp. 87-117). Mahwah, NJ: Laurence
Erlbaum.
Li, H. C. (2006). An exploration of models of mathematics academic
achievement of different ethnical schoolchildren in Taitung County.
Taiwan Journal of Sociology of E du cation, 6, 1-41.
Lloyd, G. (2008). Curriculum use while learning to teach: One student
teacher’s appropriation of mathematics curriculum materials. Journal
for Research in Mathematics Education, 39, 63-94.
Ministry of Education (2003). The learning domain of mathematics of
grade 1-9 curriculum guidelines. Taipei: Ministry of Education in
Taiwan.
National Council of Teacher of Mathematics (2000). Principles and
standards for scho ol mathematics. Reston, VA: NCTM.
Rodriguez, A. J. (2005). Teachers’ resistance to ideological and peda-
gogical change: In A. J. Rodriguez, & R. S. Kitchen (Eds.), Prepar-
ing mathematics and science teachers for diverse classrooms: Prom-

W.-M. HSU ET AL.
672
ising strategies for transformative pedagogy (pp. 1-16). Mahwah, NJ:
Lawrence Erlbaum.
Stein, M., Remillard, J., & Smith M. (2007). How curriculum influ-
ences student learning. In F. K. Lester Jr. (Ed.), Second handbook of
research on mathematics teaching and learning (pp. 319-369).
Gweenwich, CT: Information Age.
Stuart, C., & Thrulow, D. (2000). Making it their own: Preservice
teachers’ experiences, beliefs, and classroom practices. Journal of
Teacher Education, 51, 113-121.
http://dx.doi.org/10.1177/002248710005100205
Tan, G. D., & Lin, M. F. (2002). Traits of learning styles of aboriginal
children: A study on Atayal children in Hualien County. Bulletin of
Educational Resear c h , 48, 233-261.
White, P., & Mitchelmore, M. C. (2010). Teaching for Abstraction: A
Model. Mathematical T hi n k in g an d Learning, 12, 205-226.
http://dx.doi.org/10.1080/10986061003717476
Wiske, M. S., Franz, K. R., & Breit, L. (2005). Teaching for under-
standing with technolog y. San Francisco, CA: Jossey-Bass.