Advances in Historical Studies
2013. Vol.2, No.3, 131-139
Published Online September 2013 in SciRes (http://www.scirp.org/journal/ahs) http://dx.doi.org/10.4236/ahs.2013.23017
Over and Undershot Waterwheels in the 18th Century.
Science-Technology Controversy
Danilo Capecchi
Dipartimento di Ingegneria Strutturale e Geotecnica, Università La Sapienza, Rome, Italy
Received May 26th, 2013; revised July 1st, 2013; accepted July 10th, 2013
Copyright © 2013 Danilo Capecchi. This is an open access article distributed under the Creative Commons At-
tribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the
original work is properly cited.
The present paper concerns the development of theory and experiments on water wheels in the 18th cen-
tury. At that time, as a result of a growing demand for energy, a better understanding of the functioning of
watermills, even at the theoretical level, was required in order to improve their efficiency. A hint about
the evolution of the theory of wheels in the 19th century is reported also. We have tried to clarify the role
played by some protagonists as Antoine Parent, Jean-Charles de Borda and John Smeaton. Their role has
not been fully recognised even in contemporary studies. Then some considerations are developed on the
relationships between science and technology on this particular subject, concluding that it was a happy
and well-balanced marriage.
Keywords: Waterwheels; Hydraulic Machine; Science-Technology; History of Hydraulics
Introduction
Hydraulic machines can be of different kinds; for a synthetic
description see (Singer, 1957; Strock & Teague, 1952; Card-
well, 1965; Syson, 1980). In the present paper reference is
made to waterwheels only, for their widespread diffusion and
since their development allows for an understanding of the
science-technology relationship. Mainly two types of water-
wheels exist: the undershot waterwheels and the overshot wa-
terwheels, whose difference is shown in Figure 1. There is also
another interesting wheel, intermediate between the two, the
breast wheel, in which water enters from the mid points—or
breast—of the wheel.
In the undershot machines, water flows beneath the wheel
and hits blades or paddles evenly diffused around the periphery
of the wheel. They are moved by the impulsion of the particles
of water. In the overshot machines water is led above the wheel.
Instead of blades, there are often buckets which get filled with
water and move the wheel by means of the water gravity itself.
The waterwheel steadily evolved since its introduction 2000
years ago, to pump water and mill grain. It is not clear where it
had its origin; it is clear however that it rapidly spread out as
described by Roman, Greek and Chinese sources. There is evi-
dence that the familiar vertical waterwheel developed within
the Roman Empire and rapidly spread out; the undershot wa-
terwheel was more common (Denny, 2003). Overshot wheels
required a large head (2 - 10 m), therefore they were usually
confined to hilly areas, or required extensive and expensive
auxiliary constructions. On the other hand, undershot wheels,
could operate with a small head (0.5 - 2 m), hence they could
be located on small streams in flat areas, near to population
centres. It is widely considered that the most dramatic industrial
consequences of waterwheels occurred in the Middle Ages,
when the scale of milling considerably increased with the de-
velopment of large towns. Their considerable economic and
social impact may be judged by the increased application of
waterwheels. From grinding wheat and pumping water in an-
tiquity, water powered mills evolved to forge iron, full cloth,
saw wood and stone, and for metalworking and leather tanning
(Denny, 2003: p. 194).
In the 18th century the waterwheels received new attention
because of the rising of the manufacturing industry and its in-
creasing request of energy. As soon as all the places suitable to
install wheels run out, the only way to increase energy was to
increase the efficiency, which called for an intervention of sci-
entists and technicians. The problem of efficiency of water-
wheels and their history in the 18th century has been the object
of rather recent studies (Reynold, 1979; Cardwell, 1965, 1967),
which also make general considerations on the role of the hy-
draulic energy in society. However, it must be said that in some
cases these studies present some errors when interpreting the
precise contribution of scientists. For example Parent is accused
of committing faults he is actually not guilty of while de Borda
is given undeserved merits.
The purpose of the present paper is to clarify these misun-
derstandings and also to present some reflections on the inter-
action between science and technology in this particular field,
in the 18th century. The first point has been developed by con-
sidering the contributions on theory and practice of water-
wheels published in the scientific journals of the time, mainly
the Mémories de l’Académie des science de Paris and the Phi-
losophical transactions. A great attention was paid to explain
the writings of the 18th century authors without judging them on
the basis of the modern standards of mechanics and hydraulics.
For the sake of simplicity, some concepts of mechanics of the
18th century were given for granted hoping the presentation to
be comprehensible enough for a contemporary reader. The

D. CAPECCHI
132
water stream
a)
(a)
water stream
b)
(b)
Figure 1.
Examples of undershot wheel (a) and
overshot wheel (b).
second point concerning the relationship between science and
technology is also based on the content of the mentioned scien-
tific journals. However, the conclusion drawn in such a case are
not comprehensive and a more targeted study would be neces-
sary. This notwithstanding some indications can be drawn.
Science and Technology
At the beginning of the 18th century an increasing need of
energy was felt by the emerging factories, especially in England.
Before the introduction of steam the only way to get energy
from nature was by means of the motion of water and air. Wa-
terwheels were suitable machines to collect energy form the
rivers. However the available streams of water were limited and
an increase of energy could come only by improving the effi-
ciency of the hydraulic machines.
Both scientists and engineers devoted themselves to the task.
Hydrodynamics at the beginning of the century was scarcely
developed. Only the theoretical results reported by Newton in
the second book of his masterpiece, Philosophiae naturalis
principia mathematica (Newton, 1972) and by Torricelli in his
Opera geometrica (Torricelli, 1644: p. 265) and the experi-
mental analysis of Edme Mariotte in his Traité des eaux et
autres corps fluides (Mariotte, 1686) were of some help. In this
situation scientists could consider very simplified models only.
On the other side engineers, or at least some of them, were no
longer practical men; they knew hydraulic quite enough and,
mainly, had a scientific attitude versus experiments which were
carried out using models of reduced size and accurate meas-
urements. There were thus elements for science and technique
to cooperate.
Scientists were the first to be involved. But the results they
found were useless from a practical point of view because they
were far from the actual findings. For this reason the develop-
ment of the hydraulic machines in the whole 18th century was
greatly influenced by engineers that experimented different
kinds of wheels, in particular overshot wheels and wheels with
curved blades.
Difference between theory and practice was hardly accepted
by the scientific community, thus the need to interpret the ex-
perimental results was pressing. But it took nearly a century
from the first theoretical analysis, that of Parent in 1704 (see
below), to reach a satisfactory interpretation of the hydraulic
phenomena and to suggest a way to build more efficient ma-
chines thanks to the studies of French engineers, especially
Poncelet (Poncelet, 1825, 1827).
Theoretical Studies
The first attempts to measure the efficiency of waterwheels
were carried out by scientists and contributed to the develop-
ment of hydrodynamics. For example, Edme Mariotte measured
the force of water stream by means of a counterbalancing
weight, drawing the conclusion that the force varies as the
square of the velocity of impact (Mariotte, 1686: p. 205); a re-
sult which was already provided by Newton on theoretical basis
(Newton, 1726: Part II, Theorem 27). A sophisticated analysis
on the undershot wheels was soon given by Antoine Parent in
1704 (Parent 1745: pp. 116-123, 323-338).
Antoine Parent’s Analysis of Undershot Wheels
In his memoir Sur la plus grande perfection possible des
machines (Parent 1745) Parent considered the idealized system
of wheels of Figure 2, deprived of any friction. A fluid (water)
flows through a channel from left to right; it spins the large
wheel CBD (Figure 2) as a consequence of the force exerted by
the fluid in B. The rotatory motion of FGH is transmitted by
means of teeth to the small wheel HMR, around whose axis a
rope wraps and lifts a weight. The fluid flows through the chan-
nel EB with uniform velocity V; the larger wheel CBD rotates
with constant angular velocity and the velocity at point B of the
immersed blade is equal to x, so that the relative velocity of the
water with respect to the blade is V-x. Parent wanted to calcu-
late the weight p that the smaller wheel HMR is able to lift with
velocity u.
He made the following assumptions: (a) the force exerted by
the water flow on the blade in B is proportional to the square of
the relative velocity between the blade and the water; (b) the
Galilean principle of moments (the principle of virtual veloci-
ties) can be applied—as the friction is negligible—assuming
that a steady state is reached in which the forces are balanced.
There were also some assumptions not made explicit, although
fundamental; (c) only one blade at a time is considered im-
mersed in water; (d) the stream is considered to be perpendicu-
lar to the blade. Parent indicated with P the force exerted by the
fluid in B with the blade at rest (then with a relative speed of
the fluid equal to the absolute velocity V); and called natural
effect of the fluid (effect naturelle) the product PV (Parent 1745:
p. 326). The product pu of the weight p lifted by its velocity u
is the (general) effect of the fluid. Assume with Parent: B = AB,
b = AH; C = LH; c = LI, Q the weight p necessary to equili-
brate P1.
The relation between P and Q is given by the principle of
virtual velocities as Q = αP; where α = BC/bc measures the
ratio of the virtual displacements of the blade B (horizontal) and
the weight p = Q (vertical). When the wheel CBD rotates,
1Note that in his calculations Parent used the same symbol P to indicate both
the suspended weight which equilibrates the wheel and the force exerted on
the blade. We prefer to differentiate the symbols, retaining the symbol P for
the force exerted on the blade and indicating the suspended equilibrating
weight as Q.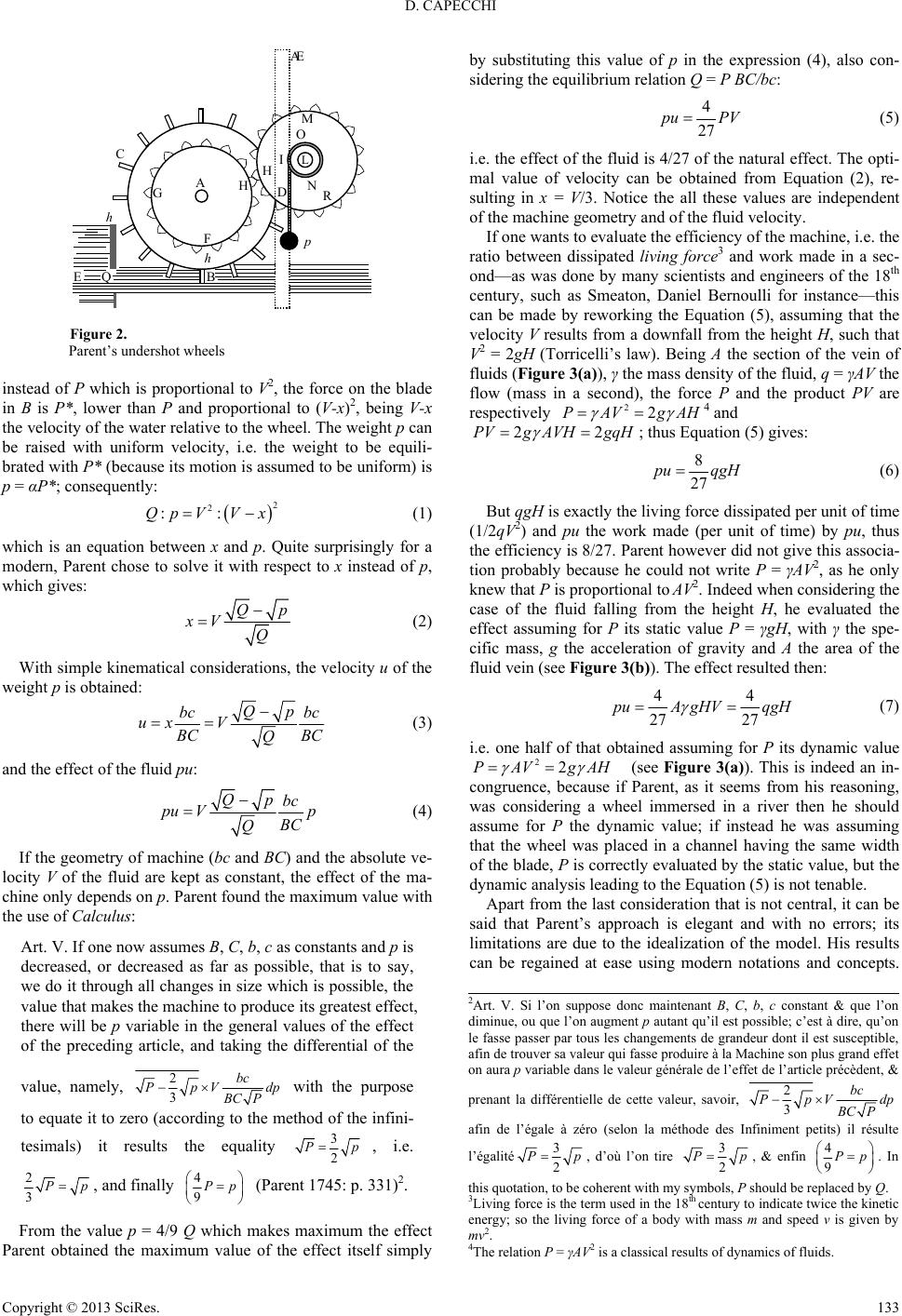

D. CAPECCHI
C
h
h
EQ
p
R
N
L
HI
H
A
E
O
M
D
A
B
G
F
Figure 2.
Parent’s undershot wheels
instead of P which is proportional to V2, the force on the blade
in B is P*, lower than P and proportional to (V-x)2, being V-x
the velocity of the water relative to the wheel. The weight p can
be raised with uniform velocity, i.e. the weight to be equili-
brated with P* (because its motion is assumed to be uniform) is
p = αP*; consequently:

2
2
::Qp VVx (1)
which is an equation between x and p. Quite surprisingly for a
modern, Parent chose to solve it with respect to x instead of p,
which gives:
Qp
xV Q

 (2)
With simple kinematical considerations, the velocity u of the
weight p is obtained:
Qp bc bc
ux V
B
CBC Q

 (3)
and the effect of the fluid pu:
Qp bc
pu Vp
B
C
Q

 (4)
If the geometry of machine (bc and BC) and the absolute ve-
locity V of the fluid are kept as constant, the effect of the ma-
chine only depends on p. Parent found the maximum value with
the use of Calculus:
Art. V. If one now assumes B, C, b, c as constants and p is
decreased, or decreased as far as possible, that is to say,
we do it through all changes in size which is possible, the
value that makes the machine to produce its greatest effect,
there will be p variable in the general values of the effect
of the preceding article, and taking the differential of the
value, namely, 2
3
bc
PpV dp
BC P
 with the purpose
to equate it to zero (according to the method of the infini-
tesimals) it results the equality 3
2
Pp, i.e.
2
3Pp, and finally 4
9Pp




(Parent 1745: p. 331)2.
From the value p = 4/9 Q which makes maximum the effect
Parent obtained the maximum value of the effect itself simply
by substituting this value of p in the expression (4), also con-
sidering the equilibrium relation Q = P BC/bc:
4
27
pu PV (5)
i.e. the effect of the fluid is 4/27 of the natural effect. The opti-
mal value of velocity can be obtained from Equation (2), re-
sulting in x = V/3. Notice the all these values are independent
of the machine geometry and of the fluid velocity.
If one wants to evaluate the efficiency of the machine, i.e. the
ratio between dissipated living force3 and work made in a sec-
ond—as was done by many scientists and engineers of the 18th
century, such as Smeaton, Daniel Bernoulli for instance—this
can be made by reworking the Equation (5), assuming that the
velocity V results from a downfall from the height H, such that
V2 = 2gH (Torricelli’s law). Being A the section of the vein of
fluids (Figure 3(a)), γ the mass density of the fluid, q = γAV the
flow (mass in a second), the force P and the product PV are
respectively 22PAV gAH
  4 and
22PVg AVHgqH


; thus Equation (5) gives:
8
27
pu qgH (6)
But qgH is exactly the living force dissipated per unit of time
(1/2qV2) and pu the work made (per unit of time) by pu, thus
the efficiency is 8/27. Parent however did not give this associa-
tion probably because he could not write P = γAV2, as he only
knew that P is proportional to AV2. Indeed when considering the
case of the fluid falling from the height H, he evaluated the
effect assuming for P its static value P = γgH, with γ the spe-
cific mass, g the acceleration of gravity and A the area of the
fluid vein (see Figure 3(b)). The effect resulted then:
44
27 27
puA gHVqgH  
(7)
i.e. one half of that obtained assuming for P its dynamic value
22PAV gAH
  (see Figure 3(a)). This is indeed an in-
congruence, because if Parent, as it seems from his reasoning,
was considering a wheel immersed in a river then he should
assume for P the dynamic value; if instead he was assuming
that the wheel was placed in a channel having the same width
of the blade, P is correctly evaluated by the static value, but the
dynamic analysis leading to the Equation (5) is not tenable.
Apart from the last consideration that is not central, it can be
said that Parent’s approach is elegant and with no errors; its
limitations are due to the idealization of the model. His results
can be regained at ease using modern notations and concepts.
2Art. V. Si l’on suppose donc maintenant B, C, b, c constant & que l’on
diminue, ou que l’on augment pautant qu’il est possible; c’est à dire, qu’on
le fasse passer par tous les changements de grandeur dont il est susceptible,
afin de trouver sa valeur qui fasse produire à la Machine son plus grand effet
on aura p variable dans le valeur générale de l’effet de l’article précèdent, &
prenant la différentielle de cette valeur, savoir, 2
3
bc
PpV dp
BC P

afin de l’égale à zéro (selon la méthode des Infiniment petits) il résulte
l’égalité3
2
Pp, d’où l’on tire 3
2
Pp, & enfin 4
9Pp




. In
this quotation, to be coherent with my symbols, P should be replaced by Q.
3Living force is the term used in the 18th century to indicate twice the kinetic
energy; so the living force of a body with mass m and speed vis given by
mv2.
4The relation P = γAV2 is a classical results of dynamics of fluids.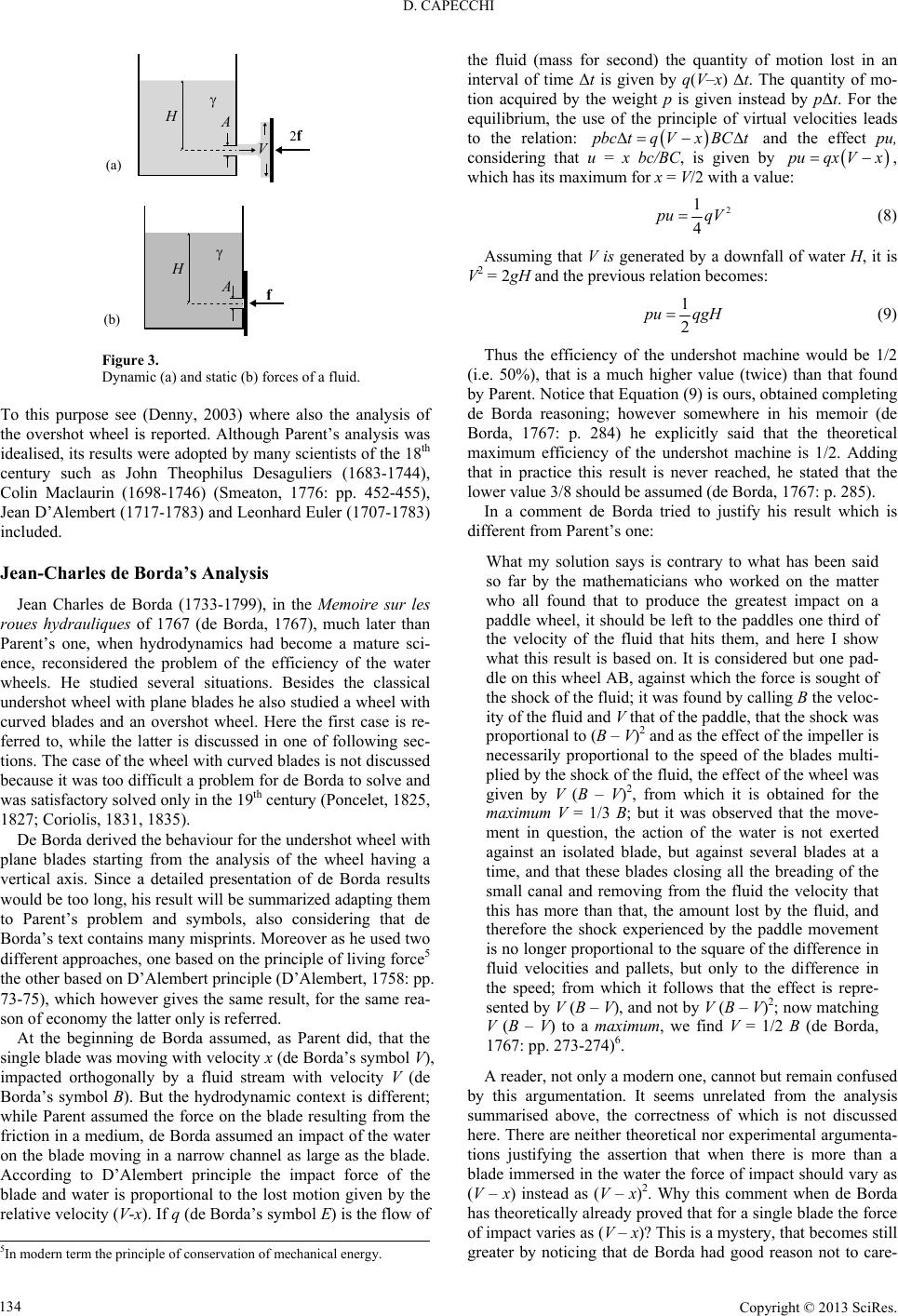

D. CAPECCHI
134
HA
2f
V

a)
(a)
b)
H
Af

(b)
Figure 3.
Dynamic (a) and static (b) forces of a fluid.
To this purpose see (Denny, 2003) where also the analysis of
the overshot wheel is reported. Although Parent’s analysis was
idealised, its results were adopted by many scientists of the 18th
century such as John Theophilus Desaguliers (1683-1744),
Colin Maclaurin (1698-1746) (Smeaton, 1776: pp. 452-455),
Jean D’Alembert (1717-1783) and Leonhard Euler (1707-1783)
included.
Jean-Charles de Borda ’s A nal ysi s
Jean Charles de Borda (1733-1799), in the Memoire sur les
roues hydrauliques of 1767 (de Borda, 1767), much later than
Parent’s one, when hydrodynamics had become a mature sci-
ence, reconsidered the problem of the efficiency of the water
wheels. He studied several situations. Besides the classical
undershot wheel with plane blades he also studied a wheel with
curved blades and an overshot wheel. Here the first case is re-
ferred to, while the latter is discussed in one of following sec-
tions. The case of the wheel with curved blades is not discussed
because it was too difficult a problem for de Borda to solve and
was satisfactory solved only in the 19th century (Poncelet, 1825,
1827; Coriolis, 1831, 1835).
De Borda derived the behaviour for the undershot wheel with
plane blades starting from the analysis of the wheel having a
vertical axis. Since a detailed presentation of de Borda results
would be too long, his result will be summarized adapting them
to Parent’s problem and symbols, also considering that de
Borda’s text contains many misprints. Moreover as he used two
different approaches, one based on the principle of living force5
the other based on D’Alembert principle (D’Alembert, 1758: pp.
73-75), which however gives the same result, for the same rea-
son of economy the latter only is referred.
At the beginning de Borda assumed, as Parent did, that the
single blade was moving with velocity x (de Borda’s symbol V),
impacted orthogonally by a fluid stream with velocity V (de
Borda’s symbol B). But the hydrodynamic context is different;
while Parent assumed the force on the blade resulting from the
friction in a medium, de Borda assumed an impact of the water
on the blade moving in a narrow channel as large as the blade.
According to D’Alembert principle the impact force of the
blade and water is proportional to the lost motion given by the
relative velocity (V-x). If q (de Borda’s symbol E) is the flow of
the fluid (mass for second) the quantity of motion lost in an
interval of time Δt is given by q(V–x) Δt. The quantity of mo-
tion acquired by the weight p is given instead by pΔt. For the
equilibrium, the use of the principle of virtual velocities leads
to the relation:


pbctq VxBCt

 
and the effect pu,
considering that u = x bc/BC, is given by


puqx Vx

,
which has its maximum for x = V/2 with a value:
2
1
4
pu qV (8)
Assuming that V is generated by a downfall of water H, it is
V2 = 2gH and the previous relation becomes:
1
2
pu qgH (9)
Thus the efficiency of the undershot machine would be 1/2
(i.e. 50%), that is a much higher value (twice) than that found
by Parent. Notice that Equation (9) is ours, obtained completing
de Borda reasoning; however somewhere in his memoir (de
Borda, 1767: p. 284) he explicitly said that the theoretical
maximum efficiency of the undershot machine is 1/2. Adding
that in practice this result is never reached, he stated that the
lower value 3/8 should be assumed (de Borda, 1767: p. 285).
In a comment de Borda tried to justify his result which is
different from Parent’s one:
What my solution says is contrary to what has been said
so far by the mathematicians who worked on the matter
who all found that to produce the greatest impact on a
paddle wheel, it should be left to the paddles one third of
the velocity of the fluid that hits them, and here I show
what this result is based on. It is considered but one pad-
dle on this wheel AB, against which the force is sought of
the shock of the fluid; it was found by calling B the veloc-
ity of the fluid and V that of the paddle, that the shock was
proportional to (B – V)2 and as the effect of the impeller is
necessarily proportional to the speed of the blades multi-
plied by the shock of the fluid, the effect of the wheel was
given by V (B – V)2, from which it is obtained for the
maximum V = 1/3 B; but it was observed that the move-
ment in question, the action of the water is not exerted
against an isolated blade, but against several blades at a
time, and that these blades closing all the breading of the
small canal and removing from the fluid the velocity that
this has more than that, the amount lost by the fluid, and
therefore the shock experienced by the paddle movement
is no longer proportional to the square of the difference in
fluid velocities and pallets, but only to the difference in
the speed; from which it follows that the effect is repre-
sented by V (B – V), and not by V (B – V)2; now matching
V (B – V) to a maximum, we find V = 1/2 B (de Borda,
1767: pp. 273-274)6.
A reader, not only a modern one, cannot but remain confused
by this argumentation. It seems unrelated from the analysis
summarised above, the correctness of which is not discussed
here. There are neither theoretical nor experimental argumenta-
tions justifying the assertion that when there is more than a
blade immersed in the water the force of impact should vary as
(V – x) instead as (V – x)2. Why this comment when de Borda
has theoretically already proved that for a single blade the force
of impact varies as (V – x)? This is a mystery, that becomes still
greater by noticing that de Borda had good reason not to care-
5In modern term the principle of conservation of mechanical energy.

D. CAPECCHI
fully scrutinise and accept his theory, as it was in good agree-
ment with experience, at least as far as efficiency and velocity
of the wheel are concerned (see Smeaton’s comments, hereafter).
In the secondary literature it is sometimes argued that Parent
made calculation errors, and the true efficiency of the undershot
wheel is 8/24 instead of 4/27 (Cardwell, 1967; Reynolds, 1979).
De Borda would instead have found exact results if he had cor-
rected the error due to the approximation in considering a wheel
at the time and a factor two which Parent had neglected:
In 1767, Borda published a short paper correcting the two
main errors of Parent and harmonising theory with expe-
riment (Cardwell, 1967: p. 212).
Parent’s theory is actually correct if properly intended. The
difference with de Borda’s one depends on the different hy-
draulic context assumed by the two scientists.
Empirical Investigations
John Smeaton Investigations on Undershot Wheels
The first systematic experiments on waterwheels were pro-
bably those of the English engineer John Smeaton (1724-1792)
who in 1759 published An experimental enquiry concerning the
natural powers of water and wind to turn mills, and other ma-
chines, depending on a circular motion, before de Borda’s me-
moir. He compared under and overshot wheels.
Smeaton’s attention to water wheels was due to the demand
of English industry for an improvement of the efficiency of
existing water wheels. Being not convinced of Parent’s results
he performed numerous experiments on the model shown in
Figure 4, where ABCD is a reservoir which collects water for
recirculation after its action on the waterwheel. Water is
pumped out of the waterwheel via a hand pump (MN is the
handle of the pump, L the pump rod) to another reservoir DE.
The water in DE was maintained at a constant level by observ-
ing the graduated rod FG, while the water released on the wheel
was controlled by the rod HI. A rope connected to the axle of
the wheel in O and led through the pulleys P and Q raised a pan
of weights, R, used for measuring the wheel’s output. The ap-
paratus could be adapted to test overshot wheels as shown by
the dotted line in the cross-sectional view.
Smeaton defined the original power of the water as the
product between the quantity of water expounded in a given
time and the height that water comes down from. The effect of
the machine is the sum of the weight raised by the action of this
water and the weight necessary to overcome the friction, multi-
plied by the height the weight will be raised to in a given time.
The efficiency is the ratio between effect and power (Smeaton,
1759: p. 106-107). In one of his experiments where the power
was 3970 pounds × inches in a minute (the product of the flow
of 264.7 lb of water multiplied by the height of fall of 15
inches), by varying the raised weight, he found that the maxi-
mum effect corresponded to 1266 pounds × inches in a minute
(the product of a weight of 9.375 lb raised to and height of 135
inches), for an efficiency of 1266/3970 = 32%, greater than that
provided by Parent (25%) but lower than that provided by de
Borda (50%). The ratio between the velocity of the blades of
the wheel and the velocity of water was often greater than that
foreseen by Parent, arriving in some cases close to 1/2 instead
of 1/3. Also the weight raised was much greater, (3/4) instead
of 4/9 of the equilibrating weight (Smeaton, 1759: p. 115).
Smeaton justified the difference between theory and experiment
as a consequence of different assumptions:
It must be remembered, therefore, that, in the present case,
the wheel was not placed in an open river, where the
natural current, after it has communicated its impulse to
the float, has room on all fides to escape, as the theory
supposes; but in a conduit or rate, to which the float being
adapted, the water cannot otherwise escape than by mov-
ing along with the wheel. It is observable, that a wheel
working in this manner, as soon as the water meets the
float, receiving a sudden check, it rises up against the float,
like a wave against a fixed object; insomuch that when the
sheet of water is not a quarter of an inch thick before it
meets the float, yet this sheet will act upon the whole sur-
face of a float, whose height is 3 inches; and consequently
was the float no higher than the thickness of the sheet of
water, as the theory also supposes, a great part of the force
would have been 10 ft, by the water’s dashing over the
float (Smeaton, 1759: pp. 113-114).
Figure 4.
Smeaton’s experimental set (Smeaton, 1756: p. 102).
6Ce que ma solution vient de me donner, est contraire à ce qu’ont dit jusqu’à
présent les Géomètres qui ont travaillé fur cette matière en effet, tous ont
trouvé, que pour faire produire à une roue à palettes le plus grand effet
p
ossible, il ne fallait laisser prendre aux palettes que le tiers de la vitesse du
fluide qui les frappait, & voici sur quoi ce résultat était fondé. On ne
considérait dans cette roue qu’une seule palette A B, contre laquelle en
cherchait la force du choc du fluide; on trouvait, en appelant B la vitesse du
fluide & V celle des palettes, que le choc était proportionnel à (B – V)2 &
comme l’effet de la roue est nécessairement proportionnel à la vitesse des
p
alettes multipliée par le choc du fluide, on avait l’effet de la roue représenté
par V (B – V)2 d’où on tirait pour le maximum V = 1/3 B; mais il fallait
observer que dans le mouvement dont il s’agit, l’action de l’eau ne s’exerce
p
as contre une palette isolée, mais contre plusieurs palettes à la fois, & que
ces palettes fermant tout le panage du petit canal & ôtant au fluide la vitesse
qu’il a de plus qu’elles, la quantité du mouvement perdu par ce fluide, & par
conséquent le choc qu’éprouvent les palettes, n’est plus proportionnel au
carré de la différence des vitesses des fluides & des palettes, mais seulement
à la différence de ces vitesses d’où il suit que l’effet est représenté par V (B
–
V) , & non pas par V (B – V)2; or égalant V (B – V) à un maximum, on
trouve V = ½B.

D. CAPECCHI
136
In a subsequent paper Smeaton reassumed his experimental
results, rhetorically exaggerating the difference between ex-
perimental and theoretical findings, asserting also that for large
wheel (as the wheels of actual mills), the efficiency is greater
arriving up to 50%:
I have found be the commonly received doctrine among
theoretical mechanics [...] that, where the velocity of wa-
ter is double, the adjutage or aperture of the sluice re-
maining the same the effect is eight time; that is not as the
square but as the cube of the velocity [...].
For if that conclusion were true, only 4/27 of the water
expended could be raised back again to the height of the
reservoir from which it had descended, exclusively of all
kinds of friction, &c. which would make the actual quan-
tity raised back again still less; that is, less than one-sev-
enth of the whole; whereas it appears from Table I of the
said volume (Smeaton, 1759), that in some of the experi-
ments here related, even upon the small scale on which
they were tried, the work done was equivalent to the rais-
ing back again about one quarter of the water expended;
and in large works the effect is still greater, approaching
towards half, which seems to be the limit for the under-
shot mills, as the whole would be the limit for the overshot
mills [emphasis added][...].
The velocity also of the wheel, which according to M.
Parent’s determination, adopted by Desaguliers and Mac-
laurin, ought to be no more than one-third of that of the
water, varies at the maximum in the above mentioned ex-
periments of table, between one third and one half but in
all the cases there related, in which the most work is per-
formed in proportion to the water expended and which
approach the nearest to the circumstances of great works,
when properly executed the maximum lies much nearer to
one half than one third (Smeaton, 1776: pp. 456-457).
Antoine de Parcieux’s and John Smeaton’s
Experiments on Overshot Wheels
The overshot waterwheel received no attention by the scien-
tists probably because there was the spread opinion that they
had the same efficiency as the undershot ones (Reynolds, 1979:
p. 274). This was the opinion of Leonhard Euler also, who in
his work of 1754 denied that the overshot wheel had any ad-
vantage over the undershot ones (Euler, 1754: p. 198). Bernard
Forest de Belidor (Belidor, 1782: p. 286) referred that an un-
dershot wheel is six times more efficient that an overshot one,
while Desaguliers on the contrary affirmed that a “well-made
overshot mill” may be ten times more efficient than an under-
shot wheel (Desaguliers, 1744: p. 532).
The first known study on the subject was that of Antoine de
Parcieux (1703-1768) which is usually classified as an engineer
though member of the Académie des sciences de Paris. The
interest of de Parcieux derived by the desire of Madame de
Pompadour to have current water from the small river the
Blaise in Crésy, raising to a height of 50 meters. Because of the
small flow of the river, an undershot wheel would not have
been able to satisfy the request.
De Parcieux was brought to think that the efficiency of the
overshot wheels should be higher than that of the current un-
dershot wheels by assimilating the water, that descends and
works as an engine for the water that should be raised, to two
weights which are located on two opposite sides of a pulley and
are connecters by a rope.
I soon saw that I could get a much better use of water
weight, considering it as weights which falling raise oth-
ers: but how has one to take the wheel (de Parcieux, 1759:
p. 607)7.
He said to have made experiments with a pulley using as
power a weight of 96 ounces which raised weights of 24, 32, 40,
etc. ounces registering the amount these weights rise in one
second, i.e. the velocity in the first part of motion. The velocity
ranged from 85 inches per second for a weight of 24 ounces to
20 inches per second for a weight of 72 ounces (de Parcieux,
1754: p. 609).
On the basis of his results de Parcieux suggested a simple
experiment by imagining two waterwheels equal to each other
but with their buckets inclined in opposite directions. The
wheel receiving the falling water was able to raise water in the
other wheel under the condition that the raised water was less
than that falling one. And, in the same way as in a pulley, the
speed of the wheels will depend on the ratio between falling
and raising weight. In the limit, if the wheels rotate very slowly,
the amount of raised water will be equal to that fallen, and the
efficiency of the overshot waterwheel will reach 100%.
The explanation, on the greater efficiency of wheels that ro-
tate slowly actually has no weight. The experiment of the pul-
ley is of course truthful, but here accelerated motions are con-
cerned. In the case of the waterwheel there is instead a station-
ary motion. In this situation it can be shown that the velocity of
the wheel, at least ideally, has no influence on the efficiency.
The greater efficiency actually registered for the overshot
wheels that rotate slowly depends on the construction methods
and operation. In (Denny 2003) the reasons for the efficiency of
the overshot wheels to decrease with the increasing of speed are
illustrated.
With his apparatus, Smeaton was able to experiment an
overshot wheel with water flowing from the tape indicated with
fg in Fi gure 4. He found that using the same wheel with plane
blades, the efficiency was double than that of the undershot
wheels and confirmed the results obtained by de Parcieux, that
the efficiency of the wheel increased by slowing its speed.
Smeaton was convinced that most of the difference between
over and under wheels were due to the loss of living force of
the water in the latter case associated to its change in shape
during impact. He also proposed an (unsatisfactory) explanation
for the increase of efficiency of the overshot wheel by slowing
the speed of the wheel, assuming that at the higher speed the
efficiency of the water pressure was lower.
When the velocity is greater [water] does not press so
much upon the bucket as when it is less, the power of the
water to produce effects will be greater in the less velocity
than in the greater: and hence we are led to this general
rule, that, caeteris paribus, the less the velocity of the
wheel, the greater will be the effect thereof (Smeaton,
1759: p. 133).
7Je vis bientôt que je pouvais tirer un bien meilleur parti du poids de
l’eau, en la considérant comme des poids qui en descendant, en
enlèveraient d’autres: mais quelle vitesse falloir il faire prendre à la
roue.

D. CAPECCHI
In the subsequent years Smeaton performed many experi-
ments on the impact of non-elastic bodies assuming that the
loss of living force in the impact was due to a change of shape
of the bodies. The following quotations resumes Smeaton’s
ideas about the energy (modern term) required to change the
shape of a body.
To obviate this, those of the old opinion seriously set
about proving, that the bodies might change their figure,
without any loss of motion in either of the striking bodies.
[…]
On the other hand, if it can be shown that the figure of a
body can be changed, without a power, then, by the same
law, we might be able to make a forge hammer work upon
a mass of soft iron, without any other power than that
necessary to overcome the friction resistance, and original
vis inertiae, of the parts of the machine to be put in mo-
tion: for, as no progressive motion is given the mass of
iron by the hammer (it being supported by the anvil). no
power call be expended that way; and if none is lost to the
hammer from changing the figure of the iron, which is the
only effect produced, then the whole power must reside in
the hammer, and it would jump back again, to the place
from which it fell, just in the same manner as if it fell
upon a body perfectly elastic, upon which, if it did fall,
the case would really happen: the power, therefore to
work the hammer would be the same whether, it fell upon
an elastic or non-elastic body; an idea so very contrary to
all experience (Smeaton, 1782: pp. 342-343).
Thanks to Smeaton, at least in Great Britain, the overshot
wheels became dominant, and contrasted the success of steam
machines.
However much Mr. Smeaton’s valuable observations may
have been disregarded by authors, they have not been lost
to practical men... [As a result of his experiments] he de-
termined to apply the water, in all cases, so that it should
act more by its weight, and less by its impulse; and the
advantage gained by that improved construction was
found to be fully equal to his expectation. It was after-
wards so generally adopted and improved upon by him-
self and by other engineers in this country, that although
undershot water-wheels were, about fifty years ago, the
most prevalent, they are now rarely to be met with; and
wherever economy of power is an object, no new ones are
made (Reynold, 1979: p. 291).
In his Hydrodinamica of 1738 Daniel Bernoulli reinterpreted
Parent’s result calculating in 8/27 the theoretical efficiency
related to the living force of the flowing water before the im-
pact upon the blades of the wheel. Bernoulli concluded that the
small efficiency of the undershot wheels had to be ascribed to
the fact that part of the water living force is lost to keep still the
high speed of the water flowing after that the impact against the
paddles of the wheel has occurred (Bernoulli, 1738: p. 193).
Johann Albrecht Euler—a son of Leonhard—in a memoir sub-
mitted in 1754 for a prize competition, which he actually won,
analysed separately undershot, gravity and reaction wheels
(Euler, 1754). For the undershot wheel he found out Parent’s
result, i.e. an efficiency equal to 8/27 (Euler, 1754: p. 12). For
the gravity wheel (the overshot ones of Figure 5) Euler con-
cluded that if the buckets were large enough to collect all the
water of the stream and if the diameter of the wheel was equal
to the height of the fall, the efficiency of the gravity wheel
would be 100%.
Smeaton did not know Daniel Bernoulli’s work, neither
probably Johann Albrecht Euler’s. Equally, at least in 1759,
Smeaton did not know de Borda’s analysis of the overshot
wheels which, though correct, needed to be interpreted.
De Borda, considering a very idealized wheel whose buckets
did not leave water, drew the conclusion that an overshot wheel
as in Figure 6, where the stream of water MN is tangent to the
wheel, reaches its maximum effect when BH = 0 and the wheel
rotates with zero velocity, confirming that the efficiency of this
kind of wheel increases by lowering the speed of rotation. Ac-
tually de Borda was not precise. Indeed, after a substantially
correct analysis, he concluded that the effect of the overshot
wheel, using Parent’s symbols is given by (de Borda, 1770: p.
281):

2Hh pu qgh xgx
x







 (10)
with h = BH and H = BE, with reference to Figure 6. He cor-
rectly concluded that the maximum of the efficiency is reached
for h = 0; but incorrectly that x should be zero. Indeed for h = 0
the previous relation should be rewritten as:
H
puq gxqgH
x



 (11)
A
a
b
c
d
e
f
g
h
i
k
lm
M
RQ
S
L
K
I
H
GFE
D
OC
B
Figure 5.
Euler’s gravity wheel (Euler, 1754: Tab. II)
MB
A
HN
CB
X
E
O
P
Figure 6.
de Borda’s wheel (de Borda, 1767: p. 286).

D. CAPECCHI
138
which indicates an efficiency of 100% and the independence of
the velocity x of the wheel. However, at the end of his paper (de
Borda, 1770: p. 286), De Borda noticed that the efficiency is in
fact substantially independent of x, as its change with x is rather
small.
Further Development in the 19th Century
In the 19th century the development of the hydraulic ma-
chines was brought in the frame of applied mechanics, where
theory and practice were carried out by the same people, the
modern engineers, determining a great improvement in the
efficiency of all kind of machines. A prominent role was played
by the military engineers of the École de applications de l’Ar-
tillerie et du Génie, in particular Jean Victor Poncelet (1788-
1867) and Arthur Jules Morin (1795-1880).
These engineers were so much involved in mathematics and
physics as to consider themselves more scientists than practi-
tioners; for instance they addressed their memories to the
Académie des science instead to technological journals. They
made extensive use of experiments, but not as much as they
should to verify the effectiveness of the general mechanical
theories behind their designs. The experiments instead had two
main purposes. From the one hand they had to highlight some
minor defects of the machines to be corrected after a theoretical
review of the problem; on the other hand they should furnish
numerical values of selected correction factors which allowed
transition from theoretical to practical formulas. This was not
due to errors in the theory but to simplified assumptions. For
example very often the conservation of living forces—or the
work—was assumed and then no friction was taken into ac-
count; its effect was evaluated by performing experiments un-
der different operating conditions and arranging tables of cor-
rection factors.
After some preparatory work (Belhoste, 1994) Poncelet pre-
pared the Mémoire sur les roues verticales à palettes courbes
mues par en dessous, suivi d’expériences sur les effets mé-
caniques de ces roues concerning the undershot waterwheels. It
was presented before the Académie des science in 1824 and
published in the Annales de Chimie et Physique in 1825, with
minor revisions (Poncelet, 1825); an improved version was
published in 1827 (Poncelet, 1827). Poncelet’s purpose was to
satisfy Lazare Carnot (1753-1823) (and de Borda) requirements
for an efficient machine: avoiding loss of living force by impact
and by the release of water with significant speed. He reached
his goal by assuming curved and inclined blades as shown in
Figure 7; probably not a new idea, but a good idea that was not
pursued with the due firmness:
The idea to substitute curved blades to plane blades of the
old systems seemed so naturel and simple that one can
think that its arose to everyone; so I did not attribute a
great merit to it. But because the simplest ideas are often
those which found the most difficulties to be accepted, I
did not want limit myself to theoretical speculations (Pon-
celet: pp. 144-145)8.
With these devices the undershot wheels could reach, at least
theoretically, an efficiency of 100%. The water wheel as pro-
posed by Poncelet is now known as Poncelet wheel. Later stud-
ies and experiments highlighted some weakness of Poncelet
wheels (Belhoste, 1994), which however spread out and for a
long time were competing with the water turbine introduced by
Benoit Fourneyron (1802-1867) proposed to replace the wa-
terwheel around 1830 (Fourneyron 1840). Contrary to the com-
mon belief, turbines did not replace the waterwheels and their
design was only in the syllabus of engineering faculties at least
until 1940. They disappeared only after the Second World War.
Today new attention is paid to properly designed water-wheels,
both undershot and overshot, as an economical solution to get
energy from water streams with low head (Muller, 2004).
Conclusion
In the early 18th century, as a result of the growing demand
for energy, a better understanding of the functioning of the
waterwheels, even at the theoretical level, was required in order
to improve their efficiency. At the time there was a widespread
belief among scientists that it did not really matter which type
of wheel to study because the effectiveness would have to be
the same for all types of wheels, probably because at an intui-
tive level one could think that the living force (the kinetic en-
ergy) of water is communicated always in the same way.
Despite the theoretical knowledge of hydraulics was very
limited, in 1704 Antoine Parent could write a very interesting
work on the hydraulics of the undershot wheels that preserved
its value for most of the century. Parent’s result was not in a
perfect agreement with practice and some differences persisted
in comparison to experimental research. Smeaton work was for
sure of much interest. He finally pointed out what should have
been clear to everyone, that is that the overshot wheels could
have much higher efficiency, up to twice, than undershot, at
least as regards the wheels used in the 18th century.
Meanwhile hydrodynamics was developing thanks to the
theoretical work of Leonhard and Johann Euler and D’Alembert,
who had also made it possible theoretical investigation of the
operation of water wheels. But their theoretical work written on
the subject had a very limited impact on the technological de-
velopment of the wheels, mainly because they were not read by
engineers, in particular, they were not read by Smeaton and
were soon forgotten. Thus the legacy the experiments and the
theoretical speculations of the 18th century left to the 19th cen-
tury consists mainly of the two points concerning the optimi-
zation of the efficiency of water wheels:
a) the impact of the water upon the paddles of the wheel
should be avoided;
b) the wheel must move so that the water is unloaded with
the minimum possible speed.
These conclusions were collected by Lazare Carnot (Carnot
1786) who based his theory of machines on the conservation of
living force and impact for insensible degrees. The problem of
the efficiency of waterwheels was dealt with in a different
fashion in the 19th century by French engineers, such as Morin
and Poncelet. They clarified that the efficiency does not depend
on the kind of wheel (over or undershot) but on the way they
are designed.
If one had to summarize in a few words the role of science in
the technology of water-wheels, he would be attempted to say
that it was modest, almost none at all as claimed by Reynolds
8L’idée de substituer des palettes courbes aux palettes droites de l’ancien
système parait si naturelle et simple, qu’il y a lieu de croire qu’elle sera
venue à plus d’une personne; aussi n’ai-je pas la prétention de lui attribuer
un grand mérite; mais, comme les idées les plus simples son fort souvent
celles qui rencontrent le plus de difficultés à être admises, je n’ai pas voulu
m’en tenir à des aperçus purement théoriques.

D. CAPECCHI
Figure 7.
Poncelet wheel (Poncelet, 1827: Planche 1)
(1979) and Rupert Hall (1961). In our opinion there was instead
a fruitful interaction between science and technology. In fact,
the application of rational mechanics based on a high formal-
ization had a limited impact, which instead will have a pro-
found influence on the technology of the 19th century. On the
contrary, less formalized theoretical considerations had a deci-
sive role, despite their high degree of idealization, such as those
of Parent and de Borda. The hydrodynamical studies aimed at
assessing the pressure of fluids also had decisive importance.
Moreover, considering De Parcieux’s and Smeaton’s peers as
foreign to science, as was done by some historians asserting the
low influence of science on technology, is certainly debatable
and not shared by all. For example, Musson and Robinson (1969)
considered Smeaton’s contribution as an example of the direct
application of science to technology.
REFERENCES
Belhoste, B. (1994). La formation polytechnicienne: 1794-1994. Paris:
Dunod.
Belidor, B. F. (1782). Architecture hydraulique, ou l’art de conduire,
d’élever et de ménager les eaux pour les différens besoins de la vie
(1737-1739). Paris: L. Cellot.
Bernoulli, D. (1738). Hydrodynamica, sive de viribus et motibus flui-
dorum commentarii. Strasbourgh: Dulsseker J. R.
Cardwel, D. S. L. (1965). Power technologies and the advance of sci-
ence. Technology and Culture, 6, 188-207.
Cardwell, D. S. L. (1967). Some factors in the early development of the
concepts of power, work and energy. The British Journal for the
History of Science, 3, 209-224. doi:10.1017/S0007087400002661
Carnot, L. (1786). Essai sur les machines en général (1783). Dijon:
Defay.
Coriolis, G. (1831). Mémoire sur le principe des forces vives dans le
mouvements relatifs des machines. Journal École polytechnique, 21,
268-302.
Coriolis, G. (1835). Sur les équations du mouvement relatif des sys-
tèmes de corps. Journal de l’École Polytechnique, 24, 142-154.
D’Alembert, J. (1758). Traité de dynamique. Paris: David.
de Borda, J. C. (1770). Sur les roues hydrauliques (pp. 270-287). Me-
moires de l’Académie des Sciences de Paris.
De Parcieux, A. (1759). Mémoire dans lequel on démontre que l’eau
d’une chute destinée a faire mouvoir quelque machine (pp. 603-605).
Histoire de l’Académie Royale des Sciences de Paris, année 1754.
Paris: Imprimerie Royale.
Denny, M. (2003). The efficiency of overshot and undershot water-
wheels. European Journal of Physics, 25, 193-202.
Desaguliers, J. T. (1744). A course of experimental philosophy, vol. 2.
London: Innys W. et al.
Euler, J. A. (1754). Enodatio quaestionis quomodo vis aquae aliusve
fluidi cum maximo lucro ad molas circum agendas aliave opera
perficienda impdendi possit? Gottingen: Kubler D.F.
Euler, L. (1754). Maximes pour arranger le plus avantageusement les
machines destinées è élever de l’eau par le moyen des pompes (pp.
185-232). Mémoires de l’Académie des Sciences de Berlin. Berlin:
Auder et Spenner
Fourneyron, B. (1840). Memoires sur les turbines hydrauliques. Liege:
Leroux.
Hall, A. R. (1961). Engineering and the scientific revolution. Technol-
ogy and Culture, 2, 333-341. doi:10.2307/3100888
Mariotte, E. (1686). Traité des eaux et autres corps fluides. Paris: Es-
tienne Michallet.
Musson, A. E., & Robinson, E. (1969). Science and technology in the
industrial revolution. Manchester: University Press of Manchester.
Newton, I. (1972). Philosophiae naturalis principia mathematica (3rd
ed.). Cambridge: Cambridge University Press.
Parent, A. (1745). Sur la plus grande perfection possible des machines.
Histoire de l’Académie Royale des Sciences de Paris, année 1704.
Paris: Martin G., Coignard JB., Guerin HL., pp. 323-338.
Poncelet, J. V. (1825). Mémoire sur les roues verticales à palettes cour-
bes mues par en dessous, suivi d’expériences sur les effets mé-
caniques de ces roues. Annales de Chimie et de Physique, 30, 136-
188, 225-257.
Poncelet, J. V. (1827). Mémoire sur les roues verticales à aubes cour-
bes mues par en dessous, suivi d’expériences sur les effets mé-
caniques de ces roues. Metz: Thiel.
Reynolds, T. S. (1979). Scientific influences on technology: The case
of the overshot waterwheel, 1752-1754. Technology and Culture, 20,
270-295. doi:10.2307/3103867
Singer et al. (1957). A history of technology, Vol. 3. Oxford: Clarendon
Press.
Smeaton, J. (1759). An experimental enquiry concerning the natural
powers of water and wind to turn mills, and other machines, depend-
ing on a circular motion. Philosophical Transactions of the Royal
Society of London, 51, 100-174. doi:10.1098/rstl.1759.0019
Smeaton, J. (1776). An experimental examination of the quantity and
proportion of mechanic power necessary to be employed in giving
different degrees of velocity to heavy bodies from a state of rest.
Philosophical Transactions of the Royal Society of London, 66,
450-475. doi:10.1098/rstl.1776.0029
Smeaton, J. (1782). New fundamental experiments upon the collision of
bodies. Philosophical of the Royal Society of London, 72, 337-354.
Storck J., & Teague W. (1952). Flour for man’s bread: A history of
milling. Minneapolis: University of Minnesota Press.
Syson, L. (1980). The watermills of Britain. London: David and Charles.
Torricelli, E. (1644). Opera geometrica. Florentiae: Masse & de Landis.