Journal of Financial Risk Management
2013. Vol.2, No.3, 47-54
Published Online September 2013 in SciRes (http://www.scirp.org/journal/jfrm) http://dx.doi.org/10.4236/jfrm.2013.23008
Stock Market Volatility, Speculative Short Sellers and Weekend
Effect: International Evidence
Weili Zhai1, Hossein S. Kazemi2*, Jibao He3#, Jinghan Cai4
1Department of Finance, Shenzhen University, Shenzhen, China
2Department of Economics, Stonehill College, Easton, USA
3Shenzhen Stock Exchange, Shenzhen, China
4Department of Economics, Boston College, Boston, USA
Received June 15th, 2013; revised July 15th, 2013; accepted July 22nd, 2013
Copyright © 2013 Weili Zhai et al. This is an open access article distributed under the Creative Commons At-
tribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the
original work is properly cited.
We test the Chen and Singal (2003) hypothesis that speculative short sellers add to the selling pressure on
Mondays, and hence add to the weekend effect, by examining evidence from 60 market indices. We find
strong evidence that, until about a decade ago, the actions of short sellers could explain the weekend ef-
fect. Recently, however, the relationship between short sales and the weekend effect is gradually dissi-
pating in developed markets, probably due to the cross-market hedges of short sellers. These findings
strongly support, rather than weaken, the Chen and Singal hypothesis.
Keywords: Weekend Effect; Short Sales; Market Anomaly; Stock Market Volatility
Introduction
We provide empirical evidence, from an international per-
spective, to document the long standing weekend effect and its
relation with short sales. This paper is an expanded and en-
hanced version of the authors’ previous paper1 on this topic.
The research on the weekend anomaly begins with French
(1980), who studies the S&P 500 Index over the period 1953
through 1977, and with Gibbons and Hess (1981) who study the
S&P 500 Index and the CRSP value-and-equal-weighted in-
dexes for NYSE and AMEX securities over the period 1962
through 1978. After that, there is much evidence in support of
systematically lower returns on Mondays. Keim and Stam-
baugh (1984) find that Friday returns are lower when there is
Saturday trading. Ariel (1990) finds that the value of a signifi-
cantly larger number of stocks increases pre-holiday rather than
post-holiday. Wang et al. (1997) find that lower returns sys-
tematically occur on Mondays in the second half of a month.
In addition to the US stock market, researchers have also do-
cumented weekend effects in other equity markets. Hindmarch
et al. (1984) find a weekend effect in the Canadian market. Jaffe
and Westerfield (1985) find weekday effects similar to those in
the US market for the Canadian, British, Japanese, and Austra-
lian equity markets. Condoyanni et al. (1989)find significantly
negative Monday or Tuesday returns in a study including seven
developed markets. Chang et al. (1993) find significantly nega-
tive Monday returns in 13 of 23 international markets. Dubois
and Louvet (1996) provide further evidence of the existence of
low Monday returns for developed markets in an examination
of eleven indices from nine countries during the period 1969
through 1992. Cai et al. (2006) document a pattern similar to
that found by Wang et al. (1997), i.e., they find that, in the Chi-
nese stock market also, weekend effects mainly occur in the se-
cond half of a month.
Many researchers propose potential explanations for the wee-
kend effect. Keim and Stambaugh (1984) establish that the phe-
nomenon has been a regular feature of the financial landscape
for many years, and they reject the possibility that it arises from
measurement error. Keim (1989) finds that the bid ask bounce
can explain about 17 percent of the weekend effect. Lakonishok
and Maberly (1990), Abraham and Ikenberry (1994) and Chan
et al. (2004) attribute part of the weekend effect to the differen-
tial trading patterns or holding preferences of institutions and
individuals. Sias and Starks (1995) also document an associa-
tion between the weekend effect and institutional ownership.
More recently, Chen and Singal (2003) propose a new expla-
nation, i.e., that the weekend effect might be linked to short
sales. They argue that, unlike a long position whose potential loss
is limited, a short position faces theoretically unlimited down-
side risks. Thus short positions require closer monitoring. Since
short sellers cannot close their positions during non-trading
hours, they tend to close their positions by the weekend to avoid
the potential losses which might occur during the long period of
non-trading. Empirically, Chen and Singal (2003) find that stocks
with high short interest experience a relatively greater weekend
effect than stocks with low short interest, which directly sup-
ports their hypothesis.
However, recently some researchers have issued evidence
that is contrary to Chen and Singal’s (2003) findings. For ex-
ample, Blau et al. (2007) do not find that short selling is more
abundant on Mondays. Christophe et al. (2006) distinguish short
sales by dealers from those by customers, but they find no evi-
*Corresponding author.
#Jibao He acknowledges supports from NSFC project 71172226/G0206.
1Jinghan Cai, Hossein S. Kazemi, Jibao He and Weili Zhai “Weekend Effect
and Short Sales: International Evidence”. International Advances in Eco-
nomic Research. May 2013, Volume 19, Issue 2, 209-211.

W. L. ZHAI ET AL.
dence that the Monday-Friday return differences are closely
linked to the Monday-Friday differences in either type of short
sales.
To clarify whether short sales play a significant role in ex-
plaining the weekend effect, we extend the empirical tests. Us-
ing the daily stock index returns from 60 markets, we find that,
during the period from 1980 to 1994, the practice of short sales
can in fact explain the weekend effect; however, during the pe-
riod from 1995 to 2007, the cross-sectional weekend effect can-
not be explained by the practice of short sales. Our findings
imply that the hypothesis proposed by Chen and Singal (2003)
used to work well, but that it has actually been losing its effec-
tiveness during the last decade, especially in developed markets.
One potential explanation is that short sellers in developed mar-
kets can now hedge worldwide, and they do not need to be li-
mited to a single market, while short sellers in developing mar-
kets, in contrast, have to balance their short positions within the
local markets due to strict capital account controls. In order to
test this explanation, we separate the developed markets from
the developing markets, and test the relationship between short
sales and the weekend effect in each case. Our findings are
highly consistent with the explanation that the weakened impact
of short sales on the weekend effect is due to cross-market he-
dges. Our findings provide sound evidence to support Chen and
Singal’s (2003) hypothesis and help explain why others such as
Christophe et al. (2006) and Blau et al. (2007) cannot find re-
sults consistent with findings of (2003).
The rest of the paper is arranged as follows: Section 2 ex-
plains the data source and sample characteristics, Section 3 shows
the empirical results, Section 4 is the robust check, and Section
5 concludes.
The Data
We obtained daily index data from the CEIC Daily Database.
Our dataset contains 60 market indices from 59 countries2. For
each country, we begin our analysis on January 1st, 1980, or on
the first date for which CEIC data is available, whichever comes
later. The appendix lists a detailed list of markets and the be-
ginning date used in our paper. We define the daily return of
index i on day t as




,,
log log
it itit
rI I


,1
3.
where Ii,t refers to the price index i on day t.
The information about the availability of short sales in each
market is from Charoenrook and Daouk (2005). The details can
also be in the Appendix. We can see that of the 60 indices (60
markets from 59 countries), 27 are from markets where short
sales are allowed and practically feasible, while the other 33 are
from markets where short sales are either legally prohibited or
practically not feasible. We further divide the 60 sample indices
into two subsamples: the developed markets and the developing
markets. 27 indices are identified as coming from developed
economies, while the other 33 are from developing economies4.
Table 1 shows the basic information about the weekend ef-
fect in the 60 markets. For each market, we first run the fol-
Table 1.
Descriptive statistics.
Panel A: i

Full sample Before
1995 After 1995
Total market number 60 37 60
# of negativei

(%) 45 (75.00) 28 (75.68) 38 (63.33)
# of − & sig. i

(%) 23 (38.33) 19 (51.35) 15 (25.00)
# of + & sig. i

(%) 2 (3.33) 1 (2.70) 2 (3.33)
N
ote: Panel A is based on the coefficient of model (1).
Panel B: return Full sample Before 199 5 After 1995
Mondays (%) 0.019 −0.064 0.025
Other days (%) 0.073 0.098 0.065
t-value −3.62 −3.51 −2.60
p-value 0.001 0.001 0.012
lowing model (1):
itiiit it
r MONDUM



 (1)
Where rit is the daily return of market i on date t. MONDUMit is
a dummy variable which takes the value of 1 if date t is a Mon-
day and 0 otherwise5. We can see from the table that of the 60
markets, 45 have negative coefficients for the variable MON-
DUMit, making up 75% of the total number of markets. Among
these 45 markets, 23 have coefficients that are significantly
negative. Comparatively, only 2 out of the 60 indices carry
positive and significant coefficients for MONDUMit.
We further divide our total sample period into two sub-pe-
riods—before Jan 1, 1995 and after Jan 1, 1995—to see whe-
ther the negative coefficients happen in some specific sub-pe-
riod. The sub-period results show a change from sub-period 1
to sub-period 2. In sub-sample 1, 28 out of 37 (75.68%) indices
show negative coefficients for the variable MONDUMit6, and
19 (51.35%) are significant. However, in sub-sample 2, only 38
out of 60 coefficients (63.33%) are negative, and only 15 (25%)
are significant. These results are consistent with the existing li-
terature, which has found that the weekend effect has been get-
ting weaker recently.
In Panel B, we show the cross sectional means and medians
of average Monday returns and of other weekday returns for
each index. We can see that for the full sample, the mean of ave-
rage Monday returns for the 60 indices is 0.019%, significantly
(1% level, with t-value of −3.62) lower than that of the other
weekday returns (0.073%). The median of average Monday re-
turns is 0.018%, also significantly (1% level, with z-value of
−3.27) lower than that of the other weekday returns (0.058%).
We also test this difference in the two sub-samples, and the same
pattern appears in both.
In summary, the results obtained in this section confirm the
existing literature in the following two aspects: First, the
2For the US, we use both the Dow Jones Index and the S&P 500. For
other countries, only one major stock index is used.
3We drop any observations with the daily return higher than 20% or
lower than −20%. 48 observations are therefore deleted, comprising less
than 0.1% of the total number of observations.
4We adopt the standards from the IMF 1997 World Economic Outlook.
5Note that for markets (Bangladesh, Jordan, Egypt, Saudi Arabia and
Israel) where the first day after the weekend is not Monday, MON-
D
UMit takes the value of 1 for the first trading day after the weekend.
This applies to all the MONDUMit variables in this paper.
623 indices do not have data before Jan. 1, 1995.
48

W. L. ZHAI ET AL.
weekend effect is a world-wide phenomenon; Secondly, the
degree of the weekend effect has been getting weaker in the re-
cent decade, compared with one decade ago.
Short Sales and the Weekend Effect
Chen and Singal (2003) explain the weekend effect by intro-
ducing the potential impacts of short sellers. It is well known
that stock returns for unhedged short positions are theoretically
unbounded. Also, researches such as Asquith and Muelbroek
(1996) and DeChow et al. (2001) have noted that unhedged
short positions face higher risks. All these facts indicate that an
uncovered short exposure needs close watching to minimize the
chance of large losses due to a price increase. Non-trading hours
will therefore incur more risks than usual because short sellers
are unable to adjust their positions. Based on these arguments,
Chen and Singal (2003) believe that short sellers tend to close
their positions before the weekend, and to reopen them on
Mondays. This leads to lower average Monday returns.
In this section we test whether short sales explain the week-
end effect worldwide. If Chen and Singal’s (2003) story is true,
in the markets allowing short sales, the weekend effect will be
stronger than where short sales are prohibited. Therefore, we
introduce the following regression models:
itiiit it
r MONDUM

   (1)
itiiit iit it
rMONDUMCMON

   
ititiitiiit CMONMONDUMr
  (2)
where rit is the daily return of market i on date t. MONDUMit is
a dummy variable which takes the value of 1 if date t is a
Monday and 0 otherwise. CMON it is the interaction term of a
short sale dummy (1 if the market allows short sales and 0 oth-
erwise) and the MONDUMit.
Table 2 shows the results of a pooled regression of models
(1) and (2). For the full sample, the coefficient of MONDUMit
in model (1) is −0.066%, significant at 1% level, meaning that
the weekend effect does exist around the world. After entering
the interaction term, the coefficient of MONDUMit in model (2)
changes to −0.039%, less negative than in regression (1), mean-
ing that short sales do explain a large part of the weekend effect.
On average, the Monday return of a firm in a market allowing
Table 2.
Pooled regression results.
Full sample Before 199 5 After 1995
Unit: 10−3 (1) (2) (1) (2) (1) (2)
MONDUM −0.66**
(−8.57)
−0.39**
(−3.73)
−1.26**
(−9.37)
−0.24
(−1.03)
−0.45**
(−4.87)
−0.39**
(−3.30)
CMON
−0.50**
(−3.68) −1.46** (−5.54) −0.12 (−0.71)
Cons 0.67**
(19.73)
0.67**
(0.00)
0.83**
(14.22)
0.83**
(14.24)
0.61**
(14.90)
0.61**
(14.90)
F-value 73.47 43.50 87.87 59.30 23.7412.12
Prob > F 0.000 0.000 0.000 0.000 0.0000.000
R^2 (%) 0.03 0.04 0.15 0.20 0.01 0.01
Note: This table is based on models (1) and (2). t-values are in parentheses; *and
**represent significance level at 5% and 1% respectively. t-values are in parenthe-
ses.
short sales is 0.050% lower than the Monday return from a
marketnot allowing short sales. And the Monday return of a
firm in a short-sale allowing market is −0.089% (−0.039 +
(−0.050)) lower than the non-Monday return of a firm in a mar-
ket where short sales are not allowed.
The sub-sample results show a pattern different from the full
sample. In the first sub-sample, the coefficient of MONDUMit
in model (1) is −0.126%, meaning that the weekend effect ex-
ists around the world before 1995. After entering CMONit, the
coefficient of MONDUMit gets insignificant, and the coefficient
of CMONit is significantly negative, implying that short sales
can explain most of the weekend effect before 1995.
However, in the second sub-sample, the coefficient of
CMONit is now −0.012% (not significant), and MONDUMit is
still negatively significant. This pattern indicates that, after 1995,
the actions of short sellers cannot explain the weekend effect.
We argue that the difference in the two sub-samples actually
supports Chen and Singal’s (2003) story, rather than weakens it:
until about a decade ago, the world’s stock markets were more
isolated, and capital flows were not as convenient as they are
today. Hence, cross-market hedges were not often employed,
and short sellers had to adjust their positions mainly within the
local market. Thus the practice of closing and re-opening short
positions around the weekend was necessary and frequent, lea-
ding to the prominent weekend effect. However, with the con-
verging trend of the world’s stock markets, capital flows be-
tween markets became easier and encountered fewer obstacles.
Short sellers are now getting more comfortable at managing
their positions worldwide, rather than staying within one single
market. Also, investors have incentives to hedge their short po-
sitions across markets because by doing so they can reduce the
transaction costs incurred by closing and rebuilding short posi-
tions within a single market. This explains why the weekend
effect was more prominent a decade ago, but less prominent in
the recent decade.
Moreover, we want to separate developed from developing
markets, which is important because capital flows and cross-
market hedges will happen more in developed markets since in
most developing markets capital accounts are still under strict
control. This means that, if our explanation above is correct, the
impact of short sellers’ actions should decrease by more in de-
veloped markets than in developing ones. When it comes to de-
veloped markets, capital flows and cross-market hedges be-
come more available throughout this time period, and so short
sellers’ actions should have a decreased impact in the latter sub-
sample. We spell all this out in the predictions in Table 3.
Pattern 1: In the full sample, short sellers’ actions can ex-
plain the weekend effect in developing markets, since even at
the present time, capital accounts in developing economies are
still strictly controlled, and capital free flow is not expected.
However, in the developed markets, the impact of short sales on
the weekend effect is more ambiguous.
Table 3.
Comparison between developed and developing markets—predictions.
The impacts of short sales on
weekend effects Developed markets Developing markets
Full sample Ambiguous Strong
Before 199 5 Strong Strong
After 1995 Weak Strong

W. L. ZHAI ET AL.
Pattern 2: In sub-sample 1, (before 1995) short sellers’ ac-
tions can explain the weekend effect in developed and develop-
ing markets, since capital market conglomerations were not pre-
vailing even in developed markets, and so short sellers every-
where had to adjust their short positions mainly within a single
market only.
Pattern 3: In sub-sample 2, (after 1995) short sellers’ ac-
tions can explain the weekend effect in developing markets. For
developed markets, we do not expect to observe negative and
significant coefficients of CMONit because, after 1995, the im-
pact of short sales on the weekend effect in developed markets
is weakened due to capital market conglomerations.
In order to empirically test the patterns from Table 3, we in-
troduce Table 4 for the pooled regressions based on equations
(1) and (2) as shown earlier and all variables are defined the
same. The empirical results in Table 4 are highly consistent
with the predictions in Table 3. In the full sample, the coeffi-
cients of CMONit are not significant for the developed markets,
while the coefficients of CMONit for the developing markets
are significantly negative, confirming the predictions in Pattern
1. In the subsample before 1995, the coefficients of CMONit for
both the developing and the developed markets are negative
and significant, confirming the predictions in Pattern 2. More-
over, after controlling for the impact of short sales, the coeffi-
cients of MONDUMit become less negative, especially for the
developed markets, indicating that the impact of short sellers’
actions can explain the weekend effect to a large extent. In the
subsample after 1995, the coefficients of CMONit for developed
markets are now positive, indicating that, in the recent decade
or so, short sellers’ actions cannot explain the weekend effect.
This is because short sellers in developed markets may now
hedge in other markets, rather than adjust their positions within
one market. However, in developing markets the coefficients of
CMONit are still negative and significant, implying that in de-
veloping markets investors cannot easily hedge across markets;
short sellers still have to adjust their positions around the wee-
kends, leading to a strong impact on the weekend effect. Our
empirical results strongly support Chen and Singal’s (2003) fin-
dings that the weekend effects are partly caused by the actions
of short sellers’ position adjustments.
Robust Check
Different Sub Periods
Up until now we set the early sub-sample as the period before
Jan 1st, 1995, and the recent sub-sample as the period on or
after Jan 1st, 1995. The selection of this breaking date is some-
what arbitrary. In order to reduce potential bias, we also select-
ed different dates as breaking points, and re-examined the above-
mentioned tests. We tried Jan 1st, 1996, Jan 1st, 1997, Jan 1st,
1998, Jan 1st, 1999 and Jan 1st, 2000. The results for all these
dates are highly consistent with what we found when we used
Jan 1st 1995.
Consistent Sub-samples be fo re a nd after 1995
From Table 1 we can see that in the after 1995 sub-sample,
there are 60 indices, but that in the before 1995 sub-sample,
there are only 37 indices. The problem is that either the indices
do not date back to before 1995, or that the CEIC database does
not contain data for before 1995. This leads to the question of
whether this sample difference leads to any bias. To answer this
question, we kept only the 37 indices that have data before
1995, and re-performed all the above regressions on them alone.
The results are highly consistent with those in Table 5, indi-
cating that the different number of indices before and after 1995
does not lead to severe bias.
Short Sales and Put Options
Chen and Singal (2003) also provide a substitute explanation
besides short selling: the put option. They empirically docu-
ment that traders use options when available in preference to
short selling, possibly causing the weekend effect to dissipate.
Following this line, we also consider the substitution effect of
put options for short sales. The following model (3) is thus in-
troduced:
itiiit iit it
rMONDUMCMON




itiiit iitiitit
rMONDUM CMON PMON




(3)
whererit is the daily return of market i on date t. MONDUMit is
a dummy variable which takes the value of 1 if date t is a Mon-
day and 0 otherwise. CMONit is an interaction term of a short
sale dummy (1 if the market allows the practice of short sales
and 0 otherwise) and the MONDUMit. PMONit is an interaction
term of a put option dummy (1 if the market allows the practice
of put options and 0 otherwise) and the MONDUMit7.
Table 5 shows empirical results different from Chen and Sin-
gal’s (2003). The entering of PMONit does not affect the rela-
tionship between short sales and the weekend effect. The pat-
terns documented in Table 5 do not change at all, and the im-
pact of the put options on the weekend effect is trivial.
Discussion and Conclusions
The weekend effect is a research field full of discussions and
controversies. Ever since French (1980), many researchers do-
cument the existence of it and provide numerous potential ex-
planations. Recently, Chen and Singal (2003) propose an expla-
nation that the weekend effect might be linked to short sales.
They argue that since short sellers cannot close positions during
the weekend, they tend to close their positions before the wee-
kend and to re-open them on Mondays in order to avoid poten-
tial losses. However, other papers—such as Christophe et al.
(2006), and Blau et al. (2007)—declare that there is no empiri-
cal evidence to support Chen and Singal’s (2003) explanations.
In this paper, we examine the relationship between short
sales and the weekend effect from an international perspective.
Using 60 indices from around the world, we document the fol-
lowing patterns: In the full sample (both before and after 1995)
short sellers’ actions can explain the weekend effect in devel-
oping markets but not in developed ones. In sub-sample 1 (be-
fore 1995) the short sellers’ actions can explain the weekend ef-
fect both in developed and developing markets. In sub-sample 2,
(after 1995) short sellers’ actions can explain the weekend ef-
fect in developing markets but not in developed ones. These re-
sults are independent of various potential biases.
We propose a new potential explanation: the position adjust-
ments of short sellers cause the weekend effect if the investors
have to balance their positions in the local markets. This pheno-
menon was prevalent everywhere until about a decade ago, and
is still prevalent even today in developing markets where capi-
al accounts are strictly controlled and cross-market hedges are t
7The data about the availability of put options in each market are also from
Charoenrook and Daouk (2004).
50

W. L. ZHAI ET AL.
Table 4.
Comparison between developed and developing economies—empirical evidence.
re 1995 (37 indices) Sub-sample 2: After 1995 (60 indices) Full sample (60 indices) Sub-sample1: Befo
Unit: 10−3 Developed
ma
Developing Developed Developing Developed Developing
rkets markets markets markets markets markets
(1) (2) ) (1) ) (1) (1) ) (1) (1) (2(2(2)(2(2)
MONDUM −0.5
(−)
397
(−)
−0.8
(−)
572
(−)
1.14
(−)
115
(−)
−1.6
(−)
834
(−)
−0.2
(−)
514
(−)
−0.6
(−)
515
(−)
0.484
(13.)
0.906
(14.)
0.628
(11.)
1.507
(8.
0.406
(8.
0.807
(12. )
31 −0.
6.23 2.29
22 −0.
6.06 3.88
5 −0.
8.76 0.42
53 −0.
4.47 1.85
08 −0.
1.88 2.34
83 −0.
4.69 3.30
CMON
−0.170
(−0.89) −1.329 (−4.29) −1.207 (−4.22) −2.159 (−3.18) 0.394 (0.106) −1.081 (−3.01)
Constant 00
0.484
(13.00) 90
0.906
(14.90) 73
0.628
(11.13) 97)
1.507
(8.97) 34)
0.406
(8.34) 38
0.807
(12.38)
F-value
Prob > F
38.83 19.81
0.000
36.77 27.59
0.000
76.45 47.31 19.98 15.06
0.000
3.52 3.06 22.04 15.55
0.000 0.000 0.000 0.000 0.000 0.061 0.047 0.000 0.000
R^2 (%) 0.03 0.03 0.04 0.05 0.17 0.20 0.13 0.20 0.00 0.00 0.00 0.03
Note is the regsulton m) anluent
Full sample (60 indices) Before 1995
(37 indices)
After 1995
(60 indices)
: This table pooledression res based odels (1d (2). t-vas are in pareheses.
Table 5.
he impacts of put options.
T
Unit: 10−3 De
m
ing Develope
markets
loping
rkets
Developed
markets
eveloping
markets
veloped
arkets
Develop
markets
d Deve
ma
D
Model: (3) (3) (3) (3) (3) (3)
MONDUM (−) (−) ( (−) (−) (−)
−
Prob > F
Adj R(%)
−0.628**
2.57
−0.623**
3.65
0.612
1.23)
−1.420*
2.31
−0.821**
2.86
−0.501**
2.82
CMON −0.258
(−1.29)
−1.350**
(−4.33)
1.295**
(−4.30)
−2.025**
(−2.96)
0.171
(0.62)
−1.072**
(−2.96)
PMON 0.341
(1.34)
0.150
(0.59)
−0.768
(−1.77)
0.939
(1.40)
0.553
(1.66)
−0.045
(−0.16)
Constant 0.484**
(13.00)
0.906**
(14.90)
0.628**
(11.13)
1.507**
(8.97)
0.406**
(8.34)
0.807**
(12.38)
F-value 13.81 18.51 32.58 10.69 2.96 10.38
0.000 0.000 0.000 0.000 0.031 0.000
-square0.03 0.05 0.21 0.21 0.01 0.03
Note: *and ficance level atd 1% respectivellues are in paes.
d markets in the recent decade because short sellers can now
ey
th
short sales and the weekend effect has already largely
dissipated because of the existence of cross-markets hedging.
efore holidays: Existence and evi-
dence on possible causes. Journal of Finance, 45, 1611-1626.
doi:10.1111/j.1540-6
**represent signi 5% any. t-varenthes
not as easy. However, the weekend effect dissipates in develop- tween
e
hedge internationally rather than being limited to local markets.
This is a strong support for Chen and Singal’s (2003) story.
Recently more and more researchers tend to explain the wee-
kend effect through the investigation of intraday patterns. Th
us tend to use a relatively short sample period (one year, for
example) due to the large volume of data. However, consider-
ing the empirical results of this paper, we have enough evi-
dence to argue that too short a sample period may lead to the
study of an already weakened weekend effect (especially forde-
veloped markets). This explains why recent studies cannot find
empirical evidence to support Chen and Singal (2003). For
example, Blau, et al. (2007) use a one-year sample of the NYSE
listed stocks, and declare that they do not find abundant short
selling on Mondays. Christophe, et al. (2006) use NASDAQ
stocks from September 2000 to July 2001 and find no test
which supports the idea that the Monday-Friday return differ-
ences are closely linked to the Monday-Friday differences in
short sales. But since both of these studies employ US data (a
developed market) in the recent decade, the relationship be-
So their evidence cannot be considered to be weakening Chen
and Singal’s (2003) conclusion.
REFERENCES
Ariel, R. (1990). High stock returns b
261.1990.tb03731.x
k, L. (1996). An empiricaAsquith, P., & Muelbroel investigation of short
, 29,
interest, working paper.
Abraham, A., & Ikenberry, D. (1994). The individual investor and the
weekend effect. Journal of Financial and Quantitative Analysis
263-277. doi:10.2307/2331225
Blau, B., Van Ness, B., & Van Ness, R. (2007). Short selling and the
weekend effect for NYSE securities, working paper.
Cai, J., Li, Y., & Qi, Y. (2006). The day of the week effect: New evi-
dence from Chinese stock market. The Chinese Economy, 39, 71-88.
doi:10.2753/CES1097-1475390206
Cai, J., Kazemi, H. S., He, J., & Zhai, W. (2013). Weekend effect and
short sales: International evidence. International Advances in Eco-

W. L. ZHAI ET AL.
nomic Research, 19, 209-211. doi:10.1007/s11294-013-9398-z
han, S. H., Leung, W. K., & Wang, K. (2004). The impact of institu-
tional investors on the Monday sea
C
sonal. Journal of Business, 77,
967-986. doi:10.1086/422630
hang, E., Pinegar, J., & Ravichandran, R. (1993). International evi-
dence on the robustness of the day-of-the-week effect. Journal o
Cf Fi-
nancial and Quantitative Analysis, 28, 497-513.
doi:10.2307/2331162
haroenrook, A., & Daouk, H. (2005)C. The world pricing of short sell-
C
540-6261.00541
ing, working paper.
hen, H., & Singal, V. (2003). Role of speculative short sales in price
formation: The case of the weekend effect. Journal of Finance, 58,
685-705. doi:10.1111/1
eturns, working paper.
Christophe, S., Ferri, M., & Angel, J. (2006). Short selling and the wee-
kend effect in stock r
Condoyanni, I., O’Hanlon, J., & Ward, C. W. R. (1989). Day of the
week effects on stock returns: International evidence. Journal of Bu-
siness Finance and Acc ou nt in g , 14, 159-174.
doi:10.1111/j.1468-5957.1987.tb00536.x
eChow, P., Hutton, A. Meulbroek, L., & SloDan, R. (2001). Short sel-
lers, fundamental analysis, and stock returns. Journal of Financial
Economics, 61, 77-106. doi:10.1016/S0304-405X(01)00056-3
ubois, M., & Louvet, P. (1996). The day-of-the-
tional evidence. Journal of Banking and F
Dweek effect: Interna-
inance, 20, 1463-1484.
doi:10.1016/0378-4266(95)00054-2
ench, K. (1980). Stock returns and the weekend effect. Journal of Fi- Fr
016/0304-405X(80)90021-5nancial Economics, 8 , 55-69. doi:10.1
Gibbons, M., & Hess, P. (1981). Day of the week effects and asset re-
turns. Journal of Business, 5 4 , 579-596. doi:10.1086/296147
Hindmarch, S., Jentsch, D., & Drew, D. (1984). A note on Canadian
stock returns and the weekend effect. Journal of Business Admini-
Ja
al evidence. Journal of Fina nce, 40, 433-454.
stration, 14, 163-172.
ffe, J., & Westerfield, R. (1985). The weekend effect in common stock
returns: The internation
doi:10.1111/j.1540-6261.1985.tb04966.x
eim, D. (1989). Trading patterns, bid-ask spread, and estimated secu-
rity returns. Journal of Financial Economi
Kcs, 25, 75-89.
doi:10.1016/0304-405X(89)90097-4
eim, D., & Stambaugh, R. (1984). A further investigation
kend effect in stock returns. Journal o
K of the wee-
f Finance, 39, 819-835.
doi:10.1111/j.1540-6261.1984.tb03675.x
akonishok, J., & Maberly, E. (1990). The weekend effect: T
patterns of individual and institutional inv
Lrading
estors. Journal of Finance,
45, 231-244. doi:10.1111/j.1540-6261.1990.tb05089.x
ias, R., & Starks, L. (1995). The day of the week anomaly: The role of
institutional investors. Financial Analysts Journal, 51, 5
S
8-67.
doi:10.2469/faj.v51.n3.1906
ang, K., Li, Y., & Erickson, J. (1997). A new look at the M
effect. Journal of Finance, 52
Wonday
, 2171-2186.
doi:10.1111/j.1540-6261.1997.tb02757.x
52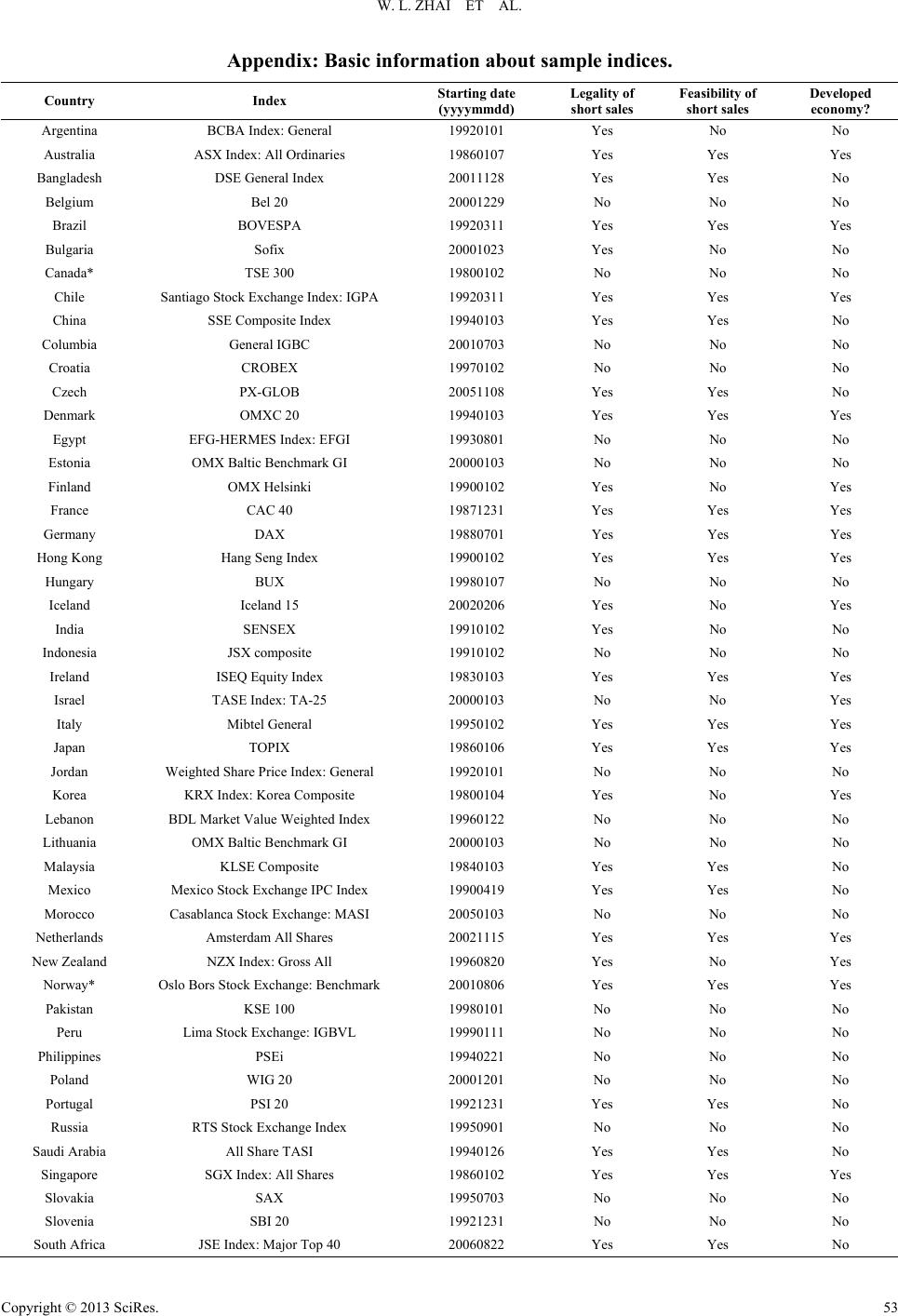

W. L. ZHAI ET AL.
Appendix: Basic information about sample indices.
Country Index
Starting date
(yyyymmdd) Legality of short sales Feasibil ity of short sales Developed economy?
Argentina BCBA Index: General 19920101 Yes No No
Australia ASX Index: All Ordinaries 19860107 Yes Yes Yes
Bangladesh DSE General Index 20011128 Yes Yes No
Belgium Bel 20 20001229 No No No
Brazil BOVESPA 19920311 Yes Yes Yes
Bulgaria Sofix 20001023 Yes No No
Canada* TSE 300 19800102 No No No
Chile Santiago Stock Exchange Index: IGPA 19920311 Yes Yes Yes
China SSE Composite Index 19940103 Yes Yes No
Columbia General IGBC 20010703 No No No
Croatia CROBEX 19970102 No No No
Czech PX-GLOB 20051108 Yes Yes No
Denmark OMXC 20 19940103 Yes Yes Yes
Egypt EFG-HERMES Index: EFGI 19930801 No No No
Estonia OMX Baltic Benchmark GI 20000103 No No No
Finland OMX Helsinki 19900102 Yes No Yes
France CAC 40 19871231 Yes Yes Yes
Germany DAX 19880701 Yes Yes Yes
Hong Kong Hang Seng Index 19900102 Yes Yes Yes
Hungary BUX 19980107 No No No
Iceland Iceland 15 20020206 Yes No Yes
India SENSEX 19910102 Yes No No
Indonesia JSX composite 19910102 No No No
Ireland ISEQ Equity Index 19830103 Yes Yes Yes
Israel TASE Index: TA-25 20000103 No No Yes
Italy Mibtel General 19950102 Yes Yes Yes
Japan TOPIX 19860106 Yes Yes Yes
Jordan Weighted Share Price Index: General 19920101 No No No
Korea KRX Index: Korea Composite 19800104 Yes No Yes
Lebanon BDL Market Value Weighted Index 19960122 No No No
Lithuania OMX Baltic Benchmark GI 20000103 No No No
Malaysia KLSE Composite 19840103 Yes Yes No
Mexico Mexico Stock Exchange IPC Index 19900419 Yes Yes No
Morocco Casablanca Stock Exchange: MASI 20050103 No No No
Netherlands Amsterdam All Shares 20021115 Yes Yes Yes
New Zealand NZX Index: Gross All 19960820 Yes No Yes
Norway* Oslo Bors Stock Exchange: Benchmark 20010806 Yes Yes Yes
Pakistan KSE 100 19980101 No No No
Peru Lima Stock Exchange: IGBVL 19990111 No No No
Philippines PSEi 19940221 No No No
Poland WIG 20 20001201 No No No
Portugal PSI 20 19921231 Yes Yes No
Russia RTS Stock Exchange Index 19950901 No No No
Saudi Arabia All Share TASI 19940126 Yes Yes No
Singapore SGX Index: All Shares 19860102 Yes Yes Yes
Slovakia SAX 19950703 No No No
Slovenia SBI 20 19921231 No No No
South Africa JSE Index: Major Top 40 20060822 Yes Yes No

W. L. ZHAI ET AL.
Continued
Spain IGBM General 19800102 Yes No Yes
Sweden SSE 30 19860930 Yes Yes Yes
Switzerland All Swiss Shares 19990628 Yes Yes Yes
Taiwan TSEC Index 19940105 Yes Yes Yes
Thailand SET Index 19871218 Yes Yes No
Turkey ISE NPI: 1st Sec: 100 19981001 Yes Yes No
UK FT 30 19860107 Yes Yes Yes
Ukraine PFTS Index 19971103 No No No
U.S. Dow Jones Index 19800101 Yes Yes Yes
U.S. S&P 500 19800101 Yes Yes Yes
Venezuela Caracas Stock Exchange Index: IBC 19940105 No No No
*Note: for Canadian market, TSE 300 is used on and before Apr. 30, 2002. On or after May 1, 2002, the TSE 300 is no longer used so we use TSX composite Index instead.
For Norway market, Norway index (total) is used on and before September 28th, 2001, after that, the Oslo Borse Stock Exchange Benchmark index is used instead because
the former is no longer utilized.
54