 Journal of Applied Mathematics and Physics, 2013, 1, 37-46 http://dx.doi.org/10.4236/jamp.2013.13007 Published Online August 2013 (http://www.scirp.org/journal/jamp) Random Attractors for the Dissipative Hamiltonian Amplitude Equation Governing Modulated Wave Instabilities with Additive Noise* Jinyan Yin, Yangrong Li, Huijun Zhao School of Mathematics and Statistics, Southwest University, Chongqing, China Email: yjy111@swu.edu.cn, liyr@swu.edu.cn, huijun88@swu.edu.cn Received June 10, 2013; revised July 15, 2013; accepted September 10, 2013 Copyright © 2013 Jinyan Yin et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT In this paper, we study the random dynamical system (RDS) generated by the dissipative Hamiltonian amplitude equa- tion with additive noise d efined on the periodic boundaries. We inv estigate the existence o f a compact random attractor for the RDS associated with the equation through introducing two functions and one process in . The com- pactness of the RDS is established by the decomposition of solution semigroup. 1 0 EHL 2 Keywords: Random Dynamical System; Random Attractor; Hamiltonian Amplitude Equation 1. Introduction The Hamiltonian amplitude equation 2 i2 xtt 0 , (1) was first proposed by Tanaka, Yajima and Wadati as a model for the nonlinear modulation of stable plane wave in unstable media [1,2]. In 1992 as an improved version of (1), the equation 2 i2 0,0 xtt xt 1 , (2) was proposed [3], which generalized (1) in the sense that ,; 0, t xt , (3) but one can show that for most initial data 0 lim, ;, tx t, (4) even if the two functions agree at t = 0. Both of these models can be derived systematically from more com- plicated Hamiltonian systems through a particular limit- ing process (nearly monochromatic waves of small am- plitude) corresponding to 0 . Even so, keeping 0 in (2) is crucial because (1) is formally integrable but ill-posed, whereas (2) is a generalization of it which is apparently not integrable but well-posed. In this paper, we consider the following dissipative Hamiltonian amplitude equation governing modulated wave instabilities perturbed by an additive white noise 2 1 i t txtxxx m jj j duudtudtudtudtfuudt hdW (5) 0 ,,, t ux uxuxux 1 , (6) and the periodic boundary condition ,u xLtu xLt ,, (7) where is an unknown complex valued function, is the unit of imaginary number, the internal ui , LL , , and are positive constants, which satisfy , the functions , , are time independent, the random functions 2 j hHI1,2, ,jm W, 1, 2j,,m , are independent two-side real-valued Wiener processes on a probability space ,, P which will be specified later, and s is , 1 C fs is real valued function which satisfies that 2 C 0 1 lim inf0,,1 s Fs s , (8) 0 1 lim inf0,,1 s sfsF ss *This work is supported by National Natural Science Foundation o China (11071199) and Natural Science Foundation of Chongqing (2009BB8105). , (9) C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 38 where 01 , 0 is a constant depended on and , and s d sf tt. The deterministic case has been studied extensively, for instance, Guo, B. L. and Dai, Z. D. [4] proved that there exists a global weak attractor 1 in 1 EHH 21 is actually a global strong attractor in 1 E. Dai, Z. D. [5proved the existence of a global attractor 0 in 12 0 EH , ande equality 01 for (5) and it] obtained t Lh . Dai, Z. DL. Huang, J. [6] obtained a global at- tractor for the unperturbed system in 0 E and 1 E re- ctivelyng, L., Dai, Z. D. [7] obtained the estimate of the Hausdorff dimension and the fractal dimension of a global attractor for the perturbed and unperturbed sys- tems separately. However, up to the best of our knowl- edge, the research for the dissipative Hamiltonian ampli- tude equation governing modulated wave instabilities with random attractors has not in volved. Recen . Yang, . Yaspe tly, many authors have studied the existence of ra ollows. In Section 2, for co ndom attractors for other equations [8-10]. In this paper, for (5), we first obtain an absorbing set in 0 E and 1 E respectively through introducing two functio and o process, then by the decomposition of solution semi- group we derive the compactness in 0 E. As far as we know, no one has studied stochastic eations through introducing two functions, so this method enriches the study of stochastic equations. This paper is organized as f ns ne qu nvenience of the reader, we recall some basic notions on function spaces and the theory of random dynamical system. In Section 3, we solve Equation (5) and get the corresponding RDS . In Section 4, we prove the exis- tence of a random attractor in 0 E for this RDS. Throughout this paper, we adopt the following nota- tions. We write 22 LLI, 11 HI, 22 HI fo dr short. Weenote byand d the norms, by , an , the inner prodts in 1 uc and 2 Lrespectively. We lso use au to denote the dular absolute v a lue of u. mo or 2. Preliminaries all some basic notions on function 2.1. Function Spaces and Operators g for (5). Let In this section, we rec spaces [4,7], the theory of RDS [11-14] and introduce the method of the existence of random attractors for the con- tinuous RDS [8,10], which we will use in this paper. We first consider the mathematical settin 2 L, 1 , 2 be usual Sobolev space, 12 0 EHL , H nd T ,uv . We define the f ts and norately: for any T 0 , i uv E and 2 scalar p 1 a uc 1 EH rod ollowing rms sepa ii T 0 ,uv E, we have 0 1212 12 ,, Euu vv , , 0 22 Euv , 2 for any T 1 , iii uv E and , we ve T 1 ,uv E ha 11 22 EDu Du, 22 12 1 ,,,v v 1 222 uv E Let 212 :2 DDA HHL , then is a positive self-adjoint operator, which has the firsigen- value t e 1 2 inf u . 12 uH u 2.2. Random Dynamical Systems Let ,, P be a probability space and : tR be a family of measur ch that ,t t , trans su t formations e preserving is measurable, 0id and tst s for all , tR. The flow t together with rresponding probability space theco ,,, t F is called a measurable dynamical system. 2.2.1 A continuous random dynamical sys- Definition tem(RDS) on a Polish space , d with Borel -algebra on ,,, t F is a measurable map :RX , ,, ,tx tx X such that ..as 1) ,id on 0 ; 2) t ,,, ssts , for all , tR (cocyc 3) le property); ,:t random compac is continuous. is a family of co A t set K mpact sets indexed by such thevery xat for the mapping ,xdxK is measurable wipect to th res . Lt ebe a random set and B . We say s B if attract lim,,0,. . ta s t dist t , where ,dist denotes the Hausdorff semi-distan in ce . We say absorbs B if there exists 0 such thall B tt B tat for , ,. , . t t s a . A random set is said to be a randoctor fo m attra r the RDS i..asf 1) i a randomompact set; s c 2) is invariant, that is, ,t t )( 3) for all t0; attracts all deterministicn bouded sets eore pact set ab B. Thm 2.2.2 If there exists a random com sorbing every bounded set B,then the RDS possesses a random attractor , , Copyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 39 0, :ts t st where is tomega-limit set oe the Equation and Generate a RDS he of B. 3. Slv We consider the probability space ,, P, where m 12 ,,,0 0 mCR , , :R and is the Borel - , while algebr a induced by the co mact opeology ofis the corresponding p Wie-n top ea P ner msure on , . Then, we identify with 12 ,, for m WtWtWtttR . ,W t Finally, we define the time shift by , ttt , a tR ontin . Then system. om dy- namical system urpose, w dimensional Ornstein-Uhlenbeck eq One may easily check that a solut by Putting ,,,ttR F is a metric dynamical cuous rand corresponding to (5). For this p We now want to establish e need to convert the stochastic equation with an addi- tive noise into a deterministic equation with a random parameter. Given 1,2,,jm, consider the stochastic station- ary solution of the one- uation jj j dzz dtdWt . (10) ion to (10) is given ed, tts . (11) jj ztWs tR 1 m j j zh z, by (10) we have 1 m j th dW . j dz zd We also need two facts 2021 ed0 2 , (12) as 2 00 jj Ez Ez . We also have lim j t zt t 0,. .as (13) Assumed . Then ding theorem 2 j hDAH , by Sobolev embed- 21 ICI, we have 1 j hCI. In particular, all Dh are bou ts a 00nded continuous functions. Thus there exis (dly onepending on h) such that 01 sup xI Dz ,,,. . m j j xtzttRa s (14) where j zhz and z is the Ornstein-Uh process defined by (11). It is also easy to prove that lenbeck 211 m j j zD zt , (15) zD z where only depends on h. a ran 10 To sat (5) generates dom tem, we let how th dynamical sys- vtu tutzt , atis es wher e is t the solution of (5), then u, v sfiu, t u 2 010 , , ,,, , t t uvu vAuuvi x xx z u vzzfuu uuvuuz (16) where 1 0 uH, 2 0 vL , and 0 T 00 ,,uv 0 E proof as . dete rministic case [4], one can et that By the same easily gfor ..sa , , the following results hold sa Theorem 3.1 If T 00 0 ,uv E, there exists a unique solution T 0 , ,tu E of (16), which ,tvt , tisfies 1 ,;CT H , ,ut ,,vt C 2 ;T L . If T 00 1 ,uv E , there exists a unique solution E T 1 ,,vt ,,tut isf of (16), which saties 2 ;uxH, ,,t CT 1 ,,;vtCT H . the solution of From the above discussion, we denote (5) by 0 ,;,ut utu (denote sometimesby , 0 ;,ut u ;ut , ,ut , ut or even if no E by u confusions). Then we can define a mapping :R 00 E 00 ,:,;0,tt 00 ,;0,;0, , 0,utuv t , ,vt , (17) by the definition 2.2.1, it is easy to show that is a continuous RDS onwith the following fact 0 E 00 ,0,;, , for T ,, ,0.uv E 00 00 4. Random Attractors ng Set in 4.1. Absorbi 0 In this subsection, we prove that the RDS defined by rb set , which . Recall that (17) has a bounded absoing absorbs, in fact, all the bounded sets 0 BE i 0 BE T 000 , ;,, ;,,, ;,tutuvtv s the solu- tion of (16) with 0 uu and vuuzv 10 0 . We then rewrite (16) as follows C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 40 ,LF u T ,,,,,,vt , (18) where , u v II L II , 2 , xx x z Fiuvzzfu u . We now can prove the absorption of RDS (defin by (17)) in . Lemma 4.1or any no random bounded , there ex ed 0 E Fset B ists a random variable 10 satisfying the fol- lowing property: for every T u B ere ex 01 ,uu 0, th ists 1 B T , such that, for any B T , the following estimate holds ..as 0 01 ;, , E Proof. Taking the innu , 1,0tt. er prodct of (18) with in , we obtain that 0 E ,, , 2d LF t 2 1d . 19) Taking the real pa ( rt of (19), we find that 22 2 2 2d Re , t uv v 2 1d 1Re , Re,Re,Re , Re ,Re,Re, xx x uv uuv zuiu vv v zvz vfuuv . (20) Since 22 1Re , 1Re , 1d 11Re 2d t uv uuu z uu t , uz , (21) and 2 2 22 22 Re , 2 2 , Re ,Re ,Re , 1d dRe ,Re , 2d t t fu uv fu uuuzRe 22 2 2 2 2 d11 d d22 2 Re , Re, Re, Re,Re ,Re, Re, 0 x x uvFuxu t uvv iuvfuuu uzzvz v fu uz 2 . (23) We introduce two functions 22 2 111 ,d 222 uvuvFux , (24) uuufuuu fuuz uxfuuufu uz t , (22) it follows from (20)-(22), we get that 22 1 2 2 ,Re, Re, Re, Re,Re,Re, Re , x x Guv uuvv iuvfuu uzzvz v fu uz , (25) an u d one process 11 m j j Ct zt . So that (23) gives (26) 11 d,, dguv Guv t0 . (27) In the following, we denote byany constant depend- ing only on the data c ,,,,, f th s e or even ine positive contant , which can be dif- ferent from line to linsame line. Now we xists can prove there e0 , d and 1 d such that 101 22 1 , d uv 1 1 ,, 2 2 Guv guv C tgtc , (28) 2 1 1,d 2 uv c , (29) where 1 is defined by (15) and t will be defined in the following p ap er, henc e we o btain 101 1 d,,, d uvguvu . (3 ) v t0 In fact we ha ve 101 1 22 222 00 ,,, Re, Re , Re ,Re,22 x x Guv guvuv uuvv iuvfu zvfu uz (31) 22 dux. 2 0dR e, Re, 2Fu xuzzv u v C opyright © 2013 SciRes. JAMP 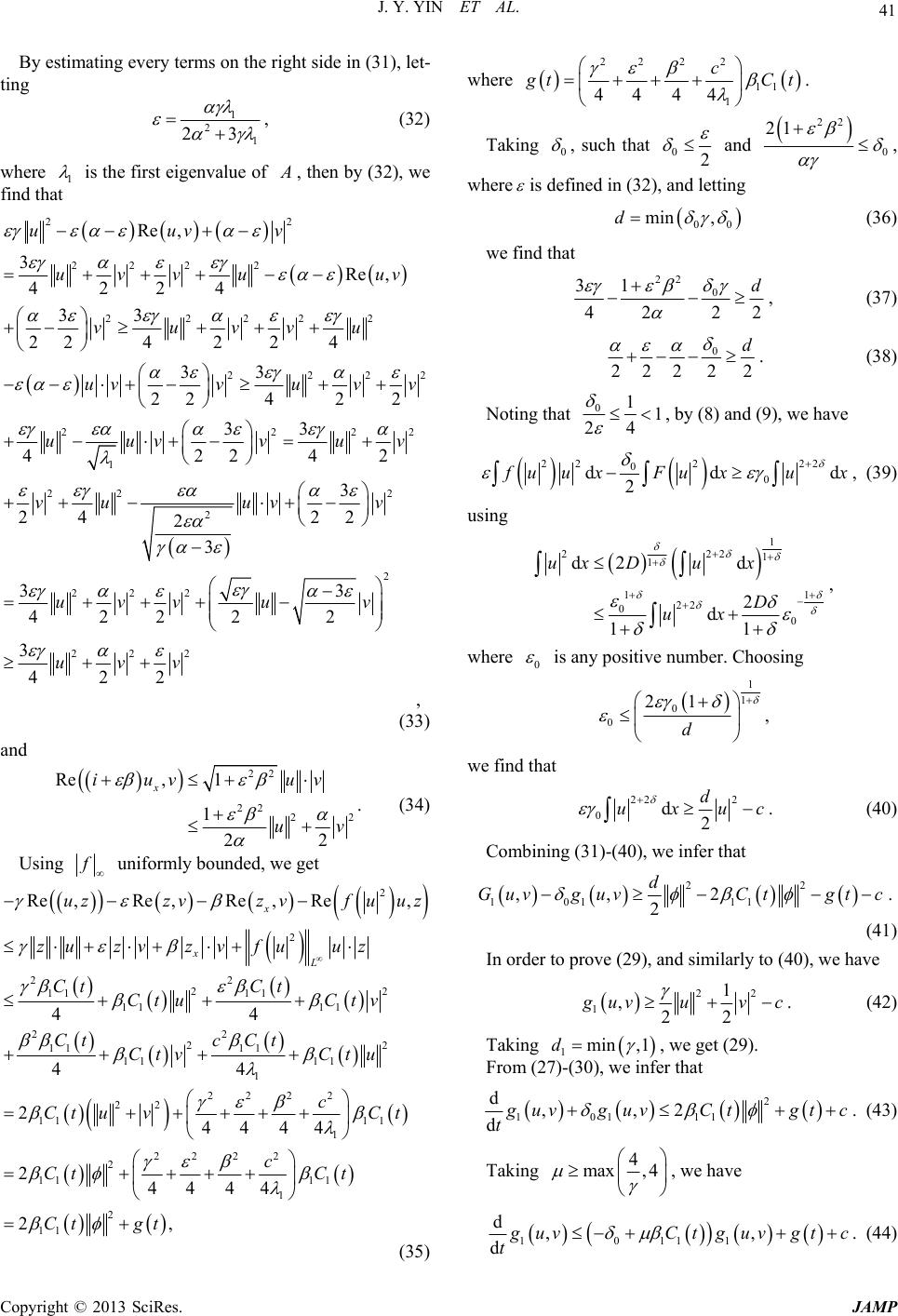 J. Y. YIN ET AL. 41 By estimating every terms on the right side in (31), let- ting 1 21 23 , (32) where 1 is the first eigenvalue of , then by (32), we find that 22 2222 2 2 1 22 2 Re , 3Re, 4224 242 24 33 2242 2 242 24 2 3 uuvv uvvuuv vuvvu uvvuvv uvvuv vu uv 22 222 33 2 2 22 222 33 42 u 2 2 222 222 3 22 33 422 22 3 422 v uvvuv uvv , (33) and 22 22 22 Re, 1 1 22 x iuv uv uv . (34) Using uniform l y bounded, we get 2 2 22 11 11 11 11 22 2 11 11 11 11 1 2 11 1 222 2 2 11 1 Re ,Re,Re ,Re, 44 44 4444 24444 x xL uzzvz vfuuz zuzvz vfuuz Ct Ct Ctu Ctv Ctc Ct CtvCt u Ct c Ct 22 2 u 22 2 22 11 2c Ct v 2222 11 1 4444 c tC . 11 2 11 2, Ct Ct gt (35) where t Taking and 22 0 21 , , such that 02 0 where isned in (32), and letting defi 00 min ,d (36) we find that 22 0 31 42 2 d 2 , (37) 0 22222 d . (38) Noting that 011 24 , by (8) and (9), we have 22222 f 00 ddd 2 uuxFuxux , (39) using 1 222 1 122 0d 11 ux 1 d2 dux Dux 1 0 2D , wh ere 0 is any positive number. Choosing 1 1 0 0 21 d , we find that 22 2 dd xuc. 02 u (40) Combining (31)-(40), we infer that 22 1 Gu 0 1 11 ,, 2 2 d vguvCtgtc . (41) In order to prove (29), and similarly to (40), we have 22 11 ,22 uvuv c . (42) ng Taki 1min,1d , we get (29). From (r that 27)-(30), we infe 2 101 11 d,,2 d uvguvCtg tc t . (43) Taking 4 max ,4 , we have 10111 ,, d d uvCtg u t v gt c . (44) C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 42 Putting 101101 1 m j j ft Ctzt , th (45) en by the Gronwall Lemma, we get, fo r t 1d 11 00 ,e , tt f guvguvg 1d e d tfss c . (46) In particular, we have, for 1, 0t, 1 , 01 01 d 11 100 0d 1 ,e, ed f fss guv cguv cg c , (47) where 0 1ec 10, , in view of the following fact: for t 00 111 000 101 0 ddd dd d eee ee ee tt t fff ff . To estimate all integration terms on the righ (47), we choos e t side in 0 such that 0 11 1 1 00 2 m j j ECE z . This is possible since by (12), 0 10EC as . Thus, since j zt ry get is stationaic, it and ergod is easy to 0 1101 1 limd 02 fEfEC 0 1 0 (48) ps th (49) By (13), , which imlieat 01d lim e0,. . fas 0 j z as , thus Ct 1 further and t is atst 1-times p moolynomoal growth at , whether with (49), implies that ..as (50) an ich, tog 01 0d 1ed, fss qgc d also implies that 012 d 2 q 1 supe fz . (51) Noting that 222 100 0 00 11 ,d 222 uvuvFux , 0010 ,uuu z 2 , and , we get 1 0 uH, 1 uL 2 100 0 ,1guvc llow , (52) then it fos from (29) and (47)-(52), we obtain that 0 01 01 0 2 22 d 10 10 d 1 2 e1 ed . E f fss cc uu uz cg cc 2 1,;, dt 0 We now take (53) 0 22e 11 2 11 2 2c cq cq dd and choose B T such that 0122 d 010 e1 fuuu , for all B T , then we get 1,0. (54) 0 22 01 ,;, , E tt 4.2. Absorbing Set in 1 In order to prove the absorption property in , we also need the following change for (18). Differentiating (18) with respect to 1 E and letting u , v , T T ,, xx uv , we have T ,, ,,,,, F t , L (55) where II L II , 2 2 , . x xxx xx z iz zfu u fu 22 u 2 fu F We now can prove the absorption of RDS (defined by (17)) in 1 E. Lemma 4.2 For anyded set B, there atisfying th no random boun exists a random variable se fol- lowing property: for ev there exists 20 ery 01 , T 0 B, 1 B T B T , such that, for any , the followingolds estimate h..as 1 02 ,;,, 1,0. E tt Proof. Taking the inner product of (55)ith w in , we obtain that 0 E C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 43 2 1d ,, 2d LF t, . (56) Taking the real part of (56), we find that 22 2 Re ,Re ,, Re,Re, . xxx xxx zz uu fu 222 2 2 2 1d 1Re , 2d e,Re , Re t i f fuu Re , Re ,Rz (57) Due to 22 1d 11 Re, 2d z t ,58) 1Re ,1Re ,tx x z ( and 22 22 222 22 222 222 22 Re, Re, 1d dRe 2d Re dd Re, , tx t t x fu ufu uz d uuxfuuux t uuuuxfuux fu uz (59) and 22 2222 22 2 Re ,Re , 1d dRed 2d dRe,. tx t x fu fu z uxfu uux t fuxfu z (60) view of (57)-(60), we get that In 22 222 22 2 2 22 22 222 222 22 22 2 d11 d22 2 1Re , 2 Re ,Re, Re ,Re,Re, 2Red Re dRe , dRe, 0. xx xxx t x x fu udx t fu dx zi zzfuu d t uuux fuuuux fu uxfu uz fuxfu z (61) Letting 22 222 2 22 11 ,d 222 1d 2 fu ux fux (62) and 2, 22 22 2 2 222 222 22 22 2 Re ,Re , Re,Re ,Re, Re, 2Red Re dd Re ,d Re , x xx xx t t x x G z izz fuufuuu x fuuuux fuux fu uzfux fu z (63) Then it comes from (61)-( 63) that 22 d,, dgG t 0 . (64) ilarly to the above arguments (Lemma wrove that there exist Now sim e can p4.1), 20 , and , such that 20d 30d 222 22 2 211 ,, ,2 2 Gg dCtgt c 0 , (65) 2 3 2,2 d c , (66) where 1 followi is defined by (15) and will be defined in the ng paper, therefore we)( 0tg have 222 2 d,, dgg t, . (67) In fact, 2222 22 22 2 222 22 222 22 ,,, Re , Re,Re,Re , 2Red Re, 22 d 22 xxx tt x Gg izz 22 Re , Re, xx zf uu 22 Red 22 2 22 22 dRe , d x uuux fuu ffu z fu ux uux fu uxfu uz u x 22 d.fu x (68) C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 44 2 , 2 d 0 , d respectivelyTaking are the same as in (36) and using , t u, u , , , 22 uf and uniformly boun in time, we ma- uded joring every term on the right side in (68) to get 22 222 2 2 222 2 2 222 2 2 1 222 Re , 3 4224 3 Re ,22 3 4224 3 22 3 4224 3 22 3, 422 (69) where is defined by (32) and 1 is the first eigen- value of , and whae ve 22 , 1 22 22 1 x Re 4 i (70) and , 222 22 2 22 22 11 11 11 11 22 11 11 11 22 2 2 11 11 2 11 0 Re,Re ,Re, Re, Re, || 2 84 4 284 4 2, xx xx xx xx xx xx LL zz z fuzfuuz zz z fuzf uuz Ct Ct Ct Ct Ct CtcCt Ctc Ct Ct gt (71) where 22 2 011 84 4 tcCt . By 4 12 23 x Lc 22 222 2 Re , , 4 L fuu fu uc (72) 4 22 22 2 2 2Red 28 t t LL L fuuu x d fu uuc 2 and young inequality, we have 2 , (73) 4 222 22 22 2 Re d 8 t t LL L fuu uux d fuu uuc 2 , (74) 4 222 2 22 22 22 d 2 28 L L fu ux d fu uc 2 , (75) 4 22 2 22 2 22 d 2 28 L L fux d fu c 2 . (76) it follows from (68)-(76) that Then 222 22 2110 22 2110 ,, 2 2 2 2 Gg dCtgt c dCtgt c 2 . (77) Similarly, with 3min ,1d , we can easily derive that 2 3 2,2 d c . (78) From (64)-(67), we inf e r that 2 222 110 d,,2 d gCtg t tc (79) it follows from (44) that, for the sam , e , we have 221120 d,, d Ct gg t t c (80) Putting . 221121 1 m j j tCt zt then by the Gronwall Lemma, we have, for , (81) t 2d 2200 ,e , f gg 2d 0ed t t tfss gc . (82) C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. 45 1, 0t , 1 , The same as (47), we have, for 02 02 d 21 200 0d 10 ,e , ed f fss gcg cg c (83) where 2 1ec , and 0 10 edc 2 0d fss qg , (84) 022 d 21 supe f x qz . (85) Noting that 200 22 222 00 000 22 22 2 100 , 11 d d 2 g uux fux , 0010 ,x z , we get 2 200 0 ,gc 1 , (86) then from (66) an d (83)- (86), we ob t ai n that 02 0d ed fss cg c 0 02 2 20 222 d 1010 10 ,;, 2 e()1 E f x dt cc z c . (87) We now take 2 2 212 22 2e 2 2c cq cq dd B T and choose such that 0222 d 010 e1 f , for all B T , then we get 1 22 02 ,;t ,, 1,0 Et . (88) 4.3. The Compactness in 0 In this subsection, we prove the compactness in through the decomposition of solution semigroup. Let be a solution of (5) with initial value 0 E ut 0 u T 01 ,uu z 12 utyt, w y t . We make the ecomposition here d 1 t and 2 t satisfy and 11 11 1011 0, ,,, t txtxx t dyydtydtydtiydt yx uxyx u 1 , x x (89) 11 ,, . yxLt yxLt 2 , 22222 2 1 22 2 , ,0, ,0, ,. t txtxxx m j j t dyy dtydtydtiydt uudt hdW yxyx yxLt (90) Lemma 4.3 For any no random bound have, for a ny yxLt ed set B, we 0 T 1 0 ,uu uB 0 3 222 1111 22 10 0000 2e t E Yyyy uuu , (91) and there exists a random variable 0 1 d 30 such that ..as for 0 22 22 0,; ,,DY Y3 E , (92) spect- Proof. Taking the whose initial va afte putation similarly as Lemobtain (9 Taking the inner product of (90) with where T 1111 ,t Yyy y and T 222 ,Yyy yz satisfy (89) and (90) re 2 inner product of (89) with 1 Y in lue is T 0 u r a simple t tively. 0 E com 01 ,uu , ma 4.1, we 1). 2 Y in 0 E T 0, z , ma 4.2, we whose initial value is after a s putation similarly as Lemobtain imp (92). le com- Let 12 B be the ball of of random ble 21 1 EH H varia 30 21 10 H E . Frombedding the compact em 21 EH HL , we see that 12 B is comp random bact. For any noounded set of , pick 0 E any tB . Fr0, t om Lemma 4.3, we have 21 00YY 12 0B , where ,t 2 Y is giveereforen by Th Lemma 4.3., again by Lemma 4.3, 3 0 2 222 010 1 inf00 2e,0. E Euuu d 0 12 0 1 0 B Y So 12 ,, 0,as. t disttB Bt Corolla ry 4.4 The RDS ,t associated with (17) pact set possesses a uniformly attracting com 12 0 BE ,t .2, Lem , so the RDS is uniformly asymp- totically compact in By applying Th 2.2ma 4.1 obtain the final con this whole pa per . 4.5 Assum S 0 E. eorem and corollary 4.4, weclusion of Theorem e j hDAHH, then the RD 12 modeling po the dissipative Hamiltonian am- plitude equation governing modulated wave instabilities ssesses a compact random attractor which C opyright © 2013 SciRes. JAMP  J. Y. YIN ET AL. Copyright © 2013 SciRes. JAMP 46 att 2 racts all bounded sets of 1 0 EHL , “So . dati, “Solitons in e Physical Society . J. Ablowitz, ation Governing M the Ph . 1187-1193. . 5. ou M. Tanaka an d N. Ya ji nt Vol. 94, 1988, pp. 138-162 [2] T. Yajima and M. Wa an Unstable dium,” Journal of thof Japan, V 1987, pp. 3069-3081. [3] M. Wadati, H. Segur and M “A New Ham- tonian Amplitude Equodulated W tabilities,” Journal of ysical Society of Japan Vol. 61, No. 4, 1992, pp Acknowledgements The authors would like to express their sincere thanks to theonym/her valuable ans referee for his comments and suggestions toimprove the paper. REFERENCES [1] ma liton Modes in an Unstable Plasma Nonlinear Phenomena in an Electron-Beam Plasma,” Progress of Theoretical Physics Suppleme, Me- . 56, ol il Ins ave , doi:10.1143/JPSJ.61.1187 [4] B. L. Guo and Z. D. Dai, “Attractor for the Dissipative Hamiltonian Amplitude Eqation Governing Modulated Wave Instabilities,” Disc Continuous Dynamical Systems, Vol. 4, No. 4, 1998, pp. u rete and 783-793. doi:10.3934/dcds.1998.4.783 [5] Z. D. Dai, “Regularity of the Attractor for the Dissipative Hamiltonian Amplitude Edulated Wavestabilities,” Acta Mathematicae Applicatae Sinica (English Series), Vol. 18, No. 2, 2002, pp. 263-272. quation Governing Mo In doi:10.1007/s102550200025 [6] Z. D. Dai, L. Yang and traJ. Huang, “Atctor for th sion of Global At- uations Governing Modulated perturbed Dissipative Hamiltonian Amplitude Wave,” Acta Mathematicae Applicatae Sinica, Vol. 27, No. 4, 2004, pp. 577-592. [7] L. Yang and Z. D. Dai, “Finite Dimen tractors for Dissipative Eq Wave,” Applied Mathematics: A Journal of Chinese Uni- versities, Vol. 18, No. 4, 2003, pp. 421-430. doi:10.1007/s11766-003-0069-3 [8] Y. R. Li and B. L. Guo, “Random Attracto nesq Equations with Multiplirs of Boussi- cative Noise,” Acta Mathe- maticae Sinica (English Series), Vol. 25, No. 3, 2009, pp. 481-490. doi:10.1007/s10114-008-6226-0 [9] Y. R. Li, and B. L. Guo, “Random Attractors for Quasi- Continuous Random Dynamical Systems and App tions to Stochastic Reaction-D lica- iffusion Equations,” Jour- nal of Differential Equations, Vol. 245, No. 7, 2008, pp. 1775-1800. doi:10.1016/j.jde.2008.06.031 [10] X. M. Fan, “Random Attractor for a Damped Sine- Gordon Equation with White Noise,” Pacific Journal of Mathematics, Vol. 216, No. 1, 2004, pp. 63-76. doi:10.2140/pjm.2004.216.63 [11] H. Crauel and F. Flandoli, “Attracors for Random Dy- namical Systems,” Probability Theory and Rel Vol. 100, No. 3, 1994, pp. 365-393.ated Fields, doi:10.1007/BF01193705 [12] H. Crauel and F. Flandoli, “Random Attractors,” Journal of Dynamics and Differential Equations, Vol. 9, No. 2, 1997, pp. 307-341. doi:10.1007/BF02219225 [13] L. Arnold, “Random Dynamical Systems,” Springer- Verlag, New York, 1998. [14] R. Temam, “Infinite-Dimensional Dynamical System in Mechanics and Physics,” Springer-Verlag, New York, 1988, pp. 90-226. doi:10.1007/978-1-4684-0313-8 e Un-
|