Circuits and Systems, 2011, 2, 1-6
doi:10.4236/cs.2011.21001 Published Online January 2011 (http://www.SciRP.org/journal/cs)
Single MO-CCCCTA-Based Electr onically Tunable
Curr ent/Tr ans-Impedance-Mode Biquad Universal Filter
Sajai Vir Singh1, Sudhanshu Maheshwari2, Durg Singh Chauhan3
1Department of Electronics and Communications, Jaypee University of Information Technology,
Waknaghat, India
2Department of Electronics Engineering, Zakir Hussain College of Engineering and Technology,
Aligarh Muslim University, Aligarh, India
3Department of Electrical Engineering, Institute of Technology, Banaras Hindu University, Varanasi, India
Received June 12, 2010; revised July 9, 2010; accepted July 19, 2010
Abstract
This paper presents an electronically tunable current/trans-impedance-mode biquad universal filter employing
only single multi-output current controlled current conveyor trans-conductance amplifier (MO-CCCCTA) and
two grounded capacitors. The proposed filter realizes all the standard filter functions i.e. low pass (LP), band
pass (BP) and high pass (HP), notch and all pass (AP) filters in the current form at high impedance output
through appropriate selection of the input signals, without any matching conditions. Simultaneously, it can also
realize all the standard filter functions in trans-impedance form from the same circuit topology. The circuit does
not require inverting-type input current signal(s) and double input current signal(s) to realize all the responses
in the design. The validity of proposed filter is verified through PSPICE simulations.
Keywords: Universal, Current-Mode, Trans-Impedance-Mode, Biquad Filter
1. Introduction
Analog electronic filters are important blocks, widely
employed in continuous time signal processing. They
are present in just about every piece of electronic equip-
ment that are obvious types of equipments, such as radios,
televisions and stereo systems. Test equipments such as
spectrum analyzers and signal generators also need fil-
ters even where signals are connected into digital form,
using digital to analog converters; analog filters are
usually needed to prevent aliasing. Universal biquadratic
filters belong to most popular analog filters, providing
all standard filter functions (LP, BP, HP, Notch and AP),
without modifying the circuit topology. Several filter
realizations either in current-mode, where the input and
the output variables are current, or in voltage-mode,
where the input and output variables are voltage, have
been reported using different active elements [1-22].
These filter circuits are classified as single input mul-
tiple output (SIMO) [1-10], multiple input single output
(MISO) [11-19] and multiple input multiple output
(MIMO) [20-22]. However, there are a number of ap-
plications in analog signal processing where it may be
desirable to have active filters with input variable as
current and output variable as voltage that is trans-
impedance filters. Such filters can be used as an inter-
face circuit connecting a current-mode circuit to a vol-
tage-mode circuit and find direct applications with some
sensors, the receiver base band (BB) blocks of modern
radio systems and D/A converters which provide a cur-
rent as output signal, avoiding a current to voltage con-
version [23,24]. There are a small number of filter to-
pologies operating in trans-impedance-mode reported in
the literature [25-27]. These filter topologies reported in
[24-26] cannot realize all the standard filter functions
(LP, BP, HP, Notch and AP). As far as the topic of this
paper is concerned, the filter circuits operated in either
current-mode or trans-impedance-mode or in both
modes simultaneously, using a single active element,
are of interest. Single active element based current-mode
filters with multi-input are reported in [18-20]. The cir-
cuits in references [18,19] use three inputs and one out-
put and realize all the standard filter functions at high
impedance output terminal. The filter circuit of [18]

S. V. SINGH ET AL.
2
employs single CCCII, two grounded capacitors and
one floating resistor and suffers from the following
disadvantages: 1) requirement of passive component
matching conditions, 2) requirement of inverting-type
input current signal, 3) use of floating resistor which is
not suitable for IC fabrications while other filter circuit
of [19] uses single CCCCTA, two grounded capacitors
and suffers from the following two disadvantages: re-
quirement of double input current signal to obtain an
all-pass response and use of one capacitor at port X
which limits the use of filter in high frequency range
since it effectively appears in series with X terminal re-
sistance [28]. Lastly, a three inputs and two outputs
current-mode single DO-CCCDTA based filter circuit
[20] also realizes all the standard filter functions at high
impedance outputs but it still require double input cur-
rent signal to obtain an all-pass response. Up until now,
no previous paper has reported a filter based on single
active element which can realize all the standard res-
ponses in current as well as trans-impedance form, to-
gether, without any matching conditions, from the
same topology. In this paper a single MO-CCCCTA-
based electronically tunable current/trans-impedance-
mode biquad universal filter is proposed. It also uses
two grounded capacitors. The proposed filter realizes all
the standard filter functions i.e. LP, BP, HP, notch and
AP filters in the current form at high impedance output
through appropriate selection of the input signals, with-
out any matching conditions. Simultaneously, it can also
realize all the standard filter functions in trans-impe-
dance form from the same circuit topology. The pro-
posed circuit does not require inverting-type input cur-
rent signal(s) and double input current signal(s) to real-
ize all the responses in the design. The proposed circuit
does not use capacitor at port X so this circuit is suitable
in high frequency range. The circuit possesses low ac-
tive and passive sensitivity. Moreover, the pole fre-
quency (ωo) can be independently tuned without dis-
turbing the parameter ωo/Q through adjusting the bias
current of MO-CCCCTA. The performance of proposed
circuit is illustrated by PSPICE simulation using 0.35 µ
CMOS parameters.
2. Proposed Circuit
CCCCTA is relatively new proposed current mode active
building block [19] which is the modified version of CCTA.
This device can be operated in both current and voltage
modes, providing flexibility. In addition, it can offer sever-
al advantages such as high slew rate, high speed, wider
bandwidth and simpler implementation. Moreover, in the
CCCCTA one can control the parasitic resistance at X (RX)
port by input bias current. The MO-CCCCTA properties
can be described in the following matrix equation
00 00 00
10 000
10 00 00
10 0000
000 00
00 000
YX
Xx Y
Za, ZcZb
Z
bZc
Ob mbOb
Ocmc Oc
II
VR V
IV
=
IV
IgV
IgV





 

 

 

 


 

 

 


 


 

 
(1)
where RX is the parasitic resistance at X
terminal. gmb and
gmc are trans-conductance of CCCCTA. The schematic
symbol of MO-CCCCTA is illustrated in Figure 1.
CMOS implementation of MO-CCCCTA is shown in
Figure 2 . For a CMOS CCCCTA [29], the R
X
, g
mb
and
g
mc
can be expressed to be
8
X
nB
1
R= β
I
, mbn Sb g=β
I
andmcn Sc g=β
I
(2)
where
nnOX
W
CL
  (3)
where μ
n
, C
OX
and W/L are the electron mobility, gate
oxide capacitance per unit area and transistor aspect
ratio, respectively. IB, ISb and ISc are the biasing currents
of MO-CCCCTA.
The proposed biquad filter circuit as shown in Figure
3 uses only single MO-CCCCTA and two grounded ca-
pacitors. By routine analysis of the circuit in Figure 3,
the output current IO and output voltage VO can be ob-
tained as
2
112223
2
12 2
mcmbmc
O
mcmb mc
I
sCCIsCgIg g
IsCCsCgg g

 (4)


2
11222 3
2
12 2
x
mcmb mc
O
mcmb mc
RIsCC IsCgIgg
VsCCsCgg g

 (5)
From Equations (4) and (5), various filter responses in
current form as well as in trans-impedance form can be
Figure 1. MO-CCCCTA symbol.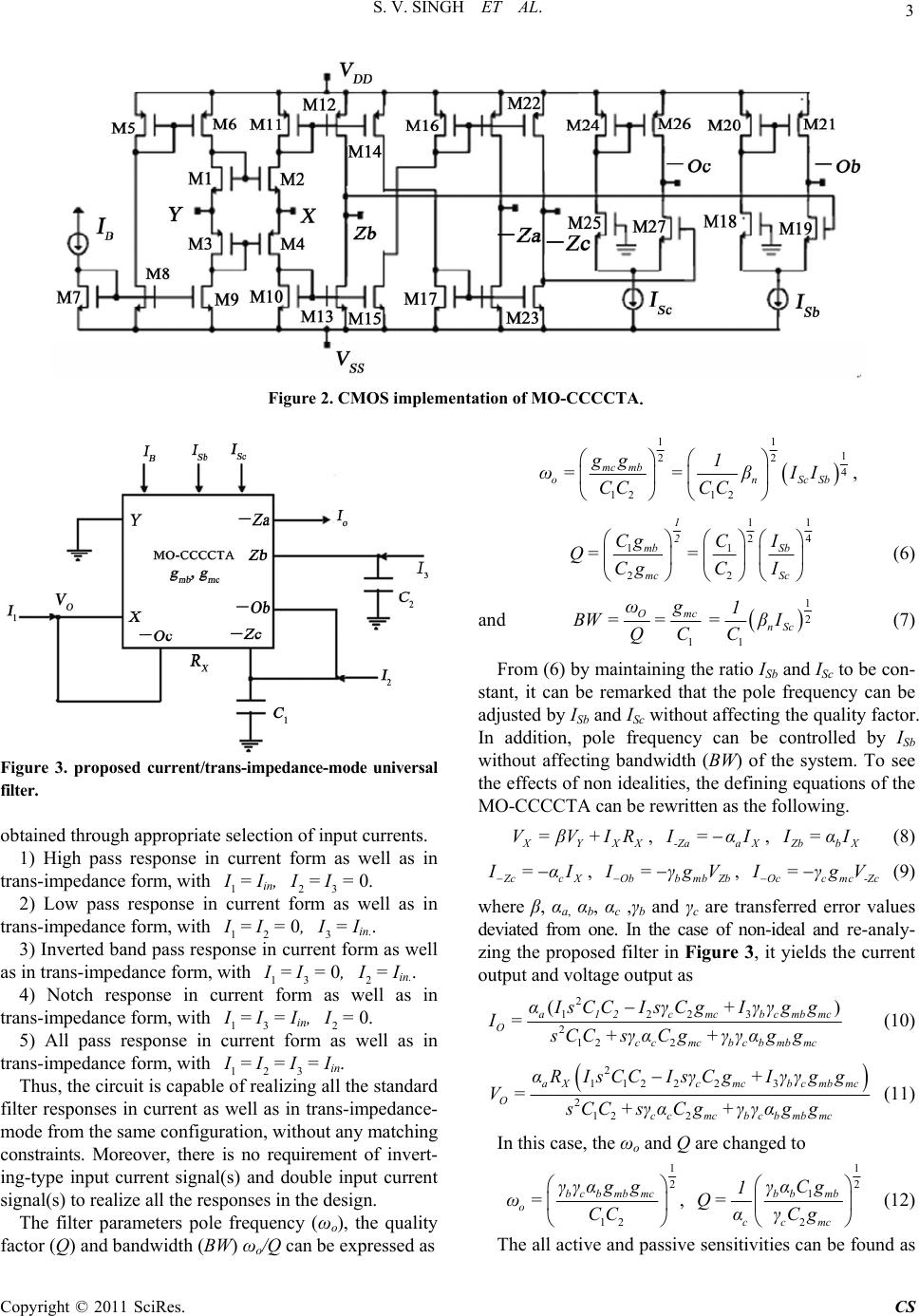

S. V. SINGH ET AL.
3
Figure 2. CMOS implementation of MO-CCCCTA.
Figure 3. proposed current/trans-impedance-mode universal
filter.
obtained through appropriate selection of input currents.
1) High pass response in current form as well as in
trans-impedance form, with 1
I
= Iin, 2
I
=3
I
= 0.
2) Low pass response in current form as well as in
trans-impedance form, with 1
I
=2
I
= 0, 3
I
= Iin..
3) Inverted band pass response in current form as well
as in trans-impedance form, with 1
I
=3
I
= 0, 2
I
= Iin..
4) Notch response in current form as well as in
trans-impedance form, with 1
I
=3
I
= Iin, 2
I
= 0.
5) All pass response in current form as well as in
trans-impedance form, with 1
I
=2
I
=3
I
= Iin.
Thus, the circuit is capable of realizing all the standard
filter responses in current as well as in trans-impedance-
mode from the same configuration, without any matching
constraints. Moreover, there is no requirement of invert-
ing-type input current signal(s) and double input current
signal(s) to realize all the responses in the design.
The filter parameters pole frequency (ωo), the quality
factor (Q) and bandwidth (BW) ωo/Q can be expressed as
1
2
12
mc mb
o
gg
ω=CC




1
1
2
4
12
nScSb
1
=βII
CC


 ,
1
2
1
2
mb
mc
Cg
Q= Cg



1 1
4
2
1
2
Sb
Sc
I C
=CI
 
 
 
(6)
and
1
Omc
ω
g
BW ==
QC

1
2
1
nSc
1
=βI
C (7)
From (6) by maintaining the ratio ISb and ISc to be con-
stant, it can be remarked that the pole frequency can be
adjusted by ISb and ISc without affecting the quality factor.
In addition, pole frequency can be controlled by ISb
without affecting bandwidth (BW) of the system. To see
the effects of non idealities, the defining equations of the
MO-CCCCTA can be rewritten as the following.
XYXX
V=βV+IR , -Zaa X I=α
I
,
Z
bbX
I=α
I
(8)
Z
ccX
I=α
I


, Obbmb Zb I=γ
g
V


, Occmc-Zc I=γ
g
V
 (9)
where β, αa, αb, αc ,γb and γc are transferred error values
deviated from one. In the case of non-ideal and re-analy-
zing the proposed filter in Figure 3, it yields the current
output and voltage output as
2
1223
2
12 2
()
a12cmcb cmbmc
O
ccmcbcbmb mc
αIsCCI sγCg +Iγγgg
I= sCC +sγαCg +γγαgg
 (10)


2
1122 23
2
12 2
aXc mcbcmbmc
O
ccmcbcb mb mc
αRIsCC IsγCg+Iγγgg
V= sCC+sγαCg+γγαgg

(11)
In this case, the ωo and Q are changed to
1
2
12
bcbmbmc
o
γγαgg
ω=CC



,
1
2
1
2
bb mb
ccmc
γαCg 1
Q= αγCg



(12)
The all active and passive sensitivities can be found as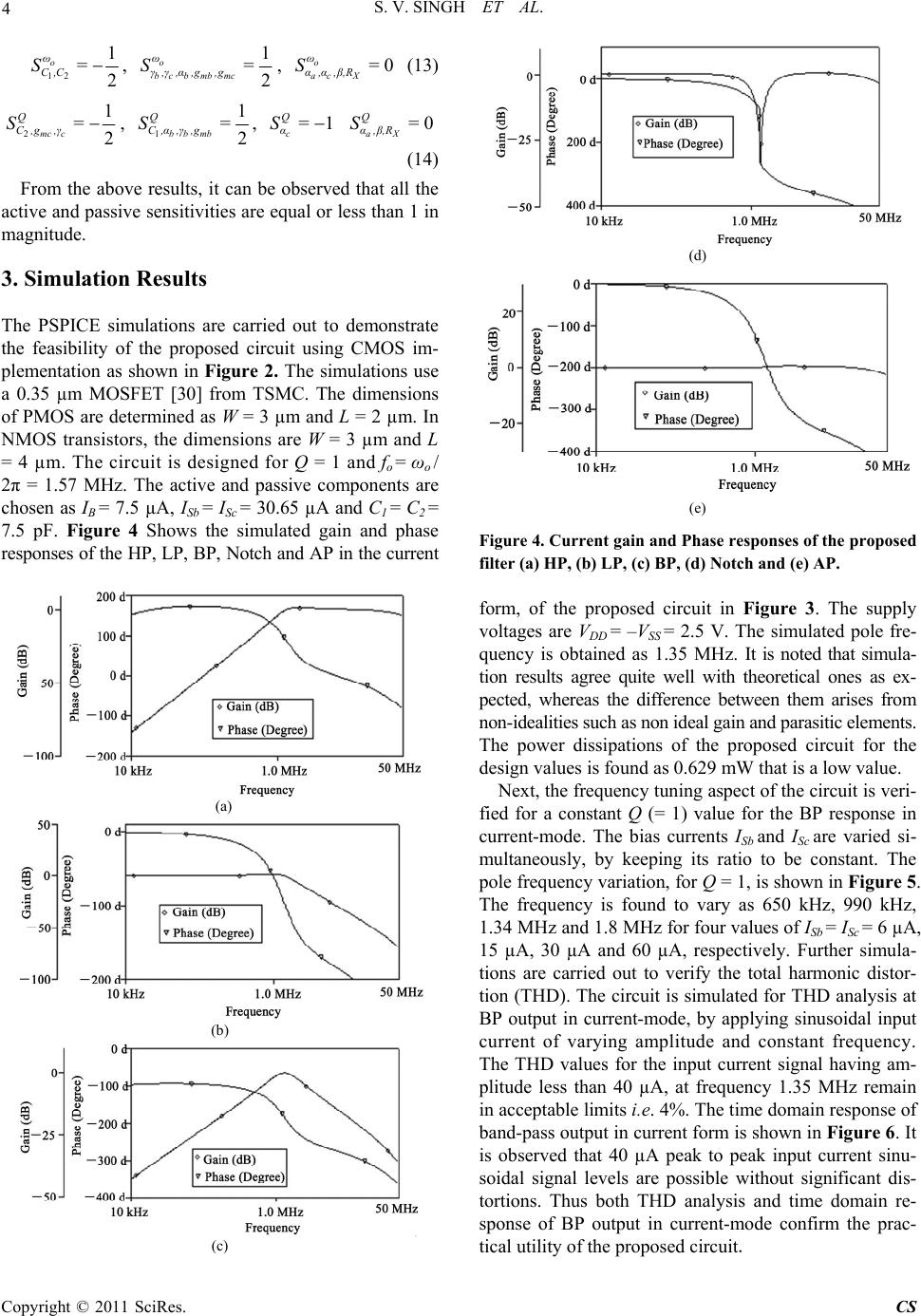

S. V. SINGH ET AL.
4
12
1
2
o
ω
C,C
S=, 1
2
o
bc bmbmc
ω
γ,γ,α,g ,g
S=, 0
o
ac X
ω
α,α,β,R
S= (13)
2
1
2
mc c
Q
C,g ,γ
S=, 1
1
2
bb mb
Q
C,α,γ,g
S=, 1
c
Q
α
S= 0
aX
Q
α,β,R
S=
(14)
From the above results, it can be observed that all the
active and passive sensitivities are equal or less than 1 in
magnitude.
3. Simulation Results
The PSPICE simulations are carried out to demonstrate
the feasibility of the proposed circuit using CMOS im-
plementation as shown in Figure 2. The simulations use
a 0.35 µm MOSFET [30] from TSMC. The dimensions
of PMOS are determined as W = 3 µm and L = 2 µm. In
NMOS transistors, the dimensions are W = 3 µm and L
= 4 µm. The circuit is designed for Q = 1 and fo = ωo /
2π = 1.57 MHz. The active and passive components are
chosen as IB = 7.5 µA, ISb = ISc = 30.65 µA and C1 = C2 =
7.5 pF. Figure 4 Shows the simulated gain and phase
responses of the HP, LP, BP, Notch and AP in the current
(a)
(b)
(c)
(d)
(e)
Figure 4. Current gain and Phase responses of the proposed
filter (a) HP, (b) LP, (c) BP, (d) Notch and (e) AP.
form, of the proposed circuit in Figure 3. The supply
voltages are VDD = –VSS = 2.5 V. The simulated pole fre-
quency is obtained as 1.35 MHz. It is noted that simula-
tion results agree quite well with theoretical ones as ex-
pected, whereas the difference between them arises from
non-idealities such as non ideal gain and parasitic elements.
The power dissipations of the proposed circuit for the
design values is found as 0.629 mW that is a low value.
Next, the frequency tuning aspect of the circuit is veri-
fied for a constant Q (= 1) value for the BP response in
current-mode. The bias currents ISb and ISc are varied si-
multaneously, by keeping its ratio to be constant. The
pole frequency variation, for Q = 1, is shown in Figure 5.
The frequency is found to vary as 650 kHz, 990 kHz,
1.34 MHz and 1.8 MHz for four values of ISb = ISc = 6 µA,
15 µA, 30 µA and 60 µA, respectively. Further simula-
tions are carried out to verify the total harmonic distor-
tion (THD). The circuit is simulated for THD analysis at
BP output in current-mode, by applying sinusoidal input
current of varying amplitude and constant frequency.
The THD values for the input current signal having am-
plitude less than 40 µA, at frequency 1.35 MHz remain
in acceptable limits i.e. 4%. The time domain response of
band-pass output in current form is shown in Figure 6. It
is observed that 40 µA peak to peak input current sinu-
soidal signal levels are possible without significant dis-
tortions. Thus both THD analysis and time domain re-
sponse of BP output in current-mode confirm the prac-
tical utility of the proposed circuit.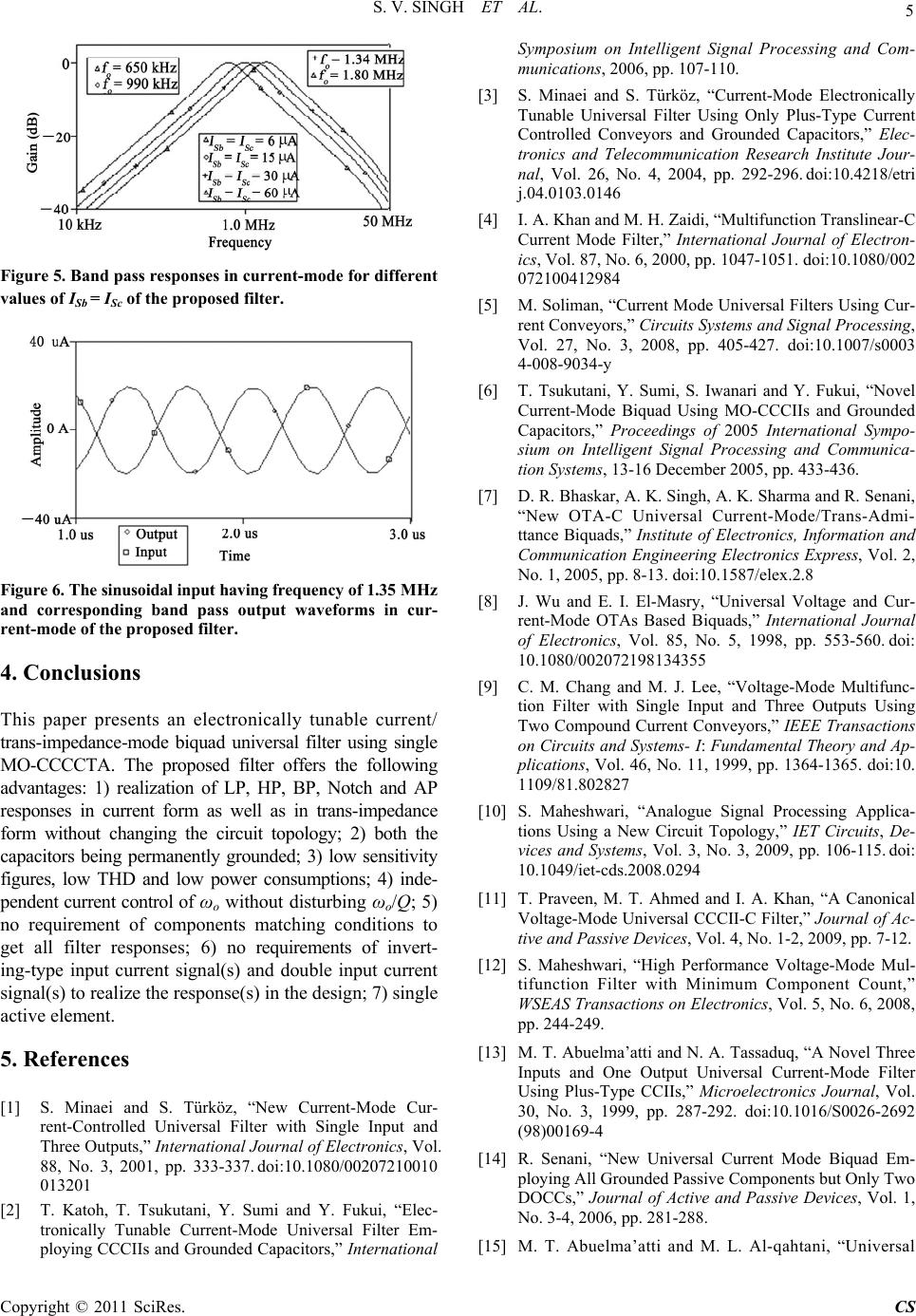

S. V. SINGH ET AL.
5
Figure 5. Band pass responses in current-mode for different
values of ISb = ISc of the proposed filter.
Figure 6. The sinusoidal input having frequency of 1.35 M H z
and corresponding band pass output waveforms in cur-
rent-mode of the proposed filter.
4. Conclusions
This paper presents an electronically tunable current/
trans-impedance-mode biquad universal filter using single
MO-CCCCTA. The proposed filter offers the following
advantages: 1) realization of LP, HP, BP, Notch and AP
responses in current form as well as in trans-impedance
form without changing the circuit topology; 2) both the
capacitors being permanently grounded; 3) low sensitivity
figures, low THD and low power consumptions; 4) inde-
pendent current control of ωo without disturbing ωo/Q; 5)
no requirement of components matching conditions to
get all filter responses; 6) no requirements of invert-
ing-type input current signal(s) and double input current
signal(s) to realize the response(s) in the design; 7) single
active element.
5. References
[1] S. Minaei and S. Türköz, “New Current-Mode Cur-
rent-Controlled Universal Filter with Single Input and
Three Outputs,” International Journal of Electronics, Vol.
88, No. 3, 2001, pp. 333-337. doi:10.1080/00207210010
013201
[2] T. Katoh, T. Tsukutani, Y. Sumi and Y. Fukui, “Elec-
tronically Tunable Current-Mode Universal Filter Em-
ploying CCCIIs and Grounded Capacitors,” International
Symposium on Intelligent Signal Processing and Com-
munications, 2006, pp. 107-110.
[3] S. Minaei and S. Türköz, “Current-Mode Electronically
Tunable Universal Filter Using Only Plus-Type Current
Controlled Conveyors and Grounded Capacitors,” Elec-
tronics and Telecommunication Research Institute Jour-
nal, Vol. 26, No. 4, 2004, pp. 292-296. doi:10.4218/etri
j.04.0103.0146
[4] I. A. Khan and M. H. Zaidi, “Multifunction Translinear-C
Current Mode Filter,” International Journal of Electron-
ics, Vol. 87, No. 6, 2000, pp. 1047-1051. doi:10.1080/002
072100412984
[5] M. Soliman, “Current Mode Universal Filters Using Cur-
rent Conveyors,” Circuits Systems and Signal Processing,
Vol. 27, No. 3, 2008, pp. 405-427. doi:10.1007/s0003
4-008-9034-y
[6] T. Tsukutani, Y. Sumi, S. Iwanari and Y. Fukui, “Novel
Current-Mode Biquad Using MO-CCCIIs and Grounded
Capacitors,” Proceedings of 2005 International Sympo-
sium on Intelligent Signal Processing and Communica-
tion Systems, 13-16 December 2005, pp. 433-436.
[7] D. R. Bhaskar, A. K. Singh, A. K. Sharma and R. Senani,
“New OTA-C Universal Current-Mode/Trans-Admi-
ttance Biquads,” Institute of Electronics, Information and
Communication Engineering Electronics Express, Vol. 2,
No. 1, 2005, pp. 8-13. doi:10.1587/elex.2.8
[8] J. Wu and E. I. El-Masry, “Universal Voltage and Cur-
rent-Mode OTAs Based Biquads,” International Journal
of Electronics, Vol. 85, No. 5, 1998, pp. 553-560. doi:
10.1080/002072198134355
[9] C. M. Chang and M. J. Lee, “Voltage-Mode Multifunc-
tion Filter with Single Input and Three Outputs Using
Two Compound Current Conveyors,” IEEE Transactions
on Circuits and Systems- I: Fundamental Theory and Ap-
plications, Vol. 46, No. 11, 1999, pp. 1364-1365. doi:10.
1109/81.802827
[10] S. Maheshwari, “Analogue Signal Processing Applica-
tions Using a New Circuit Topology,” IET Circuits, De-
vices and Systems, Vol. 3, No. 3, 2009, pp. 106-115. doi:
10.1049/iet-cds.2008.0294
[11] T. Praveen, M. T. Ahmed and I. A. Khan, “A Canonical
Voltage-Mode Universal CCCII-C Filter,” Journal of Ac-
tive and Passive Devices, Vol. 4, No. 1-2, 2009, pp. 7-12.
[12] S. Maheshwari, “High Performance Voltage-Mode Mul-
tifunction Filter with Minimum Component Count,”
WSEAS Transactions on Electronics, Vol. 5, No. 6, 2008,
pp. 244-249.
[13] M. T. Abuelma’atti and N. A. Tassaduq, “A Novel Three
Inputs and One Output Universal Current-Mode Filter
Using Plus-Type CCIIs,” Microelectronics Journal, Vol.
30, No. 3, 1999, pp. 287-292. doi:10.1016/S0026-2692
(98)00169-4
[14] R. Senani, “New Universal Current Mode Biquad Em-
ploying All Grounded Passive Components but Only Two
DOCCs,” Journal of Active and Passive Devices, Vol. 1,
No. 3-4, 2006, pp. 281-288.
[15] M. T. Abuelma’atti and M. L. Al-qahtani, “Universal

S. V. SINGH ET AL.
6
Current-Contolled Current-Mode Filter with Three Inputs
and One Output Using Current Controlled Conveyor,”
Active Passive Electronics Components, Vol. 21, No. 1,
1998, pp. 33-41. doi:10.1155/1998/83413
[16] W. Tangsriart and W. Surakampontorm, “Electronically
Tunable Current-Mode Universal Filter Employing Only
Plus-Type Current-Controlled Conveyors and Grounded
Capacitors,” Circuits Systems and Signal Processing, Vol.
25, No. 6, 2006, pp. 701-713. doi:10.1007/s00034-005-0
908-y
[17] W. Tangsrirat and W. Surakampontorm, “Low-Component
Current-Mode Universal Filter Using Current-Controlled
Conveyors and Grounded Capacitors,” Journal of Active
and Passive Devices, Vol. 4, No. 3, 2009, pp. 259-264.
[18] C. M. Chang, “Universal Active Current Filter Using
Single Second-Generation Current Controlled Conveyor,”
International Journal of Circuits, Systems and Signal
Processing, Vol. 1, No. 1, 2007, pp. 194-198.
[19] M. Siripruchyanun and W. Jaikla, “Current Controlled
Current Conveyor Transconductance Amplifier (CCCCTA):
A Building Block for Analog Signal Processing,” Elec-
trical Engineering, Vol. 90, No. 6, 2008, pp. 443-453.
doi:10.1007/s00202-007-0095-x
[20] M. Siripruchyanun and W. Jaikla, “Electronically Con-
trollable Current-Mode Universal Biquad Filter Using
Single DO-CCCDTA,” Circuits System Signal Processing,
Vol. 27, No. 1, 2008, pp. 113-122. doi:10.1007/s00034-0
08-9014-2
[21] W. Tangsriart, “Current-Tunable Current-Mode Multi-
function Filter Based on Dual-Output Current-Controlled
Conveyors,” International Journal of Electronics and
Communications, Vol. 61, No. 8, 2007, pp. 528-533.
doi:10.1016/j.aeue.2006.09.005
[22] T. Tsukutani, Y. Sumi and N. Yabuki, “Versatile Current
Mode Biquadratic Circuit Using Only Plus Type CCCIIs
and Grounded Capacitors,” International Journal of
Electronics, Vol. 94, No. 12, 2007, pp.1147-1156. doi:10.
1080/00207210701789998
[23] Y. Wang and R. Raut, “A Design of Transresistance Am-
plifier for High Gain-Bandwidth Applications,” Pro-
ceedings of the 2003 10th IEEE International Conference
on Electronics, Circuits and Systems, 14-17 December
2003, pp. 185-188.
[24] A. Carlosena and E. Cabral, “Novel Transimpedance
Filter Topology for Instrumentation,” IEEE Transactions
on Instrumentation and Measurement, Vol. 46, No. 4,
1997, pp. 862-867. doi:10.1109/19.650789
[25] M. Soliman, “Mixed-Mode Biquad Circuits,” Microelec-
tronics Journal, Vol. 27, No. 6, 1996, pp. 591-594. doi:10.
1016/0026-2692(95)00121-2
[26] J. Ramirez-Angulo, M. Robinson and E. Sanchez-Sinencio,
“Current-Mode Continuous-Time Filters: Two Design
Approaches,” IEEE Transactions on Circuits and Sys-
tems-II: Analog and Digital Signal Processing, Vol. 39,
No. 6, 1992, pp. 337-341. doi:10.1109/82.145290
[27] S. Minaei, G. Topcu and O. Cicekoglu, “Low Input Im-
pedance Trans-Impedance Type Multifunction Filter Us-
ing Only Active Elements,” International Journal of
Electronics, Vol. 92, No. 7, 2005, pp. 385-392. doi:10.
1080/00207210412331334789
[28] A. Fabre, O. Saiid and H. Barthelemy, “On the Frequency
Limitations of the Circuits Based on Second Generation
Current Conveyors,” Analog Integrated Circuits and
Signal Processing, Vol. 7, No. 2, 1995, pp. 113-129. doi:1
0.1007/BF01239166
[29] M. Siripruchyanun, P. Silapan and W. Jaikla, “Realiza-
tion of CMOS Current Controlled Current Conveyor
Transconductance Amplifier and Its Application,” Jour-
nal of Active and Passive Devices, Vol. 4, No. 1-2, 2009,
pp. 35-53.
[30] E. Yuce, S. Tokar, A. Kizilkaya and O. Cicekoglu,
“CCII-Based PID Controllers Employing Grounded Pas-
sive Componensts,” International Journal of Electronics
and Communications, Vol. 60, No. 5, 2006, pp. 399-403.
doi:10.1016/j.aeue.2005.03.017