 J. Electromagnetic Analysis & Applications, 2011, 3, 27-32 doi:10.4236/jemaa.2011.31005 Published Online January 2011 (http://www.SciRP.org/journal/jemaa) Copyright © 2011 SciRes. JEMAA 27 Correlation of Magnetoresistance and Thermoelectric Power in La1-xLixMnOy Compounds Ahmed Mohamed Ahmed1*, Mahrous Rashad Ahmed1,2, Saad Abed El Rahman Ahmed1 1Physics Department, Faculty of Science, Sohag University, Sohag, Egypt; 2Physics Department, University College, Um-Elqura University, Makkah, KSA. Email: *Fikry_99@yahoo.com Received November 25th, 2010; revised December 1st, 2010; accepted December 20th, 2010. ABSTRACT The temperature dependences of the thermoelectric power, TEP, (S) and magnetoresistance (MR) effect of La1-xLixMnOy (x = 0.05, 0.1, 0.15, 0.2 & 0.25 at %) fixed valence doped compounds were studied between 80K and 320K. X-ray powder diffraction (XRD) showed that the samples are single phase. We found the correlation of structures to the mag- netoresistance (MR) and the thermoelectric power (S) which we assigned to change the specific branches of the magnon /phonon spectra with x. We also observed a splitting of the Curie and the metal insulating temperature TC > Tms. Keywords: Thermoelectric Power, Magnetoresistance, the Curie Temperature 1. Introduction In general, the thermal variation of the electrical resis- tivity for both divalent and monovalent lanthanum man- ganites are known to be dominated by polaronic transport for T > Tc [15,20], while below Tc, electron–electron and electron–magnon interactions are usually believed to do- minate the conductivity [21-23]. Seebeck coefficient has also been subject of several studies [15,21,24-26], par- ticularly in divalent-doped manganites. In the high tem- perature (T > TC), most of the reports on thermoelectric power suggested a small polaron hopping [27,28], while, below TC in the ferromagnetic region obtained from the TEP is governed by the coexistence of both phonon drag and magnon drag effects [26]. Recently, the rediscovery of colossal magnetoresistance (CMR) in perovskite manganese oxides R1-xAxMnO3 (where R is a trivalent rare-earth element and A is a di- valent element such as Ca, Sr, Ba, or Pb) has generated a considerable interest because of their various electronic, magnetic and structural properties and potential applica- tions [1-4]. It has long been thought that the magnetic structure and electronic transport properties of R1-xAxMnO3 are correlated via the double-exchange (DE) mechanism [5], i.e. the hopping of eg electrons between Mn3+ and Mn4+ ions mediated by oxygen anions. However, recent studies have proved that the strong Jahn–Teller type electron–phonon coupling [6], orbital degree of freedom [7,8], cation size mismatch [9-11], oxygen non-stoichiometry [12-14], etc. can strongly influence the behavior of these types of systems under the external conditions such as temperature, magnetic field, electric fields …. etc. In case of doping the compound La +3 1-x A+ 1 x MnO3 by monovalent alkali (A) atoms like Li1+, Na1+, Rb1+ because of larger valence difference between La+3 and alkali-metal ions, fewer alkali-metal ions are required to obtain a specific carrier concentration com- pared to divalent doping and a consequent increase in conductivity is achieved with less inhomogeneity [15-19]. 2. Experimental The polycrystalline La1-xLixMnOy samples (x = 0.05, 0.1, 0.15, 0.2 & 0.25 at %) have been prepared by standard solid state reaction method. Stoichiometric amounts of La2O3, Li2CO3 and MnCO3 powders (all having 99.99% purity) were thoroughly mixed and ground. After grind- ing, the powders were pressed into pellets with a pressure of 2 tones cm-2 and calcined at 1273K for 15 h in air. Followed by cooling to room temperature, they were re- ground and again pressed into pellets with a pressure of 7 tones cm-2 and subsequently annealed at 1373K for 10 h. The samples were examined by X-ray powder diffraction 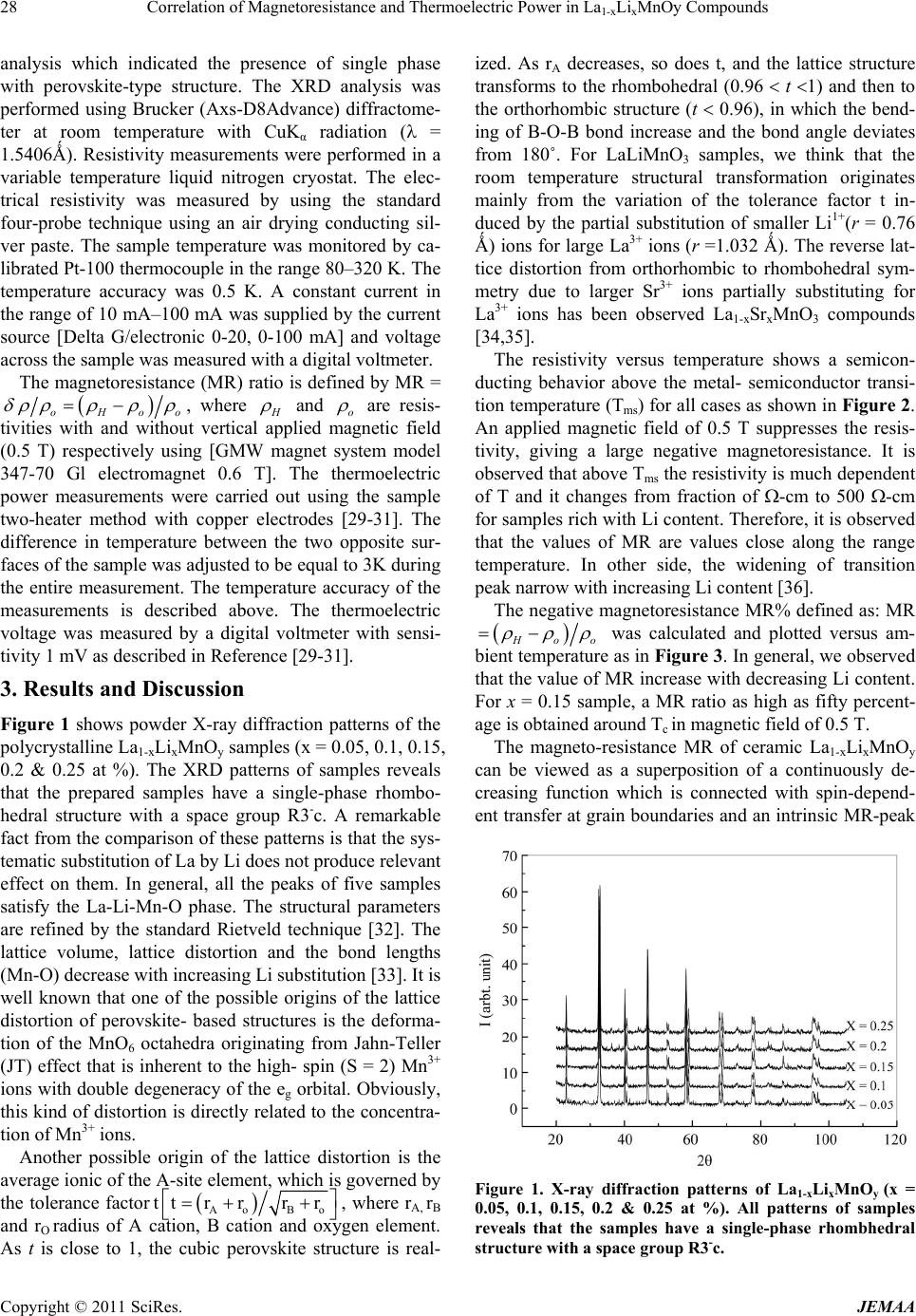 Correlation of Magnetoresistance and Thermoelectric Power in LaLi MnOy Compounds 28 1-x x analysis which indicated the presence of single phase with perovskite-type structure. The XRD analysis was performed using Brucker (Axs-D8Advance) diffractome- ter at room temperature with CuKα radiation ( = 1.5406Ǻ). Resistivity measurements were performed in a variable temperature liquid nitrogen cryostat. The elec- trical resistivity was measured by using the standard four-probe technique using an air drying conducting sil- ver paste. The sample temperature was monitored by ca- librated Pt-100 thermocouple in the range 80–320 K. The temperature accuracy was 0.5 K. A constant current in the range of 10 mA–100 mA was supplied by the current source [Delta G/electronic 0-20, 0-100 mA] and voltage across the sample was measured with a digital voltmeter. The magnetoresistance (MR) ratio is defined by MR = oHoo , where and o are resis- tivities with and without vertical applied magnetic field (0.5 T) respectively using [GMW magnet system model 347-70 Gl electromagnet 0.6 T]. The thermoelectric power measurements were carried out using the sample two-heater method with copper electrodes [29-31]. The difference in temperature between the two opposite sur- faces of the sample was adjusted to be equal to 3K during the entire measurement. The temperature accuracy of the measurements is described above. The thermoelectric voltage was measured by a digital voltmeter with sensi- tivity 1 mV as described in Reference [29-31]. 3. Results and Discussion Figure 1 shows powder X-ray diffraction patterns of the polycrystalline La1-xLixMnOy samples (x = 0.05, 0.1, 0.15, 0.2 & 0.25 at %). The XRD patterns of samples reveals that the prepared samples have a single-phase rhombo- hedral structure with a space group R3-c. A remarkable fact from the comparison of these patterns is that the sys- tematic substitution of La by Li does not produce relevant effect on them. In general, all the peaks of five samples satisfy the La-Li-Mn-O phase. The structural parameters are refined by the standard Rietveld technique [32]. The lattice volume, lattice distortion and the bond lengths (Mn-O) decrease with increasing Li substitution [33]. It is well known that one of the possible origins of the lattice distortion of perovskite- based structures is the deforma- tion of the MnO6 octahedra originating from Jahn-Teller (JT) effect that is inherent to the high- spin (S = 2) Mn3+ ions with double degeneracy of the eg orbital. Obviously, this kind of distortion is directly related to the concentra- tion of Mn3+ ions. Another possible origin of the lattice distortion is the average ionic of the A-site element, which is governed by the tolerance factor Ao Bo ttrrrr , where rA, rB and rO radius of A cation, B cation and oxygen element. As t is close to 1, the cubic perovskite structure is real- ized. As rA decreases, so does t, and the lattice structure transforms to the rhombohedral (0.96 t 1) and then to the orthorhombic structure (t 0.96), in which the bend- ing of B-O-B bond increase and the bond angle deviates from 180˚. For LaLiMnO3 samples, we think that the room temperature structural transformation originates mainly from the variation of the tolerance factor t in- duced by the partial substitution of smaller Li1+(r = 0.76 Ǻ) ions for large La3+ ions (r =1.032 Ǻ). The reverse lat- tice distortion from orthorhombic to rhombohedral sym- metry due to larger Sr3+ ions partially substituting for La3+ ions has been observed La1-xSrxMnO3 compounds [34,35]. The resistivity versus temperature shows a semicon- ducting behavior above the metal- semiconductor transi- tion temperature (Tms) for all cases as shown in Figure 2. An applied magnetic field of 0.5 T suppresses the resis- tivity, giving a large negative magnetoresistance. It is observed that above Tms the resistivity is much dependent of T and it changes from fraction of -cm to 500 -cm for samples rich with Li content. Therefore, it is observed that the values of MR are values close along the range temperature. In other side, the widening of transition peak narrow with increasing Li content [36]. The negative magnetoresistance MR% defined as: MR oo was calculated and plotted versus am- bient temperature as in Figure 3. In general, we observed that the value of MR increase with decreasing Li content. For x = 0.15 sample, a MR ratio as high as fifty percent- age is obtained around Tc in magnetic field of 0.5 T. The magneto-resistance MR of ceramic La1-xLixMnOy can be viewed as a superposition of a continuously de- creasing function which is connected with spin-depend- ent transfer at grain boundaries and an intrinsic MR-peak Figure 1. X-ray diffraction patterns of La1-xLixMnOy (x = 0.05, 0.1, 0.15, 0.2 & 0.25 at %). All patterns of samples reveals that the samples have a single-phase rhombhedral structure with a space group R3-c. Copyright © 2011 SciRes. JEMAA 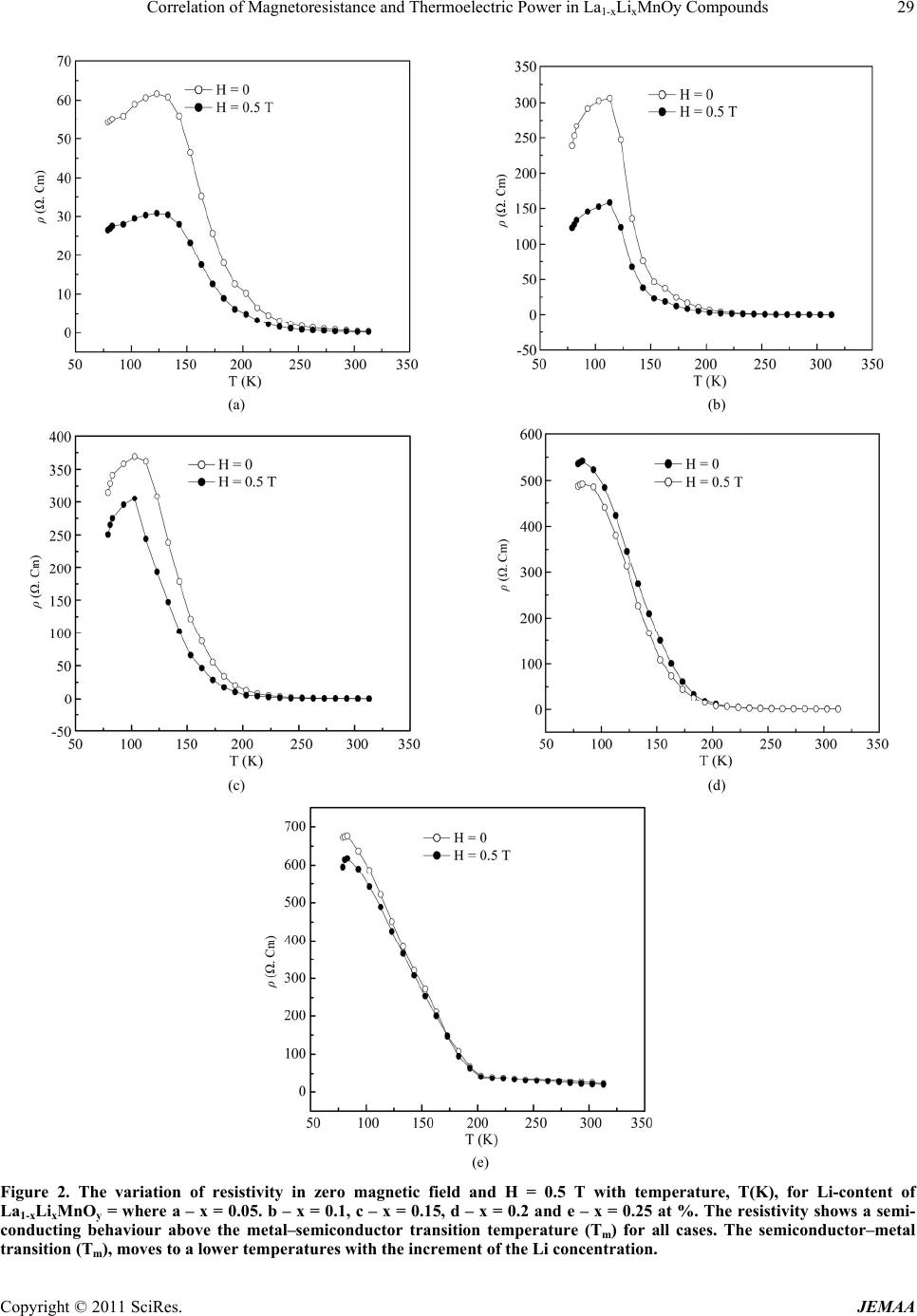 Correlation of Magnetoresistance and Thermoelectric Power in La1-xLixMnOy Compounds Copyright © 2011 SciRes. JEMAA 29 (a) (b) (c) (d) (e) Figure 2. The variation of resistivity in zero magnetic field and H = 0.5 T with temperature, T(K), for Li-content of La1-xLixMnOy = where a – x = 0.05. b – x = 0.1, c – x = 0.15, d – x = 0.2 and e – x = 0.25 at %. The resistivity shows a semi- conducting behaviour above the metal–semiconductor transition temperature (Tm) for all cases. The semiconductor–metal transition (Tm), moves to a lower temperatures with the increment of the Li concentration.  Correlation of Magnetoresistance and Thermoelectric Power in LaLi MnOy Compounds 30 1-x x at Tc [37]. The superposition was observed for all the five composition (i-MR) as in Figure 3. That means that there are additional structures in the i-MR. This superposition corresponds to the first peak of S(T) in Figures 3,4. In other side we observe that while the values of S(T) in- crease with decreasing Li-content the value of MR de- crease. As shown in Figure 4 there are two transitions for each composition. The first peak has a value of TC corre- sponds to that value obtained from the magnetoresistance o (T) curve [38] can be identified with the (highest) peak of the (bulk quantity) i-MR (at TC). The second peak of S(T) corresponds to the m-s transition which de- termined of o as shown in from Figure 2.This obser- vation shows that there are a good correlation between MR and TEP. However, both the value of Seebeck coefficient (S) and the value of resistivity (ρ) increase with increasing dop- ing of Lithium as in Figures 2,4. We expect that when the Li-content increase not only the La-content decreases but also the charge carrier density does [38]. Therefore, the La/Li mixture ions play a prominent role in control- ling the resistivity. However, the Li-doping can also have beneficial ef- fects in regard to the magnetoresistance: 1) we find that MR increase with decreasing Li content and 2) the low Li-substitution tends to broaden the magnetoresistive peak around Tc (Figure 2); both effects are helpful if one thinks about the robust magnetoresistive sensors. In an effort to understand this behavior we found an unex- pected correlation of structures in MR and the thermoe- lectric power TEP results; which gives us an important clue to the origin of this broaden magnetoresistive and the fine structure which agrees with it. The values of metal-semiconductor transition tem- perature (Tm-S) and the ferromagnetic transition tempera- ture (Tc) tabulated in Table 1. Generally the value of both Tc and Tms which are determined from Seebeck or resistivity measurements decreases with increasing Li content. In addition, the values of Tc (S) are higher than Tc(MR) and also the values of Tms(S) are equal or higher than Tms(ρ). We believe that the lithium alters the Mn4+/Mn3+ ratio which is one of the factors which deter- mine the transport and magnetic properties of samples. The decrease in Tms with increasing of Li-content can be interpreted due to an increasing strength of the Mn–O– Mn bond with decreasing the average radius average rA of ions of A-sites because of the partial substitution of smaller Li1+ ions for larger La3+ ions. This substitution causes a narrowing of the bandwidth, thus, decreasing of, eg electrons which, in turn, results in a weakening of the double exchange interaction magnetism [9]. Figure 3. The variation of magnetoresistance with tem- perature, T(K), for Li-content of La1-xLixMnOy. the value of MR increase with decreasing Li content. The MR ratio of the La1-xLixMnOy reaches to 55% under a low field (H = 0.5 Tesla) for low dopin g Li-c ontent. Figure 4. The variation of Seebeck coefficient with tem- perature, T(K), for Li-content of La1-xLixMnOy. The values of S (T) increase with increasing Li concentration. Tm1 cor- responds to the Curie temperature but Tm2 corresponds to m-s transition temperature. Table 1. Curie and M-S transition temperature as function of doping, x. T c (M)(K) Tc (S) Tc (MR) Tms(S) Tms(ρ) x = 0.05 - 203 223 123 123 x = 0.1 215 203 213 113 113 x = 0.15 211 173 203 113 103 x = 0.2 150 173 193 103 83 x = 0.25 163 183 93 83 Copyright © 2011 SciRes. JEMAA  Correlation of Magnetoresistance and Thermoelectric Power in LaLi MnOy Compounds31 1-x x 4. Conclusions The thermoelectric power and magnetoresistance of mo- novalent alkali metal (Li) substituted LaMnO3 polycrys- talline pellets prepared by solid-state reaction procedure has been studied between 80 and 320K. X-ray diffraction patterns showed a single-phase rhombohedral structure of all samples. The M–S transition temperature decreases with increasing Li content. This is due to the partial sub- stitution of smaller Li1+ ions for larger La3+ions or due to Zener bond blocking. The MR ratio of the La1-xLixMnOy is about 55% under a low field (H = 0.5 Tesla) for low doping Li-content. This result opens new opportunities to improve performance of colossal magnetoresistive de- vices. The S(T) curves show that Seebeck coefficient is positive sign. In addition, there are two transition the first we point it correspond to Tc which determine from MR measurements, and the second peak correspond to Tms(ρ). The two corresponds of transition temperature confirm that there are a correlation of structures in MR and the thermoelectric power TEP; which gives us an important clue as to the origin of this broaden magneto resistive and the fine structure which goes with it. 5. Acknowledgements The authors would like to thank H. F. Mohamed for his continuous help. REFERENCES [1] G. C. Xiong, Q. Li, H. L. Ju, S. N. Mao, L. Senapati, X. X. Xi, R. L. Greene and T. Venkatesan, “Giant Magnetore- sistance in Epitaxial Nd0.7Sr0.3MnO3−δ Thin Films,” Ap- plied Physics Letter, Vol. 66, 1995, pp. 1427-1429. doi:10.1063/1.113267 [2] Asamitsu, Y. Moritomo, Y. Tomioka, T. Arima and Y. Tokura, “A Structural Phase Transition Induced by an External Magnetic Field,” Nature, Vol. 373, No. 6613, 1995, pp. 407-409. doi:10.1038/373407a0 [3] V. Caignaert, A. Maignan and B. Raveau, “Up to 50 000 Percent Resistance Variation in Magnetoresistive Poly- crystalline Perovskites Ln2/3Sr1/3MnO3 (Ln = Nd; Sm),” Solid State Communications, Vol. 95, No. 6, 1995, pp. 357-359. doi:10.1016/0038-1098(95)00291-X [4] J. M. D. Coey, M. Viret and S. von Molnar, “Mixed-Valence Manganite,” Advanced Physics, Vol. 48, No. 2, 1999, pp. 167-293. doi:10.1080/000187399243455 [5] Zener, “Interaction between the d-Shells in the Transition Metals. II. Ferromagnetic Compounds of Manganese with Perovskite Structure,” Physical Review B, Vol. 82, No. 3, 1951, pp. 403-405. [6] A. J. Millis, B. I. Shraiman and R. Mueller, “Dynamic Jahn-Teller Effect and Colossal Magnetoresistance in La1-xSrxMnO3,” Physical Review Letter, Vol. 77, No. 1, 1996, pp. 175-178. doi:10.1103/PhysRevLett.77.175 [7] S. Yunoki, A. Moreo and E. Dagotto, “Phase Separation Induced by Orbital Degrees of Freedom in Models for Manganites with Jahn-Teller Phonons,” Physical Review Letter, Vol. 81, No. 25, 1998, pp. 5612-5615. doi:10.1103/PhysRevLett.81.5612 [8] Y. Endoh, K. Hirota, S. Ishihara, S. Okamoto, Y. Mura- kami, A. Nishizawa, T. Fukuda, H. Kimura, H. Nojiri, K. Kaneko and S. Maekawa, “Transition between Two Fer- romagnetic States Driven by Orbital Ordering in La0.88Sr0.12MnO3,” Physical Review Letter, Vol. 82, No. 21, 1999, pp. 4328-4331. doi:10.1103/PhysRevLett.82.4328 [9] H.Y. Hwang, S.-W. Cheong, P.G. Radaelli, M. Marezio and B. Batlogg, “Lattice Effects on the Magnetoresistance in Doped LaMnO3,” Physical Review Letter, Vol. 75, No. 5, 1995, pp. 914-917. doi:10.1103/PhysRevLett.75.914 [10] Z. Jira´ k, F. Damay, M. Hervieu, C. Martin, B. Raveau, G. Andre´ and F. Boure´, “Magnetism and Charge Order- ing in Pr0.5CaxSr0.5-xMnO3 (x = 0.09 and 0.5),” Physical Review B, Vol. 61, No. 2, 2000, pp. 1181- 1188. [11] R. Laiho, K. G. Lisunov, E. La¨ hderanta, P. A. Petrenko, J. Salminen, M. A. Shakhov, M. O. Safontchik, V. S. Stamov, M. V. Shubnikov and V. S. Zakhvalinskii, “Variable-Range Hopping Conductivity in La1−xCaxMn1−y FeyO3: Evidence of a Complex Gap in Density of States Near the Fermi level,” Journal Physics: Condensed Mat- ter, Vol. 14, 2002, p. 8043. [12] L. Malavasi, M. C. Mozzati, C. B. Azzoni, G. Chiodelli and G. Flor, “Role of Oxygen Content on the Transport and Magnetic Properties of La1−xCaxMnO3+δ Mangan- ites,” Solid State Communications, Vol. 123, No. 8, 2002, pp. 321-326. [13] J. Yang, W. H. Song, R. L. Zhang, Y. Q. Ma, B. C. Zhao, Z. G. Sheng, G. H. Zheng, J. M. Dai and Y. P. Sun, “The Effect of Oxygen Stoichiometry on Electrical Transport and Magnetic Properties of La0.9Te0.1MnOy,” Solid State Communications, Vol. 131, No. 6, 2004, pp. 393- 398. doi:10.1016/j.ssc.2004.05.030 [14] R. Dhahri, F. Halouni, “Study of the Effect of Oxygen Deficiencies on the La1−x(Sr,Ca)xMnO3−δ□δ Manganites Properties,” Journal of Alloys and Compounds ,Vol. 385, 2004, pp. 48-52. doi:10.1016/j.jallcom.2004.04.125 [15] N. Abdelmoula, A. Cheikhrouhou and L. Reversat, “Structural, Magnetic and Magnetoresistive Properties of La0.7Sr0.3-xNaxMnO3 Manganites,” Journal Physics: Con- densed Matter, Vol. 13, No. 3, 2001, pp. 449-458. doi:10.1088/0953-8984/13/3/307 [16] R. J. H. Voorhoeve, J. P. Remeika, L. E. Trimble, A. S. Cooper, F. J. Disalvo and P. K. Gallagher, “Perovs- kite-Like La1−xKxMnO3 and Related Compounds: Solid State Chemistry and the Catalysis of the Reduction of NO by CO and H2,” Journal of Solid State Chemistry, Vol. 14, No. 4, 1975, pp. 395-406. doi:10.1016/0022-4596(75)90061-4 [17] T. Shimura, T. Hayashi, Y. Inaguma and M. Itoh, “Magnetic and Electrical Properties of LayAxMnwO3(A = Na, K, Rb, and Sr) with Perovskite-Type Structure,” Journal of Solid State Chemistry, Vol. 124, No. 2, 1996, pp. 250-263. doi:10.1006/jssc.1996.0234 Copyright © 2011 SciRes. JEMAA  Correlation of Magnetoresistance and Thermoelectric Power in La1-xLixMnOy Compounds Copyright © 2011 SciRes. JEMAA 32 [18] G. H. Rao, J. R. Sun, K. Ba¨ rner and N. Hamad, “Crystal Structure and Magnetoresistance of Na-doped LaMnO3,” Journal Physics: Condensed Matter, Vol. 11, No. 6, 1999, pp. 1523-1528. doi:10.1088/0953-8984/11/6/016 [19] S. Roy, Y. Q. Guo, S. Venkatesh and N. Ali, “Interplay of Structure and Transport Properties of Sodium-Doped Lanthanum Manganite,” Journal Physics: Condensed Matter, Vol. 13, No. 42, 2001, pp. 9547- 9559. doi:10.1088/0953-8984/13/42/314 [20] A. Banerjee, S. Pal, S. Bhattacharya, B. K. Chaudhuri and H. D. Yang, “Magnetoresistance and Magnetothermoe- lectric Power of La0.5Pb0.5Mn1-xCrxO3,” Physical Review B, Vol. 64, No. 10, 2001, pp. 104428- 104435. doi:10.1103/PhysRevB.64.104428 [21] M. Jaime, P. Lin, M. B. Salamon and P. D. Han, “Low-Temperature Electrical Transport and Double Ex- change in La0.67 (Pb,Ca)0.33MnO3,” Physical Review. B, Vol. 58, No. 10, 1998, pp. R5901-R5904. doi:10.1103/PhysRevB.58.R5901 [22] G. Zhao, V. Smolyaninova, W. Prellier and H. Keller, “Electrical Transport in the Ferromagnetic State of Man- ganites: Small-Polaron Metallic Conduction at Low Temperatures,” Physical Review Letter, Vol. 84, No. 26, 2000, pp. 6086-6089. doi:10.1103/PhysRevLett.84.6086 [23] G. J. Snyder, R. Hiskes, S. DiCarolis, M. R. Beasley and T. H. Geballe, “Intrinsic Electrical Transport and Mag- netic Properties of La0.67Ca0.33MnO3 and La0.67Sr0.33 MnO3 MOCVD Thin Films and Bulk Material,” Physical Review B, Vol. 53, 1996, pp. 14434-14444. doi:10.1103/PhysRevB.53.14434 [24] S. Bhattachyrya, A. Banerjee, S. Pal, R. K. Mukherjee and B. K. Chaudhuri, “Thermoelectric Power of Na-Doped La0.7Ca0.3−yNayMnO3 both in the Presence and the Absence of Magnetic Field,” Journal of Applied Physics, Vol. 93, No. 1, 2003, pp. 356-361. doi:10.1063/1.1527220 [25] R. Horyn, A. J. Zaleski, C. Sulkowski, E. Bukowska and A. Sikora, “Magnetic Features of La1−2xNaxKxMnO3+δ Solid Solution,” Physica C, Vol. 387, No. 1-2, 2003 , pp. 280-283. doi:10.1016/S0921-4534(03)00694-4 [26] P. Mandal, “Temperature and Doping Dependence of the Thermopower in LaMnO3,” Physical Review B, Vol. 61, No. 21, 2000, pp. 14675-14680. doi:10.1103/PhysRevB.61.14675 [27] M. Jaime, M. B. Salamon, K. Pettit, M. Rubenstein, R. E. Treece, J. S. Horwitz and D. B. Chrisey, “Effects on the Transport Properties of (R,Sr)3Mn2O7 (R=La, Eu, and Pr),” Applied Physics Letter, Vol. 68, 1996, pp. 1576-1578. doi:10.1063/1.116686 [28] M. Jaime, M. B. Salamon, M. Rubenstein, R. E. Treece, J. S. Horwitz and D. B. Chrisey, “High-Temperature Ther- mopower in La2/3Ca1/3MnO3 Films: Evidence for Pola- ronic Transport,” Physical Review B, Vol. 54, No. 17, 1996, pp. 11914-11917. doi:10.1103/PhysRevB.54.11914 [29] A. M. Ahmed, S. A. Saleh, E. M. M. Ibrahim, E. Bontem- pib and H. F. Mohamed, “The Effect of Annealing Proc- ess on the Physical Properties of La1-xNaxMnOy,” Journal of Magnetism and Magnetic Materials, Vol. 320, No. 6, 2008, pp. L43-L49. doi:10.1016/j.jmmm.2007.08.031 [30] A. M. Ahmed, S. A. Saleh, E. M. M. Ibrahim and H. Mo- hamed, “Crystal Structure and Some Transport Properties of Na-doped LaMnOy,” Journal of Magnetism and Mag- netic Materials, Vol. 301, No. 2, 2006, pp. 452-457. doi:10.1016/j.jmmm.2005.07.021 [31] A. M. Ahmed, “Bi-doping Effects on the Transport Prop- erties in La0.7-xBixSr0.3MnOy,” Physica B, Vol. 352, No. 1-4, 2004, pp. 330-336. doi:10.1016/j.physb.2004.08.008 [32] D. B. Wiles, R. A. Young, “New Computer Program for Rietveld Analysis of X-Ray Powder Diffraction Patterns,” Journal of Applied Crystallography, Vol. 14, 1981, pp. 149-151. doi:10.1107/S0021889881008996 [33] S. L. Ye, W. H. Song, J. M. Dai, S. G. Wang, K. Y. Wang, C. L. Yuan and Y. P. Sun, “Effect of Li Substitution on the Crystal Structure and Magnetoresistance of LaMnO3,” Journal Applied Physics, Vol. 88, No. 10, 2000, pp. 5915-5919. doi:10.1063/1.1319971 [34] A. Urushibara, Y. Moritomo, T. Arima, A. Asamitsu, G. Kidom and Y. Tokuro, “Insulator-Metal Transition and Giant Magnetoresistance in La1-xSrxMnO3,” Physical Re- view B, Vol. 51, No. 20, 1995, pp. 14103- 14109. doi:10.1103/PhysRevB.51.14103 [35] J. F. Mitchell, D. N. Argyriou, C. D. Potter, D. G. Hinks, J. D. Jorgensen and S. D. Bader, “Structural Phase Dia- gram of La1-xSrxMnO3+δ: Relationship to Magnetic and Transport Properties,” physical Review B, Vol. 54, No. 9, 1996, pp. 6172-6183. [36] A. M. Ahmed, A. K. Diab and H. F. Mohamed, “Effect of Li-Substitution on the Resistivity and Magnetoresistance of LaMnOy,” Accepted in Journal of Superconductivity and Novel Magnetism, 2010. [37] H. Y. Hwang, S. W. Cheong, N. P. Ong and B. Batlogg, “Spin-Polarized Intergrain Tunneling in La2/3Sr1/ 3MnO3,” Physical Review Letter, Vol. 77, No. 10, 1996, pp. 2041-2044. doi:10.1103/PhysRevLett.77.2041 [38] G. H. Rao, J. R. Sun, A. Kattwinkel, L. Haupt, K. B. arner, E. Schmitt and E. Gmelin, “Magnetic,Electric and Ther- mal Properties of La0.7Ca0.3Mn1−xFexO3 Compounds,” Physica B, Vol. 269, No. 3-4, 1999, pp 379-385. doi:10.1016/S0921-4526(99)00119-2
|