Theoretical Economics Letters, 2013, 3, 233-244
http://dx.doi.org/10.4236/tel.[phone] Published Online August 2013 (http://www.scirp.org/journal/tel)
Economic and Emotional Rationality: An Application to
Wealth Concentration
Jose Rigoberto Parada-Daza1, Miguel Ignacio Parada-Contzen2
1Universidad de Concepcion, Concepcion, Chile
2Technische Universität Berlin (TUB), Berlin, Germany
Received May 29, 2013; revised June 29, 2013; accepted July 17, 2013
Copyright © 2013 Jose Rigoberto Parada-Daza, Miguel Ignacio Parada-Contzen. This is an open access article distributed under the
Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the
original work is properly cited.
ABSTRACT
This Paper presents a theoretical outline regarding the Emotional Well-being (EW) function as an extension of the eco-
nomic utility function. EW includes habitual factors that are always present in everyday decision making. Firstly, an
analytical-mathematical conceptualization of EW is carried out, followed by a study of the concept of emotional secu-
rity, in order to define a new idea of emotional rationality as a complement to economic rationality. An explanation is
put forth, as an application, of the concentration of wealth phenomenon according to the focus on economic and emo-
tional rationality. The conclusion is that EW is a theoretical approach which can clarify the understanding of the deci-
sion making process in economics activities.
Keywords: Utility Function; Emotional Well-Being; Economic Rationality; Emotional Rationality; Concentration of
Wealth
1. Introduction
The relation between levels of wealth and utility can be
explained conceptually, from an exclusively economic
point of view, by adopting the economic utility function
concept, based on the theory of rational investment deci-
sions measured in utilitarian units. This is the foundation
of the argument for financial and economic decision
making theory. However, this vision can change if vari-
ables are incorporated that are not completely explained
by rationality of economic man when the interpretation
of a particular economic fact is attempted. Sometimes it
is not completely convincing that the person or persons
behave solely as economic agents when making eco-
nomic decisions.
As such, following the economic utility function, new
variables can be incorporated into the analysis in order to
explain the relation between wealth and utility. In this
perspective, Parada-Daza [1], a function is developed
that incorporates the economic rationale adding an ele-
ment to interpret other variables that influence in making
decisions during an economic act. This new factor in-
cludes factors such as: ethics, social responsibility and
other characteristics related to people. This function has
been titled the Emotional Well-being Function. The EW
function has been used in literature to evaluate corporate
social responsibility Parada-Daza [2], the sustainable
development of small and medium enterprises, Brilius [3]
and to evaluate the economic and non-economic behavior
of various countries, Parada-Daza [4].
In this paper analytically distinct characteristics of the
EW function are developed. Emphasis is placed on two
new concepts; emotional rationality and emotional secu-
rity to propose an application of the Emotional Well Be-
ing function that allows understanding, from a different
perspective, what motivates the concentration of wealth
and the perception that the actions of rich and poor citi-
zens must be separated.
2. Prior Definitions and Literary Analysis
To substantiate the propositions of this paper, some con-
cepts must be defined. These are extrapolated from con-
sulted literature and new concepts incorporated here.
2.1. Emotional Well-Being
Personal Emotional Well-being (EW) is understood as
the level of satisfaction that is felt when undertaking any
act of daily life. This includes economic and global emo-
tional satisfaction. This is evaluated by emotional pleas-
C
opyright © 2013 SciRes. TEL

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 234
ure. Higher the level of emotional pleasure, higher the
level of emotional wellbeing. It is considered here that
EW is a variable that is dependent on an individuals’
wealth.
2.2. Relative Wealth
Let wa,i be the wealth of individual i, valued in absolute
monetary units a, in an economy comprised of n indi-
viduals and W the total wealth in the economy in the fol-
lowing form:
,
1
n
ai
i
wW



Thus, a variable is defined as


,0,1 ri
w which re-
presents the relative wealth of an individual i as:
,
,
ai
ri
w
wW , such that ,
1
1
n
ri
i
w


.
The relative wealth of the individual i permits the
grouping of people as more wealthy and less wealthy.
Therefore if an individual i has a wealth relative to 0.9 it
indicates that, of the entire wealth of a society of n indi-
viduals, this individual i has accumulated 90% and the
rest of the n
 1 individuals, possess 10% of the total
wealth.
2.3. Utility Function and Its Literary Discussion
2.3.1. Economic Utility
In economic decision making theory, the utility function
is used as the basis of analysis for explanation under
risky conditions. A set of axioms has been developed to
explain the choice or decision theory, Copeland and
Weston [5] established a quadratic utility function as
well as the logarithmic utility function that has been
widely used in literature. Theories have been developed
based on this type of function that reflect the behavior of
people from an economic rationality standpoint, giving
understanding as to why people are essentially maximiz-
ers and only move through this type of function.
Using the concept of relative wealth, for the purposes
of this paper a utility function is defined as:


:0,1U  and .

rr
wUUw
Thus, U is differentiable up to at least second grade
and is strictly increasing. i.e.

d0, 0,1
dr
r
Uw
w
In this approach, mathematically, utility is a function
of wealth and it is supposed that the greater the wealth,
the greater the utility. Each point shows the value that
corresponds to each level of wealth. Economic man
moves over the combination of these points. In regards to
the previously mentioned it is assumed that the utility
function is both increasing and limited. This implies that
the greater the wealth, the greater the degree of economic
satisfaction. The fact that it is limited means that certain
levels exist where people consider a certain amount of
satisfaction is acceptable.
Another characteristic of this function is that there is a
diminishing marginal utility. That is, compared to an
increase of wealth, an augment in utility will be gradu-
ally decreasing. Mathematically this is represented by a
negative value of the second derivative of the utility
function of which corresponds graphically to a convex
function:

2
2
d0, 0,1
dr
r
Uw
w
Some utility functions commonly used in economic
and financial literature are: logarithmic function, quad-
ratic function, exponential negative function and poten-
tial function, Marin & Rubio [6]. In continuation, the two
most common functions used in the economic literature
are detailed.
2.3.2. Logarithmic Function



 

ln1, with,0,
rr
Uwa wcac

  (1)
D. Bernoulli (1730-1731) applied the logarithmic util-
ity function, i.e. U(w) = ln(w). Given that this paper util-
izes relative wealth, which is defined between zero and
one, the use of a logarithmic function is not possible be-
cause the function would take negative values. To avoid
this problem, the argument of the logarithmic function is
augmented in one unity as shown in Equation (1). The
logarithmic function is used because it is increasing
throughout its domain, is convex and has no relative
maximum point or inflection.
Figure 1 shows the graph of this function. The dotted
line represents the utility function value when wr = 1.
2.3.3. Quadrat i c Function
 
2
1,with
rr
Uwawaa0

  (2)
Figure 1. Function U(wr) = ln(wr + 1) + c.

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 235
The quadratic function has been used by several au-
thors such as R. Merton [7], Copeland-Weston [5], R.
Jarrow [8]. W. Sharpe [9], Also represents the utility
function by a quadratic function. The main feature of this
is that it presents a maximum point of utility for a level
of wealth. Beyond this point the function graph decreases
and, therefore, the utility decreases with increasing
wealth. Regarding this, W. Sharpe [9] said: this is clearly
unacceptable. This is explained following the concept of
economic rationality which is an intersection between a
normative and a positive approach, as there are people in
real life who behave according to this standard however
others will not. Thus their everyday economic actions are
not fully explained by the assumption of economic ra-
tionality and the ethic that this standard implies. Figure 2
shows the graph of this function.
2.3.4. Discussion
Thus, personal economic performance reduces when
maximum utility is obtained. This is methodologically
explained through the mathematic maximization of the
desired utility of an event various alternative propositions.
The expected utility implies that, faced with two possi-
bilities of obtaining compensation for a decision that is
made, that which has a higher expected utility is appro-
priate. The aforementioned is that which is defined as the
expected utility hypothesis which represents the “rational
behavior” of a person faced with uncertainty. With re-
spect to this, Lafont [10] poses the following:
a) The definition of the utility function with these nor-
mative assumptions is a working hypothesis and there-
fore it is necessary to deduce verifiable empirical impli-
cations. If the empirical study doesn’t dismiss, it can be
concluded that people act as if they will maximize the
expected utility.
b) The utility function is a normative interpretation
that consists of demonstrating that rational agents “should
maximize” their expected utility.
The concept of rational behavior rests within the pre-
viously mentioned postulations and is defined as the abil-
Figure 2. Function U(wr) = a(wr + 1)2 – a.
ity to choose as if it were a lottery characterized by vari-
ous paths of retribution. This interpretation is an eco-
nomic definition and the rational concept should not be
understood as a synonym for terms such as: reasonable,
prudent, just and fair amongst others. Thus, a being is
“rational” only if they behave according to the economic
rule or standard, which from a Theory of Knowledge
point of view, is based on a rational and empirical model.
These two aspects of regulation and maximization are
essential for understanding and reasoning what, under
these suppositions, implies the concept of rational eco-
nomic man.
Carroll [11] follows Max Weber in “The Protestant
Ethic and the Spirit of Capitalism”, which explains that
people’s search for wealth is for their own use and pos-
session, and that this is the main cause of both the system
and of individuals. Robinson [12] indicates that the maxi-
mization of profit is a metaphorical concept of impene-
trable circularity. Debreu [13] shows that there are con-
tinuous and non-continuous utility functions. Markowitz
[14] based his approach on the utility function. Pratt and
Arrow [15] establish ways of measuring risk reward.
“Powerful utility functions” have been developed with
complex mathematical formulas under the same norma-
tive principles as indicated in Ait-Sahalia & Brandt [16],
Ang [17], Mehra & Prescott [18], Friend & Blume [19].
Hwang and Satchell [20] elaborate from the Utility
function, how much should be paid in order to acquire
information. The utility function has been used to explain
donations and signal that these have a similar behavior to
luxury goods. Inhaber and Carroll [21] signal that luxury
goods are generally associated with assets such as art,
jewelry, sporting equipment, etc. Which are always
goods in an economic sense and therefore, assimilable to
any other economic good. Carroll [11] indicates that the
love of wealth as motivation is assuredly an extreme po-
sition; there are other types of motivation such as: job
satisfaction, status, philanthropic ambition, power, etc.
Considering the aforementioned, the utility function
theory has a conceptual and philosophical foundation but
is also regulative, forcing the understanding of people’s
behavior exclusively as rational economic entities. In this
way, the utility function explains any other type of non-
economic motivation which would be well represented
under its assumptions. From a Theory of Knowledge
stand point, the utility function theory is a mix between
rationalism and empiricism, where an intersection can be
made between the normative and the positive. The ra-
tionalism and empiricism approach is present in financial
theory.
The interpretation of an economic act from a solely ra-
tional economic point of view and its methodological
representation through the utility function can at times
give partial results. For which, these acts should be ana-

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 236
lyzed from a view point that encompasses both rational
economic standards and their implicit ethics, as with
other ethic views that can fulminate distinct decisions to
those that would suggest an analysis from a purely eco-
nomic view point.
3. Emotional Well-Being Function
3.1. Definition
From the postulations of Sharpe (Op.Ci.), who indicates
that no investor is located on the descending part of the
utility function curve, a more global function of eco-
nomic utility is discussed here. This new function ex-
plains the performance of people and businesses simul-
taneously incorporating both the facets of man with eco-
nomic rationality and the view of people and businesses
that also act motivated by other ethical values, Parada [1].
This same model has been used to explain the economic
crisis in small and medium businesses and their sustain-
able politics, Brilius [3], in order to assess the social re-
sponsibility of the business, Parada-Daza [2], and by this
same author in order to evaluate the economic behaviour
of people in different countries, Parada-Daza [4].
An Emotional Well-being (EW) function considers
that there are economic sacrifices in exchange for bene-
fits provoked by different reasons and exclusively eco-
nomic motives. This is translated mathematically in that
the function is not necessarily strictly increasing through-
out its domain.


 
:0,1
,
rrrer
U
wUUwUwf




 (3)
where Ur(wr) corresponds to a classic utility function as
previously described. The term Ue(wr, f) joins all cultural,
ethical, social, emotional or practical characteristics of a
distinct nature to the strictly economic, that can cause the
individual to be separated from the behavior of an eco-
nomic man. Unlike the original article of Parada-Daza
[1], an innovation is introduced here, making a generali-
zation of both components and incorporating the f pa-
rameter to synthesize the non-economic characteristics
mentioned.
For analysis, the following function is considered be-
cause the conclusions derived from it are easily gener-
alizable to the types of functions described above.



 
EW :0,1
EW
ln1sin 2
rr
rr
wU w
awbfw c







(4)
With parameters of the function and a
+ b = 1.
,,,abc f
Parameters a an d b are interpreted as the relative im-
portance that people or organizations submit to economic
rationality and the respective emotional component. In
effect, if a = 1, then b = 0, a classic utility function is
obtained as previously expressed. The parameter c repre-
sents the minimum satisfaction that is independent from
the level of wealth of each person or business. This could
be zero, in which case it is implied that emotional well-
being only depends on wealth. The coefficient c is inter-
preted as a “Satisfaction of Belonging” for being an inte-
gral part of a business or society that provides emo-
tional satisfaction to the person, independent to their
wealth. This enjoyment can be explained by such factors
as: social and business prestige, business and social his-
tory and tradition, social and business culture and other
specific factors and characteristics of each person or
business.
The parameter f is interpreted as the frequency with
which the person or organization allows the aversion of
strictly economic rationality. Distinct values of f involve
distinct types of behavior. While higher the value of f,
higher will be the oscillation of the emotional variable
with respect to a purely economic component.
The approach of (4) is an extension of the Emotional
Well-being function introduced by Parada-Daza [1], be-
cause in this paper, the parameter f is considered perma-
nently in f = 1/2 and, given that it was working with ab-
solute wealth (in general, w > 1), the displacement of the
algorithmic component was not considered. However, in
this paper it has been decided to open the spectral analy-
sis to include the parameter f and with it explain why the
valor f = 1/2 has been previously used. Therefore, in
contrary to the Parada-Daza [1] paper, in this contribu-
tion the relative wealth concept has been adopted, with


0,1
r
w, and the original approach has been modified
adding 1 to the argument of the logarithmic function, in
such a way to adjust it in order to work without the EW
function assuming negative values, Mao [22].
3.2. Coordinates of Emotional Well-Being:
Enveloping Functions
The family of curves (4), defined by the parameter f, in-
cludes superior and inferior enveloping functions. This is
explained by the sinusoidal component of the EW func-
tion that has a bounded range in [−1,1], independent of
its argument.
A curve is said to be enveloping a family of plane
curves if it is tangent with all the lines of said family,
additionally each point of the enveloping function has
contact with some of the lines of the family that is ex-
amined, Demidovich [23].
For a family of plane curves depending on the pa-
rameter α that complies with the following equation:


,, 0fxy 

.
The enveloping equation is determined by way of the
equation systems:


,, 0fxy   and

,, 0fxy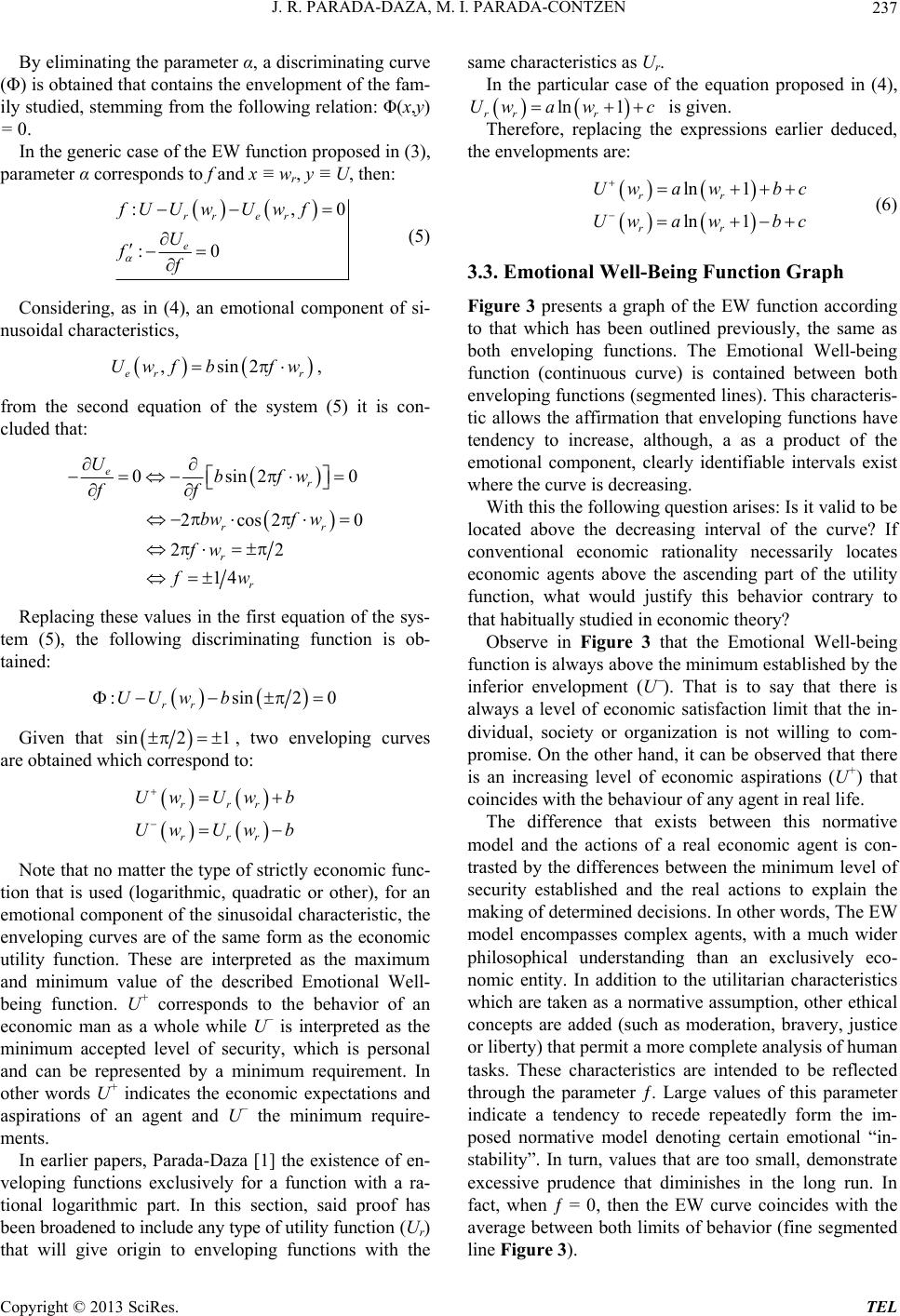




.

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 237
By eliminating the parameter α, a discriminating curve
(Φ) is obtained that contains the envelopment of the fam-
ily studied, stemming from the following relation: Φ(x,y)
= 0.
In the generic case of the EW function proposed in (3),
parameter α corresponds to f and x ≡ wr, y ≡ U, then:
 
:,
:0
rr er
e
fUUwUw f
U
ff

 



0

(5)
Considering, as in (4), an emotional component of si-
nusoidal characteristics,
 
,sin2
er r
Uwf bfw ,
from the second equation of the system (5) it is con-
cluded that:


0sin2
2cos2
22
14
e
r
rr
r
r
Ubfw
ff
bwf w
fw
fw

 


 


0
0
Replacing these values in the first equation of the sys-
tem (5), the following discriminating function is ob-
tained:
 
:sin
rr
UUw b 20
Given that

sin2 1 , two enveloping curves
are obtained which correspond to:



 
rrr
rrr
UwUwb
UwUwb




Note that no matter the type of strictly economic func-
tion that is used (logarithmic, quadratic or other), for an
emotional component of the sinusoidal characteristic, the
enveloping curves are of the same form as the economic
utility function. These are interpreted as the maximum
and minimum value of the described Emotional Well-
being function. U+ corresponds to the behavior of an
economic man as a whole while U− is interpreted as the
minimum accepted level of security, which is personal
and can be represented by a minimum requirement. In
other words U+ indicates the economic expectations and
aspirations of an agent and U− the minimum require-
ments.
In earlier papers, Parada-Daza [1] the existence of en-
veloping functions exclusively for a function with a ra-
tional logarithmic part. In this section, said proof has
been broadened to include any type of utility function (Ur)
that will give origin to enveloping functions with the
same characteristics as Ur.
In the particular case of the equation proposed in (4),




ln 1
rr r
Uwawc

 is given.
Therefore, replacing the expressions earlier deduced,
the envelopments are:



ln 1
ln 1
rr
rr
Uwa wbc
Uwa wbc






(6)
3.3. Emotional Well-Being Function Graph
Figure 3 presents a graph of the EW function according
to that which has been outlined previously, the same as
both enveloping functions. The Emotional Well-being
function (continuous curve) is contained between both
enveloping functions (segmented lines). This characteris-
tic allows the affirmation that enveloping functions have
tendency to increase, although, a as a product of the
emotional component, clearly identifiable intervals exist
where the curve is decreasing.
With this the following question arises: Is it valid to be
located above the decreasing interval of the curve? If
conventional economic rationality necessarily locates
economic agents above the ascending part of the utility
function, what would justify this behavior contrary to
that habitually studied in economic theory?
Observe in Figure 3 that the Emotional Well-being
function is always above the minimum established by the
inferior envelopment (U

). That is to say that there is
always a level of economic satisfaction limit that the in-
dividual, society or organization is not willing to com-
promise. On the other hand, it can be observed that there
is an increasing level of economic aspirations (U+) that
coincides with the behaviour of any agent in real life.
The difference that exists between this normative
model and the actions of a real economic agent is con-
trasted by the differences between the minimum level of
security established and the real actions to explain the
making of determined decisions. In other words, The EW
model encompasses complex agents, with a much wider
philosophical understanding than an exclusively eco-
nomic entity. In addition to the utilitarian characteristics
which are taken as a normative assumption, other ethical
concepts are added (such as moderation, bravery, justice
or liberty) that permit a more complete analysis of human
tasks. These characteristics are intended to be reflected
through the parameter ƒ. Large values of this parameter
indicate a tendency to recede repeatedly form the im-
posed normative model denoting certain emotional “in-
stability”. In turn, values that are too small, demonstrate
excessive prudence that diminishes in the long run. In
fact, when ƒ = 0, then the EW curve coincides with the
average between both limits of behavior (fine segmented
line Figure 3).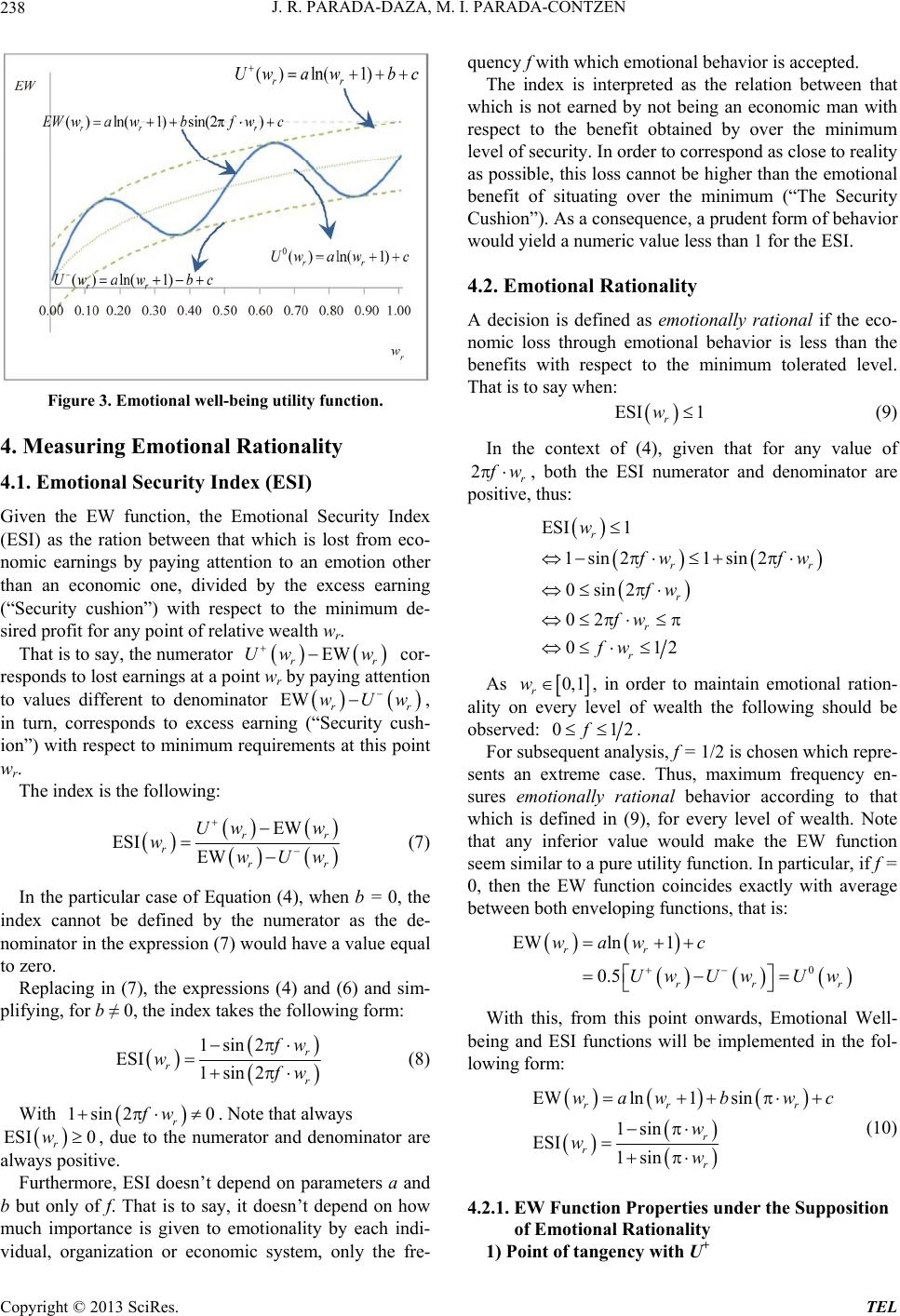

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 238
Figure 3. Emotional well-being utility function.
4. Measuring Emotional Rationality
4.1. Emotional Security Index (ESI)
Given the EW function, the Emotional Security Index
(ESI) as the ration between that which is lost from eco-
nomic earnings by paying attention to an emotion other
than an economic one, divided by the excess earning
(“Security cushion”) with respect to the minimum de-
sired profit for any point of relative wealth wr.
That is to say, the numerator



EW
rr
Uw w


cor-
responds to lost earnings at a point wr by paying attention
to values different to denominator


wEW r wU

r ,
in turn, corresponds to excess earning (“Security cush-
ion”) with respect to minimum requirements at this point
wr.
The index is the following:



 

EW
ESI EW
r
r
rr
Uw w
wwUw




r

(7)
In the particular case of Equation (4), when b = 0, the
index cannot be defined by the numerator as the de-
nominator in the expression (7) would have a value equal
to zero.
Replacing in (7), the expressions (4) and (6) and sim-
plifying, for b ≠ 0, the index takes the following form:
 

1sin2
ESI 1sin2
r
r
r
f
w
w
f
w


(8)
With . Note that always
r, due to the numerator and denominator are
always positive.

1sin2 0
r
fw

0ESI w
Furthermore, ESI doesn’t depend on parameters a and
b but only of f. That is to say, it doesn’t depend on how
much importance is given to emotionality by each indi-
vidual, organization or economic system, only the fre-
quency f with which emotional behavior is accepted.
The index is interpreted as the relation between that
which is not earned by not being an economic man with
respect to the benefit obtained by over the minimum
level of security. In order to correspond as close to reality
as possible, this loss cannot be higher than the emotional
benefit of situating over the minimum (“The Security
Cushion”). As a consequence, a prudent form of behavior
would yield a numeric value less than 1 for the ESI.
4.2. Emotional Rationality
A decision is defined as emotionally rational if the eco-
nomic loss through emotional behavior is less than the
benefits with respect to the minimum tolerated level.
That is to say when:


ESI 1
r
w (9)
In the context of (4), given that for any value of
2r
f
w

, both the ESI numerator and denominator are
positive, thus:


 


ESI 1
1sin2 1sin2
0sin2
02
012
r
rr
r
r
r
w
f
wf
fw
fw
fw

 
 
 
 
w
As


0,1
r
w, in order to maintain emotional ration-
ality on every level of wealth the following should be
observed: 012f

.
For subsequent analysis, f = 1/2 is chosen which repre-
sents an extreme case. Thus, maximum frequency en-
sures emotionally rational behavior according to that
which is defined in (9), for every level of wealth. Note
that any inferior value would make the EW function
seem similar to a pure utility function. In particular, if f =
0, then the EW function coincides exactly with average
between both enveloping functions, that is:




 
0
EWln 1
0.5
rr
rr
wawc
Uw UwUw





r
With this, from this point onwards, Emotional Well-
being and ESI functions will be implemented in the fol-
lowing form:




 

EWln1 sin
1sin
ESI 1sin
rr r
r
r
r
wawbw
w
ww
c

 


(10)
4.2.1. EW Function Properties under the Supposition
of Emotional Rationality
1) Point of tangency with U+

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 239
The EW function is tangent with U+ in wr = 1/2. In-
deed, in order to have:
 
EW rr wUw


By (6) and (10) then:




ln1sin 2
ln 1
rr
r
aw bfw
aw bc
 

c
Reducing, the following is obtained:
.

sin 21
r
fw 
This complies with the function domain only if:
22
r
fw .
Additionally, f = 1/2, thus: 12
r
w.
That is, the EW function reaches a superior level (U+)
at a solitary point within the domain when the emotional
component reaches its maximum value. Additionally, at
this point, the marginal growth rate for both functions is
the same:



12
12 12
12
12
dEW
d
cos
1
1
d
d
r
rr
r
r
r
rw
r
rww
rw
r
rw
w
w
abw
w
a
w
Uw
w












These growth rates are interpreted as marginal emo-
tional and economic wellbeing. That is, for this point, the
marginal emotional wellbeing is exactly the same as the
marginal emotional wellbeing for an economic man.
2) Determining the maximum level of emotional
wellbeing
Deriving EW with respect to relative wealth, the fol-
lowing is obtained:

dEW 2cos2
d1 r
rr
a
f
bfw
ww
 

On the other hand, the second derivative of the EW
function corresponds to:
 
2
22
22
dEW 4sin2
d1r
rr
a
f
bfw
ww



The derived functions can be disassembled into two
parts u1 y u2 such as:
1
dEW
dr
uu
w
With:

12
and2cos 2
1r
r
a
uufb
w
 
fw
The first expression of (11), u
1, is interpreted as the
rate of change in EW as a product of the emotional
component of the behavior. Note that this change is al-
ways strictly positive. That is to say, the economic com-
ponent of the behavior is strictly increasing in all the
domain and that in the behavior of individuals, organiza-
tions or economies that approach the paradigm of eco-
nomic rationality (i.e. when a → 1 and b → 0), the EW
function will have a maximum level only in the outer
lying reaches of its domain when .
1
r
w
Moreover, the second component of (11), u2, corre-
sponds to the EW rate of change produced by the emo-
tional component of the behavior. This change can be
both positive or negative, so it can be presumed that
within the defined domain, , the EW function
can have a maximum or minimum point in w* when:
[0,1]
r
w
 
12
*
dEW 0*
dr
rww
uw uw
w
 

*
Note that the value of w* depends on the parameters of
the function a, b = (1 – a) and f. this equation does not
have a direct analytical solution, for which an analysis of
all possible parameter values is not carried out.
Furthermore, when wr → 0 the EW derivative is posi-
tive and therefore, the EW function is increasing in a
vicinity of 0. Also, under the supposition of emotional
rationality stated earlier (f = 1/2), the second derivative is
negative throughout the EW domain, thus the EW func-
tion graph is convex. In other words, these properties
ensure that there is a point where the function reaches its
maximum value within the domain [0, 1].
Therefore the EW function will be increasing in the
interval [0, w*] and decreasing in the interval [w*, 1]. In
which case with the supposition of emotional rationality
the w* point complies with:
 
1cos
*1
aaw
w



*
The solution to this equation depends exclusively on
the value of parameter a. An extreme case is given when
w* = 1. In this situation, the function will be strictly in-
creasing throughout its domain. If the solution to the
equation is such that w* > 1, therefore the maximum
value of EW will correspond to EWmax = EW (w = 1),
since the function would be increasing throughout the
entire domain. From the latter it can be deduced that a
value limit exists for the parameter a for which a maxi-
mum wellbeing is reached for total wealth (wr = 1). In-
deed, making w* = 1 from the last expression is obtained
2
(11)

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 240
that: 20.862
21
a 
 .
This means that, under the supposition of emotional
rationality, for values of a superior to 0.862 the EW
function will be strictly increasing throughout its domain
and will reach maximum in w* = 1.
3) EW function graph
Figure 4 shows an EW function under supposed emo-
tional rationality function (continuous blue line) together
with both enveloping functions (continuous green lines).
Note the tangent point in wr = 1/2 and that the function
have a maximum value close to wr = 0.7.
4.2.2. ESI Properties under Supposed Emotional
Rationality
Note that the properties expressed in continuation are
valid when f = 1/2.
1) Increasing and decreasing intervals
Considering that


0,1
r
w, the function is decreasing
when:




2
dESI 0
d
2cos 0
1sin
2cos 0
02
012
r
r
r
r
r
r
r
w
w
w
w
w
w
w
 

 






 
Analogous to the previous, the function is increasing
when:

dESI01 21
drr
r
ww
w


2) Maximum and minimum points
The wr = 1/2 point is a singular point, in effect:

12
dESI 0
dr
r
rw
w
w


Taking this into consideration as well as the increasing
and decreasing intervals previously calculated, the maxi-
mum and minimum points of the function are given by:



Maximum: 0ESI1
Minimum :12ESI0
Maximum:1ESI1
rr
rr
rr
ww
ww
ww
 
 
 
From this, it can be deduced that the ESI range corre-
sponds to
 


Range ESI0,1
r
w.
3) Symmetry of the ESI
Figure 4. EW under supposed emotional rationality.
The ESI shows symmetric behavior in relation to the
wr = 1/2 point. This is demonstrated in continuation.
According to the definition of symmetry in differential
calculus, a function f(x) is symmetric with respect to x0
when






00
f
xx fxx .
Let δ > 0, thus
 

 
   


1sin 2
ESI1 21sin 2
1sin2 cossincos2
1sin2 cossincos2
1cos
1cos









 















 
 




1sin 2
ESI1 21sin 2
1sin2cossincos2
1sin2cossincos2
1cos 1cos
1cos 1cos









 




 




 

 
Therefore


ESI1 2ESI1 2




 and the func-
tion is symmetric with respect to wr = 1/2. Precisely for
wr = 1/2, the EW function is tangent to its superior limit
or utility function. That is to say, the loss produced by
distancing from economic behavior is minimized at this
point.
Note that if points are defined as wr,1 y wr,2, these two
points are symmetric (that is, wr,1 = 1/2  δ and wr,2 =
1/2 + δ) if and only if wr,1 + wr,2 = 1. In other words, two
different levels of wealth exist for which the ESI reaches
the same value. These levels are such that together they
represent all economic wealth, as it was previously de-
fined that ,
1
1
n
ri
i
w


.
4) ESI Graph

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 241
It is necessary to note that the index is a relative meas-
ure and not absolute. In symmetric intervals, who has
more wealth (wr → 1) has their EW balanced the same as
those with less wealth (wr → 0), however with different
levels of economic demands and expectancies. These
levels of demands are given by U− and exclusively eco-
nomic expectancies by U+. This implies that, even if they
behave in different wealth dimension, both agents are
equally satisfied because they share the same relationship
between economic compromise and security cushion.
In order to explain the previous, the following example
is used. Suppose that two individuals exist. One individ-
ual has 20% of the total wealth; on the contrary, the other
individual has 80%. For symmetry, both individuals have
the same level of emotional security. This is represented
directly in Figure 5. In this case
. However, the individ-
ual who possesses 80% of the wealth has an EW value
superior to the individual with 20% as can be observed in
Figure 4. This is additionally accompanied by a higher
level of minimum demand (U

) and an economic expec-
tancy of (U+).
 
ESI 0.2ESI 0.80.25962
4.3. Concentration of Wealth
4.3.1. Differential Growth
In order to understand the way in which people behave
between the two utility functions, U+ y U

, it is necessary
to analyze the marginal growth of both curves for each
given interval of wealth.
By marginal growth (decline) of a function f(x) in the
interval [x0, x
f], is understood the positive (negative)
value calculated through the expression:




0
0
0
,f
ff
f
xfx
xx fx

 (12)
4.3.2. Symmetric Interval Application
For the emotional Wellbeing Functions and their higher
Figure 5. ESI(wr) function under supposed emotional ra-
tionality.
and lower levels, marginal growth can be calculated at a
symmetric interval defined between wr and (1 – wr), with


12 1
rr
ww , as:







 


 





1
,1
ln 2ln1
ln 1
EW 1EW
,1 EW
ln 2ln1
ln1 sin
1
,1
ln 2ln1
ln 1
rr
rr
U
r
rr
r
rr
EW rr
r
rr
rr
rr
rr
U
r
rr
r
UwUw
ww Uw
awaw
aw bc
ww
ww w
awaw
awbw c
UwUw
ww Uw
awaw
aw bc








 



 
 


 

The numerator of the second expression (ΔEW) is ob-
tained through simplification using the property:



  


 
sinsin cossincos
sin sin
xxx
xx


 
Note that:


 


 




 


1sin 1
ln1ln1 sin
ln 1
1
ln 1
1
ln1 sin
1
ln 1
ln 2ln1
ln 1
ln 2ln1
ln1 sin
ln 2ln1
ln 1
r
rr
r
r
rr
r
rr
r
rr
rr
rr
r
w
awbcawbw c
aw bc
aw bc
awbw c
aw bc
awaw
aw bc
awaw
awbwc
awaw
aw bc

r

 


 

 

 
 
 


EW
,1,1 ,1
rrrrrr
UU
wwwwww

 
(13)
Expression (13) implies that the increase in demand


U
 is superior to the increase of EW (ΔEW) and su-
perior to the increase of economic expectations


U
through analysis of symmetric intervals. This implication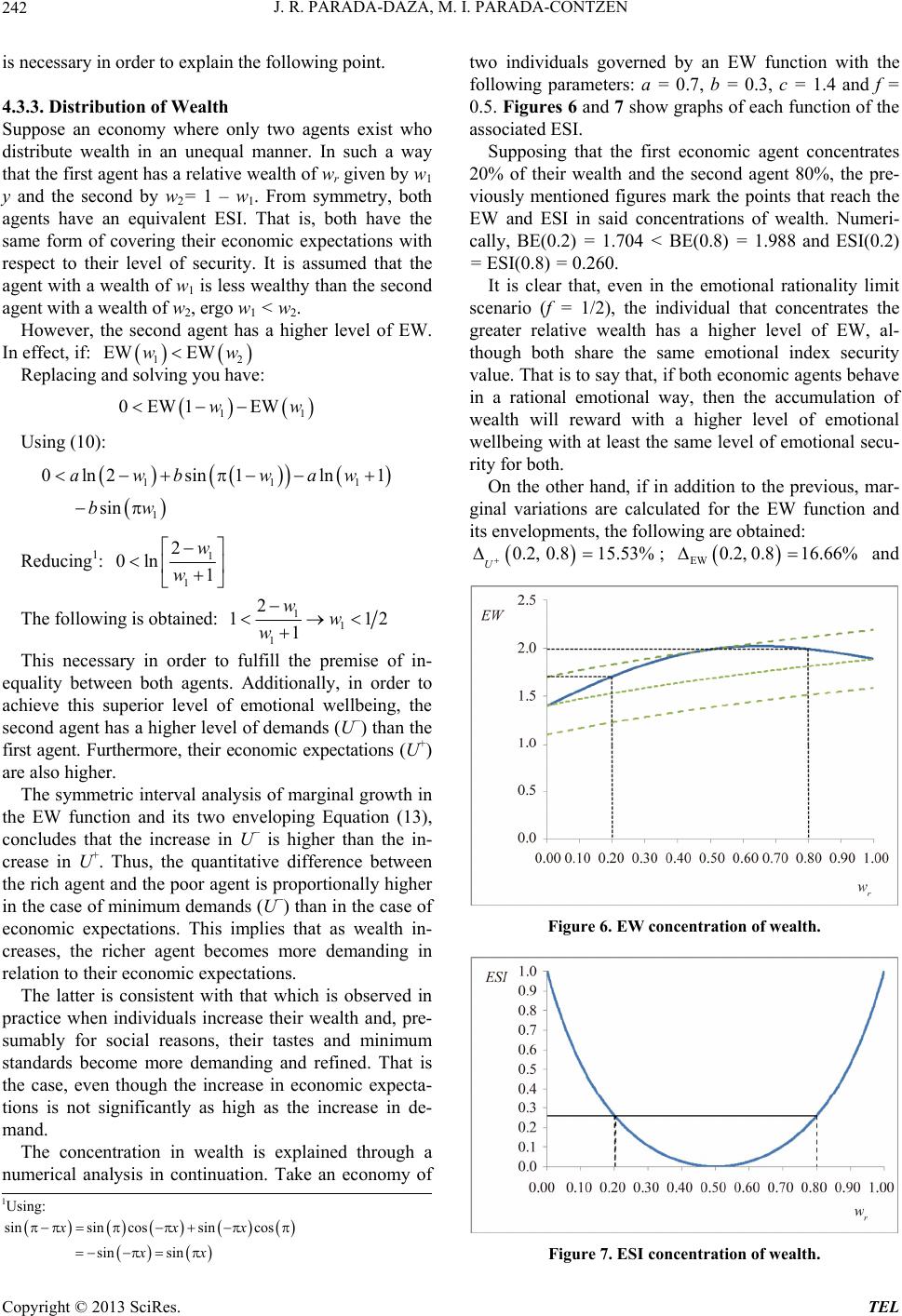

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 242
is necessary in order to explain the following point.
4.3.3. Distribution of Wealth
Suppose an economy where only two agents exist who
distribute wealth in an unequal manner. In such a way
that the first agent has a relative wealth of wr given by w1
y and the second by w2= 1 – w1. From symmetry, both
agents have an equivalent ESI. That is, both have the
same form of covering their economic expectations with
respect to their level of security. It is assumed that the
agent with a wealth of w1 is less wealthy than the second
agent with a wealth of w2, ergo w1 < w2.
However, the second agent has a higher level of EW.
In effect, if:
 
12
EW EWww
Replacing and solving you have:
 
11
0EW1 EWww

Using (10):


  

11
1
0ln2sin1ln 1
sin
awb waw
bw
 

1
Reducing1: 1
1
2
0ln 1
w
w





The following is obtained: 1
1
1
2
11
1
ww
w


2
This necessary in order to fulfill the premise of in-
equality between both agents. Additionally, in order to
achieve this superior level of emotional wellbeing, the
second agent has a higher level of demands (U−) than the
first agent. Furthermore, their economic expectations (U+)
are also higher.
The symmetric interval analysis of marginal growth in
the EW function and its two enveloping Equation (13),
concludes that the increase in U− is higher than the in-
crease in U+. Thus, the quantitative difference between
the rich agent and the poor agent is proportionally higher
in the case of minimum demands (U−) than in the case of
economic expectations. This implies that as wealth in-
creases, the richer agent becomes more demanding in
relation to their economic expectations.
The latter is consistent with that which is observed in
practice when individuals increase their wealth and, pre-
sumably for social reasons, their tastes and minimum
standards become more demanding and refined. That is
the case, even though the increase in economic expecta-
tions is not significantly as high as the increase in de-
mand.
The concentration in wealth is explained through a
numerical analysis in continuation. Take an economy of
two individuals governed by an EW function with the
following parameters: a = 0.7, b = 0.3, c = 1.4 and f =
0.5. Figures 6 and 7 show graphs of each function of the
associated ESI.
Supposing that the first economic agent concentrates
20% of their wealth and the second agent 80%, the pre-
viously mentioned figures mark the points that reach the
EW and ESI in said concentrations of wealth. Numeri-
cally, BE(0.2) = 1.704 < BE(0.8) = 1.988 and ESI(0.2)
= ESI(0.8) = 0.260.
It is clear that, even in the emotional rationality limit
scenario (f = 1/2), the individual that concentrates the
greater relative wealth has a higher level of EW, al-
though both share the same emotional index security
value. That is to say that, if both economic agents behave
in a rational emotional way, then the accumulation of
wealth will reward with a higher level of emotional
wellbeing with at least the same level of emotional secu-
rity for both.
On the other hand, if in addition to the previous, mar-
ginal variations are calculated for the EW function and
its envelopments, the following are obtained:


0.2, 0.815.53%
U
; and

EW 0.2, 0.816.66%
Figure 6. EW concentration of wealth.
1Using:
 
 
sinsin cossincos
sin sin
xxx
xx

  Figure 7. ESI concentration of wealth.

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 243

0.2,0.8 23.12%
U

This indicates that the change from a position of pov-
erty to a position of relative wealth is accompanied by an
increase of minimum demands by the agent. In this way
indicating that the rich agent is comparatively more de-
manding that the poor agent and that continuing to con-
centrate wealth provides greater comfort.
5. Conclusions
This paper has presented a theoretic outline in reference
to the Emotional Wellbeing function as an extension of
the economic utility function. After analytical-mathe-
matical conceptualization of the new function, a concept
of emotional security has been developed which, in turn,
is used to define a new idea of emotional rationality as a
complement to economic rationality. This concept allows
for the explanation of a more integrated vision with re-
spect to economic decisions whether they are related to
business or other everyday acts.
On the other hand, an analytical explication has been
put forth of the concentration of wealth phenomenon
through a function of rational emotional wellbeing.
In the introduction, it was stated that the economic
utility function may represent a biased approach when
trying to describe the behavior, for example, of a man-
ager in the decision making process. Using a utility func-
tion can lead to an incomplete description of the process
of economic decision. An Emotional welfare function,
however, includes factors usually neglected by the eco-
nomic rationalist approach, but always present in any
business with or without a profitable outcome. Therefore
it has been proposed that the role of Emotional Well-be-
ing may represent phenomenon related to both economic
and non-economic satisfaction of agents who make deci-
sions. In conclusion, this paper is an analytical attempt of
integration between a rational economic approach and a
management approach to explaining decision making in
different daily acts, whether they are for business or
other activities. Indeed, this paper uses an analytical lan-
guage, typical of the utility function, to show that mathe-
matical models are applicable to human behavior, and
that they can collect various influences of ethical schools
distinct to economic maximizing utilitarianism. In par-
ticular, the studied function of EW suggests that there are
at least two ways to consider the influence of these other
schools of thought on human behavior.
The first is the relationship between the a and b coef-
ficients of the function. It is shown that through the con-
sideration of these elements, a greater (or lower) relative
importance an agent or company gives to both the eco-
nomic maximizing component as well as the emotional
component of their behavior. Furthermore, it is con-
cluded that the parameter f represents the “type” of emo-
tionality that the economic agent values in their decisions.
This parameter is closely related to the concepts of emo-
tional security and emotional rationality.
These aspects were synthesized through the inclusion
and development of an emotional security index (ESI).
Regardless of the values of a and b, this is an indicator of
economic sacrifice in exchange for emotional satisfaction
obtained for each level of wealth. Thus, this indicator is a
periodic function that depends only on f. That is, the type
of emotionality of the individual. This indicator corre-
sponds to a theoretical novelty as it had not appeared
before in literary references. This is an important finding
because from the index develops the concept of emo-
tional rationality, which allows for a more convincing
justification of the strictly non-maximizing behavior of
economic agents. This new concept of emotional ration-
ality complements the economic rationality approach and
allows an integrated view of the explanation of decisions
to be generated.
A conclusion that is also relevant, thanks to the con-
cept of relative wealth introduced in this paper, is that an
application on the concentration of wealth is presented.
Indeed, it is concluded as the proposed EW function
helps to explain the phenomenon of concentration of
wealth through an emotional incentive to do so. This will
complement the analysis carried out to show the practical
and conceptual validity of the proposition of EW.
This paper concludes that the function of EW has a
new application, not thought of at first, that joins with
other applications mentioned in literature. Another con-
clusion of the analytical work, that whatever the form
adopted by utility functions most commonly used in
economic literature (quadratic, logarithmic, power, nega-
tive exponential or other), they are also envelopments of
an emotional wellbeing function. From this it can be
concluded that the utility functions are an explanation of
edge (or limit) behavior of people during their economic
acts.
Lastly, a sinusoidal characteristic of the emotional part
of the EW function is proposed here. However, the defi-
nition of EW function that has been given opens the op-
tion to use other features. In particular, it is only required
that the emotional characteristic is a bounded function on
its range to ensure the existence of enveloping functions.
Thus it is possible to define other characteristics (Fourier
series, stochastic functions, etc.) that can better represent
a particular decision making process.
REFERENCES
[1] J. R. Parada-Daza, “The Utility Function and the Emo-
tional Well-Being Function,” Electronic Journal of Busi-
ness Ethics and Organization Studies, Vol. 9, No. 2, 2004,
pp. 22-29.
[2] J. R. Parada-Daza, “A Valuation Model for Corporate

J. R. PARADA-DAZA, M. I. PARADA-CONTZEN 244
Social Responsibility,” Social Responsibility Journal, Vol.
5, No. 3. 2009, pp. 284-299.
[3] P. Brilius, “Economic Crisis and SMES Sustainability Po-
licies: Application of Emotional Well-Being Function for
Analysis,” Journal of Advanced Research in Management,
Vol. 1, No. 1, 2010, pp. 16-29.
[4] J. R. Parada-Daza, “Economic Ethics and Emotional
Well-Being,” Journal of Behavioral Studies in Business,
Vol. 6, No. 1, 2012, pp. 1-12.
[5] T. E. Copeland and J. F. Weston, “Finantial Theory and
Corporate Policy,” 3rd Edition, Addison-Wesley Publish-
ing Company, New York, 1992, p. 89.
[6] J. M. Marín and G. Rubio, “Economía Financiera,”
Antoni Bosch Editor, Barcelona, 2001, pp. 777-781.
[7] R. Merton, “Continuous-Time Finance,” Blackwell Pub-
lishers Ltd., Oxford, 1996, p. 138.
[8] R. Jarrow, “Finance Theory,” Prentice-Hall International
Editions, Upper Saddle River, 1988, p. 199.
[9] W. F. Sharpe, “Teoría de Cartera y del Mercado de
Capitales,” Ediciones Deusto S.A., Bilbao,1976, p. 237.
[10] J.-J. Laffont, “The Economics of Uncertainty and Infor-
mation,” The MIT Press, Cambridge, 1995, p. 8.
[11] C. D. Carroll, “Why Do the Rich Save Much?” The Johns
Hopkins University, Baltimore, 1998.
[12] J. Robinson, “Economic Philosophy,” Aldine, Chicago,
1962.
[13] G. Debreu, “La Théorie de la Valeur,” Dunod, Paris,
1966.
[14] H. Markowitz, “Portfolio Selection,” Yale University
Press, New Haven, 1959.
[15] J. W. Pratt, “Risk Aversion in the Small and in the Large,”
Econometrica, Vol. 32, No. 1-2, 1964, pp. 122-136.
doi:10.2307/1913738
[16] J. Ait-Sahalia and M. Brandt, “Variable Selection for Port-
folio Choise,” National Bureau of Economic Research
(NBER), 2001.
[17] A. Ang, G. Bekaert and J. Liu, “Why Stocks May Disap-
point?” EFA 0669, 2000.
[18] R. Mehra and E. Prescott, “The Equity Premium: A Puz-
zle,” Journal of Monetary Economy, Vol. 15, No. 2, 1985,
pp. 145-161. doi:10.1016/0304-3932(85)90061-3
[19] I. Friend and M. Blume, “The Demand for Risky Assets,”
American Economic Review, Vol. 65, No. 5, 1975, pp.
900-922.
[20] S. Hwang and S. Satchell, “Valuing Information Using
Utility Functions: How Much Should We Pay for Lineal
Factor Model?” The European Journal of Finance, Vol.
11, No. 1, 2005, pp. 1-16.
doi:10.1080/1351847042000286630
[21] H. Inhaber and S. Carroll, “How Rich Is Too Rich? In-
come and Wealth in America,” Prarger Publisher, New
York, 1992.
[22] J. C. T. Mao, “Quatitative Analysis of Financial Decision,”
The Macmillan Co., New York, 1969.
[23] B. Demidovich, “Análisis Matemático,” Undécima Edi-
ción, Edit Paraninfo, Madrid, 1993, p. 247.