Optics and Photonics Journal, 2013, 3, 50-52
doi:10.4236/opj.2013.32B012 Published Online June 2013 (http://www.scirp.org/journal/opj)
Nonlinear Cascaded Femtosecond Third Harmonic
Generation by Multi-grating Periodically Poled
MgO-doped Lithium Niobate
Shuanggen Zhang1*, Wenchao You1, Zhangchao Huang2
1School of Electronic Information Engineering, Tianjin University of Technology, Tianjin Key Laboratory of Film
Electronic and Communication Device, Engineering Research Center of Communication Devices Ministry of Education,
Tianjin University of Technology, Tianjin, China
2School of Physics, Nankai University, Tianjin, China
Received 2013
ABSTRACT
Nonlinear cascaded femtosecond third harmonic generation was experimentally investigated pumped by 100 fs pulses at
optical communication band 1550 nm using a multi-grating 5 mol. % MgO-doped periodically poled lithium niobate
crystal. The optimized efficiency of 10.8% was achieved with the simultaneous phase-matching of the second harmonic
and sum frequency process. And the third harmonic spectrum reached as broad as 8.7 nm because of the choosing of a
small group velocity mismatching between the fundamental and second harmonic pulses. Nonlinear cascaded method
will provide a reference for the efficient frequency conversion in the high intensity range.
Keywords: Femtosecond; Third Harmonic Generation; Second Harmonic Generation; Cascaded Nonlinear Process
1. Introduction
Frequency upconversion is a useful nonlinear process to
achieve short wavelength, and third harmonic generation
(THG) is one of the most effective upconversion process,
which can be used to generate optical emission with shorter
wavelength. Generally, third-order susceptibility of ma-
terial contributes to THG process, i. e., direct THG. Besides
direct THG method, cascaded THG by second harmonic
and sum frequency process is a more efficient method.
Higher conversion efficiency can be achieved with quad-
ratic nonlinearity using two cascaded nonlinear processes.
However, the most efficient conversion of cascaded
THG is achieved when the phase-matching conditions
for both second harmonic and sum frequency processes
are satisfied. In case of cw or quasi-cw regimes, the si-
multaneous phase-matching of the two parametric proc-
esses has been realized in several different structures in
the past two decades [1-3]. For two-dimension nonlinear
photonic crystals, they are often utilized to realize the
noncollinear THG [4-6]. Recently, collinear THG was
also achieved by a short-range-ordered two-dimension
nonlinear photonic crystal [7]. However, there are few
reports on cascaded THG of ultrashort pulses. N. Fujioka
realized noncollinear cascaded THG of femtosecond
pulses using two-dimension periodically poled lithium
niobate and THG efficiency of 8% with spectral width of
4 nm was obtained [8].
In this paper, we demonstrated a collinear cascaded
THG of femtosecond pulses with high intensity in a 5
mol. % MgO-doped periodically poled lithium niobate
crystal. In the single pass scheme, the optimal THG effi-
ciency of 10.8% was obtained at the input intensity of 74
GW/cm2. The TH spectral width reached as broad as 8.7
nm with a small group velocity mismatch (GVM) be-
tween fundamental pulses and SH pulses.
2. Experimental Configuration
In general, a periodic QPM material can provide only
one effective wave vector for a parametric process.
However, a QPM material with a period of

is also
possible to provide two effective wave vectors contrib-
uting to the two QPM processes, respectively. In such a
periodic QPM marital, the cascaded THG, which in-
volves the simultaneous SHG and SFG processes, with
the respective
I
k

and
I
I
k

as follows:
21 1
21 11
3212
32112
2
4()/2/
2(32)/2/
IQPM
II QPM
kk kk
nn m
kkkkk
nnnm



 
 


 
(1)
*Corresponding author.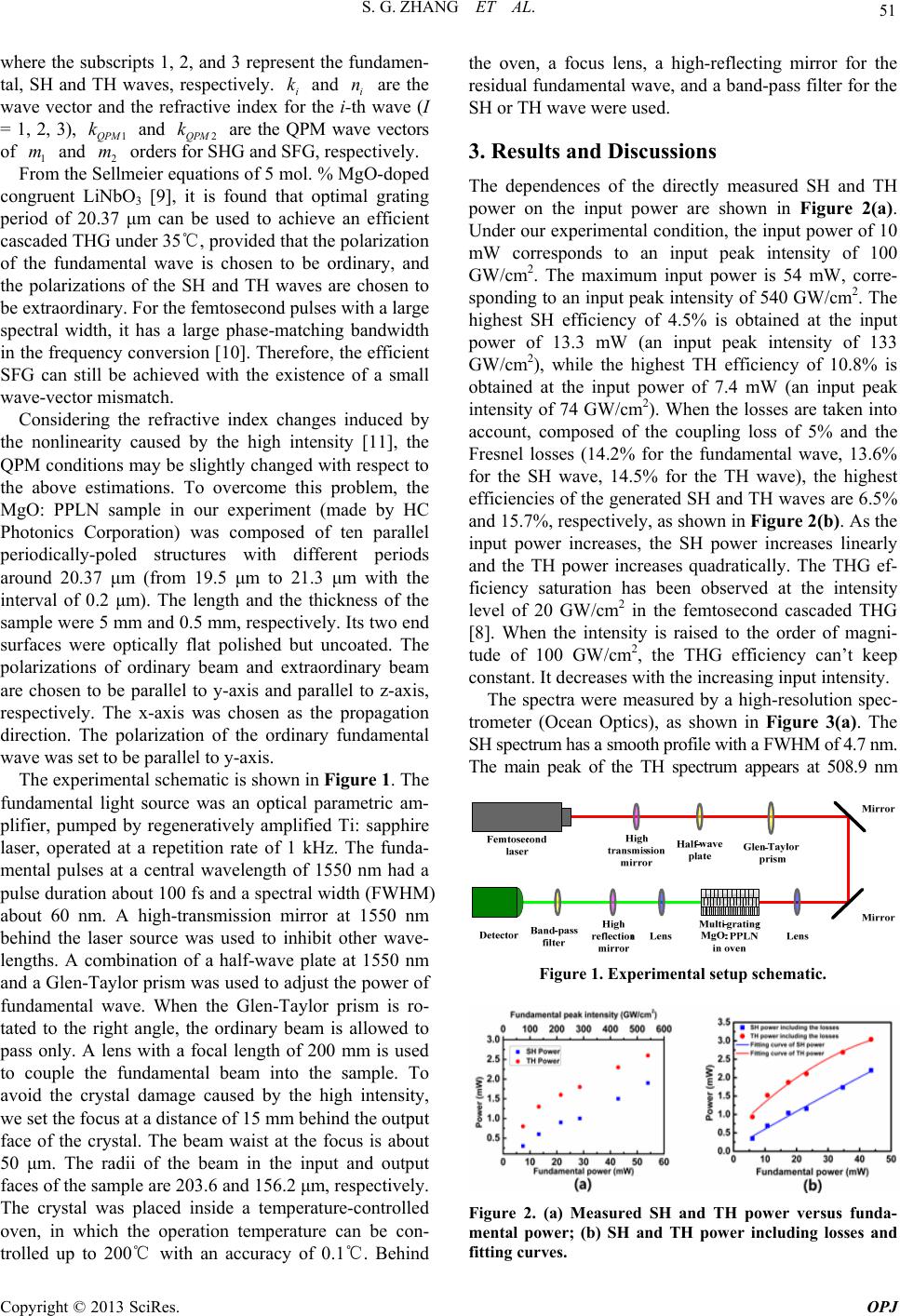

S. G. ZHANG ET AL. 51
where the subscripts 1, 2, and 3 represent the fundamen-
tal, SH and TH waves, respectively. i and i are the
wave vector and the refractive index for the i-th wave (I
= 1, 2, 3), 1 and 2QPM are the QPM wave vectors
of and orders for SHG and SFG, respectively.
k n
QPM
kmk
1 2
From the Sellmeier equations of 5 mol. % MgO-doped
congruent LiNbO3 [9], it is found that optimal grating
period of 20.37 μm can be used to achieve an efficient
cascaded THG under 35℃, provided that the polarization
of the fundamental wave is chosen to be ordinary, and
the polarizations of the SH and TH waves are chosen to
be extraordinary. For the femtosecond pulses with a large
spectral width, it has a large phase-matching bandwidth
in the frequency conversion [10]. Therefore, the efficient
SFG can still be achieved with the existence of a small
wave-vector mismatch.
m
Considering the refractive index changes induced by
the nonlinearity caused by the high intensity [11], the
QPM conditions may be slightly changed with respect to
the above estimations. To overcome this problem, the
MgO: PPLN sample in our experiment (made by HC
Photonics Corporation) was composed of ten parallel
periodically-poled structures with different periods
around 20.37 μm (from 19.5 μm to 21.3 μm with the
interval of 0.2 μm). The length and the thickness of the
sample were 5 mm and 0.5 mm, respectively. Its two end
surfaces were optically flat polished but uncoated. The
polarizations of ordinary beam and extraordinary beam
are chosen to be parallel to y-axis and parallel to z-axis,
respectively. The x-axis was chosen as the propagation
direction. The polarization of the ordinary fundamental
wave was set to be parallel to y-axis.
The experimental schematic is shown in Figure 1. The
fundamental light source was an optical parametric am-
plifier, pumped by regeneratively amplified Ti: sapphire
laser, operated at a repetition rate of 1 kHz. The funda-
mental pulses at a central wavelength of 1550 nm had a
pulse duration about 100 fs and a spectral width (FWHM)
about 60 nm. A high-transmission mirror at 1550 nm
behind the laser source was used to inhibit other wave-
lengths. A combination of a half-wave plate at 1550 nm
and a Glen-Taylor prism was used to adjust the power of
fundamental wave. When the Glen-Taylor prism is ro-
tated to the right angle, the ordinary beam is allowed to
pass only. A lens with a focal length of 200 mm is used
to couple the fundamental beam into the sample. To
avoid the crystal damage caused by the high intensity,
we set the focus at a distance of 15 mm behind the output
face of the crystal. The beam waist at the focus is about
50 μm. The radii of the beam in the input and output
faces of the sample are 203.6 and 156.2 μm, respectively.
The crystal was placed inside a temperature-controlled
oven, in which the operation temperature can be con-
trolled up to 200℃ with an accuracy of 0.1℃. Behind
the oven, a focus lens, a high-reflecting mirror for the
residual fundamental wave, and a band-pass filter for the
SH or TH wave were used.
3. Results and Discussions
The dependences of the directly measured SH and TH
power on the input power are shown in Figure 2(a).
Under our experimental condition, the input power of 10
mW corresponds to an input peak intensity of 100
GW/cm2. The maximum input power is 54 mW, corre-
sponding to an input peak intensity of 540 GW/cm2. The
highest SH efficiency of 4.5% is obtained at the input
power of 13.3 mW (an input peak intensity of 133
GW/cm2), while the highest TH efficiency of 10.8% is
obtained at the input power of 7.4 mW (an input peak
intensity of 74 GW/cm2). When the losses are taken into
account, composed of the coupling loss of 5% and the
Fresnel losses (14.2% for the fundamental wave, 13.6%
for the SH wave, 14.5% for the TH wave), the highest
efficiencies of the generated SH and TH waves are 6.5%
and 15.7%, respectively, as shown in Figure 2(b). As the
input power increases, the SH power increases linearly
and the TH power increases quadratically. The THG ef-
ficiency saturation has been observed at the intensity
level of 20 GW/cm2 in the femtosecond cascaded THG
[8]. When the intensity is raised to the order of magni-
tude of 100 GW/cm2, the THG efficiency can’t keep
constant. It decreases with the increasing input intensity.
The spectra were measured by a high-resolution spec-
trometer (Ocean Optics), as shown in Figure 3(a). The
SH spectrum has a smooth profile with a FWHM of 4.7 nm.
The main peak of the TH spectrum appears at 508.9 nm
Figure 1. Experimental setup schematic.
Figure 2. (a) Measured SH and TH power versus funda-
mental power; (b) SH and TH power including losses and
fitting curves.

S. G. ZHANG ET AL.
52
Figure 3. (a) SH spectra and mode; (b) TH spectra and
mode.
with a FWHM of 8.7 nm and another peak locates at
516.1 nm. The TH spectral width is more than two times
as the reported value of 4 nm by the cascaded THG of
118 fs pump pulses with a FWHM of 51 nm in the
two-dimension PPLN [8]. The measured TH mode in the
output face of the sample is shown in inset of Figure
3(b). A nearly circular profile indicates a good spatial
intensity distribution of THG.
During the cascaded processes, the TH bandwidth is
limited by GVM among fundamental, SH and TH pulses.
However, the GVM between the fundamental and SH
pulses is the most important because it directly deter-
mines the effective interaction length of the SFG process
[8]. In our experiment, the small GVM between the fun-
damental and SH pulses mainly results in the generation
of the broadband TH wave. We choose the SHG type
(o+o--e) that the fundamental and SH pulses have dif-
ferent polarizations. In the 5 mol. % MgO-doped con-
gruent lithium niobate crystal, the GVM between ordi-
nary fundamental wave (1550 nm) and extraordinary SH
wave (775 nm) is only 155.6 fs/cm, while that is 3034
fs/cm for the SHG type (e+e--e) that the polarizations of
the fundamental and SH pulses are both extraordinary [12].
4. Conclusions
Efficient cascaded femtosecond THG using a 5 mol. %
MgO-doped multi-grating periodically poled crystal was
experimentally demonstrated. The optimal TH efficiency
of 10.8% was obtained in case of simultaneous phase
matching of SHG and the SFG. The TH spectral width
reached as abroad as 8.7 nm with a small GVM between
the fundamental and SH pulses. Such nonlinear cascaded
process will provide a reference for the efficient fre-
quency conversion in the high intensity range.
5. Acknowledgements
This work was supported by the National Natural Sci-
ence Foundation of China (11004152), Program of Tian-
jin Municipal Education Commission (20090715).
REFERENCES
[1] K. Kintaka, M. Fujimura, T. Suhara and H. Nishihara,
“Third Harmonic Generation of Nd: YAG Laser Light in
Periodically Poled LiNbO3”, Electronic Letters, Vol. 33,
No. 17, 1997, pp. 1459-1461. doi:10.1049/el:19970990
[2] S. N. Zhu, Y. Y. Zhu and N. B. Ming, “Quasi-Phase-
Matched Third Harmonic Generation in a Quasi- Periodic
Optical Superlattice,” Science, Vol. 278, No. 5339, 1997,
pp. 843-846. doi:10.1126/science.278.5339.843
[3] C. Zhang, H. Wei, Y. Y. Zhu, H. T. Wang, S. N. Zhu and
N. B. Ming, “Third-Harmonic Generation in A General
Two-Component Quasi-Periodic Optical Superlattice,”
Optics Letters, Vol. 26, No. 12, 2001, pp. 899-901.
doi:10.1364/OL.26.000899
[4] N. G. R. Broderick, R. T. Bratfalean, T. M. Monro, D. J.
Richardson and C. M. de Sterke, “Temperature and
Wavelength Tuning of Second-, Third-, and Fourth-Har-
monic Generation in A Two-Dimensional Hexagonally
Poled Nonlinear Crystal,” Journal of the Optical Society
of America B, Vol. 19, No. 9, 2002, pp. 2263-2272.
doi:10.1364/JOSAB.19.002263
[5] A. H. Norton and C. M. de Sterke, “Optimal Poling of
Nonlinear Photonic Crystals for Frequency Conversion,”
Optics Letters, Vol. 28, No. 3, 2003, pp. 188-190.
[6] A. H. Norton and C. M. de Sterke, “Two-Dimensional
Poling Patterns for 3rd and 4th Harmonic Generation,”
Optics Express, Vol. 11, No. 9, 2003, pp. 1008-1014.
doi:10.1364/OE.11.001008
[7] Y. Sheng, S. M. Saltiel and K. Koynov, “Cascaded
Third-Harmonic Generation in A Single Short-Range
-Ordered Nonlinear Photonic Crystal,” Optics Letters,
Vol. 34, No. 5, 2009, pp. 656-658.
doi:10.1364/OL.34.000656
[8] N. Fujioka, S. Ashihara, H. Ono, T. Shimura and K. Ku-
roda, “Cascaded Third-Harmonic Generation of Ul-
trashort Optical Pulses in Two-Dimensional Quasi-Phase-
Matching Gratings,” Journal of the Optical Society of
America B, Vol. 24, No.9, 2007, pp. 2394-2405.
doi:10.1364/JOSAB.24.002394
[9] D. E. Zelmon, D. L. Small and D. Jundt, “Infrared Cor-
rected Sellmeier Coefficients for Congruently Grown
Lithium Niobate and 5 mol.% Magnesium Oxide –Doped
Lithium Niobate,” Journal of the Optical Society of
America B, Vol. 14, No. 12, 1997, pp. 3319-3322.
doi:10.1364/JOSAB.14.003319
[10] Z. C. Huang, C. H. Tu, S. G. Zhang, Y. N. Li, F. Y. Lu, Y.
X. Fan and E. B. Li, “Femtosecond Second-Harmonic
Generation in Periodically Poled Lithium Niobate
Waveguides Written by Femtosecond Laser Pulses,” Op-
tics Letters, Vol. 35, No. 6, 2010, pp. 877-879.
doi:10.1364/OL.35.000877
[11] DeSalvo, A. A. Said, D. J. Hagan, E. W. Van Stryland,
and M. Sheik-Bahae, “Infrared to Ultraviolet Measure-
ments of Two-Photon Absorption and N2 in Wide Band-
gap Solids,” IEEE Journal of Quantum Electronics,
Vol.32, No. 8, 1996, pp. 1324-1333.
doi:10.1109/3.511545
[12] N. E. Yu, J. H. Ro, M. Cha, S. Kurimura and T. Taira,
“Broadband Quasi-Phase-Matched Second-Harmonic
Generation in MgO-Doped Periodically Poled LiNbO3 at
the Communications Band,” Optics Letters, Vol. 27, No.
12, 2002, pp. 1046-1048. doi:10.1364/OL.27.001046