Journal of Modern Physics, 2013, 4, 22-27
http://dx.doi.org/10.4236/jmp.2013.47A2004 Published Online July 2013 (http://www.scirp.org/journal/jmp)
Local Stability of Curzon-Ahlborn Cycle with Non-Linear
Heat Transfer for Maximum Power Output Regime
Delfino Ladino-Luna, Pedro Portillo-Díaz, Ricardo T. Páez-Hernández
Universidad Autónoma Metropolitana-Atzcapotzalco, Física de Procesos Irreversibles, Cd. México, México
Received May 7, 2013; revised June 9, 2013; accepted July 4, 2013
Copyright © 2013 Delfino Ladino-Luna et al. This is an open access article distributed under the Creative Commons Attribution
License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
ABSTRACT
The study of local stability of thermal engines modeled as an endoreversible Curzon and Ahlborn cycle is shown. It is
assumed a non-linear heat transf er for heat flux es in the system (eng ine + env iron ments). A semisum of two expression s
of the efficiency found in the literature of finite time thermo dynamics for the maximum power output regime is consid-
ered in order to make the analysis. Expression of variables for local stability and power output is found even graphic
results for important parameters in the analysis of stability, and a phase plane portrait is shown.
Keywords: Local Stability; Thermal Engines; Non-Linear Heat Transfer
1. Introduction
As it is known the limits in the performance of thermal
engines in th e Clas sica l Equ ilibrium Thermodynamics con-
text correspond to reversible processes [1-4]. This situa-
tion represents a very hard obstacle in the analysis of
thermal engines and leads to non adequate values for va-
riables of processes whose values far from to the experi-
mental values were reported in the literature. These limits
have been partially overcome by helping of the named
Finite Time Thermodynamics [5-7]. In order to analyze
the performance of thermal engines, many papers in this
context have considered that the heat flux between the
system and its environs is made by Newton heat transfer
law [5-14], for the named Curzon and Ahlborn engine [7].
Nevertheless a more real model has to consider all possi-
bilities of heat transfer. Thus, some authors have used
particularly the Dulong and Petit heat transfer law be-
cause it allows a better model than the Newton heat trans-
fer model is [15-18]. In [15-17] numerical results appear
near to the experimental values reported in the literature
for power plants working at maximum power output, and
in [17,18] for nuclear plants working at maximum ecolo-
gical function [14].
It is important to point out that all of the above-cited
papers have been focused on the thermodynamics prop-
erties of the system, through an objective function to an a-
lyze the performance of thermal engines, and only the
steady state has been analyzed.
Nevertheless, other authors have analyzed the intrinsic
properties of the systems as the response to a perturba-
tion on the steady state of important quantities for the per-
formance of thermal engines [19,20]. More recently the
local stability of thermal engines has been made consi-
dering Newton heat transfer [21-24] and Stefan-Boltz-
mann heat transfer [25], besides it has been made con-
sidering a working substance different to ideal gas [26].
In the present paper, we consider a heat transfer like
the Dulong-Petit heat transfer law in order to make the
analysis of a thermal engine for local stability. The im-
portant quantities in the performance of the thermal en-
gine are found by the heat transfer cited, as the expres-
sion of power output and the dynamic equations for an
endoreversible Curzon and Ahlborn engine. We assume
for simplicity an expression of efficiency as a semi-sum
of two expressions found by two different authors [16-
18], which contains the same necessary parameters of the
present work, including a comparison by plotting of them.
To make this paper self-contained, a review of some well-
known results on the Carnot, and Curzon and Ahlborn
engines concerning to steady state variables is also inclu-
ded.
2. Properties of the Steady States
2.1. Steady States Variables
Let us consider a system which consists of two reservoirs
at temperatures (hot reservoir) and (cold reser-
1
T2 T
C
opyright © 2013 SciRes. JMP

D. LADINO-LUNA ET AL. 23
voir), which are related as 12 ; and the thermal en-
gine working at temperatures x and y, which are related
as
TT
x
y
xyT
. There is a resistance for the heat flow between
the thermal engine and its reservoirs with a heat conduc-
tance denoted by α, as is shown in Figure 1. In case of
Carnot engine, 1 and 2 T

, and in case of Cur-
zon and Ahlborn engine the temperatures are related by
12
. The engine produces the work W. The
heat 1 flows from the hot reservoir to the engine and
the heat 2 flows from the engine to the cold reservoir,
assuming a constant thermal conductance by the given
parameter
Tx
Q
y
Q
T

in both fluxes.
According to the first and second laws of thermody-
namics, for Carnot engine the heats exchanged in the sys-
tem and are given by
1
Q2 Q
1
x
QW
x
y
 (1a)
and
2.
y
QW
x
y
 (1b)
For the endorev ersible Curzon and Ahlborn eng ine we
can consider an engine working in steady state, so the
temperatures are now
x
and
y
with 12 Tx
[20]. Here, and thereafter, the variables with over-bars
represent steady state quantities. The endoreversible hy-
pothesis is now,
yT
12
12
J
J
TT
 (2)
which asserts that an engine working between two reser-
voirs at temperatures
x
and
y
behaves as a Carnot
Figure 1. Thermal engine working between both x and y
temperatures. The reservoirs are at temperatures and
. The fluxes to the engine and from the engine are respec-
tively
T1
2
T
J
1 and
J
2.
engine, despite the fact that it works in finite-time cycles
[10].
J
and 21
J
are the steady state heat flows from
the
x
to the engine and from the engine to
y
, respec-
tively, and because the Curzon and Ahlborn engine is
usually supposed working in steady state, the fluxes be-
fore mentioned are


11
J
Tx 
(3a)


and


22
J
.yT   (3b)
This means that
1
x
J
P (4a)
x
y

and
2
y
J
P (4b)
x
y

P is the power output in steady state. The efficiency
in steady state for this internally reversible Curzon and
Ahlborn engine is written as
y
(5) 1 


x
and by using (4b) we can write
2
1
1JWP
J
  
1
P
or , (6)  
J
and it follows that
121
1
21
TTT
x






(7a)
and

121
11
21
TTT
y 


 



. (7b)
2.2. Effect of a Non-Linear Heat Transfer Law
Consider now a heat transfer law as,

0
d,
d
k QTT
t
  (8)
which contains as a particular case the Dulong and Petit
heat transfer law, where ddQt is the rate of heat trans-
fer,

is the thermal conductance, 0 is the tempera-
ture of environs, T is the temperature of th e body and k is
a parameter as , which in case of Dulong and Petit
heat transfer is as
T
1k
1.1 1.6k [27,28].

Heat fluxes
J
12
,J can be written now as

11
k
J
Tx   and

22
,1
k
JyTk   , (9)
and (6) with (9) per mits

D. LADINO-LUNA ET AL. 24


1
2
,
k
k
Tx
xy
yT


 (10)
and then,


1
1,
11
k
k




1
1
xT (11)
and,


1
1,
11
k
k




1
1
1yT
  (12)
where has been defini t e 21
TT

.
On other hand, the Curzon and Ahlborn engine gives
more realistic values of efficiency with this heat transfer
than it gives with the Newton heat transfer, in case of
maximum power output regime [15]. Results in [14,15]
are compared with different reported values of power
plants. More recently, at maximum power output regime
and at maximum ecological function regime, assuming
54k, analytical approximated expressions for the ef-
ficiency were found as [16,18],
2981
10
   1
1
OPDP


 (13a)
and
2646 1
1.
  1 649
36
OEDP



 (13b)
Besides, by a variational approach, the efficiencies in
the maximum power output regime and in the ecological
function regi me were obtained res pect i vel y [1 7] as,


1
88
MP

12
160,


   (14a)
and


1
1
88
ME

4 041.


   (14b)
Analyzing the results of several studies in the literature
of finite time thermodynamics, it is found that the effi-
ciency of Curzon and Ahlborn cycle CAN

, named Cur-
zon-Ahlborn-Novikov efficiency, is adequate for con-
ventional plants, and the named ecological efficiency
E

is adequate for modern plants (including nuclear
plants). So, we consider hereafter a thermal engine in the
maxim um power output r egime.
Comparing the efficiencies in (13a) and (14a) the fol-
lowing property is found,

,
OBS MP

 
OPDP (15)
and the semisum



 12
SOPDPMP
is closer than
the efficiencies in (15) to experimental efficiencies.
Table 1 shows a comparison between efficiencies in
(15), for the case of some conventional power plants
working in a maximum power output regime, reported in
[16,17]. Even more, the difference between the eficien-
cies (13a) and (14a) is shown in Figure 2. As can be seen,
most of the numerical values of half the sum of the above
cited efficiencies are an approximated constant ratio of
Curzon and Ahlborn efficiency as,



1 20.88,
OPDP MPCAN

   (16)
where the Curzon-Ahlborn-Novikov efficiency is
1   . Besides, if the Carnot efficiency CCAN


is
considered, a numerical factor of the semisum appears
also as an approximated constant ratio of this efficiency.
It can be verified that the semisum


12 PDPMP

 
SO
is related with the Carnot efficiency as,



1 20.55.
OPDP MPC

 
(17) 
So, in order to analyze the local stability for a Curzon
and Ahlborn engine it can be assumed the previous value
for the efficiency, when the Dulong and Petit heat trans-
fer law is considered. Figure 3 shows the difference of
efficiencies in (17) as function of the parameter

,
where it can be appreciated that the difference goes to
zero, when 111
b
aba
2
T1 T

.

Using the linear approximation

, in
Table 1. Efficiency at maximum power output regime.
OPDP

OBS
Power plant

M
P


Steam power plant, West
Thurrock, U K 298838 0.37625 0.335770.360
Geothermal steam plant,
Lardarello, Italy 353523 0.16198 0.145300.160
Steam power plant, USA298923 0.40380 0.360060.400
Combined cycle plant
(steam-mercury), USA 298783 0.35620 0.318040.340
Central steam power (UK
1936-1940) 298698 0.32089 0.286780.280
Figure 2. Graphic comparison of the efficiencies
.

,,
OPDPMP CAN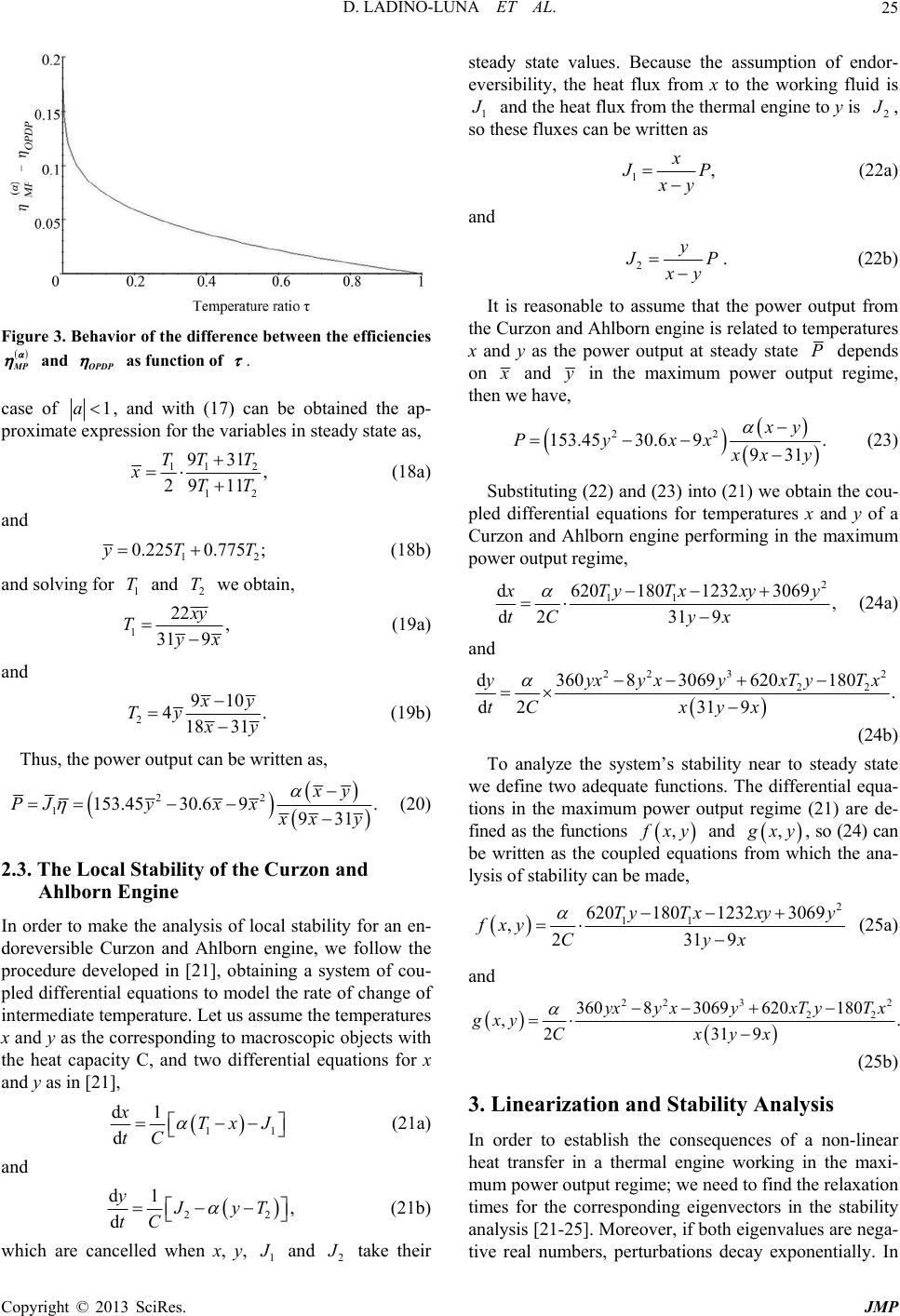



D. LADINO-LUNA ET AL. 25
Figure 3. Behavior of the difference between the efficiencies

M
P


and OPDP

as function of

.
case of 1a, and with (17) can be obtained the ap-
proximate expression for the variables in steady state as,
11 2
12
931
,
29 11
TT T
TT

  x (18a)
and
12
0.775 ;yTT
1
T2
0.225 (18b)
and solving for and T we obtain,
122 ,
31 9
x
y
yx
 T (19a)
and
2910
4.
18 31
x
y
Ty
x
y


 (19b)
Thus, the power output can be written as,



22
1153.4530.69PJyx x.
931
xy
x
xy
 

    (20)
2.3. The Local Stability of the Curzon and
Ahlborn Engine
In order to make the analysis of local stability for an en-
doreversible Curzon and Ahlborn engine, we follow the
procedure developed in [21], obtaining a system of cou-
pled differential equations to model the rate of change of
intermediate temperature. Let us assume the temperatures
x and y as the corresponding to macroscopic objects with
the heat capacity C, and two differential equations for x
and y as in [21],

11
Tx J

d1
d
x
tC
  (21a)
and

22
,Jy T   

1
d1
d
y
tC
 (21b)
which are cancelled when x, y,
J
and 2
J
take their
steady state values. Because the assumption of endor-
eversibility, the heat flux from x to the working fluid is
1
J
and the heat flux from the thermal engine to y is 2
J
,
so these fluxes can be written as
1,
x
J
P
x
y
 (22a)
and
2
y
J
P. (22b)
x
y

It is reasonable to assume that the power output from
the Curzon and Ahlborn engine is related to temperatures
x and y as the power output at steady state P depends
on
x
and
y
in the maximum power output regime,
then we have,



22
153.4530.6 9.
931
xy
Pyxx
x
xy
 
 (23)
Substituting (22) and (23) into (21) we obtain the cou-
pled differential equations for temperatures x and y of a
Curzon and Ahlborn engine performing in the maximum
power output regime,
2
11
6201801232 3069 d,
d2 319
Ty Txxyy x
tC yx
  
  (24a)
and

22 32
22
36083069 620180d.
d2 319
y
xyxyxTy Txy
tC xyx
  
 
(24b)
To analyze the system’s stability near to steady state
we define two adequate functions. The differential equa-
tions in the maximum power output regime (21) are de-
fined as the functions


,xy

, and
f
g
xy

, so (24) can
be written as the coupled equations from which the ana-
lysis of stability can be made,
2
11
6201801232 3069
,2319
Ty Txxyy
fxyCyx
  
 

(25a)
and

22 32
22
36083069 620180
,.
2319
yxyxyxTyT x
gxy Cxyx
  
 
(25b)
3. Linearization and Stability Analysis
In order to establish the consequences of a non-linear
heat transfer in a thermal engine working in the maxi-
mum power output regime; we need to find the relaxation
times for the corresponding eigenvectors in the stability
analysis [21-25]. Mor eover, if both eig envalues are nega-
tive real numbers, perturbations decay exponentially. In

D. LADINO-LUNA ET AL. 26
this case it is possible to identify the characteristic time
scales for each eigendirections as,
11
1t

 (26a)
and
22
1t


,
(26b)
where 12

 are the corresponding eigenvectors. In the
present case with the heat transfer law assumed we ob-
tain the following results for the derivative of definite
functions (25):




22
672
9 11,
57 10

,
528.55 931000
8918.65 101.0
xxy
fC 





  (27a)



67
,
61
14 314 4
4.95
8918.65 101.05710
1.7983 101.5415 107
1.83 101.1175 10
yxy
fC


2
0132
.478 10

 

 

 
,
(27b)







2
2
672
22
775
10
0.66884 ,


14
,
2
2
1981175109 40
8918.65 101.057
9 111.6356
931
xxy
g
C










 (28a)




22
2
672
22
9 11
.057 10
.





0.5
,
2
198940775
8918.65 101
1.6356 0.66884
931
yxy
g
C






(28b)
Substituting into the characteristic equation [22-26],
and taking as an example


, we obtain the eigen-
values,
7
1.042710 ,
5202.6




62
0.99956
.0509 10.


1
2






 (29)
and their respective eigenvectors,
7
1
2
4.7452 10,
9.9994 10,1

 

 


u
u (30)
Because both of the eigenvalues are real values, we
can conclude that the fixed po int is stable. Equation s (17)
and (20), respectively, determine the steady-state effi-
ciency,

, and the steady state power output, P, as
functions of 21
TT

 for an endore versible Curzon and
Ahlborn engine working in a maximum-power-like re-
gime. It is straightforward shows that both

and
are decreasing function of

parameter, as is shown in
Figure 4.
P
Both eigenvectors, 1

and 2

are function of

and
consequently relaxation times also are. There is an inter-
val of values for relaxation times 1 and 2
t in which
they are monotone-function of t

, and in the values
01


 it is, as we can appreciate in Figure 5. Finally,
Figure 6 shows how all the trajectories slowly approach
the origin in a tangential direction, so we can conclude
that the origin is a stable point
4. Concluding Remarks
The present work was focused on the analysis of conse-
quences in the stability of thermal engine when a non-li-
near heat transfer law is assumed. Graphic analysis shows
that the engine working in these conditions is near to th e
steady state as it is shown in Figures 5 and 6. Combining
two expressions for efficiency as a middle of them near
to experimental results was an adequate decision because
it permits us to have an expression as fraction of Carnot’s
efficiency. A comparison with other results in the litera-
ture is necessary. It is also necessary to point out that in
(23) and (24) some terms in these expressions were ap-
Figure 4. Steady state power output and efficiency as func-
tion of

.
Figure 5. Relaxation times and , in units of t1t2C

vs

.

D. LADINO-LUNA ET AL. 27
[10] M. Rubin, Physical Review A, Vol. 19, 1979, pp. 1277-
1289. doi:10.1103/PhysRevA.19.1277
[11] P. Salamon, A. Nitzan, B. Andresen and R. S. Berry, Phy-
sical Review A, Vol. 21, 1980, pp. 2115-2129.
doi:10.1103/PhysRevA.21.2115
[12] J. L. Torres, Revista Mexicana de Física, Vol. 34, 1988,
pp. 18-24.
[13] F. Angulo-Brown, Revista Mexicana de Física, Vol. 37,
1991, pp. 87-96.
[14] F. Angulo-Brown, Journal of Applied Physics, Vol. 69,
1991, pp. 7465-7469. doi:10.1063/1.347562
[15] F. Angulo-Brown and R. T. Páez-Hernández, Journal of
Applied Physics, Vol. 74, 1993, pp. 2216-2219.
doi:10.1063/1.354728
[16] D. Ladino-Luna, Revista Mexicana de Física, Vol. 48,
2003, pp. 87-91.
Figure 6. Qualitative phase portrait of


xt and


yt
for a Curzon-Ahlborn cycle using the efficiency in (17). [17] L. A. Arias-Hernández, G. Ares and F. Angulo-Brown, Open Systems & Information Dynamics, Vol. 10, 2003,
pp. 351-375. doi:10.1023/B:OPSY.0000009556.27759.11
proximated without losing the objective of numerical cal-
culation. [18] D. Ladino-Luna, Journal of the Energy Institute, Vol. 81,
2008, pp. 114-117. doi:10.1179/174602208X301961
[19] A. Lifschitz and E. Hameiri, Physical Fluids A, Vol. 3,
1991, pp. 2644-2651. doi:10.1063/1.858153 5. Acknowledgements
[20] G. Gallavotti, “New Methods in Noequilibrium Gases and
Fluids,” arXiv:chao-dyn/9610018v1 [accesed 30.10.1996].
Authors thank the partial support of CONACYT (México)
by SNI program. [21] M. Santillán, G. Maya and F. Angulo-Brown, Journal of
Physics D, Vol. 34, 2001, pp. 2068-2072.
doi:10.1088/0022-3727/34/13/318
REFERENCES [22] R. T. Páez-Hernández, F. Angulo-Brown and M. Santil-
lán, The Journal of Non-Equilibrium Thermodynamics,
Vol. 31, 2006, pp. 173-188.
[1] S. Carnot, “Réflexions sur la Puissance Motrice du feu et
sur les Machines Propres à Développer Cette Puissanc,”
Spanish Translation, IPN Press, México, 1998. [23] Y. Huang and D. Sun, The Journal of Non-Equilibrium
Thermodynamics, Vol. 33, 2008, pp. 61-74.
[2] R. Clausius, “Théorie Mécanique de la Chaleur, 1ére Par-
tie,” Lacroix, 1868. [24] Y. Huang, D. Sun and Y. Kang, Journal of Applied Phys-
ics, Vol. 102, 2007, pp. 349051-349056.
doi:10.1063/1.2767622
[3] E. Fermi, “Thermodynamics,” Columbia University Press,
New York, 1936.
[4] R. C. Tolman and P. C. Fine, Reviews of Modern Physics,
Vol. 20, 1948, pp. 51-77.
doi:10.1103/RevModPhys.20.51
[25] L. Guzmán-Vargas, I. Reyes-Ramírez and N. Sánchez,
Journal of Physics D: Applied Physics, Vol. 38, 2005, pp.
1282-1295. doi:10.1088/0022-3727/38/8/028
[5] I. I. Novikov, Journal of Nuclear Energy II, Vol. 7, 1958,
pp. 125-128. [26] R. T. Páez-Hernández, D. Ladino-Luna and P. Portillo-
Díaz, Physica A, Vol. 390, 2011, pp. 3275-3282.
doi:10.1016/j.physa.2011.05.019
[6] P. Chambadal, “Les Centrales Nucleares,” Armand Colin,
1957. [27] L. W. Taylor, “Manual of Adva nced Undergraduate Expe-
riments in Physics,” Addison-Wesley, 1959, p. 115.
[7] F. L. Curzon and B. Ahlborn, American Journal of Phys-
ics, Vol. 43, 1975, pp. 22-24. doi:10.1119/1.10023 [28] M. Nelson and P. Parker, “Advanced Level Physics,” 4th
Edition, Heinemann, New Orleans, 1977, p. 194.
[8] D. Gutkowics-Krusin, I. Procaccia and J. Ross, Journal of
Chemical Physics, Vol. 69, 1978, pp. 3898-3906.
doi:10.1063/1.437127
[9] M. Rubin, Physical Review A, Vol. 19, 1979, pp. 1272-
1276. doi:10.1103/PhysRevA.19.1272