Journal of Modern Physics, 2010, 1, 357-359
doi:10.4236/jmp.2010.16050 Published Online December 2010 (http://www.SciRP.org/journal/jmp)
2∆0/kBTc Ratio and Temperature Dependence of the
Superfluid Density in Overdoped La2-xSrxCuO4
Mikhail Eremin, Daniil Sunyaev
Institute of Physics, Kazan Federal University, Kazan, Russia
Received August 10, 2010; revised September 15, 2010; accepted September 20; 2010
Abstract
Using band structure parameters extracted from photoemission data by Yoshida et al., Phys. Rev. B., 2006, we
have analyzed the temperature dependence of the superfluid density in overdoped La2-xSrxCuO4.
We point out that the temperature behavior 1/λab2 is very sensitive to the ratio 2∆0/kBTc and have estimated this
quantity, using experimental data obtained previously by Panagopoulos et al., Phys. Rev. B., 1999. We com-
pare the results with those obtained from NMR/NQR (Nuclear Magnetic Resonance/Nuclear Quadrupole
Resonance) (Mayer et al., J. Phys, Cond. Mat., 2007) and scanning tunneling spectroscopy (Kato et al., Physica
C, 2007) data.
2
/1 abs n  
Keywords: Supefluid Density, Overdoped La2-xSrxCuO4, Short Range Pairing
1. Introduction
Up to now there is no consensus about the nature of su-
perconductivity in layered cuprates. In this context it is
important to study the temperature dependencies of the
superfluid density because this quantity is directly related
to superconductivity. At the same time, its analysis re-
quires preliminary information about the Fermi surface
(band structure parameters), the symmetry and tempera-
ture dependence of the superconducting gap and a trans-
parent description of the London penetration depth
within tight binding approximation. At the moment all
this information is available for La2-xSrxCuO4 supercon-
ductor and in what follows we analyze the temperature
dependence of superfluid density and compare the results
with available experimental data by Panagopoulos [1].
Won and Maki [2] were first who pointed out that in case
d-wave pairing the ratio 2Δ0/kBTc can be different from
its standard BCS value 3.52. However their calculations
were performed in week coupling approximation which
in the strict sense is not appreciable for layered cuprates.
Our main result is that the temperature behavior of
1/λab2 depends sensitively on the ratio 2∆0/kBTc and we
estimate this quantity for La2-xSrxCuO4 with for the dop-
ing concentration x = 0.20, x = 0.22 and 0.24.
2. Calculations of Superfluid Density
The superconducting current density is proportional to a
vector potential and written as (London’s equation):
2.
4
sc
j

 A (1)
Here λ – is a so-called London’s penetration depth of
external magnetic field into a superconductor (magnetic
penetration depth). This quantity is measured by various
experimental techniques [1,3]. Obviously its temperature
and doping dependencies contain important information
about fundamental microscopic properties of a super-
conductor. The microscopic expression for superfluid
density ns ~ 1/λab2 for layered cuprates is discussed in
detail in [4] (and Refs there in). It is written as follows:
2
2
22
22
1()
4.
2
1tanh 2
kk
x k
k
xk
k
k
xx
k
k
kk B
k E
k
e
ck
E
E
EE kT











 


 





   




 
 





 



 







 (2)
Here it is assumed that the magnetic field is applied
along the x-axis in CuO2-plane (ab). εk is the energy dis-
persion of quasiparticles in the normal state, μ is the
chemical potential,

22
kk k
E   (3)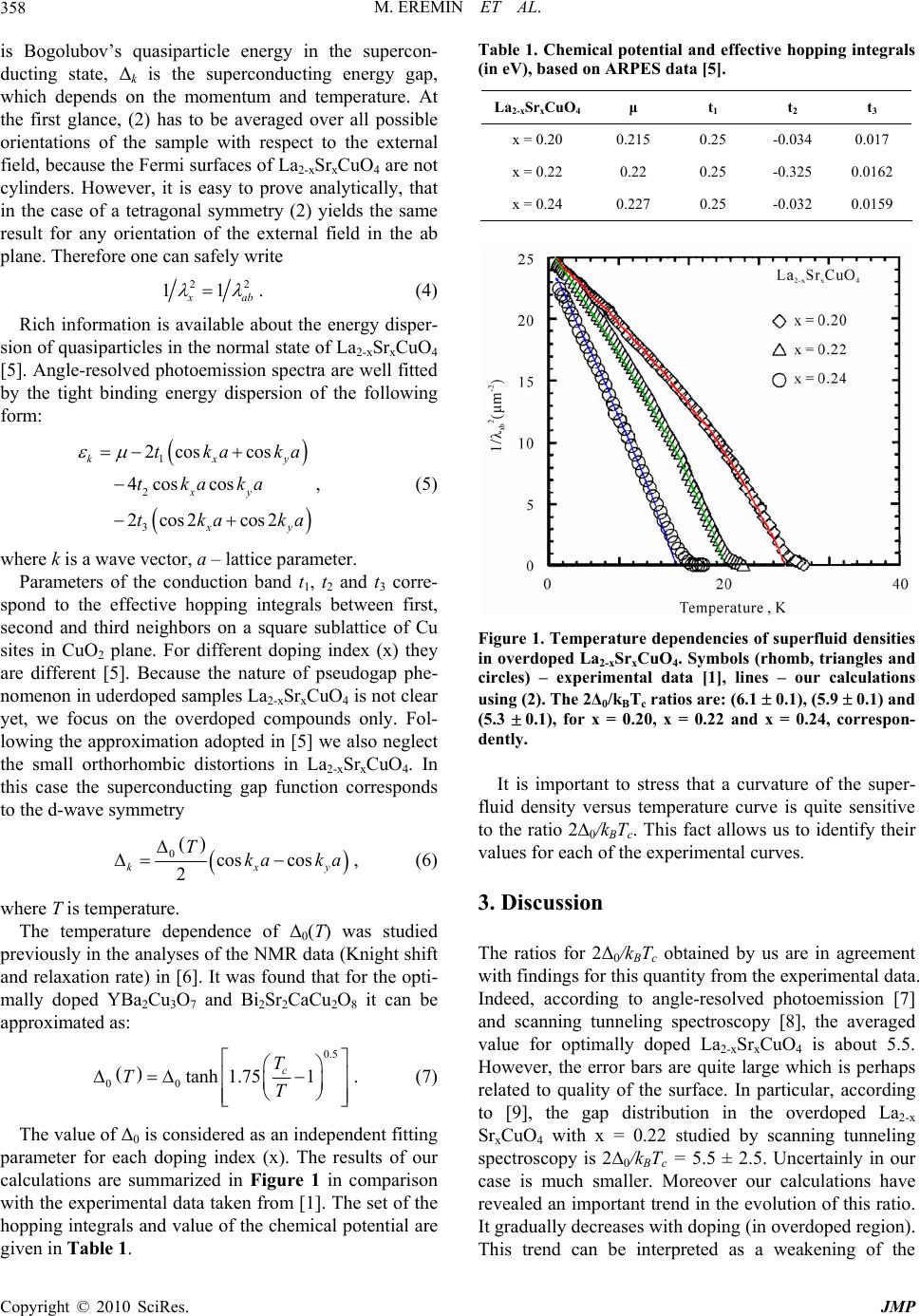

M. EREMIN ET AL. 358
is Bogolubov’s quasiparticle energy in the supercon-
ducting state, Δk is the superconducting energy gap,
which depends on the momentum and temperature. At
the first glance, (2) has to be averaged over all possible
orientations of the sample with respect to the external
field, because the Fermi surfaces of La2-xSrxCuO4 are not
cylinders. However, it is easy to prove analytically, that
in the case of a tetragonal symmetry (2) yields the same
result for any orientation of the external field in the ab
plane. Therefore one can safely write
2
11
2
x
ab

 . (4)
Rich information is available about the energy disper-
sion of quasiparticles in the normal state of La2-xSrxCuO4
[5]. Angle-resolved photoemission spectra are well fitted
by the tight binding energy dispersion of the following
form:


1
2
3
2cos cos
4coscos
2 cos2cos2
kx
xy
xy
tkaka
tkaka
tkak
  


y
a
, (5)
where k is a wave vector, a – lattice parameter.
Parameters of the conduction band t1, t2 and t3 corre-
spond to the effective hopping integrals between first,
second and third neighbors on a square sublattice of Cu
sites in CuO2 plane. For different doping index (x) they
are different [5]. Because the nature of pseudogap phe-
nomenon in uderdoped samples La2-xSrxCuO4 is not clear
yet, we focus on the overdoped compounds only. Fol-
lowing the approximation adopted in [5] we also neglect
the small orthorhombic distortions in La2-xSrxCuO4. In
this case the superconducting gap function corresponds
to the d-wave symmetry


0cos cos
2
kx
Tka ka 
 y , (6)
where T is temperature.
The temperature dependence of Δ0(T) was studied
previously in the analyses of the NMR data (Knight shift
and relaxation rate) in [6]. It was found that for the opti-
mally doped YBa2Cu3O7 and Bi2Sr2CaCu2O8 it can be
approximated as:

0.5
00
tanh 1.751
c
T
TT


 





. (7)
The value of Δ0 is considered as an independent fitting
parameter for each doping index (x). The results of our
calculations are summarized in Figure 1 in comparison
with the experimental data taken from [1]. The set of the
hopping integrals and value of the chemical potential are
given in Table 1.
Table 1. Chemical potential and effective hopping integrals
(in eV), based on ARPES data [5].
La2-xSrxCuO4μ t
1 t 2 t 3
x = 0.20 0.215 0.25 -0.034 0.017
x = 0.22 0.22 0.25 -0.325 0.0162
x = 0.24 0.227 0.25 -0.032 0.0159
Figure 1. Temperature dependencies of superfluid densities
in overdoped La2-xSrxCuO4. Symbols (rhomb, triangles and
circles) – experimental data [1], lines – our calculations
using (2). The 2Δ0/kBTc ratios are: (6.1 0.1), (5.9 0.1) and
(5.3 0.1), for x = 0.20, x = 0.22 and x = 0.24, correspon-
dently.
It is important to stress that a curvature of the super-
fluid density versus temperature curve is quite sensitive
to the ratio 2∆0/kBTc. This fact allows us to identify their
values for each of the experimental curves.
3. Discussion
The ratios for 2Δ0/kBTc obtained by us are in agreement
with findings for this quantity from the experimental data.
Indeed, according to angle-resolved photoemission [7]
and scanning tunneling spectroscopy [8], the averaged
value for optimally doped La2-xSrxCuO4 is about 5.5.
However, the error bars are quite large which is perhaps
related to quality of the surface. In particular, according
to [9], the gap distribution in the overdoped La2-x
SrxCuO4 with x = 0.22 studied by scanning tunneling
spectroscopy is 2∆0/kBTc = 5.5 ± 2.5. Uncertainly in our
case is much smaller. Moreover our calculations have
revealed an important trend in the evolution of this ratio.
It gradually decreases with doping (in overdoped region).
This trend can be interpreted as a weakening of the

M. EREMIN ET AL. 359
5. Acknowledgments
strong correlation effect in overdoped side of phase dia-
gram for La2-xSrxCuO4. It is interesting to compare the
ratio 2Δ0/kBTc extracted by us with those obtained from a
solution of the BCS equation
We thank Josef Roos, and Ilya Eremin for discussions.
This work was supported in part by the Russian Founda-
tion for Basic Research, Grant # 09-02-00777-a and
Swiss National Science Foundation, Grant IZ73Z0
128242.

'
'
1'tanh
22
k
kkkB
E
Jk k
NE

 
 

'k
kT

. (8)
Here J(q) = 2J(cos(qxa)+cos(qya)) is a Fourier trans-
form of the short-range pairing potential, which included
all possible interactions between nearest neighbors in
CuO2 plane (for example superexchange, screened Cou-
lomb repulsion and phonon mediated interaction). The
solutions of (8) are presented in Table 2. Note that the
momentum and temperature dependencies fairly well
correspond to (6-7).
6. References
[1] B. D. Rainford, J. R. Cooper, W. Lo, J. L. Tallon, J. W.
Loram, J. Betouras, Y. S. Wang and C. W. Chu, “Effects
of Carrier Concentration on the Superfluid Density of
High-Tc cuprates”, Physical Review B, Vol. 60, No. 21,
1999, pp. 14617-14620.
[2] H. Won and K. Maki, “D-Wave Superconductor as a
Model of High-Tc Superconductors,” Physical Review B,
Vol. 49, No. 2, 1994, pp. 1397-1402.
The analysis of the temperature dependencies of the
superfluid density ns ~ 1/λab2 in YBa2Cu3O6+x and single
layer tetragonal compound Tl2Ba2CuO6+δ (Tc = 78 K),
performed in [4], showed that in the overdoped com-
pound Tl2Ba2CuO6+δ (Tc = 78 K) the ratio 2Δ0/kBTc de-
creases with respect to that of an optimally doped
YBa2Cu3O6+x (Tc = 92 K). In present paper we have
found the same trend for overdoped La2-xSrxCuO4. This
fact seems to reflect a quite general property of layered
cuprates.
[3] J. Hofer, K. Conder, T. Sasagawa, Guo-meng Zhao, M.
Willemin, H. Keller and K. Kishio, “Oxygen-Isotope Ef-
fect on the In-Plane Penetration Depth in Underdoped
La2-xSrxCuO4 Single Crystals”, Physical Review Letters,
Vol. 84, No. 18, 2000, pp. 4192-4195.
[4] M. V. Eremin, I. A. Larionov and I. E. Lyubin, “London
Penetration Depth in the Tight Binding Approximation:
Orthorhombic Distortion and Oxygen Isotope Effects in
Cuprates”, Journal of Physics: Condensed Matter, Vol.
22, No. 18, 2010, pp. 185704-185709.
This conclusion is also supported by the fact that the
temperature dependence of the superconducting energy-
gap (7) in overdoped La2-xSrxCuO4 is described fairly
well by (8).
[5] T. Yoshida, X. J. Zhou, K. Tanaka, W. L. Yang, Z. Hus-
sain, Z.-X. Shen, A. Fujimori, S. Sahrakorpi, M. Lindroos,
R. S. Markiewicz, A. Bansil, Seiki Komiya, Yoichi Ando,
H. Eisaki, T. Kakeshita, and S. Uchida, “Systematic
Doping Evolution of the Underlying Fermi Surface of
La2-xSrxCuO4”, Physical Review B, Vol. 74, No. 22, 2006,
pp. 224510-1–224510-5.
4. Conclusion
[6] T. Mayer, M. Eremin, I. Eremin and P. F. Meier, “Spin
Dynamics of Itinerant Holes in HTSC Cuprates: the
Singlet-Correlated Band Model and Its Applications”,
Journal of Physics: Condensed Matter, Vol. 19, No.11,
2007, 116209-116227.
In summary, we have analyzed the temperature depend-
ence of the superfluid density in overdoped La2-xSrxCuO4.
Our calculations have revealed important trend of
changes the ratio 2Δ0/kBTc. It gradually decreases with
doping in overdoped region. The temperature depend-
ence of the energy gap is fairly well described by simple
BCS equation with short range pairing potential yielding
a d-wave symmetry of the superconducting gap.
[7] A. Damascelli, Z. Hussain, Z.-X. Shen, “Angle-Resolved
Photoemission Studies of the Cuprate Superconductors”.
Reviews of Modern Physics, Vol. 75, No. 2, 2003, pp.
473-541.
[8] O. Fischer, M. Kugler, I. Maggio-Aprile, C. Berthod and
C. Renner, “Scanning Tunneling Spectroscopy of High-
Temperature Superconductors”, Reviews of Modern
Physics, Vol. 79, No. 1, 2007, pp. 353-419.
Table 2. Solutions of Equation (8) for short range interac-
tion.
La2-xSrxCuO4 T
c, K J, meV 2Δ0/kBTc
x = 0.20 34 85 4.02 0.01
x = 0.22 25 91 4.21 0.01
x = 0.24 18 93.7 4.36 0.01
[9] T. Kato, T. Noguchi, R. Saito, T. Machida and H. Sakata.
“Gap Distribution in overdoped La2-xSrxCuO4 Observed
by Scanning Tunneling Spectroscopy”. Physica C Vol.
460-462, 2007, pp. 880-881.