Open Journal of Applied Sciences, 2013, 3, 102-105
doi:10.4236/ojapps.2013.31B1021 Published Online April 2013 (http://www.scirp.org/journal/ojapps)
Nonsingular Positon Solutions of a Variable-Coefficient
Modified KdV Equation
Yi Lin, Chuanzhong Li, Jingsong He
Department of Mathematics, Ningbo University, Ningbo, China
Received 2013
ABSTRACT
The determinant representation of three-fold Darboux transformation for a variable-coefficient modified KdV equation
is displayed based on the technique used to solve Ablowitz-Kaup-Newell-Segur system. Additionally, the nonsingular
positon solutions of the variable-coefficient modified KdV equation are firstly discovered analytically and graphically.
Keywords: Variable-Coefficient KdV Equation; Lax Pair; Darboux Transformation; Positon; Soliton-Positon
1. Introduction
The differential nonlinear evolution equations (NLEEs)
have been researched extensively in the past decades.
Recently, investigators are shifting their focus in the
NLEEs with variable coefficients as in [1]. It is found
that NLEEs with variable coefficients can provide more
powerful and realistic models than the ones with constant
coefficients in describing more complex and real phe-
nomenon. It is shown that solitons of NLEEs with vari-
able coefficients can be effectively controlled through
changing their variable parameters.
In this paper, we will concentrate on a variable-coef-
ficient modified KdV (vc-mKdV) equation
2
()()()() 0,
t xxxxx
ututuutu tu     (1)
where ,, ,

t 

t 

t 


t

are all time-dependent
analytic functions.
In fact, much attention has been paid to research on
different forms of vc-mKdV equations. Particularly, as
long ago as 1996, K. Porsezian investigated the N-soliton
solution of a vc-mKdV equation is derived through the
Hirota method in [2]. And the double-Wronskian-typed
soliton of the vc-mKdV Equation (1) are constructed
recently in [3].
Besides the soliton solution of NLEEs, another kind of
solution called positon, is researched in lots of papers as
well, such as [4-6]. As we know, positon relates very
closely to soliton, i.e. the interdependence among spec-
tral parameters of soliton gives rise to positon, so we can
also call positon “degenerate soliton” [7].
This letter is organized as follows. In Section 2, we
construct the detailed Darboux Transformation and its
determinant representation of Equation (1). Furthermore,
the nonsingular positon and soliton-positon solutions of
Equation (1) are derived firstly in Section 3, a series of
pictures are also displayed for the better understanding.
Section 4 is devoted to the conclusions and discussions.
2. Darboux Transformation of the vc-mKdV
Equation
2.1. Lax pair of the vc-mKdV Equation
It’s known that the Lax pair plays a vital role in studying
the integrable properties of NLEEs such as the Hamilto-
nian structures, conservation laws, symmetry and Dar-
boux Transformations show in [7-10]. In the following
research, we will use the lax pair presented in [3], which
is constructed with the help of the Ablowitz-Kaup-New-
ell-Segur (AKNS) approach under the following constraint
2()
2
0
() 6()tdt tCte



, (2)
where 00C

is an arbitrary constant. We will choose
01C

in this paper for simplicity.
Then the linear eigenvalue problems for Equation (1)
can be expressed as
, .
xt
M
N


(3)
where

12
,T

f
f , T denotes the transpose of the ma-
trix, and M and N are shown as follows:
()
() , ,
tdt
tdt
eu AB
MN
CA
ev








 








(4)
with
2() 3
2()
() 2
2() 2
() 2
2() 2
4()[() 2()]
()
[4()2( )( )
2()()]
[4()2 ()()
2()()
tdt
tdt
xx
tdt
x
xx
tdt
tdt
x
xx
tdt
Atte tuv
euvvu
B
etutu
etuvtu
C etvtvtv
etuvt






 

 

 







 

 

 
]v
tu

Y. LIN ET AL. 103
The spectral parameter

is independent of x and t.
With constraint (2), it is easy to prove that the compati-
bility condition (also called the zero curvature equation)
of Equations (3) and (4).


,
tx
MN MN 0
(5)
gives rise to Equation (1) for by direct compu-
tation. The bracket represents the usual matrix commu-
tator, and the Lax pair (3) can guarantee the complete
integrability of Equation (1).
uv
2.2. Three-fold Darboux Transformation for the
vc-mKdV Equation
First of all, we need to introduce the eigenfunctions
which stasify the Lax pair (3)

1
2
k
kk
k
f
f







(6)
of all the eigenvalues k

, we let km 1, 2,, 6,k



if and k km

 . Additionally, the eigenfunctions
are linearly independent i.e. k

and m  are linearly
independent if . According to the knowledge of
DT, with the help of Cramer's rule and iterative computa-
tions, the explicit new solutions and of the
vc-mKdV Equation (1) can be derived as
km
[3]
u[3 v]
[3]
66
[3]
66
2,
2.
uuUW
vvVW




(7)
where
23
11121 111 12111111
23
21222 212 22221221
23
3132 331 332 331331
623
41424 414 42441441
23
51525 515 52551551
23
61626 616 62661661
,
f
fff ff
f
fffff
f
fff ff
U
f
fffff
f
fff ff
f
fff ff







23
11121 111 12112112
23
2122 221222222 222
23
31323 313 32332332
623
4142 441442442 442
23
51525 515 52552552
23
6162 661662 662 662
.
f
ffff f
f
fff ff
f
fff ff
V
f
fff ff
f
fff ff
f
fff ff
 
 
 
 
 
 

Next, it's very easy to turn out that if ,
,
k+1 1k 2
f = - f
_k+1 2k 1
f = f1kk


 , then

1, 3, 5k[3][3] uv



2
u

2
v
will be realized, so the three-fold DT of the vc-mKdV
equation (1) is accomplished completely. Additionally,
we can get the analogous expressions of and
when , so the two-soliton can be arrived as
well.
k=1,2,3,4
3. Positon and Soliton-positon Solutions of
the vc-mKdV Equation
Matveev expounded “positon” in [4] for the KdV equa-
tions. As we know, most of the known positon solutions
are singular. For various important integrable systems
such as the KdV equations and the mKdV equations,
there is no nonsingular positon found, though [6] gives
the nonsingular positon for the coupled KdV system. In
this section, the positon solutions of the vc-mKdV Equa-
tion (1) will be displayed for the first time using the re-
sult obtained in section 2. It's happy that they are non-
singular.
Choosing the zero seed solution of Equation (1),
Equation (3) is solved by

1
2
,
k
kk
k
f
f





(8a)
3
( 4()())
1,
kk k
ttdtx
k
fe
   

 (8b)
3
(4()())
2.
kk k
ttdtx
k
fe
  

 (8c)
with


1, 2,, 6
kk
  are arbitrary spectral Parameters.
In the construction of two-soliton solutions, if the
second spectral parameter 3

is colse to the first spec-
tral parameter 1

, then the one-positon solution of vc-
mKdV Equation (1) can be generated by doing the Tay-
lor expansion of the wave function (8b) to the first order
up to 1

. That is to say, firstly, taking 31



 in
the used eigenvalue of the two-fold DT. Secondly, using
the Taylor expansion of 3
f
and 4
f
up to the first order
of in terms of 1 

. Finally, substituting these ma-
nipulations into the two-fold DT, we get the one-positon
solution in the form of
()
1111 11
[8 (2sinh( )2sinh( )
tdt
pos
ueh PxP

 


22
111
cosh())] /(18()cosh(2))
1
P
hx P  
(9)
with


2
11
12 ()(),htt    
dt
k
3
2(4()())2, 1,3
kkkk
Ptttxd   

From Equation (9), we can know that ()t

will
change the amplitude of the positon solution, just as the
plot (a) in Figure 1 vividly shown. What's more, there is
no zero point in the denominator of Equation (9), that
means this one-positon solution is nonsingular. Positons
of KdV are defined as long-range analogues of solitons
and are slowly decreasing, oscillating solutions in [5].
From Figure 1, it is clear that two peaks in one-positon
separate according to a different way in the two soliton.
Using the same method in the process of the positon
above, fixing 5

, and calculating the limit 31

 , we
obtain the soliton-positon solution of vc-mKdV Equation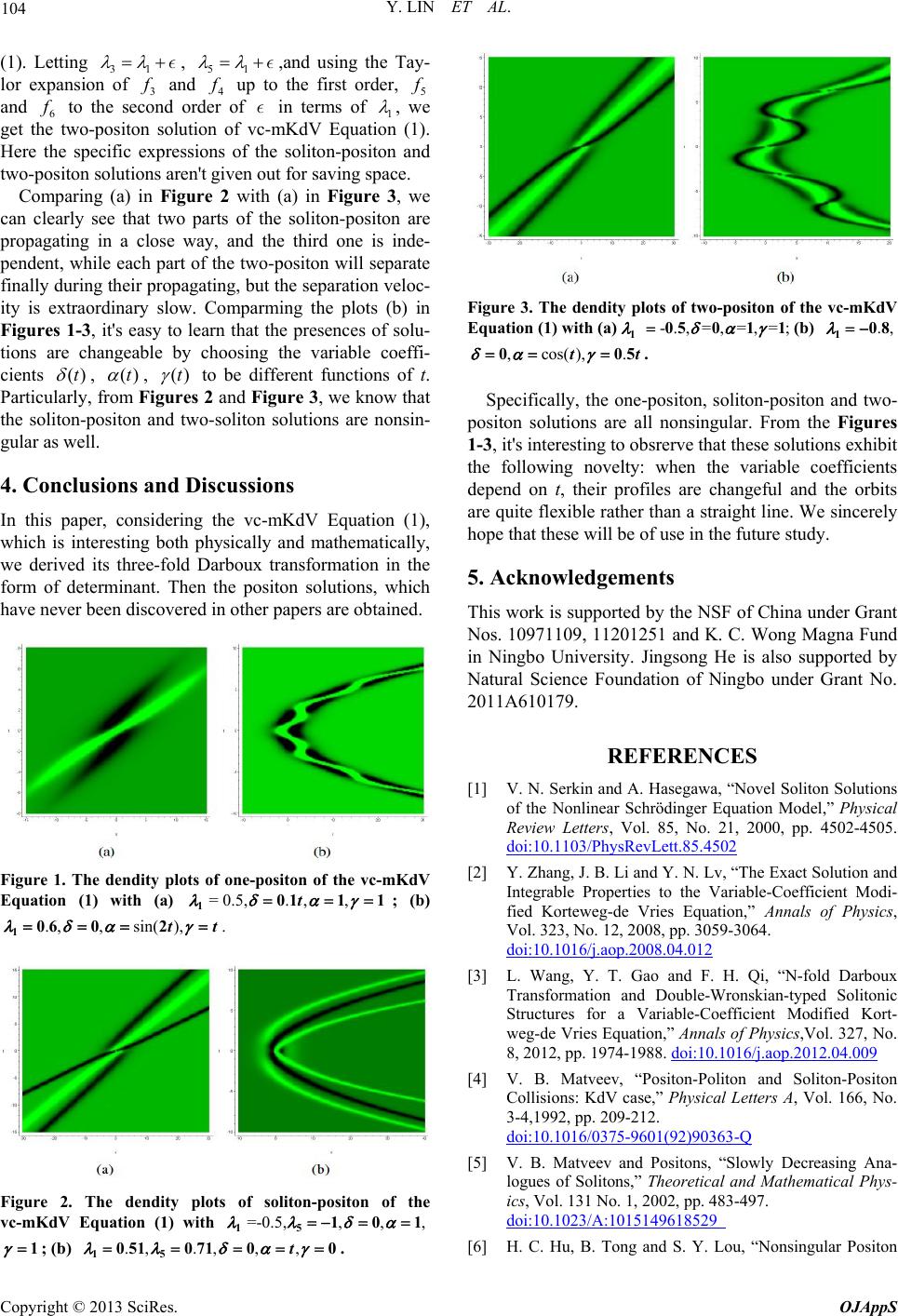

Y. LIN ET AL.
104
(1). Letting 31

 , 51

 ,and using the Tay-
lor expansion of 3
f
and 4
f
up to the first order, 5
f
and 6
f
to the second order of in terms of 1 

, we
get the two-positon solution of vc-mKdV Equation (1).
Here the specific expressions of the soliton-positon and
two-positon solutions aren't given out for saving space.
Comparing (a) in Figure 2 with (a) in Figure 3, we
can clearly see that two parts of the soliton-positon are
propagating in a close way, and the third one is inde-
pendent, while each part of the two-positon will separate
finally during their propagating, but the separation veloc-
ity is extraordinary slow. Comparming the plots (b) in
Figures 1-3, it's easy to learn that the presences of solu-
tions are changeable by choosing the variable coeffi-
cients ()t

, ()t

, ()t

to be different functions of t.
Particularly, from Figures 2 and Figure 3, we know that
the soliton-positon and two-soliton solutions are nonsin-
gular as well.
4. Conclusions and Discussions
In this paper, considering the vc-mKdV Equation (1),
which is interesting both physically and mathematically,
we derived its three-fold Darboux transformation in the
form of determinant. Then the positon solutions, which
have never been discovered in other papers are obtained.
Figure 1. The dendity plots of one-positon of the vc-mKdV
Equation (1) with (a) = 0.5,
1. ,,011t1




; (b)
.,06 ,sin( ),0 2
1tt

    .
Figure 2. The dendity plots of soliton-positon of the
vc-mKdV Equation (1) with =-0.5
1, 5
  ,1,,10 
1

; (b) ., .,
15
051071,,0 0t

  .
Figure 3. The dendity plots of two-positon of the vc-mKdV
Equation (1) with (a) -.,=,=,=;
10501 1



(b) .,
108



,cos(), .005tt



.
Specifically, the one-positon, soliton-positon and two-
positon solutions are all nonsingular. From the Figures
1-3, it's interesting to obsrerve that these solutions exhibit
the following novelty: when the variable coefficients
depend on t, their profiles are changeful and the orbits
are quite flexible rather than a straight line. We sincerely
hope that these will be of use in the future study.
5. Acknowledgements
This work is supported by the NSF of China under Grant
Nos. 10971109, 11201251 and K. C. Wong Magna Fund
in Ningbo University. Jingsong He is also supported by
Natural Science Foundation of Ningbo under Grant No.
2011A610179.
REFERENCES
[1] V. N. Serkin and A. Hasegawa, “Novel Soliton Solutions
of the Nonlinear Schrödinger Equation Model,” Physical
Review Letters, Vol. 85, No. 21, 2000, pp. 4502-4505.
doi:10.1103/PhysRevLett.85.4502
[2] Y. Zhang, J. B. Li and Y. N. Lv, “The Exact Solution and
Integrable Properties to the Variable-Coefficient Modi-
fied Korteweg-de Vries Equation,” Annals of Physics,
Vol. 323, No. 12, 2008, pp. 3059-3064.
doi:10.1016/j.aop.2008.04.012
[3] L. Wang, Y. T. Gao and F. H. Qi, “N-fold Darboux
Transformation and Double-Wronskian-typed Solitonic
Structures for a Variable-Coefficient Modified Kort-
weg-de Vries Equation,” Annals of Physics,Vol. 327, No.
8, 2012, pp. 1974-1988. doi:10.1016/j.aop.2012.04.009
[4] V. B. Matveev, “Positon-Politon and Soliton-Positon
Collisions: KdV case,” Physical Letters A, Vol. 166, No.
3-4,1992, pp. 209-212.
doi:10.1016/0375-9601(92)90363-Q
[5] V. B. Matveev and Positons, “Slowly Decreasing Ana-
logues of Solitons,” Theoretical and Mathematical Phys-
ics, Vol. 131 No. 1, 2002, pp. 483-497.
doi:10.1023/A:1015149618529
[6] H. C. Hu, B. Tong and S. Y. Lou, “Nonsingular Positon

Y. LIN ET AL.
105
and Complexi-Ton Solutions for the Coupled KdV Sys-
tem,” Physics Letters A, Vol. 351, No. 6, 2006, pp.
403-412. doi:10.1016/j.physleta.2005.11.047
[7] J. S. He, H. R. Zhang, L. H. Wang, K. Porsezian and A. S.
Fokas, “A Generating Mechanism for Higher Order
Rogue Waves,” 2013.
[8] J. S. He, L. Zhang, Y. Cheng and Y. S. Li, “Determinant
Representation of Darboux Transformation for the AKNS
System,” Science in China Series A: Mathematics, Vol.
49, No. 12, 2006, pp. 1867-1878.
doi:10.1007/s11425-006-2025-1
[9] K. Porsezian and K. Nakkeeran, “Optical Solitons in
Presence of Kerr Dispersion and Self-Frequency Shift,”
Physical Review Letters, Vol. 76, No. 21, 1996, pp.
3955-3958. doi:10.1103/PhysRevLett.76.3955
[10] M. J. Ablowitz, D. J. Kaup, A .C. Newell, H. Seger,
“Nonlinear-Evolution Equations of Physical Signifi-
cance,” Physical Review Letters, Vol. 31, No. 2, 1973, pp.
125-127. doi:10.1103/PhysRevLett.31.125
[11] H. H. Chen and C. S. Liu, “Soliton in Nonuniform
Media,” Physical Review Letters, Vol. 37, No.11,
1976, pp. 693-697. doi:10.1103/PhysRevLett.37.693