Open Journal of Applied Sciences, 2013, 3, 99-101
doi:10.4236/ojapps.2013.31B1020 Published Online April 2013 (http://www.scirp.org/journal/ojapps)
Rogue Waves of the Kundu-DNLS Equation
Shibao Shan, Chuanzhong Li, Jingsong He
Department of Mathematics, Ningbo University, Ningbo, China
Received 2013
ABSTRACT
In this paper, we give the Lax pair and construct the Darboux transformation of the Kundu-DNLS equation. Further-
more, the rogue wave solutions of the Kundu-DNLS equation are derived by using the Taylor expansion of the breather
solution. What's more, the triangular and the circular patterns of the third rouge solution are displayed.
Keywords: Kundu-DNLS Equation; Darboux Transformation; Rogue Waves
1. Introduction
As one of the most important integrable systems in many
branches of physics and applied mathematics, the deriva-
tive nonlinear Schr\"{o}dinger (DNLS) equation


0
2 x
xxt qqiqiq

(1)
has been studied in optics, water wave and so on [1,2].
Considering the significance of the higher order nonlin-
earities in physical system, the DNLS equation yields an
integrable higher nonlinear equation, i.e. Kundu-DNLS
equation [3,4]
0)2(
)()(
*2
2*2


QQiQ
QiQQiQiQ
xx
xxxtxxxt


(2)
by means of a nonlinear transformation of the field
with arbitrary gauge function
 i
qeQq  

.
For example, setting
 xdxQ  

2

, Kundu-DNLS
equation implies Eckhaus-Kundu (EK) equation.
Here denotes the complex conjugate of Q, and α s
a real parameter.
*
Q
Rogue waves are one of those fascinating destructive
phenomena in nature that have not been fully explained
so far. Understanding the initial conditions that foster
rogue waves could be useful both in attempts to avoid
them by seafarers and in generating highly energetic
pulses in optical fibers. There are several method to
solve the integrable equations, for instance, Hirota
method [5], inverse scattering transformation [6], bilin-
ear method [7], Darboux transformation [8]. In this paper,
we have given the rogue waves of the DNLS and the
coupled system of Hirota and Maxwell-Bloch equations
[2,9,10]. Inspired by the importance of these recent in-
teresting developments about the analysis of rogue waves
of the NLS-type equations, we shall construct the rogue
wave solutions of the Kundu-DNLS equation with the
help of the Darboux transformation.
2. Darboux Transformation and Lax Pair
The Darboux transformation is a powerful method used
to generate the soliton solutions for integrable equations.
Inspired by classical Darboux transformation for the DNLS
equation, we consider the coupled Kundu-DNLS equa-
tion,
0)2(
)()(
2
22


RQiQ
QiRQiQiQ
xx
xxxtxxxt


(3)
0)2(
)()(
2
22


QRiR
RiQRiRiR
xx
xxxtxxxt


(4)
where

is a arbitrary gauge function. This form of the
equation is very extensive, which is reduced to the equa-
tion (2) for with the sign of the nonlinear term
changed. The Kundu-DNLS equation can be obtained if
α is a real parameter.
*
QR 
We first present a general framework for deriving the
required conservation rule for the DNLS equation. We
start with the linear set of Lax equations:
,, 




VU tx (5)
where U and V depend on the complex constant eigen-
value parameter λ.














































0
0
10
01
48
,
0
Re0
2
10
01
4
*
2
4
2
G
G
iQRiV
Qe
i
iU i
i






,
with

 .2)(2
4
*2 


i
x
ii
x
ieQQiQeeQiQeG 

S. B. SHAN ET AL.
100
where λ is the eigenvalue, Φ is the eigenfunction corre-
sponding to λ.
In general, considering the universality of Darboux
transformation, according to the Kundu-DNLS equation
(3) and (4) , we can start from
,
00
00
11
11 2
22
22

























dc
ba
dc
ba
dc
ba
T 
(6)
where are functions
of
222211110000,,,,,,,,,,, dcbadcbadcba
.t ,
x
From

,
1TUTUTx (7)
and

,
1TVTVTt

(8)
then the now solutions




11,RQ are given by


.
,
2
21
1211
2
21
2
11
1
2
11
2221
2
11
2
21
1

















i
i
e
QR
e
QQ
(9)
Here
.,
,,
22
2
2
11
2
1
22
222
111
21
22
2
2
11
2
1
12
222
111
11










So far, we discussed about the determinant construc-
tion of one-fold Darboux transformation of Kundu-
DNLS equation. As an application of these transforma-
tions of Kundu-DNLS equation, rogue wave solutions
will be constructed in the next section.
3. Rogue Wave Solutions
In this section, we construct the rogue wave solution of
Kundu-DNLS equation . This kind of solution only ap-
pears in some special region of time and space and then
drown into a fixed non-vanishing plane. By making use
of the Taylor expansion for the breather solution, one
order rogue wave solution of for the Kundu-DNLS
equation is obtained
 1
r
Q

,
2
)2(
1
1
v
ev
Q
txi
r


 (10)
,8812484
4888883
2334
422222
1
tititixix
x
txtxtixtitxxv




.44488
888481
44322
222
2
ix
x
tix
x
t
txxititxititv


The picture of one order rogue wave solution of the
Kundu-DNLS equation and its corresponding density
graph are plotted in Figure 1.
When we take ,1,1,1,2 





ca the picture of
second rogue wave solution for the Kundu-DNLS equa-
tion is displayed in Figure 2.
Next, we examine third-order rogue waves. In this
case, form the figures, We can get third-order rogue
wave solution with the help of
,1,1,2



ca the
picture of third-order rogue wave solution is displayed in
Figure 3.
Figure 1. The One order rogue wave solution of the Kundu-
DNLS equation with a = –2, c = 1, ξ = 1, η = 1.
Figure 2. The second order rogue wave solution of the Kundu-
DNLS equation with a = –2, c = 1, ξ = 1, η = 0.8.
Figure 3. The third order rogue wave solution |Qr3|2 f the
Kundu-DNLS equation with a = –2, c = 1, ξ = 0.8, η = 0.8.

S. B. SHAN ET AL.
101
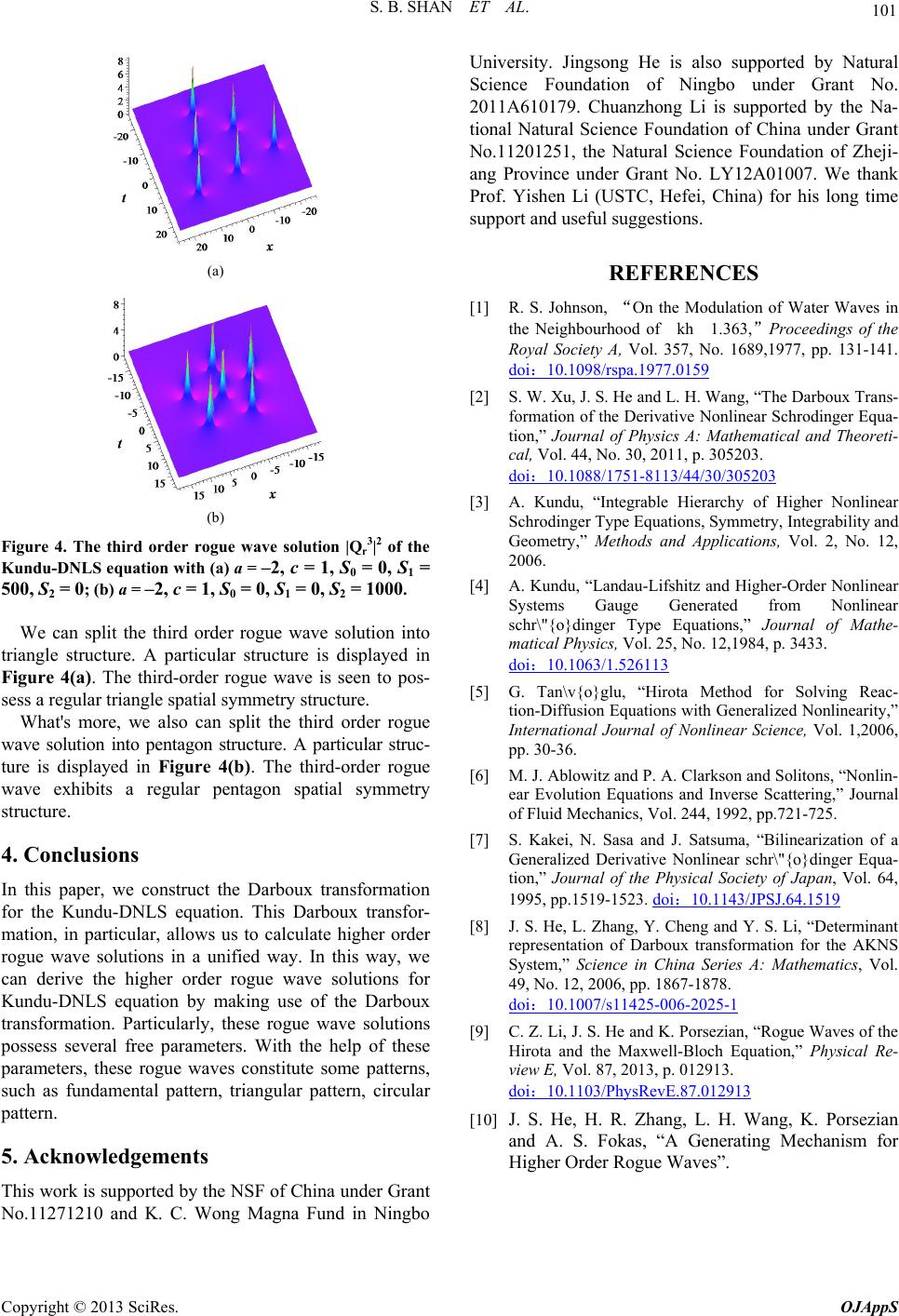
(a)
(b)
Figure 4. The third order rogue wave solution |Qr3|2 of the
Kundu-DNLS equation w ith (a) a = –2, c = 1, S0 = 0, S1 =
500, S2 = 0; (b) a = –2, c = 1, S0 = 0, S1 = 0, S2 = 1000.
We can split the third order rogue wave solution into
triangle structure. A particular structure is displayed in
Figure 4(a). The third-order rogue wave is seen to pos-
sess a regular triangle spatial symmetry structure.
What's more, we also can split the third order rogue
wave solution into pentagon structure. A particular struc-
ture is displayed in Figure 4(b). The third-order rogue
wave exhibits a regular pentagon spatial symmetry
structure.
4. Conclusions
In this paper, we construct the Darboux transformation
for the Kundu-DNLS equation. This Darboux transfor-
mation, in particular, allows us to calculate higher order
rogue wave solutions in a unified way. In this way, we
can derive the higher order rogue wave solutions for
Kundu-DNLS equation by making use of the Darboux
transformation. Particularly, these rogue wave solutions
possess several free parameters. With the help of these
parameters, these rogue waves constitute some patterns,
such as fundamental pattern, triangular pattern, circular
pattern.
5. Acknowledgements
This work is supported by the NSF of China under Grant
No.11271210 and K. C. Wong Magna Fund in Ningbo
University. Jingsong He is also supported by Natural
Science Foundation of Ningbo under Grant No.
2011A610179. Chuanzhong Li is supported by the Na-
tional Natural Science Foundation of China under Grant
No.11201251, the Natural Science Foundation of Zheji-
ang Province under Grant No. LY12A01007. We thank
Prof. Yishen Li (USTC, Hefei, China) for his long time
support and useful suggestions.
REFERENCES
[1] R. S. Johnson, “On the Modulation of Water Waves in
the Neighbourhood of kh 1.363,”Proceedings of the
Royal Society A, Vol. 357, No. 1689,1977, pp. 131-141.
doi：10.1098/rspa.1977.0159
[2] S. W. Xu, J. S. He and L. H. Wang, “The Darboux Trans-
formation of the Derivative Nonlinear Schrodinger Equa-
tion,” Journal of Physics A: Mathematical and Theoreti-
cal, Vol. 44, No. 30, 2011, p. 305203.
doi：10.1088/1751-8113/44/30/305203
[3] A. Kundu, “Integrable Hierarchy of Higher Nonlinear
Schrodinger Type Equations, Symmetry, Integrability and
Geometry,” Methods and Applications, Vol. 2, No. 12,
2006.
[4] A. Kundu, “Landau-Lifshitz and Higher-Order Nonlinear
Systems Gauge Generated from Nonlinear
schr\"{o}dinger Type Equations,” Journal of Mathe-
matical Physics, Vol. 25, No. 12,1984, p. 3433.
doi：10.1063/1.526113
[5] G. Tan\v{o}glu, “Hirota Method for Solving Reac-
tion-Diffusion Equations with Generalized Nonlinearity,”
International Journal of Nonlinear Science, Vol. 1,2006,
pp. 30-36.
[6] M. J. Ablowitz and P. A. Clarkson and Solitons, “Nonlin-
ear Evolution Equations and Inverse Scattering,” Journal
of Fluid Mechanics, Vol. 244, 1992, pp.721-725.
[7] S. Kakei, N. Sasa and J. Satsuma, “Bilinearization of a
Generalized Derivative Nonlinear schr\"{o}dinger Equa-
tion,” Journal of the Physical Society of Japan, Vol. 64,
1995, pp.1519-1523. doi：10.1143/JPSJ.64.1519
[8] J. S. He, L. Zhang, Y. Cheng and Y. S. Li, “Determinant
representation of Darboux transformation for the AKNS
System,” Science in China Series A: Mathematics, Vol.
49, No. 12, 2006, pp. 1867-1878.
doi：10.1007/s11425-006-2025-1
[9] C. Z. Li, J. S. He and K. Porsezian, “Rogue Waves of the
Hirota and the Maxwell-Bloch Equation,” Physical Re-
view E, Vol. 87, 2013, p. 012913.
doi：10.1103/PhysRevE.87.012913
[10] J. S. He, H. R. Zhang, L. H. Wang, K. Porsezian
and A. S. Fokas, “A Generating Mechanism for
Higher Order Rogue Waves”.