Communications and Network, 2013, 5, 48-52
doi:10.4236/cn.2013.52B009 Published Online May 2013 (http://www.scirp.org/journal/cn)
A Set of Space-Time Block Codes for the High-Rate
Transmission Scheme with One Information Bit
Yier Yan1,3, Xueqin Jiang2,3, Moon Ho Lee3
1School of Mechanical and Electrical Engineering of Guangzhou University, Guangdong Guangzhou, China
2School of Information Science and Technology of DonghuaUniversity, Shanghai, PR China
3Electrical and Computer Engineering of Chonbuk National University, Repulic of Korea
Received 2013
ABSTRACT
In [1], the authors have proposed one high rate transmission scheme for Space-Time Block Codes (STBC) without ad-
ditional system source such as power, bandwidth and time slot. To maintain the full rank property of the coding gain
matrix, we propose a set of STBCs for 4 transmit antennas transmission to transmit one additional information bit
achieving rate-9/8. Another orthogonal STBC code with rate-1 is proposed in this paper within the set. It shows by
computer simulation results that by employing the set of STBCs, it achieves better bit error rate (BER) performance and
throughput th an that of [1] with a valid BER improvement at the high SNR region above 20dB.
Keywords: Antennas; Orthogonal; STBC; BER; High Rate
1. Introduction
Since transmit diversity for wireless communication has
been introd uced in [2,3]. Space-tim e bloc k cod ing (ST BC)
is an efficient transmit diversity scheme to combat detri-
mental effects of wireless fading channels. STBC from
orthogonal designs are attracting wider attention due to
their amenability for fast maximum likelihood (ML) de-
coding algorithm and full diversity [3,4]. STBCs are a set
of practical signal design techniques aimed at approach-
ing the information theoretic capacity for multiple-input
and multiple-output (MIMO) channels. Alamouti code is
an elegant and seminal STBC design for a two-transmit
antenna system [4]. It achieves full-rate, i.e. rate one, full
diversity transmission using two time slots for signals
with complex constellations, which are employed in most
current commercial wireless systems. The orthogonal code
design from Tarokh et al. is a generalization of Alamouti
code for systems with an arbitrary number of transmit
antennas [2,3]. It has been proved however, the orthogonal
design for complex signals with linear decoding com-
plexity achieving full-rate full-diversity transmission is
not available for the number of antennas more than two
[3]. The system with higher number of antennas has to
either suffer from rate loss or put up with more decoding
complexity.
The class of linear STBC is the major category of
space-time codes and can be divided into subclasses like
Linear Dispersion Codes [5], Orthogonal STBC (OSTBC)
[2-4] and Quasi Orthogonal STBC (QOSTBC) [6] which
is typical designed for more than two antenna systems
with increased, but not exponentially, d ecoding complex-
ity [4-6].
A class of STBCs [1,7] is proposed for a high rate>1
transmission scheme by exploiting the inherent algebraic
structure for 2 and 4 transmit antennas. In order to main-
tain the full rank property of coding gain matrix for two
STBC codewords selected from two STBCs, respectively,
one of two STBCs should be scaled by weighted factor
and rotated by an angle. To compare the high rate trans-
mission scheme with the rate-1 case such as Alamouti
and Jafarkhani schemes, the system performance is at-
tenuated due to rotating or scaling one transmit matrix
selected from the class. In order to compensate the loss
of system performance, we propose another orthogonal
STBC matrix with rate-1 as one candidate in the set to
maintain the full rank property of coding gain matrix for
4 transmit antennas case while two codewords are se-
lected from two candidate matrices, respectively. Simu-
lation results show that the BER performance is similar
with Jafarkhani code in the high SNR region (20-25dB)
due to full rank property of coding gain matrix.
2. System Model
Consider a MIMO system with N transmitting and M
receiving antennas with transmit vector 1
[, ,]
N
x
xx
and flat block-fading channel [4] can be expressed as

YXHN (1)

Y. YAN ET AL. 49
is 1(1 )
j
x
e

 is 2(1 ).
j
x
e


gain matrix bet
where (2)
[(1),,()]
[(1),,()]
[(1),,()]
TT
TT
TT
T
T
T



Yy y
Xx x
Nn n



T
T
T
are the matrices of the received signals, transmitted sig-
nals, and noise, respectively, is the com-
plex channel matrix which is composed of i.i.d complex
Gaussian entries, and is a vector of size M repre-
senting the additive n oise, T is the block length. Here,
HNM
()tn
1
( )[( ),,( )],
M
tyt yty
1
( )[( ),,( )],
N
txt xtx
1
( )[( ),,( )],
M
tnt ntn
are the complex row vectors of the received signal, trans-
mitted signal, and noise, respectively.
3. Original Works
The author proposed one transmission scheme to transmit
one additional information bit without additional source.
The transmission scheme employs two STBC matrices
1 and 2
 to represent the information of bit b for 0 or
1, respectively

1234
21 43
11234 3412
4321
(, ,,,0),
x
xxx
x
xxx
xxxxb
x
xxx
x
xxx
 


















(4
where each row represents an antenna index and e
(3)
and
12 34
21 43
21234
3412
4321
(, ,, ,1),
jj
jj
jj
jj
xe xexx
xe xexx
xxxxb xxxexe
xxxexe




 













)
ach
column represents either a time instance for STBC, and
1234
,,,
x
xxx are selected from an alphabet (QPSK
Superscript  denotes complex conjugate.
The system performance is mainly dependent on the
coding gain matrix between 1
 and 2 . The coding
gain defined in [2]
Modulation).
1212
()()
H
CG CCCC (5)
must be a full rank matrix with the determinant as large
as possible, where 1
C and 2 C are STBC codewords [1]
corresponding to theTBC mrix. It is easy to check the
coding gain matrix for 1
 and 2.
ˆ
Sat
12
21
12 12
21
ˆ00
ˆˆ00
,
ˆˆ 00
ˆˆ 00
xx
xx
CC
x
x
x
x











1
ˆ
x
and 2
ˆ
x

(6)
where
Reme co
and is one full rank matrix, the
uldfferent depending on the values of
arks: Although thding ween
determinate
1

wo 2

be di .
j
e

The
m performance is weak because there are mny null
thg matri
4. m
mulation results [1,7] show
d Jafarkhani code [6]
SNR region (15-25dB).
syste a
parts ine codinx.
Transission Scheme
To check previous works [1,7], the diversity gain of high
rate transmission of STBCs is weak compared to Jafark-
hani code with rate-1. The si
that the BER gap between [1] an
has an almost 3dB gap in the high
We propose a new set of STBCs in this paper to transmit
one additional information bit b with an improvement of
BER performance compared to [1].
4.1. A Set of STBC Matrices
Similar to the method of [1], we consider a set of 44

STBCs consisting of two STBC matrices QOSTBC
[6] and Here, we propose 11

OSTBC as a
ission
22.22

s tr
tion
can-
scheme didate and use 11
 for 4-antenna
to transmit one additional informaansm bit
12 4
21 43
11 1234
3412
(,, , ,0).
3
4321
x
xxx
x
xxx
xxxxb
x
xxx
x
xx
 
 










 (7)
x


)
The is the Alamouti code [4] and shown as

0
22 1 2340
(,) 0
(, ,, ,1).
0(,
yz
xxxxb yz






 (8)
0(,)yz

12
21
123 4
0
3412
(,)
jj
jj
xe xxex
yz xexxe x







  

 (9)

 
jj 




12
21
12 3 4
0
3412
(,) jj
xe xxex
yz xexxex




 



j
(10)
where we denote y as 1
12
x
ex

and denote z as
2
34
.
j
x
ex


rate properIt is easy to chorthogonality and full ty of eck the
22

22
2
22
00
0
,
00 0
y
yz






2
22
22
000
000
H
yz
z
yz









(11)
where denotes absolute value and H denotes the


Y. YAN ET AL.
50
Hermitian of a matrix.
4.2. Angle Selections for 1

and 2

To observe equation (11), denote as the first transmit
codeword with signal 1
C
234
,
1
,,
x
xx
deword x with
and denote as
the second transmit cosignal 2
C
234
,,
1,
x
xx

an x
 
terminemploying transmit me de
gatrix 22
. Tht of
coding ain matrix for 1
C and 2 C is zero if 12 ,
x
x

34123
, , .
4
x
xx xxx 
 
Otherwise, the determinant is
extremely large if 1 2
, ,
341 2
,34 .
x
x x
, thxx xx
 In
1
x

 

forder to avoid this probleme values o

and 2

1
j
should be constrained to he make t12
x
ex


and
2
34
j
x
ex

 none zeros employinQPSK modulation.
Then, 1
g

and 2

must satisfy 14k

  and 24,k



0,1,2,..., .kN The set of these two ST

11 22
, is used to transmne additional bit with
optimization of coding ga in [1] achieving high
f the additional bit b comes
from these two canion
of the additional bit b is 0 the candidate (7) is lected
the transmitted matrix. Otherwise, the c8) is
another transmitted matrix to transmit additional
bit b = 1. To achieve good divrmance
coding gain defined in [2] 12 ()CGC C
BC codes
rate-
rmat
(
STB
it
in
n o
te matr
ersity
o
ices. W
per
9/8
fo as
te
f Cs
(QPSK). The informatio
dida
selected as
in the set , the
hile
fo
th
and
e in
se
ida
o


12
()
H
CC mu
g
the STBC m
of d
st be a full rank matrix. The drawback in
[1] is that while 1 C and 2 C are selected from 11  and
22 , respectively, the coding g ain matrix CG is singular
or null in submatrix to lose full rank property. To solve
this problem in [1], the authors proposed a criterion to
scale or rotate the transmitted symbols in 11  or 22 ,
by a weighted factor and an angle to guarantee full rank
property [2]. Due to the weighted factor and rotated angle
the codingain matrix also lo ses lit tle divers
ultiplied with a weighted factor individually
transmits to the receiver. In rder to compensate the loss
iversity gain we also propose another orthogonal
STBC matrix 22  to make the coding gain matrix be
full rank. If this coding gain matrix without a weighted
factor or null submatrices is also full rank, the design
does not lose divers ity gain.
4.3. Analysis of Coding Gain Matrix
In order to select the optimum v alues of 1
ity gai lein wh
o

and 2

， the
determinate of the coding gain matrix should be as large
as possible,
1212
12 12
max( )( )
0, 2;,4,
H
CCCC
subject tok  

 (12)
where and are selected from and
deriv
fi
1 2
respectively. We will neglect th e mathematical
C C11 
n be
22 ,
ation
ed by to solve the problem, but these values caveri
computer simulation results. Then, these optimum values
are 138

  and 238,

  respectively. Assume
that 1 C is selected from matrix and is selected
from with same informatmhe coding
gainx is given by (13)

11 
ion sy2
C
bols, t


ma22 tri
2
x
x


1122 3434
()xxxx xxxx
x xx
 
  
 


3
4 11243
34112234
43234112
1122 3434
234 1124
()
()
()
()
()
xx xxxxx
CG xxxxxxxx
xxxxxxxx
xxxx xxxx
x xxx


 

  






 
 



 

3
34112234
43234112
()
()
H
xxxxxx
xxxxxxxx

  

 



 
xx






(13)
where 1
11
j
x
xe


 and 2
33
.
j
x
xe



note 11
To simpe equation
(13 e2
lify th
), let 1 y d
x
xx
 a denote nd 2
y
234
().
x
xx


e sam

h O e form
bvi gairix (13) ously, this
as (7), codingn m
4
3
2
1
x
x
at
has t
123
21 4
12 341
432
yyx
yy x
CC
x
xyy
x
xyy





n i










(14)
milar OSTBC
t dulatios
n,
and is to Qwith full rank property.
Assume thaQPSK mo employed in the
tranthe terms of 112
si
issiosm
x
xx
 and 234
()
x
xx



are none zeros for all combinations of 1234
,,,.
x
xxx
Furthermore, the coding gaintrix ma12
()C CCG


12
()
H
CC
r all fo maintains full rank
1
without null submatrices
11
C and 22

2 C

ou
oue ST
a
erty
with
gh th
trix form
. It can
t
to [1].
h
versity be
c
stem
,
los
BC
22

show
s of coding
matrix gain com
involves t
achieve
, we
p
fu
ared
e ort
ll di
Alth
ogonal m
prop
22

does not
n from the
h
simulation result that there is a small gap between the
11
 and 22 
4.4. Decoding Algorithm
Hereonsider the problem of calculating the metric
for the sy model (1)
2
min YHX ()
for the implementation of a sequential decoding algo-
rithm. The decoding algorithm can be divided into two
sub-decoding procedures: 1) We select all possible se-
nces f
14
quer o1234
,,,
x
xxx from the first candidate relying
mbols on (7) to decode the transmitted sy2
1,YHX
where X corresponds to th
1 1
2) Similarly, we also use this ML principle to decode
other possible transmitted
e first candidate STBC
symbols selected from
candi
;
another
date 2
2,YHX where
date STBC3) To 2 corresponds to the decode the additional
X
first candi 1;
information bit, we also have to compare these two met-
rics for decoding it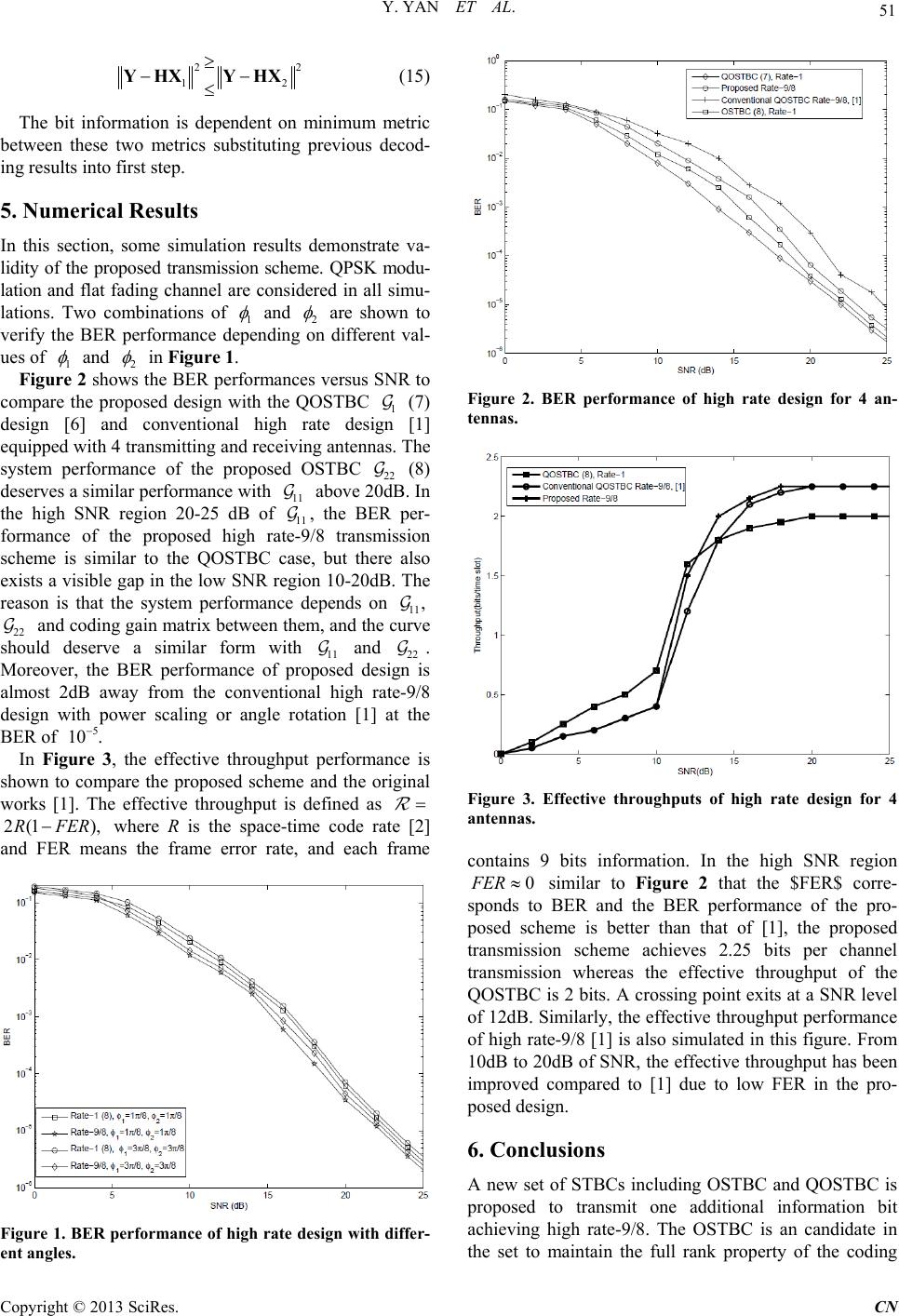

Y. YAN ET AL. 51
22
2

YHX (15)
The b information is dependent on minimum me
between these two metrics substituting previous decod-
ing results into first step.
5. Numerical Results
In this section, some simulation results demonstrate va-
lidity of the propose
1
YHX
it tric
d transmission scheme. QPSK modu-
lation and flat fading channel are consid
lations. Two combinations of ered in all simu-
1

and 2

are shown to
verify the BER performance depending on different val-
ues of 1

and 2

in Figure 1.
Figure 2 shows the BER performances versus SNR to
compare the proposed design with the QOSTBC (7)
nal high rate desi1]
and receiving anhe
gh
vaR 10-2he
re
1

gn [
tennas. T
0dB. T
ate
design [6] and conventio
equipped with 4 transmitting
system performance of the proposed OSTBC 22
 (8)
deserves a similar performance with 11  above 20dB. In
the high SNR region 20-25 dB of 11 , the BER per-
formance of the proposed hi rate-98 transmission
scheme is similar to the QOSTBC case, but there also
exists aisible gp in the low SN
/
region
ason is that the system performance depends on 11,
22
 and coding gain matrix between them, and the curve
should deserve a similar form with 11
 and 22 .
Moreover, the BER performance of proposed design is
almost 2dB away from the conventional high r-9/8
design with power scaling or angle rotation [1] at the
BER of 5
10 .

In Figure 3, the effective throughput performance is
shown to compare the proposed scheme and the original
works [1]. The effective throughput is defined as


2(1 ),RFER where R is the space-time code rate
FER means the frame error rate, and each frame
[2]
and
Figure 2. BER performance of high rate design for 4 an-
tennas.
Figure 3. Effective throughputs of high rate design for 4
ntennas.
contains 9 bits information. In the high SNR region
a
0FER

similar to Figure 2 that the $FER$ corre-
sponds to BER and the BER performance of the pro-
posed scheme is better than that of [1], the proposed
transmission scheme achieves 2.25 bits per channel
transmission whereas the effective throughput of the
QOSTBC is 2 bits. A crossing point exits at a SNR level
of 12dB. Similarly, the effectiv e throughput performance
of high rate-9 /8 [1] is also simulated in this figure. From
10dB to 20dB of SNR, the effective throughput has been
improved compared to [1] due to low FER in the pro-
posed design.
6. Conclusions
to transmit one additional information bit
A new set of STBCs including OSTBC and QOSTBC is
proposed
achieving high rate-9/8. The OSTBC is an candidate in
the set to maintain the full rank property of the coding
Figure 1. BER performance of high rate design w ith differ-
ent angles.

Y. YAN ET AL.
52
gain matr
-20014-0, BSRP 2010-0020942 NRF, Ko-
02521, NRF Korea, National Nature
of China (61201249), and Natural
ix. From the simulation results, the system per-
formance is improved with a similar BER performance to
Jafarkhani scheme in the high SNR region. In the future
works, we are interested in enlarging the set of STBC
matrices and combining some error correcting codes to
reduce the coding rate to achieve better system perform-
ance by exploiting the additional information bit.
7. Acknowledgements
This work was supported by World Class University
R32-2012-000
rea, MEST 2012-0
Science Foundation
Science Foundation of Guangdong Province (S201104
0004068), Chin a.
REFERENCES
[1] S. Das, N. Al-Dhahir and A. Calderbank, “Novel Full-
Diversity High Rate STBC for 2 and 4 Transmit Anten-
nas,” IEEE Communication Letters, Vol. 10, No. 3, 2006,
pp.171-173. doi：10.1109/LCOMM.2006.1603374
[2] V. Tarokh, N. Seshedri and A. R. Calderbank,
“Space-Time Codes for High Data Rate Wireless Com-
munication: Performance Criterion and Code Construc-
tion,” IEEE Tra, Vol. 44, nsactions on Information Theory
No. 2, 1998, p/18.661517p. 744-765.doi：10.1109
[3] V. Tarokh and A. R. Calderbank, “Space-Time Block
Codes from Orthogonal Designs,” IEEE Transactions on
Information Theory, Vol. 45, No. 5, 1999, pp.
1456-1467.doi：10.1109/18.771146
[4] S. M. Alamouti, “A Simple Transmit Diversity Technique
for Wireless Communications,” IEEE Journal on Select
Areas in Communications, Vol. 16, No. 8, 1998,
pp.1451-1458.doi：10.1109/49.730453
[5] B. Hassibi, B. M. Hochwald, A. Shokrollahi and W.
Space Time Block
Sweldens, “Representation Theory for High-rate Multi-
ple- antenna Code Design,” IEEE Transations on Infor-
mation Theory, Vol. 47, No. 9, 2001, pp. 2335-2367.
[6] H. Jafarkhani, “A Quasi-orthogonal
Code,” IEEE Transactions on Communication, Vol. 49,
No.1, 2001, pp. 1-4. doi：10.1109/26.898239
[7] H. N. Duy, Na. H. Nguyen, T. Nguyen and D.
Hoang, “High-Rate Space-Time Block Coding
Schemes,” IEICE Transactions on Fundamentals of
Electronics, Communications and Computer Sci-
ences, Vol. E91-A, No. 11, 2008, pp. 3393-3397.