Applied Mathematics, 2010, 1, 464-469
doi:10.4236/am.2010.16061 Published Online December 2010 (http://www.SciRP.org/journal/am)
Some Wgh Inequalities for Univalent Harmonic
Analytic Functions
Poonam Sharma
Department of Mathematics and Astron o my, Uni versi ty of Lucknow , L ucknow , In di a
Received August 2, 2010; revised September 27, 2010; accepted October 2, 2010
Abstract
In this paper, some Wgh inequalities for univalent harmonic analytic functions defined by Wright's genera-
lized hypergeometric (Wgh) functions to be in certain classes are observed and proved. Some consequent
results are also discussed.
Keywords: Harmonic Functions, Harmonic Starlike Functions, Wright’s Generalized Hypergeometric
Functions
1. Introduction and Preliminaries
Let u and v be real valued harmonic functions in a
simply connected domain Din the complex plane,
then a continuous function
f
uiv

 is called a com-
plex valued harmonic map in D. Clunie and Sheil-
Small [1] introduced a class SH of complex valued
harmonic maps
f
which are univalent and sense-pre-
serving in the open unit disk

:,1zz z

 and
assume a normalized representation hgwhere
11
11
(),1, (),1
nn
nn
nn
hzhz hgzgzg


 

(1)
are analytic and univalent in

. Let *()SH

denotes
the class of maps
f
hgSH satisfying the condi-
tion

'
() ()
arg( ())ImRe()
()
i
i
i
fre zf z
fre fz
fre






 










for ,0 1,02
i
zre r


  and 01

, where
'''
()() ()zfzzh zzgz.
Denote by TSH the subclass of function
f
hgSH such that
21
(), ().
nn
nn
nn
hzzh zgzgz


 

Also denote ** () ()THSSH TSH  .
We have following result from the work of Jahangiri
[2]:
Lemma 1. Let
f
hgSH

 , ()hz and ()
g
z are
given by (1), satisfies


11
10
21
nnn n
nn
nh ghg







 (2)
then
f
is sense preserving, harmonic univalent in

and *()fSH

. Furthermore, *()fTSH

 if and
only if (2) holds.
For some


1,2, 3,k , corresponding to ()hz
and ()
g
z defined in (1), let
() () ()
kkk
f
zhzgzSH

 (3)
where for z

,

1
1
0
() ()*1
nk
knk
kn
z
hz hzhz
z




 (4)

1
1
0
() ()*1
nk
knk
kn
z
g
zgz gz
z




 (5)
'*' stands for convolution. Since
1
11
11
10 10
k
nknk m
nknk m
nn mn
n
hzhzhz
n
 



 

 
()
k
hzand()
k
g
zfor some 2k in (3), represent series
of missing terms which increase with k. Involving
()
k
f
z, defined in (3), a class ()
k
SH

is defined as
follows:
Definition 1. A function
f
hgSH is said to
be in the class ()
k
SH

, if it satisfies the condition
'()
Re ()
k
zf z
fz







, 01,

 (6)

P. SHARMA
465
where for some k, ()
k
f
z is defined by (3). Func-
tions in the class ()
k
SH

are called harmonic starlike
functions with respect to k-symmetric points of order
.

Note that
*
1() ()SH SH

 ,2() ()
s
SH SH



and ()
k
TSH


() ,
k
SH TSH

() ().
ss
TSHSH TSH

  The class
()
s
SH

is studied by Ahuja and Jahangiri in [3] (see also
[4]). They also proved following result in [3].
Lemma 2. Let
f
hgSH , ()hz and ()
g
z are
given by (1), satisfies


21 21
10
21
nnn n
nn
nh ghg





 
 (7)
then
f
is sense preserving, harmonic univalent in

and ()
s
fSH

. Furthermore, ()
s
fTSH

 if and
only if (7) holds.
Shaqsi and Darus in [5,6] proved that for 01


,
k if ()
k
fSH

, then *()
k
fSH

 and proved
following result.
Lemma 3. Let
f
hgSH , ()hz and ()
g
z are
given by (1), satisfies for some k,


11
10
21
n nnknk
nn
nh ghg






 (8)
then
f
is sense preserving, harmonic univalent in

and ()
k
fSH

. Furthermore, ()
k
fTSH

 if and
only if (8) holds.
Obviously Inequality (8) is a generalized inequality
ensuring
f
to be in classes *()SH

and ()
s
SH

for 1k and 2k respectively. We see that if in-
equality (8) holds, inequality (2) must hold for any
01

and for 0

both are same. Hence, inequality
(8) for kand 01

 , ensures that
*()fSH

and thus it is used in this study.
If ()0,,gz zwe denote()()
kk
SH S

  which
is studied by Wang et al. [7] for

1(12)
() (1 )
z
zz



in
the respective class. The class *
2(0)
s
SS is introduced
by Sakaguchi [8] whose members satisfy the condition
'
2
()
Re 0
()
zh z
hz




,z
where
2
()()
() .
2
hzh z
hz



Connectivity of hypergeometric functions with har-
monic functions is seen through some of the recent pa-
pers [9-11]. Specially involvement of Wright’s genera-
lized hypergeometric (Wgh) functions is studied in
[12-23]. Some Wgh inequalities for starlike and convex
classes have already been obtained in [21,23] for certain
harmonic functions.
The Wright’s generalized hypergeometric (Wgh) func-
tion [24,25], for positive real numbers
,( 1,2,,)
ii
aAi p

 and ,( 1,2,,)
ii
bBi q
with
11
10
qp
ii
ii
BA



 , is defined by
 

  




112 2
112 2
1
1
1
,,,,, ;
,,,, ,
,;,
,!
pp
pp
pn
ii
ii iq
n
ii ii
i
aAaAa A
pq Z
bBbBb B
anAz
aA
pq Zz
bB bnBn




















(9)
Referring to [26], the series in (9) is absolutely con-
vergent z

 if
11
10
qp
ii
ii
BA



 and if
11
10
qp
ii
ii
BA



 , it is absolutely convergent for
1
1
i
i
q
B
i
ipA
i
i
B
z
A




and for 1
1
i
i
q
B
i
ipA
i
i
B
z
A




,
11
1
22
qp
ii
ii
pq
ba




 .
Involving Wgh functions as defined in (9), we consid-
er a univalent, harmonic function ()Wz of the form:
()()()WzHz GzSH (10)
where



1
1
11,
,,1,1
() ,
() ;
1,
()
qii n
iqn
n ii
iiq
aA
bq
H
zzz z
qbB
a






 



 
(11)


1,
1,
,,(1,1)
1,
1
()
() ;
()
iis
iis
scC
iss dD
ii
d
Gz z
c









1
,1
n
n
n
z  




 (12)
and



1
(1) ()
:(1) ()
qiii
niiii
anAb
bnB a



 
 (13)



1
(1) ()
:(1) ()
qiii
niiii
cnC d
dnDc



 
. (14)
Denote for some

00j and for any
k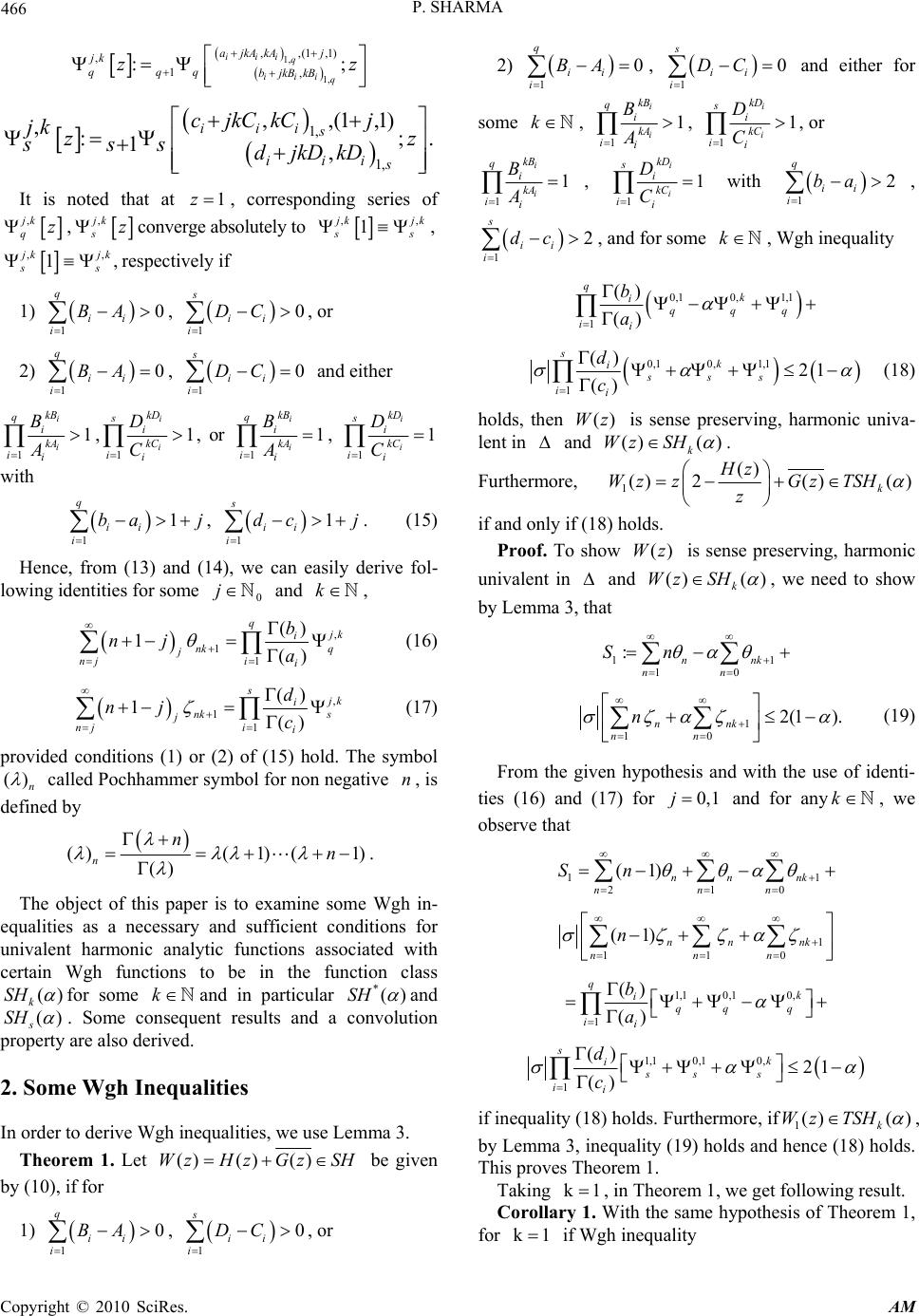

,

P. SHARMA
466



1,
1,
,,(1,1)
,
1,
:;
iii
q
iii
q
ajkAkA j
jk
qqqbjkBkB
z
z









1,
1,
,,(1,1)
,:;.
1,
iii
s
iii
s
cjkC kCj
jk zz
sss djkD kD







It is noted that at 1z, corresponding series of


,jk
qz,


,jk
szconverge absolutely to


,,
1
j
kjk
s
s
,


,,
1,
j
kjk
ss
respectively if
1)

1
0
q
ii
i
BA


,

1
0
s
ii
i
DC



, or
2)

1
0
q
ii
i
BA


,

1
0
s
ii
i
DC



 and either
1
1
i
i
kB
qikA
ii
B
A


,
1
1
i
i
kD
sikC
ii
D
C


, or
1
1
i
i
kB
qikA
ii
B
A


,
1
1
i
i
kD
sikC
ii
D
C



with

1
1
q
ii
i
ba j


,

1
1
s
ii
i
dc j


. (15)
Hence, from (13) and (14), we can easily derive fol-
lowing identities for some 0
j

 and k,

,
1
1
()
1()
q
j
k i
nk q
j
nj ii
b
nj a 




 

 (16)

,
1
1
()
1()
s
j
k
i
nk s
j
nj ii
d
nj c 




 

 (17)
provided conditions (1) or (2) of (15) hold. The symbol
()
n

called Pochhammer symbol for non negative n, is
defined by

()(1) (1)
()
n
nn 




.
The object of this paper is to examine some Wgh in-
equalities as a necessary and sufficient conditions for
univalent harmonic analytic functions associated with
certain Wgh functions to be in the function class
()
k
SH

for some kand in particular *()SH

and
()
s
SH

. Some consequent results and a convolution
property are also derived.
2. Some Wgh Inequalities
In order to derive Wgh inequalities, we use Lemma 3.
Theorem 1. Let ()()()WzHz GzSH be given
by (10), if for
1)

1
0
q
ii
i
BA


,

1
0
s
ii
i
DC



, or
2)

1
0
q
ii
i
BA



,

1
0
s
ii
i
DC


 and either for
some k

,
1
1
i
i
kB
qikA
ii
B
A


,
1
1
i
i
kD
sikC
ii
D
C


, or
1
1
i
i
kB
qikA
ii
B
A


,
1
1
i
i
kD
sikC
ii
D
C


 with

1
2
q
ii
i
ba



,

1
2
s
ii
i
dc



, and for some k, Wgh inequality

0,10, 1,1
1
()
()
qk
iqqq
ii
b
a




 




0,10, 1,1
1
() 21
()
sk
isss
ii
d
c





 

 (18)
holds, then ()Wz is sense preserving, harmonic univa-
lent in

and ()( )
k
Wz SH


.
Furthermore, 1
()
()2()( )
k
Hz
WzzGz TSH
z





if and only if (18) holds.
Proof. To show ()Wz is sense preserving, harmonic
univalent in

and ()( )
k
Wz SH


, we need to show
by Lemma 3, that
11
10
:nnk
nn
Sn 






1
10
2(1 ).
nnk
nn
n











 (19)
From the given hypothesis and with the use of identi-
ties (16) and (17) for 0,1j

and for anyk

, we
observe that
11
210
(1)
nn nk
nnn
Sn 




 

1
110
(1)
nn nk
nnn
n 





 





1,1 0,10,
1
()
()
qk
iqq q
ii
b
a






 





1,1 0,10,
1
() 21
()
sk
iss s
ii
d
c




  



if inequality (18) holds. Furthermore, if1()( )
k
Wz TSH


,
by Lemma 3, inequality (19) holds and hence (18) holds.
This proves Theorem 1.
Taking k1

, in Theorem 1, we get following result.
Corolla ry 1. With the same hypothesis of Theorem 1,
for k1

if Wgh inequality

P. SHARMA
467




0,11,1
1
0,1 1,1
1
() 1
()
()
(1)2 1,
()
qiqq
ii
siss
ii
b
a
d
c







 



(20)
holds, then ()Wz is sense preserving, harmonic univa-
lent in  and * ()( )Wz SH

. Furthermore,
*
1
()
()2()( )
Hz
WzzGz TSH
z




 if and only if
(20) holds.
Remark 1. Taking (1,2,,)
ii
A
Bi q

 and
(1,2,,)
ii
CDi s, the inequality of Corollary 1
coincides with Theorem 3.1 in [23] for
p
1.
Taking k2 in Theorem 1, we get following result.
Corolla ry 2. With the same hypothesis of Theorem 1,
for k2 if Wgh inequality

0,10,2 1,1
1
()
()
qiqqq
ii
b
a







0,10,2 1,1
1
() 21
()
sisss
ii
d
c



 

 (21)
holds, then ()Wz is sense preserving, harmonic univa-
lent in  and ()( )
s
Wz SH

.
Furthermore, 1
()
()2()()
s
Hz
WzzGz TSH
z





if and only if (21) holds.
3. Consequences of Wgh Inequalities
Involving Mittag-Leffler functions [25]: 11
1,1
,()
Bb
Ez


11
(1,1)
11 ,;
bB z

 ,

11 11
1,1 (1,1)
,11
,
() ;
Dd dD
Ez z



, for positive
real numbers 111 1 ,and,bB dD, we consider a univalent,
harmonic function ()Ez for 1   of the form:
111 1
1,1 1,1
1, 1,
()()()()()
Bb Dd
EzzbEzzd EzSH   (22)
Denote for some

00j and k,

11 1111
1,1 (1,1)
,11
,
(); ,
jj
kB bjkBbjkBkB
Ez z







 11 1111
1,1 (1,1)
,11
,
(); .
jj
kD djkDdjkDkD
Ez z







At 1z, corresponding series of 111
1,1
,()
j
kB bjkB
Ez

,
11 1
1,1
,()
j
kD djkD
Ez

 converge absolutely to
11 111 1
1,11,1
,,
(1) ,
jj
kB bjkBkB bjkB
EE


11 111 1
1,1 1,1
,,
(1) ,
jj
kD djkDkDdjkD
EE



respectively. Following result can be directly obtained
from Theorem 1.
Corolla ry 3. Let ()Ezbe defined by (22), if for some
k

, inequality




111111 1
1111111
1,11,1 2,1
1,,,
1,11,1 2,1
1,,,
()
()21 ,
BbkBbBb B
DdkDdDd D
bEEE
dEE E







(23)
holds, then ()Ez is sense preserving, harmonic univa-
lent in

and ()( )
k
Ez SH


. Furthermore,

111 1
1
1,1 1,1
1, 1,
()
2() ()()()()
BbDd k
Ez
zbEzzdEzTSH



 
if and only if (23) holds.
Results similar to the Corollaries 1 and 2, for
()Ez and 1()Ezcan be obtained by taking k1

and
k2

respectively in Corollary 3.
On taking1
ii
AB

,1, 2, 3,,iq

 and 1
ii
CD

,
1,2, 3,,is

, ()Wzreduces to
 
( )([]),([]),,
qi si
F
zzFaz zFczSH   
(24)
which involve the generalized hypergeometric functions:



1,
1,
,1,(1,1)
1,1
([ ]),;
iq
iq
a
qiqq b
F
az z




,



1,
1,
,1, (1,1)
1,1
([ ]),;
is
is
c
sissd
F
cz z




.
Also, if ==1,
ii
AB =1,2,3, ,iqand==1,
ii
CD
=1,2,3,, ,isfor some

00j andk

,
we get



,,
=1 =1
()
=(1) ,
()
qq
ijk ijk jk
qq
ii
iijk
a bjF
ab






,,
=1 =1
()
=(1) ()
ss
ijk
ijk jk
ss
ii
iijk
c
djF
cd



,
where
,,
=0 =1
()(1)
:=([]),
()!
q
jk jkilk l
qqi
liilk
ajk j
FFa bjkl



,,
=0 =1
()(1)
:=([])()!
s
jk jkilk l
ssi
liilk
cjk j
FFc djk l



provided

1
1
q
ii
i
ba j



,

1
1
s
ii
i
dc j


.
From Theorem 1, we obtain following result.
Corollary 4. Let ()
F
z be defined by (24), if for
some k

 and

1
2
q
ii
i
ba



,

1
2
s
ii
i
dc


,
inequality
0,10, 1,1
=1
q
ki
qqq
ii
a
FFF
b
 


P. SHARMA
468

0,10, 1,1
=1
21
s
ki
sss
ii
c
FFF
d



 


 (25)
holds, then ()
F
z is sense preserving, harmonic univa-
lent in  and ()()
k
Fz SH

. Furthermore,



1( )2([]),([]),() qisi k
FzzFa zzFcz TSH

 
if and only if (25) holds.
Results similar to the Corollaries 1 and 2, for
()
F
zand 1()
F
zcan be obtained by taking k1

and
k2 respectively in Corollary 4.
Further, taking 2qs,22 1bd, in Corollary 4,
we get following result for a harmonic univalent function
defined by Gauss hypergeometric functions.
Corollary 5. Let for positive real values of
12112 1
,,,,,aabccd and for 1 

, a harmonic univalent
function:

21121 21121
(),;;,;;.GzzFaabzzFccdz SH   
If for some kand

112
>1,baa

11 2
>1,dcc
inequality

0,1 0, 12
22
112
1 ([]) ([])
1
k
ii
aa
F
aFa
baa








0,1 0, 12
22
11 2
||1 ([])([])
1
k
ii
cc Fc Fc
dcc



 







2(1 ),

 (26)
holds, then ()Gz is sense preserving, harmonic
univalent in  and ()().
k
Gz SH


Furthermore,



12112121121
()2,;;,;;( )
k
GzzFaabzzFccdz TSH

 
if and only if (26) holds.
Results similar to the Corollaries 1 and 2, for
()Gzand 1()Gzcan be obtained by taking k1

and
k2 respectively in Corollary 5.
4. Convolution Property
In this section, we obtain a covolution property for
functions belonging to the class ().
k
SH

Theorem 2. A function=()
k
fhgSH

 for some
k if and only if




2
12 1
() 1
1k
zz
hz z
z













2
121
() 0,
1
1k
zz
gz z
z




 




=1,1,0< <1.z  
Proof. From the definition of the function class
(),
k
SH

f

(),
k
SH

if and only if
1()1
,
(1)()1
'
k
zf z
fz












for =1,1,0< <1.z
  Hence by simple
calculations, we get
 
1() ()1(1)()0.
'
kk
zfzf zf z 





Using (3), we get

 
1()() ()()
11
''
kk
zz
zhzzgzh zg z
zz





















 
1(1) ()()0
11
kk
zz
hz gz
zz




 




which easily derives the result.
Based on Theorem 2, we get that harmonic functions,
()()()WzHz Gz
111 1
1,1 1,1
1, 1,
()()()()()
Bb Dd
EzzbEzzdEz  , and
 
( )([]),([]),,
qi si
F
zzFaz zFcz   defined in (10),
(22) and (24) respectively belong to the class ()
k
SH

for some k

 if and only if for
=1,1,0< <1,z  




2
12 1
() 1
1k
zz
Hz z
z









 







2
12 1
() 0,
1
1k
zz
Gz z
z














11
1,1
1, 2
12 1
() ()1
1
Bb k
zz
zbE zz
z


















11
1,1
1, 2
121
() ()0
1
1
Dd k
zz
zdE zz
z






 




and





2
12 1
([]),1
1
qi k
zz
zFa zz
z



















2
121
([ ]),0
1
1
si k
zz
zFc zz
z











respectively hold.

P. SHARMA
469
5. References
[1] J. Clunie and T. Sheil-Small, “Harmonic Univalent Func-
tions,” Annales Academiae Scientiarum Fennicae. Series
A I. Mathematica, Vol. 9, 1984, pp. 3-26.
[2] J. M. Jahangiri, “Harmonic Functions Starlike in the Unit
Disk,” Journal of Mathematical Analysis and Applica-
tions, Vol. 235, No. 2, 1999, pp. 470-477.
[3] O. P. Ahuja and J. M. Jahangiri, “Sakaguchi-Type Har-
monic Univalent Functions,” Scientiae Mathematicae
Japonicae, Vol. 59, 2004, pp. 239-244.
[4] H. Ö. Ġuney, “Sakaguchi-Type Harmonic Univalent
Functions with Negative Coefficients,” International
Journal of Contemporary Mathematical Sciences, Vol. 2,
No. 10, 2007, pp. 459-463.
[5] M. Al. Shaqsi and M. Darus, “On Subclass of Harmonic
Starlike Functions with Respect to K-Symmetric Points,”
International Mathematical Forum, Vol. 2, No. 57, 2007,
pp. 2799-2805.
[6] M. Al. Shaqsi and M. Darus, “On Harmonic Univalent
Functions with Respect to K-Symmetric Points,” Interna-
tional Journal of Contemporary Mathematical Sciences,
Vol. 3, No. 1-4, 2008, pp. 111-118.
[7] Z. G. Wang, C. Y. Gao and S. M. Yuan, “On Certain
Subclasses of Close-to-Convex and Quasi-Convex Func-
tions with Respect to K-Symmetric Points,” Journal of
Mathematical Analysis and Applications, Vol. 322, No. 1,
2006, pp. 97-106.
[8] K. Sakaguchi, “On Certain Univalent Mapping,” Math-
matical Society of Japan, Vol. 11, 1959, pp. 72-75.
[9] O. P. Ahuja, “Planar Harmonic Convolution Operators
Generated by Hypergeometric Functions,” Integral
Transforms and Special Functions, Vol. 18, No. 3, 2007,
pp. 165-177.
[10] O. P. Ahuja, “Harmonic Starlike and Convexity of
Integral Operators Generated by Hypergeometric Series,”
Integral Transforms and Special Functions, Vol. 20, No.
8, 2009, pp. 629-641.
[11] O. P. Ahuja and H. Silverman, “Inequalities Associating
Hypergeomatric Functions with Planer Harmonic Map-
pings,” Journal of Inequalities in Pure and Applied Ma-
thematics, Vol. 5, No. 4, 2004.
[12] M. K. Aouf and J. Dziok, “Distortion and Convolutional
Theorems for Operators of Generalized Fractional Cal-
culus Involving Wright Function,” Journal of Applied
Analysis, Vol. 14, No. 2, 2008, pp. 183-192.
[13] M. K. Aouf and J. Dziok, “Certain Class of Analytic
Functions Associated with the Wright Generalized
Hypergeometric Function,” Journal of Mathematics and
Applications, Vol. 30, 2008, pp. 23-32.
[14] J. Dziok and R. K. Raina, “Families of Analytic Func
tions Associated with the Wright Generalized Hyper-
geometric Function,” Demonstratio Mathematica, Vol.
37, No. 3, 2004, pp. 533-542.
[15] J. Dziok and R. K. Raina, “Some Results Based on First
Order Differential Subordination with the Wright’s Ge-
neralized Hypergeometric Function,” Commentarii Math-
ematici Universitatis Sancti Pauli, Vol. 58, No. 2, 2009,
pp. 87-94.
[16] J. Dziok, R. K. Raina and H. M. Srivastava, “Some
Classes of Analytic Functions Associated with Operators
on Hilbert Space Involving Wright’s Generalized Hyper-
geometric Function,” Proceedings of the Jangjeon Ma-
thematical Society, Vol. 7, 2004, pp. 43-55.
[17] G. Murugusundaramoorthy and R. K. Raina, “On a Sub-
class of Harmonic Functions Associated with the Wr-
ight’s Generalized Hypergeometric Functions,” Hacet-
tepe Journal of Mathematics and Statistics, Vol. 38, No.
2, 2009, pp. 129-136.
[18] G. Murugusundaramoorthy and K. Vijaya, “A Subclass
of Harmonic Functions Associated with Wright’s Hyper-
geometric Functions,” Applied Mathematics, Vol. 1, No.
2, 2010, pp. 87-93.
[19] R. K. Raina, “Certain Subclasses of Analytic Functions
with Fixed Argument of Coefficients Involving the
Wright’s Function,” Tamsui Oxford Journal of Mathe-
matical Sciences, Vol. 22, No. 1, 2006, pp. 51-59.
[20] R. K. Raina, “On Generalized Wright’s Hypergeometric
Functions and Fractional Calculus Operators,” East Asian
Journal of Mathematics, Vol. 21, No. 2, 2005, pp. 191-
203.
[21] R. K. Raina and P. Sharma, “Harmonic Univalent Func-
tions Associated with Wright’s Generalized Hyper- geo-
metric Functions,” Integral Transform and Special Func-
tions, Communicated for Publication.
[22] P. Sharma, “A Class of Multivalent Analytic Functions
with Fixed Argument of Coefficients Involving Wright’s
Generalized Hypergeometric Functions,” Bulletin of Ma-
thematical Analysis and Applications, Vol. 2, No. 1, 2010,
pp. 56-65.
[23] P. Sharma, “Multivalent Harmonic Functions Defined by
M-Tuple Integral Operators,” Commentationes Mathe-
maticae, Vol. 50, No. 1, 2010, pp. 87-101.
[24] E. M. Wright, “The Asymptotic Expansion of the Gene-
ralized Hypergeometric Function,” Proceedings London
Mathematical Society, Vol. 46, No.1, 1946, pp. 389-408
[25] H. M. Srivastava and H. L. Manocha, “A Treatise on
Generating Functions,” Halsted Press, Ellis Horwood
Limited, Hichester, 1984.
[26] A. A. Kilbas, M. Saigo and J. J. Trujillo, “On the Genera-
lized Wright Function,” Fractional Calculus and Applied
Analysis, Vol. 5, No. 4, 2002, pp. 437-460.