Applied Mathematics, 2010, 1, 456-463
doi:10.4236/am.2010.16060 Published Online December 2010 (http://www.SciRP.org/journal/am)
Thermal Effect on Free Vibration of Non-Homogeneous
Orthotropic Visco-Elastic Rectangular Plate of
Parabolically Varying Thickness
Arun Kumar Gupta, Pooja Singhal
Department of Mathematics, M. S. College, Saharanpur, India
Received August 31, 2010; revised September 17, 2010; accepted September 21, 2010
Abstract
A simple model presented here is to study the thermal effect on vibration of non-homogeneous orthotropic
visco-elastic rectangular plate of parabolically varying thickness having clamped boundary conditions on all
the four edges. For non-homogeneity of the plate material, density is assumed to vary linearly in one direc-
tion. Using the separation of variables method, the governing differential equation has been solved for vibra-
tion of non-homogeneous orthotropic visco-elastic rectangular plate. An approximate frequency equation is
derived by using Rayleigh-Ritz technique with a two-term deflection function. Results are calculated for time
period and deflection at different points, for the first two modes of vibration, for various values of tempera-
ture gradients, non-homogeneity constant, taper constant and aspect ratio and shown by graphs.
Keywords: Thermal Gradient, Vibration, Non-Homogeneous, Orthotropic, Visco-Elastic, Rectangular Plate,
Variable Thickness
1. Introduction
In modern technology an interest towards the effect of
high temperatures on non-homogeneous plates of varia-
ble thickness is developed due to applications in various
engineering branches such as nuclear, power plants,
aero-nautical, chemical etc. where metals and their alloys
exhibits visco-elastic behavior. Therefore for these
changes the structures are exposed to high intensity, heat
fluxes and material properties undergo significant
changes.
The materials are being developed, depending upon
the requirement and durability, so that these can be used
to give better strength, flexibility, weight effectiveness
and efficiency. So some new materials and alloys are
utilized in making structural parts of equipment used in
modern technological industries like space craft, jet en-
gine, earth quake resistance structures, telephone indus-
try etc. Applications of such materials are due to reduc-
tion of weight and size, low expenses and enhancement
in effectiveness and strength. It is well known that first
few frequencies of structure should be known before
finalizing the design of a structure. The study of vibra-
tion of plate structures is important in a wide variety of
applications in engineering design. Elastic plates are
widely employed nowadays in civil, aeronautical and
marine structures designs. Complex shapes with variety
of thickness variation are sometimes incorporated to re-
duce costly material, lighten the loads, and provide ven-
tilation and to alter the resonant frequencies of the struc-
tures. Dynamic behavior of these structures is strongly
dependent on boundary conditions, geometric shapes,
material properties etc.
As technology develops new discoveries day by day
like in jet engine, field of spacecraft and nuclear power
plants etc., the time dependent behavior of materials has
become of great importance. Thus, the need of the study
of vibration of visco-elastic plates (it may be rectangular,
circular, elliptical etc.) of certain aspect ratios with some
simple boundary conditions has been increased rapidly.
Vibration phenomenon, common in mechanical de-
vices and structures, is undesirable in many cases, such
as machine tools. But this phenomenon is not always
unwanted; for example, vibration is needed in the opera-
tion of vibration screens.
In the course of time, engineers have become increa-
singly conscious of the importance of an elastic behavior
of many materials and mathematical formulations have

A. K. GUPTA ET AL.
457
been attempted and applied to practical problems. Since
plastics and new materials are widely used in the con-
struction of equipment and structures, so the develop-
ment of the application of visco-elasticity is needed to
permit rational design.
Leissa’s monograph [1] contains an excellent discus-
sion of the subject of vibrating plates with elastic edge
support. Leissa [2] has given the solution for rectangular
plate of variable thickness. Gupta, Johri and Vats [3] have
discussed the thermal effect on vibration of non- homo-
geneous orthotropic rectangular plate having bi- direc-
tional parabolically varying thickness. Sobotka [4] dis-
cussed the free vibration of visco-elastic orthotropic rec-
tangular plates were discussed. Tomar and Gupta [5-7]
solved the vibration problem of orthotropic rectangular
plate of varying thickness subjected to a thermal gradient.
Lal [8] has studied the transverse vibrations of ortho-
tropic non-uniform rectangular plates with continuously
varying density.
Visco-elasticity, as its name implies, is a generaliza-
tion of elasticity and viscosity. The ideal linear elastic
element is the spring. When a tensile force is applied to it,
the increase in distance between its two ends is propor-
tional to the force. The ideal linear viscous element is the
dashpot.
The plate type structural components in aircraft and
rockets have to operate under elevated temperatures that
cause non-homogeneity in the plate material, i.e., elastic
constants of the materials becomes functions of space
variables. In an up-to-date survey of literature, authors
have come across various models to account for non-
homogeneity of plate materials proposed by researchers
dealing with vibration but none of them consider
non-homogeneity with thermal effect on visco-elastic
plates.
Gupta and Khanna [9] have studied the effect of li-
nearly varying thickness in both directions on vibration
of visco-elastic rectangular plate. Gupta and Kaur [10]
studied the effect of thermal on vibration of clamped
viscoelastic rectangular plate with linearly thickness in
both directions. Gupta, Kumar and Gupta [11] have con-
sidered the vibration of visco-elastic parallelogram plate
with parabolic thickness variation. Recently, Gupta and
Singhal [12] discussed the effect of non-homogeneity on
thermally induced vibration of orthotropic visco-elastic
rectangular plate of linearly varying thickness.
The object of the present study is to determine the
thermal effect on vibration of non-homogeneous ortho-
tropic visco-elastic rectangular plate of parabolically
varying thickness. It is clamped supported on all the four
edges. The assumption of small deflection and linear
orthotropic visco-elastic properties are made. It is further
assumed that the visco-elastic properties of the plates are
of the Kelvin type. Time period and deflection for the
first two modes of vibration are calculated for the various
values of thermal gradients, non-homogeneity constant,
aspect ratio and taper constant.
2. Analysis
The equation of motion of a visco-elastic rectangular
plate of variable thickness is [12]:
44 4
4422
333
22 3
2
2
2 322
32222
2
2' 2' 22 2
11
22 22
2
222
0
2
4
xy
x
yy
x
xy
WW W
DD H
xyxy
D HW HWW
xyx xyx yxhp W
DD
D WWW
yyxxyy
D
DD WW W
xy xy xy yx


 
 




  
 
  




 





 
  


   

(1)
and
.. ~
20TpDT

 (2)
where Equations (1) and (2) are the differential equations
of motion for orthotropic plate of variable thickness and
time function for visco-elastic orthotropic plate for free
vibration respectively.
Here p2 is a constant and
'
12
x
y
H
DD ,

3
12 1
x
x
x
y
Eh
Dvv ,
is called the flexural rigidity of the plate in x-direction,

3
12 1
y
y
x
y
Eh
Dvv ,
is called the flexural rigidity of the plate in y-direction,
3
12
xy
xy
Gh
D,
is called the torsion rigidity,


'
1
x
yyx
DvD vD ,
~
D is Rheological operator and
&
x
y
EE are the modules of elasticity in x- and
y-direction respectively,
x

and
y

are the Poisson
ratios &
x
y
G is the shear modulus.
Assuming steady one dimensional temperature distribu-
tion along the length, i.e., x-direction for the plate as
0
x
ττ1a




(3)

A. K. GUPTA ET AL.
458
where τ denotes the temperature excess above the ref-
erence temperature at any point at distance
x
a and
0
τ denotes the temperature excess above reference
temperature at the end, i.e., x=a.
Temperature dependence of the modulus of elasticity
for most of engineering materials can be expressed in
this form

11 x
EE

 ,

21 y
EE

 ,

01 xy
GG

  (4)
Here E1 and E2 are values of the Young’s moduli re-
spectively along the x and y axis at the reference temper-
ature, i.e., at τ = 0 and γ is the slope of the variation of
modulus of elasticity with τ.
Thus modulus variation become
 
111 x
Ex Exa  



,
 
211 y
Ex Exa  



,
 
011 xy
Gx Gxa  



 (5)
where

001
  , a parameter ,known as ther-
mal gradient.
The expression for the strain energy V and Kinetic
energy P in the plate are [1].
 
2 2
,,
00
1
2
ab
xxx yyy
VDWDW






2
1, ,,
24
xx yyxyxy
DW WDWdxdy


 (6)
22
00
1
2
ab
PphWdxdy   (7)
Assuming thickness and density varies parabolically
and linearly in the x-direction respectively, therefore one
can take


2
01hh xa
  (8)
and

01
1
x
a    (9)
where β is the taper constant and α1 is non-homogeneity
constant.
3. Solution and Frequency Equation
To find a solution, we use Rayleigh-Ritz technique. This
method requires that maximum strain energy must be
equal to the maximum kinetic energy. So, it is necessary
for the problem under consideration that

0VP   (10)
for arbitrary variations of W satisfying relevant geome-
trical boundary conditions which are
0
x
WW at 0,
x
a
0
y
WW at 0,yb (11)
and the corresponding two term deflection function is
taken as [12].

2
11Wxaybxayb








12 11
A
Axayb xayb



 (12)
The non-dimensional variables are
X
xa

, Yyb

, /, /WWahha
, ρa


(13)


*
11
1
x
y
EE vv,

*
22
1
x
y
EE vv
***
21xy
EvE vE
By using Equations (5), (8), (9) and (13) in (6) and (7),
one gets


2 1/
25 2
00
11 + 1
2
ba
oo
Ppha XXWdXdY 






(14)
and

 


2
3 1/ 2,
00 1 - 11
ba
XX
VRXX W 


 





 
2
** **
,,,
21 1
2
YYXX YY
EE WEEWW


 

2
*,
1
4XY
o
GE WdXdY


 (15)
where


3
*
1
112
2o
REha (16)
On substituting the values of P and V from Equations (14)
and (15) in Equation (10), we get


22
11
0VpP 

 (17)


 

2 3
1/ 2,
100 1 - 11
ba
XX
VXXW 


 







2
** **
,,,
21 1
2
YYXX YY
EE WEEWW
 

2
*,
1
4XY
o
GE WdXdY


 (18)


2 1/ 2
11
00 1 + 1
ba
PXX WdXdY 




 (19)
where
4
2
2
*
1
12 o
o
a
Eh

  (20)
Equation (17) involves the unknown A1 and A2 arising
due to the substitution of W(x,y) from Equation (12).
These two constants are to be determined from Equation
(17) as follows:

A. K. GUPTA ET AL.
459

22
11
n
VpP0
A


 where 1, 2n (21)
On simplifying (21) we get
112 20 nn
bAb A (22)
where 1, 2n, bn1, bn2 involves parametric constants
and the frequency parameter p. For a non-trivial solution,
the determinant of the coefficient of Equation (22) must
be zero. So, we get the frequency equation as
11 12
21 22
0
bb
bb
 (23)
On solving Equation (23) one gets a quadratic equa-
tion in p2, which gives two values of p2. On substituting
the value of 11A, by choice, in Equation (12) one get
21112
A
bb and hence W becomes:

2
11
aa
WXYX Y
bb








11
12
111
baa
XYX Y
bb b


 
 
 
 
  


(24)
4. Time Functions of Vibration of
Viscoelastic Plates
Equation (2) is defined as general differential equation of
time functions of free vibrations of visco-elastic ortho-
tropic plates. It depends on visco-elastic operator ~
D
.
One has, for Kelvin’s model [12],
~
1d DGdt
 






(25)
where  is visco-elastic constant and G is shear modulus.
Taking temperature dependence of viscoelastic constant
η and shear modulus G is the same form as that of
Young’s moduli, we have
 
01
1GG

 ,

 
02
1

   (26)
where G0 is shear modulus and 0 is visco-elastic con-
stant at some reference temperature, i.e., at
 = 0, 
1 and

2 are slope variation of
 with G and  respectively.
Substituting the value of
 from Equation (3) and using
Equation (13) in Equation (26), one gets:

05
11GG X   

, where 510

 ,
5
01

 and


04
11
X
   

,
where 420

 , 4 01

 (27)
Here 4 and 5 are thermal constants.
After using Equation (25) in Equation (2), one obtains
22
0TpkTpT
 

 (28)
where



04
05
11
11
X
kGGX








 




(29)
Equation (28) is a differential equation of second order
for time function T.
Solution of Equation (28) will be




1
1121
cos sin
at
TteCbt Cbt (30)
where 2
12apk (31)
2
112
pk
bp  


(32)
and C1 ,C2 are constants which can be determined easily
from initial conditions of the plate.
Assuming initial conditions as
1T

and 0T

 at 0t (33)
Using Equation (33) in Equation (30), one obtains
121
1
1&CCab

 (34)
One has


1
1111
cos sin
at
Ttebta bbt



 (35)
after using Equation (34) in Equation (30).
Thus, deflection of vibrating mode w(x,y,t), which is
equal to W(x,y)T(t), may be expressed as
 
2
11wXYab X Yab







 
11 12
111bbXY abXYab










1
1111
cos sin
at
ebtabbt




 (36)
by using Equations (24) & Equation (35).
Time period of the vibration of the plate is given by
2
K
p


(37)
where p is the frequency given by Equation (23).
5. Numerical Evaluations
The values of time period (K) and deflection (w) (at two
different instant of time) for a clamped visco-elastic or-
thotropic non-homogeneous rectangular plate for differ-
ent values of taper constant
 , thermal constants (α, α4,
α5), non homogeneity constant α1 and aspect ratio a/b at
different points for first two modes of vibrations are cal-
culated.
The following orthotropic material parameters have
been taken as [1].
**
21
0.32EE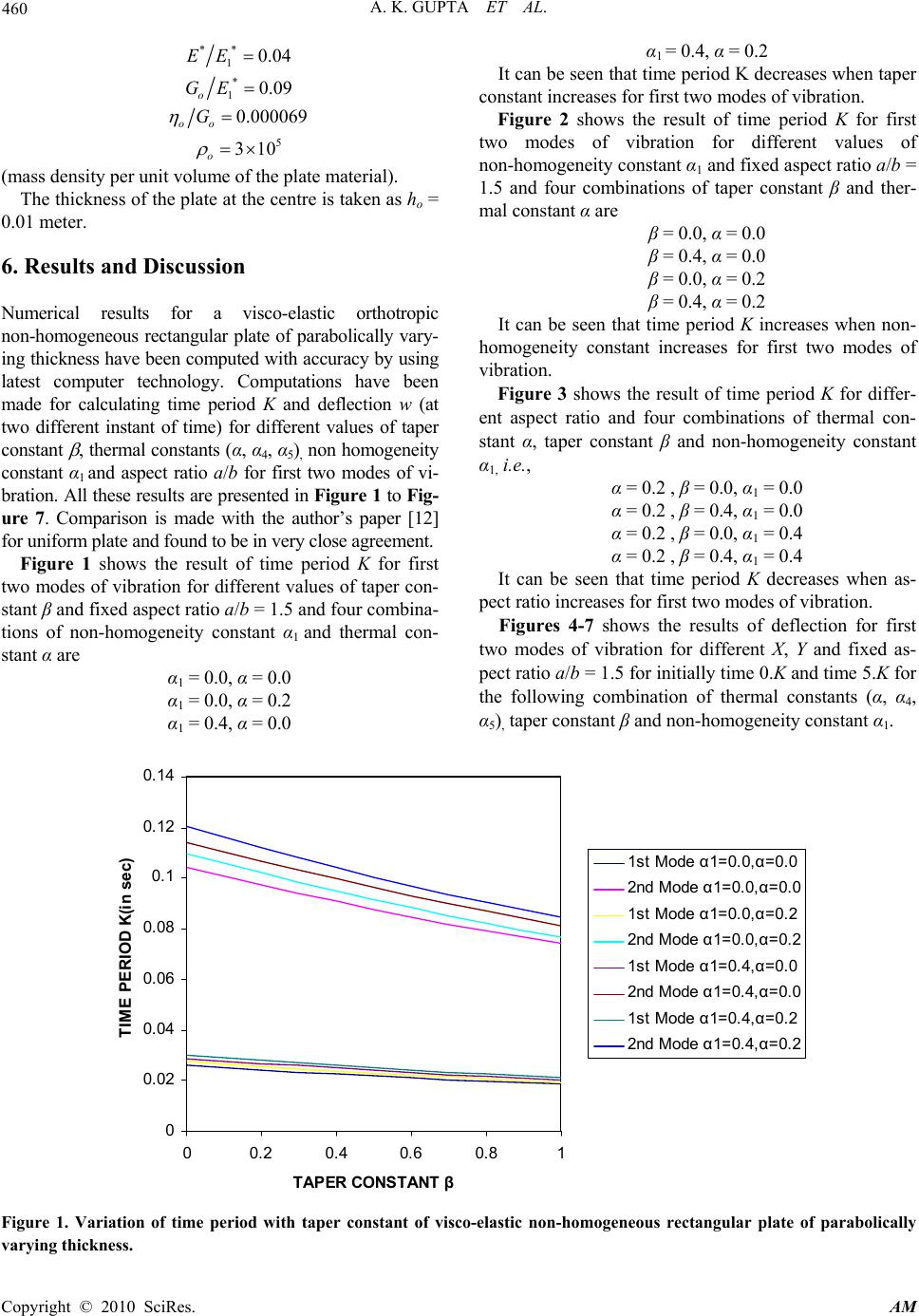

A. K. GUPTA ET AL.
460
**
10.04EE
*
10.09 o
GE
0.000069
oo
G


5
310
o
 
(mass density per unit volume of the plate material).
The thickness of the plate at the centre is taken as ho =
0.01 meter.
6. Results and Discussion
Numerical results for a visco-elastic orthotropic
non-homogeneous rectangular plate of parabolically vary-
ing thickness have been computed with accuracy by using
latest computer technology. Computations have been
made for calculating time period K and deflection w (at
two different instant of time) for different values of taper
constant  , thermal constants (α, α4, α5), non homogeneity
constant α1 and aspect ratio a/b for first two modes of vi-
bration. All these results are presented in Figure 1 to Fig-
ure 7. Comparison is made with the author’s paper [12]
for uniform plate and found to be in very close agreement.
Figure 1 shows the result of time period K for first
two modes of vibration for different values of taper con-
stant β and fixed aspect ratio a/b = 1.5 and four combina-
tions of non-homogeneity constant α1 and thermal con-
stant α are
α1 = 0.0, α = 0.0
α1 = 0.0, α = 0.2
α1 = 0.4, α = 0.0
α1 = 0.4, α = 0.2
It can be seen that time period K decreases when taper
constant increases for first two modes of vibration.
Figure 2 shows the result of time period K for first
two modes of vibration for different values of
non-homogeneity constant α1 and fixed aspect ratio a/b =
1.5 and four combinations of taper constant β and ther-
mal constant α are
β = 0.0, α = 0.0
β = 0.4, α = 0.0
β = 0.0, α = 0.2
β = 0.4, α = 0.2
It can be seen that time period K increases when non-
homogeneity constant increases for first two modes of
vibration.
Figure 3 shows the result of time period K for differ-
ent aspect ratio and four combinations of thermal con-
stant α, taper constant β and non-homogeneity constant
α1, i.e.,
α = 0.2 , β = 0.0, α1 = 0.0
α = 0.2 , β = 0.4, α1 = 0.0
α = 0.2 , β = 0.0, α1 = 0.4
α = 0.2 , β = 0.4, α1 = 0.4
It can be seen that time period K decreases when as-
pect ratio increases for first two modes of vibration.
Figures 4-7 shows the results of deflection for first
two modes of vibration for different X, Y and fixed as-
pect ratio a/b = 1.5 for initially time 0.K and time 5.K for
the following combination of thermal constants (α, α4,
α5), taper constant β and non-homogeneity constant α1.
0
0.02
0.04
0.06
0.08
0.1
0.12
0.14
00.2 0.40.6 0.81
TAPER CONSTANT β
TIME PERIOD K(in sec)
1st Mode α1=0.0,α=0.0
2nd Mode α1=0.0,α=0.0
1st Mode α1=0.0,α=0.2
2nd Mode α1=0.0,α=0.2
1st Mode α1=0.4,α=0.0
2nd Mode α1=0.4,α=0.0
1st Mode α1=0.4,α=0.2
2nd Mode α1=0.4,α=0.2
Figure 1. Variation of time period with taper constant of visco-elastic non-homogeneous rectangular plate of parabolically
varying thickness.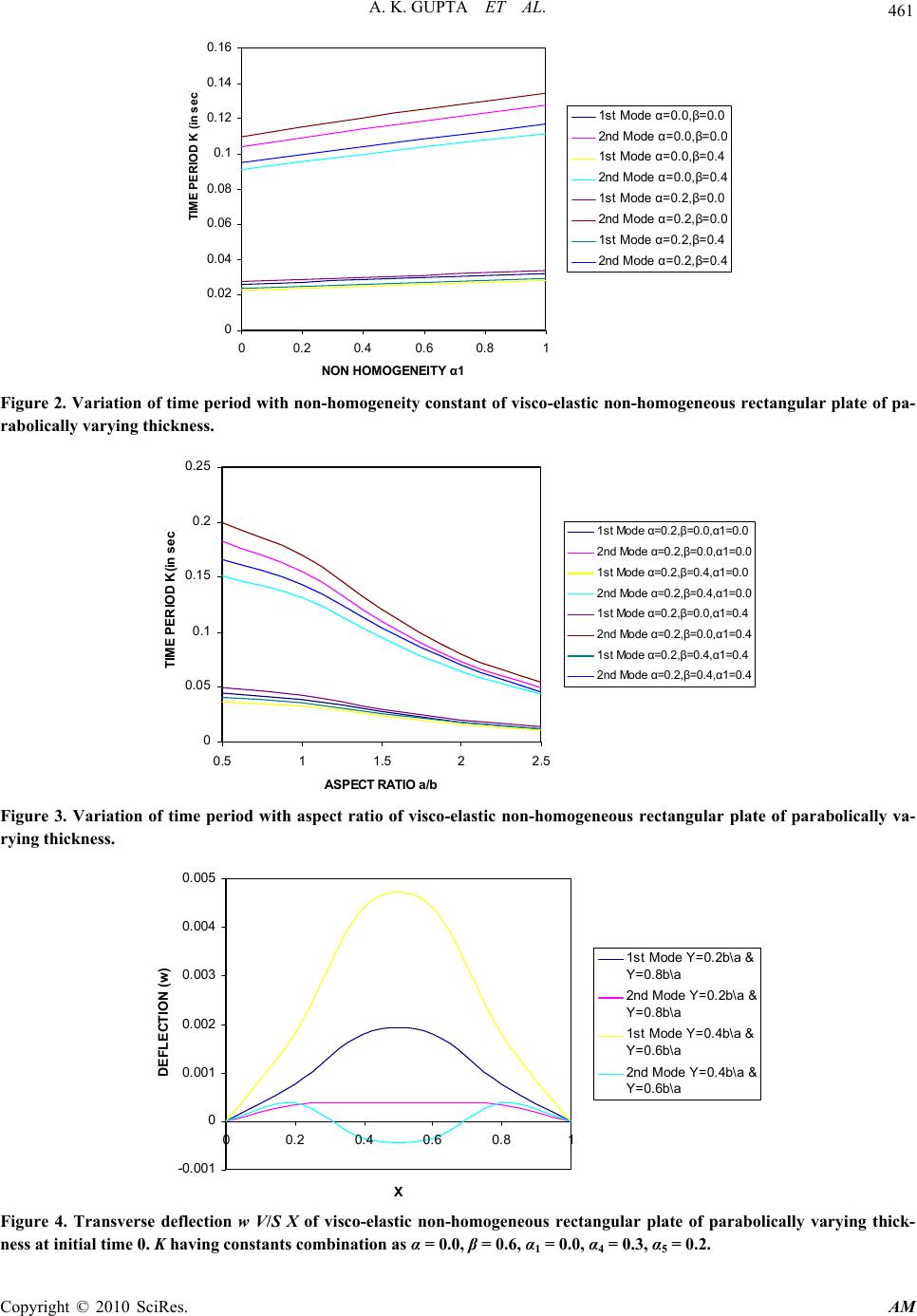

A. K. GUPTA ET AL.
461
0
0. 02
0. 04
0. 06
0. 08
0. 1
0. 12
0. 14
0. 16
00.20.40.6 0.81
NON HOMOGENEITY α1
TIME PERIOD K (in sec
)
1st Mode α=0.0,β=0.0
2nd Mode α=0.0,β=0.0
1st Mode α=0.0,β=0.4
2nd Mode α=0.0,β=0.4
1st Mode α=0.2,β=0.0
2nd Mode α=0.2,β=0.0
1st Mode α=0.2,β=0.4
2nd Mode α=0.2,β=0.4
Figure 2. Variation of time period with non-homogeneity constant of visco-elastic non-homogeneous rectangular plate of pa-
rabolically varying thickness.
0
0.05
0.1
0.15
0.2
0.25
0.511.522.5
ASPECT RATIO a/b
TIME PERIOD K(in sec
)
1st Mode α=0.2,β=0.0,α1=0.0
2nd Mode α=0.2,β=0.0,α1=0.0
1st Mode α=0.2,β=0.4,α1=0.0
2nd Mode α=0.2,β=0.4,α1=0.0
1st Mode α=0.2,β=0.0,α1=0.4
2nd Mode α=0.2,β=0.0,α1=0.4
1st Mode α=0.2,β=0.4,α1=0.4
2nd Mode α=0.2,β=0.4,α1=0.4
Figure 3. Variation of time period with aspect ratio of visco-elastic non-homogeneous rectangular plate of parabolically va-
rying thickness.
-0.0 01
0
0. 00 1
0. 00 2
0. 00 3
0. 00 4
0. 00 5
00.20.4 0.60.81
X
DEFLECTION (w)
1st Mode Y=0.2b\a &
Y=0.8b\a
2nd Mode Y=0.2b\a &
Y=0.8b\a
1st Mode Y=0.4b\a &
Y=0.6b\a
2nd Mode Y=0.4b\a &
Y=0.6b\a
Figure 4. Transverse deflection w V/S X of visco-elastic non-homogeneous rectangular plate of parabolically varying thick-
ness at initial time 0. K having constants combination as α = 0.0, β = 0.6, α1 = 0.0, α4 = 0.3, α5 = 0.2.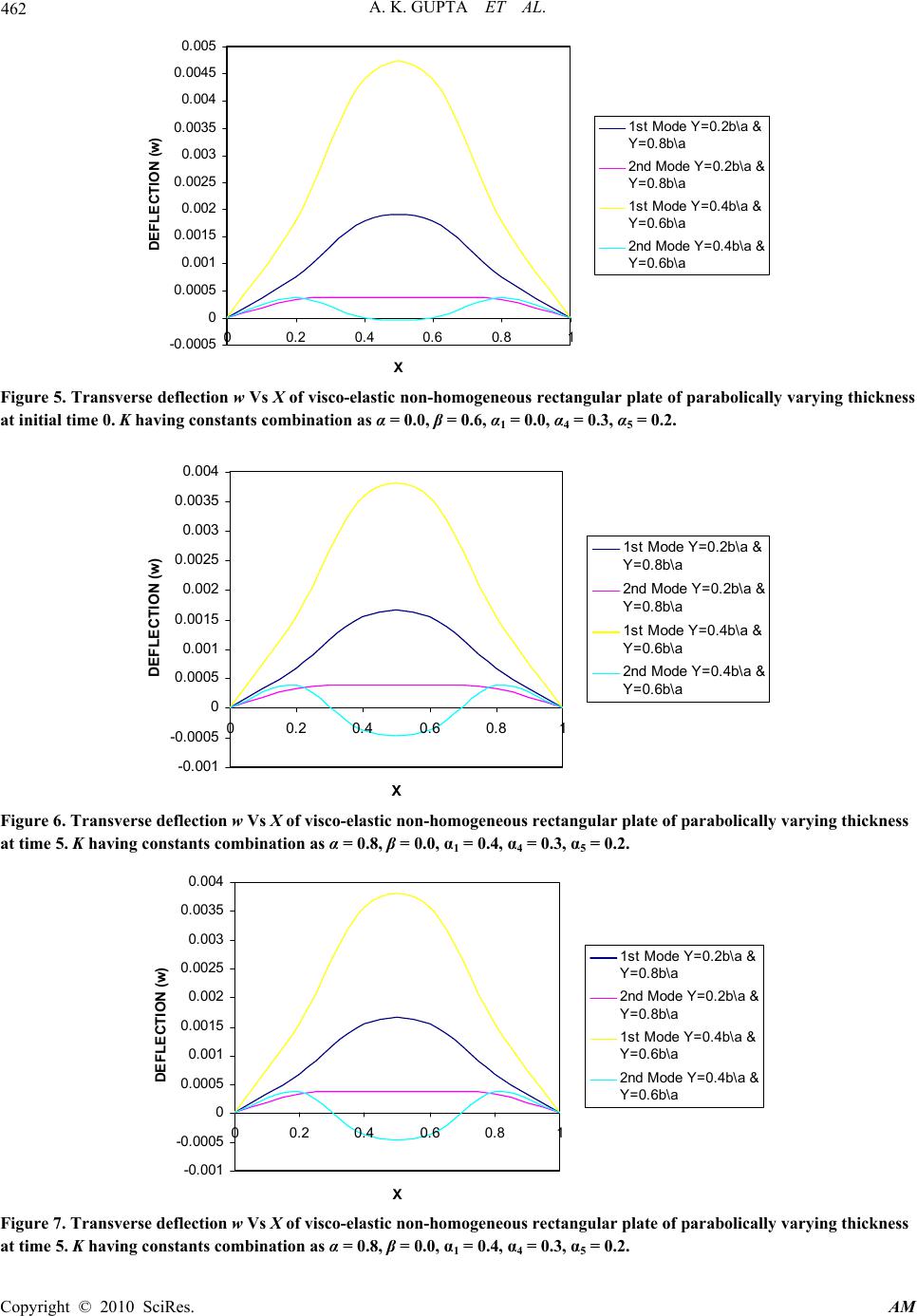

A. K. GUPTA ET AL.
462
-0.0005
0
0.0005
0.001
0.0015
0.002
0.0025
0.003
0.0035
0.004
0.0045
0.005
00.20.40.6 0.81
X
DEFLECTION (w)
1st Mode Y=0.2b\a &
Y=0.8b\a
2nd Mode Y=0.2b\a &
Y=0.8b\a
1st Mode Y=0.4b\a &
Y=0.6b\a
2nd Mode Y=0.4b\a &
Y=0.6b\a
Figure 5. Transverse deflection w Vs X of visco-elastic non-homogeneous rectangular plate of parabolically varying thickness
at initial time 0. K having constants combination as α = 0.0, β = 0.6, α1 = 0.0, α4 = 0.3, α5 = 0.2.
-0.001
-0.0005
0
0. 0005
0. 001
0. 0015
0. 002
0. 0025
0. 003
0. 0035
0. 004
00.20.40.6 0.81
X
DEFLECTION (w)
1st Mode Y=0.2b\a &
Y =0.8b\a
2nd Mode Y=0.2b\a &
Y =0.8b\a
1st Mode Y=0.4b\a &
Y =0.6b\a
2nd Mode Y=0.4b\a &
Y =0.6b\a
Figure 6. Transverse deflection w Vs X of visco-elastic non-homogeneous rectangular plate of parabolically varying thickness
at time 5. K having constants combination as α = 0.8, β = 0.0, α1 = 0.4, α4 = 0.3, α5 = 0.2.
-0.001
-0.0005
0
0.0005
0. 001
0.0015
0. 002
0.0025
0. 003
0.0035
0. 004
00.2 0.40.6 0.81
X
DEFLECTION (w)
1st Mode Y=0.2b\a &
Y=0.8b\a
2nd Mode Y=0.2b\a &
Y=0.8b\a
1st Mode Y=0.4b\a &
Y=0.6b\a
2nd Mode Y=0.4b\a &
Y=0.6b\a
Figure 7. Transverse deflection w Vs X of visco-elastic non-homogeneous rectangular plate of parabolically varying thickness
at time 5. K having constants combination as α = 0.8, β = 0.0, α1 = 0.4, α4 = 0.3, α5 = 0.2.

A. K. GUPTA ET AL. 463
7. References
[1] A. W. Leissa, NASA SP-60, Vibration of Plate, 1969.
[2] A. W. Leissa, “Recent Studies in Plate Vibration 1981-
1985 Part II, Complicating Effects,” Shock and Vibration
Dig., Vol. 19, No. 3, 1987, pp. 10-24.
[3] A. K. Gupta, T. Johri and R. P. Vats, “Thermal Effect on
Vibration of Non-Homogeneous Orthotropic Rectangular
Plate Having Bi-Directional Parabolically Varying Thick-
ness,” Proceeding of International Conference in World
Congress on Engineering and Computer Science, San-
Francisco, October 2007, pp. 784-787.
[4] Z. Sobotka, “Free Vibration of Visco-Elastic Orthotropic
Rectangular Plates,” Acta Technica, CSAV, Vol. 23, No.
6, 1978, pp. 678-705.
[5] J. S. Tomar and A. K. Gupta, “Effect of Thermal Gra-
dient on Frequencies of Orthotropic Rectangular Plate
Whose Thickness Varies in Two Directions,” Journal of
Sound and Vibration, Vol. 98, No. 2, January 1985, pp.
257-262.
[6] J. S. Tomar and A. K. Gupta, “Thermal Effect on Fre-
quencies of an Orthotropic Rectangular Plate of Linearly
Varying Thickness,” Journal of Sound and Vibration, Vol.
90, No. 3, October 1983, pp. 325-331.
[7] J. S. Tomar and A. K. Gupta, “Effect of Exponential
Temperature Variation on Frequencies of an Orthotropic
Rectangular Plate of Exponentially Varying Thickness,”
Proceeding of the Workshop on Computer Application in
Continum Mechanics, Roorkee, March 1986, pp. 183-
188.
[8] R. Lal, “Transverse Vibrations of Orthotropic Non-Uni-
form Rectangular Plate with Continuously Varying Den-
sity,” Indian Journal of Pure and Applied Mathematics,
Vol. 34, No. 4, 2003, pp. 587-606.
[9] A. K. Gupta and A. Khanna, “Vibration of Visco-Elastic
Rectangular Plate with Linearly Thickness Variations in
Both Directions,” Journal of Sound and Vibration, Vol.
301, No. 3-5, April 2007, pp. 450-457.
[10] A. K. Gupta and H. Kaur, “Study of the Effect of Ther-
mal Gradient on Free Vibration of Clamped Visco-Elastic
Rectangular Plate with Linearly Thickness Variation in
Both Directions,” Meccanica, Vol. 43, No. 4, 2008, pp.
449- 458.
[11] A. K. Gupta, A. Kumar and Y. K. Gupta, “Vibration of
Visco-Elastic Parallelogram Plate with Parabolic Thick-
ness Variation,” Applied Mathematics, Vol. 1, No. 2,
2010, pp. 128-136.
[12] A. K. Gupta and P. Singhal, “Effect of Non-Homogeneity
on Thermally Induced Vibration of Orthotropic Visco-
Elastic Rectangular Plate of Linearly Varying Thick-
ness,” Applied Mathematics, Vol. 1, No. 4, 2010, pp.
326-333.