J. Mod. Phys., 2010, 1, 281-289
doi:10.4236/jmp.2010.14039 Published Online October 2010 (http://www.SciRP.org/journal/jmp)
Study of Propagation of Ion Acoustic Waves in Argon
Plasma
N. S. Suryanarayana, Jagjeet Kaur, Vikas Dubey
Department of Physi cs , Govt. Vishwanath Yadav Tamaskar Post Graduate
Autonomous College, DURG (C.G), India
Received June 25, 2010; revised July 22, 2010; accepted August 19, 2010
Abstract
The properties of small amplitude acoustic waves (IAW) in unmagnetised plasma have been discussed in
detail. An experimental set up to study the propagation of IAW in argon plasma has been descried. The
speed of IAW under different conditions of discharge current and pressure has been measured from the
time-of flight technique. From these measurements, electron temperatures have been calculated. The results
have been compared with those obtained by single probe method, and were found to be in good agreement
with each other so IAW speeds can be used to calculate plasma parameters.
Keywords: IAW in Argon Plasma, Study of Propagation
1. Introduction
Plasma can support a great variety of wave motion. Both
high frequency (  pe) and low frequency (  pi)
electromagnetic and electrostatic waves may propagate
in plasma. The primary emphasis has been placed on the
study of electrostatic waves because the ease with which
such waves may be excited and detected and because the
collision less damping of waves predicted by Landau can
be conveniently studied [1-10].
Ion waves are low frequency pressure waves in plasma.
If the ion plasma frequency (pi) greatly exceeds the
wave frequency, both the electrons and ions oscillate
almost in phase. At this stage some of the characteristics
of the ion waves are similar to those of the ordinary
sound waves. So they are called Ion Acoustic Waves
(IAW).
The difference between ordinary Acoustic waves and
Ion acoustic waves arise from the electric field induced
by a slight charge separation. The electron component of
the ion acoustic wave tends to propagate faster than the
ion component. The electric field retards the electron
motion, forcing the two species to propagate together.
Ion Acoustic waves were first predicted theoretically
by Tonks & Langmuir [11]. They were first observed, in
gas discharge plasma by Rewans [12]. Landau damping
in plasmas was predicted by Fried & Gould [13] and
experimentally demonstrated in a high temperature ce-
sium plasma by wong et al. [14]. Ion Acoustic waves
were observed in cylindrical discharge tubes by many
workers [15-17] and in magnetically supported plasmas
by Alesef & Neidgh [18] dispersive effects at high fre-
quencies were studied by sessler [19] Tanaca et al. [20]
and Jones et al. [21]. Ion Acoustic so lution s were excited
and their propagation in plasma was studied by many
others [22-28]. Ion acoustic wave propagation in dusty
plasmas [29,33] was studied to find charge fluctuation
and a new damping mechanism. The possibility of the
turbulent development of an Ion Acoustic Wave that
yields particle fluxes as well as energy fluxes has been
used for characterization of the acoustic wave propaga-
tion in presheath region [30]. Dust acoustic solitary
waves [31] and nonplaner ion acoustic waves in plasma
[32] were studied by many others.
In the propagation of these ion acoustic waves (IAW),
depending on the details of the velocity distribution
function; en ergy may flow from the wave into the ions or
in the opposite direction. If the phase velocity of the
wave greatly exceeds the average thermal speed of the
ions, the interaction is weak and energy exchange is be-
tween resonant ions and the electric field of the wave. If
the phase velocity of the wave is comparable in magni-
tude the average ion speed, the interaction is strong and
occurs by phase mixing and also by resonant exchange.
The phase velocity of a small amplitude plane ion acous-
tic wave propagating along the field lines can be derived

N. S. SURYANARAYANA ET AL.
282
from the plasma fluid equations [34].

1/2
1/2
3
ee ii
si
ee i
i
kT kT
C
km
kT T
m
 

 



 


(1)
Where

the ratio of specific heats, k is the wave
number.
1.1. Wave Excitation
Electrostatic excitation of low frequency waves by elec-
trodes outside the plasma is extremely inefficient be-
cause currents on the plasma periphery shield the plasma
interior from the applied fields. Excitation by modulation
of magnetic field is possib le only if the confining field is
weak and only for long wave-lenghths [35]. Various other
techniques have also been reported [36,37 ]. In almost all
experiments on externally controlled ion waves the grids
are placed inside the plasma column with the plane of the
grid normal to the axis of the column. This technique
was introduced by Hatta et al. [38] and extensively used
by wong [39] and others. The transmission of the grid is
a function of the applied potential, greater negative po-
tentials decrease the transmission. If time varying poten-
tials are applied, it would lead to density variations. If  
pi, finite transit time of ion s through the sheaths around
the grid introduces velocity modulation [40]. Grid exci-
tation was accomplished at frequencies 0.1
p
i

  (7
to 130 KHz) for all conditions. Detection of ion acoustic
waves was done either by another biased grid or with a
Langmuir probe. Grid detection generally produces an
improved signal-to noise ratio.
1.2. Measuring Techniques
Phase and amplitude measurements were made by re-
ceiving probe along the plasma column. Phase velocity
has been inferred simply by measuring the time of flight
of the perturbation between two fixed grids. Capacitive
coupling between the grids often presents a pickup prob-
lem41, especially if the exciter frequency is > 100 KHz
and the grid separation is < 1 cm. Plasma noise is not a
problem in wave experiments but to detect extremely
faint signals the signal-to-noise ratio should greatly be
improved.
1.3. Wave Damping
The most significant contribution of the work of wong et
al and Alexeff et al., Y.Nakamura et al. [42] and others
[43] to the experimental stu dy of ion wav es was the care -
ful measurement of wave damping under conditions in
which collisional damping of ion waves could be com-
pletely neglected. The collisionless damping distance
was of the order of a few centimeters or much shorter
than the mean free path for ion-neutral charge exchange
collisions ( > 1 m).The ion drift between the grids cre-
ates a non-negligib le correction to the damping rate. It is
convenient to have the average damping constant. Then
the characteristics of the observed ion acoustic waves
appear to match closely the properties predicted for the
ion acoustic wave eigenmodes. Confirmation of these
results has b een reported by Sat o [ 44] and others [45,4 6].
1.4. Effect of Collisions on Ion Acoustic Waves
In the works of wong et al. [39] and Aleseff et al it was
shown that collisions played no role in the propagation
of ion acoustic waves. Collisions between ions and
electrons occur frequently

16
310
e
x
sec 
 but do
not affect the ion dynamics unless the electron ion en-
ergy equipartion time is comparable to 1


, which
does not occur unless 13 3 10ncm

 5
~10
s
ec 
. Heavy
particle collisions cannot be neglected. Collisions be-
tween ions do not necessarily increase the attenuation
of ion waves, in fact for ei TT ion collisions decrease
the damping rate because they reduce the resonant in-
teraction [47]. Accelerated ions and the background
ions would increase rather than decrease the wave
damping. The effect of ion-atom collisions on ion
waves was extensively studied by Anderson et al. [48].
The ion temperature could be deduced from the drop in
ion acoustic wave phase velocity.
1.5. Theoretical Considerations [49,50]
A dispersion relation is obtained using fluid approxima-
tions and then a useful handy formula for the velocity of
ion acoustic wave derived. Langmuir probe I-V charac-
teristic have been used to calculate the Te and ne (ni) and
hence pe, pi and D. Pe and pi are defined as Electron
plasma frequency
1/2
2
4e
pe
n
m








 (2)
Ion plasma frequency
1/2
2
4i
pi i
n
m








 (3)
Since Ion Acoustic Waves propagate only below the
ion plasma frequencypi ( << pi) both electrons and
ions move together. If Te >> Ti, the ion wave is not
strongly damped. Integrating equation of motion for

N. S. SURYANARAYANA ET AL.
283
electrons, we get the Boltzmann relation.
 
00
expexp 1
ee e
n nekTnekT   (4)
Ion acoustic perturbation follow
22 4
22
2222
0
ii i
sD
nn n
C
txxt

 
 

(5)
Where Cs = (Te/M) 1/2 and

1/2
4
De e
Tn   is the
ion acoustic speed and Debye length. By solving the
above equation the disp ersion relation is obtained
22
2
22
1s
D
Ck
k


 (6)
If k2 D2 << 1; phase velocity of IAW equals the cs and
is constant and if k2 D2  1 the wave becomes dispersive.
The handy f o rmul a for Cs is
 
1/2
3
ei ei
i
s
kT kTkT T
C
kmm



 


(7)
1.6. Single Langmuir Probe
A small electrode is inserted in to the plasma and the
current is measured as a function of the applied voltage.
The voltage is measur ed with respect to some convenient
reference point, which is often the cathode of the dis-
charge. The log I – V characteristic is shown in Figure 2.
The plasma potential (Vp) is obtained. Another potential,
which is shown as Vf, is the floating potential is also
obtained. Let us consider a perfectly reflecting probe. If
the electrons have a Maxwellian distribution the Boltz-
mann’s relation can be used


0exp ee
nneV kT (8)
Thus as we approach the probe, electron density de-
creases. Let us consider now an absorbing probe. When
the mean free path is large compared with the size of the
probe, the number hitting the absorbing probe is essen-
tially the same. Thus the electron s will mov e to the prob e
from regions much further away than the plasma bound-
ary without making a collision. But as the probe absorbs
the electrons the local electron density is depleted with
an absorbing probe the current density is given by
0exp
e
eV
II kT



(9)
Taking logarithm on both sides, the equation is

0
log loge
I
IeVkT (10)
Thus the slope of the plot of log I (Vs) V shown in
Figure 2 gives the electron temperature. Location of the
knee of the curve gives the plasma potential. The other
handy formulas used to calculate the plasma frequency
pe and Debye length De are
1/2
2
4e
pe
n
m









1/2
9000( )sec.
p
fn or
Where n = number of electrons per cm3.
1/2
2
4e
De
e
kT
n







 (11)

1/2
6.9
De Tn
  (12)
2. Experimental Setup
The experimental set up has been shown in Figure 1.
The dimensions of the vacuum chamber are 30 cm di-
ameter; 50 cm length of stainless steel. First it was
evacuated to a pressure of 2 × 10-5 torr. The experiment
was performed in the pressure range of 2 × 10-4 to 3 ×
10-3 torr. of Argon. Argon was flown continuously for
half an hour before the production of plasma. 10 fila-
ments of Tungsten (4 cm length and 0.05 cm diameter)
were symmetrically distributed and fixed to the two
Aluminium supporting rings. First they were heated
through supplying heater current ( 1.5 Amp. max. per
filament). The discharge was created by applying the
voltage between the heated filaments and wall of the
chamber. The discharge current was varied between 0.75
Amps. to 0.075 Amps. So the plasma was quiescent.
Plasma was spread into the chamber symmetrically. The
potential across the filament was 4 V. The discharge
voltage was around 60 volts.
Figure 1. Photograph of experimental setup.

N. S. SURYANARAYANA ET AL.
284
Figure 2. Log I–V Curve of langmuir probe.
A 1 cm diameter thin aluminium disk was used as the
Langmuir probe. The probe voltage was varied between
–70 V to + 25 V. The probe current has been measured
by a Keithly multimeter.
For the launching of Ion Acoustic waves a 30 KHz
pulse from the pulse generator was applied to the nega-
tively biased transmitting probe. The biasing can also be
floating. This probe was situated at the centre of the
plasma and normal to the axis of the chamber and it was
fixed in the cha mber through a feed through.
For collecting the ion acoustic waves, the Langmuir
probe was used. It was biased positive to collect the
electrons (electron current). The voltage developed at the
ends of 1 k resistance was given to the CRO Y1 termi-
nal, through a 100 PF capacitor. The pulse, directly from
pulse generator was fed to the Y2 of CRO. Both the
traces were locked through the adjustment of H. F. sync.
and level controls of CRO. The received voltage was in
the 20 mV to 50 mV range. While the transmitted volt-
age was < 1 V.
3. Observations & Results
Table 1 presents a comprehensive list of Te (electron
temperature) calculated through (1) Ion Acoustic wave
and (2) By single Langmuir probe for various discharge
current at various operating pressures.
1) a) Probe current was obtained at different Langmuir
probe voltages and discharge currents at three different
pressures.
b) A sample log I – V characteristic of Langmuir
probe has been shown in Figure 2.
c) The electron density/ion density, electron tempera-
tures, plasma frequency, plasma ion frequency, Debye
length were calculated from log I – V characteristics.
2) The traces of the transmitted wave and received ion
acoustic wave, appearing on the CRO screen, were care-
fully drawn by putting a transparent paper on the CRO
screen, at different probe separations, for varying dis-
charge currents and pressures. Time taken by ion acous-
tic wave to travel from transmitting grid to receiving grid
was obtained by the time of the flight techniqu es. Veloc-
ity of the IAW was calculated and tabulated .
3) Electron temperature was calculated for different
sets of readings.
4) The electron temperature obtained by Langmuir
probe method was compared with that obtained from the
time of – flight techniques.
4. Discussion and Conclusions
1) The calculation of electron temperature by IAW
propagation studies Figure 3 to Figure 8 and by Lang-
muir probe techniques Figure 1 indicate that the two
values are in fair agreement. However Te calculated from
Langmuir probe techniques is slightly more (10 to 15%)
than that calculated by IAW propagation speeds. Lang-
muir probe suffers from a number of defects such as-
a) Laboratory plasma deviates from some of the as-
sumptions made, while developing theory, such as
Maxwellian distribution of energy and velocity, isotropy
of plasma, homogeneity and quasinutrality of plasma.
b) Probe Size: It is assumed that the presence of probe
does not change the distribution and that the size of the
probe is negligible in comparison with reference elec-
trode. But finite probe size always as important role and
disturbs the distribution.
c) Sheath: In general a transition region called sheath
appearing around the probe, is assumed to be independ-
ent of probe potential. But at strong negative probe po-
tentials the central part of the sheath has increased elec-
tron density due to the presence of repelled electrons
from the probe and incoming electrons to the probe i.e.
the energy distribution near the probe may not be equal
to that existing in undisturbed plasma. Further, probe
current is also affected by secondary emission from
probe by the action of photons , ions and m etastable at oms.

N. S. SURYANARAYANA ET AL.
285
Table 1. A comprehensive list of Te (electron temperature) calculated by (1) Ion Acoustic wave and (2) By single Langmuir
probe for various discharge current at various operating pressures.
Gas used = Argon Temperature = 306 K
S.No. Pressure in torr Discharge
Current Amp
Velocity of IAW by
time–of–flight measurement
× 105 cm/sec.
Te obtained by
velocity of IAW
(eV)
Te obtained by
Langmuir probe
(eV) Ion temperature
1 2.5 × 10-4 0.5 2.96 3.65 4.3
2 6.5 × 10-5 0.5 2.34 2.27 -
3 1 × 10-3 0.5 2.14 1.90 2.1
4 6 ×10-4 0.35 2.6 2.84 3.5
5 2.5 × 10-3 0.35 1.95 1.59 -
6 1 × 10-3 0.35 2.03 1.72 -
7 1.5 × 10-4 0.35 2.78 3.23
8 3 × 10-4 0.11 1.82 1.38 1.5
9 7 × 10-4 0.11 1.67 1.16
10 2.5 × 10-3 0.11 1.54 0.99 -
11 3 × 10-3 0.075 1.43 0.85 0.8
12 3 × 10-3 0.2 1.46 0.89 -
13 3 × 10-4 0.2 2.27 2.15 -
14 2.5 × 10-3 0.7 2.83 3.34 -
15 6 × 10-4 0.75 2.84 3.37 -
16 2.5 × 10-4 0.75 2.84 3.37 4.0
 800 K
Figure 3. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.5 amp).

N. S. SURYANARAYANA ET AL.
286
Figure 4. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.25 amp).
Figure 5. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.11 amp).

N. S. SURYANARAYANA ET AL.
287
Figure 6. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.15 amp).
Figure 7. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.075 amp).
d) In the measurements: The probe potential as meas-
ured by an instrument may not represent the true poten-
tial difference between the electrodes and probe. There
may be contract potentials. A part of the voltage applied
to the probe is drop ped across the sheath and it is an un-
known quantity to the experiments. The space potential
Vs may vary due to the presence of fluctuations and drift
with in the bulk plasma and more intrinsically by the
driving probe current through the plasma without infinite
conductivity.
e) The equivalent plasma resistance, R, between probe
and reference electrode plays a significant role on the
determination of Te. R in the measuring circuit of the
probe depends on both the plasma conductivity and con-
figuration of the prob e.
f) Probe surface contamination also plays an important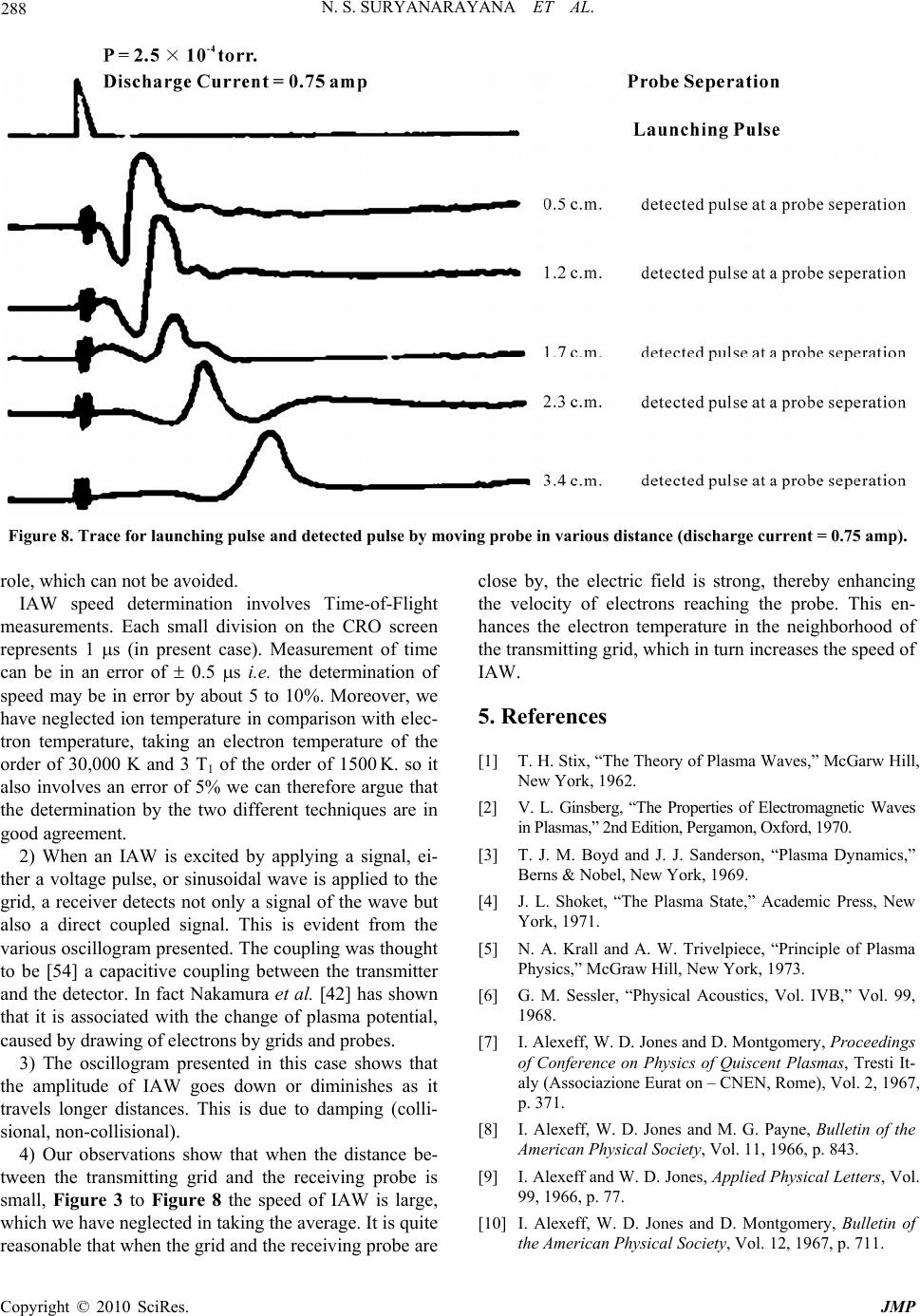

N. S. SURYANARAYANA ET AL.
288
Figure 8. Trace for launching pulse and detected pulse by moving probe in various distance (discharge current = 0.75 amp).
role, which can not be avoided.
IAW speed determination involves Time-of-Flight
measurements. Each small division on the CRO screen
represents 1 s (in present case). Measurement of time
can be in an error of  0.5 s i.e. the determination of
speed may be in error by about 5 to 10%. Moreover, we
have neglected ion temperature in comparison with elec-
tron temperature, taking an electron temperature of the
order of 30,000 K and 3 T1 of the order of 1500 K. so it
also involves an error of 5% we can therefore argue that
the determination by the two different techniques are in
good agreement.
2) When an IAW is excited by applying a signal, ei-
ther a voltage pulse, or sinusoidal wave is applied to the
grid, a receiver detects not only a signal of the wave but
also a direct coupled signal. This is evident from the
various oscillogram presen ted. The coupling was thought
to be [54] a capacitive coupling between the transmitter
and the detector. In fact Nakamura et al. [42] has shown
that it is associated with the change of plasma potential,
caused by drawing of electrons by grids and probes.
3) The oscillogram presented in this case shows that
the amplitude of IAW goes down or diminishes as it
travels longer distances. This is due to damping (colli-
sional, non-collisional).
4) Our observations show that when the distance be-
tween the transmitting grid and the receiving probe is
small, Figure 3 to Figure 8 the speed of IAW is large,
which we have neglected in taking th e averag e. It is quite
reasonable that when the grid and the receiving probe are
close by, the electric field is strong, thereby enhancing
the velocity of electrons reaching the probe. This en-
hances the electron temperature in the neighborhood of
the transmitting grid, wh ich in turn increases th e speed of
IAW.
5. References
[1] T. H. Stix, “The Theory of Plasma Waves,” McGarw Hill,
New York, 1962.
[2] V. L. Ginsberg, “The Properties of Electromagnetic Waves
in Plasmas,” 2nd Edition, Pergamon, Oxford, 1970.
[3] T. J. M. Boyd and J. J. Sanderson, “Plasma Dynamics,”
Berns & Nobel, New York, 1969.
[4] J. L. Shoket, “The Plasma State,” Academic Press, New
York, 1971.
[5] N. A. Krall and A. W. Trivelpiece, “Principle of Plasma
Physics,” McGraw Hill, New York, 1973.
[6] G. M. Sessler, “Physical Acoustics, Vol. IVB,” Vol. 99,
1968.
[7] I. Alexeff, W. D. Jones and D. Montgomery, Proceedings
of Conference on Physics of Quiscent Plasmas, Tresti It-
aly (Associazione Eurat on – CNEN, Rome), Vol. 2, 1967,
p. 371.
[8] I. Alexeff, W. D. Jones and M. G. Payne, Bulletin of the
American Physical Society, Vol. 11, 1966, p. 843.
[9] I. Alexeff and W. D. Jones, Applied Physical Letters, Vol.
99, 1966, p. 77.
[10] I. Alexeff, W. D. Jones and D. Montgomery, Bulletin of
the American Physical Society, Vol. 12, 1967, p. 711.

N. S. SURYANARAYANA ET AL.
289
[11] L. Tonks and I. Langmuir, Physical Review, Vol. 33,
1929, p. 195.
[12] R. W. Revans, Physical Review, Vol. 44, 1933, p. 798.
[13] B. D. Fried and R. W. Gould, Physics of Fluids, Vol. 4,
1961, p. 139.
[14] A. Y. Wong, R. W. Motley and N. D’Angelo, Physical
Review, Vol. 133, 1964, p. A436.
[15] Y. Hatta and N. Sato, Proceedings of 5th International
Conference on Ionization Phenomenon in Gases, H.
Maecker, Ed., North Holland Publishing Co., Amsterdam,
Vol. 1, 1962, p. 478.
[16] F. W. Crawford and S. A. Self, Proceedings of 6th Inter-
national Conference on Ionization Phenomenon in Gases,
P. Hubert and Cremeien Bureau des., Ed., Paris, Vol. 3,
1964, p. 129.
[17] P. J. Barrett and P. F. L ittle, Physical Re view Letter, Vol.
14, 1965, p. 365.
[18] I. Alexeff and R. V. Neidigh, Physical Review, Vol. 129,
1963, p. 516.
[19] G. M. Sessler, Physical Review Letters, Vol. 17, 1966, p.
243.
[20] H. Tanaca, M. Koganei and A. Hirose, Physical Review
Letters, Vol. 16, 1966, p. 1079.
[21] W. D. Jones and I. Alexeff, Proceedings of 7th Interna-
tional Conference on Ionization Phenomenon in Gases,
Gradevinskknija, Yugoslavia, Vol. 2, 1966, p. 330.
[22] H. Ikezi, R. J. Taylor and D. R. Baker, Physical Review
Letters, Vol. 25, 1970, p. 11.
[23] R. J. Taylor, K. R. Mackenzie and H. Ikezi, Review of
Scientific Instruments, Vol. 43, 1972, p. 1675.
[24] H. Ikezi, Physics of Fluids, Vol. 10, 1973, p. 1668.
[25] M. Q. Tran, Physica Scripta, Vol. 20, 1979, p. 317.
[26] Y. Nakamura and T. Ogino, Plasma Physics, Vol. 24,
1982, p. 1295.
[27] Y. Nakamura, IEEE Transactions on plasma science PS–
Vol. 10, 1982, p. 180.
[28] S. Watanabe, Journal of Plasma Physics, Vol. 14, 1975, p.
353.
[29] J.-J. Li, D.-L. Xiao, Y.-F. Li and J.-X. Ma, Chinese
Physical Letters, Vol. 24, 2007, p. 151.
[30] U. Deka, C. B. Dwivedi and H. ramchandran, Physica
Scripta, Vol. 73, 2006, p. 87.
[31] A. P. Mishra and A. R. Chowdhury, Physical Plasmas,
Vol. 13, 2006, pp. 62307-62314.
[32] A. P. Mishra and C. Bhowmik, Physical Letters A, Vol.
369, 2007, pp. 90-98.
[33] A. P. Mishra, H. Bailung and J. Chutia, Physical Plasmas,
Vol. 17, 2010, pp. 44502-44505.
[34] L. Landau , Journal of Physics, Vol. 10, 1946, p. 25.
[35] L. Spitzer, “The Physics of Fully Ionized Gases,” Wiley
Inter science, New York, 1962.
[36] P. F. Little, Proceedings of 5th International Conference
Ionization Phenomenon in Gases, North Holland Pub-
lishing Co., Amsterdam, 1962
[37] J. M. Buzzi, H. J. Doucet and W. D. Jones, Proceedings
of 3rd International Conference on Quiscent Plasmas
Elsinore, Gjellerup, Copenhagen, 1971.
[38] R. N. Franklin, G. J. Smith, S. M. Hamberger and G.
Lampis, Physical Letters A, Vol. 36, 1971, p. 473.
[39] H. Ikezi, N. takahashi and K. Nishikawa, Physics of Flu-
ids, Vol. 12, 1969, p. 853.
[40] A. Y. Wong and D. R. Baker, Physical Review, Vol. 188,
1969, p. 326.
[41] I. B. Bernstein, J. L. Hirschfield and J. H. Jacob, Physics
of Fluids, Vol. 14, 1971, p. 628.
[42] Y. Nakamura and Y. Nomura, Physical Letters A, Vol. 65,
1978, p. 415.
[43] Y. Nakamura, Y. Nomura and K. E. Lonngren, Research
Note, ISAS, RNO of Tokyo.
[44] L. Scott, Review of Scientific Instruments, Vol. 51, 1980,
p. 383.
[45] N. Sato, H. Ikezi, Y. Takanashi and Yamashita, Physical
Review, Vol. 183, 1969, p. 278.
[46] J. F. Wendt and A. B. Cambel, Proceedings of Interna-
tional Conference on Physics of Quiescents, Plasmas
Frascati, Lab Gas Ionization Rome, 1967.
[47] A. Y. Wong, Applied Physical Letters, Vol. 6, 1965, p.
147.
[48] R. W. Motley and A. Y. Wong, Proceedings of 6th Inter-
national Conference on Ionization Phenomena in Gases-
bureau des. Editions, France, 1963, Vol. 3, p. 133.
[49] H. K. Andersen, N. D. Angelo, V. O. Jensen, P. Michel-
sen and P. Nielsen, Physics of Fluids, Vol. 11, 1968, p.
1177.
[50] H. Ikezi, “Solitons in Action,” K. E. Lonngren and A.
Scott, Ed., Academic Press, New York, 1978, p. 153.
[51] F. F. Chen, “Introduction to Plasma Physics,” Plemun
Press, New York, 1974.
[52] J. E. Allan, Plasma Physics, B. E. Keen, Ed., Conference
Series, Institute of Physics, London, 1974, p. 131.
[53] J. D. Swift and M. J. R. Schwar, “Electrical Probs for
Plasma Diagnostics,” Iliffe, London, 1969.
[54] I. Alexeff, W. D. Jones, K. Lonngren and D. Montgom-
ery, Physics of Fluids, Vol. 12, 1969, p. 345.