Journal of Modern Physics, 2013, 4, 522-527
http://dx.doi.org/10.4236/jmp.2013.44074 Published Online April 2013 (http://www.scirp.org/journal/jmp)
Non Local Corrections to the Electronic Structure of
Non Ideal Electron Gases: The Case of
Graphene and Tyrosine
Yamila García1, John Cuffe1,2*, Francesc Alzina1, Clivia Marfa Sotomayor-Torres1,3,4
1Catalan Institute of Nanotechnology (CIN2-CSIC), Campus UAB, Bellaterra, Spain
2Department of Physics, Tyndall National Institute, University College Cork, Cork, Ireland
3Instituciò Catalana de Recerca i Estudis Avançats (ICREA), Barcelona, Spain
4Department of Physics, Universitat Autonoma de Barcelona, Bellaterra, Spain
Received January 4, 2013; revised February 6, 2013; accepted February 14, 2013
Copyright © 2013 Yamila García et al. This is an open access article distributed under the Creative Commons Attribution License,
which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
ABSTRACT
We introduce a formal definition of a non local functional and show that the non local exchange-correlation potential
functional, derived within Density-Functional Theory, is non local in the space of electronic densities. A previously
developed non local exchange-correlation potential term, is introduced to approach the exact density-functional poten-
tial. With this approach, the electronic structure of the graphene surface and the tyrosine amino acid are calculated.
Keywords: Nonlocality; Interfaces; Exchange-Correlation Potential; Graphene
1. Introduction
Functional architectures at the nanoscale are natural can-
didates to overcome the limitations encountered by the
conventional road map of micro technologies. The effi-
cient realization of nanoscale devices demands the deve-
lopment of theoretical methods with enough precision to
predict properties in a regime where physical interfaces
play a key role. However, it is precisely the description
of interfaces that is the main obstacle to rely in current
theoretical methods. This is due to limitations in model-
ing and numerically assess the required quantum defini-
tion of electronic states. On the one hand, reduced mod-
els suffer from the inability to include actual many body
potentials while retaining numerical efficiency. On the
other hand, ab-initio approaches, while exactly introduc-
ing many body interactions, suffer from the lack of effi-
ciency in terms of computational resources. Consensually
[1], an efficient, elegant, and exact alternative involves
ab-initio calculations by means of Density-Functional
Theory (DFT).
While in principle, DFT can yield exact electron-elec-
tron interactions, its effectiveness relies in a combination
of the Hohenberg-Kohn (HK) theorems [2] and the
Kohn-Sham (KS) scheme [3]. The HK theorems reduce
any property of the many-body interacting system to a
functional of the ground state electronic density and then,
the KS scheme replaces the original many-body problem
by an auxiliary independent-particle problem, whereby
all many-body interactions are mapped in an effective
single-particle potential, namely, the exchange-correla-
tion (xc) potential,
x
c

[4]. This xc potential, which
contains all the information regarding electron-electron
interactions, is implicitly defined as a functional deriva-
tive of the xc energy, Exc, in the space of electronic den-
sities,
 

xc
xc
Enr
vnr nr





 (1)
However, in most general cases, an algebraic form for


xc
Enr


 is unknown. Thus, the definition of



xc
nnr is by far the most important task for an ef-




fective DFT.
Initially, the manageability of DFT arises from the in-
troduction of the Local-Density Approximation (LDA) to
x
c

[3]. LDA, and further extensions beyond it within
local exchange models, such as generalized gradient ap-
proximations (GGA) [5] and Meta-GGA [6], work fairly
well for homogeneous and well-behaved electronic den-
sities [7]. While this is probably the case of bulk systems,
*Present address: Department of Mechanical Engineering, Massachu-
setts Institute of Technology, Cambridge, Massachusetts, 02139, USA.
C
opyright © 2013 SciRes. JMP

Y. GARCÍA ET AL. 523


U
it is no longer the expected behaviour for confined sys-
tems, such as nanostructures and interfaces, [8], therefore,
failures within DFT can be attributed to the inability of
local xc potentials, to retain all many body effects when
the many body interacting wave function representation
is mapped to a mean-field density-based representation
[9]. Recent attempts to overcome such difficulties and to
manage DFT are framed within the context of the con-
trolled addition of the non local Fock exchange to current
local xc potentials [10]. To some extent, such hybrid po-
tentials correct the electronic structure, even when such
improvements are not handled systematically [11,12].
Therefore, the purpose of this work is twofold. First,
we provide a means to understand the role that non local-
ity plays in DFT by introducing a formal definition of a
non local functional which, as far as we know, is not
presently available in the literature. The use of our defi-
nition provides a rigorous frame to assess the failure of
DFT when describing non homogeneous electron sys-
tems. Secondly, we use a method developed previously
by some of us to devise hybrid functionals in a system-
atic manner [13]. In this way, we analyze the role that
non locality plays in the electronic characterization of
two benchmark systems, graphene and tyrosine. The cho-
ice of these two systems responds to the emergence of
these materials as platforms for effective molecular elec-
tronics [14], and thus pay particular attention to graphene
interfaced with bio-systems [15,16].
2. Definition of Non Local Functional by Its
Opposite
A fundamental issue to address when dealing with a
mean field theory is the definition of effective potentials.
DFT is a mean field theory within which the selection of
the effective
x
c

potential is controversial [9,17], with
continuous efforts towards improvement in accuracy [11,
12,18-20]. It is our point of view that all such efforts suf-
fer from the lack of a rigorous and explicit definition of
one of the analytic properties that a
x
c

functional must
fulfill, namely the non locality in the position space [9].
Thus, in this section we introduce a definition for a non
local functional. This definition should be sufficient to
connect DFT with other effective theories and gain in-
sights in the physical implications that the inclusion of
non local effects has on mean field theories.
Two complementary definitions are given for a local
functional as follows:
1) A local functional from F to :

n
CR

A functional


n
:
F
CR 
n
UR
 is local if for any
open set and fields

and
F

, so that




UU UU
 

  (2)
This means that

is determined by the values
of

in an arbitrarily set . U
:
2) A local functional from F to R:
F
R A functional

 is local if it is given by the
integral,





d
n
R
x
x   

:n
(3)
of a local functional
F
CR 

 (4)
This means that the functionals which do not fulfill
either of these two conditions in the fields where they are
defined are called non local functionals. These two defi-
nitions should contribute to elucidate controversies con-
cerning the inclusion of non local effects in current ex-
change-correlation potentials.
3. Non Locality in the Exchange-Correlation
Potential
In the fundamentals of DFT by Hohenberg and Kohn [2],
the analytical expression for the xc energy functional is
determined for two particular cases, the electron gas with
almost constant density and the electron gas with slowly
varying density. By using the first of these two cases, we
will show the non local character of the most general
exchange-correlation functional.
The gas with almost constant density is defined in ac-
cordance with the conditions that follow,


0
nrn nr  (5a)
with


0
1
nr
n

 (5b)
and


d0nr r
 (5c)

where

nr is the electronic density as a function of the
position. HK proposed a formal expansion for Exc pro-
vided such conditions above are met [2]. It is written as,







0dd
,, ddd
xc xc
EnEnKrrnrnr rr
Lrr rnrnrnrrrr


 




 
(6)

In order to capture the essential physics of the problem
in question, and to avoid tedious mathematical treatments,
we reduce all the expressions above to their one dimen-
sional (1D) form. Then, by doing the functional deriva-
tive we obtain an enlightening expression for the xc po-
tential. It is then written as,





d
,, dd
xc
vnx Kxxnxx
Lxxxnxnxx x

 

 





 
(7)

Y. GARCÍA ET AL. 524
Noticeably, the spatial integrals of the electronic den-
sity products are, by themselves, non local mathematical
operations in the space in which they are defined, see
definition of non locality above. Thus, for the most gen-
eral case, this analytical expression for
x
c

is non local
in the x-space provided that

ñ
x
is different from
zero.
4. Non Local xc Potentials for Interfaces
The choice of the xc functional describing the most gen-
eral physical system must fulfill the following conditions:
1) it has to be non local in the space of electronic densi-
ties [9]; 2) it should correct self-interaction errors in ex-
change functionals [10]; 3) it should contain a fraction of
the Fock potential to approach exact exchange [13]; and
4) it must agree with the adiabatic connection theorem
[21]. With these conditions in mind, and as an improve-
ment to the first hybrid approaches introduced by Becke
[22], we propose the following analytical equation to
evaluate numerically
x
c

,
 
Fock 1
xc x
vv  

local local
x c
vv   
Fock
v
local
(8)
Here x is the Fock-like potential contribution,
which accounts for non local effects, the ,
x
c contribu-
tions are given by any of the available exchange (x) or
correlation (c) local functionals. Finally, α is a single
fitting parameter to be determined by exact rules.
v

HOMO LUMO
To estimate α, we follow an exact methodology within
mean-field Green’s function schemes [23,24]. The value
is chosen so that HOMO-LUMO matches the charge gap,
GAP. This quantities are defined as
HOMO-LUMO

  (9)
and



0
11E N
00
2GAPE NE N (10)
respectively, where N represents the number of electrons,
E0 is the total energy for the selected ground states in the
Kohn-Sham system of independent electrons with single
particle energies denoted as HOMO

and LUMO

, for the
Highest Occupied Molecular Orbital (HOMO) and Low-
est Unoccupied Molecular Orbital (LUMO) respectively
[4]. The choice of the finite region in which to search for
α is constrained by two conditions: 1) it must contain the
zone which confines the electron and 2) the HOMO and
LUMO orbitals must be well localized in to the cluster
model.
Finally, we should remark that from the reasoning pre-
sented here we could not derive an explicit mathematical
relation between the non local corrections to
x
c

re-
quired by seminal DFT [2], and our adjustable hybrid
approach defined by Equation (8). However, we note that
both xc potentials are non local functionals of the elec-
tronic density. It is this common property that justifies an
algebraic connection between both equations. Moreover,
the fundamental physical relation between both correc-
tions is now clear: in both cases non local corrections are
included beside the causal effects, when non ideal elec-
tron gases are described in terms of the electronic den-
sity.
5. Numerical Results and Discussion
In this section the influence of the gradual inclusions of
non local effects in
x
c

upon the electronic structure of
two confined systems, tyrosine and graphene, is discussed.
To do this, we analyze the evolution of the O-LUMO ,
when the non local Fock contribution to HOM



xc

 varies
[see Equation (8)].
The code GAUSSIAN09 is used for numerical DFT
calculations [25]. The local DFT functional introduced to
define
x
c

in the Equation (8) is PW91 [26], which is
used either to account for local exchange and local cor-
relation effects.
In regard to the other two inputs required to manage
DFT approaches, i.e., 1) finite cluster models definition
and 2) the selection of basis sets, the following reasoning
was followed. First, the atomic structure of the amino
acid reported in the pdb data base is selected [27], and a
finite cluster model for the ideal semi-infinite graphene is
chosen. To this end, we calculate the position of the
ionization potential 00

1
I
PEN EN when the
size of graphene cluster models is increased [see Figure
1].
Graphene is known to have an IP close to 5 eV [28].
Thus, this reference value was used to estimate the level
of certainty of our finite model approach to the semi-
infinite graphene system [29]. Using these results, see
Figure 1, we select a 10 × 10 unit cells cluster to model
graphene1. Second, the basis sets are selected such that
they are 3 - 21 g for all the atoms in the amino acid and
graphene systems. Higher order basis sets were in- tro-
duced to prove the robustness of our results for all the
calculations in this work2. The convergence criterium
was set to the 10 percent for the single particle energy le-
vels as well as for ground state energies calculated with
fully local
x
c potentials.

Figures 2 and 3 show HOMO-LUMO

and GAP energies
calculated for graphene and tyrosine respectively, using a
1Bigger cluster sizes will certainly increase the accuracy of the calcula-
tions but will also increase the computational time by orders of magni-
tude. e.g. Using 3 - 21 g basis sets [25], and going from a cluster with 1
carbon atom [0 unit cells] to clusters with 240 carbon atoms [10 × 10
unit cells], the time consumption is increased by 3 orders of magnitude.
In addition, improvements to the accuracy of self-consistent energies do
not affect the main message of this work.
2The electronic structure has been optimized using localized-atomic-
orbitals-
b
asis sets for all the atoms in the structure. The basis selected
where sto-3 g, 3 - 21 g, 6 - 31 g and cc-pvtz.
http://www.emsl.pnl.gov/forms/basisform.html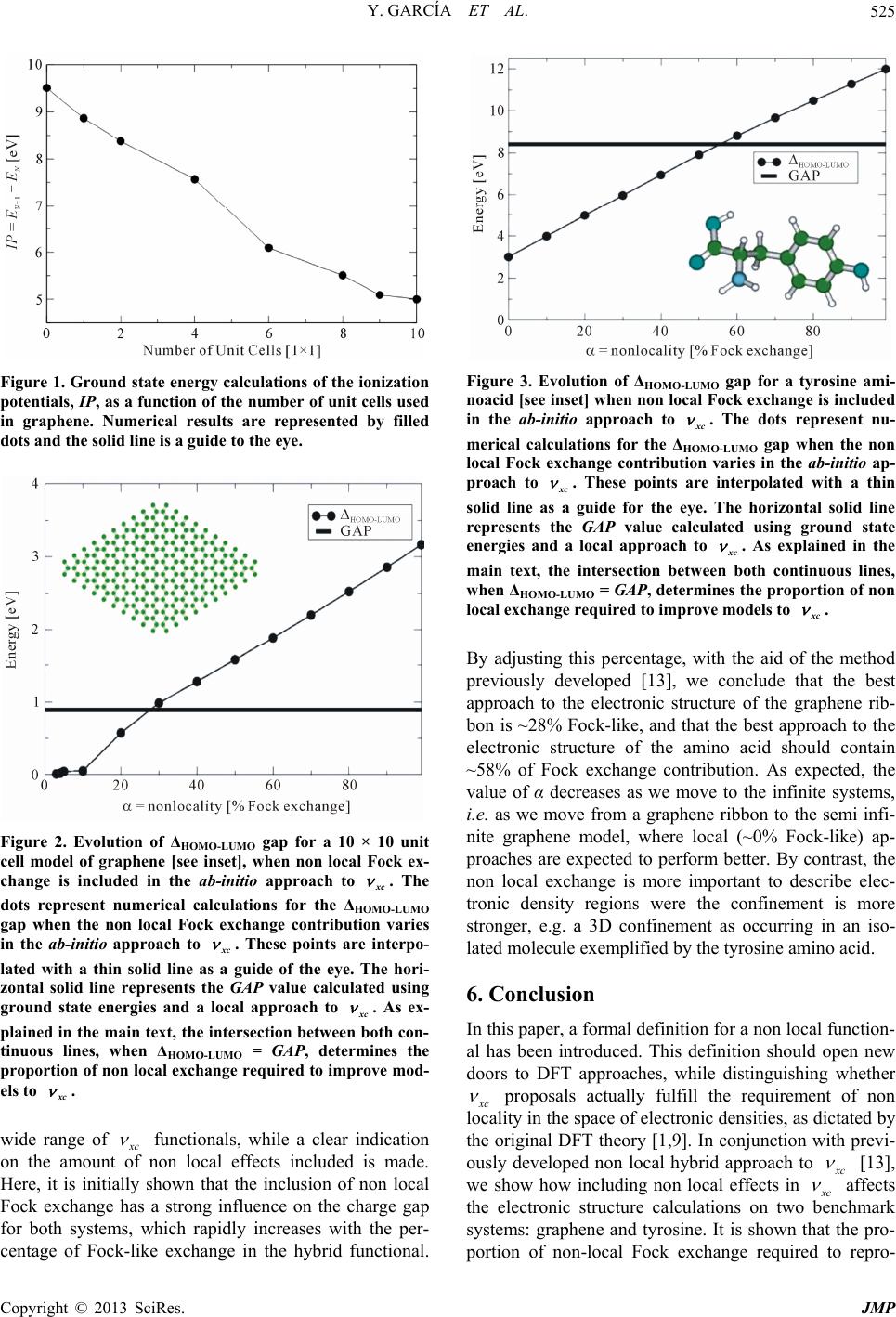

Y. GARCÍA ET AL. 525
Figure 1. Ground state energy calculations of the ionization
potentials, IP, as a function of the number of unit cells used
in graphene. Numerical results are represented by filled
dots and the solid line is a guide to the eye.
Figure 2. Evolution of ΔHOMO-LUMO gap for a 10 × 10 unit
cell model of graphene [see inset], when non local Fock ex-
change is included in the ab-initio approach to xc

. The
dots represent numerical calculations for the ΔHOMO-LUMO
gap when the non local Fock exchange contribution varies
in the ab-initio approach to xc

. These points are interpo-
lated with a thin solid line as a guide of the eye. The hori-
zontal solid line represents the GAP value calculated using
ground state energies and a local approach to xc

. As ex-
plained in the main text, the intersection between both con-
tinuous lines, when ΔHOMO-LUMO = GAP, determines the
proportion of non local exchange required to improve mod-
els to xc

.
wide range of
x
c
Figure 3. Evolution of ΔHOMO-LUMO gap for a tyrosine ami-
noacid [see inset] when non local Fock exchange is included
in the ab-initio approach to xc

. The dots represent nu-
merical calculations for the ΔHOMO-LUMO gap when the non
local Fock exchange contribution varies in the ab-initio ap-
proach to xc

. These points are interpolated with a thin
solid line as a guide for the eye. The horizontal solid line
represents the GAP value calculated using ground state
energies and a local approach to xc

. As explained in the
main text, the intersection between both continuous lines,
when ΔHOMO-LUMO = GAP, determines the proportion of non
local exchange required to improve models to xc

.
By adjusting this percentage, with the aid of the method
previously developed [13], we conclude that the best
approach to the electronic structure of the graphene rib-
bon is ~28% Fock-like, and that the best approach to the
electronic structure of the amino acid should contain
~58% of Fock exchange contribution. As expected, the
value of α decreases as we move to the infinite systems,
i.e. as we move from a graphene ribbon to the semi infi-
nite graphene model, where local (~0% Fock-like) ap-
proaches are expected to perform better. By contrast, the
non local exchange is more important to describe elec-
tronic density regions were the confinement is more
stronger, e.g. a 3D confinement as occurring in an iso-
lated molecule exemplified by the tyrosine amino acid.
6. Conclusion
In this paper, a formal definition for a non local function-
al has been introduced. This definition should open new
doors to DFT approaches, while distinguishing whether
x
c

proposals actually fulfill the requirement of non
locality in the space of electronic densities, as dictated by
the original DFT theory [1,9]. In conjunction with previ-
ously developed non local hybrid approach to
x
c

functionals, while a clear indication
on the amount of non local effects included is made.
Here, it is initially shown that the inclusion of non local
Fock exchange has a strong influence on the charge gap
for both systems, which rapidly increases with the per-
centage of Fock-like exchange in the hybrid functional.

[13],
we show how including non local effects in
x
c

affects
the electronic structure calculations on two benchmark
systems: graphene and tyrosine. It is shown that the pro-
portion of non-local Fock exchange required to repro-

Y. GARCÍA ET AL. 526
duce correctly the gap energy increases with increasing
the degree of confinement in the system. The discrepan-
cies between our non local corrected calculations and the
local calculation results, suggest that care should be
taken when forthcoming local density-functional appro-
aches in favour of those results obtained by means of
hybrid density-functional approaches. However, in light
of the results discussed here, it is still difficult to assess
to what extent current hybrids
x
c

potentials can de-
scribe the electronic states in a more general frame of
non ideal electron gases, e.g. nanostructures and inter-
faces, where the amount of non local corrections is ex-
pected to vary. Therefore, further developments of hybrid
functionals should also include the spatial dependence of
non local contributions while exactly approaching the xc
potentials.
7. Acknowledgements
We are indebted with E. Hawkins (University of York)
for his contribution on defining non local functionals as
well as helpful discussions on the mathematical issues
here addressed. We used the computational facilities
from “Centro de Supercomputación de Galicia” (CESGA).
We aknowledge financial support from the MICINN-
Spain (ACPHIN FIS2009-10150 and NANOTHERM
CSD2010-00044), and EU grants (NAPANIL 214249,
NANOPACK 216176, NANOPOWER FP7-ICT-2009-5
256959), and MINECO-Spain (TAPHOR: MAT2012-
31392).
REFERENCES
[1] W. Kohn, “An Essay on Condensed Matter Physics in the
Twentieth Century,” Reviews of Modern Physics, Vol. 71,
No. 2, 1999, pp. S59-S77.
doi:10.1103/RevModPhys.71.S59
[2] P. Hohenberg and W. Kohn, “Inhomogeneous Electron
Gas,” Physical Reviews, Vol. 136, No. 3B, 1964, pp. B864-
B871. doi:10.1103/PhysRev.136.B864
[3] W. Kohn and L. J. Sham, “Self-Consistent Equations In-
cludeing Exchange and Correlation Effects,” Physical
Review, Vol. 140, No. 4A, 1965, pp. A1133-A1138.
doi:10.1103/PhysRev.140.A1133
[4] F. M. Bickelhaupt, “Understanding Reactivity with Kohn-
Sham MO Theory. The E2-SN2 Mechanistic Spectrum
and Other Concepts,” Journal of Computational Chemis-
try, Vol. 20, No. 1, 1999, pp. 114-128.
doi:10.1002/(SICI)1096-987X(19990115)20:1<114::AID
-JCC12>3.0.CO;2-L
[5] J. P. Perdew, J. A. Chevary, S. H. Vosko, K. A. Jackson,
M. R. Pederson, D. J. Singh and C. Fiolhais, “Atoms,
Molecules, Solids, and Surfaces: Applications of the Gen-
eralized Gradient Approximation for Exchange and Cor-
relation,” Physical Review B, Vol. 46, No. 11, 1992, pp.
6671-6687. doi:10.1103/PhysRevB.46.6671
[6] V. N. Staroverov, G. E. Scuseria, J. Tao and J. P. Perdew,
“Comparative Assessment of a New Nonempirical Den-
sity Functional: Molecules and Hydrogen-Bonded Com-
plexes,” Journal of Chemical Physics, Vol. 119, No. 23,
2003, pp. 12129-12137. doi:10.1063/1.1626543
[7] R. W. Godby, M. Shluter and L. J. Sham, “Self-Energy
Operators and Exchange-Correlation Potentials in Semi-
conductors,” Physical Review B, Vol. 37, No. 17, 1988,
pp. 10159-10175. doi:10.1103/PhysRevB.37.10159
[8] N. Sai, M. Zwolak, G. Vignale and M. Di Ventra, “Dyna-
mical Corrections to the DFT-LDA Electron Conductance
in Nanoscale Systems,” Physical Review Letters, Vol. 94,
No. 18, 2005, Article ID: 186810.
doi:10.1103/PhysRevLett.94.186810
[9] R. K. Nesbet, “Beyond Density Functional Theory: The
Domestication of Nonlocal Potentials,” Modern Physics
Letters B, Vol. 18, No. 2-3, 2004, pp. 73-82.
doi:10.1142/S021798490400669X
[10] J. Jaramillo, G. E. Scuseria and M. Ernzerhof, “Local
Hybrid Functionals Based on Density Matrix Products,”
Journal of Chemical Physics, Vol. 118, No. 3, 2003, pp.
1068-1073. doi:10.1063/1.1528936
[11] A. D. Becke, “A New Mixing of Hartree-Fock and Local
Density-Functional Theories,” Journal of Chemical Phys-
ics, Vol. 98, No. 2, 1993, pp. 1372-1377.
doi:10.1063/1.464304
[12] T. Yanai, D. P. Tew and N. C. Handy, “A New Hybrid
Exchange-Correlation Functional Using the Coulomb-
Attenuating Method (CAM-B3LYP),” Chemical Physics
Letters, Vol. 393, No. 1-3, 2004, pp. 51-57.
doi:10.1016/j.cplett.2004.06.011
[13] Y. García and J. C. Sancho-García, “On the Role of the
Nonlocal Hartree-Fock Exchange in Ab-Initio Quantum
Transport: H2 in Pt Nanocontacts Revisited,” Journal of
Chemical Physics, Vol. 129, No. 3, 2008, Article ID:
034702.
[14] A. Nitzan and M. A. Ratner, “Electron Transport in Mo-
lecular Wire Junctions,” Science, Vol. 300, No. 5624,
2003, pp. 1384-1389. doi:10.1126/science.1081572
[15] N. Mohanty and V. Berry, “Graphene-Based Single- Bac-
terium Resolution Biodevice and DNA Transistor: Inter-
facing Graphene Derivatives with Nanoscale and Micro-
scale Biocomponents,” Nano Letters, Vol. 8, No. 12, 2008,
pp. 4469-4476. doi:10.1021/nl802412n
[16] C. Lu, H. Yang, C. Zhu, X. Chen and G. Chen, “A Gra-
phene Platform for Sensing Biomolecules,” Angewandte
Chemie International Edition, Vol. 48, No. 26, 2009, pp.
4785-4787. doi:10.1002/anie.200901479
[17] A. W. Ghosh and S. Datta, “Molecular Conduction: Para-
digms and Possibilities,” Journal of Computational Elec-
tronics, Vol. 1, No. 4, 2002, pp. 515-525.
doi:10.1023/A:1022961608941
[18] J. P. Perdew and A. Zunger, “Self-Interaction Correction
to Density-Functional Approximations for Many-Electron
Systems,” Physical Review B, Vol. 23, No. 10, 1981, pp.
5048-5079. doi:10.1103/PhysRevB.23.5048
[19] J. Da-Chai and M. Head-Gordon, “Long-Range Corrected
Hybrid Density Functionals with Damped Atom-Atom

Y. GARCÍA ET AL. 527
Dispersion Corrections,” Physical Chemistry Chemical
Physics, Vol. 10, No. 44, 2008, pp. 6615-6620.
doi:10.1039/b810189b
[20] G. Vignale and W. Kohn, “Current-Dependent Exchange-
Correlation Potential for Dynamical Linear Response
Theory,” Physical Review Letters, Vol. 77, No. 10, 1996,
pp. 2037-2040. doi:10.1103/PhysRevLett.77.2037
[21] C. Adamo and V. Barone, “Towards Reliable Adiabatic
Connection Models Free from Adjustable Parameters,”
Chemical Physics Letters, Vol. 274, No. 1-3, 1997, pp.
242-250. doi:10.1016/S0009-2614(97)00651-9
[22] A. D. Becke, “Density-Functional Thermochemistry. III.
The Role of Exact Exchange,” Journal of Chemical Phys-
ics, Vol. 98, No. 7, 1993, pp. 5648-5651.
doi:10.1063/1.464913
[23] F. M. Bickelhaupt and E. J. Baerends, “Kohn-Sham Den-
sity Functional Theory: Predicting and Understanding,”
Reviews of Computational Chemistry, Vol. 15, 2000, pp.
1-86. doi:10.1002/9780470125922.ch1
[24] W. Schattke, M. A. Van Hove, F. J. G. de Abajo, R. Díez
Muiño and N. Mannella, “Solid-State Photoemission and
Related Methods: Theory and Experiment,” Wiley-VCH,
Berlin, 2003. doi:10.1002/9783527602506
[25] M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria,
M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B.
Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X.
Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng,
J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fu-
kuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O.
Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr., J. E.
Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers,
K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Nor-
mand, K. Raghavachari, A. Rendell, J. C. Burant, S. S.
Iyengar, J. Tomasi, M. Cossi, N. Rega, J. M. Millam, M.
Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J.
Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J.
Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L.
Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P.
Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, A.
Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J.
Fox, “Gaussian 09, Revision A.1,” Gaussian, Inc., Wal-
lingford, 2009.
[26] J. P. Perdew and Y. Wang, “Accurate and Simple Density
Functional for the Electronic Exchange Energy: General-
ized Gradient Approximation,” Physical Review B, Vol.
33, No. 12, 1986, pp. 8800-8802.
doi:10.1103/PhysRevB.33.8800
[27] Protein data bank. www.pdb.org
[28] Y. Yu, Y. Zhao, S. Ryu, L. E. Brus, K. S. Kim and P.
Kim, “Tuning the Graphene Work Function by Electric
Field Effect,” Nano Letters, Vol. 9, No. 10, 2009, pp.
3430-3434. doi:10.1021/nl901572a
[29] F. J. Owens, “Electronic and Magnetic Properties of Arm-
chair and Zigzag Graphene Nanoribbons,” Journal of
Physical Chemistry, Vol. 128, No. 19, 2008, pp. 194701-
194704. doi:10.1063/1.2905215