Creative Education, 2010, 2, 121-127
doi:10.4236/ce.2010.12018 Published Online September 2010 (http://www.SciRP.org/journal/ce)
121
Investigating Numeric Relationships Using an
Interactive Tool: Covering Number Sense
Concepts for the Middle Grades
Hui Fang Huang “Angie” Su1, Carol A. Marinas2, Joseph M. Furner3
1Nova Southeastern University, North Miami Beach, Florida, USA; 2Barry University, Miami Shores, USA; 3Florida Atlantic
University, Jupiter, USA.
Received July 1st, 2010; revised August 24th, 2010; accepted August 30th, 2010.
ABSTRACT
This article investigates intriguing number patterns utilizing an emerging technology called the Square Tool. Middle
School math tea chers will find th e Square Tool useful in making connections and bridging the gap from the co ncrete to
the abstract. Pattern recognition helps students discover mathematical concepts. With the development of puzzles,
games, and computer programs, students learn and practice the skills that are created in the mathematics classrooms.
By studying patterns that exist within different number arrangements, consistencies are observed among them. Middle
School students will investig ate various mathematics rela tionships found in numb ered squares including multip les, fac-
tors, ratios of comparative numbers, and other mathematical concepts.
Keywords: Number Sense, Number Patterns and relationships, Puzzles, Games, Computer Programs, Number Arrangements,
Multiples, Factors, Ratios of Comparative Numbers, Investigation, Number Squares, Number Games
1. What is the Square Tool?
The Square Tool (see Appendix) is an emerging Java-
Script technology that allows users to discover many
numeric relationships. It is based on the idea of arrays of
consecutive numbers such that the program can config-
ure any N × N area up to 20 × 20, like the Hundreds
Chart. A simple hundreds chart would be a 10 × 10 area
listing the numbers 1 to 100.
In Figure 1, the interactive Square Tool creates
squares of consecutive numbers (Su, Marinas, & Chraibi,
2008) [1] http://mcs-research.barry.edu/squares/squares
99 html. In the case of a 9 × 9 square, the sum of either
major diagonal is 369. While the Square Tool explores
patterns, the tool has the ability to add numbers and in-
teractively change square sizes.
2. Investigating Patterns and Numeric
Relationships
Using the Square Tool, teachers will discover many pos-
sibilities for its use in which to educate children in in-
vestigating patterns and numeric relationships. It can
help students improve recognition of similarities promptly
and correctly, apply patterns to different subjects and
venues, and form the fundamental concept of patterns,
such as counting by tens or recognizing sums of numbers.
The National Council of Teachers of Mathematics
(NCTM) Standards (2000) [2] document has made one
of its principles for teaching mathematics the use of
technology during math instruction. The Square Tool can
be used at any grade level and employs technology as stu-
dents discover intriguing number patterns.
Figure 1. A 9 × 9 Square showing the sum of either major
diagonal is 369.

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades
122
Furner (2005) [3] and Ozel, Yetkiner, and Capraro (2008)
[4] discuss the importance of using technology in the
classroom and creating connections by bridging the gap
from the concrete to the abstract using such promising
technologies. The Square Tool helps students explore
many fascinating number patterns and concepts. Students
can create and extend number models and patterns in a
progressively deeper and more meaningful manner. They
can create a variety of visually interesting patterns that
express fundamental mathematical ideas in today’s math
curriculum.
At the early grade levels, students should transition
from hands-on activities to semi-concrete representations
like virtual manipulative, to finally abstract numerical
concepts. “It is like magic, I’ve made a 5 × 5 grid and
clicked every other number and the red numbers are all
even and the other numbers are all odd,” said Jose in the
4th grade. The Square Tool adds distinct numbers and
examines patterns to advance higher-level thinking skills.
It can also be used to perform operations and show nu-
meric relationships including the basic operations of
addition, multiplication, and division. (Ta ble 1)
2.1. Properties of Numbers
Since number theory is the study of the properties of
numbers, the Square Tool is a practical instrument for
discovering prime and composite numbers (Table 2), the
Sieve of Eratosthenes, multiples, and factors. Mathema-
ticians such as Euler, Gauss, Fermat, Euclid, and Py-
thagoras were fascinated by number patterns and pro-
vided important contributions to number theory. Wall
(2010) [5] feels that number theory ideas should be
introduced in the elementary grades as a foundation that
leads to more abstract reasoning in the middle grades.
Overbay and Brod (2007) [6] contend that exploring
magic squares and recognizing number patterns with
middle school students is motivating and fascinating for
this age group. This lays an important foundation for
work with number theory concepts at higher levels.
While the Father of Primes and Composites Explora-
tion was claimed to be Gauss, Eratosthenes developed
the organized method to find the prime numbers (Figure
3). The system is known as the Sieve of Eratosthenes. By
using the Square Tool, one can easily find prime numbers
using the Sieve of Eratosthenes technique. An example
of finding primes less than 100 would look like this:
1) At the Square Tool site, select the 10 × 10 grid.
2) The number 1 is not classified as a prime number,
so the smallest prime number is 2. Highlight all the
multiples of two, excluding 2 (4,6,8, etc.).
3) The next non-highlighted number is a prime number,
3. Highlight all multiples of 3, excluding the num-
ber 3 (6,9,12,…99). Some of the numbers are al-
ready highlighted as they are also multiples of 2.
4) Highlight all the multiples of next prime, 5 up to
100, excluding the number 5. (10,15,20,…100).
Some of the numbers are already highlighted as they
are also multiples of 2 and / or 3.
5) Highlight all the multiples of next prime, 7 up to
100, excluding the number 7. Since most of the
numbers are already highlighted previously, the on-
ly remaining multiples of 7 are 49, 77, and 91.
6) The remaining numbers on the grid are prime
(shown in black in Figure 4).
3. Classroom Implementation
As we visited the classrooms of our pre-service teachers,
a class of 7th graders discovered that they can play inte-
ger games using the grid. For example, one team clicks
on 2 pairs of numbers and turning them red (they called
Table 1. Numeric relationship in addition, multiplication
and division.
Operations Relationship / Activity
Addition Add two even numbers together. What kind of
number is the result? Add two different even
numbers. What kind of number is this result? Do
this for several more pairs of even numbers. Write
a conjecture about the type of number you get
when you add two even numbers. Explain why the
result of two even numbers always seems to be an
even number. Explain why the result of two odd
numbers always seems to be an even number.
Explain why the result of adding one even and one
odd number always seems to be an odd number.
Using the Square Tool to look for a pattern when
one even and one number are added.
MultiplicationMultiplication is based on repeated adding of
groups. Students develop concrete ideas about
multiplication by using hands-on grouping activi-
ties. The Square Tool guides students to the ab-
stract view of multiplication using numbers. To
multiply 5 • 3, make a 5 × 5 square. (Figure 2)
Each row represents a group of 5 items. So 3
groups of 5 leads to the last number in row 3 or 15 .
Division Since multiplication and division are inverse
operations, the tool will explore this relationship.
In Example 1, let’s use the tool to visually calcu-
late 17 ÷ 5. Make a 5 × 5 square (Figure 2), 17 is
in the 4th row, 2nd column. There are three com-
plete rows and two additional numbers in the next
row written as 3 remainder 2. This leads to 17 = 5
• 3 + 2 and extended to 17 = 5 • □ + □. Students
build a foundation for Algebra by filling in the
boxes with the numbers that make the equation
true. In Example 2, the Square Tool can be used
to calculate 9 ÷ 5. There is 1 complete row and 4
additional numbers in the next row. The answer is
1 remainder 4, which means 1 whole row and 4 /
5 of another row.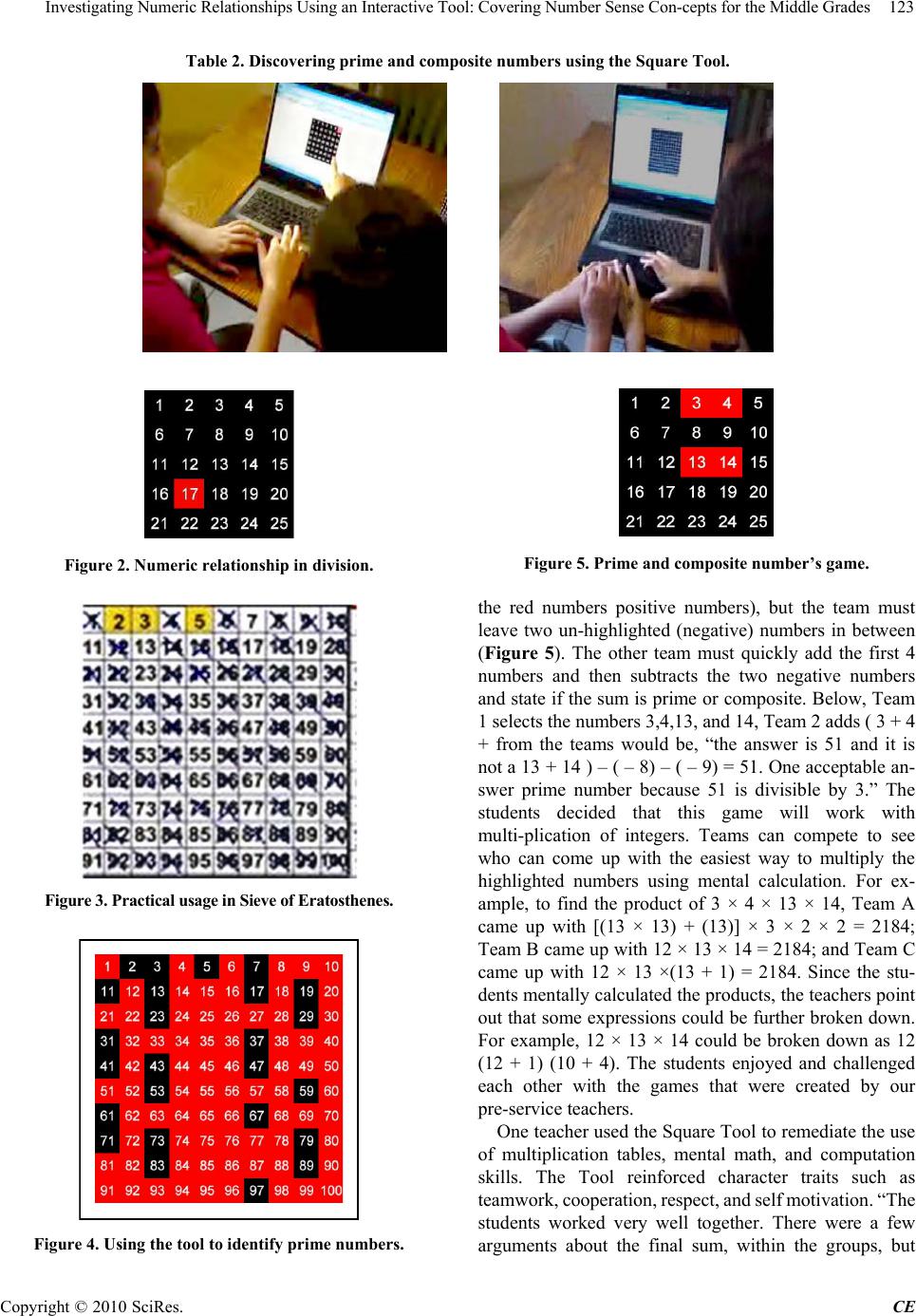

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades
123
Table 2. Discovering prime and comp osite numbe r s using the Squar e Tool.
Figure 2. Numeric relationship in division.
Figure 3. Practical usage in Sieve of Eratosthenes.
Figure 4. Using the tool to identify prime numbers.
Figure 5. Prime and composite number’s game.
the red numbers positive numbers), but the team must
leave two un-highlighted (negative) numbers in between
(Figure 5). The other team must quickly add the first 4
numbers and then subtracts the two negative numbers
and state if the sum is prime or composite. Below, Team
1 selects the numbers 3,4,13, and 14, Team 2 adds ( 3 + 4
+ from the teams would be, “the answer is 51 and it is
not a 13 + 14 ) – ( – 8) – ( – 9) = 51. One acceptable an-
swer prime number because 51 is divisible by 3.” The
students decided that this game will work with
multi-plication of integers. Teams can compete to see
who can come up with the easiest way to multiply the
highlighted numbers using mental calculation. For ex-
ample, to find the product of 3 × 4 × 13 × 14, Team A
came up with [(13 × 13) + (13)] × 3 × 2 × 2 = 2184;
Team B came up with 12 × 13 × 14 = 2184; and Team C
came up with 12 × 13 ×(13 + 1) = 2184. Since the stu-
dents mentally calculated the products, the teachers point
out that some expressions could be further broken down.
For example, 12 × 13 × 14 could be broken down as 12
(12 + 1) (10 + 4). The students enjoyed and challenged
each other with the games that were created by our
pre-service teachers.
One teacher used the Square Tool to remediate the use
of multiplication tables, mental math, and computation
skills. The Tool reinforced character traits such as
teamwork, cooperation, respect, and self motivation. “The
students worked very well together. There were a few
arguments about the final sum, within the groups, but

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades
124
overall they did well. The students thought the Square
Tool was a neat mathematical tool.”
3.1. Prime Numbers
While many interactive websites identify prime numbers
by using the Sieve of Eratosthenes, the automated process
eliminates the understanding of multiples by doing all
the hands-on work of removing the multiples. Through
observations, we as teachers feel that students should use
the Square Tool to help develop and then reinforce their
own understanding of “prime numbers” by creating their
own Sieve manually.
Students can use colored tiles as concrete examples to
explore the concept of multiples. By making differing
square sizes, the Square Tool helps the teacher discuss
multiples of the square size. The teacher can make paper
copies of the 10 × 10 square and allow the students to
find multiples by using different colors. For example, all
multiples of three can be blue and all multiples of five
can be red, then making multiples of fifteen purple.
They then can transfer to the Square Tool, make the
appropriate grid and click on the numbers to identify the
multiples.
In connecting the bridge from the concrete to the
semi-concrete, students can first use colored tiles to de-
velop a concrete understanding of the concept of factors.
For example, the factors of 12 are 1,2,3,4,6, and 12, stu-
dents can make the areas or arrays of each. Then they
can use the Square Tool to determine which method is
more efficient and why. Proper Factors are factors of n
other than itself. The proper factors of 14 are {1,2,7}.
Teachers may enjoy allowing students to learn about
abundant, deficient, perfect, and amicable numbers after
learning about proper factors as well.
3.2. Factors
This leads to Greatest Common Factors (GCF). Students
can then identify the GCF of two or more numbers. The
GCF is the largest factor that divides evenly into two or
more numbers. For example, the GCF for 15 and 24 is 3,
as 3 is the largest number both 15 and 24 can be divided
by evenly without a remainder.
By using the Square Tool, students can play games
that reinforce the concepts of proper factors and Greatest
Common Factor. In an urban school from southeastern
region of the United States, one writer of this article
during a class demonstration lesson found that the sev-
enth grade students picked up the GCF concept quickly
and had fun doing it to the point that they did not realize
that they were learning math (Table 3). They were testing
each other using the Square Tool by taking turns select-
ing 2 random numbers on the grid and see who can iden-
tify the GCF from the two random numbers within the
shortest time frame. This class of regular twenty-one
seventh graders in this Title One school, generated their
own factor problems and initiated the idea of testing
their conjectures with different grids. For example, Us-
ing a 7 × 7 grid, Mary, a student in this class, chal-
lenged her classmates to identify the number for the
proper factors {1,2,4,7,8,14, and 28}. Mary’s math
Table 3. Classroom implementation of the Square Tool.
7th graders using the Tool for prime numbers Student using the tool to investigate patterns
Students highlighted Multiples of 4 in red Students the Tool to create mathematical games

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades 125
classmates responded with, “56 is a composite number, it
has factors other than 1 and itself, therefore it is not a
prime number.” Students can see the 8 factors of 56 are
1,2,4,7,8,14,28 and 56. The factor pairs of 56 are 1 × 56, 2
× 28, 4 × 14, and 7 × 8. They went on and said that the
number for proper factors {1,2,4,7,8,14, and 28} is 56.
They also identified the prime factors of 56, which are
2,2,2, and 7.
3.3. Connections to Magic Squares
The Square Tool can create non-Magic Squares, since the
diagonals, horizontals, or verticals are not necessarily
equal to one another. Loly and Steeds (2005) [7] says that
there are an interesting class of purely pandiagonal, i.e.
non-magic squares, counting number squares of orders
(row / column dimension) that exist and many patterns
can be discovered while observing them. Based on re-
viewing the hundreds square which arranges the first 100
numbers respectively into 10 rows and 10 columns
(Figure 6), students can look for patterns and draw ap-
propriate conjectures.
One feature of the Square Tool is to add selected
numbers. The following number patterns and relation-
ships can be discovered using any size square. In this
example of a 7 × 7 square (Figures 7, 8, 9, and 10).
1) Numbers in the opposite ends of diagonals add to
50, for example in Figure 7:
1 + 49 = 50 or 7 + 43 = 50
2) In Figure 8, the sum of the major diagonals add to
175.
1 + 9 + 17 + 25 + 33 + 41 + 49 = 175 or,
7 + 13 + 19 + 25 + 31 + 37 + 43 = 175
3) If you take an “M × M” square within an “N × N”
square in Figures 9 and 10, the diagonal numbers at
the each corner add to the same number. In the 2 ×
2 square at the top corner of the 7 × 7 square, 1 + 9
= 10 and 8 + 2 = 10. In Figure 10, the 3 × 3 square
with vertices 17,19,31, and 33 have diagonal num-
bers with the same sum 17 + 33 = 50 and 19 + 31 =
50.
Figure 6. The Tool and connection 10 × 10 Square.
Figure 7. Sum of corner diagonal numbers are the same.
Figure 8. Sum of diagonals are the same.
Figure 9. Sum of diagonal numbers are the same.
Figure 10. Sum of diagonal numbers are the same.
4. Square Tool Tutorial
The Square Tool serves two major mathematical con-
cepts: addition and grouping.
1) Pick the size of your square from the pull-down
menu and then click on Create Grid Button.

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades
126
2) Highlight the numbers (it will change to a red back-
ground) that you want to add. The sum of those
numbers will be found in the Sum of Selected Cells
display.
3) If you want to remove just one cell, click on it again
and it will be deselected.
4) If you want all the cells cleared, select the Reset
button.
The size is 6 × 6 grid and click on Create Grid But-
ton.
Click on 4, 14, and 29. They will be highlighted with a
red background. In the Sum of Selected Cells display,
sum of the three highlighted numbers is 47 (Example 1).
a) The size is 6 × 6 grid and click on Create Grid
Button.
b) Click on the numbers down a major diagonal from 6
to 31. They will be highlighted with a red back-
ground. In the Sum of Selected Cells display, sum
of the highlighted cells is 111. If you Reset the Grid
and select the major diagonal from 1 to 36, the sum
will again be 111 (Example 2).
The sums of the diagonals of a square are equal.
1 + 36 = 6 + 31
Grouping is helpful with multiplication and divi-
sion.
1) Pick the size of your square from the pull-down
menu and then click on Create Grid Button.
2) Highlight the number under consideration.
3) Use the visual aspects of this number in relationship
to rows and columns to help with multiplication and
division problems (Example 3).
Other Examples
a) The size is 6×6 grid and click on Create Grid
Button.
b) If the number 18 is selected, 18 equals 3*6 because
it is found on the 3rd row of 6 numbers.
If the number 26 is selected, 26 ÷ 6 = 4 remainder 2 (4
completed rows and 2 additional numbers in the fifth row)
(Example 4).
Example 1. In the Sum of Selected Cells display, sum of the
three highlighted numbers is 47.
Example 2. In the Sum of Selected Cells display, sum of the
highlighted cells is 111.
Example 3. The sums of the diagonals of a square are equal.
1 + 36 = 6 + 31.
Example 4. If the number 18 is selected, 18 equals 3*6 be-
cause it is found on the 3rd row of 6 numbers.

Investigating Numeric Relationships Using an Interactive Tool: Covering Number Sense Con-cepts for the Middle Grades
127
5. Summary
As an interactive program, the Square Tool puts the
learner in charge of his / her own exploration of number
patterns at all mathematics grade levels. Number theory,
the study of properties of integers, is taught at the college
level but its foundation is developed in the middle grades.
To provide this foundation, math teachers can use the
Square Tool to investigate numeric relationships ranging
from simple operations to primes and multiples and be-
yond. The Square Tool’s main goal is to connect the
concrete number ideas using Color Tiles and other mani-
pulatives to students’ abstract understanding of number
theory, thus serving as a bridge or semi-concrete repre-
sentation of these mathematical ideas in an interactive
fashion. The Square Tool can serve as a semi- concrete
bridge connecting the concrete to the abstract for stu-
dents.
REFERENCES
[1] H. F. Su, C. Marinas and C. Chraibi, “Exploring Nu-
merical Relationship through Interactive Numbered
Squares of Differing Sizes,” Proceeding of International
Conference on Technology in Collegiate Mathematics,
San Antonio, Vol. 20, March 2008, pp. 28-32.
[2] National Council of Teachers of Mathematics, Principles
and Standards for School Mathematics, NCTM: Reston,
VA, 2000.
[3] J. M. Furner, “Geometry Sketching Software: It’s Ele-
mentary Dear Euclid,” World Conference on Educational
Multimedia, Hypermedia & Telecommunications, Mont-
real, June 30, 2005.
[4] S. Ozel, Z. E. Yetkiner and R. M. Capraro, “Technology
in K-12 Mathematics Classrooms,” School Science and
Mathematics, Vol. 108, No. 2, 2008, pp. 80-85.
[5] E. Wall, “Number Theory for Elementary School Teach-
ers,” McGraw-Hill Company, New York, 2010.
[6] S. R. Overbay and M. J. Brod, “Magic with Mayan
Math,” Mathematics Teaching in the Middle School, Vol.
12, No. 6, 2007, pp. 340-347.
[7] P. D. Loly and M. J. Steeds, “A New Class of Pandiagonal
Squares,” International Journal of Mathematical Educa-
tion in Science and Technology, Vol. 36, No. 4, 2005, pp.
375-388.