Journal of Electromagnetic Analysis and Applications
Vol.5 No.3(2013), Article ID:29205,6 pages DOI:10.4236/jemaa.2013.53017
Chiral Dirac Equation Derived from Quaternionic Maxwell’s Systems
![]()
University School of Electrical and Electronic Engineering, Arica, Chile.
Email: htorres@uta.cl
Received December 8th, 2012; revised January 10th, 2013; accepted January 23rd, 2013
Keywords: Quaternion; Dirac Equation; Maxwell System
ABSTRACT
In the present article, we propose a simple equality involving the Dirac operator and the Maxwell operators under chiral approach. This equality establishes a direct connection between solutions of the two systems and moreover, we show that it is valid when the natural relation between the frequency of the electromagnetic wave and the energy of the Dirac particle is fulfilled if the electric field E is parallel to the magnetic field H. Our analysis is based on the quaternionic form of the Dirac equation and on the quaternionic form of the Maxwell equations. In both cases these reformulations are completely equivalent to the traditional form of the Dirac and Maxwell systems. This theory is a new quantum mechanics (QM) interpretation. The below research proves that the QM represents the electrodynamics of the curvilinear closed chiral waves. It is entirely according to the modern interpretation and explains the particularities and the results of the quantum field theory. Also this work may help to clarify the controversial relation between Maxwell and Dirac equations while presenting an original way to derive the Dirac equation from the chiral electrodynamics, leading, perhaps, to novel conception in interactions between matter and electromagnetic fields. This approach may give a reinterpretation of Majorana equation, neutrino mass, violation of Heinsenberg’s measurement-disturbation relationship and mass generation in systems like graphene devices.
1. Introduction
In mathematical physics, the relation between the two most important first order systems of partial differential equations, (Dirac equation and Maxwell’s equations), is among those topics which attract attention because of their general significance and solutions of particular problems concerning physical models. The connection between solutions of massless Dirac and Maxwell equations was well established [1]. It is shown that the massless Dirac equation is invariant under three different representation of Poincaré algebra. Two coupled Dirac equations with masses m and –m has also possesses this symmetries. The Maxwell equations can be represented in a Dirac like form in different ways (e.g., [1-10]). The Beltrami equations are another approach [11]. Also, solutions of Maxwell’s system can be related to solutions of the Dirac equation through some nonlinear equations (e.g., [12]).
However, in [13] we find that the author shows that in the formulation of [12], based on spinor form there is no physically meaningful way to transform Maxwell’s and Dirac’s equations into each other. However, this statement is valid for standard Maxwell fields, but not for parallel electromagnetic fields that will be discussed in this paper.
Maxwell’s equations are formulated in a number of different representations: a) As a single four-component spinor equation whose transformation properties are almost identical with those of the Dirac equation; b) As a pair of uncoupled two-component spinor equations, in two different representations. One of these is similar to the Weyl equation for the neutrino field and the other to the two-component spinor form of the Dirac equation; c) As a single equation in which the field variables are 2 × 2 matrices.
Nevertheless, in spite of these significant efforts there remain some important conceptual questions. For example, what is the meaning of this close relation between the Maxwell system and the Dirac equation and how this relation is connected with the wave-particle dualism. In the present article we propose a simple equality involving the Dirac quaternionic operator and the Maxwell quaternionic operators under chiral approach. Dirac derived the linear relativistic wave equation for fermions by “taking the square root” of the Klein-Gordon equation, which is quadratic in time and space derivatives. In this paper, we find a chiral electromagnetic wave equation of fourth order which can be linearized to the Dirac way to obtain two linear Beltrami equations.
We propose to find an equivalence between the Dirac equation and the Beltrami equations in quaternionic coordinates. This equality establishes a direct connection between solutions of the two systems and moreover, we show that it is valid when a quite natural relation between the frequency of the electromagnetic wave and the energy of the Dirac particle is fulfilled. This condition is satisfied when E is parallel to H. Our analysis is based on the quaternionic form of the Dirac equation [8] and on the quaternionic form of the Maxwell equations [7,8] (see also [9]). In both cases our quaternionic reformulations are completely equivalent to the traditional form of the Dirac and Maxwell systems. Chiral approach means that our Universe is observable area of basic space-time where temporal coordinate is positive and all particles bear positive masses (energies). The mirror Universe is an area where temporal coordinate is negative and all particles bear negative masses. Also, from viewpoint of our world observer, the mirror Universe is a world with reverse flow of time, where particles travel from future into past in respect to us. The two worlds are separated by a membrane—an area of space-time inhabited by light-like particles that travel along light-like right or left-handed (isotropic-chiral) spirals. On the scales of elementary particles such space can be attributed to particles that possess spirality (e.g. Chiral photons). The membrane prevents mixing of positive and negativemass particles and thus their total annihilation. The link between both universes is the chiral factor defined by  (see Section 3).
(see Section 3).
The aim of this work is to link the Dirac equation in the Weyl representation with Maxwell’s equations in the chiral formulation of Born Fedorov and show that only when the E field and H field are spatially parallel, we have that this field distribution can generate mass and the Dirac equation can be obtained from the chiral electrodynamics. These fields are circulatory and stationary. Under this condition we have no radiation and the vector Poynting  is zero.
is zero.
2. The Dirac Equation in Quaternionic Form
The algebra of complex quaternions is denoted by 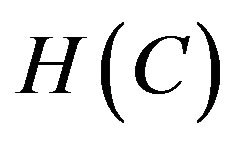 . Each complex quaternion
. Each complex quaternion  is of the form
is of the form 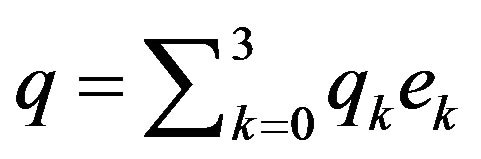 where
where ,
,  is the unit and
is the unit and  are the quaternionic imaginary units:
are the quaternionic imaginary units:
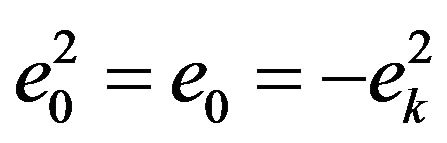 ;
;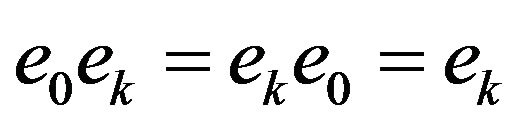 ,
,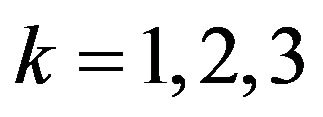 ;
;
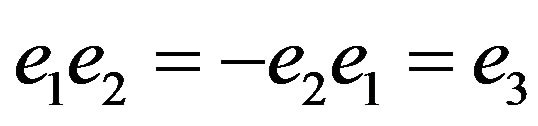 ;
;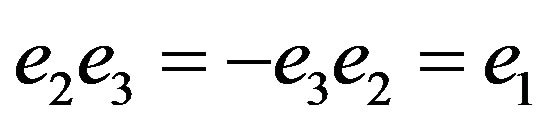 ;
; 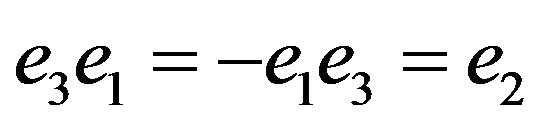
The complex imaginary unit  commutes with
commutes with 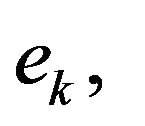
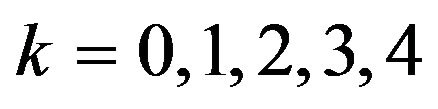 . We further introduce a complex conjugate operation
. We further introduce a complex conjugate operation which takes
which takes 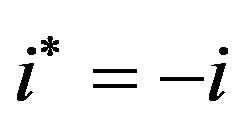 but leaves
but leaves 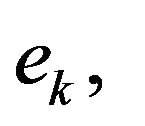
 unchanged, as well as a quaternionic conjugation operation
unchanged, as well as a quaternionic conjugation operation , which leaves i unchanged but takes
, which leaves i unchanged but takes
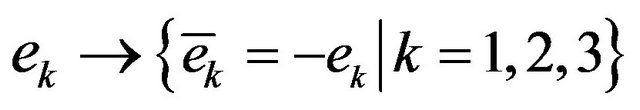
We will use the vector representation of complex quaternions: , where
, where  and
and
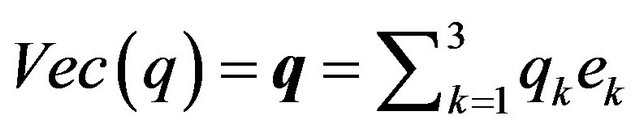 .
.
That is each complex quaternion is a sum of its scalar part and its vector part. Complex vectors we identify with complex quaternions whose scalar part is equal to zero. In vector terms, the multiplication of two arbitrary complex quaternions 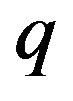 and
and 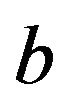 can be written as follows:
can be written as follows:
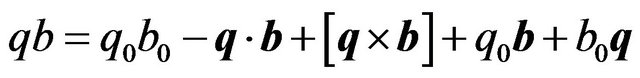 where
where
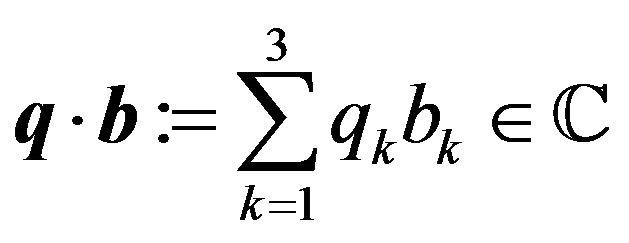 ,
,
We shall consider continuously differentiable  - valued functions depending on three real variables
- valued functions depending on three real variables 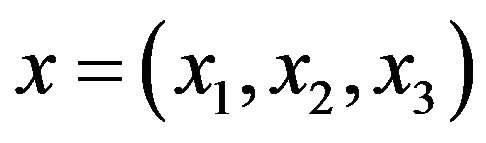 . On this set the well known (see, e.g., [1,2,5,8,9]) Moisil-Theodoresco operator is defined by the expression
. On this set the well known (see, e.g., [1,2,5,8,9]) Moisil-Theodoresco operator is defined by the expression
 ,
, where
where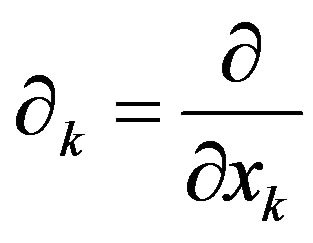 .
.
The action of the operator  on an
on an 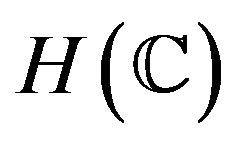 -valued function
-valued function 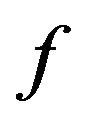 can be written in a vector form:
can be written in a vector form:
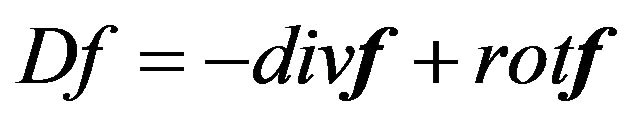 . (1)
. (1)
In a good number of physical applications the operators 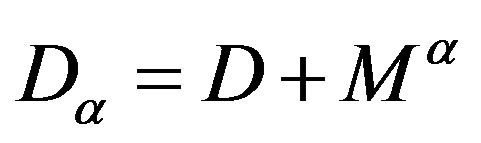 and
and 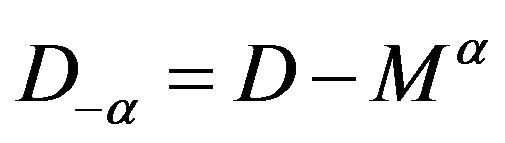 are needed, where
are needed, where 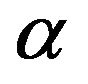 is a complex quaternion and
is a complex quaternion and 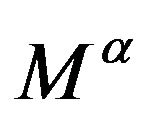 denotes the operator of multiplication by
denotes the operator of multiplication by 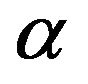 from the right-hand side:
from the right-hand side: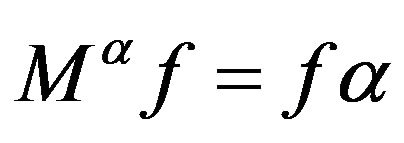 . Here we will be interested in two special cases when
. Here we will be interested in two special cases when 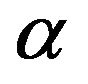 is a scalar, that is
is a scalar, that is 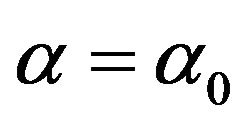 or when
or when 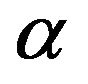 is a vector
is a vector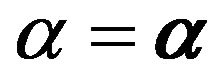 . The first case corresponds to the Maxwell equations and the second to the Dirac equation (see [4,8]).
. The first case corresponds to the Maxwell equations and the second to the Dirac equation (see [4,8]).
Following [8,14,15], but by considering the chiral representation, the Dirac equation in its covariant form
 .
.
For a wave function with a given energy we have
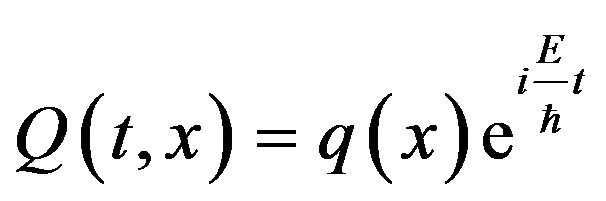 , where
, where 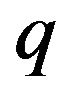 satisfies the time harmonic Dirac equation
satisfies the time harmonic Dirac equation
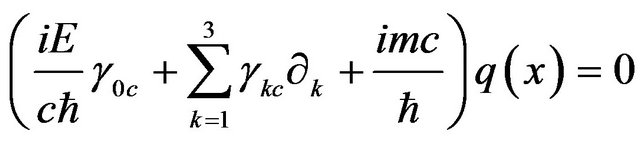 . (2)
. (2)
Denote
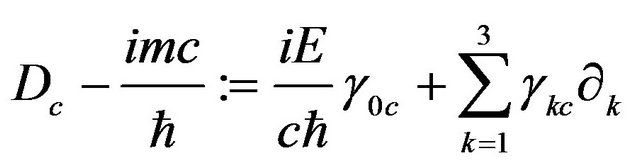 .
.
where the gamma matrices are given in the chiral representation. Also, 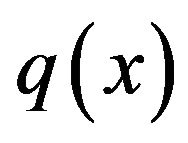 is written in terms of the two component complex spinor
is written in terms of the two component complex spinor 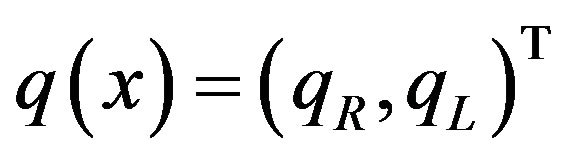


With 0 and 1 are the 2 × 2 zero and unit matrix and 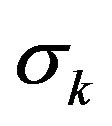 the Pauli matrices.
the Pauli matrices.
Equation (2) can be written as a two component Weyl equation

Let us introduce an auxiliary notation  . The transformation which allows us to rewrite the Dirac Equation (2) in a quaternionic form we denote as
. The transformation which allows us to rewrite the Dirac Equation (2) in a quaternionic form we denote as  defined as a function
defined as a function 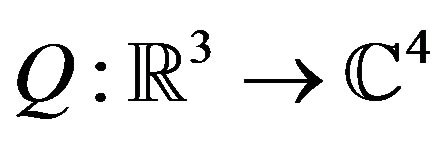 which is transformed into a function
which is transformed into a function 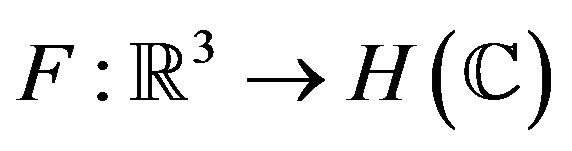 by the rule
by the rule ,[7].
,[7].
The inverse transformation  is
is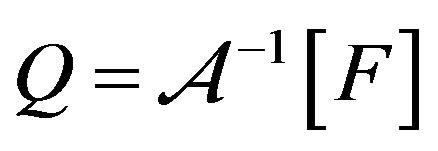 . The introduced transformations relates the components of a
. The introduced transformations relates the components of a  -valued function
-valued function  with the components of an
with the components of an 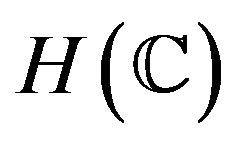 -valued function
-valued function :
:
Following [7,8] an important equality is obtained
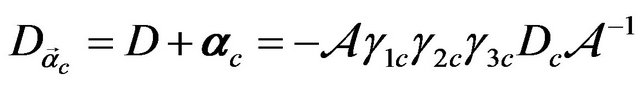 where
where
 (3)
(3)
The difference between this  and
and 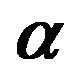 of [8,14,15] is that here the Dirac Equation (2) is in the Weyl or chiral representation. This equality shows that instead of Equation (2) we can consider the equivalent quaternionic equation
of [8,14,15] is that here the Dirac Equation (2) is in the Weyl or chiral representation. This equality shows that instead of Equation (2) we can consider the equivalent quaternionic equation
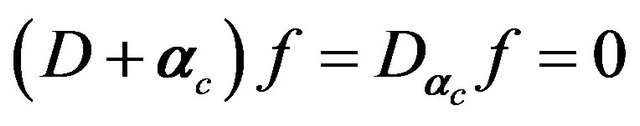
This last equation can be written as the two-component field 
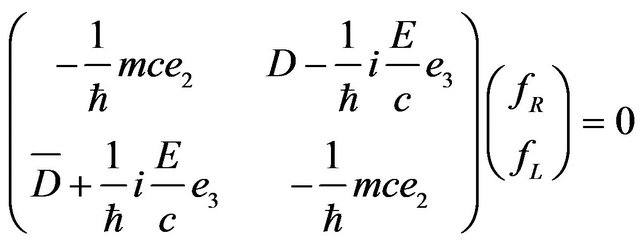 (4)
(4)
and the relation between solutions of (2) and (4) is established by means of the invertible transformation 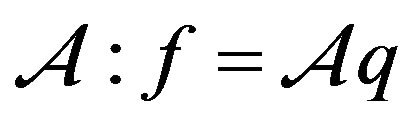 .
.
Next we develop the Maxwell equations in quarternionic representation.
3. The Maxwell Equations in Chiral Quaternionic Form
Chiral materials, in which the mirror reflection symmetry is broken, are ubiquitous in nature. Even the quantum vacuum of the standard model of particle physics is chiral [16], so that the behavior of the left-handed and righthanded elementary particles (quarks and leptons) are essentially different. One of the consequences is the chiral anomaly—the anomalous nonconservation of a chiral current, as first described by Adler [17] and by Ginzburg and Landau [18]. The chiral anomaly provides one explanation for the baryogenesis in the early Universe and the huge excess of matter over antimatter in the present Universe. Here, we consider a chiral vacuum. A Chiral Vacuum will be defined as a vacuum for which the constitutive matrices represented by  are not zero, but for which there are no real charge densities or current densities. Such an assumption, which if applicable to the vacuum, would imply that the chiral vacuum, and there for the universe itself, may not have a center of symmetry. The concept of spontaneous symmetry breakdown’ has proved to be extraordinarily fruitful in many areas of physics and I consider it worthwhile to try to incorporate it into chiral electromagnetism The modern formulation of this concept appears to originate with the work of V. Ginzburg and L. Landau [18] and A. Zee [19].
are not zero, but for which there are no real charge densities or current densities. Such an assumption, which if applicable to the vacuum, would imply that the chiral vacuum, and there for the universe itself, may not have a center of symmetry. The concept of spontaneous symmetry breakdown’ has proved to be extraordinarily fruitful in many areas of physics and I consider it worthwhile to try to incorporate it into chiral electromagnetism The modern formulation of this concept appears to originate with the work of V. Ginzburg and L. Landau [18] and A. Zee [19].
First we consider the chiral factor  as an scalar parameter and then we extend the analysis to a chiral matrix. We will consider the Maxwell equations for a sourceless anisotropic chiral homogeneous medium with
as an scalar parameter and then we extend the analysis to a chiral matrix. We will consider the Maxwell equations for a sourceless anisotropic chiral homogeneous medium with 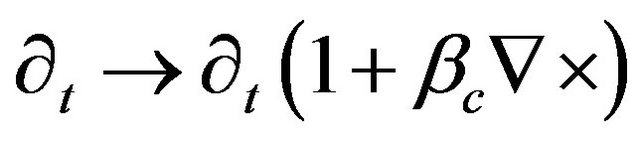 [20-22].
[20-22]. 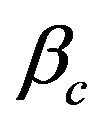 is a chiral parameter so the Maxwell’s equations without charges are expressed as:
is a chiral parameter so the Maxwell’s equations without charges are expressed as:
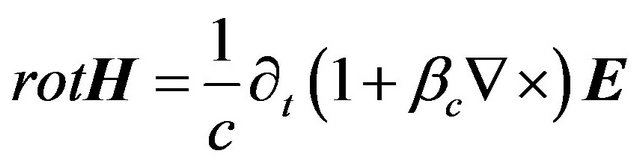 ,
, 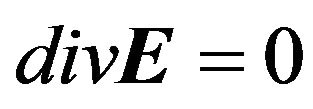
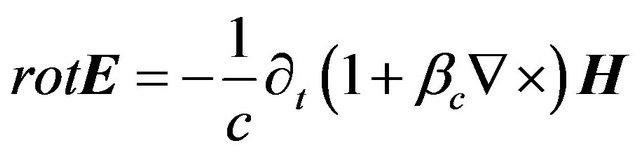 ,
, 
So the time-harmonic Maxwell equations are
 , (5)
, (5)
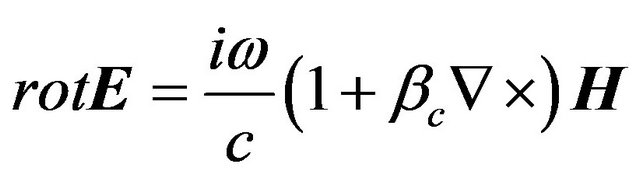 , (6)
, (6)
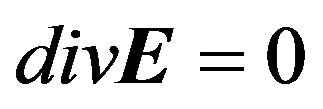 , (7)
, (7)
 . (8)
. (8)
Here, 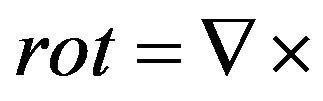 ,
,  is the chiral scalar parameter,
is the chiral scalar parameter, 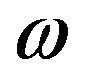 is the frequency. Application of rot to (5) and (6) allows us to separate the equations for
is the frequency. Application of rot to (5) and (6) allows us to separate the equations for  and
and  and to obtain in this way the wave equation for chiral medium.
and to obtain in this way the wave equation for chiral medium.
 (9)
(9)
this chiral generalization represents an equation of fourth order. When
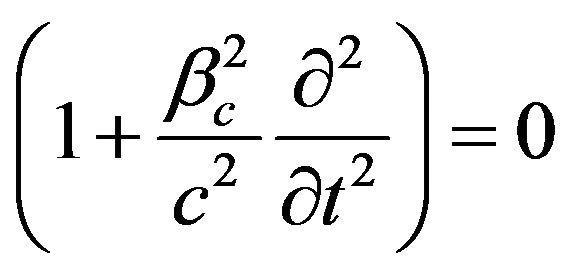 (10)
(10)
we have an important linearization which transforms a fourth order equation to a first order one.
 (11)
(11)
This result corresponds to a self dual electromagnetic fields represented by standing waves possessing zero Poynting vector [23]. In this case, we can link the Dirac Equation (4) with the Beltrami Equation (11). Obviously system (11) can be written in the form of a quaternionic equation if we define
 ,
,  ,
, 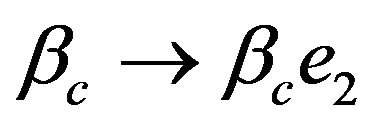 , so Equation (10) is transformed to
, so Equation (10) is transformed to
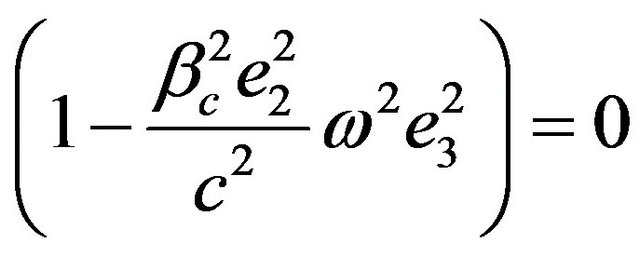 (12)
(12)
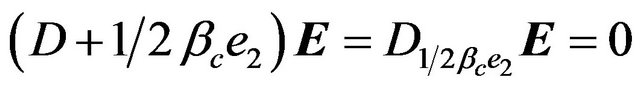 (13)
(13)
where
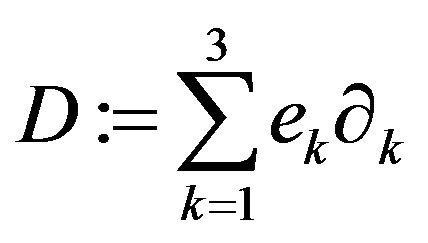
A similar equation can be obtained for . Taking into account (1) and
. Taking into account (1) and  and
and  simultaneously, we introduce the following quaternionic operator
simultaneously, we introduce the following quaternionic operator

If we consider the purely vectorial biquaternionic function 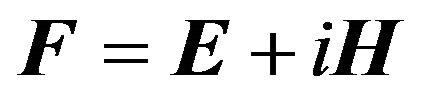 and the complex conjugate
and the complex conjugate 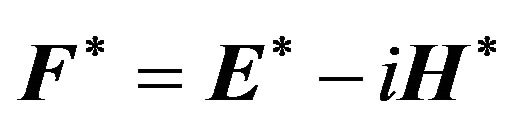 we have
we have
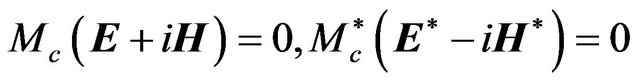 (14)
(14)
The first equation is equivalent to the Maxwell system (5)-(8). We can rewrite this system in matrix form as
 , (15)
, (15)
Equation (15) can be diagonalized and transformed in the following way [5,6] (see also [8,14]). Following [5] we define
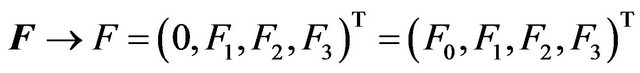
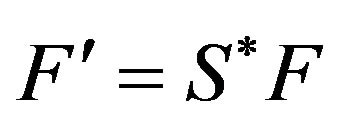
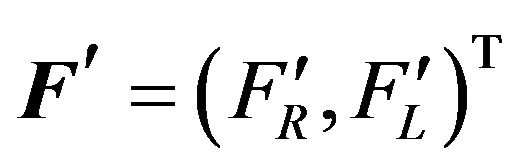
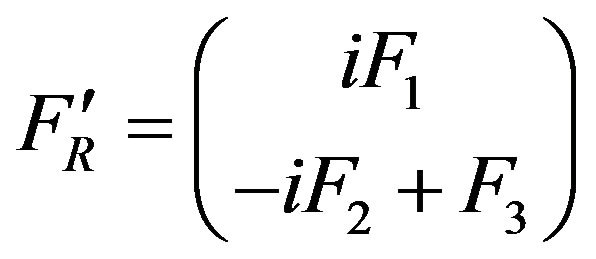 ;
;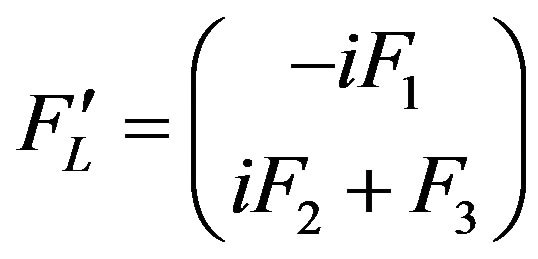 (16)
(16)
Where the unitary matrix 
 is given by
is given by
 (17)
(17)
Thus we are transformed the quaternionic Maxwell Equation (15) in the form of two component equations
 (18)
(18)
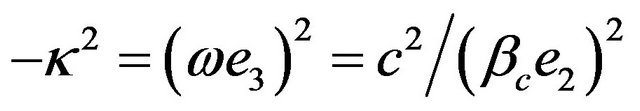 is the square wave number. Applying the operator
is the square wave number. Applying the operator  and
and 
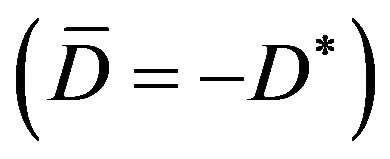 to the functions
to the functions  and
and 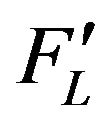 respectively one can see that
respectively one can see that  satisfies the equation
satisfies the equation
 , (19)
, (19)
and  satisfies the equation
satisfies the equation
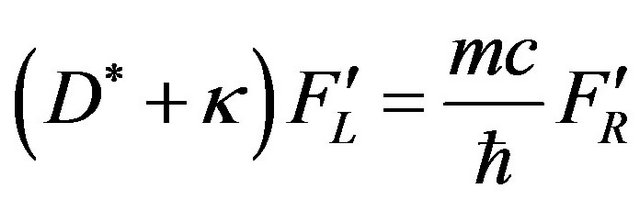 . (20)
. (20)
Solutions of (19) and (20) are called the Beltrami fields (see, e.g., [11]). Thus, if we compare (19) and (20) with Equation (4), we infer that for parallel fields, the coupled system (19-20) is equivalent to Equation (4). R and L, subscripts are associated with circularly polarized photons (right or left-handed spirals or chiral photons).
4. The Chiral Electromagnetic Dirac Equation
In the preceding sections it was shown that the Dirac Equation (2) is equivalent to the quaternionic equation
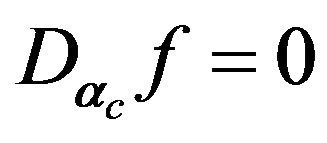 with
with 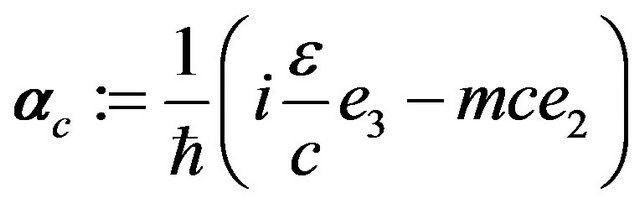 and the Maxwell Equations (5)-(8) are equivalent to the pair of quaternionic Equations (19) and (20) when the electric field
and the Maxwell Equations (5)-(8) are equivalent to the pair of quaternionic Equations (19) and (20) when the electric field  is parallel to the magnetic field
is parallel to the magnetic field ,
,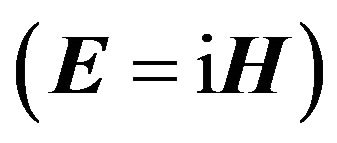 . If f is a solution of (4), then
. If f is a solution of (4), then  are solutions of Equations (19) and (29) with
are solutions of Equations (19) and (29) with 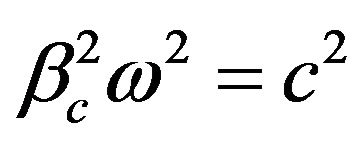 and the coupled Equations (19) and (20) are equivalent to the chiral Dirac Equation (4).
and the coupled Equations (19) and (20) are equivalent to the chiral Dirac Equation (4).
Now we will show a simple relation between these objects. Observing Equation (10) or (12) we have
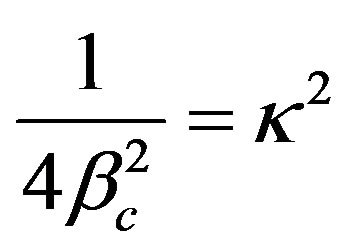 . (21)
. (21)
Note that from Equation (3)
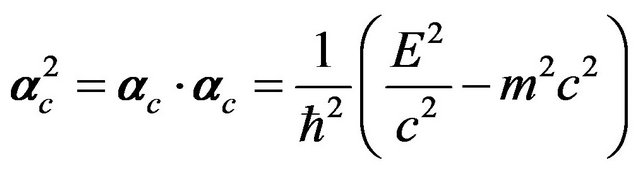 . (22)
. (22)
Thus, when  is parallel to
is parallel to , (21) is equal to (22) we have the important result
, (21) is equal to (22) we have the important result
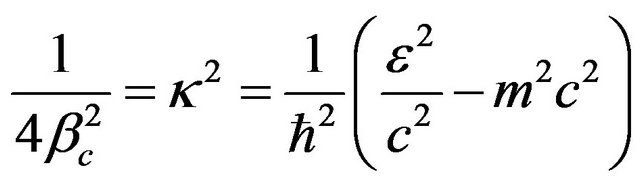 (23)
(23)
Thus, relation between the Dirac operator and the Maxwell operators is valid if the condition (23) is fulfilled. This happens if and only if  is parallel to
is parallel to , that is
, that is  and the vector Poynting is null.
and the vector Poynting is null.
In general, if in (23) we formally use the de Broglie equality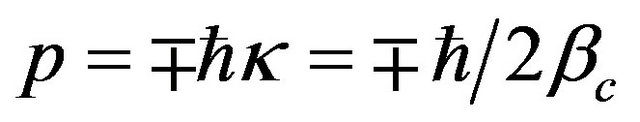 , we again obtain the fundamental Einstein relation
, we again obtain the fundamental Einstein relation
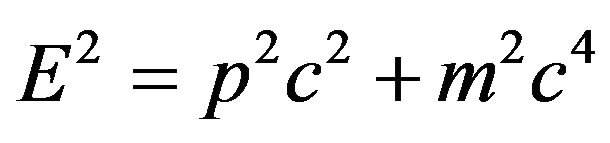 . (24)
. (24)
From  we obtain naturally the value of the electron spin
we obtain naturally the value of the electron spin  and the positron spin
and the positron spin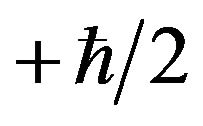 .
.
We can note that 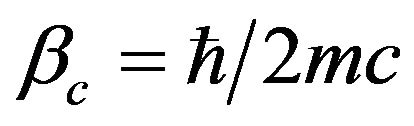 and
and 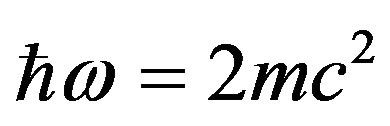 so the original energy photon is transformed in the pair particle-antiparticle
so the original energy photon is transformed in the pair particle-antiparticle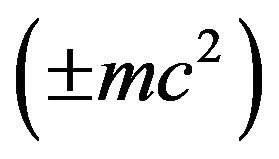 .
.
If we consider  and
and  as arbitrary observable operators
as arbitrary observable operators  and
and , a new Heisenberg uncertainty principle (HUP) is obtained
, a new Heisenberg uncertainty principle (HUP) is obtained
 (25)
(25)
While there is a rigorously proven relationship about uncertainties intrinsic to any quantum system often referred to as “Heisenberg’s uncertainly principle” HUP, recently it has been shown experimentally violation of Heisenberg’s measurements-disturbance relationship by weak measurements, MDR [24,25].
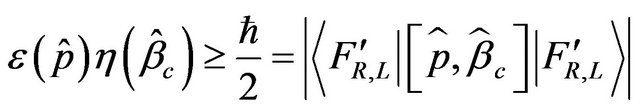
It is formally incorrect because the disturbance
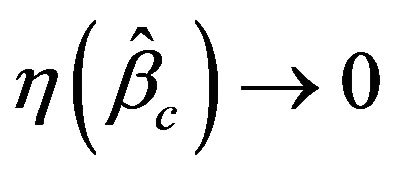 in some eigenstate
in some eigenstate .
.
Equation (25) is appropriate to obtain the corrected MDR
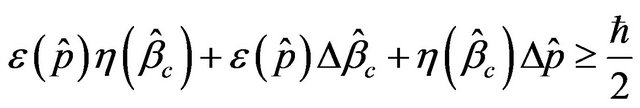
The authors [24,25] have designed an apparatus to measure the polarization of a single photon. They needed to measure how much that apparatus disturbed that photon. To do this, they needed to measure the photon before the apparatus but how that measurement would also disturb the photon. Our approach is related precisely to the polarization of photons (see Equations (19) and (20)). Our theory may be important on study of uncertainly relations, specifically in the setting of quantum information and quantum cryptography.
Also, with this theory we can explore the neutrino physics. The importance of neutrino electromagnetic properties was first mentioned by Pauli in 1930. Systematic theoretical studies of neutrino electromagnetic properties started after it was shown that in the extended Standard Model with right-handed neutrinos the magnetic moment of a massive neutrino is, in general, non vanishing and that its value is determined by the neutrino mass [26].
As in known, uncharged fermions having non-zero mass and spin 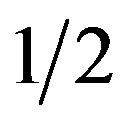 may be subject to one of the two equations: the Dirac equation, similar to that for charged particles such electron (Equation (18)), and the Majorana equation in which the inversion (on our case of
may be subject to one of the two equations: the Dirac equation, similar to that for charged particles such electron (Equation (18)), and the Majorana equation in which the inversion (on our case of ) takes helicity particles into antiparticles (in our theory if we take
) takes helicity particles into antiparticles (in our theory if we take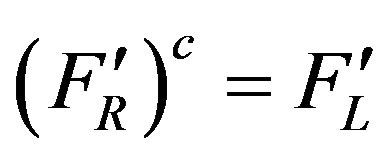 ).
).
Neutrino electromagnetic properties are important because they are directly connected to fundamentals of particle physics. For example, neutrino electromagnetic properties can be used to distinguish Dirac and Majorana neutrinos and also as probes of new physics that might exist beyond the Standard Model. Equation (18) is well suited to study the electromagnetic properties of neutrinos. Our result Equation (18) is appropriate to study Dirac and Majorana particles. As  our theory lends itself to study mass generation in graphene devices [22]. Also, classically the Heisenberg expression can be written in terms of time and energy and can be useful in problems of shear waves [27].
our theory lends itself to study mass generation in graphene devices [22]. Also, classically the Heisenberg expression can be written in terms of time and energy and can be useful in problems of shear waves [27].
5. Conclusions
The main result of this paper is that the Dirac equation can be derived from the Maxwell’s equation under chiral quaternionic approach. Equations (15)-(20) are our main results which are compared with Equation (4). With this theory we can study electromagnetic interactions in neutrinos which can be considered as Majorana fermions, favoured by simplicity because they have only two degrees of freedom.
Also, our approach is related precisely to the polarization of photons so this theory may be important on study of uncertainly relations, specifically in the setting of quantum information and quantum cryptography.
Also, our theory lends itself to study mass generation in graphene devices.
The author thanks the anonymous reviewer for helpful comments.
REFERENCES
- W. I. Fushchyld, “On the Connection between Solutions of Dirac and Maxwell Equations,” Scientific Works, Vol. 4, No. 1, 2002, pp. 320-336.
- A. A. Campolattaro, “Generalized Maxwell Equations and Quantum Mechanics,” International Journal of Theoretical Physics, Vol. 29, No. 2, 1990, pp. 141-155. doi:10.1007/BF00671324
- V. V. Dvoeglazov, “Generalized Maxwell and Weyl Equations for Massless Particles,” Revista Mexicana de Física, Vol. 49S1, No. 6, 2003, pp. 99-103.
- R. H. Good, “Particle Aspect of the Electromagnetic Field Equations,” Physical Review, Vol. 105, No. 6, 1957, pp. 1914-1919. doi:10.1103/PhysRev.105.1914
- H. E. Moses, “Solution of Maxwell’s Equations in Spinor Notation,” Physical Review, Vol. 113, No. 6, 1959, pp. 1670-1679. doi:10.1103/PhysRev.113.1670
- K. Imaeda, “A New Formulation of Classical Electrodynamics,” Nuovo Cimento, Vol. 32B, No. 1, 1976, pp. 138- 162.
- H. Campos, V. Kravchenko and L. Méndez, “Complete Families of Solutions of the Dirac Equation: An Application of Bicomplex Pseudoanalytic Function Theory and Transmutation Operators,” Advances in Applied Clifford Algebras, Vol. 22, No. 3, 2011, pp. 557-594.
- V. V. Kravchenko, “On the Relation between the Maxwell System and the Dirac Equation,” WSEAS Transaction on Systems, Vol. 1, No. 2, 2002, pp. 115-118.
- V. V. Kravchenko and M. P. Ramirez, “On Bers Generating Functions for First Order Systems of Mathematical Physics,” Advances in Applied Clifford Algebras, Vol. 21, No. 3, 2011, pp. 547-559. doi:10.1007/s00006-010-0261-5
- I. Yu. Krivsky and V. M. Simulik, “Unitary Connection in Maxwell-Dirac Isomorphism and the Clifford Algebra,” Advances in Applied Clifford Algebras, Vol. 6, No. 2, 1996, pp. 249-259.
- A. Lakhtakia, “Beltrami Fields in Chiral Media,” World Scientific Series in Contemporary Chemical Physics, Vol. 2, No. 1, 1994.
- J. Vaz Jr. and W. Rodrigues Jr., “Equivalence of Dirac and Maxwell Equations and Quantum Mechanics,” International Journal of Theoretical Physics, Vol. 32, No. 6, 1993, pp. 945-959. doi:10.1007/BF01215301
- A. Gsponer, “On the ‘Equivalence’ of the Maxwell and Dirac Equations,” International Journal of Theoretical Physics, Vol. 41, No. 4, 2002, pp. 689-964. doi:10.1023/A:1015232427515
- V. V. Kravchenko and H. Oviedo, “On the Quaternionic Reformulation of Maxwell’s Equations for Chiral Media and Its Applications, Zeischrift für Analysis und ihre Anwendungen,” Journal for Analysis and Its Applications,” Vol. 22, No. 3, 2003, pp. 569-589.
- B. Schneider and E. Karapinar, “A Note on Biquaternionic MIT Bag Model,” International Journal of Contemporary Mathematical Sciences, Vol. 1, No. 10, 2006, pp. 449-461.
- T. D. Lee and C. N. Yang, “Question of Parity Conservation in Weak Interactions,” Physical Review, Vol. 104, No. 1, 1956, pp. 254-258. doi:10.1103/PhysRev.104.254
- S. Adler, “Axial-Vector Vertex in Spinor Electrodynamics,” Physical Review, Vol. 177, No. 5, 1969, pp. 2426- 2438. doi:10.1103/PhysRev.177.2426
- V. Ginzburg and L. Landau, “On the Theory of Superconductivity,” Zhurnal Eksperimentalnoi i Teoreticheskoi Fisiki. Vol. 20, No. 1, 1950, p. 1064.
- A. Zee, “Broken-Symmetric Theory of Gravity,” Physical Review Letter, Vol. 42, No. 7, 1979, pp. 417-421. doi:10.1103/PhysRevLett.42.417
- H. Torres-Silva and D. Torres, “Chiral Current in a Graphene Battery,” Journal of Electromagnetic Analysis and Applications, Vol. 4, No. 10, 2012, pp. 426-431.
- H. Torres-Silva, “Chiral Transverse Electromagnetic Standing Waves with E II H in the Dirac Equation and the Spectra of the Hydrogen Atom,” In: A. Akdagli, Ed., Behavior of Electromagnetic Waves in Different Media and Structures, Chapter 15, Book Intech, Rijeka, 2011, pp. 301-324.
- H. Torres-Silva, “Chiral Waves in Graphene Medium and Optical Simulation with Metamaterial,” In: A. Kishk, Ed., Solutions and Applications of Scattering, Propagation, Radiation and Emission of Electromagnetic Waves, Chapter 2, Book Intech, Rijeka, 2012, pp. 25-55.
- E. Chubykalo, et al., “Self Dual Electromagnetic Fields,” American Journal of Physics, Vol. 78, No. 8, 2010, pp. 858-861.
- L. Rozena, et al., “Violation of Heisenbergs’s Measurement-Disturbance Relationship by Weak Measurements,” Physical Review Letters, Vol. 109, 2012, Article ID: 100 404.
- J. Erhart, et al., “Experimental Demonstration of a Universally Valid Error-Disturbance Uncertainty Relation in Spin Measurements,” Nature Physics, Vol. 8, 2012, pp. 185-189. doi:10.1038/nphys2398
- A. Studenikin, “Neutrino Magnetic Moment,” Nuclear Physics B, Vol. 188, No. 1, 2009, pp. 220-222.
- H. Torres-Silva and D. T. Cabezas, “Chiral Seismic Attenuation with Acoustic Metamaterials,” Journal of Electromagnetics Analysis and Applications, 2013, in Press.

