Received 28 September 2015; accepted 26 October 2015; published 29 2015

ABSTRACT

For steady frictionless flow along a straight line, when a constant acceleration is applied parallel to that line, a term needs to be added to the standard form of Bernoulli’s equation. After so modifying, the equation then predicts that along a streamline, when the speed is high, the pressure is significantly lower than that if there were no acceleration. For example, one might think of a dense fluid falling down through a less dense fluid under gravity. Potential applications to vertical motions of the atmosphere, such as down bursts of cold dry air and warm humid updrafts in the eye of a hurricane, are mentioned.

Keywords:

Bernoulli’s Equation, Accelerated Motion

1. Introduction

Frictional correction terms to be added to Bernoulli’s equation have been available for over 50 years [1] . More recently a surface tension term has been added to Bernoulli’s equation in order to help explain the vena contracta [2] . Here, apparently for the first time, an acceleration term, constant in magnitude and directed parallel to the streamlines, has been added to the standard (steady and inviscid) form of the Bernoulli equation: where the speed is greatest, the pressure is least, and vice versa. Potential applications to the tornado and to the hurricane are mentioned below.

Considering strictly vertical fluid motion, the additional term to be put into Bernoulli’s equation involves the acceleration of gravity multiplied by the density, or difference in two densities, that are taken constant.

It should be pointed out that since before 1850 theoretical studies of surface gravity waves have used Bernoulli’s equation as a boundary condition at the air/water interface in which a gravity term was included. However, for waves of small slope gravity acts basically perpendicular, rather than parallel, to the surface streamline.

2. Equation Developed

As is well known Bernoulli’s equation for steady frictionless flow can be viewed as an energy balance with a collection of kinetic and potential energy terms. Therefore it can be written down at once with the new term put in:

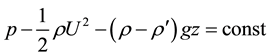(1)

where p is pressure, U is flow speed, g is acceleration of gravity and and are the different densities of two fluids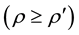. Equation (1) applies along a streamline. On the LHS of (1) the third term added in is a potential energy quantity. A visual image that may be helpful in reading (1) is to consider a heavier fluid falling vertically and accelerating through a lighter fluid, where z is the vertical coordinate. Alternatively (3) can be derived in the normal way from the fluid dynamic equations of motion.

For a fluid falling under reduced gravity the speed is given by

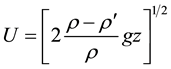(2)

Which becomes familiar when the smaller of the two densities goes to zero (i.e. the water faucet problem). Combining (2) and (1) produces two alternate forms of the Bernoulli equation for this problem:

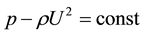(3)

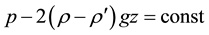
The first equation of (3) looks similar to the normal Bernoulli one except that the factor of 1/2 is missing that usually multiplies the velocity term. For example, for a given increase in speed along a streamline (3) predicts twice the decrease in pressure compared to the non-accelerated case. On the other hand, the second equation of (3) looks like a “reduced” hydrostatic pressure law except for the factor of 2 in the gravity term.

3. Discussion

Is the above development an academic exercise or does the possibility of a practical application exist? There is considerable doubt about this concerning the tornado, which is almost outside the bounds of scientific study, at least experimentally, since the magnitude of the horizontal winds at ground level are so destructive of instrumentation. However, if it turns out that at the core of a tornado relatively cool dry air falls down from higher to lower elevation through warm humid air, then a partial application might eventually be made excluding the rotary motion about the core.

A different type of downward vertical motion occasionally occurs in the atmosphere which probably does not have a rotary component. Although not much is known about it, certain evidence is very clear. From southwestern Colorado a photograph was sent to me showing a circular patch in the middle of a forest where all the trees had been completely knocked down. This event may not have been witnessed by any people, but such a scar will remain visible for at least 40 years. Total area of the blown down patch of trees was much larger than the square footage of a typical house. In the future Equation (3) may be more applicable to down bursts of this sort.

Ascending motion inside the eye of a hurricane is a phenomenon with a possibility for applying Equation (3) also. Recently an hypothesis was put forward for the initiation of North Atlantic hurricanes [3] . Involved in the hypothesis is a blanket of warm dry air from the Sahara Desert that moves west over the ocean and comes to rest above a cool moist marine layer next to the sea surface. Laboratory studies have shown that water vapor diffuses significantly faster in air than heat does. Therefore, water vapor is expected to rise up into dry air and this could cause it to become gravitationally unstable. If instability does happen, upward accelerated motion will occur. Then only the sign of the density difference in (3) needs to be reversed.

4. Conclusion

When the speed is high, the pressure is lower for accelerated than non-accelerated flow in steady inviscid motion along a straight line. Bernoulli’s equation is adapted to the circumstance by adding a potential energy term. Practical applications to certain vertical motions of the atmosphere are suggested.

Cite this paper

Kern E.Kenyon, (2015) Bernoulli’s Equation with Acceleration. Natural Science,07,488-490. doi: 10.4236/ns.2015.710050

References