Journal of Modern Physics
Vol.08 No.04(2017), Article ID:74714,24 pages
10.4236/jmp.2017.84030
The Riemann Hypothesis and Emergent Phase Space
Daniel Brox
PhD Electrical Engineering, The University of British Columbia, Vancouver, Canada

Copyright © 2017 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: January 22, 2017; Accepted: March 12, 2017; Published: March 15, 2017
ABSTRACT
By interpreting multifractal L-function zero alignment as a decoherence pro- cess, the Riemann hypothesis is demonstrated to imply the emergence of classical phase space at zero alignment. This provides a conception of emergent dynamics in which decoherence leads to classical system formation, and classical system trajectories are characterized by modular forms.
Keywords:
Riemann Hypothesis, Emergent, Phase Space, L-Functions, Modular Forms

1. Introduction
Throughout the twentieth century, a preoccupation of theoretical physics has been to identify the fundamental constitutents of matter and understand how they behave. This preoccupation has led to the construction and operation of increasingly larger particle colliders with which these constituents have been studied with greater and greater precision, and ultimately, to the discovery and validation of the Standard Model of particle physics. This model stands as a testament to the efforts of many people, and some might claim it constitutes a theory of everything once a consensus is reached on how to incorporate the gravitational force [1] .
Notably, at the root of this claim, there lies a reductionist view of the natural world, born out of extensive agreement of atomic models with experiment, and the direct observation of atoms and elementary particle tracks with scanning tunneling microscopes and particle colliders. For some, this evidence is strong enough to conclude that the Standard Model of particle physics constitutes an understanding of all biology, and even consciousness in that it describes in principle all biochemical mechanisms at an atomic level. Of course, this point of view is not universal, since scientists studying natural phenomena whose features of interest are not explained by atom-scale models may draw different conclusions, and regard such claims about human understanding as scientific overreach.
Interestingly, despite the many successes of quantum physics, there are basic theoretical questions surrounding it that remain unresolved. For instance, there is no entirely satisfactory explanation for how a measured quantum system collapses into an observable state. Secondly, though often taken for granted, it is a feature of all closed quantum systems that they undergo unitary evolution in time, because the eigenvalues of the time evolution operator are complex numbers lying on a circle of unit radius. This time evolution operator is deter- mined by the interaction and kinetic energies of a configuration of particles in space, and its success as a descriptor of atomic physical systems provides the theoretical basis for reductionism.
Given this situation, the purpose of this paper is to apply number theory to investigate the possibility that non-unitary evolution is the prime mover driving physical change. Our investigation proceeds via the study of open quantum systems which exhibit non-unitary evolution in time. From a conventional perspective, this non-unitary evolution, known as decoherence, or state mixing, is a consequence of unitary evolution of the open quantum system and its en- vironment considered as a whole. However, in this paper we’ll present a different point of view, from which quantum unitary evolution emerges as a special limit of non-unitary evolution.
In terms of layout, Chapters 2 - 4 outline research interests that motivated this work. For example, understanding how the physics of open quantum systems may be relevant to the workings of biological systems intricately coupled to their environment is discussed. Switching modes, Chapter 5 introduces the theory of solitary waves, and Chapter 6 elaborates on this discussion, introducing tau functions, modular forms and L-functions. Using these ideas, Chapter 7 introduces an alignment process analogous to state mixing that leads to the emergence of quantum unitary evolution and classical phase space, and a conjecture is made about how this emergence relates to the Standard Model. Chapter 8 concludes with a summary of results, and explains why they are of scientific interest.
2. Time and Space: Continuous or Discrete?
Classical physical theories such as electrodynamics describe physical systems as configurations of particles and fields. In these theories, a particle such as an electron or proton is idealized as a point in three dimensional space, as shown in Figure 1, and electric and magnetic fields are time dependent spatial vectors determining the direction of particle motion. For consistency with experimental observation, the real time evolution of the spatial configuration of particles and fields should obey Maxwell’s equations [2] . These equations describe a dynamic interplay between particles and fields whereby the manner in which the fields
Figure 1. Classical model of particles moving in continuous time and space.
influence particle motion and particle motion influences fields are taken into account simultaneously.
Importantly, Maxwell’s equations are differential equations describing smooth evolution of particle and field configurations in time and space. Mathematically, this relies on the assumption that time and space dimensions are coordinatized by 4 real numbers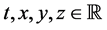 . Physically, this is interesting, because it is not clear that particle motion in time and space is truly continuous. For instance, rather than being a continuum, we can imagine that time and/or space consists of a discrete lattice of points so finely placed that discontinuous motion of particles is impossible to detect. In this event, Maxwell’s equations could arise as approximations of underlying difference equations on the lattice, and we would be unable to discern the discrete quality of time and/or space.
. Physically, this is interesting, because it is not clear that particle motion in time and space is truly continuous. For instance, rather than being a continuum, we can imagine that time and/or space consists of a discrete lattice of points so finely placed that discontinuous motion of particles is impossible to detect. In this event, Maxwell’s equations could arise as approximations of underlying difference equations on the lattice, and we would be unable to discern the discrete quality of time and/or space.
Interestingly, this issue is not particular to classical electrodynamics, but persists generally in classical and quantum mechanical descriptions of Nature, where we can similarly imagine the differential equations describing physical systems in time and space are approximations of underlying difference equations. This situation is not entirely satisfying, because it leaves us ignorant as to whether time and space are continuous, discrete, or better understood from a different point of view.
3. Mechanics of Physical Systems
3.1. Classical Systems
In classical mechanics, a point particle constrained to move in one dimension is described by its position and momentum at any given moment in time. That is, assuming its position and momentum are coordinatized by real numbers , the description of its motion is given by assigning time dependence to these coordinates, making them functions
, the description of its motion is given by assigning time dependence to these coordinates, making them functions 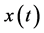 and
and  of time
of time . Geometrically, this assignment results in time flow of the point
. Geometrically, this assignment results in time flow of the point 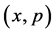 in the plane
in the plane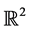 . Similarly, for more complicated physical systems consisting of
. Similarly, for more complicated physical systems consisting of 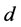 point particles moving in one dimension, the collective system motion is described by the motion of a point
point particles moving in one dimension, the collective system motion is described by the motion of a point 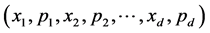 in a hyperdimen- sional Euclidean space
in a hyperdimen- sional Euclidean space 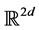 parameterizing the positions and momenta of all particles in the system simultaneously. This higher dimensional space in which the entire system is treated as a single point is known as a classical phase space, and the vector field directing real time evolution of this point is known as a Hamiltonian vector field. The components of this vector field are determined by a classical Hamiltonian function
parameterizing the positions and momenta of all particles in the system simultaneously. This higher dimensional space in which the entire system is treated as a single point is known as a classical phase space, and the vector field directing real time evolution of this point is known as a Hamiltonian vector field. The components of this vector field are determined by a classical Hamiltonian function  defining the system’s energy [3] .
defining the system’s energy [3] .
Practically speaking, classical mechanics is well equipped to model closed physical systems, but not open systems. For instance, to usefully model cell division with classical mechanics, we are forced to somewhat arbitrarily partition the phase space of the cell and its environment together into separate cell and environmental phase spaces. That is, it is necessary to identify all of the particles playing a role in the cell’s division, and model this division as a process governed by interactions between these particles and some average environmental effect. Unfortunately, this description does not allow for unpredictable variations in temperature, pressure, or particle exchange between the cell interior and exterior, making precise modeling impossible. Moreover, in the case of cell division, these sources of imprecision are complicated by the extremely large number of particles involved in all phases of the process. This situation is illustrated in Figure 2 [4] , where high resolution images of three phases of cell division are shown.
Another interesting feature of classical mechanics is that system time evolution tends to be disordered. That is, classical system trajectories are generically chaotic, filling entire  -dimensional regions of the phase space
-dimensional regions of the phase space , while lower dimensional trajectories expressing some degree of order are determined by Hamiltonian vector fields satisfying special symmetry constraints [5] . This is interesting, because we generally think of biological systems as maintaining a high degree of order in the presence of an ever changing environment, suggesting there may be some intricate maintenance of order inherent in the interplay between system and environmental variables that is not captured by the classical modeling approach.
, while lower dimensional trajectories expressing some degree of order are determined by Hamiltonian vector fields satisfying special symmetry constraints [5] . This is interesting, because we generally think of biological systems as maintaining a high degree of order in the presence of an ever changing environment, suggesting there may be some intricate maintenance of order inherent in the interplay between system and environmental variables that is not captured by the classical modeling approach.
Finally, as a technical point, we note that while classical physics describes the real time evolution of fields as well as particles, there is no clear choice of configuration space in which field configurations flow like there is for particles. That is, if we ask what the set of physically realizable electric field configurations across the Euclidean space 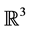 is at some point in time, it is not obvious how to rigorously define this set. This is because the configuration space of the electric field is a space of functions from
is at some point in time, it is not obvious how to rigorously define this set. This is because the configuration space of the electric field is a space of functions from  to itself, and it is not obvious what mathe-
to itself, and it is not obvious what mathe-
Figure 2. High resolution image of cell division [4] , demonstrating why it is difficult to partition the phase space into system and environment.
matical criteria these functions should satisfy, or how to define a probability measure on this function space as necessary to describe the statistical behavior of the field in a thermal environment.
3.2. Quantum Systems
In quantum mechanics, the Heisenberg uncertainty principle states that precise position and momentum coordinates of particles are not simultaneously specifiable. Consequently, the mathematical description of quantum particles is given in terms of position or momentum probability distribution functions, not points in phase space, and closed multi-particle systems are described by wave functions of position or momentum coordinates that evolve in time according to the dictate of a Hamiltonian energy operator rather than a Hamiltonian vector field. This operator defines real valued system energy levels, and unitary evolution of wave functions according to Schrodinger’s equation. A similar mathematical formalism describes the time evolution of quantum fields, though computations are typically performed via evaluation of Feynman diagrams rather than directly solving the Schrodinger equation. As in the case of classical mechanics, quantum mechanical modeling of biological systems with environ- mental interactions is awkward when system and environmental variables are difficult to distinguish.
One crucial difference between quantum and classical descriptions of physical systems is the effect of measurement on these systems. This difference stems from the description of quantum particles as wave functions spread out over all of position space, whereby a particle-like quality of these entities is only realized upon measurement with an experimental apparatus. The prototypical example of this is the observation of particle position on a detecting screen in Young’s double slit experiment, in which observation of classical particle-like behavior absent in the mathematical description of wave functions is referred to as wave function collapse. Intuitively, one expects collapse to be a consequence of the interaction of a quantum mechanical system with its measurement apparatus, as required to observe the system. For this reason, collapse and our experimental observation of particles is inherently related to the behavior of open quantum systems. Philosophically, this is important, because it leaves open the possibility that our classical notion of “particle” emerges from a description of open quantum systems in which this notion is not fundamental.
4. Mixing and Measuring
Turning to the study of open quantum systems, it is common to use density matrices instead of wave functions to describe system evolution, because this formalism can account for environmentally induced state transitions [6] . Typically, this evolution is described using a master’s equation derived from the Hamiltonian evolution 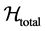 of the open system and its environment together:
of the open system and its environment together:

by averaging over environmental degrees of freedom. The upshot of this description is that most pure quantum states of the open system are unstable, and evolve into statistical mixtures of pointer states that are stable against further mixing [7] . These pointer states are clearly defined when the system 



As mentioned, in the commuting case, state mixing results in the off-diagonal decay of the system density matrix written in a pointer state basis. Furthermore, in the event the environment acts as a heat bath at thermal equilibrium, the diagonal weights of the density matrix evolve towards an equilibrium distribu- tion in which each pointer state is weighted by a Boltzmann factor. This process, known as relaxation, typically takes place on timescales much longer than dephasing. Figure 4 shows a rough conceptualization of the state mixing process, in which dephasing eliminates the off-diagonal elements of the density matrix, and relaxation adjusts the pointer state weights along the diagonal from 

Importantly, evolution of a system density matrix into a statistical mixture of pointer states is mathematically distinct from the projection of a density matrix into a pure quantum state that occurs with measurement of the system. This projection, known as collapse, has the effect of restoring a pure quantum state that can once again evolve into a mixed state upon environmental interaction. From a theoretical point of view, it is understood why measured quantum sys-
Figure 3. Schematic illustration of an open quantum system and its environment that together constitute a closed quantum system.
Figure 4. Non-unitary density matrix evolution in pointer state basis. Dephasing eliminates off diagonal elements, and relaxation adjusts diagonal weights to equilibrium levels.
tems evolve into statistical mixtures of pointer states, because they are necessarily open to their environment. However, it is not clear how collapse into a single observable outcome occurs, and this absence of clarity lies at the heart of the measurement problem.
To obtain a classical approximation of a quantum system, the Wigner trans- form can be applied to the quantum system density matrix to construct a classical trajectory distribution on classical phase space. Depending on the mixed state represented by the density matrix, this construction is not always physically meaningful. However, in the event the density matrix represents a statistical mixture of spatially localized pointer states, applying the Wigner transform yields a time varying probability distribution on classical phase space that describes the likelihood of the system taking different classical trajectories. Conventionally, such spatially localized pointer states are called coherent states.
Remarkably, there are similarities between the theory of open quantum systems and number theory, whereby commuting 






5. Driving
To explain how the alignment process described in Chapter 7 is driven, we turn to the theory of solitary waves (i.e. solitons). This theory is useful to us because differential equations describing the motion of solitons define geometric objects called Riemann surfaces 

To begin explaining this, let’s take a look at the Korteweg de-Vries (KdV) equation, the prototypical soliton equation describing non-dispersive propaga- tion of waves in shallow water [8] . This equation is the nonlinear differential equation:

where 

in differential operators 




This Lax equation has time independent solutions of the form:

where 




or:

using the relationship between 







To better understand the relationship between the KdV equation and elliptic curves, let’s assume the differential operators 



In this event, according to a result of Burchnall and Chaundy, the operators 





of degree 3 in 


Figure 5. Solitons form in long period limits 
More generally, we can construct soliton equations:

solved by functions























in which the differential operators 







satisfying:

Similarly, fixing


satisfying:

Because conjugation of an operator does not change its eigenvalues, the eigenvalues of the operators 



Assuming the differential operators 



Figure 6. Covering of the Riemann sphere 

curve. Moreover, since there are 








whose 









whose solution 








To introduce these ideas, let’s imagine that a state mixing process takes place in the Q-analog limit






defines a differentiation operator in 


More specifically, upon substituting


where 





Locally, 









Figure 7. Poles of local solutions 


Remarkably, these branches resemble centromeres aligning chromosomes at metaphase, as shown in Figure 2.
6. Meta-Physics
This chapter introduces tao functions, explaining their relationship to soliton equations, theta functions, and modular forms. It also provides a brief introduc- tion to L-functions and the Riemann hypothesis, as necessary for understanding the discussion in Chapter 7. Note that tao functions are more commonly known as tau functions, but the name tao, meaning great waves, has been adopted here to avoid confusion with the modular parameter
6.1. Tao Functions
Tao functions generate solutions to soliton equations. For example, a tao function 

generates solutions to KdV Equation (3) via the relation:

For example, the Jacobi theta function appearing in Equation (9) is an example of a tao function solving Equation (23).
More generally, soliton equations of type (12) with 


as:

where 









Tao functions can also be defined when

whenever they solve the bilinear KP equation:

Once again, Riemann theta functions provide viable examples of tao func- tions.
6.2. Modular Forms
Modular forms are functions 



for some discrete subgroup 












Demonstrating that the number of independent modular forms increases with weight 


Other examples of weight 
Figure 8. Image of the real part of a modular form called the modular discrminant 

in which


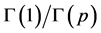








This modular function satisfies a polynomial equation whose coefficients depend on the j-invariant



In physics, modular functions arise as renormalization flow limits of Ising model partition functions [15] . To understand this, recall that the Ising energy 




Summing over all possible spin configurations, this Ising energy generates a quantum partition function

that depends on the parameters 

Assuming the spins 



where 

The renormalization transformation associated with this decimation and rescaling is:


and this transformation gives rise to regular and chaotic flows below and above the curve



Formally, under repeated iteration of renormalization transformation (35), the partition function 








6.3. L-Functions
Artin L-functions 


in that they are expressible as infinite products over primes. Technically, we can associate an L-function with each representation 









where 


Figure 9. One dimensional Ising model renormalization flow.
Interestingly, it is conjectured that all non-trivial zeros of Artin L-functions lie along the critical line
known as the generalized Riemann hypothesis, has close ties with physics. For example, it has been proposed that alignment of the Riemann zeros is related to the Hermiticity of quantum operators and/or the alignment of Yang-Lee zeros of Ising model partition functions [20] [21] . In addition, it has been conjectured that every Artin L-function 




7. Emergence
In this chapter, our goal is to explain how the Riemann hypothesis is related to the emergence of classical phase space, and how this emergence is driven. To this end, Figure 11 shows the zeros of a Langlands L-function 
Figure 10. An illustration of the Riemann hypothesis: the Riemann zeta function does not have any non-trivial zeroes lying off the critical line 
Figure 11. Conjecturally, the zeros of a Langlands L-function 

graphically projected onto the surface of a Riemann sphere. Conjecturally, these critically aligned zeros act as attractors for a multifractal L-function zero flow carrying the zeros of 





To understand the relationship between zero flow and state mixing, let’s assume the automorphic waveforms 

on the adelic group 












To relate multifractal zero alignment to the emergence of classical phase space, we’d like to associate zero flows with geometric objects 




the Galois representation 






acted on by the discrete group


Figure 12.Emergence of the 2-dimensional phase space
In the special case














Geometrically, we can understand classical system formation in 



With this in mind, let’s consider a variant of twistor theory in which twistors are replaced by continuous paths in 

of 






Visually, we can imagine system formation in 


Figure 13. (top) Resonant splitting of a KAM torus [28] . (middle) Dual spirals direct the the trajectory of a classical system formed in
dence in a plane 


Because the particle trajectory in Figure 13-middle is a vortex, we may suspect it has a characteristic period of rotation. In fact, up to anomalous factors, the Rogers-Ramanujan modular forms:


are Gaussian hypergeometric functions defining periods of classical rotational motion [30] . For this reason, we can imagine one of these periods characterizes the rotation of the golden particle around the central axis in Figure 13-middle. More generally, we’ll conjecture the rotational motion of the system formed in 







Intuitively, this conjecture is motivated by noting hypergeometric tao functions are combinatorial generating functions of signed Hurwitz numbers [31] . That is, the 
















To understand this in greater detail, let’s imagine Equation (18) is integrated in the complex 




into matrices 



and Equation (47) is the Riemann-Hilbert factorization of 


solving KZ differential equations, whose ratio is a unitary character of the Virasoro algebra [36] . Such characters are quantum partition functions associat- ed with unitary representations of loop groups, and conjecturally, emerge in conjunction with unitary mixing. This situation is illustrated schematically in Figure 14, in which the flow and crash operators are indicated by blue arrows, and unitary representations of loop groups emerge in conjunction with the pointer states
Algebraically, the determinant of Riemann-Hilbert factorization (47) is a relation between scattering amplitudes in the Hopf algebra of Feynman diagrams


Figure 14. Artistic rendering of flow and crash operators. https://permies.com/t/44266/Wood-Heat-DIY-Rocket-Mass
Figure 15. Artistic rendering of a “diamond’’ fan. https://www.quantamagazine.org/20130917-a-jewel-at-the-heart-of-quantum-physics/
Interestingly, there are cases in which the the aforementioned relation between scattering amplitudes is a 



satisfied by













As an example, let’s assume



generate a modular function field of degree 3 at 

and the ratio:

is a cyclotomic unit of degree 3 in 


Figure 16. Modular deformation of a limit set defined by a Fuschian group 

is conjectured to have a continued fraction expansion for an appropriate choice of the partition function 
8. Conclusions
Blending ideas from math and physics, this paper suggests state mixing is the fundamental process underlying the time evolution of physical systems. Formally, this is achieved by replacing quantum density matrices with multifrac- tal L-functions



Physically, the results of this paper are of interest because they highlight a connection between open quantum systems and number theory. Specifically, commuting system and environmental interaction operators 





Mathematically, the drive towards multifractal zero alignment is explained using the theory of solitons. This is done by identifying the root space 



Outside the realm of pure science, the results of this paper may also have real world applications. For example, as described, unitary mixing instills emergent classical systems with a balance between ordered and chaotic behavior that may be relevant to understanding the presence of self organized criticality in Nature [42] . Should this prove to the case, areas of pure mathematics that have traditionally been regarded as the preoccupation of ex-centrics may find application across scientific disciplines.
Cite this paper
Brox, D. (2017) The Riemann Hypothesis and Emergent Phase Space. Journal of Modern Physics, 8, 459-482. https://doi.org/10.4236/jmp.2017.84030
References
- 1. Lisi, G. (2007) An Exceptionally Simple Theory of Everything.
- 2. Feynman, R., Leighton, R. and Sands, M. (1964) The Feynman Lectures on Physics. Vol. 2, Addison-Wesley, Reading.
- 3. Arnold, V. (1989) Mathematical Methods of Classical Mechanics. Springer Science & Business Media, Berlin.
https://doi.org/10.1007/978-1-4757-2063-1 - 4. Vassilis, R., Misteli, T. and Schmidt, C. (2010) Trends in Cell Biology, 20, 503-506.
https://doi.org/10.1016/j.tcb.2010.06.008 - 5. Gutzwiller, M. (2013) Chaos in Classical and Quantum Mechanics. Vol. 1, Springer Science & Business Media, Berlin.
- 6. Mahler, G. and Rainer, W. (1998) VLSI Design, 8, 191-196.
https://doi.org/10.1155/1998/28384 - 7. Zurek, W. (2003) Reviews of Modern Physics, 75, 715.
- 8. Clifford, G., Greene, J., Kruskal, M. and Miura, R. (1967) Physical Review Letters, 19, 1095.
- 9. Kasman, A. (2010) Glimpses of Soliton Theory. AMS, Providence.
https://doi.org/10.1090/stml/054 - 10. Olivier, B., Bernard, D. and Talon, M. (2003) Introduction to Classical Integrable Systems. Cambridge University Press, Cambridge.
https://doi.org/10.1017/CBO9780511535024 - 11. Sauloy, J. (2004) Séminaires et Congrès, 14, 249-280.
- 12. Moser, J. (1979) American Scientist, 67, 689-695.
- 13. Koblitz, N. (2012) Introduction to Elliptic Curves and Modular Forms. Vol. 97, Springer Science & Business Media, Berlin.
- 14. Duke, W. (2005) Bulletin of the American Mathematical Society, 42, 137-162.
https://doi.org/10.1090/S0273-0979-05-01047-5 - 15. Kadanoff, L. (2013) Studies in History and Philosophy of Science Part B: Studies in History and Philosophy of Modern Physics, 44, 22-39.
https://doi.org/10.1016/j.shpsb.2012.05.002 - 16. Dolan, B. (1995) Physical Review E, 52, 4512.
- 17. Schumayer, D. and Hutchinson, D. (2011) Reviews of Modern Physics, 83, 307.
- 18. Cogdell, J. (2007) L-Functions and Non-Abelian Class Field Theory, from Artin to Langlands.
- 19. Bombieri, E. (2006) Problems of the Millennium: the Riemann Hypothesis. Institute for Advanced Study, Princeton, 106.
- 20. Montgomery, H. (1973) Proceedings of Symposia in Pure Mathematics, 24, 181-193.
https://doi.org/10.1090/pspum/024/9944 - 21. Bena, I., Droz, M. and Lipowski, A. (2005) International Journal of Modern Physics B, 19, 4269-4329.
https://doi.org/10.1142/S0217979205032759 - 22. Borel, A. and Casselman, W. (1979) Automorphic Forms, Representations, and L-Functions. Vol. 2, American Mathematical Society, Providence.
https://doi.org/10.1090/pspum/033.1 - 23. Lapidus, M. (2008) In Search of the Riemann Zeros: Strings, Fractal Membranes and Noncommutative Spacetimes. American Mathematical Society, Providence.
https://doi.org/10.1090/mbk/051 - 24. Bernatska, J. and Holod, P. (2007) Geometry and Topology of Coadjoint Orbits of Semisimple Lie Groups. Proceeding of 9th International Conference on Geometry Integrability and Quantization, Varna, 8-13 June 2007, 146-166.
- 25. Harris, M. (2016) Automorphic Galois Representations and the Cohomology of Shimura Varieties.
https://webusers.imj-prg.fr/~michael.harris/2014.pdf - 26. Penrose, R. (1999) Chaos, Solitons & Fractals, 10, 581-611.
https://doi.org/10.1016/S0960-0779(98)00333-6 - 27. Vilenkin, A. (1982) Physics Letters B, 117, 25-28.
https://doi.org/10.1016/0370-2693(82)90866-8 - 28. Preskill, J. (2015) Introduction to Dynamical Systems and Hamiltonian Chaos.
http://www.theory.caltech.edu/~preskill/ph106b/106b-chaos-part4.pdf - 29. Edwards, L. (1993) The Vortex of Life. Nature’s Patterns in Space and Time. Floris Books, Edinburgh.
- 30. Kontsevich, M. and Zagier, D. (2001) Periods.
- 31. Harnad, J. and Orlov, A. (2015) Communications in Mathematical Physics, 338, 267-284.
https://doi.org/10.1007/s00220-015-2329-5 - 32. Milanov, T. and Ruan, Y. (2011) Gromov-Witten Theory of Elliptic Orbifold P1 and Quasi-Modular Forms.
- 33. Varchenko, A. (1990) Multidimensional Hypergeometric Functions in Conformal Field Theory, Algebraic K-Theory, Algebraic Geometry. Proceedings of the International Congress of Mathematicians, Vol. 1, Kyoto, 21-29 August 1990, 281-300.
- 34. Connes, A. and Kreimer, D. (2001) Communications in Mathematical Physics, 216, 215-241.
https://doi.org/10.1007/PL00005547 - 35. Kac, V. (1992) Modular Invariance in Mathematics and Physics. Proceedings of the AMS Centennial Symposium, Vol. 337, Providence, 8-12 August 1988, 337-350.
- 36. Schechtman, V. and Varchenko, A. (1990) Letters in Mathematical Physics, 20, 279-283.
https://doi.org/10.1007/BF00626523 - 37. Arkani-Hamed, N., Bourjaily, J., Cachazo, F., Goncharov, A., Postnikov, A. and Trnka, J. (2012) Scattering Amplitudes and the Positive Grassmannian.
- 38. Atiyah, M. and Bott, R. (1984) Topology, 23, 1-28.
https://doi.org/10.1016/0040-9383(84)90021-1 - 39. Griffin, M., Ono, K. and Warnaar, O. (2016) Duke Mathematical Journal, 165, 1475-1527.
https://doi.org/10.1215/00127094-3449994 - 40. Mumford, D., Series, C. and Wright, D. (2002) Indra’s Pearls: The Vision of Felix Klein. Cambridge University Press, Cambridge.
https://doi.org/10.1017/CBO9781107050051 - 41. Berndt, B. (2001) Flowers Which We Cannot Yet See Growing in Ramanujan’s Garden of Hypergeometric Series, Elliptic Functions, and q’s. Special Functions 2000: Current Perspective and Future Directions. Springer, Dordrecht, 61-85.
- 42. Bak, P. (2013) How Nature Works: The Science of Self-Organized Criticality. Springer Science & Business Media, Berlin.


















