Journal of Modern Physics
Vol.06 No.15(2015), Article ID:61796,7 pages
10.4236/jmp.2015.615221
On the Relationship between the Geometry of Space-Time and Its Information Content
Andreas E. Schlatter
Burghaldeweg 2F, Küttigen, Switzerland

Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).


Received 3 November 2015; accepted 6 December 2015; published 9 December 2015

ABSTRACT
We use an information-consistency or, equivalently, a thermodynamic equilibrium condition to derive Einstein’s equations, both in case of a gravitational and an electrostatic field. We thus show the equivalence of an information-theoretic and a thermodynamic viewpoint in the analysis of the geometry of space-time.
Keywords:
Entropy, Quantum Gravity, Information, Einstein Equations

1. Introduction
In recent years, we have seen the demonstration of profound relationships between the geometry of space-time and thermodynamics or quantum information [1] [2] . In all cases it results in a derivation of Einstein’s equations as a consequence either of a thermodynamic equilibrium condition at a causal horizon, or of counting the number of possible flips in a space-time volume and changing the area of a space-like surface by a suitable multiple of that number. In this paper we are going to combine these approaches to show that Einstein’s equations actually follow from an information consistency condition under a thermal evolution. In addition we will also see that it does not make a difference whether we consider a mass and its gravitational field or a charge and its Coulomb and (induced) magnetic fields. Both sources of energy influence the geometry of space-time by the same mechanism.
There has been recent work done around the concept of thermal time and its relations to other, geometric time-flows [3] -[7] . We will make the thermal flow of a physical system, represented by a density operator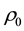 , the starting point of our considerations.
, the starting point of our considerations.
Process Velocity
Let there be a physical system with density operator
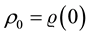 and a Hamiltonian H. The cosine of the angle between the initial state
and a Hamiltonian H. The cosine of the angle between the initial state
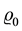 and any further state
and any further state
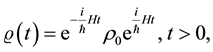 under the Schroedinger evolution is given by the scalar product
under the Schroedinger evolution is given by the scalar product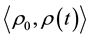 . A future state
. A future state
 is called distinguishable from
is called distinguishable from , if it is orthogonal, i.e.
, if it is orthogonal, i.e.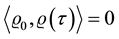 . The minimal time
. The minimal time
 needed for
needed for
 to evolve into an orthogonal state is given by the Margolus-Levitin bound [8] ,
to evolve into an orthogonal state is given by the Margolus-Levitin bound [8] ,
 (1)
(1)
Here





considers passing through a long sequence of orthogonal states, then the minimal time turns out to be

We now suppose that the system is part of a heat bath




In this equation


Expression (3) further allows us to define a very general equilibrium condition between two systems [6] . Given two systems







Note that condition (4) can also be viewed as a consistency condition for two sub-systems of an overall system in thermal equilibrium, as indicated in [9] . It is important that with a relation like (4) we actually consider classical information in form of distinguishable states, which appears natural, if a macroscopic theory like relativity is in scope.
2. Information and Space-Time
Equation (4) is a relational expression, which we now apply to the situation of observers in a four-dimensional space-time. A space-time allows an equilibrium, if it is static [6] [10] , i.e. if its local line-element does not explicitly depend upon the time-coordinate and is therefore of the form

From Equation (5) it is immediately evident that for two observers along their time-like world-lines with



This is the well-known Tolman-Ehrenfest effect [10] . This elegant derivation already indicates the usefulness of definition (4).
We now consider a situation, where the space-time is actually a flat Minkowski space-time and the observers move with constant acceleration. To generate a static environment we work in the Rindler co-moving coordinate frame and hence in the Rindler-wedge. In Rindler-coordinates


For the constant acceleration a there holds
For an observer along a time-like world-line with



By a calculation to normalize states, the value chosen for the constant is


2.1. Gravity
Our next step is now to consider a test particle of mass m at a distance R from a central object of mass M at a point


Along a small segment


Together with the definition of the Planck-length


An expression, which originally served the purpose to count orthogonal states in equilibrium, thus turns into an energy-entropy relationship of the form

If we again use (8) to express temperature


Note that, if energy is quantized,


In a general Lorentz space-time relation (13) is still locally valid, since we can in a small neighborhood around





Let us shortly summarize before we go on. Our formula to count the (maximal) number of orthogonal states (bits) per unit-time under a thermal evolution (3), together with a consistency or equilibrium definition across different observers (4), applied to a test particle in a gravitational field in the Newtonian approximation (inverse-square law), has lead to Equation (13) and Equation (14) which allows us to formally identify entropy with a surface area and establish an equilibrium relation with total energy. This is quite remarkable and delivers the preconditions to follow the approach in e.g. [1] [12] . We start with rewriting Equation (14)

and for completeness sake sketch the steps.
We may chose R in such a way,5 that in a small neighborhood of any space-like 2-surface P around the space-time point












For the variation of the horizon area there holds with


Now, the equation of geodesic deviation, applied to the null geodesic congruence generating the horizon, leads to the Raychaudhuri equation [13] .

where







Now, by Equation (15) there holds


Therefore

This holds for all null

Since




These are Einstein’s equations with a cosmological constant
2.2. Electrostatics
It is interesting to see what happens in the similar situation of another inverse square law, namely when we consider the electrostatic (Coulomb) force between a test particle of mass m and charge q at a distance R from a central charge Q. The equivalent to the to the gravitational constant G is the electric field (Coulomb) constant


the case of gravity, where the mass m drops out of the equations and hence Equation (23) is universally valid even for massless particles, the mechanism in electrostatics will be test particle-dependent. In analogy of Equation (10) we get

The quantity


Multiplying both sides with


This is the analogue to Equation (11). By using again the Unruh relation for temperature

This equation is the analogue to Equation (13) with





Due to the fact that the formulation of electrodynamics happens also in Minkowski-space, we can follow the argument in paragraph 2.1. Note that a dynamic change of charge

late the flux through the horizon we will need the energy momentum tensor







3. Conclusions
In the last couple of years, we have seen a number of different ways to derive Einstein’s equation by means of the holographic principle and thermodynamic equilibrium assumptions [1] [14] . A flux of energy through a (local) causal horizon causes some kinds of gravitational lensing effect in order to maintain the thermodynamic equilibrium. It seems that the other direction of reasoning, i.e. to derive the holographic principle from Einstein’s equations, is much harder and the principle lies deeply hidden in the structure. Another approach starts from an information-theoretic basis, counts the maximal number of flips in a space-time volume and then carves out a corresponding number of area-elements from a horizon surface to cause it to curve [2] . The two approaches seem little related at first.
In this paper we combine the two perspectives insofar, as we follow the thermodynamic arguments to derive Einstein’s equations from the holographic principle but, instead of assuming it to start with, we derive it by an information theoretic approach. We define a consistency relation across different observers, which observe different systems in thermal equilibrium, by demanding that they all agree on the same maximal number of orthogonal states (flips), which their system can pass in thermal evolution per unit of (proper) time. We apply this definition to observers in a Rindler-frame and if acceleration happens because of a gravitational or an electrostatic force, then the observers identify the available information or entropy with a quotient of the horizon area and some fundamental area units, the Planck or Compton spheres.
In fact expression (10) is very instructive and can be interpreted in the following way: if a falling observer feels an acceleration



space-time and has an elementary unit of
Cite this paper
Andreas E.Schlatter, (2015) On the Relationship between the Geometry of Space-Time and Its Information Content. Journal of Modern Physics,06,2184-2190. doi: 10.4236/jmp.2015.615221
References
- 1. Jacobson, T. (1995) Physical Review Letters, 75, 1260-1263.
http://dx.doi.org/10.1103/PhysRevLett.75.1260 - 2. Lloyd, S. (2012) arXiv:1206.6559 [gr-qc]
- 3. Connes, A. and Rovelli, C. (1994) Classical and Quantum Gravity, 11, 2899-2917.
http://dx.doi.org/10.1088/0264-9381/11/12/007 - 4. Martinetti, P. and Rovelli, C. (2003) Classical and Quantum Gravity, 20, 4919-4931.
http://dx.doi.org/10.1088/0264-9381/20/22/015 - 5. Smerlak, M. and Rovelli, C. (2011) Classical and Quantum Gravity, 28, Article ID: 075007.
http://dx.doi.org/10.1088/0264-9381/28/17/178001 - 6. Haggard, H. and Rovelli, C. (2013) Physical Review Letters, 87, Article ID: 084001.
- 7. Schlatter, A. (2015) Physics Essays, 28, 296-299.
http://dx.doi.org/10.4006/0836-1398-28.3.296 - 8. Margolus, N. and Levitin, L. (1998) Physica D, 120, 188-195.
http://dx.doi.org/10.4006/0836-1398-28.3.296 - 9. Menicucci, N., Olson, S. and Milburn, G. (2014) arXiv:1108.0883 [gr-qc]
- 10. Tolman, R.C. and Ehrenfest, P. (1930) Physical Review, 36, 1791-1798.
http://dx.doi.org/10.1103/PhysRev.36.1791 - 11. Jacobson, T. and Parenti, P. (2003) Foundations of Physics, 33, 323-348.
http://dx.doi.org/10.1023/A:1023785123428 - 12. Unruh, W.G. (1976) Physical Review D, 14, 870-892.
http://dx.doi.org/10.1103/PhysRevD.14.870 - 13. Raychaudhuri, A.K. (1955) Physical Review, 98, 1123-1126.
http://dx.doi.org/10.1103/PhysRev.98.1123 - 14. Verlinde, E. (2011) Journal of High Energy Physics. arXiv:1001.0785 [hep-th]
NOTES
1Expression (1) assumes that the lowest eigenvalue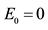 , otherwise there holds
, otherwise there holds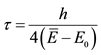 .
.
2Note that at this place the definition of temperature as the average energy per bit of information,
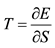 , appears very natural.
, appears very natural.
3For more information on Rindler-coordinates see e.g. [11] .
4Since the equilibrium condition (4) is relational, there is always a gauge freedom of multiplication by a constant, non-zero function.
5Typically R will be small and hence the accelereation gR big.
6We assume that all energy is transported through the horizon by a matter flux.
7Since we have tacitly assumed in the derivation, that the groundenergy state of our systems satisfies






