Applied Mathematics
Vol.05 No.19(2014), Article ID:51203,6 pages
10.4236/am.2014.519279
Amenability and the Extension Property
Antoine Derighetti
EPFL SB-DO, MA A1 354, Station 8, CH-1015 Lausanne, Switzerland
Email: antoine.derighetti@epfl.ch
Copyright © 2014 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 4 September 2014; revised 26 September 2014; accepted 6 October 2014
ABSTRACT
Let G be a locally compact group, H a closed amenable subgroup and u an element of the Herz Figà-Talamanca algebra of H with compact support, we prove the existence of an extension of u to G, with a good control of the norm and of the support of the extension.
Keywords:
Convolution Operators, Locally Compact Groups, Abstract Harmonic Analysis, Amenable Groups

1. Introduction
Let G be a locally compact group and H a closed subgroup, this paper is concerned with the problem of extending coefficients of the regular representation of H to G. Suppose H normal in G. In 1973 [1] C. Herz proved that for
 with compact support, for every
with compact support, for every
 and for every U neighborhood of
and for every U neighborhood of
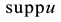 in G there is
in G there is
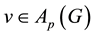 with
with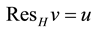 ,
,
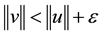 and
and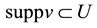 . In this work we want to treat the case of non normal subgroups. We succeed assuming that the subgroup H is amenable (Theorem 5). C. Fiorillo obtained [2] already this result assuming however the unimodularity of G and of H. But the AN part of the Iwasawa decomposition of
. In this work we want to treat the case of non normal subgroups. We succeed assuming that the subgroup H is amenable (Theorem 5). C. Fiorillo obtained [2] already this result assuming however the unimodularity of G and of H. But the AN part of the Iwasawa decomposition of
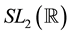 was out of reach. Even for G amenable our result is new: the case of the non-normal copy of
was out of reach. Even for G amenable our result is new: the case of the non-normal copy of
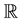 in the
in the
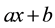 -group was also out of reach.
-group was also out of reach.
Without control of norm and support of the extension, the theorem has been obtained in 1972 by McMullen [3] . With control of the norm, but not considering the supports, the statement is due Herz [1] (see also [4] ).
2. A Property of Amenable Subgroups
We denote by
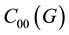 the set of all complex valued continuous functions on G with compact support. We choose a positive continuous function q on G such that
the set of all complex valued continuous functions on G with compact support. We choose a positive continuous function q on G such that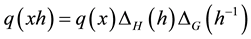 , left invariant measures on G and H and a measure
, left invariant measures on G and H and a measure
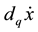 on
on
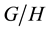 as in Chapter 8 of [5] . The following Lemma will be used in the proof of our main result. See below the steps
as in Chapter 8 of [5] . The following Lemma will be used in the proof of our main result. See below the steps
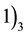 and
and
 of the proof of Lemma 2.
of the proof of Lemma 2.
Lemma 1 Let











Proof. Let












where

3. Approximation Theorem for Convolution Operators Supported by Subgroups
We refer to [7] for







We define a family of linear maps




subgroup of







Definition 1. Let






Then







Lemma 2. Let












for every


Proof. Let




symmetric neighborhood of


















Let




for every

for every







We put





By the preceding Lemma there is



is smaller than
and also smaller than
for every




1) For every




From
we obtain indeed


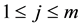

We have

But for every
and therefore
consequently


As above
taking in account that








2) For every


By the Corollary 6 of section 7.2 p.112 of [7]
Consequently
But by definition of


3) End of the proof of Lemma 2. We are now able to define the functions






Clearly



But for
hence



Theorem 3 Let











the series





for every
Proof. We choose

1) There is




for every
There are



and
for every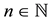


By Lemma 2 there is



and such that
for every


2) End of the proof of Theorem 3. It suffices to put



4. The Main Result
Definition 2 Let





where
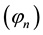




Then








Corollary 4 Let












Proof. There are sequences








is




for every
Consider an arbitrary


and
we get


The following theorem is the main result of the paper.
Theorem 5 Let











Proof. This proof is identical with the one of Proposition


















Cite this paper
AntoineDerighetti, (2014) Amenability and the Extension Property. Applied Mathematics,05,2945-2951. doi: 10.4236/am.2014.519279
References
- 1. Herz, C.S. (1973) Harmonic Synthesis for Subgroups. Annales de l'institut Fourier, 23, 91-123.
http://dx.doi.org/10.5802/aif.473 - 2. Fiorillo, C. (2009) An Extension Property for the Figà-Talamanca Herz Algebra. Proceedings of the American Mathematical Society, 137, 1001-1011.
http://dx.doi.org/10.1090/S0002-9939-08-09679-2 - 3. McMullen, J.R. (1972) Extensions of Positive-Definite Functions. Memoirs of the American Mathematical Society, 117.
- 4. Delaporte, J. and Derighetti, A. (1992) On Herz’ Extension Theorem. Bollettino dell’Unime Matematica Italiana, (7) 6-A, 245-247.
- 5. Reiter, H. and Stegman, J.D. (2000) Classical Harmonic Analysis and Locally Compact Groups. Clarendon Press, Oxford.
- 6. Derighetti, A. (2004) On Herz’s Projection Theorem. Illinois Journal of Mathematics, 48, 463-476.
- 7. Derighetti, A. (2011) Convolution Operators on Groups. Lecture Notes of the Unione Matematica Italiana, 11, Springer-Verlag, Berlin, Heidelberg.
- 8. Delaporte, J. and Derighetti, A. (1995) p-Pseudomeasures and Closed Subgroups. Monatshefte für Mathematik, 119, 37-47.
http://dx.doi.org/10.1007/BF01292767
















































