Applied Mathematics
Vol.4 No.9A(2013), Article ID:36698,6 pages DOI:10.4236/am.2013.49A003
On the Behavior of Positive Solutions of a Difference Equations System 
Institute of Systems Science and Mathematics, Naval Aeronautical and Astronautical University Yantai, Yantai, China
Email: #jwqyikeshu@163.com
Copyright © 2013 Decun Zhang et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received March 5, 2013; revised April 5, 2013; accepted April 12, 2013
Keywords: Difference Equation; System; Monotonicity; Periodicity; Oscillatory
ABSTRACT
Motivated by an open problem in the literature “Dynamics of Second Order Rational Difference Equations with Open Problems and Conjecture”, we introduce a difference equation system: 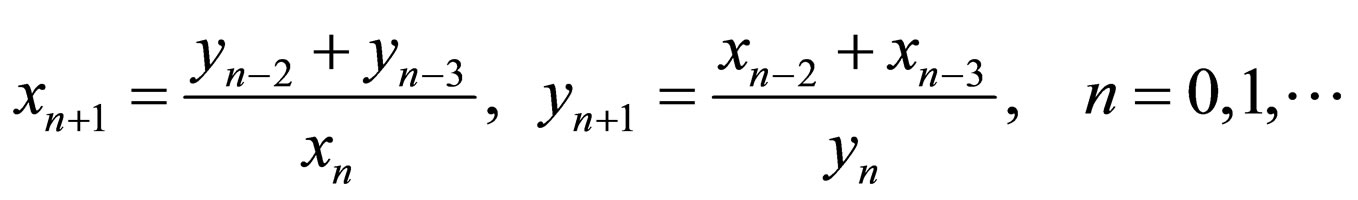 * where
* where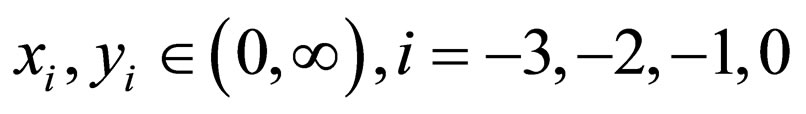 . We try to find out some conditions such that the solution of system converges to periodic solution. This model can be applied to the two species competition and population biology.
. We try to find out some conditions such that the solution of system converges to periodic solution. This model can be applied to the two species competition and population biology.
1. Introduction
In the monograph of Dynamics of Second Order Difference Equation [1], M. R. S. Kulenović and G. Ladas gave an open problem (see [1], p. 199) as following:
Open problem 11.4.8:
Determine whether every positive solutions of the following equation converges to a periodic solution of the corresponding equation:
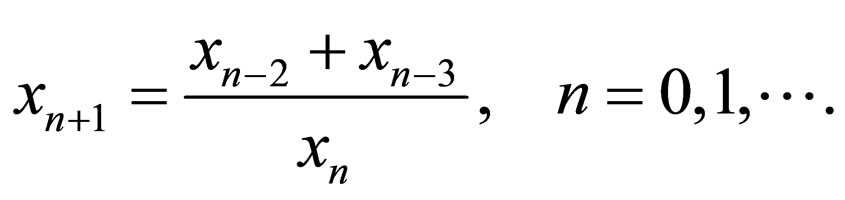 (1)
(1)
Motivated by the Open Problem, we introduce the difference equation system:
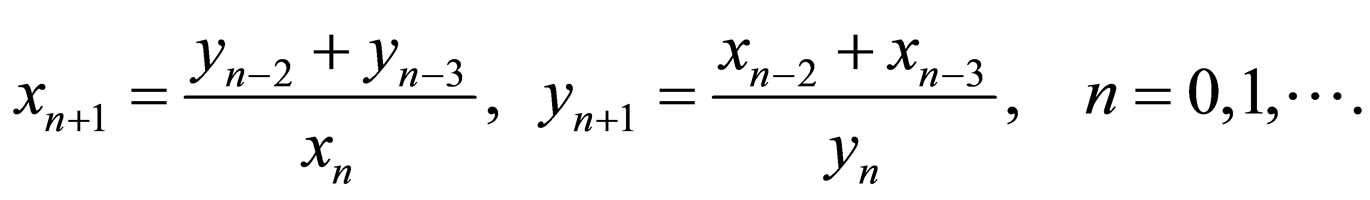 (2)
(2)
where the initial points 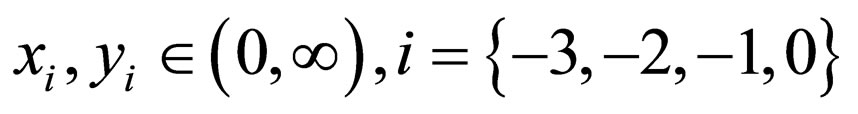
Recently, there has been great interest in studying difference equation systems. One of the reasons for this is the necessity for some techniques that can be used in investigating equations arising in mathematical models describing real life situations in population biology, economics, probability theory, etc. There are many papers related to the difference equations system for example, such as [2-9].
In [2], Cinar studied the solutions of the system of difference equations:
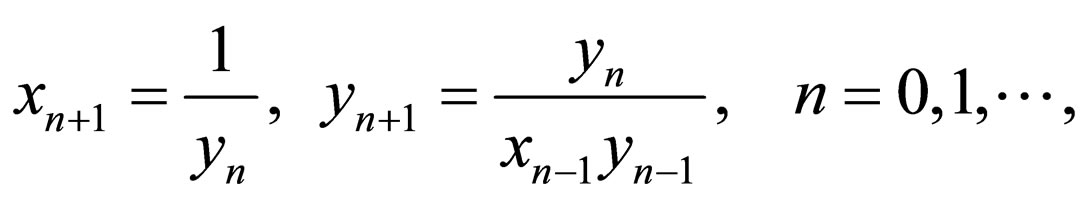 (3)
(3)
In [3], E. Camouzis and Papaschinnopoulos studied the global asymptotic behavior of positive solution of the system of rational difference equations:
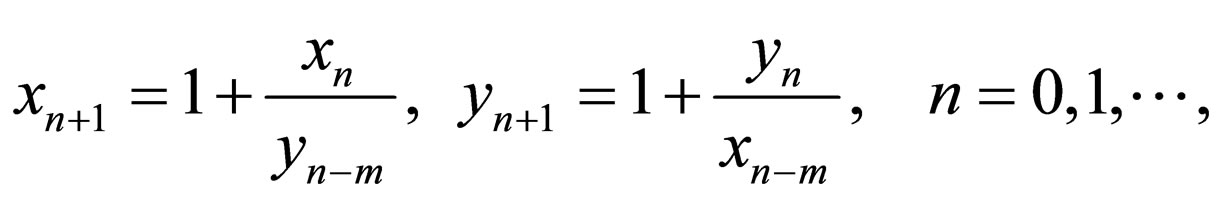 (4)
(4)
In [4], Ahmet Yasar Ozban studied the system of rational difference equations:
 (5)
(5)
In [5], Abdullah Selcuk Kurbanli et al. studied the behavior of positive solutions of the system of rational difference equations:
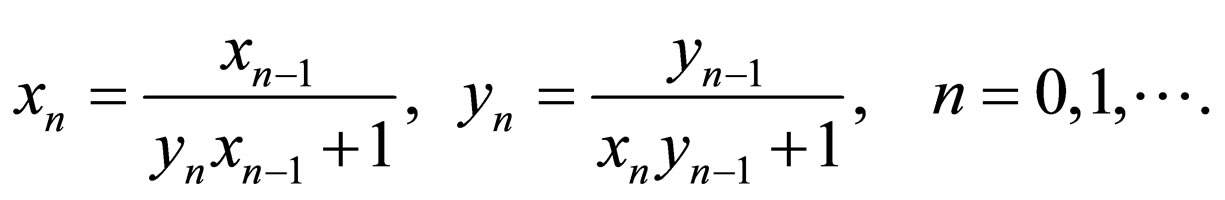 (6)
(6)
In this paper, we try to find out some conditions such that the solution of system (2) converges to periodic solution. At the same time, we can get the oscillatory of system (2).
Before giving some results of the system (2), we need some definitions as follows [6]:
Definition 1.1 A pair of sequences of positive real numbers 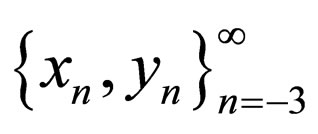 that satisfies system (2) is a positive solution of system (2). If a positive solution of system (2) is a pair of positive constants
that satisfies system (2) is a positive solution of system (2). If a positive solution of system (2) is a pair of positive constants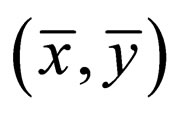 , that solution is the equilibrium solution.
, that solution is the equilibrium solution.
Definition 1.2 A “string” of consecutive terms 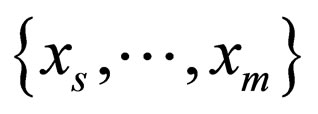 (resp.
(resp.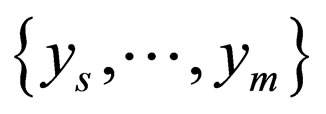 ), (
), (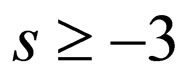 ,
,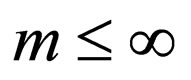 ) is said to be a positive semicycle if
) is said to be a positive semicycle if 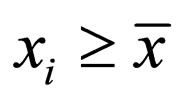 (resp.
(resp.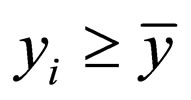 ),
), 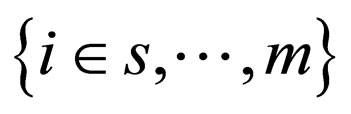 ,
, 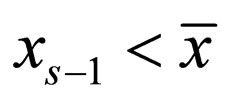 (resp.
(resp.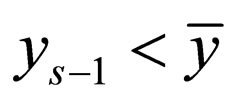 ), and
), and 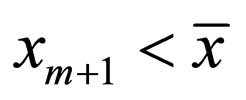 (resp.
(resp.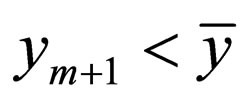 ). Otherwise, that is said to be a negative semicycle.
). Otherwise, that is said to be a negative semicycle.
A “string” of consecutive terms 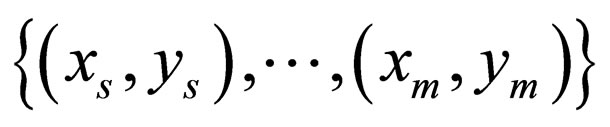 is said to be a positive(resp.negative) semicycle if
is said to be a positive(resp.negative) semicycle if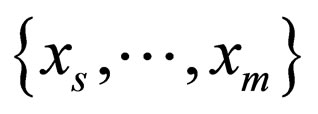 ,
, 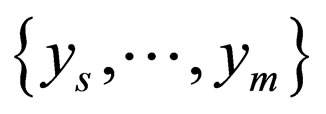 are positive (resp.negative) semicycle.
are positive (resp.negative) semicycle.
A solution 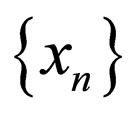 (resp.
(resp.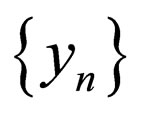 ) oscillates about
) oscillates about 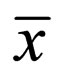 (resp.
(resp.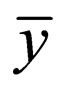 ) if for every
) if for every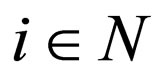 , there exist
, there exist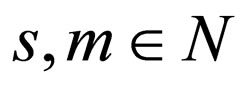 ,
, 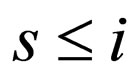 ,
, 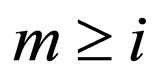 , such that
, such that 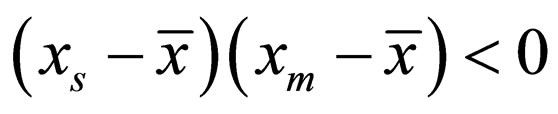 (resp.
(resp.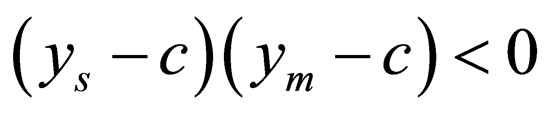 ). We say that a solution
). We say that a solution
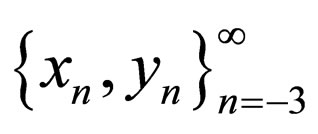 of system oscillates about
of system oscillates about 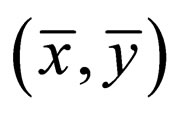 if
if 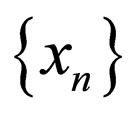
oscillates about 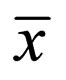 or
or 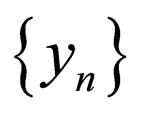 oscillates about
oscillates about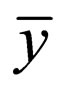 .
.
2. Some Lemmas
Lemma 2.1 The system (2) has a unique positive equilibrium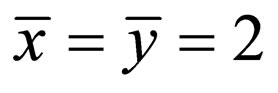 .
.
The proof of lemma 2.1 is very easy, so we omit it.
Lemma 2.2 If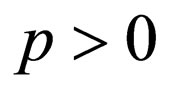 ,
, 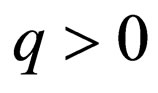 ,
, 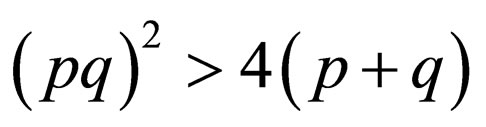 , Then ever positive solution of system (2) with prime period two takes the forms
, Then ever positive solution of system (2) with prime period two takes the forms
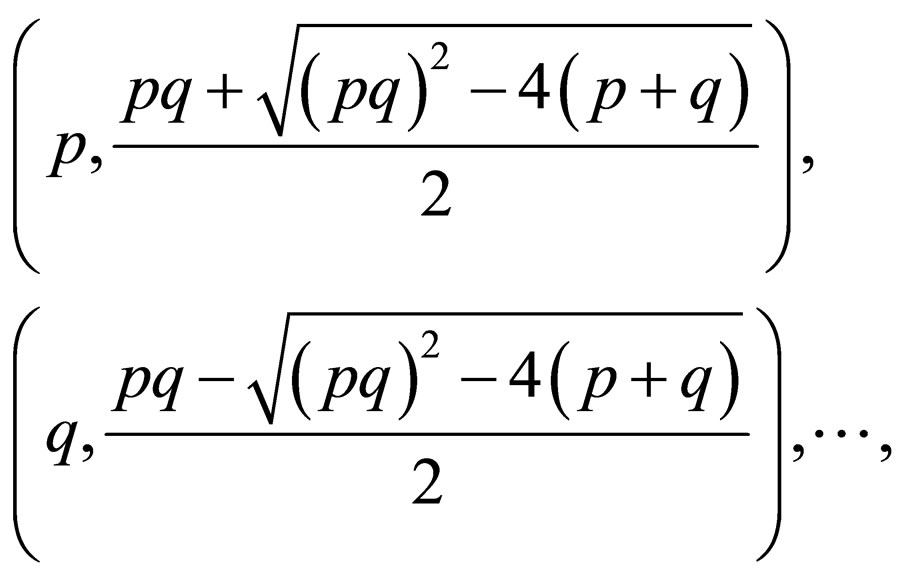
or
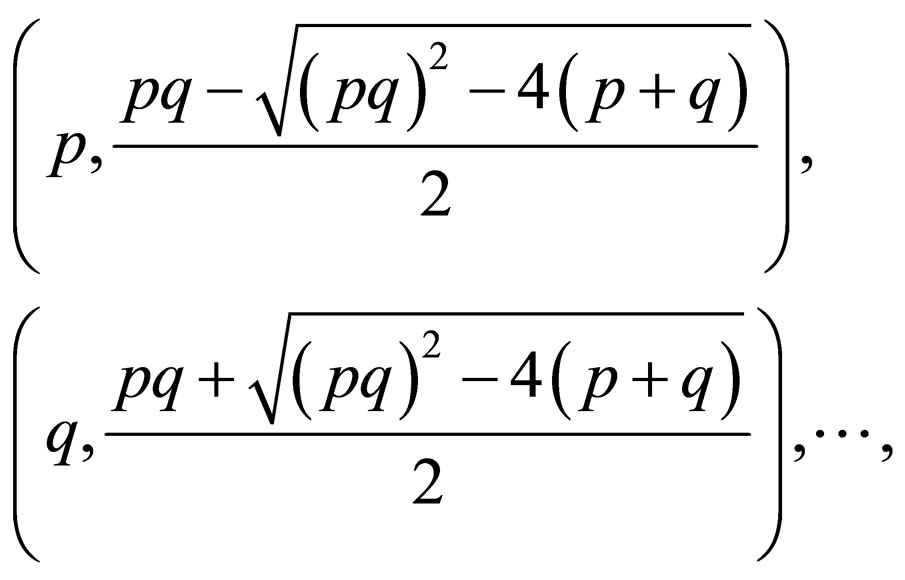
is a period-two solution of system (2).
Proof: Let 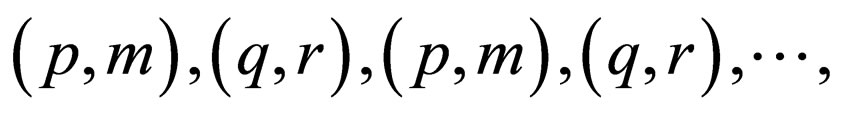 be a period-two solution of system (2).
be a period-two solution of system (2).
Then, by system (2) we get
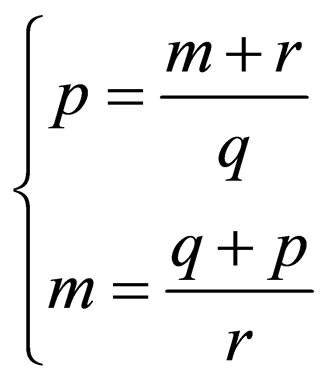 (7)
(7)
We can see that (7) can be changed to
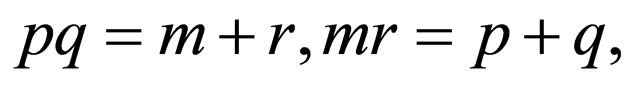 (8)
(8)
Form (8), we can obtain
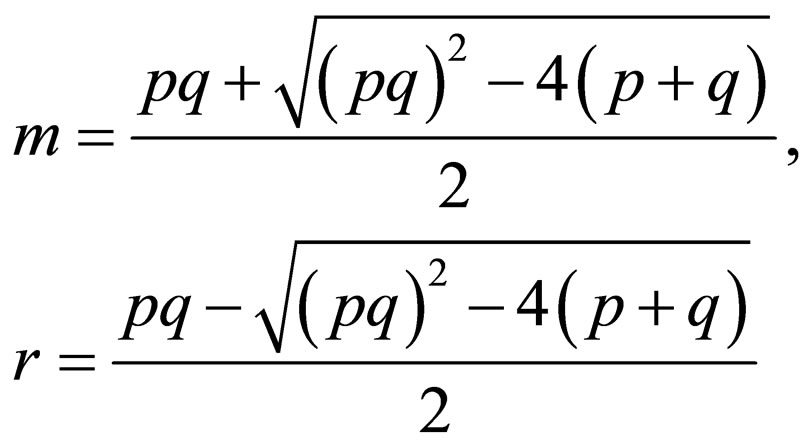
or
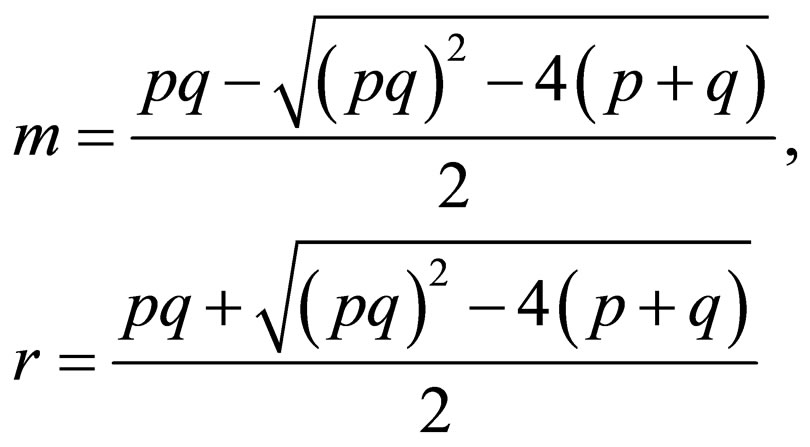
and 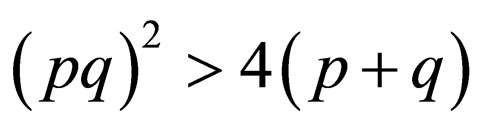
Therefore, we complete the proof.
Lemma 2.3 Assume that the initial points 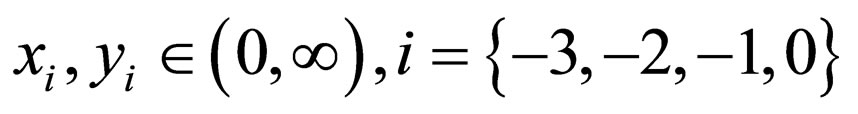 , and
, and 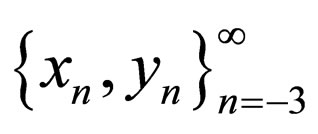 is a positive solution of system (2). Then the following cases are true:
is a positive solution of system (2). Then the following cases are true:
(a) If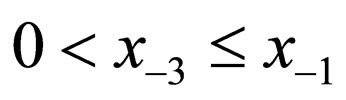 ,
, 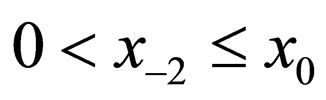 ,
,
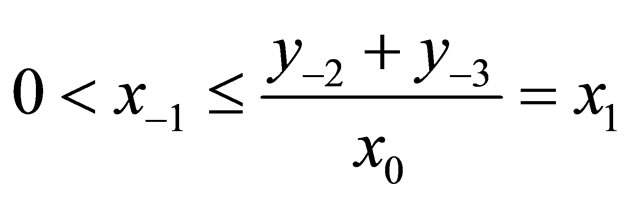 ;
;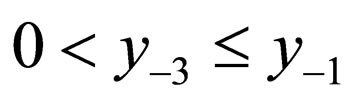 ,
, 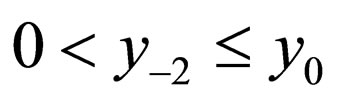 ,
, 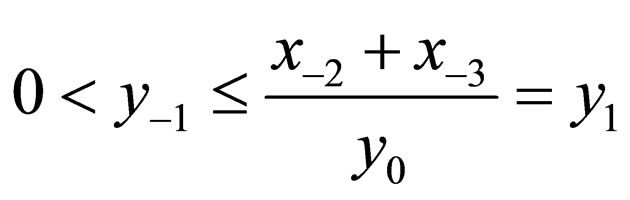 , then
, then 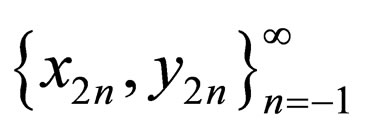 and
and
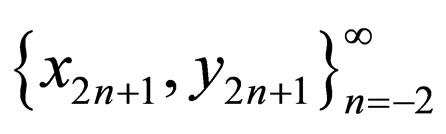 are both increasing.
are both increasing.
(b) If ,
, 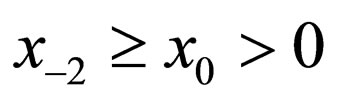 ,
,
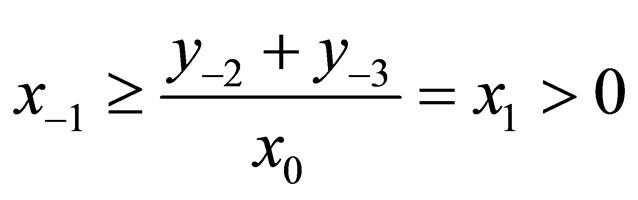 ;
;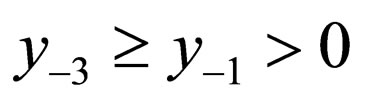 ,
, 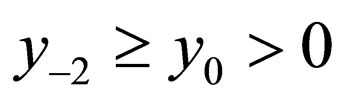 ,
, 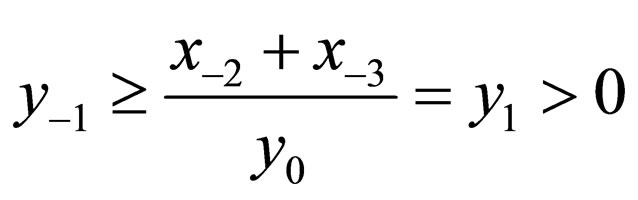 , then
, then 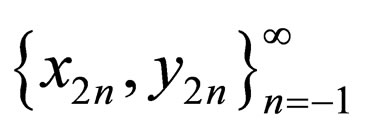 and
and
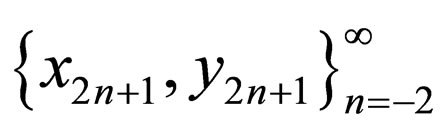 are both decreasing.
are both decreasing.
Proof: (a) By system (2), we can get
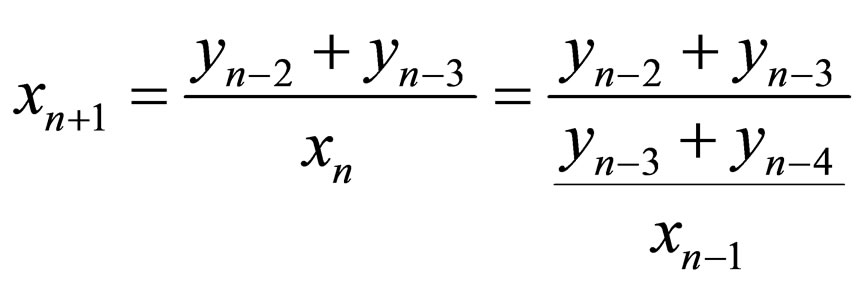
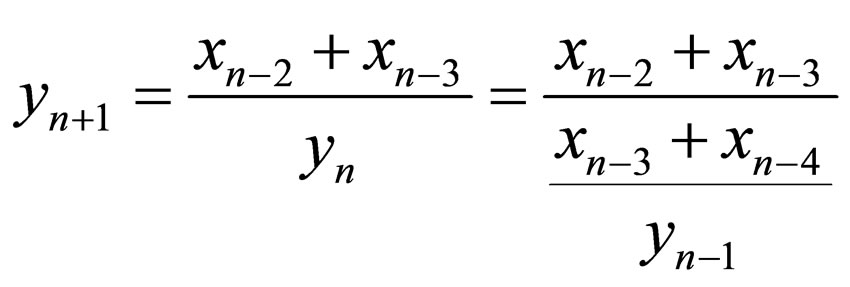
i.e.
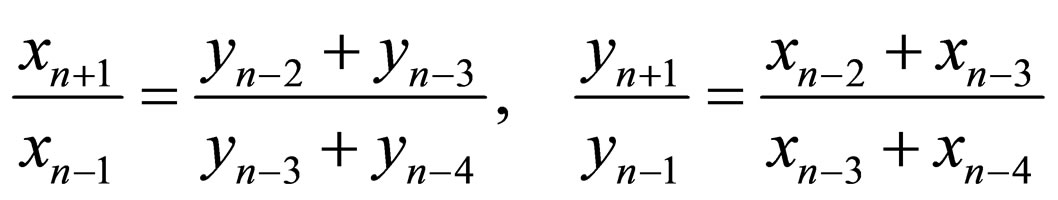 (9)
(9)
where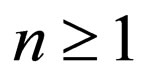 .
.
By condition 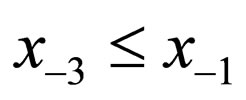 and (9), we get:
and (9), we get:
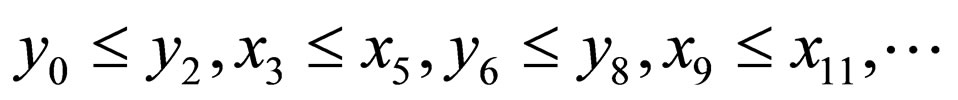 (10)
(10)
By condition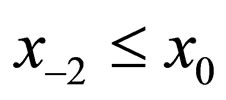 , and (9), we get:
, and (9), we get:
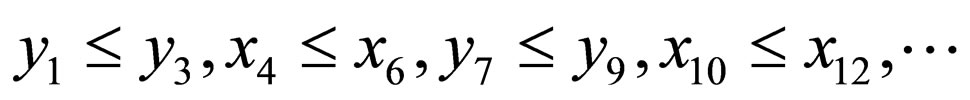 (11)
(11)
By condition 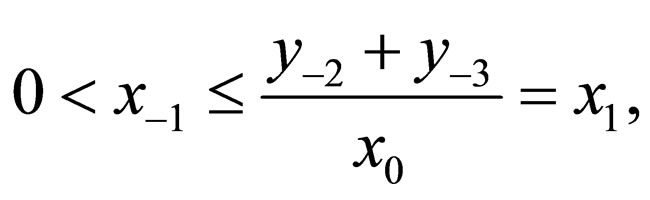 and (9), we get:
and (9), we get:
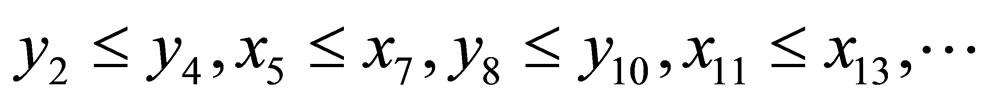 (12)
(12)
Equally, we can get:
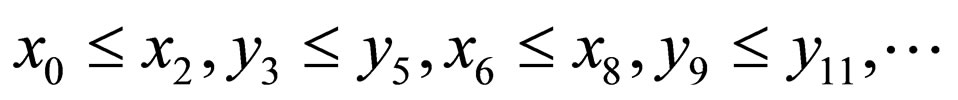 (13)
(13)
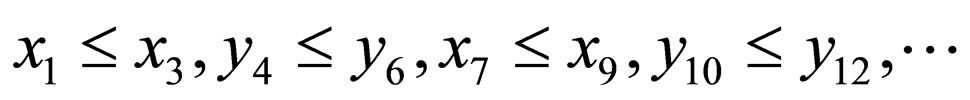 (14)
(14)
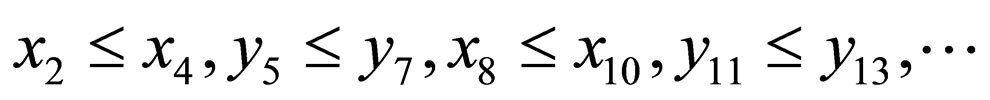 (15)
(15)
Hence, by induction and (10)-(15), we proof that
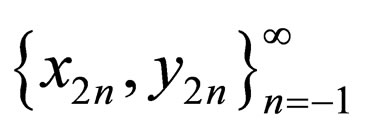 and
and 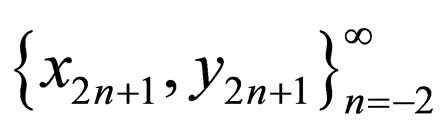 are both increasing.
are both increasing.
Using the same method, we can prove that case (b) holds.
Therefore, we complete the proof.
Lemma 2.4 Assume that
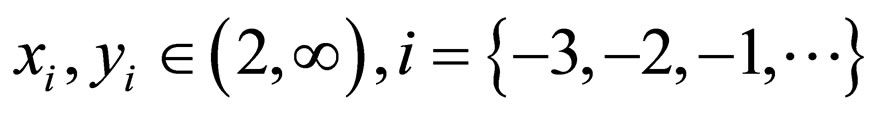 . Then there does not exist a positive solution
. Then there does not exist a positive solution 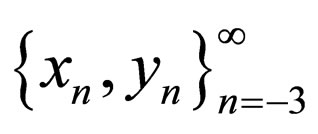 of system (2) such that
of system (2) such that 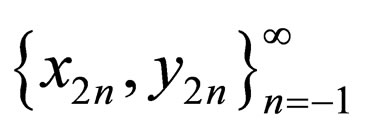 and
and 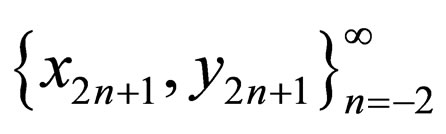 are both increasing or both decreasing.
are both increasing or both decreasing.
Proof: By Equation (9), we can get 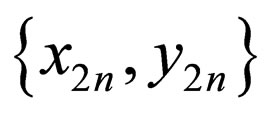 and
and 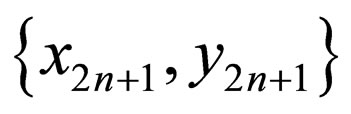 have the same monotonous.
have the same monotonous.
Firstly, we proof that there does not exist positive solution 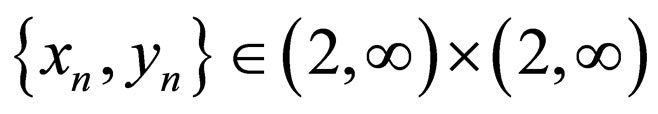 such that
such that
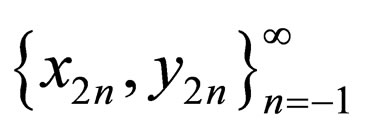 and
and 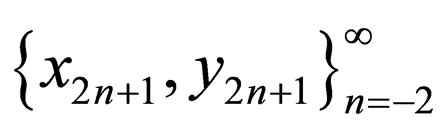 are both increasing.
are both increasing.
Assume, for the sake of contradiction, that we have the following results:
(i) 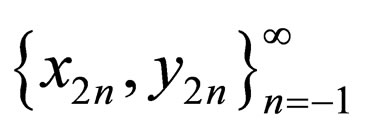 is increasing;
is increasing;
(ii)  is also increasing.
is also increasing.
By system (2), we obtain
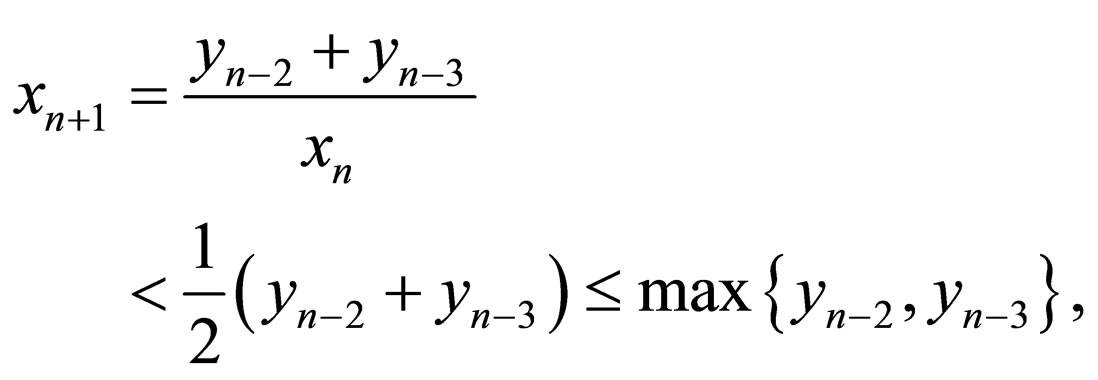 (16)
(16)
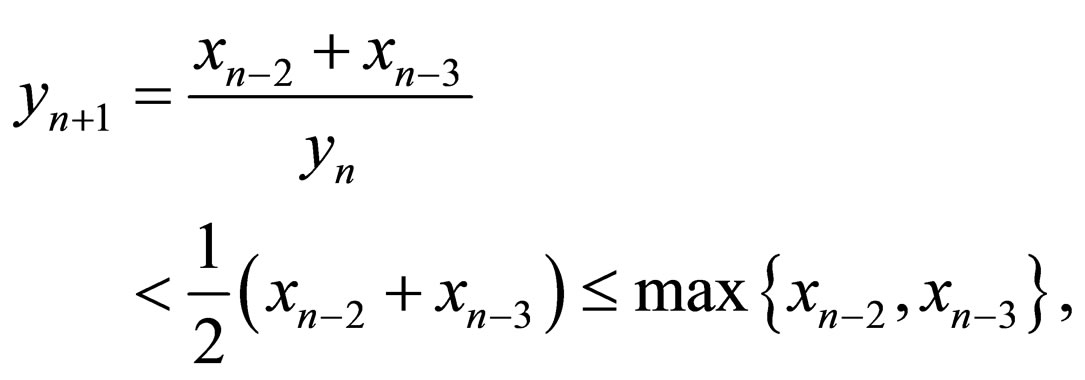 (17)
(17)
in Equations (16) and (17), it implies that:
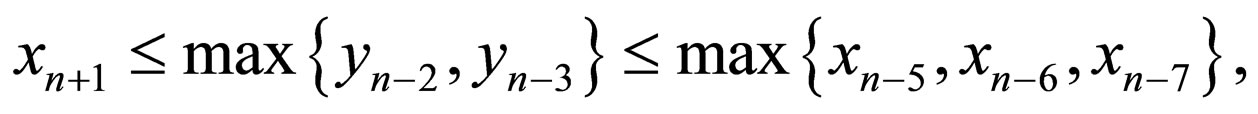
Because of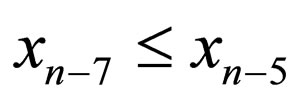 ,
, 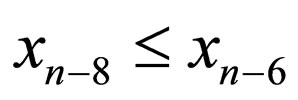 , we can get
, we can get
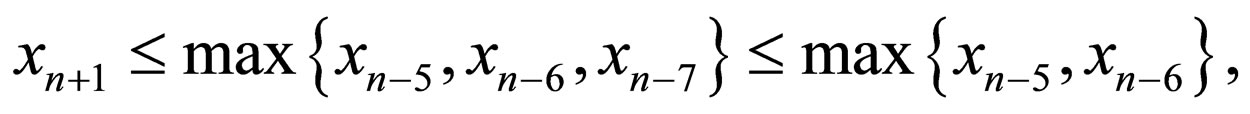
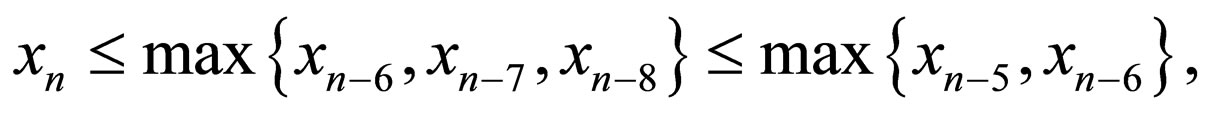
i.e
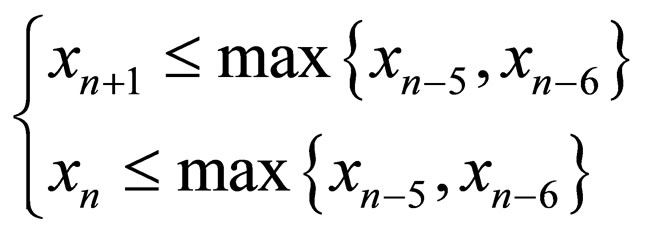 (18)
(18)
Also, we can get
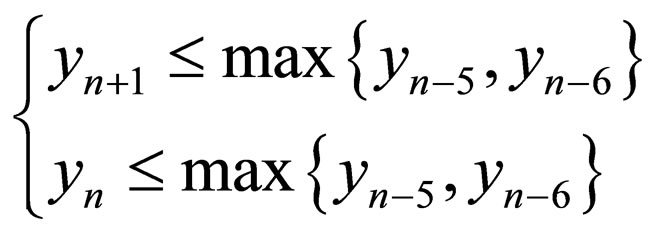 (19)
(19)
Because of the assumptions (i) and (ii), it is easy to see that (18) and (19) do not hold.
This is a contradiction and we proof the case of increasing does not hold.
Next, we proof there does not exist positive solution of system (2) such that  and
and
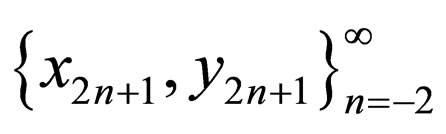 are both decreasing.
are both decreasing.
Assume, for the sake of contradiction, that we have the following results:
(i) 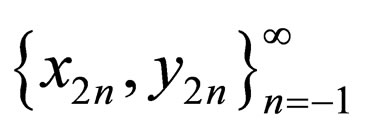 is decreasing;
is decreasing;
(ii) 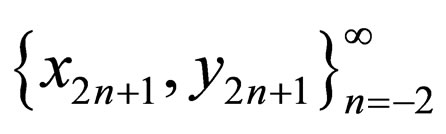 is also decreasing.
is also decreasing.
By the Limiting Theorem we know that 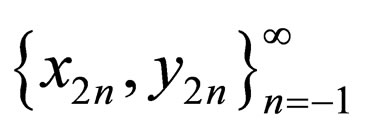
and 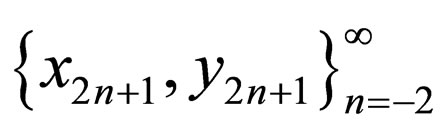 are both decreasing into a pair of constants.
are both decreasing into a pair of constants.
We set ,
, 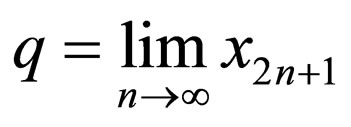 ,
, 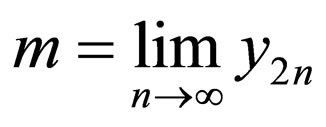 ,
,
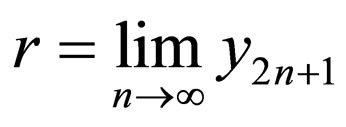 , and
, and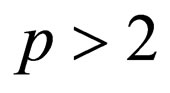 ,
, 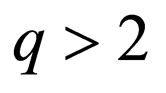 ,
, 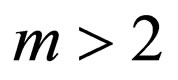 ,
,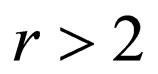 .
.
By system (2), we know that these constants satisfy the system (2)i.e.
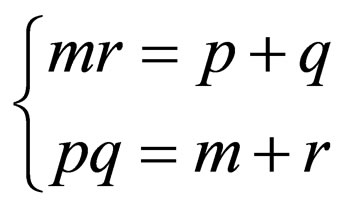 (20)
(20)
However, if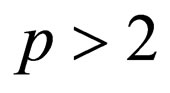 ,
, 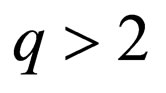 ,
, 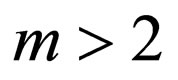 ,
, 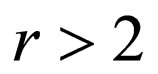 , Equation (20) do not holds, which is contradiction.
, Equation (20) do not holds, which is contradiction.
Hence, we complete the proof of lemma 10.
Lemma 2.5 Assume that
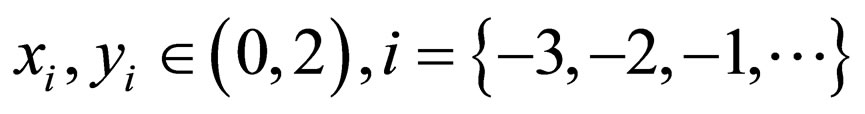 . Then there does not exist a positive solution
. Then there does not exist a positive solution 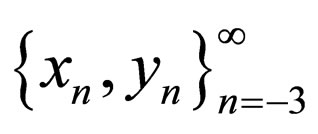 of system (2) such that
of system (2) such that 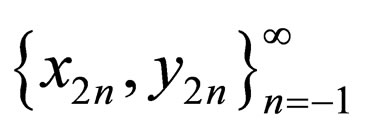 and
and 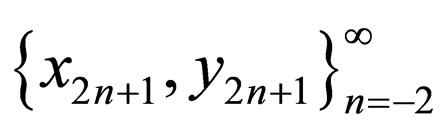 are both decreasing or both increasing.
are both decreasing or both increasing.
Proof: First, we proof there does not exist positive solution of system (2) such that 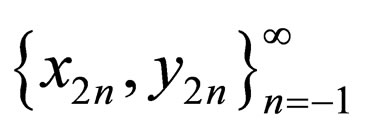 and
and 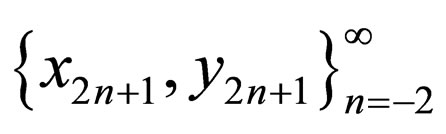 are both decreasing, the proof of increasing is similar, so we omit it.
are both decreasing, the proof of increasing is similar, so we omit it.
Assume, for the sake of contradiction, that we have the following results:
(i)  is decreasing;
is decreasing;
(ii) 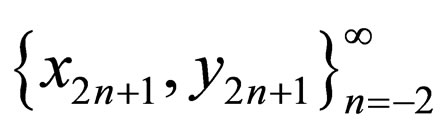 is also decreasing.
is also decreasing.
We set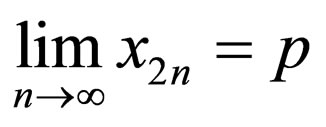 ,
, 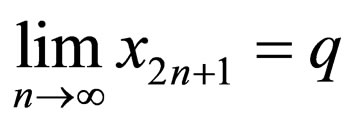 ,
,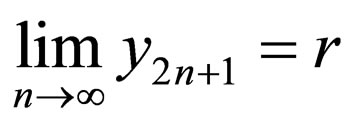
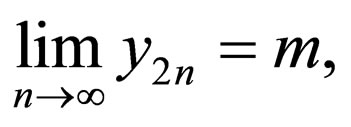 .
.
By Limit Theorem,we know that 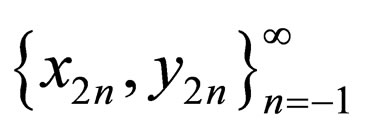 and
and 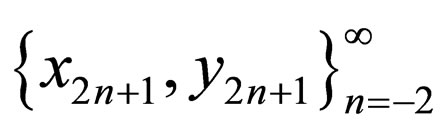 are both decreasing into a pair of constants.
are both decreasing into a pair of constants.
Obviously, the limits of 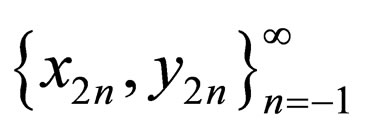
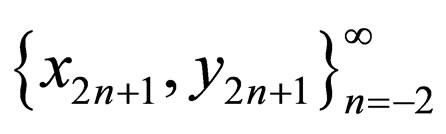 can not decrease into zero.
can not decrease into zero.
By system (2), we can get
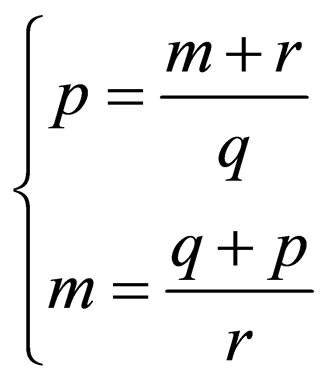 (21)
(21)
where , which can be changed to
, which can be changed to
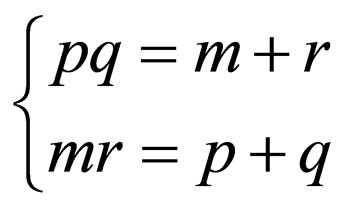 (22)
(22)
However, if , Equation (22) can not hold.
, Equation (22) can not hold.
This is a contradiction and we complete the proof.
The the proof of the case of increasing is similar with the proof of the the case of decreasing, so we omit it.
In addition to the method above, we can proof the Lemma 2.5 by the method of Lemma 2.4. Here, we omit it.
3. Main Results
Theorem 3.1 Assume that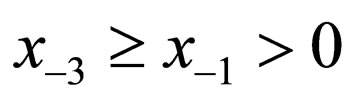 ,
, 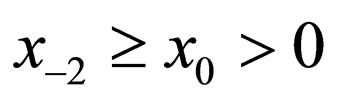 ,
,
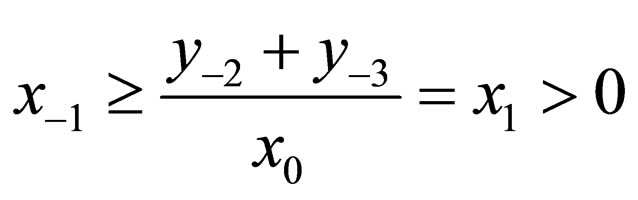 ;
;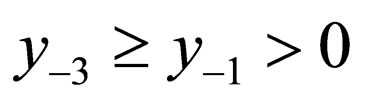 ,
, 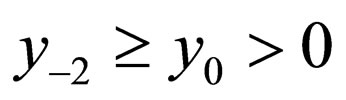 ,
, 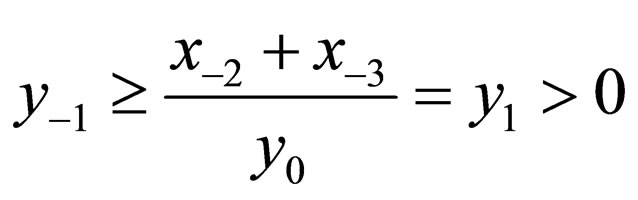 , and
, and  is a positive solution of system (2). Then
is a positive solution of system (2). Then  and
and
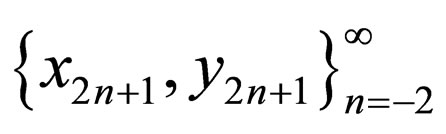 are both decreasing; and
are both decreasing; and 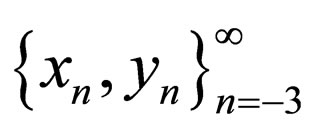 converges to a period-two solution as following
converges to a period-two solution as following
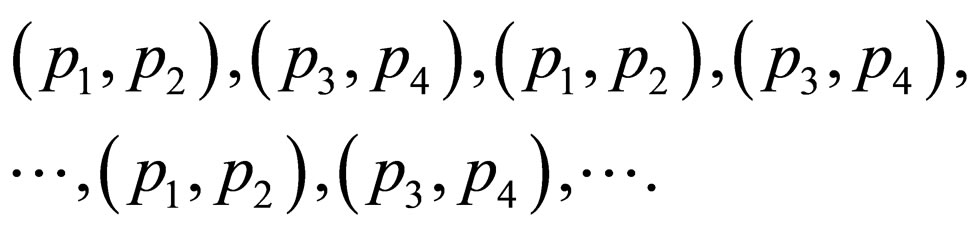
where 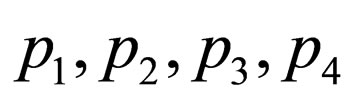 satisfy
satisfy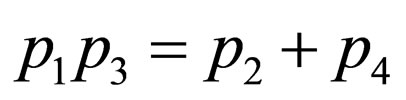 ,
,
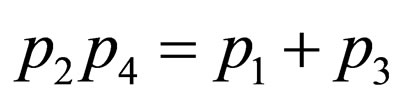 .
.
Proof: By lemma 2.3(a), we can obtain that
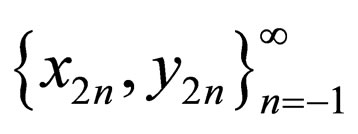 and
and 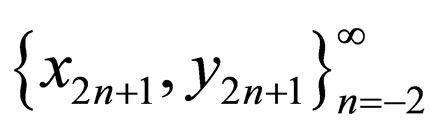 are both decreasing.
are both decreasing.
Then by the Limit Theorem, we can get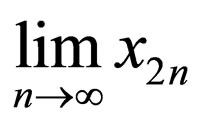 ,
, 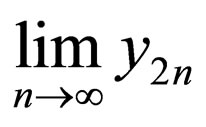 ,
, 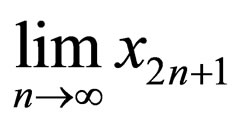 , and
, and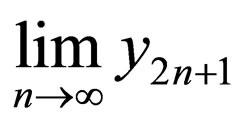 , all exist and are positive.
, all exist and are positive.
We can set
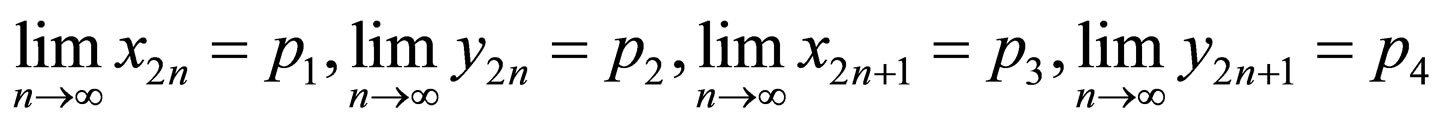
By lemmas 2.4 and 2.5, we know that there does not exist a positive solution 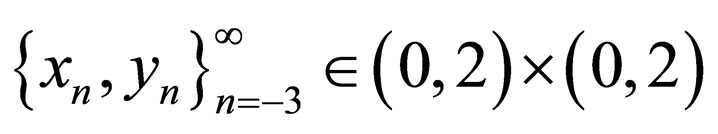 or
or
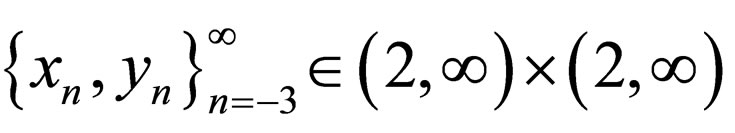 such that
such that  and
and
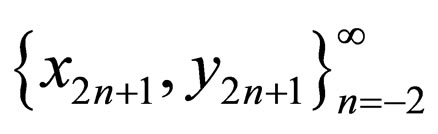 are both decreasing.
are both decreasing.
Hence, there is at least one of 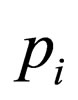 satisfy
satisfy 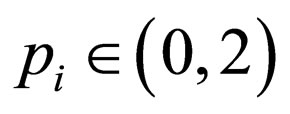 and at least one of
and at least one of 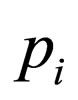 satisfy
satisfy 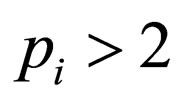
By system (2), we get
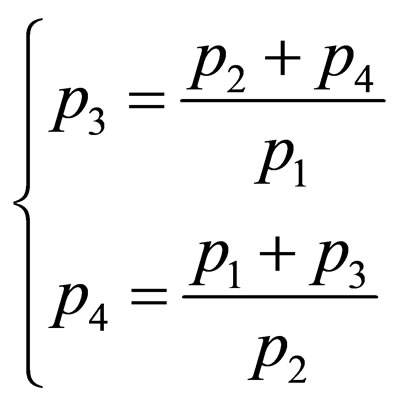 (23)
(23)
It is to see that 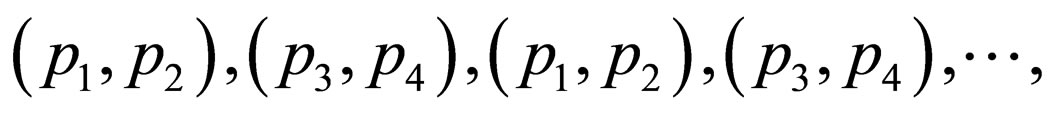 is a period-two solution of system (2), and
is a period-two solution of system (2), and 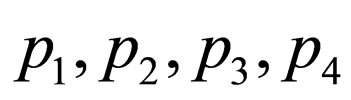 satisfy
satisfy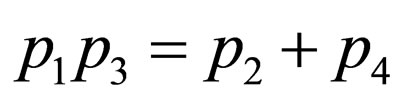 ,
,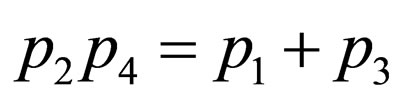 .
.
We complete the proof.
Corollary 3.1 Suppose that 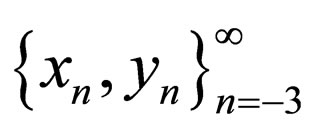 is a positive solution of system (2). Then the following statement is true:
is a positive solution of system (2). Then the following statement is true:
If 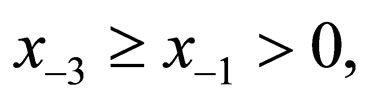
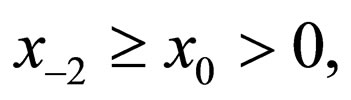
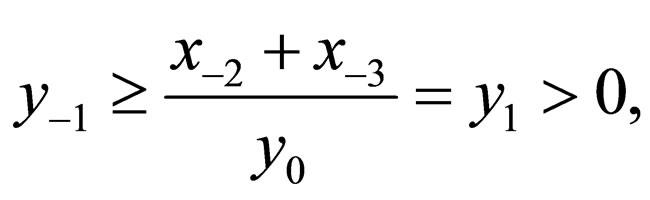
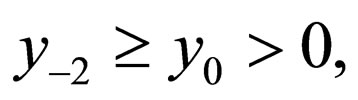
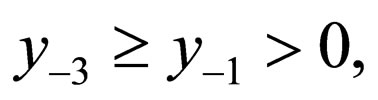
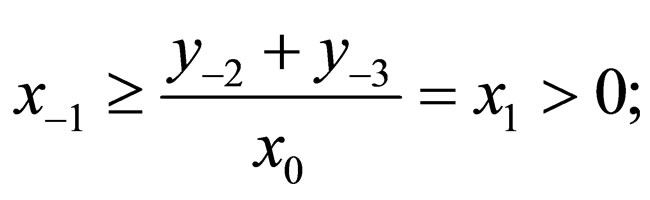 the solution of system (2)
the solution of system (2)
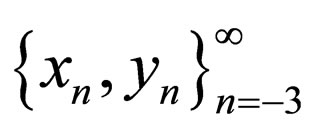 eventually oscillates about equilibrium
eventually oscillates about equilibrium
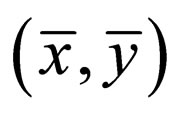 .
.
Theorem 3.2 Assume that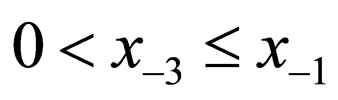 ,
, 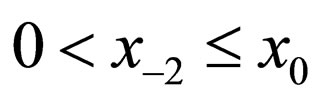 ,
,
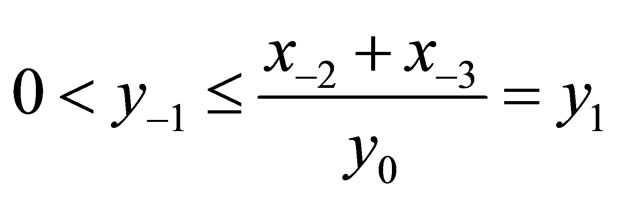
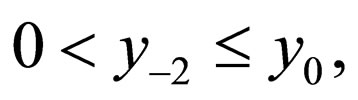
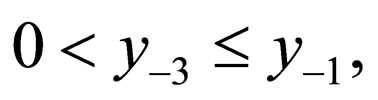
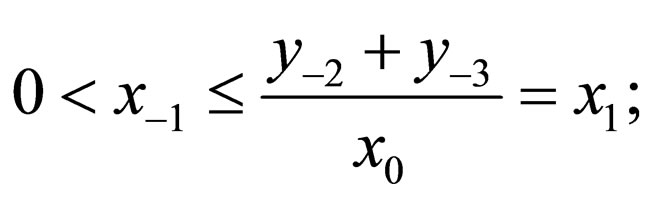 , and
, and 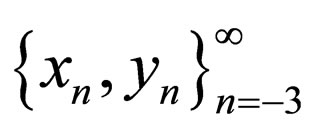 is a positive solution of system (2). Then
is a positive solution of system (2). Then 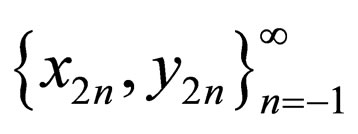 and
and
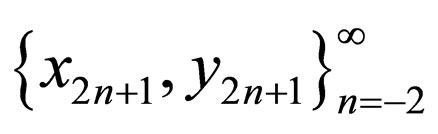 are both increasing; and
are both increasing; and 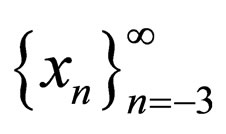
converges to a period-two solution as following
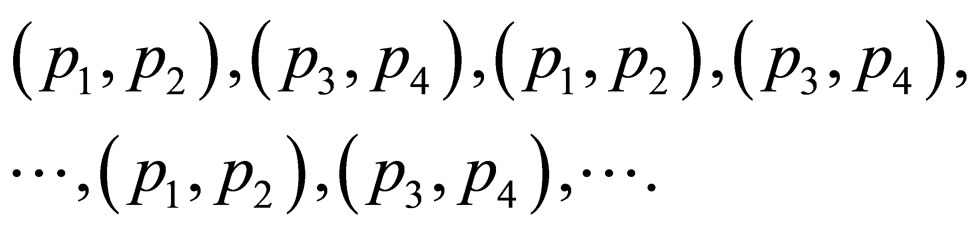
where 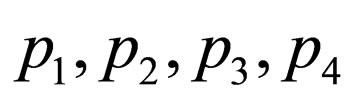 satisfy
satisfy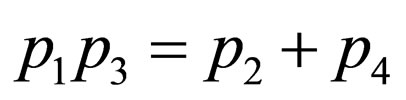 ,
,
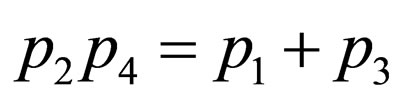 .
.
Proof: By lemma 2.3(a), we obtain that 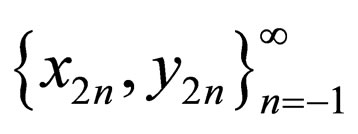 and
and 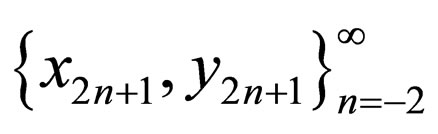 are both increasing.
are both increasing.
We set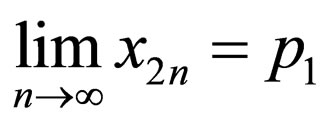 ,
, 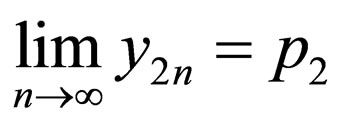 ,
, 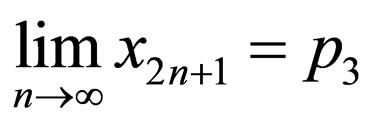 ,
, 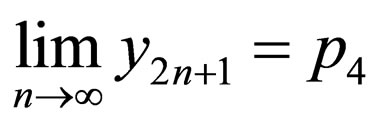
By Equation (9), we can get
 (24)
(24)
which can be changed into:
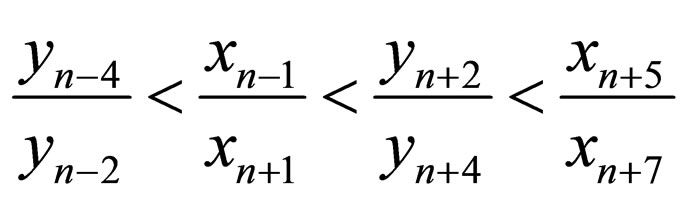 (25)
(25)
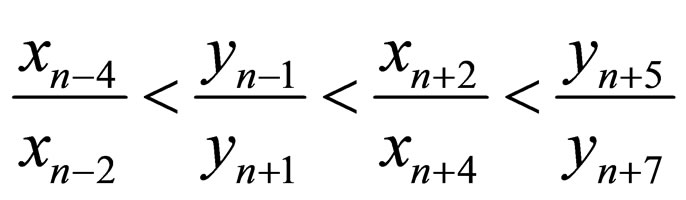 (26)
(26)
By the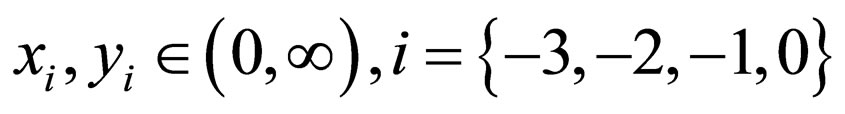 , we can get
, we can get
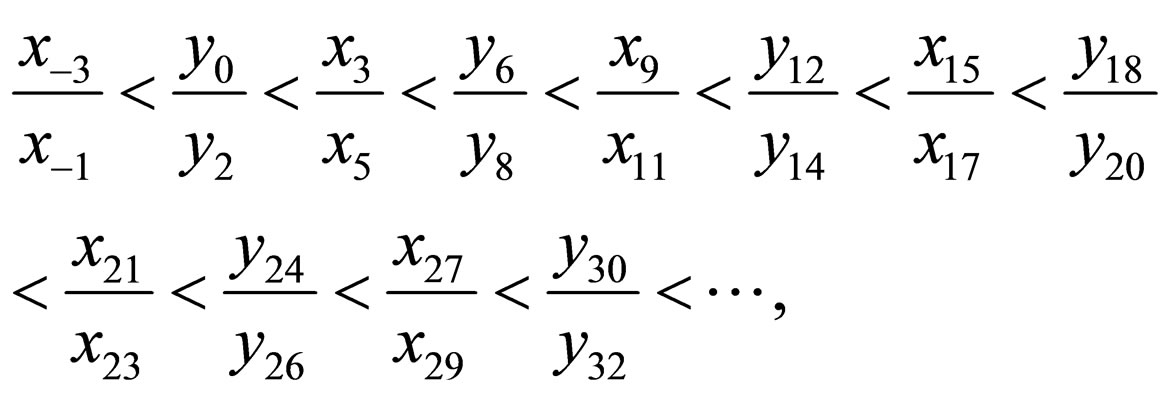
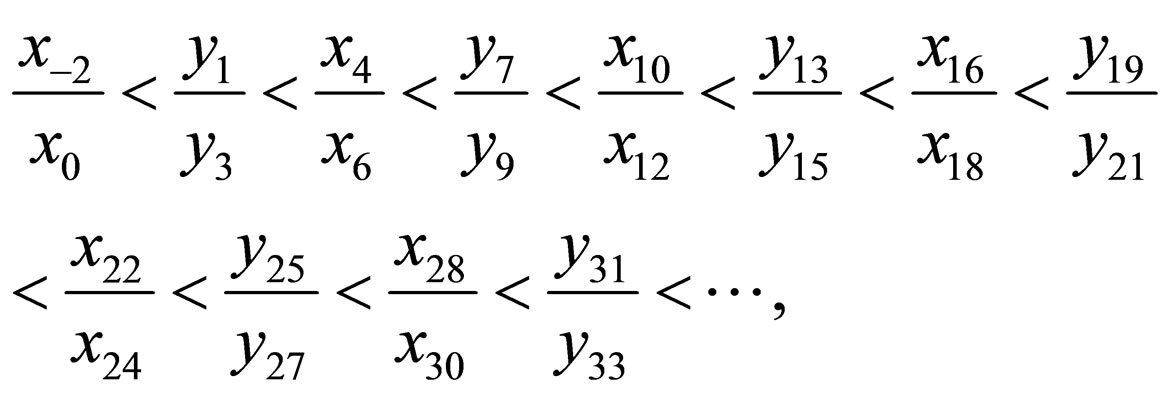
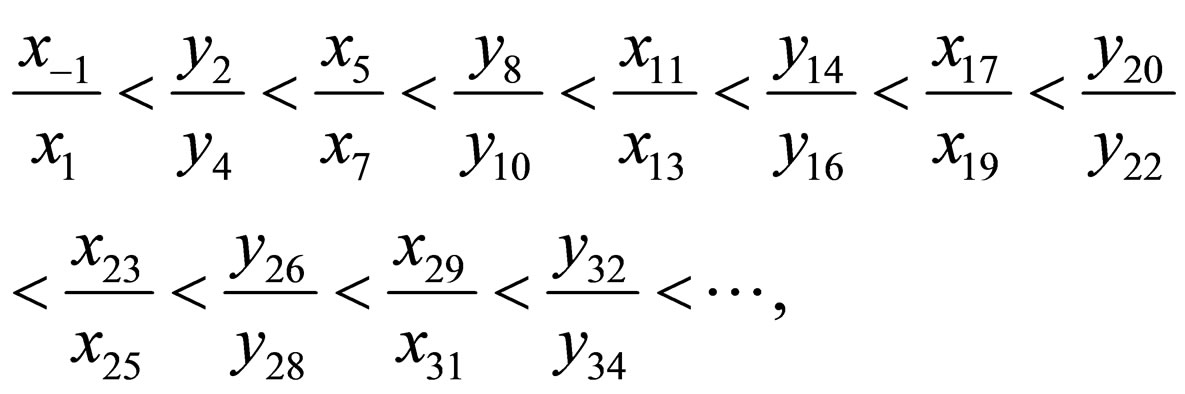
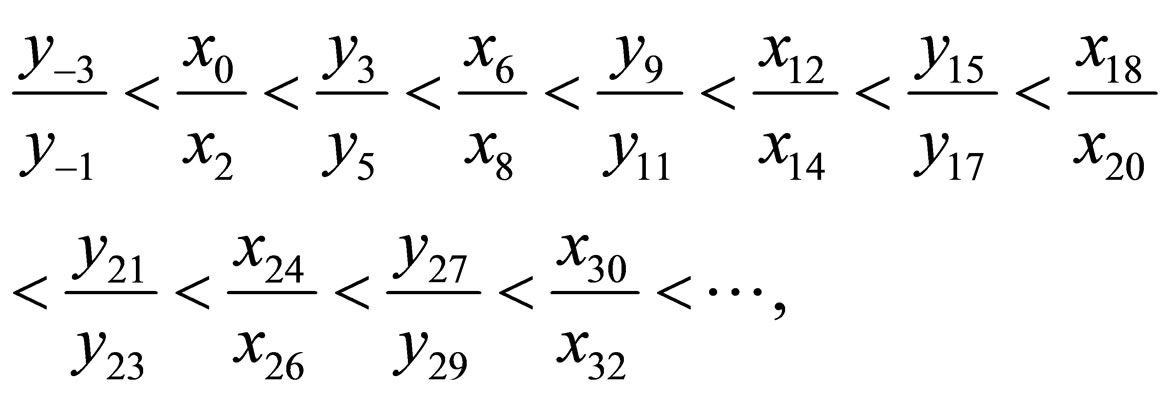
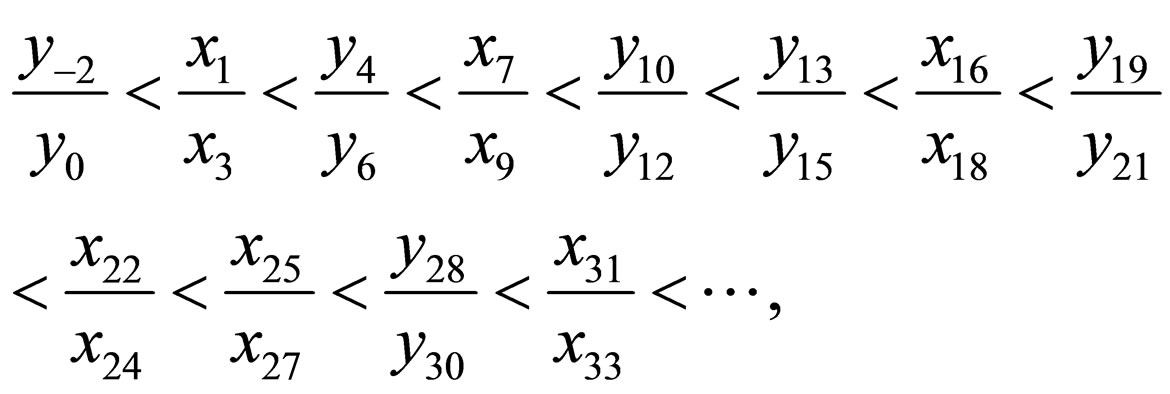
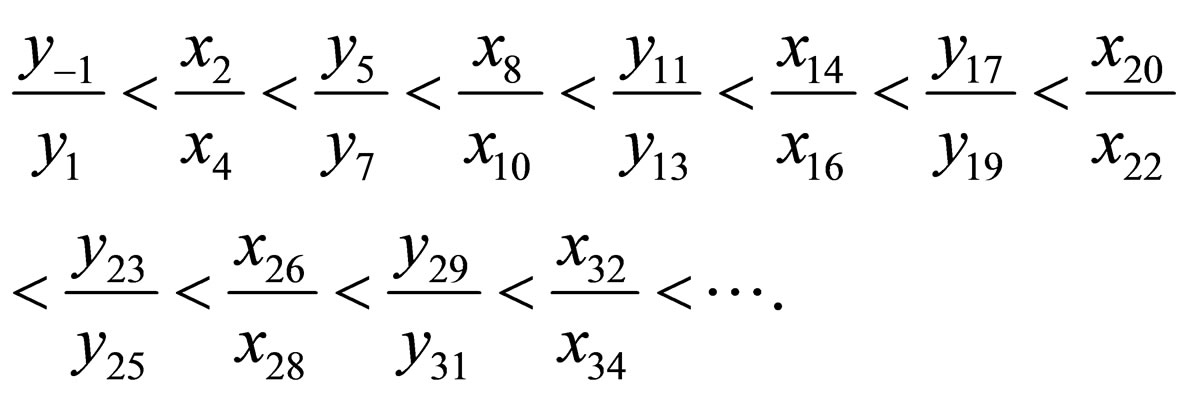
By induction, we can get
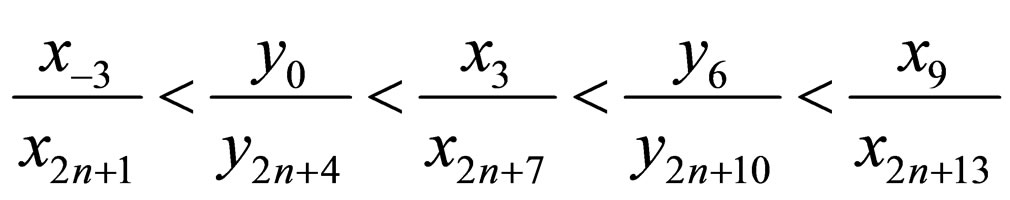 (27)
(27)
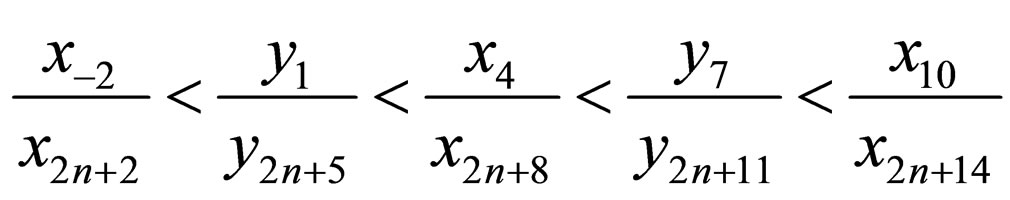 (28)
(28)
From Lemma 10, we know that there at least one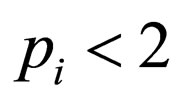 . Then by Limiting Theorem, we can get at least one of the limiting of
. Then by Limiting Theorem, we can get at least one of the limiting of 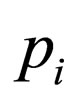 must exist. With no loss generality, we set the limit of
must exist. With no loss generality, we set the limit of 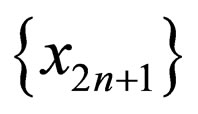 exist,we can know
exist,we can know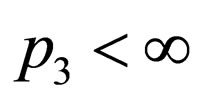 .
.
By limiting Equation (27), we can get
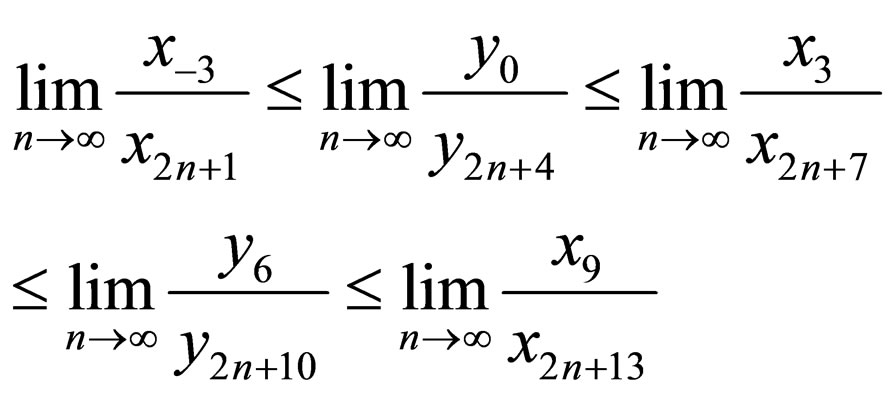 (29)
(29)
Hence, we can get
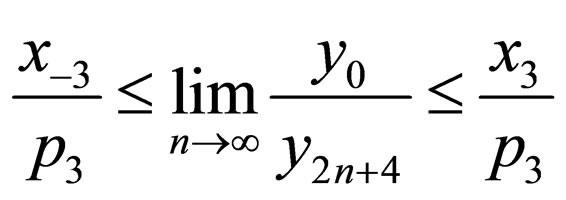
i.e.
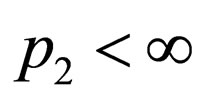
Next, we try to proof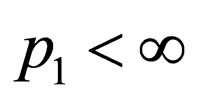 , and
, and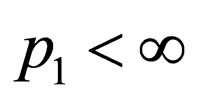 .
.
By system (2), we get
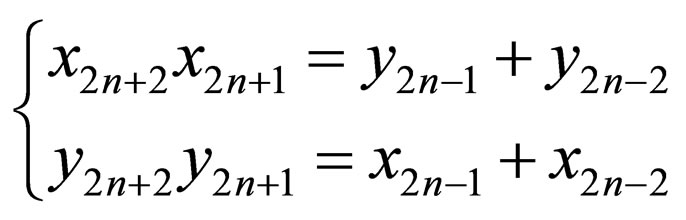 (30)
(30)
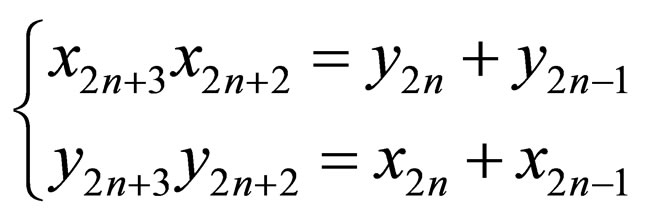 (31)
(31)
By (30) and (31), we can get
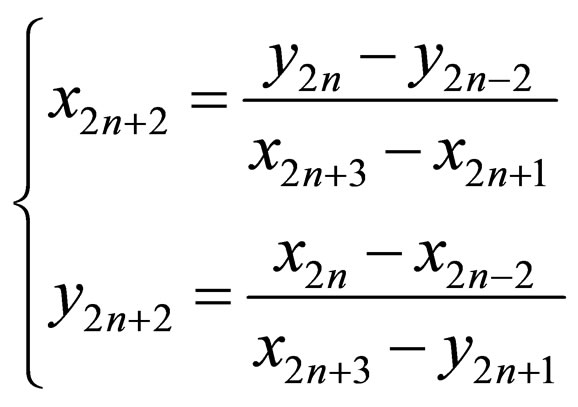 (32)
(32)
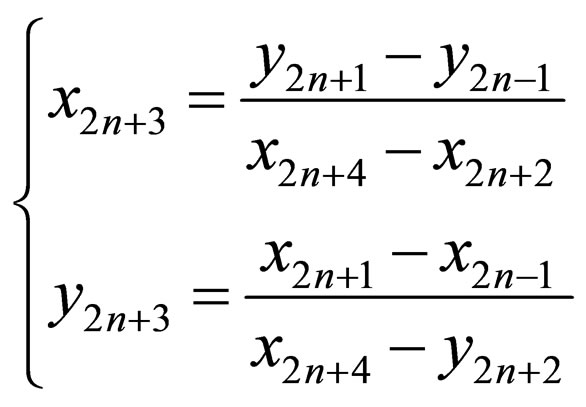 (33)
(33)
which can be changed into
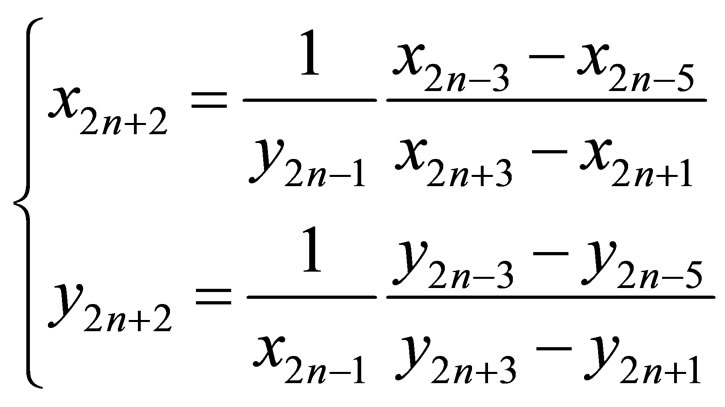 (34)
(34)
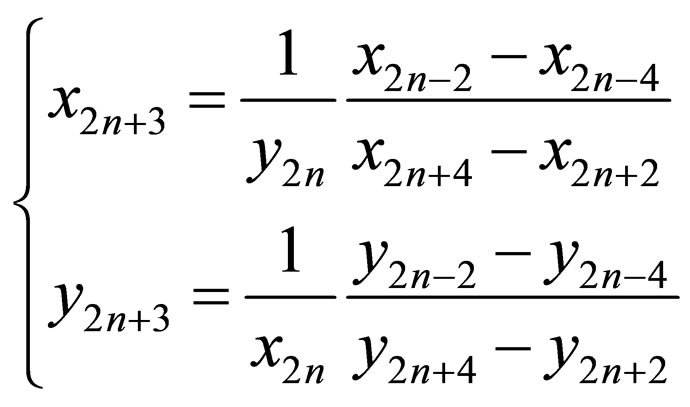 (35)
(35)
By the both side of Equation (35), we can get
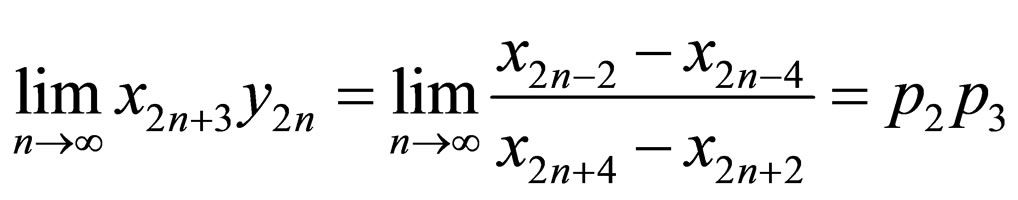 (36)
(36)
Assume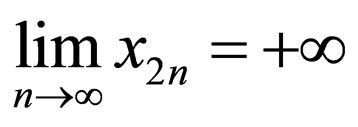 , by Stolz Theorem we obtain that
, by Stolz Theorem we obtain that
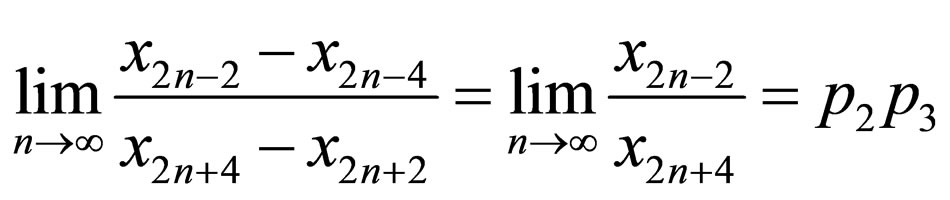 (37)
(37)
Because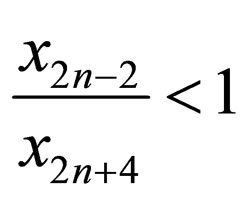 , then we can get the limit of
, then we can get the limit of 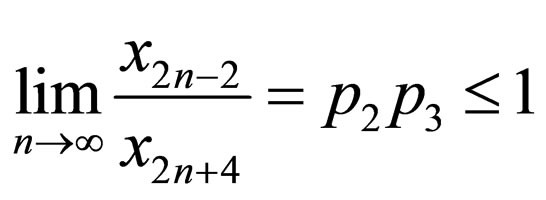
However, there exist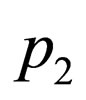 ,
, 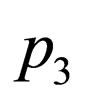 such that
such that , which is conduction.
, which is conduction.
Hence, the assume does not hold. We obtain
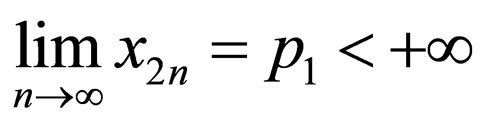 .
.
Use the same method, we can also get
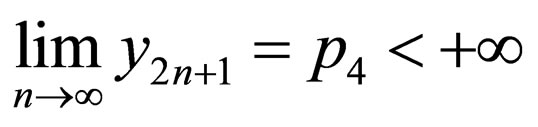 .
.
By system (2), we get
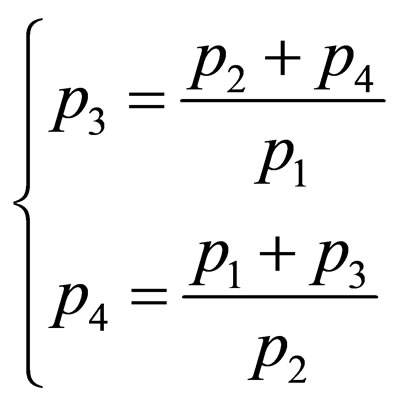 (38)
(38)
It is to see that 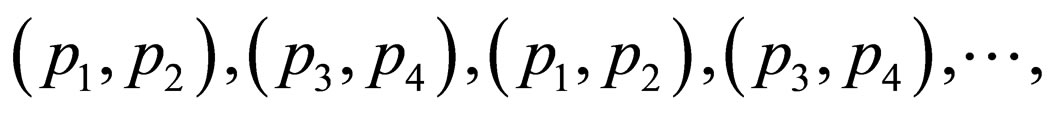 is a period-two solution of system (2), and
is a period-two solution of system (2), and 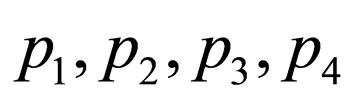 satisfy
satisfy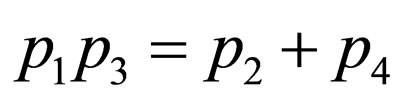 ,
,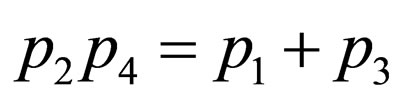 .
.
Therefore, we complete the proof.
Corollary 3.2 Suppose that 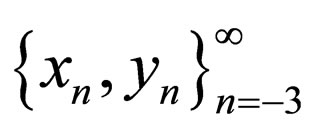 is a positive solution of system (2). Then the following statement is true:
is a positive solution of system (2). Then the following statement is true:
If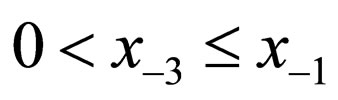 ,
,  ,
,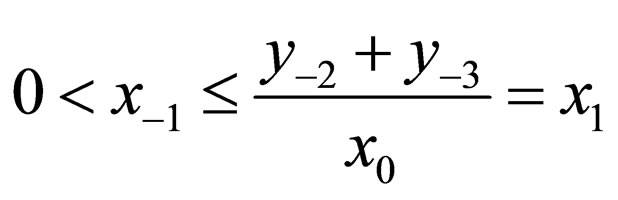 ;
;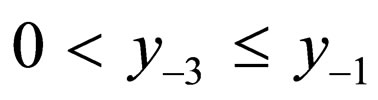 ,
, 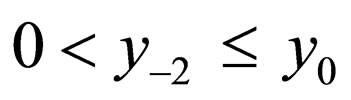 ,
, 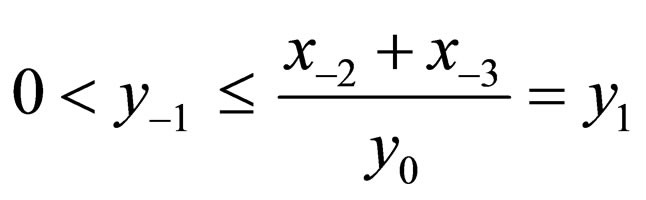 then the solution of system (2) oscillates about equilibrium
then the solution of system (2) oscillates about equilibrium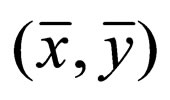 .
.
Theorem 3.3 Assume that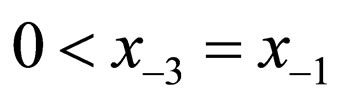 ,
, 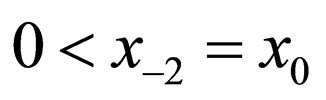 ,
,
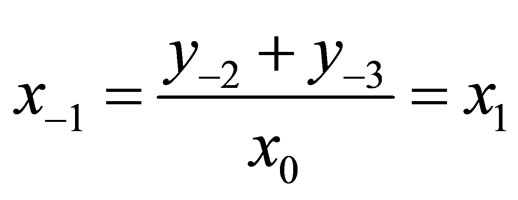 ;
;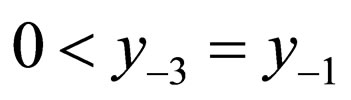 ,
, 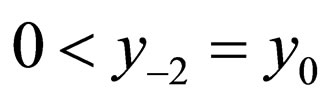 ,
,
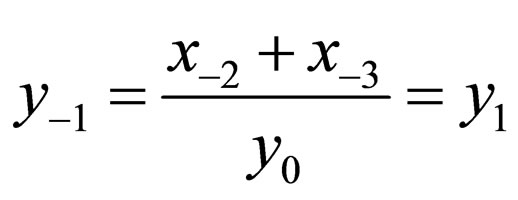 , and
, and  is a positive solution of system (2). Then the system (2) has prime period two solutions, and
is a positive solution of system (2). Then the system (2) has prime period two solutions, and 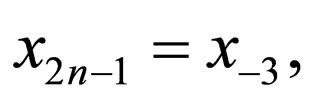
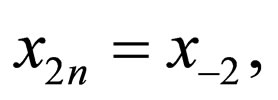

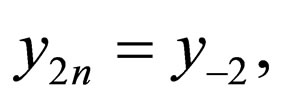 for
for 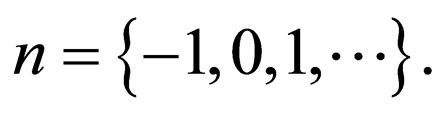
Proof: By the lemma 8, we can complete the proof. Here, we omit it.
REFERENCES
- M. R. S. Kulenović and G. Ladas, “Dynamics of Second Order Rational Difference Equation with Open Problems and Conjectures,” CRC Press, Chapman Hall, 2002.
- C. Cinar, “On the Positive Solutions of the Difference Equation System
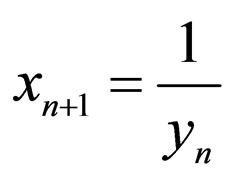 ,
, 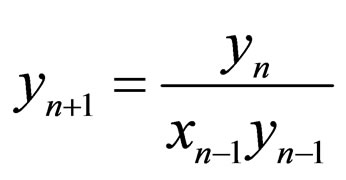 ,” Applied Mathematics and Computation, Vol. 158, 2004, pp. 303- 305.
,” Applied Mathematics and Computation, Vol. 158, 2004, pp. 303- 305. - E. Camouzis and G. Papaschinopoulos, “Global Asymptotic Behavior of Positive Solutions on the System of Rational Difference Equations
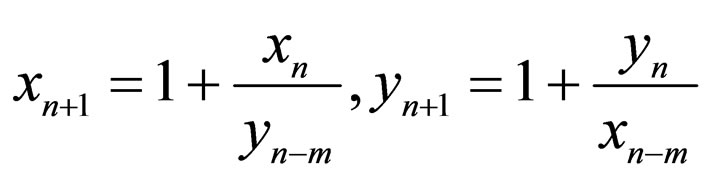 ,” Applied Mathematics and Letters, Vol. 17, 2004, pp. 733-737.
,” Applied Mathematics and Letters, Vol. 17, 2004, pp. 733-737. - A. Y. Ozban, “On the System of Rational Difference Equations
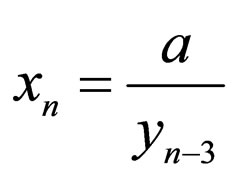
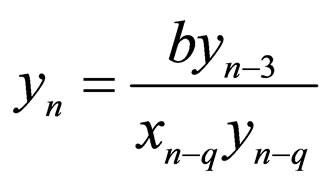 ,” Applied Mathematics and Computation, Vol. 188, 2007, pp. 833-837.
,” Applied Mathematics and Computation, Vol. 188, 2007, pp. 833-837. - A. S. Kurbanli, C. Cinar and I. Yalcinkaya, “On the Behavior of Positive Solutions of the System of Rational Difference Equations
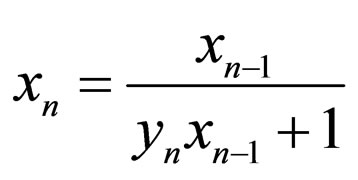 ,
, 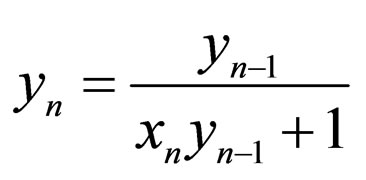 ,” Mathematical and Computer Modelling, Vol. 53, 2011, pp. 1261-1267.
,” Mathematical and Computer Modelling, Vol. 53, 2011, pp. 1261-1267. - G. Papaschinopoulos, “On a System of Two Nonlinear Difference Equations,” Journal of Mathematicsal Analysis and Applications, Vol. 219, 1998, pp. 415-426.
- G. Papaschinopoulos, M. Radin and C. J. Schinas, “Study of the Asymptotic Behavior of the Solutions of Three Systems of Difference Equations of Exponential Form,” Applied Mathematics and Computation, Vol. 218, 2012, pp. 5310-5318.
- E. Camouzis, C. M. Kent, G. Ladas and C. D. Lynd, “On the Global Character of Solutions of the System:
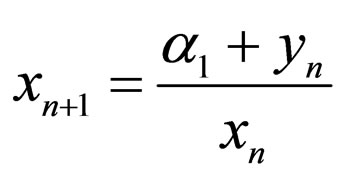
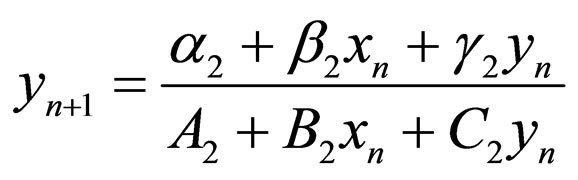 ,” Journal of Difference Equations and Applications, Vol. 18, 2012, pp. 1205-1252.
,” Journal of Difference Equations and Applications, Vol. 18, 2012, pp. 1205-1252. - E. Camouzis, E. Drymonis, G. Ladas and W. Tikjha, “Patterns of Boundedness of the Rational System
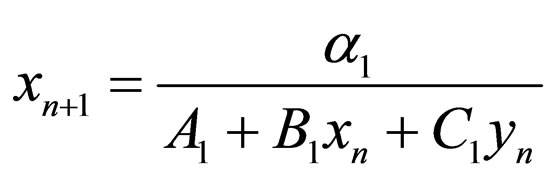
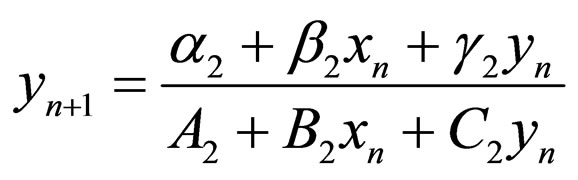 ,” Journal of Difference Equations and Applications, Vol. 18, 2012, pp. 89-110.
,” Journal of Difference Equations and Applications, Vol. 18, 2012, pp. 89-110.
NOTES
*Research supported by Distinguished Expert Foundation and Youth Science Foundation of Naval Aeronautical and Astronautical University.
#Corresponding author.

