Journal of Environmental Protection
Vol.5 No.11(2014), Article ID:48988,13 pages DOI:10.4236/jep.2014.511096
Ammonia Diffusion Phenomena through NalophanTM Bags Used for Olfactometric Analyses
Selena Sironi1*, Lidia Eusebio1, Laura Capelli1, Emanuela Boiardi1, Renato Del Rosso1, Jean-Michel Guillot2
1Department of Chemistry, Materials and Chemical Engineering “Giulio Natta”, Politecnico di Milano, Milano, Italy
2Laboratory of Industrial Environment Engineering, Ecole des Mines d’Alès, Alès cedex, France
Email: *selena.sironi@polimi.it
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 28 April 2014; revised 26 May 2014; accepted 21 June 2014
ABSTRACT
The ammonia loss through NalophanTM bags has been studied. Ammonia was chosen as target compound in order to be representative of odorous molecules of small dimensions. The losses observed for storage conditions and times as allowed by the reference standard for dynamic olfactometry (EN 13725:2003) indicate that odour concentration values due to the presence of small molecules may be significantly underestimated if samples are not analysed immediately after sampling. The diffusion coefficient of ammonia through the NalophanTM film was evaluated using the Fick’s law, and it turned out to be equal to 2.38E−12 (m2/s). The results and their theoretical interpretation indicate that concentration losses due to ammonia diffusion through the NalophanTM film can be decreased by using large bags and filling them up to their maximum capacity.
Keywords:Sampling Bag, Diffusion, Odour Sampling, Ammonia, Nalophan

1. Introduction
Even though environmental odours are generally not harmful to health [1] , in the last 30 years odour pollution has become a serious environmental concern because it may be the cause of physiological stress to the population. For this reason, during the last years, several studies have been undertaken to assess how to control and monitor odour emissions [2] . For many years, researchers have tried to characterize odour using chemical and physical techniques like GC and GC-MS. Although dozens, sometimes hundreds of odorants can be identified, such identification mostly fails to predict odour as it is perceived by human nose [3] [4] . This is the reason why sensorial odour measurement using human observers has become the main tool to quantify odours. Dynamic olfactometry has therefore been consolidated as the best analysis method to quantify odour emissions in terms of odour concentration or odour emission rate [5] .
Because of the difficulties associated with the conduction of olfactometric analyses on site, samples are generally collected and then stored in suitable containers until they are analysed in an olfactometric laboratory [5] -[9] . The European Standard on dynamic olfactometry [10] fixes the general requirements relevant to the materials used for the realization of sampling equipment. According to the European Standard, the materials used for olfactometry shall be odourless, they shall be selected to minimize the physical or chemical interaction between sample components and sampling materials, have low permeability in order to minimize sample losses caused by diffusion and smooth surface.
The materials allowed for realizing sample containers (bags) and listed in point 6.3.1 of the actual standard are: tetrafluoroethylene hexafluoropropylene copolymer (FEP); polyvinylfuoride (PVF, TedlarTM) and polyethyleneterephthalate (PET, Nalophan™).
According to the European Standard these materials shall be tested for suitability, by verifying they can hold a mixture of odourants with minimal changes for periods of storage of 30 hours, which is the maximum storage time allowed by the European Standard.
Some authors have been studying the characteristics of the materials listed in the EN 13725 [10] with the aim to verify their suitability for olfactometric measurements. Previous studies have shown that FEP bags are quite inert but not very robust and rather expensive [11] . PVF bags are more robust but they have a background odour caused by the use of solvents during production [12] . These disadvantages are the reason why PET, which is relatively cheap and odourless, is actually the most widely used material for the realization of sample bags [11] [13] -[16] .
Many studies have been conducted in order to assess the diffusion of odorous molecules through polymeric films [11] [17] -[23] . In these studies, chemical analyses have been performed to quantify the losses of specific compounds over time and to compare the recovery efficiency of different materials [11] [17] -[19] [24] -[30] .
Despite of its inertia and cost effectiveness NalophanTM has been proved to allow the diffusion of specific molecules, such as water, and its permeability has been studied.
Both the nature of the polymer and nature of the diffusing molecule affect the diffusion rate through the material that is expressed by the diffusion coefficient D [31] .
Water can diffuse quickly through polymeric films because of its structure [14] . Also other molecules having a dimension similar to water, such as ammonia (NH3) and hydrogen sulphide (H2S) [14] [32] [33] , which are typically found in emissions from several operations such as solid waste and waste water treatment, can diffuse easily.
The characteristics of the polymer itself affecting the diffusion processes are: the chemical nature of the polymer, its crystalline structure and orientation, the free volume, the molecular cohesion, the relative humidity, temperature, hydrogen bonding, polarity, solubility parameter, solvent size and shape [34] -[36] .
The experiments described in this paper have the aim to investigate the diffusion phenomena through NalophanTM, which is one of the most widespread materials used for the realization of sampling bags (EN 13725:2003), thereby calculating the diffusion coefficient relevant to this material. Ammonia was chosen as target compound for the study, which involved both an experimental part aiming to calculate the specific D coefficient through NalophanTM as well as to evaluate the influence of the surface/volume ratio on the diffusion kinetics. The described approach is important in order to increase knowledge in this field, suggesting possible technical expedients to reduce diffusion, and possibly improve regulatory issue.
2. Materials and Methods
2.1. Materials
The NalophanTM used to fabricate the bags employed for the experimental tests consists in a one-layer foil of polyterephtalic ester copolymer with 20-µm thickness supplied by Tilmmanns S.p.A.
The bags were obtained starting from a tubular film cut in different lengths.
One end was equipped with a clamp closure while the other end is provided with a Teflon inlet tube for sample collection (Figure 1).
The NH3 decay over time was evaluated using gas-chromatography (GC) for the quantification of NH3 concentration inside the bag. The ammonia concentration was measured using a HP Agilent 6890 gas chromatograph equipped with an Agilent HP-5MS fused silica capillary column (CP 7591-PoraPlot Amines, length 25 m, internal diameter 0.32 mm, film thickness 10 μm). The oven temperature follows a three steps program: 100˚C for 12 minutes, from 100˚C to 200˚C with a rate of 8˚C/min, 200˚C for 5 minutes. The carrier gas was helium with a constant flow of 3 mL/min (pressure of 1.21 atm and mean velocity of 53 cm/s). The gaseous mixture inside the bags was analysed by a GC, equipped with a TCD detector, at specific time intervals, in order to evaluate the variations of NH3 concentration (ppm) over time.
A calibration curve was built to relate the area of the GC peak with the NH3 concentration (ppm). Instrument calibration was performed analysing different standard concentrations of NH3 in air ranging from 10,000 to 60,000 ppm. Standards were obtained starting from different liquid mixtures of NH3 in water and analysing the headspace obtained in a fixed volume of air where the liquid was inserted and then kept at a controlled temperature.
All the tested samples were realized by filling the NalophanTM bags with a gaseous mixture of ammonia in wet air, with an ammonia concentration of about 55,000 ppmV and a relative humidity of 60%, which will be defined as the “test mixture”. The test mixture was prepared using the headspace technique. The liquid phase was realized at room temperature mixing 10.5 ml of a liquid solution of NH3 at a concentration of 30% w/w and 50 ml of distilled water.
During storage, physical parameters like temperature and relative humidity were kept under control using a climatic chamber (Chamber GHUMY by Fratelli Galli, Milano, Italy).
2.2. Methods
All tests were conducted by measuring the NH3 concentration at different time intervals after sample preparation. More in detail, NH3 was analysed, every hour, from 0 to 26 h. Each measurement involved the withdrawal of 300 µl of the test mixture by means of a syringe and the injection in the GC.
The diffusion of ammonia was first evaluated through a NalophanTM bag having a capacity of about 6000 cm3 and a surface equal to 2580 cm2. This bag was filled with 6000 cm3 of the above defined test mixture and then stored at a constant temperature of 23˚C and an external relative humidity of 60%. The external relative humidity was set equal the internal relative humidity in order to avoid water diffusion during storage and its potential influence on ammonia diffusion. Based on the experimental data of residual NH3 concentration inside the bag and on the Fick’s law, the diffusion coefficient D of ammonia through NalophanTM was calculated. The measurements were repeated three times each and the diffusion coefficient D is averaged over the 26 hours.
The role of the exchange surface (i.e., the bag surface area) on the NH3 concentration decay inside the bag was evaluated by realizing bags having different surface areas, i.e. 1900 cm2, 2580 cm2, 3520 cm2, respectively. These bags have different capacities (3000, 6000 and 9000 cm3), but they were filled with the same amount (3000 cm3) of the test mixture, thus realizing bags with a different surface-to-volume ratio.
Table1 Experimental data relevant to NH3 diffusion over time in a NalophanTM bag with VN = 6000 cm3 and SN = 2580 cm2

Figure 1. NalophanTM bags.
Also in this case, all tested samples were stored at a T of 23˚C and RH of 60% for the whole duration of the test. The ammonia concentrations over time were measured according to the above described test protocol.
3. Calculations
The diffusion phenomena through a polymeric film are described by the Fick’s law. According to it, the specific molar flow is defined as:
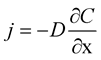 (1)
(1)
where:
• j is the specific molar flow (mol/m2/s)
• D is the diffusion coefficient of the compound through the film (m2/s)
• C is the concentration of the diffusing compound (mol/m3)
• x is the differential thickness of the film.
The film thickness can therefore be expressed as:
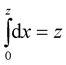 (2)
(2)
where z is the film thickness (m);
Referring to Figure 2, which schematizes the diffusion phenomenon through thethin filmwhich constitutes the sampling bag, we can define:
• SN is the surface of the polymeric film (m2)
• zN is the thickness of the film (m)
• CN is the concentration in the inside volume (mol/m3)
• CN+1 is the concentration outside the film (mol/m3), for a single bag it is generally considered negligible (CN+1 = 0)• jN is the specific molar flow through the film (mol/m2/s).
If the film thickness can be considered as negligible, then the accumulation term inside the material is negligible, as well.
With this assumption jN is constant along the film (x).
By integrating Equation (1) in dx between 0 and ZN, the specific molar flow jN can be expressed as:
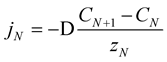 (3)
(3)
jN is relevant to an infinitesimal portion of the exchange surface dS.
Assuming that the internal molar concentration CN is constant inside the whole internal volume VN and also the external concentration CN+1 is constant inside the external volume VN+1, then the global flow J through the exchange surface SN can be calculated by integrating as follows:
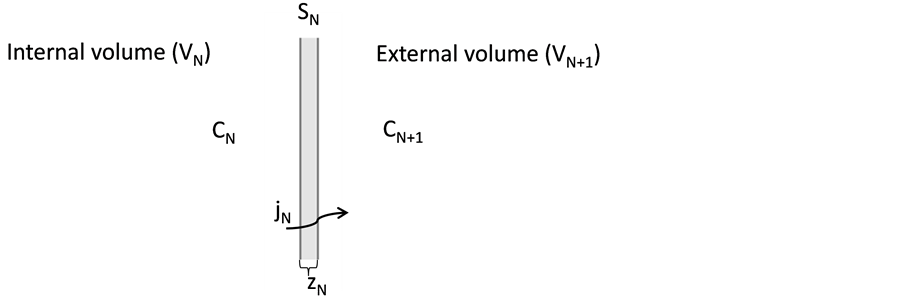
Figure 2. Schematization of diffusion through the thin film of the bag.
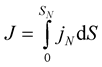 (4)
(4)
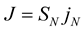 (5)
(5)
Combining Equation (3) with Equation (5), the molar flow through the surface is expressed as:
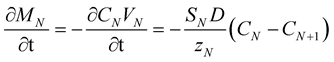 (6)
(6)
If the external concentration CN+1 is assumed to be equal to zero (CN+1 = 0), and if the volume VN+1 is taken equal to infinity (VN+1 = ∞), which is the case if the bag is placed in a neutral environment (where the presence of NH3 may be considered negligible), then Equation (6) can be rewritten as:
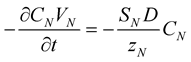 (7)
(7)
According to this model, the concentration decay over time turns out to be a function of the surface area (SN), the volume of the sampled gas VN, the film thickness (zN), the time (t), the diffusion coefficient (D) that depends on the characteristics of the material, and the concentration gradient through the polymeric barrier (∆C).
The boundary conditions considered for the integration of Equation (7) are:
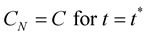 (8)
(8)
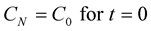 (9)
(9)
The integration of Equation (7) allows the calculation of the concentration trend over time:
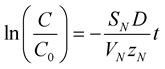 (10)
(10)
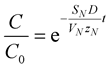 (11)
(11)
4. Results and Discussion
Table 1 shows the NH3 concentration values measured at different time intervals ti. Each concentration value reported in the table is the average of three replicate measurements.
The last column of Table 1 reports the diffusion coefficient Dti for each time interval ti calculated according to the following equation:
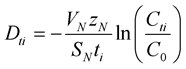 (12)
(12)
where ti is the time interval and Cti is the concentration measured after ti.
In order to give a better representation of the diffusion phenomena through the polymeric film, as well as to make it possible to compare results obtained with different bag filling volumes (VN), it was decided to make all further considerations about the bag contents considering the number of moles (n) instead of the concentrations.
For this reason, the third column of Table 1 reports the number of millimoles (mmol) contained in the bag, and the fourth column represents the number of moles divided by the bag surface (n/S). This parameter allows highlighting the differences obtained with different bag surfaces.
The fifth column reports ∆n, which is the difference between the number of moles at t0 = 0 h and the number of moles at time t (n0-n) and therefore represents the number of moles that have crossed the film.
∆n/t represents the number of moles passed through the film during the whole time interval t, thus representing the direction coefficient of the line connecting n0 and n, i.e. the average speed at which the moles have crossed the film.
The diffusion coefficient of ammonia through NalophanTM is finally calculated as the average of the different values of Dti weighted on the corresponding storage time ti:
.
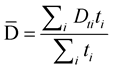 (13)
(13)
The resulting value for
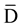 is equal to 2.38 10−8 cm2/s, with a standard deviation
equal to 3.70 10−11 cm2/s.
is equal to 2.38 10−8 cm2/s, with a standard deviation
equal to 3.70 10−11 cm2/s.
The percent NH3 loss through the bag over time can be expressed as:
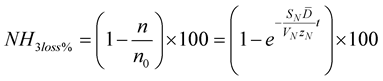 (14)
(14)
Figure 3 shows the experimental NH3 losses over time during the test period of 26 h. These results were obtained using a bag with a surface of 2580 cm2 filled with 6000 cm3 of the test mixture (surface-to-volume ratio equal to 0.430 cm−1). The frequency of the measurements of the ammonia losses was focused on the first hours of the storage time (1 - 7 h) in order to investigate the initial concentration decrease trend, and close to the limit storage time (23 - 26 h) imposed by the European norm, which is 30 h, in order to evaluate the cumulative losses.
The loss percentage of NH3 (%) after 26 h turns out to be equal to about 37%.
This trend is coherent with other data reported in scientific literature dealing with the same subject. As an example, a study by Akdezin et al. [37] also dealing with NH3 losses through polymeric films, reports losses of about 25% after 48 h. This value is lower compared to the 37% found in this study and reported in Figure 3. This may be due to the fact that the starting NH3 concentration is much lower (ppb) than in our case (thousands of ppm), thus resulting in a lower concentration gradient, which is the driving force of the diffusion phenomenon.
A similar trend was observed in other studies by Beghi and Guillot [14] [33] , which investigate H2S diffusion through different NalophanTM film having a different thickness. Also in this case, the reported H2S losses through a 20 µm thick NalophanTM film are lower, presumably due to the lower starting concentration.
Figure 4 represents the experimental data relevant to the number of NH3 moles over time (third column of Table 1). The experimental data show a good correspondence with the theoretical trend derived from Equation (11), which can be alternatively expressed as:
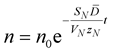 (15)
(15)
The theoretical trend is shown in Figure 4 as a continuous line. This trend was obtained by inserting, in Equation (15), the averaged diffusion coefficient calculated as described above (Equations (12) and (13)).
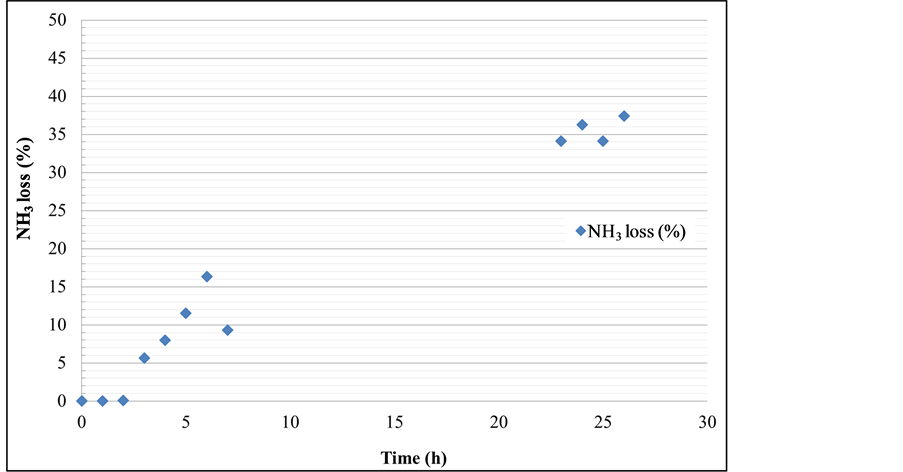
Figure 3. NH3 loss (%) over time from the NalophanTM bag with VN = 6000 cm3 and SN = 2580 cm2.
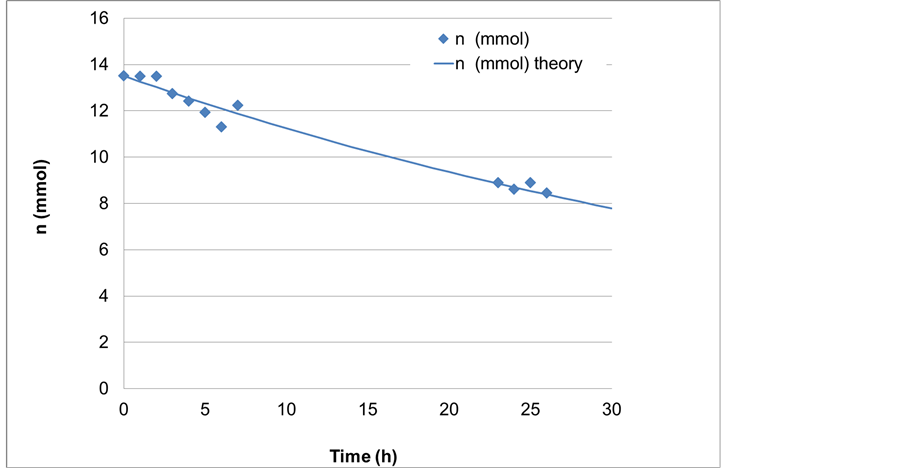
Figure 4. Number of NH3 moles over time: experimental data (dots) vs. theoretical trend (continuous line) in a NalophanTM bag with VN = 6000 cm3 and SN = 2580 cm2.
As described by Equation (15), the variation of moles inside the bag depends on
the surface-to-volume ratio ( , hereafter defined simply as
, hereafter defined simply as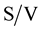 ) of the bag.
) of the bag.
In order to quantify this effect, different tests were performed using bags having different surface-to-volume ratios. This was realized by fabricating bags having different surface areas (i.e. S = 1900 cm2, 2580 cm2, 3520 cm2, respectively) and therefore different capacities, and then filling them with the same amount (V = 3000 cm3) of the test mixture. Thus the surface-to-volume ratio was changed: the surface area SN was varied while the gas volume V was kept constant.
Table 2 reports the number of NH3 moles (n) and their percent variation over time (Δn%) for the three bags having different surface-to-volume ratios, as described above. It is possible to observe that the percent variation of ammonia in the considered 26 h interval increases with the bag exchange surface S, passing from 51% for the bag with the smaller surface (S = 1900 cm2) to 72% for the bag with the larger surface (S = 3520 cm2).
Table 2 also reports the ratio between NH3 moles and the bag surface (n/S): this ratio allows better discriminating the experimental data relevant to the bags having different surface-to-volume ratios, which are shown in Figure 5. Figure 5 also illustrates the theoretical trends, calculated based on Equation (15), thereby the surface S for each bag, and the average diffusion coefficient obtained from the experimental data (2.38 10−8 cm2/s).
A good correspondence is observed between experimental and theoretical trends.
Figure 6 illustrates the ammonia loss percentage over time for bags filled with the same amount (V = 3000 cm3) of the test mixture, but with different surfaces. As already mentioned, diffusion is accentuated in the bags with a larger exchange surface.
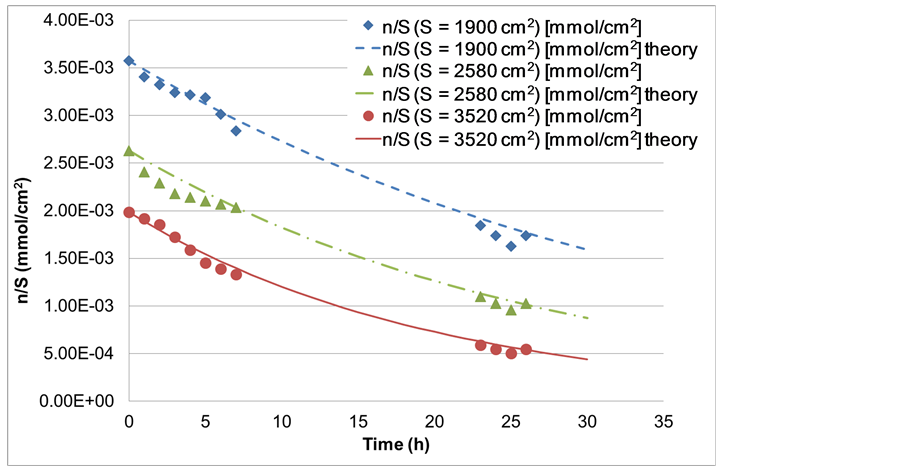
Figure 5. n/S trends for the three bags having different surfaces (1900 cm2, 2580 cm2 and 3520 cm2, respectively) and different S/V ratios (0.63 cm−1, 0.86 cm−1 and 1.17 cm−1, respectively).
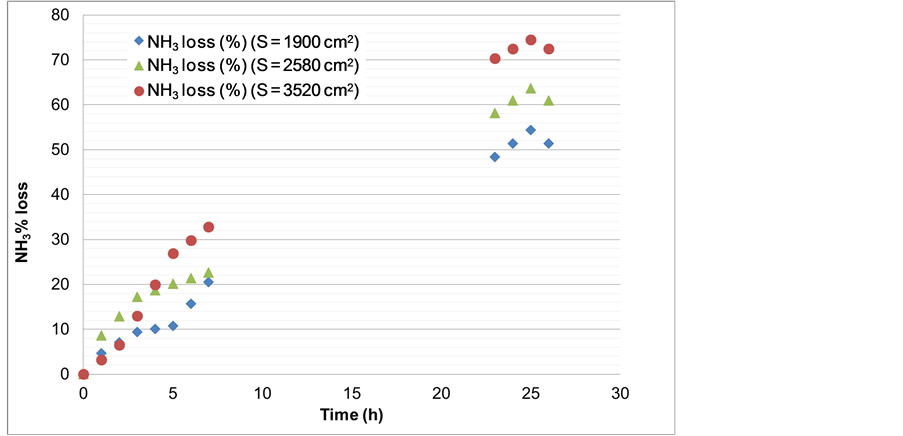
Figure 6. NH3 loss (%) over time from the three bags having different surfaces (1900 cm2, 2580 cm2 and 3520 cm2, respectively) and different S/V ratios (0.63 cm−1, 0.86 cm−1 and 1.17 cm−1, respectively).
Table3 Number of moles and average permeation speed for different S/V ratios
The bag with a surface of 1900 cm2 and a surface-to-volume ratio of 0.633 cm−1 gives a NH3 loss percentage for prolonged storage times (26 h) of about 50%. This loss increases to about 60% for the bag with a surface of 2580 cm2 and a surface-to-volume ratio of 0.860 cm−1, and to about 70% for the bag with a surface of 3520 cm2 and a surface-to-volume ratio of 1.17 cm−1. The experimental data are in agreement with the theoretical trend expressed by Equation (15), which indicates that, at a given time t, n/n0 is lower, i.e. the NH3 loss percentage is higher, for higher surface-to-volume ratios SN/VN. The same assumption is discussed in Sironi et al. [23] .
In order to evaluate the diffusion as a function of the surface-to-volume (S/V) ratio, it is possible to use the data relevant to the different tests described in this section (see Table 1 and Table 2) by fixing the time t, and then comparing the different values of Δn (i.e. the total number of moles that have crossed the film at time t) or Δn/t (i.e. the average speed at which the moles have crossed the film) obtained for the bags having different S/V ratios. In order to make the results of the first test (Table 1) comparable with those of the other tests (Table 2), given the different number of initial moles n0, the data have to be normalized with respect to n0, thus representing Δn/n0 or Δn/t/n0. As an example, Table 3 reports the experimental values of Δn/n0 and Δn/t/n0 measured after 7 hours for the different bags (having different S/V). The last columns of Table 3 report the theoretical data, as calculated based on Equation (15).
The values of Δn/t/n0, i.e. the average permeation speed in the first 7 hours of storage normalized with respect to n0, both measured experimentally and calculated with Equation (15), are also represented in Figure 7.
The data reported in Table 3 and Figure 7 further prove how the surface-to-volume ratio affects diffusion: the average diffusion speed increases with the S/V ratio, thus resulting in higher percent losses after a given storage time t.
This means that, for a given material, to which corresponds a given diffusion coefficient D, one way to reduce diffusion over time is trying to reduce the surface-to-volume ratio.
For bags of given dimensions, i.e., same surface and same maximum capacity, the only way to minimize S/V is to fill the bags to their maximum capacity. Bags filled only partially will have a higher S/V and therefore higher losses over time.
Another way to reduce S/V is to realize bigger bags. As an example, if cylindrical bags having a slenderness ratio (i.e., h/D) equal to 2 are considered, the bag surface will be:
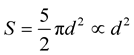
where d is the diameter of the bag. If the bag is filled completely with the sample gas, then gas volume V is equal to the bag volume:

Figure 7. Average permeation speed (t = 7 h) normalized with respect to n0 for different values of S/V.
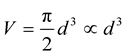
The surface-to-volume ratio in this case depends on the diameter:
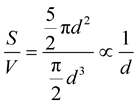
And will therefore be proportional to the inverse of the diameter, i.e. decrease with the bag capacity.
Moreover, for a given d, S/V may be reduced by reducing the slenderness ratio, giving that the lowest S/V ratio is obtained for a slenderness ratio equal to 1, i.e. h = D.
Similar considerations can be made considering other bag shapes, always giving that diffusion phenomena can be reduced by using bigger bags, filled to their maximum capacity.
5. Conclusions
This study allowed to evaluate and to quantify the phenomenon of ammonia diffusion through NalophanTM films.
The experimental determinations allowed the calculation of the diffusion coefficient of ammonia through NalophanTM according to the Fick’s law, which turned out to be equal to 2.38 10−8 cm2/s at a temperature of 23˚C and a relative humidity of 60%.
The ammonia losses from the NalophanTM sampling bag always turned out to be significant; for instance, in the case of a bag with a surface of 2580 cm2 filled with 6000 cm3 of gas (i.e. a “test mixture” of ammonia in air, at fixed temperature and relative humidity), the percent ammonia loss after 26 h was 37%. This value is not negligible especially considering that the European Norm EN 13725:2003 allows a maximum storage time of 30 hours, thus assuming that the sampled mixture remains almost unaltered for 30 hours.
This study discusses the effect of the exchange surface on diffusion, by highlighting to which extent the surface-to-volume ratio affects the diffusion rate.
Diffusion was tested in bags with different S/V giving that the bag with a surface-to-volume ratio of 0.633 cm−1 has a NH3 loss percentage after 26h of about 50%, while this loss increases to about 70% for the bag with a surface-to-volume ratio of 1.17 cm−1. The experimental data are in agreement with the theoretical trend derived from the Fick’s law, which indicates that, at a given time t, n/n0 is lower, i.e. the percent NH3 loss is higher, for higher surface-to-volume ratios SN/VN. Of course, the percentages of losses obtained during the presented experiments corresponds to a range of ammonia concentration. These losses can present different values if ammonia concentrations are in other ranges (higher or lower).
This means that, for a given material, to which corresponds a given diffusion coefficient D, one way to reduce diffusion over time is trying to reduce the surface-to-volume ratio. As a consequence, diffusion phenomena can be reduced by using bigger bags, filled to their maximum capacity. For cylindrical bags obtained from tubular NalophanTM, S/V can be minimized by realizing bags with a slenderness ratio (h/D) equal to 1.
References
- Capelli, L., Sironi, S., Del Rosso, R., Centola, P., Rossi, A. and Austeri, C. (2011) Olfactometric Approach for the Evaluation of Citizens’ Exposure to Industrial Emissions in the City of Terni, Italy. Science of the Total Environment, 409, 595-603. http://dx.doi.org/10.1016/j.scitotenv.2010.10.054
- Nicell, J.A. (2009) Assessment and Regulation of Odour Impacts. Atmospheric Environment, 43, 196-206. http://dx.doi.org/10.1016/j.atmosenv.2008.09.033
- Schulz, T.J. and Harreveld, A.P.V. (1996) International Moves towards Standardisation of Odour Measurement Using Olfactometry. Water Science and Technology, 34, 541-547. http://dx.doi.org/10.1016/0273-1223(96)00594-X
- Sironi, S. and Botta, D. (2001) Biofilter Efficiency in Odor Abatement at Composting Plants. Compost Science & Utilization, 9, 149-155. http://dx.doi.org/10.1080/1065657X.2001.10702029
- Capelli, L., Sironi, S., Del Rosso, R., Centola, P. and Bonati, S. (2010) Improvement of Olfactometric Measurement Accuracy and Repeatability by Optimization of Panel Selection Procedures. Water Science and Technology, 61, 1267-1278. http://dx.doi.org/10.2166/wst.2010.023
- Bokowa, A.H. (2008) The Effect of Sampling on the Measured Odour Concentration. Chemical Engineering Transactions, 23, 43-48.
- Bourgeois, W., Romain, A.C., Nicolas, J. and Stuetz, R.M. (2003) The Use of Sensor Arrays for Environmental Monitoring: Interests and Limitations. Journal of Environmental Monitoring, 5, 852-860. http://dx.doi.org/10.1039/b307905h
- Laor, Y., Ozer, Y., Ravid, U., Hanan, A. and Orenstein, P. (2010) Methodological Aspects of Sample Collection for Dynamic Olfactometry. Chemical Engineering Transactions, 23, 55-60.
- Kim, Y.-H. and Kim, K.-H. (2012) Experimental Approach to Assess Sorptive Loss Properties of Volatile Organic Compounds in the Sampling Bag System. Journal of Separation Science, 35, 2914-2921. http://dx.doi.org/10.1002/jssc.201200388
- CEN (2003) EN13725 Air Quality—Determination of Odour Concentration by Dynamic Olfactometry. Comite Europe en de Normalisation (CEN), Brussels.
- van Harreveld, A.P. (2003) Odor Concentration Decay and Stability in Gas Sampling Bags. Journal of the Air & Waste Management Association, 53, 51-60. http://dx.doi.org/10.1080/10473289.2003.10466121
- Juarez-Galan, J.M., Martinez, J.V., Amo, A. and Valor, I. (2008) Background Odour from Sampling Bags. Influence on the Analysis of Odour Concentration. Chemical Engineering Transactions, 15, 87-94.
- van Harreveld, A.P., Heeres, P. and Harssema, H. (1999) A Review of 20 Years of Standardization of Odor Concentration Measurement by Dynamic Olfactometry in Europe. Journal of the Air & Waste Management Association, 49, 705-715.
- Guillot, J.M. and Beghi, S. (2008) Permeability to Water Vapour and Hydrogen Sulphide of Some Sampling Bags Recommended by EN 13725. Chemical Engineering Transactions, 15, 79-86.
- Ghosh, S., Kim, K.H. and Sohn, J.R. (2011) Some Insights into Analytical Bias Involved in the Application of Grab Sampling for Volatile Organic Compounds: A Case Study against Used Tedlar Bags. The Scientific World Journal, 11, 2160-2177. http://dx.doi.org/10.1100/2011/529532
- Wang, C., Lai, P.C., Syu, S.H. and Leu, J. (2011) Effects of CF4 Plasma Treatment on the Moisture Uptake, Diffusion, and WVTR of Poly(ethylene terephthalate) Flexible Films. Surface and Coatings Technology, 206, 318-324. http://dx.doi.org/10.1016/j.surfcoat.2011.07.026
- Koziel, J.A., Spinhirne, J.P., Lloyd, J.D., Parker, D.B., Wright, D.W. and Kuhrt, F.W. (2005) Evaluation of Sample Recovery of Malodorous Livestock Gases from Air Sampling Bags, Solid-Phase Microextraction Fibers, Tenax TA Sorbent Tubes, and Sampling Canisters. Journal of the Air & Waste Management Association, 55, 1147-1157. http://dx.doi.org/10.1080/10473289.2005.10464711
- Cariou, S. and Guillot, J.M. (2006) Double-Layer Tedlar Bags: A Means to Limit Humidity Evolution of Air Samples and to Dry Humid Air Samples. Analytical and Bioanalytical Chemistry, 384, 468-474. http://dx.doi.org/10.1007/s00216-005-0177-4
- Trabue, S.L., Anhalt, J.C. and Zahn, J.A. (2006) Bias of Tedlar Bags in the Measurement of Agricultural Odorants. Journal of Environmental Quality, 35, 1668-1677. http://dx.doi.org/10.2134/jeq2005.0370
- Mochalski, P., Wzorek, B., Sliwka, I. and Amann, A. (2009) Suitability of Different Polymer Bags for Storage of Volatile Sulphur Compounds Relevant to Breath Analysis. Journal of Chromatography B: Analytical Technologies in the Biomedical and Life Sciences, 877, 189-196. http://dx.doi.org/10.1016/j.jchromb.2008.12.003
- Parker, D.B., Perschbacher-Buser, Z.L., Cole, N.A. and Koziel, J.A. (2010) Recovery of Agricultural Odors and Odorous Compounds from Polyvinyl Fluoride Film Bags. Sensors, 10, 8536-8552. http://dx.doi.org/10.3390/s100908536
- Hansen, M.J., Adamsen, A.P.S., Feilberg, A. and Jonassen, K.E.N. (2011) Stability of Odorants from Pig Production in Sampling Bags for Olfactometry. Journal of Environmental Quality, 40, 1096-1102. http://dx.doi.org/10.2134/jeq2010.0497
- Sironi, S., Eusebio, L., Dentoni, L., Capelli, L. and Del Rosso, R. (2014) Ammonia Diffusion through NalophanTM Bags. Water Science and Technology, 69, 486-494. http://dx.doi.org/10.2166/wst.2013.700
- Sulyok, M., Haberhauer-Troyer, C., Rosenberg, E. and Grasserbauer, M. (2001) Investigation of the Storage Stability of Selected Volatile Sulfur Compounds in Different Sampling Containers. Journal of Chromatography A, 917, 367-374. http://dx.doi.org/10.1016/S0021-9673(01)00654-9
- Sulyok, M., Haberhauer-Troyer, C. and Rosenberg, E. (2002) Observation of Sorptive Losses of Volatile Sulfur Compounds during Natural Gas Sampling. Journal of Chromatography A, 946, 301-305. http://dx.doi.org/10.1016/S0021-9673(01)01541-2
- Kim, K.H. (2006) A Study of Sorptive Loss Patterns for Reduced Sulfur Compounds in the Use of the Bag Sampling Method. Environmental Monitoring and Assessment, 123, 259-269. http://dx.doi.org/10.1007/s10661-006-9195-8
- Kim, K.H., Choi, G.H., Choi, Y.J., Song, H.N., Yang, H.S. and Oh, J.M. (2006) The Effects of Sampling Materials Selection in the Collection of Reduced Sulfur Compounds in Air. Talanta, 68, 1713-1719. http://dx.doi.org/10.1016/j.talanta.2005.08.037
- Saìz, J., Ferrando, J.L., Atoche, J.C., Torre, M. and Ruiz, C.G. (2011) Study of Losses of Volatile Compounds from Dinamite. Investigation of Cross-Contamination between Dynamites Stored in Polyethylene Bags. Forensic Science International, 211, 27-33. http://dx.doi.org/10.1016/j.forsciint.2011.04.007
- Jo, S.H., Kim, K.H., Shon, Z.H. and Parker, D. (2012) Identification of Control Parameters for the Sulfur Gas Storability with Bag Sampling Methods. Analytica Chimica Acta, 738, 51-58. http://dx.doi.org/10.1016/j.aca.2012.06.010
- Kim, Y.H., Kim, K.H., Jo, S.H., Jeon, E.C., Sohn, J.R. and Parker, D.B. (2012) Comparison of Storage Stability of Odorous VOCs in Polyester Aluminum and Polyvinyl Fluoride Tedlar (R) Bags. Analytica Chimica Acta, 712, 162-167. http://dx.doi.org/10.1016/j.aca.2011.11.014
- Crank, J. and Park, G.S. (1968) Diffusion in Polymers. 1st Edition, Academic Press, London and New York.
- Beghi, S. and Guillot, J.M. (2006) Sample Water Removal Method in Volatile Organic Compound Analysis Based on Diffusion through Poly(vinyl fluoride) Film. Journal of Chromatography A, 1127, 1-5. http://dx.doi.org/10.1016/j.chroma.2006.05.102
- Beghi, S. and Guillot, J.M. (2008) Use of Poly(ethylene terephthalate) Film Bag to Sample and Remove Humidity from Atmosphere Containing Volatile Organic Compounds. Journal of Chromatography A, 1183, 1-5. http://dx.doi.org/10.1016/j.chroma.2007.12.051
- Igwe, I.O., Ewulonu, C.M. and Igboanugo, I. (2006) Studies on the Diffusion Characteristics of Some Aromatic Solvents into Polypropylene Film. Journal of Applied Polymer Science, 102, 1985-1989. http://dx.doi.org/10.1002/app.24593
- Mallia, I. (2010) Sviluppo di una nuova metodologia per la misura della permeabilita di film plastici ad uso alimentare. Thesys Dissertation, Universita degli studi di Catania, Catania.
- Piergiovanni, L. and Limbo, S. (2010) Proprieta chimiche dei materiali di packaging. Food packaging, Springer-Verlag, Milan.
- Akdeniz, N., Janni, K.A., Jacobson, L.D. and Hetchler, B.P. (2011) Comparison of Gas Sampling Bags to Temporarily Store Hydrogen Sulfide, Ammonia, and Greenhouse Gases. Transactions of the ASABE, 54, 653-661. http://dx.doi.org/10.13031/2013.36468
NOTES

*Corresponding author.


