Advances in Pure Mathematics
Vol.3 No.5(2013), Article ID:35060,12 pages DOI:10.4236/apm.2013.35065
The Second Hochschild Cohomology Group for One-Parametric Self-Injective Algebras
Department of Mathematics, Taif University, Taif, KSA
Email: dak12le@hotmail.co.uk
Copyright © 2013 Deena Al-Kadi. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received March 12, 2013; revised April 30, 2013; accepted June 23, 2013
Keywords: Hochschild Cohomology; Self-Injective Algebras; Socle Deformation
ABSTRACT
In this paper, we determine the second Hochschild cohomology group for a class of self-injective algebras of tame representation type namely, which are standard one-parametric but not weakly symmetric. These were classified up to derived equivalence by Bocian, Holm and Skowroński in [1]. We connect this to the deformation of these algebras.
1. Introduction
This paper determines the second Hochschild cohomology group for all standard one-parametric but not weakly symmetric self-injective algebras of tame representation type. Bocian, Holm and Skowroński give, in [1], a classification of these algebras by quiver and relations up to derived equivalence. The algebras in [1] are divided into two types, namely the algebra 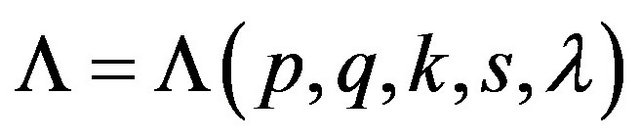 where
where  are integers such that p,
are integers such that p, 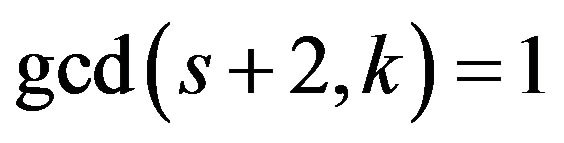
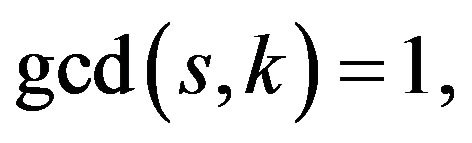
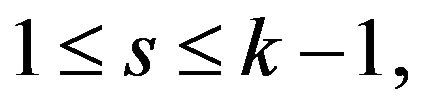
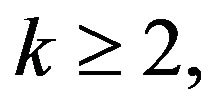
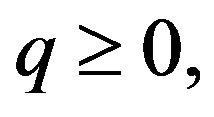 and
and 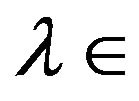
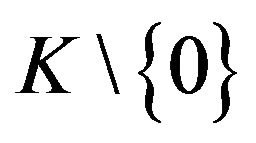 and the algebra
and the algebra 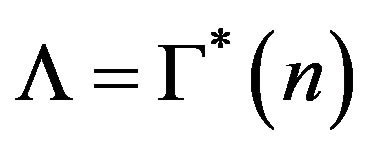 where
where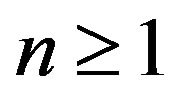 . Thus the second Hochschild cohomology group will be known for all the classes of the algebras given in [1]. We remark that an algebra of the type
. Thus the second Hochschild cohomology group will be known for all the classes of the algebras given in [1]. We remark that an algebra of the type 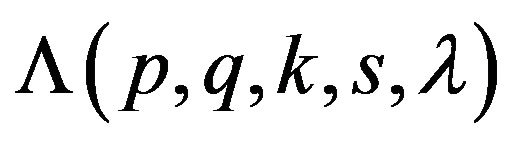 is never isomorphic to an algebra of the type
is never isomorphic to an algebra of the type 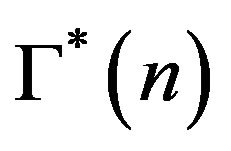 as their stable Auslander-Reiten quivers are not isomorphic. We refer the reader to [1] which gives precise conditions for two algebras of the same type
as their stable Auslander-Reiten quivers are not isomorphic. We refer the reader to [1] which gives precise conditions for two algebras of the same type 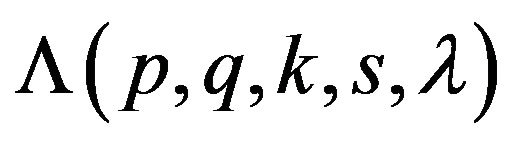 or
or 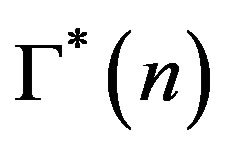 to be isomorphic.
to be isomorphic.
We start, in Section 2, by introducing the algebras , for both types, by quiver and relations. Section 3 of this paper describes the projective resolution of [2] which we use to find
, for both types, by quiver and relations. Section 3 of this paper describes the projective resolution of [2] which we use to find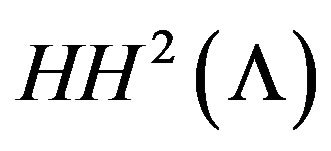 . In the third section, we determine
. In the third section, we determine 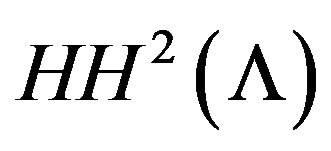 for the algebra
for the algebra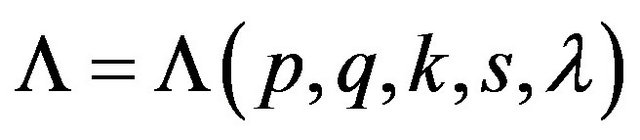 , considering separately the cases
, considering separately the cases 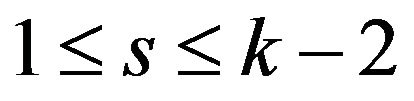 and
and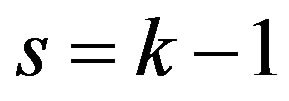 . The main result in this section is Theorem 4.9, which shows that
. The main result in this section is Theorem 4.9, which shows that 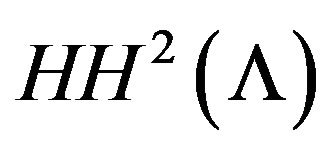 has dimension 1 for
has dimension 1 for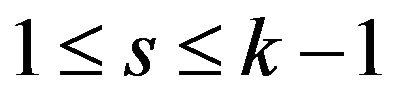 . This group measures the infinitesimal deformations of the algebra
. This group measures the infinitesimal deformations of the algebra ; that is, if
; that is, if 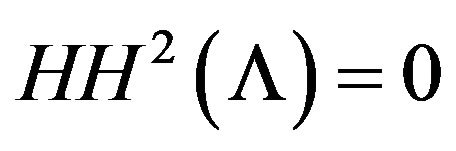 then
then  has no non-trivial deformations, which is not the case here. We include, in Section 4, Theorem 4.10 where we find a non-trivial deformation
has no non-trivial deformations, which is not the case here. We include, in Section 4, Theorem 4.10 where we find a non-trivial deformation  of
of  associated to our nonzero element
associated to our nonzero element  in
in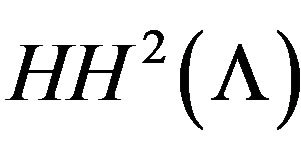 . This illustrates the connection between the second Hochschild cohomology group and deformation theory. In the final section, we determine
. This illustrates the connection between the second Hochschild cohomology group and deformation theory. In the final section, we determine 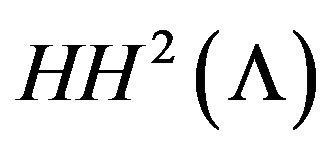 for
for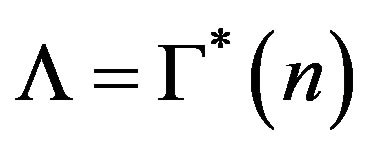 . The main result in Section 5 is Theorem 5.4 which shows that
. The main result in Section 5 is Theorem 5.4 which shows that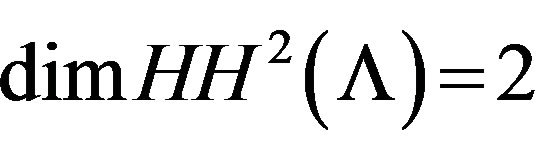 . The results we found in this paper are in contrast to the majority of self-injective algebras of finite representation type (see [3]). Since Hochschild cohomology is invariant under derived equivalence, the second Hochschild cohomology group is now known for the standard one-parametric but not weakly symmetric self-injective algebras of tame representation type which are derived equivalent to the algebra of the type
. The results we found in this paper are in contrast to the majority of self-injective algebras of finite representation type (see [3]). Since Hochschild cohomology is invariant under derived equivalence, the second Hochschild cohomology group is now known for the standard one-parametric but not weakly symmetric self-injective algebras of tame representation type which are derived equivalent to the algebra of the type 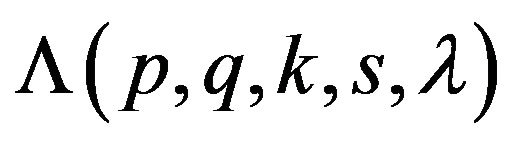 or
or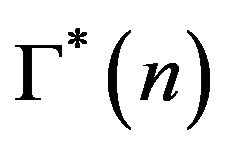 .
.
2. The One-Parametric Self-Injective Algebras
In this chapter we describe the algebras of [1]. We start with the algebra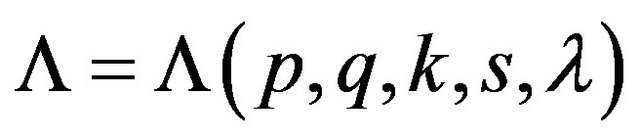 . Let K be an algebraically closed field and let
. Let K be an algebraically closed field and let 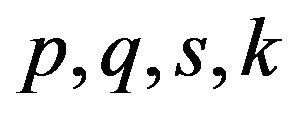 be integers such that p,
be integers such that p, 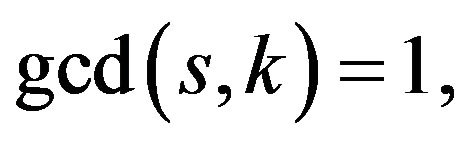
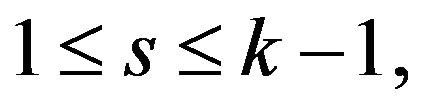
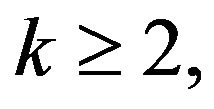
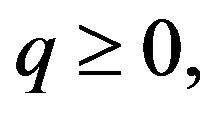
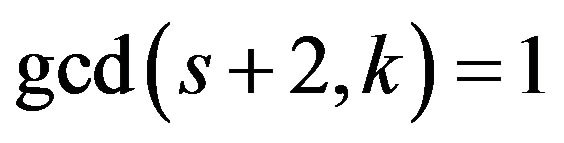 and
and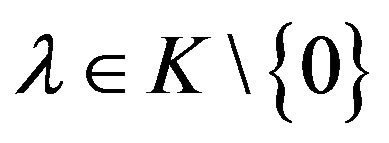 . From [1, Section 5],
. From [1, Section 5], 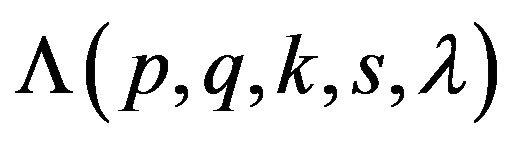 has quiver
has quiver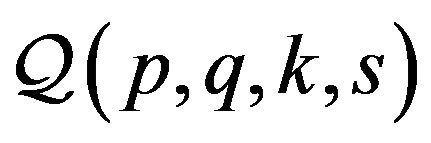 :
:
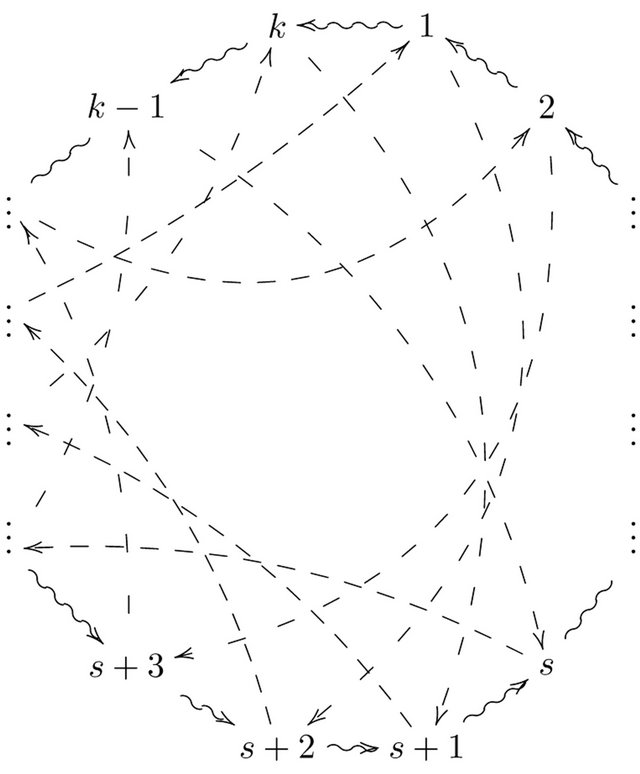
where, for any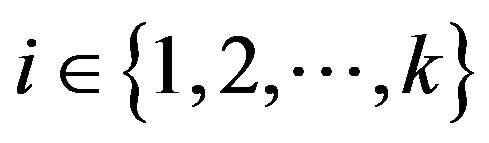 ,
,  denotes the path
denotes the path
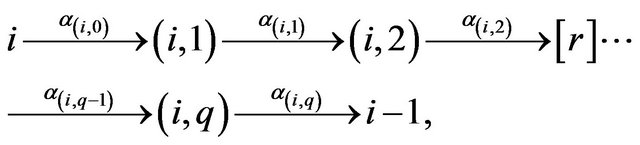
and 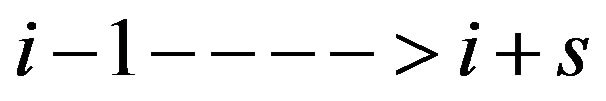 denotes the path
denotes the path
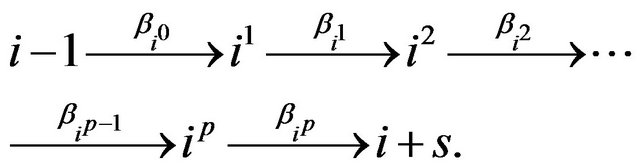
Then 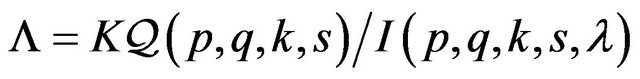 where
where 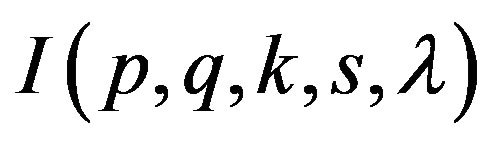 is the ideal generated by the relations
is the ideal generated by the relations
• ![]() , for
, for •
• 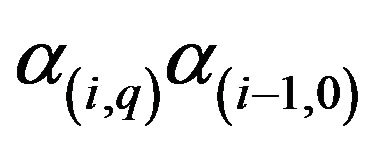 , for
, for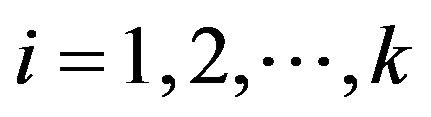 •
• 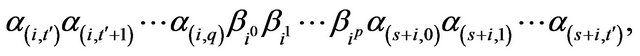 for
for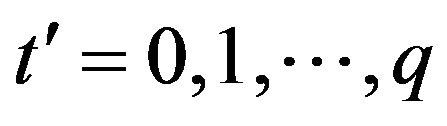 ,
, 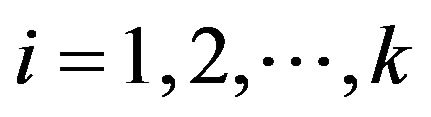 ,
,
![]()
![]() for
for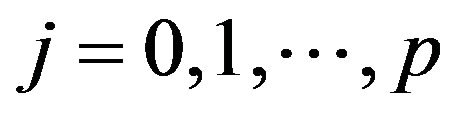 ,
, 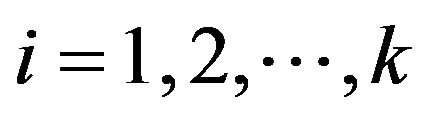 ,
,
![]()
![]() for
for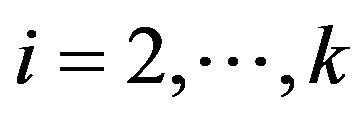 , and
, and
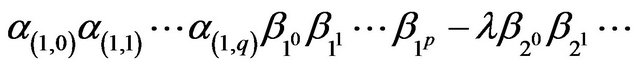
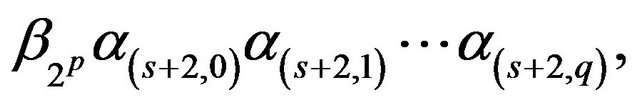 where
where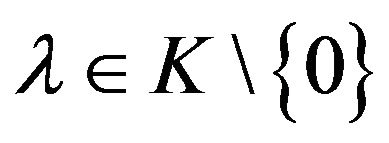 .
.
Next we describe the algebra 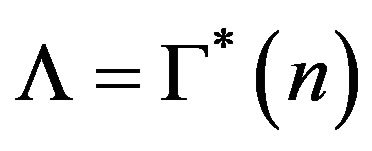 For
For ,
, 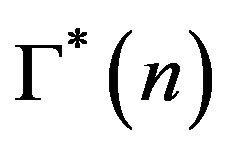 is given in [1, Section 6] by the quiver
is given in [1, Section 6] by the quiver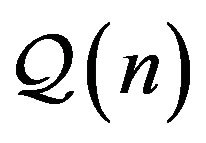 :
:
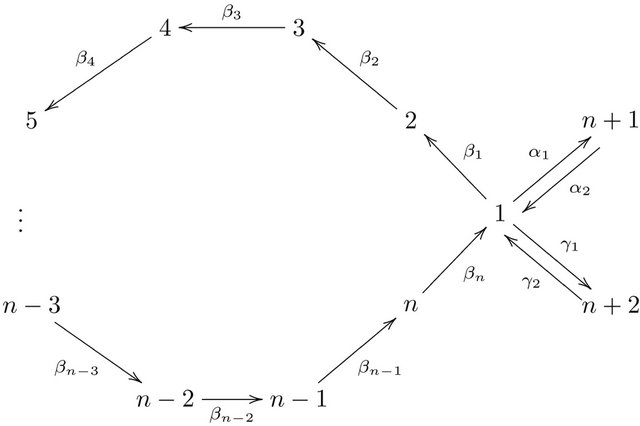
Then  where
where 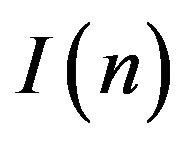 is the ideal generated by the relations:
is the ideal generated by the relations:
1) 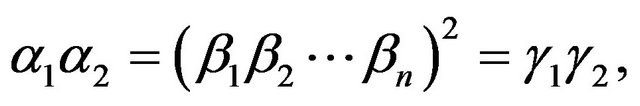
2) 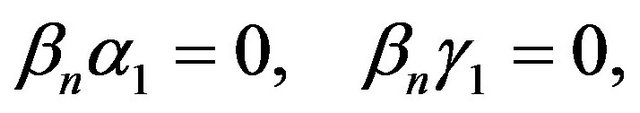
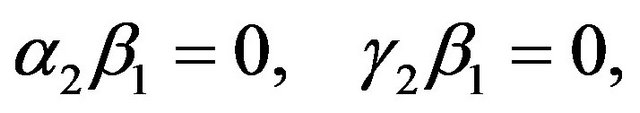
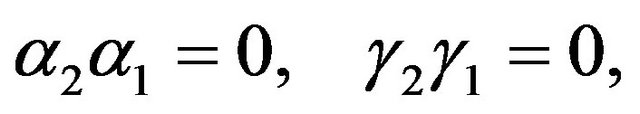
3) for all 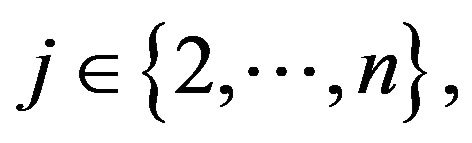
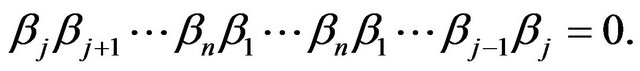
Note that we write our paths from left to right.
In order to compute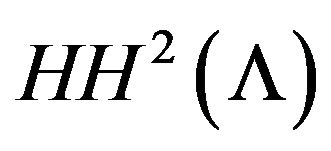 , the next section gives the necessary background required to find the first terms of the projective resolution of
, the next section gives the necessary background required to find the first terms of the projective resolution of  as a
as a 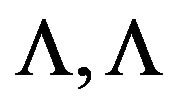 -bimodule. Section 4 and Section 5 uses this part of a minimal projective bimodule resolution for our algebras to determine the second Hochschild cohomology group and provides the main results of this paper.
-bimodule. Section 4 and Section 5 uses this part of a minimal projective bimodule resolution for our algebras to determine the second Hochschild cohomology group and provides the main results of this paper.
3. Projective Resolutions
To find the second Hochschild cohomology group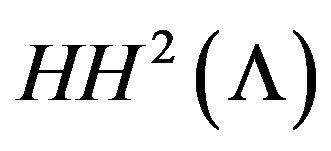 , we could use the bar resolution given in [4]. This bar resolution is not a minimal projective resolution of
, we could use the bar resolution given in [4]. This bar resolution is not a minimal projective resolution of  as
as 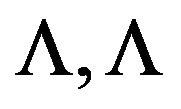 -bimodule. In practice, it is easier to compute the Hochschild cohomology group if we use a minimal projective resolution. So here we use the projective resolution of [2]. More generally, let
-bimodule. In practice, it is easier to compute the Hochschild cohomology group if we use a minimal projective resolution. So here we use the projective resolution of [2]. More generally, let 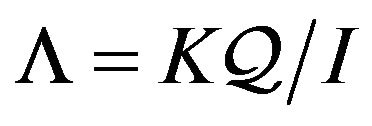 be a finite dimensional algebra, where K is an algebraically closed field,
be a finite dimensional algebra, where K is an algebraically closed field,  is a quiver, and I is an admissible ideal of
is a quiver, and I is an admissible ideal of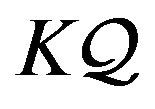 . Fix a minimal set
. Fix a minimal set 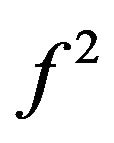 of generators for the ideal I. Let
of generators for the ideal I. Let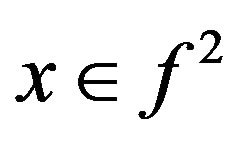 . Then
. Then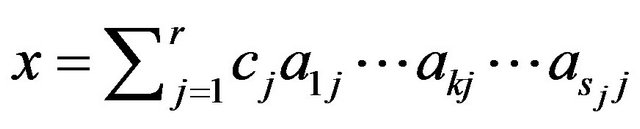 , that is, x is a linear combination of paths
, that is, x is a linear combination of paths 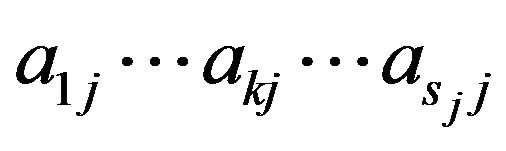 for
for 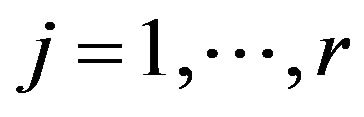 and
and 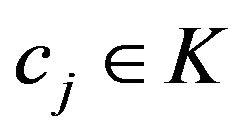 and there are unique vertices v and w such that each path
and there are unique vertices v and w such that each path  starts at v and ends at w for all j. We write
starts at v and ends at w for all j. We write 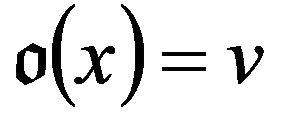 and
and 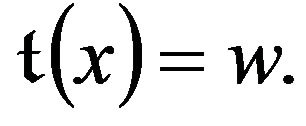 Similarly
Similarly 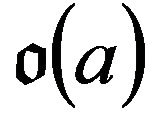 is the origin of the arrow a and
is the origin of the arrow a and 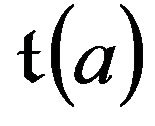 is the end of a.
is the end of a.
In [2, Theorem 2.9], it is shown that there is a minimal projective resolution of  as a
as a  -bimodule which begins:
-bimodule which begins:
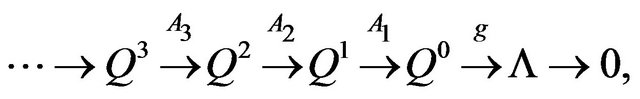
where the projective 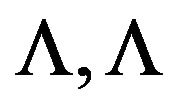 -bimodules
-bimodules 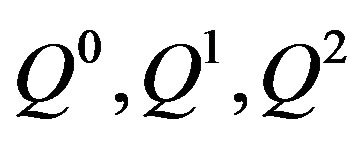 are given by
are given by

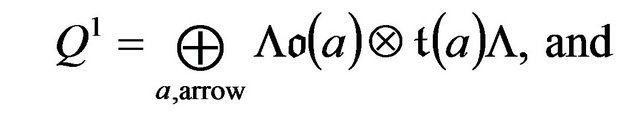
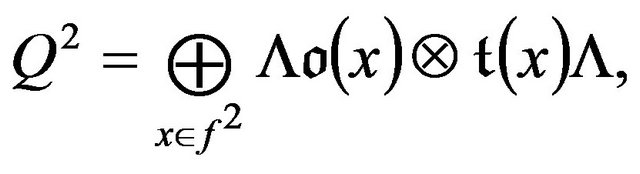
and the maps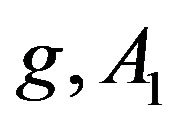 ,
, 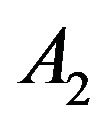 and
and 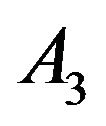 are
are 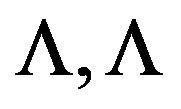 -bimodule homomorphisms, defined as follows. The map
-bimodule homomorphisms, defined as follows. The map 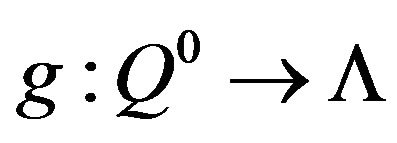 is the multiplication map so is given by
is the multiplication map so is given by . The map
. The map 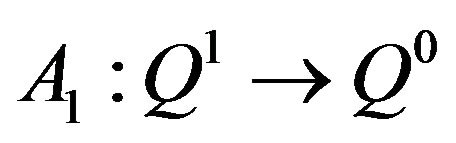 is given by
is given by

for each arrow![]() . With the notation for
. With the notation for 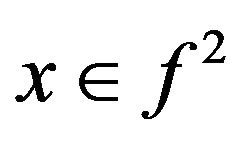 given above, the map
given above, the map 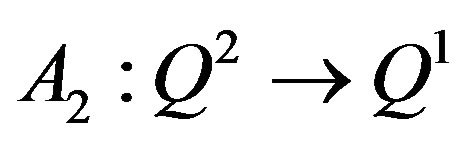 is given by
is given by
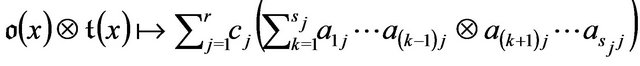 where
where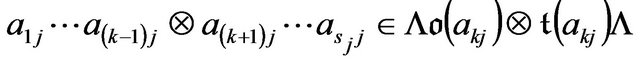 .
.
In order to describe the projective bimodule  and the map
and the map 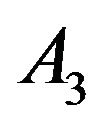 in the
in the 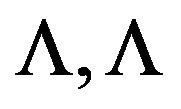 -bimodule resolution of
-bimodule resolution of  in [2], we need to introduce some notation from [5]. Recall that an element
in [2], we need to introduce some notation from [5]. Recall that an element 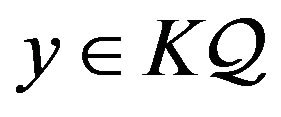 is uniform if there are vertices
is uniform if there are vertices 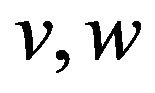 such that
such that 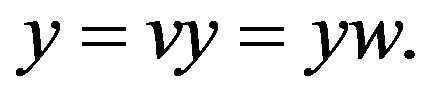 We write
We write 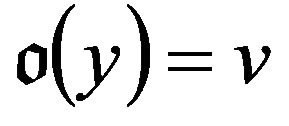 and
and . In [5], Green, Solberg and Zacharia show that there are sets
. In [5], Green, Solberg and Zacharia show that there are sets  in
in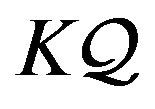 , for
, for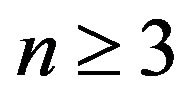 , consisting of uniform elements
, consisting of uniform elements 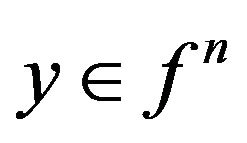 such that
such that
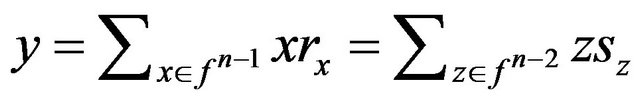
for unique elements 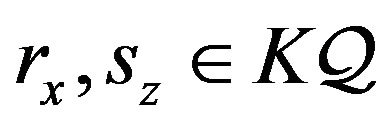 such that
such that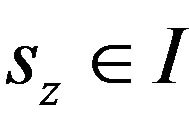 . These sets have special properties related to a minimal projective
. These sets have special properties related to a minimal projective  -resolution of
-resolution of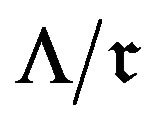 , where
, where  is the Jacobson radical of
is the Jacobson radical of . Specifically the n-th projective in the minimal projective
. Specifically the n-th projective in the minimal projective  -resolution of
-resolution of 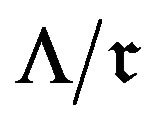 is
is
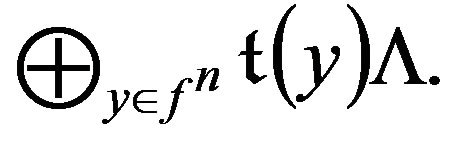
In particular, to determine the set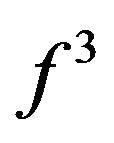 , we follow explicitly the construction given in [5, §1]. Let
, we follow explicitly the construction given in [5, §1]. Let 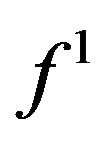 denote the set of arrows of
denote the set of arrows of . Consider the intersection
. Consider the intersection
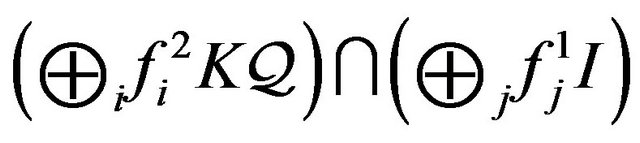 . Set this intersection equal to some
. Set this intersection equal to some . We then discard all elements of the form
. We then discard all elements of the form  that are in
that are in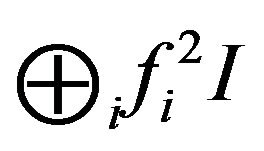 ; the remaining ones form precisely the set
; the remaining ones form precisely the set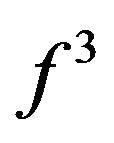 .
.
Thus, for 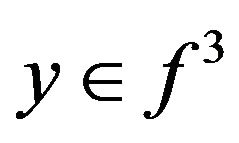 we have that
we have that
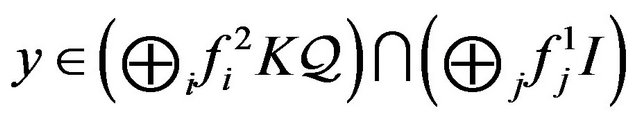 . So we may write
. So we may write
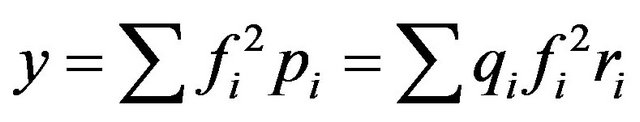 with
with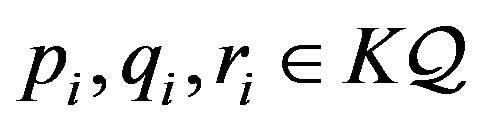 , such that
, such that 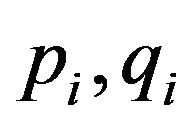 are in the ideal generated by the arrows of
are in the ideal generated by the arrows of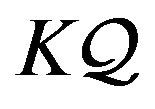 , and
, and 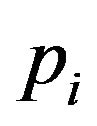 unique. Then [2] gives that
unique. Then [2] gives that
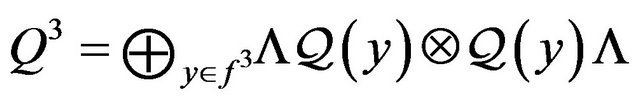 and, for
and, for 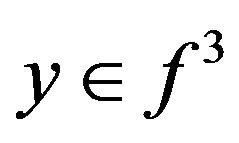 in the notation above, the component of
in the notation above, the component of 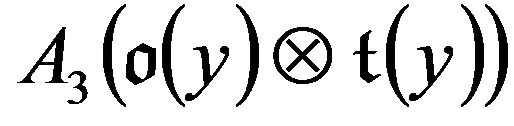 in the summand
in the summand 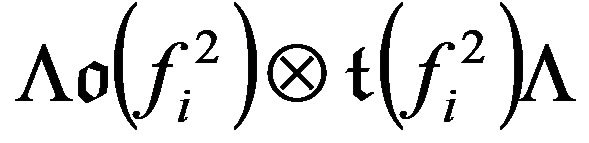 of
of  is
is
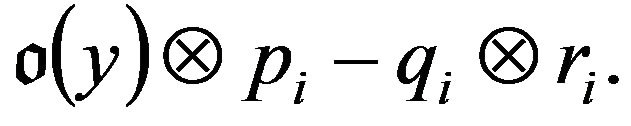
Applying 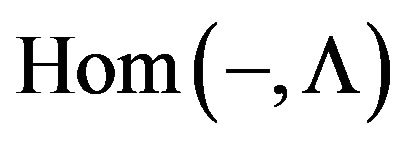 to this part of a minimal projective bimodule resolution of
to this part of a minimal projective bimodule resolution of  gives us the complex
gives us the complex
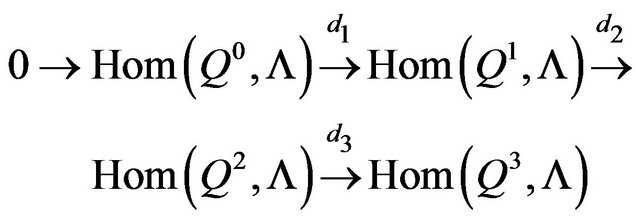
where 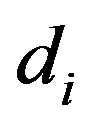 is the map induced from
is the map induced from 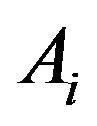 for
for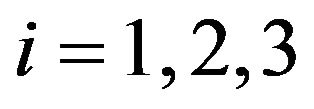 . Then
. Then 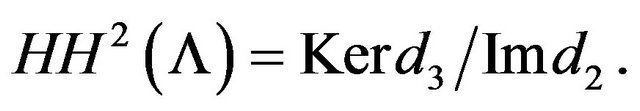
Throughout, all tensor products are tensor products over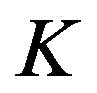 , and we write
, and we write  for
for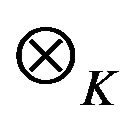 . When considering an element of the projective
. When considering an element of the projective 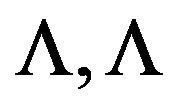 -bimodule
-bimodule
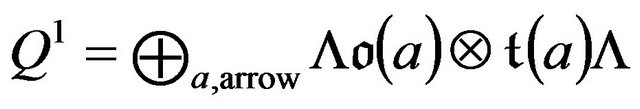 it is important to keep track of the individual summands of
it is important to keep track of the individual summands of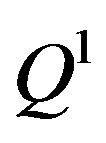 . So to avoid confusion we usually denote an element in the summand
. So to avoid confusion we usually denote an element in the summand 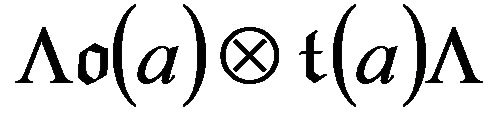 by
by 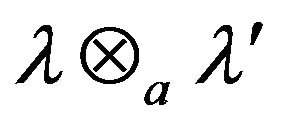 using the subscript “a” to remind us in which summand this element lies. Similarly, an element
using the subscript “a” to remind us in which summand this element lies. Similarly, an element 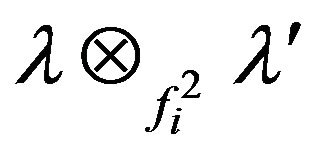 lies in the summand
lies in the summand
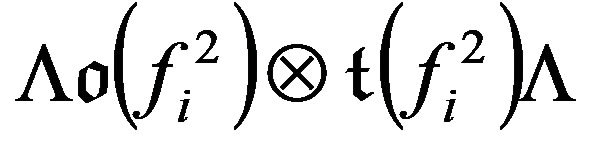 of
of 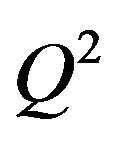 and an element
and an element 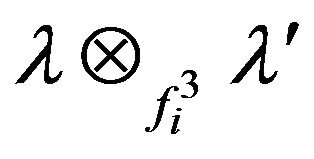 lies in the summand
lies in the summand 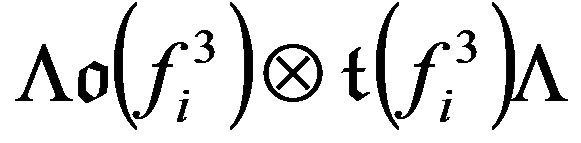 of
of . We keep this notation for the rest of the paper.
. We keep this notation for the rest of the paper.
4. 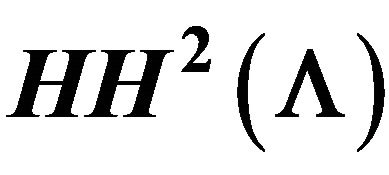 for
for ![]()
We have given 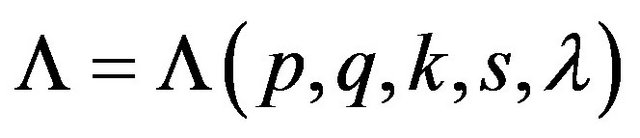 by quiver and relations in Section 2. However, these relations are not minimal. So next we will find a minimal set of relations
by quiver and relations in Section 2. However, these relations are not minimal. So next we will find a minimal set of relations 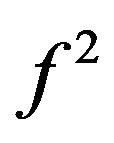 for this algebra.
for this algebra.
Let
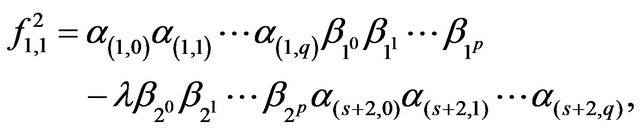
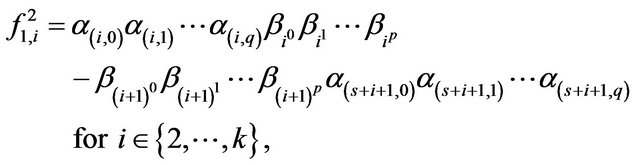
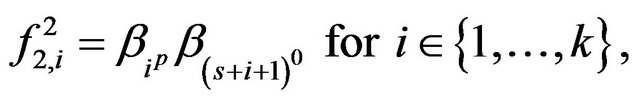
![]()
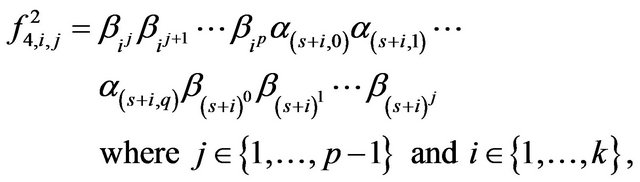
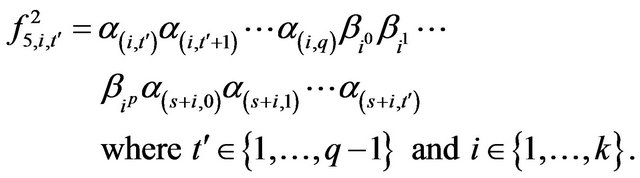
The remaining relations given in Section 2 are all linear combinations of the above relations. For example, the relation ![]() can be written as
can be written as
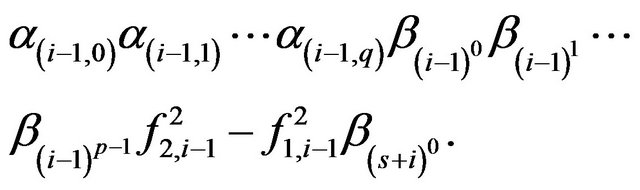
So this relation is in I and is not in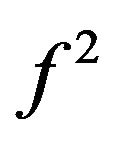 .
.
Proposition 4.1 For 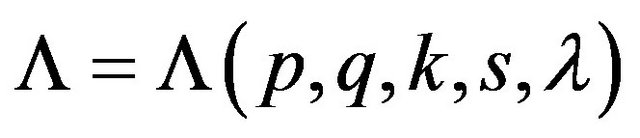 and with the above notation, the minimal set of relations is
and with the above notation, the minimal set of relations is
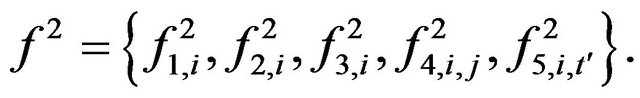
In contrast to the majority of self-injective algebras of finite representation type, we will show that the algebra 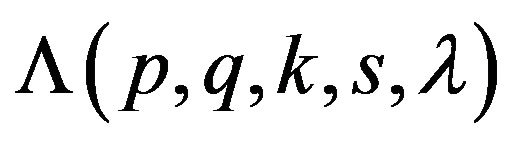 has non-zero second Hochschild cohomology group (see [3, Theorem 6.5]). Recall that
has non-zero second Hochschild cohomology group (see [3, Theorem 6.5]). Recall that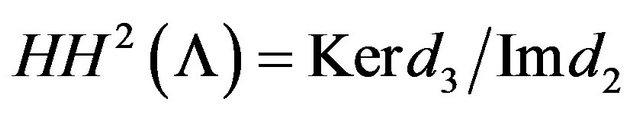 , where
, where
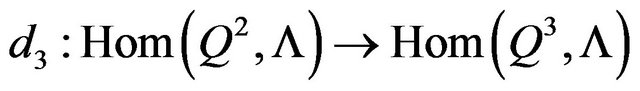
is induced by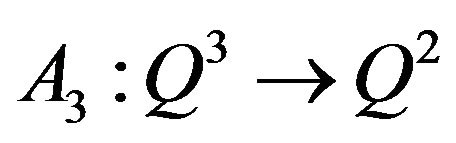 .
.
First we will find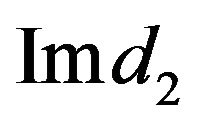 . Since
. Since
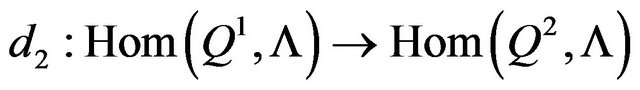 let
let 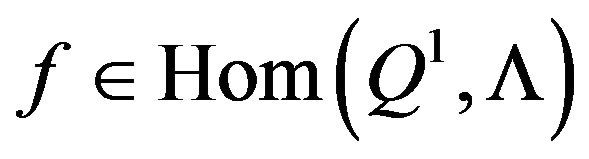 so that
so that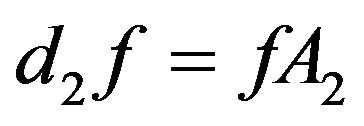 . We consider the cases
. We consider the cases 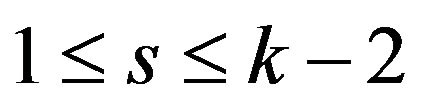 and
and 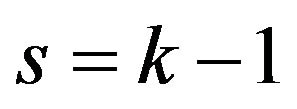 separately.
separately.
Let 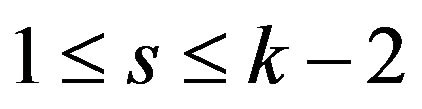 and
and
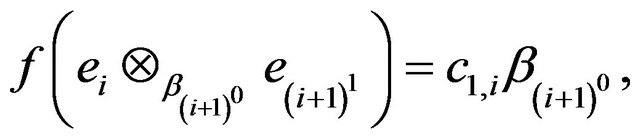
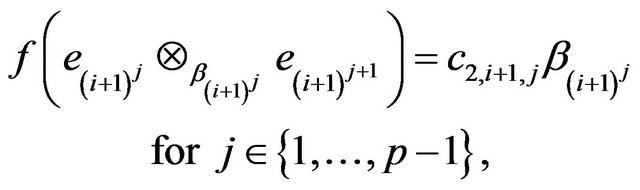
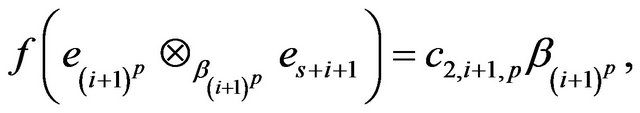
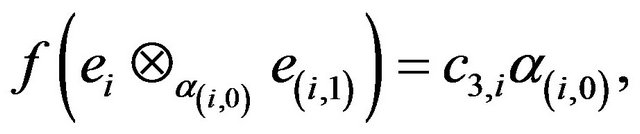

where all coefficients 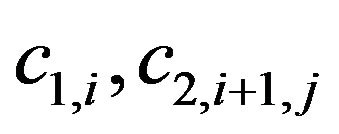 for
for 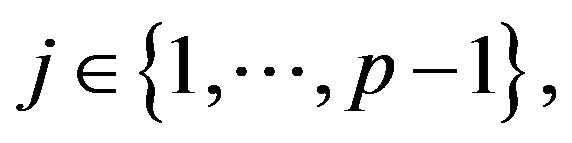
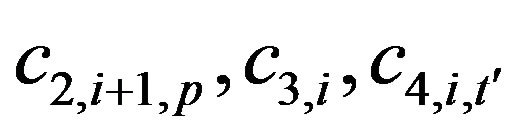 for
for 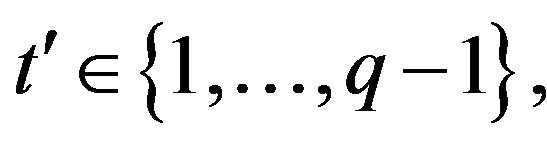
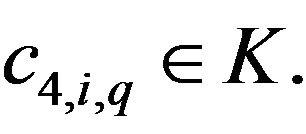 Now we find
Now we find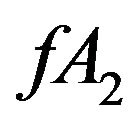 .
.
First we have,
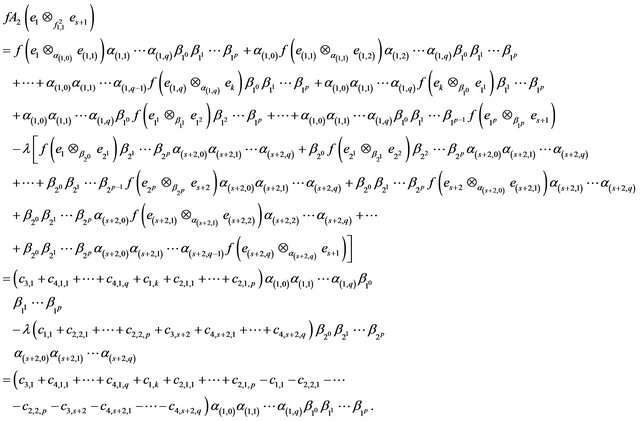
Similarly for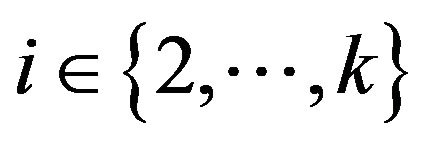 ,
,
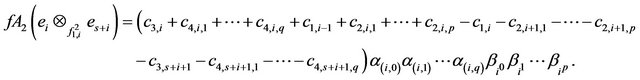
For the remaining terms, 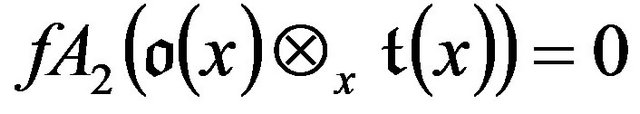 where
where 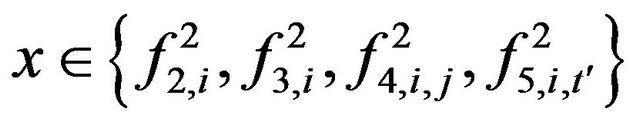 for all
for all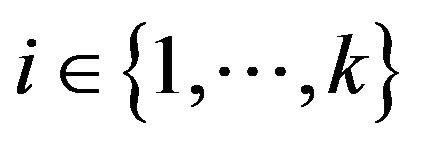 ,
,
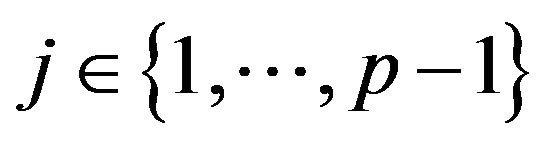 and
and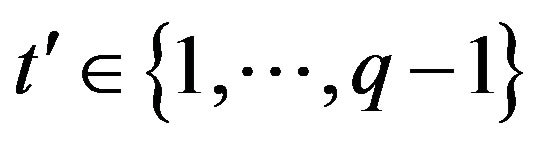 .
.
Let
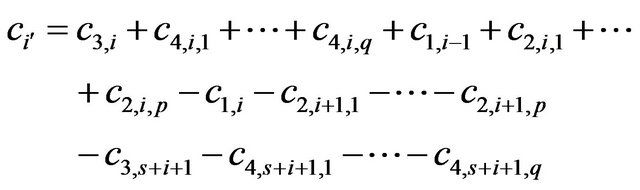
for 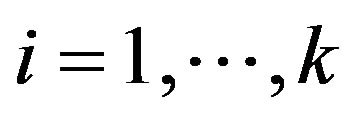 and
and
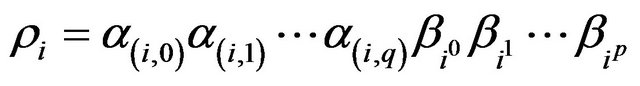
for 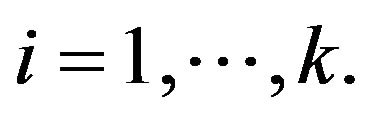
Thus for 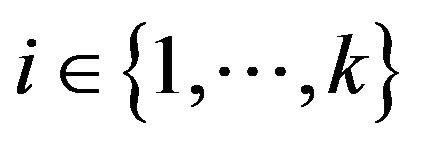 and
and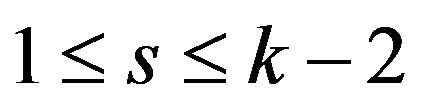 , fA2 is given by
, fA2 is given by
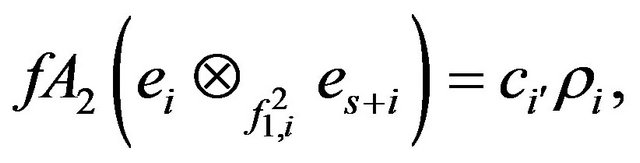
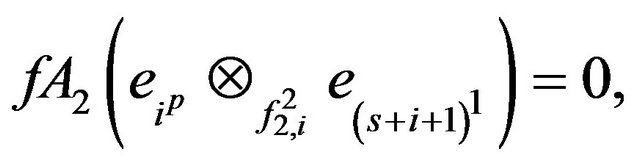
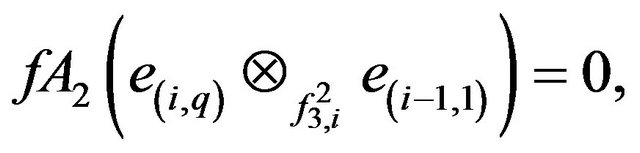
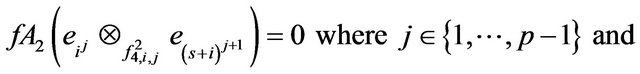
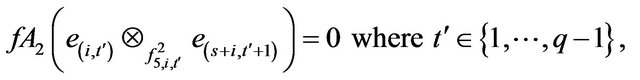
where 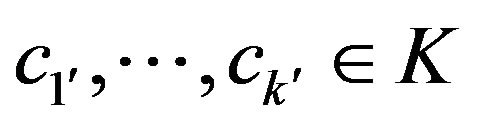 with
with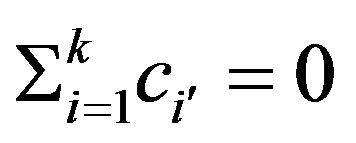 . So
. So
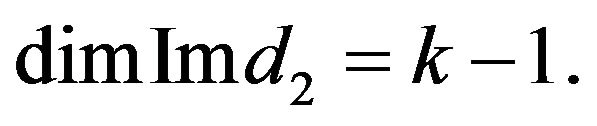
For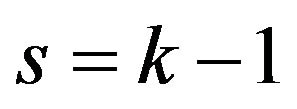 , we let
, we let

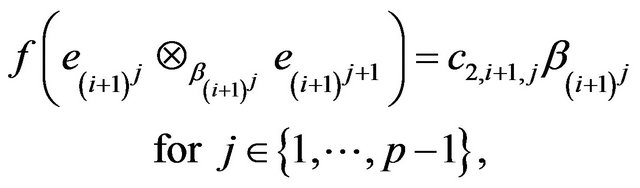
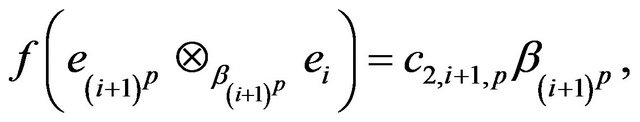
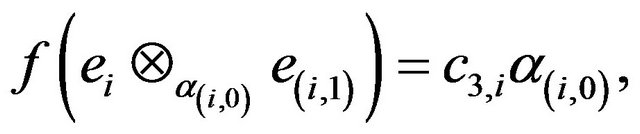
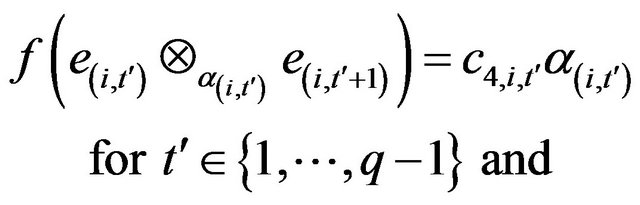

where for all 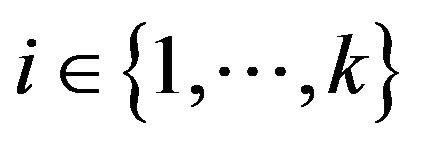 the coefficients
the coefficients 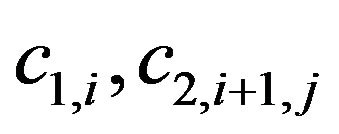 for
for 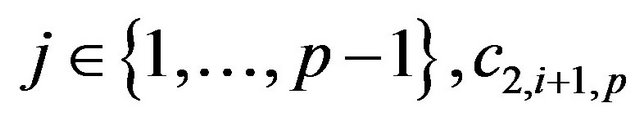
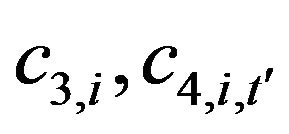 for
for 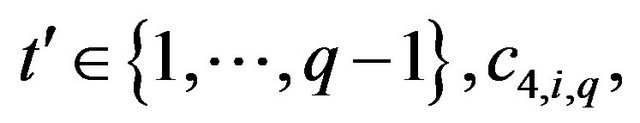
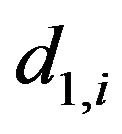 are in
are in 
Then we can find 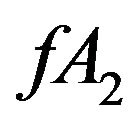 for
for 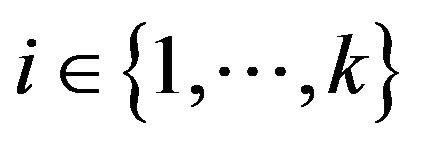 in the same way as the previous case to see that it is given by
in the same way as the previous case to see that it is given by
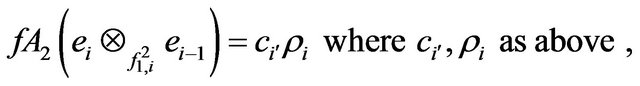
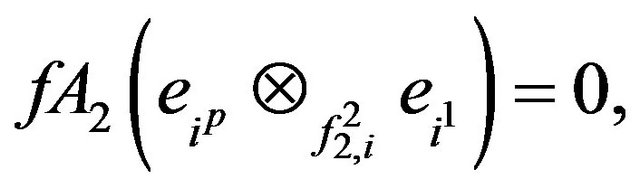
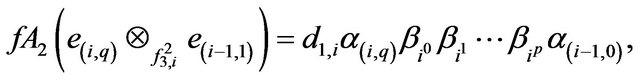
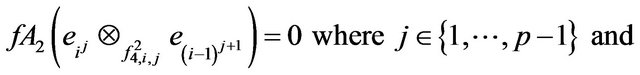
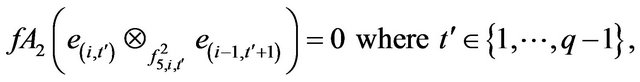
where 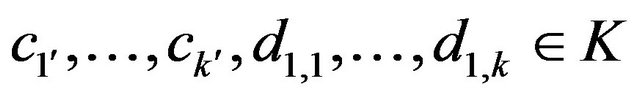 with
with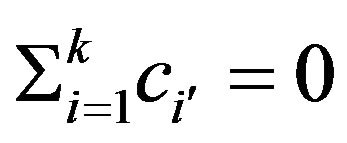 . Note that there is no dependency between the
. Note that there is no dependency between the 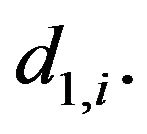 So
So 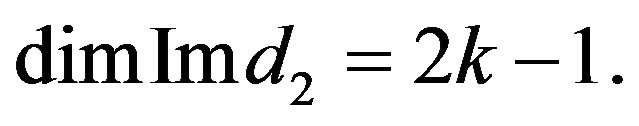
Proposition 4.2 If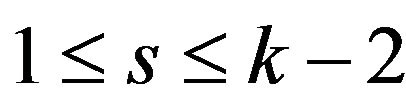 , we have
, we have 
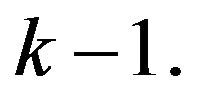 If
If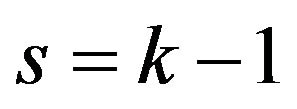 , we have
, we have 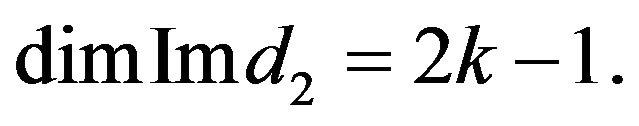
Next we find 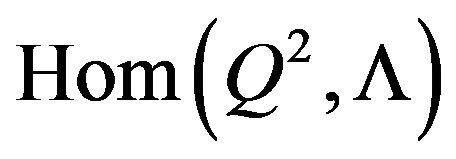 and again consider the two cases separately. Let
and again consider the two cases separately. Let 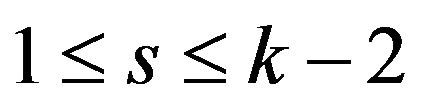 and
and 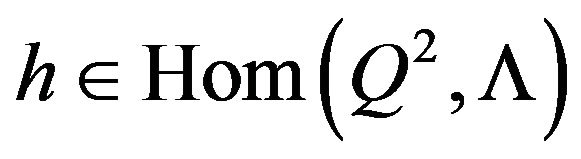 . Then
. Then  is defined by
is defined by
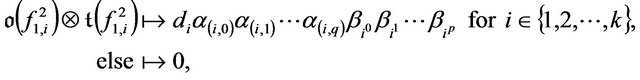
where .
.
Therefore 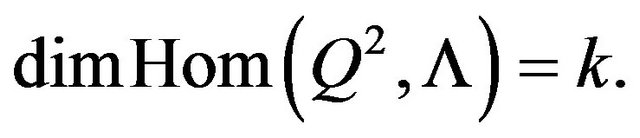 Hence,
Hence, 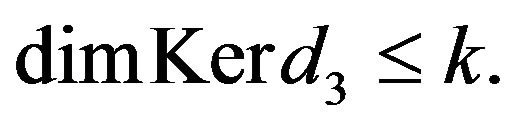
For 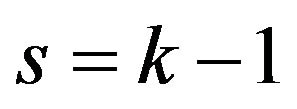 and
and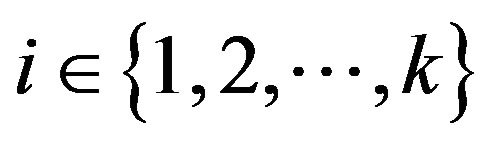 ,
, 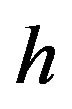 is given by
is given by
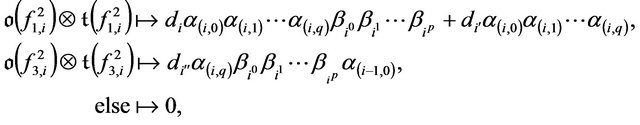
where 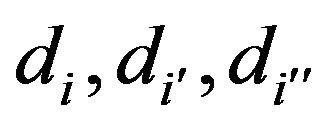 are in K for
are in K for 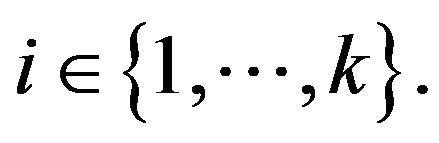 Thus
Thus 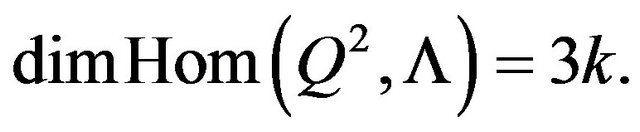
Proposition 4.3 If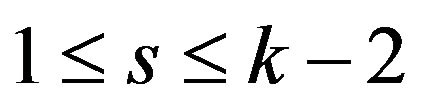 , we have
, we have
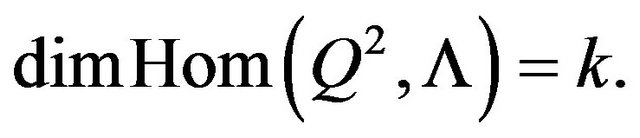 If
If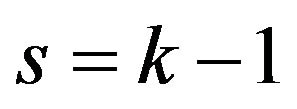 ,
,
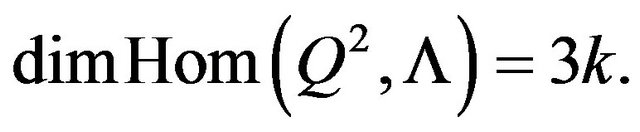
Corollary 4.4 If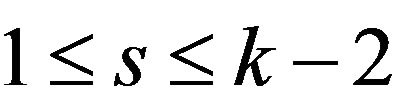 , we have
, we have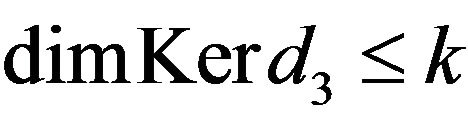 . If
. If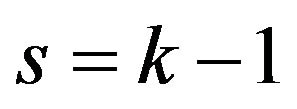 ,
, 
In order to find Kerd3 and hence determine 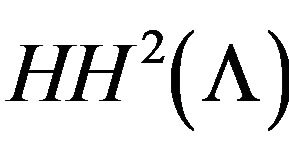 we start by giving a non-zero element in
we start by giving a non-zero element in 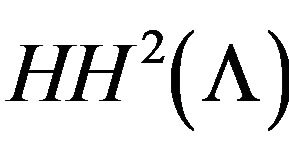 for all s.
for all s.
Proposition 4.5 Define 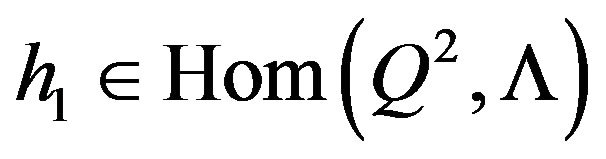 by
by
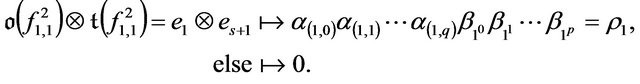
Then ![]() is in
is in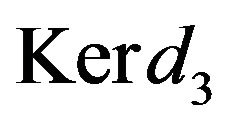 .
.
Proof. We note that 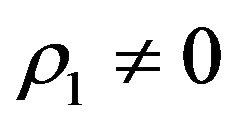 so
so ![]() is a non-zero map. To show that
is a non-zero map. To show that 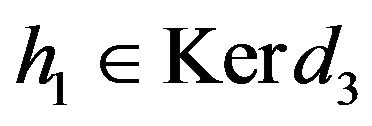 we show that
we show that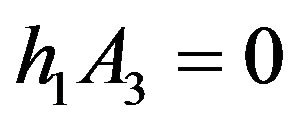 . First, observe that
. First, observe that ![]() and
and 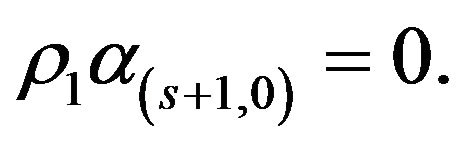 Hence
Hence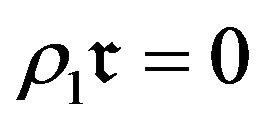 . Similarly we have
. Similarly we have 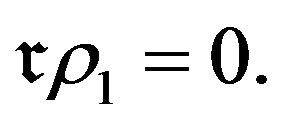
Recall that ![]() where
where
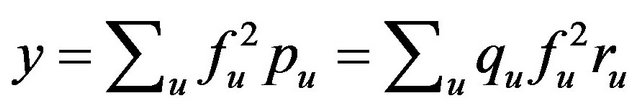 and
and  are in the ideal generated by the arrows. For
are in the ideal generated by the arrows. For 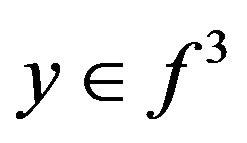 the component of
the component of
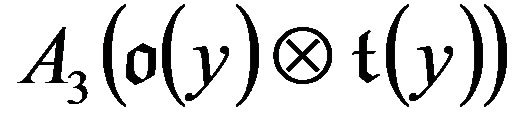 in
in 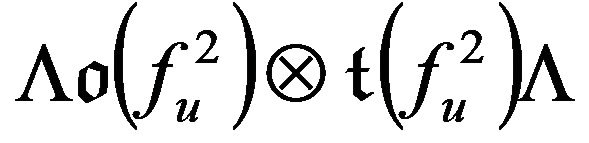 is
is
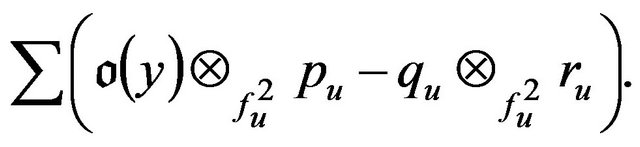
Then
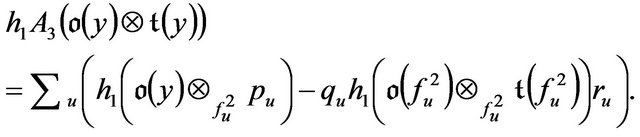
Thus

As  is in the arrow ideal of
is in the arrow ideal of ,
, 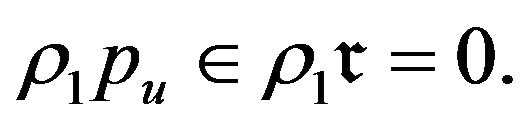 So we have
So we have 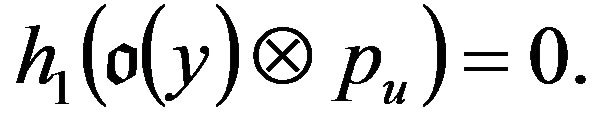 Similarly
Similarly
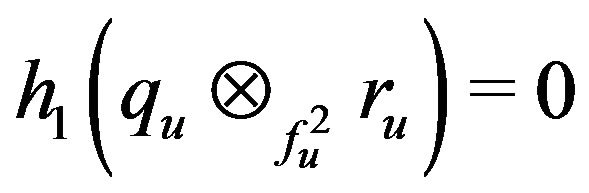 as
as 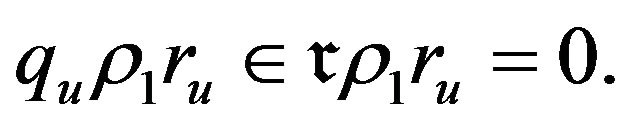 Therefore
Therefore
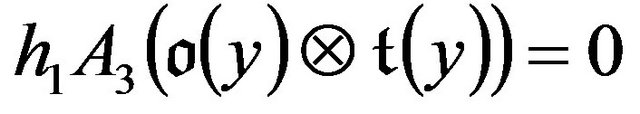 for all
for all 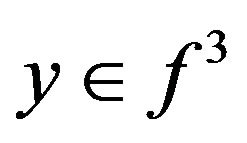 so
so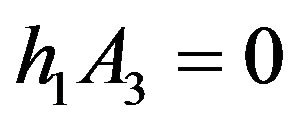 . Thus
. Thus 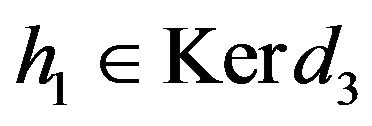 as required.
as required.
Theorem 4.6 For 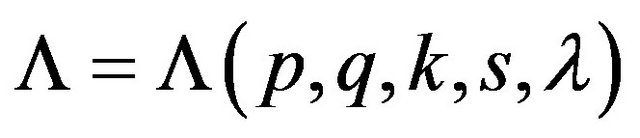 where
where 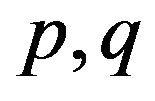 are positive integers,
are positive integers, 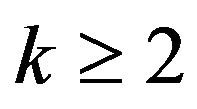 ,
, 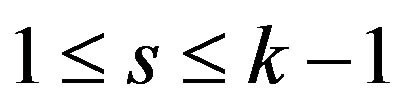 with
with
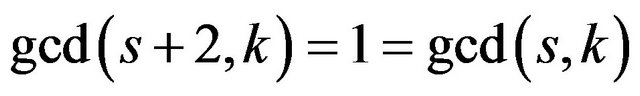 and
and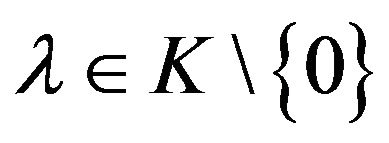 , we have
, we have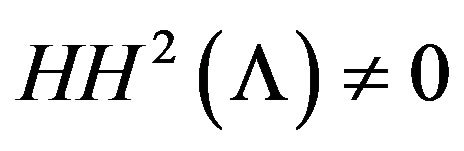 .
.
Proof. Consider the element 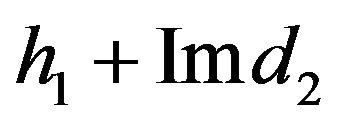 of
of 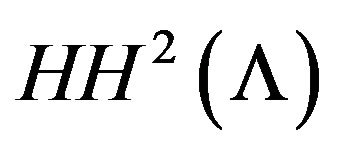
where ![]() is given as in Proposition 4.5 by
is given as in Proposition 4.5 by
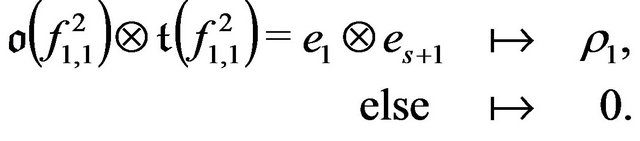
Suppose for contradiction that 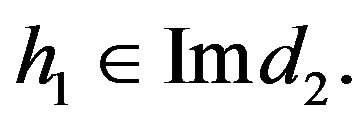 Then
Then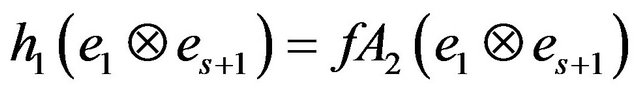 . So
. So 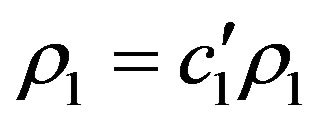 and so
and so
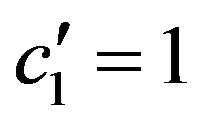 . Also
. Also 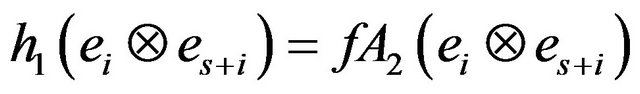 where
where 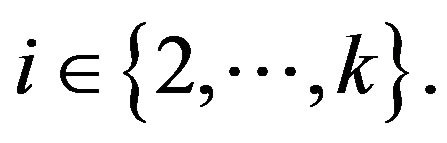 Then
Then 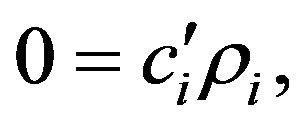 where
where 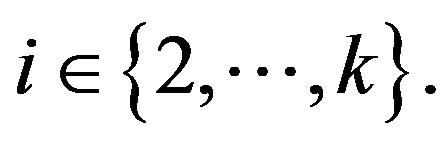 But this contradicts having
But this contradicts having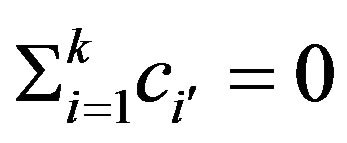 . Therefore
. Therefore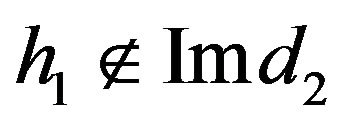 , that is,
, that is,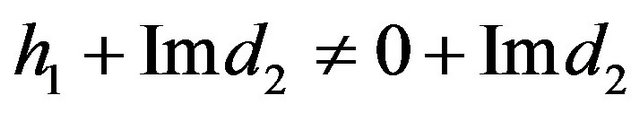 . So
. So 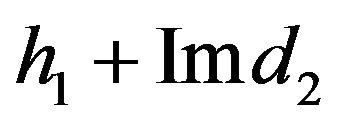 is a nonzero element in
is a nonzero element in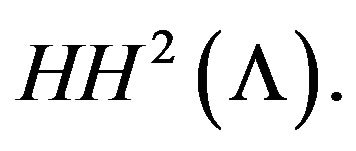 □
□
Note that we can also define maps 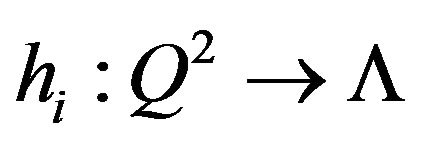 by
by
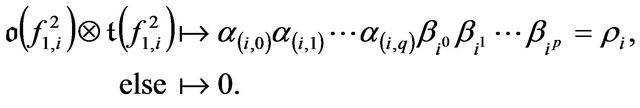
for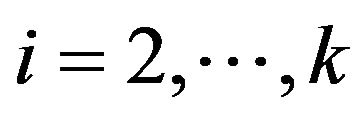 . However,
. However, 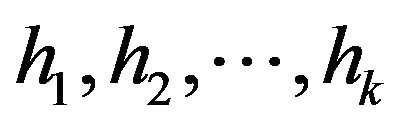 all represent the same element
all represent the same element 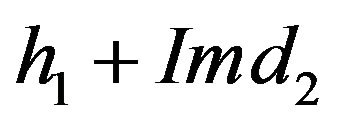 of
of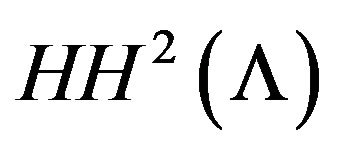 .
.
As we have found a non-zero element in 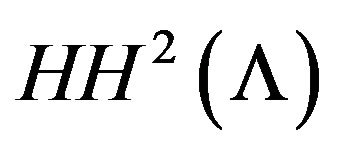 we know that
we know that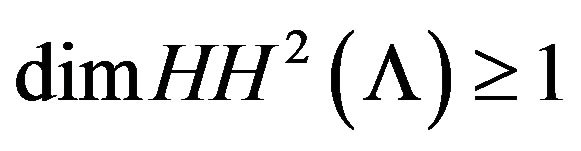 . In the case
. In the case
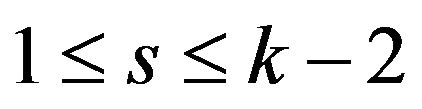 we have the following result, the proof of which is immediate from Proposition 4.2, Corollary 4.4 and Theorem 4.6.
we have the following result, the proof of which is immediate from Proposition 4.2, Corollary 4.4 and Theorem 4.6.
Proposition 4.7 For 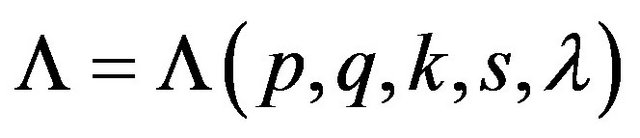 where
where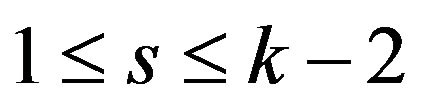 , we have
, we have 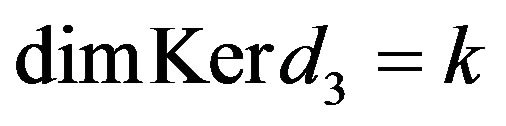 and
and

For the case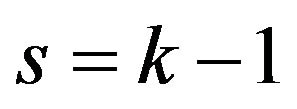 , we need more details to find
, we need more details to find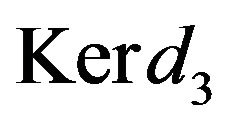 . Following [5] we may choose the set
. Following [5] we may choose the set 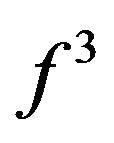 to consist of the following elements:
to consist of the following elements:
 where
where
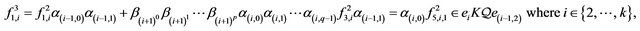
![]()
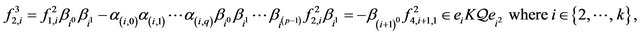
![]()
![]()
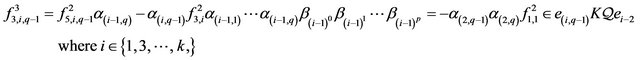
![]()
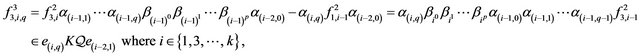
![]()
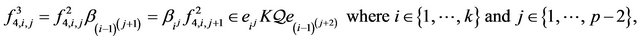
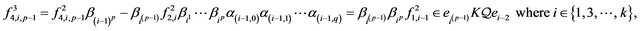
![]()
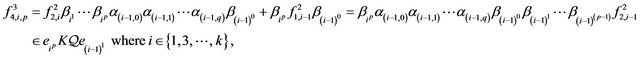
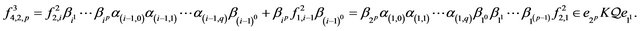
Thus the projective bimodule  is
is 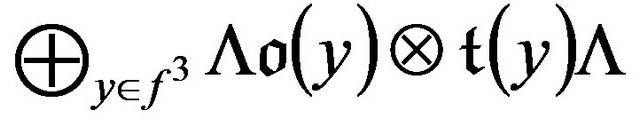
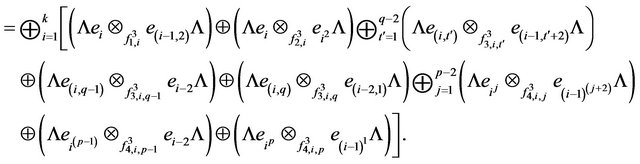
Now we determine  in the case
in the case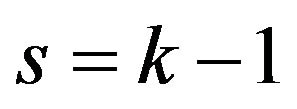 . Let
. Let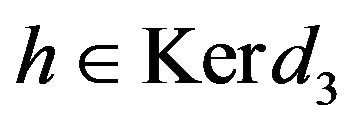 , so
, so 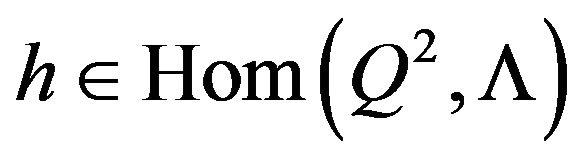 and
and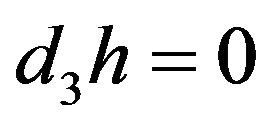 . Recall that for
. Recall that for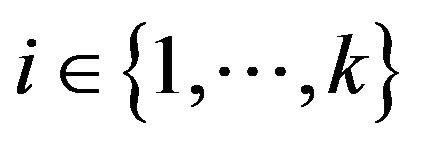 ,
, 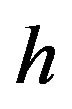 is given by
is given by
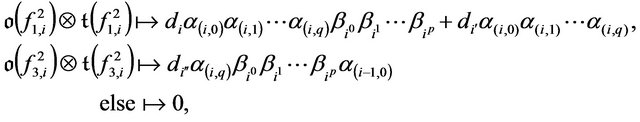
where 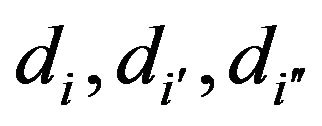 are in
are in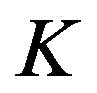 .
.
Then for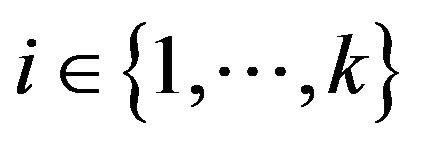 , we have
, we have 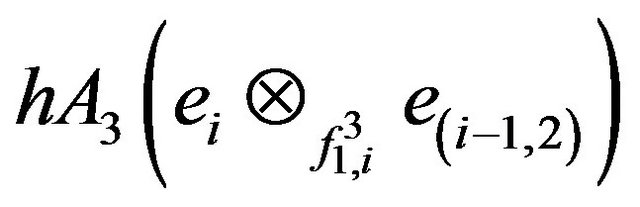
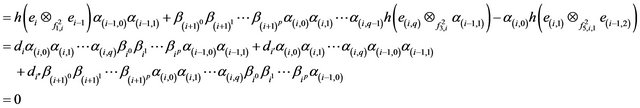
In a similar way we can show that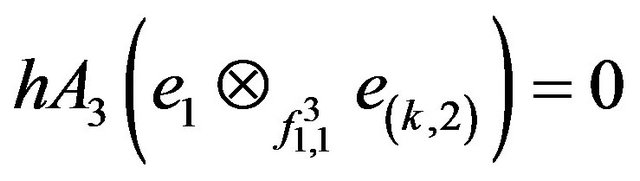 .
.
For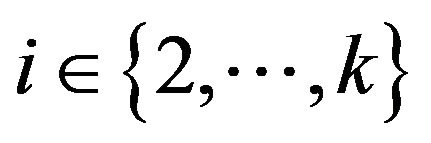 , we have
, we have 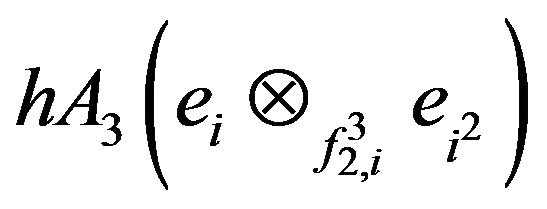

As 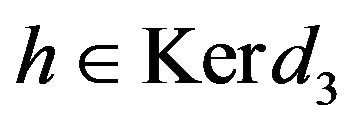 we have
we have 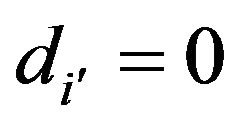 for
for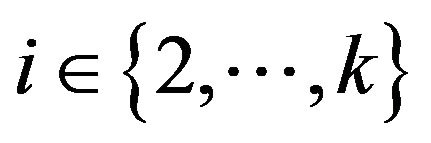 .
.
Similarly it can be shown that
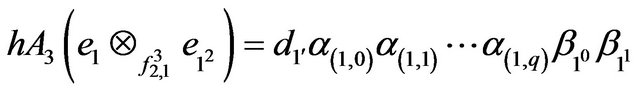
so that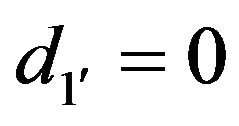 .
.
We also have 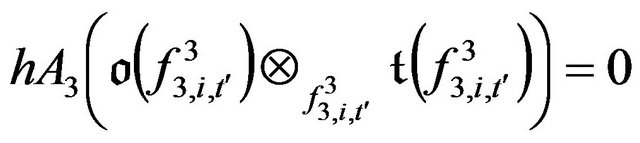 for
for
 and
and  Finally, putting
Finally, putting
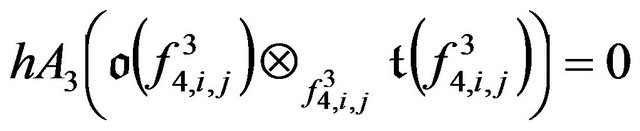
does not give any new information for ,
,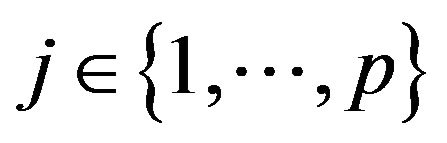 .
.
Thus h is given by
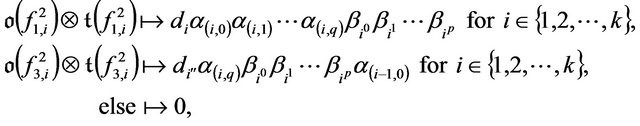
where 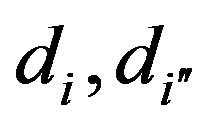 for
for 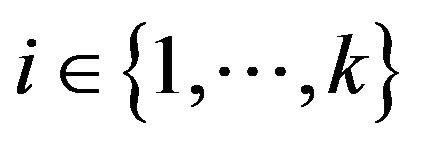 are in K. It is clear that there is no dependency between
are in K. It is clear that there is no dependency between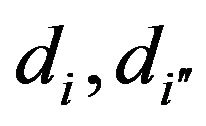 , and therefore
, and therefore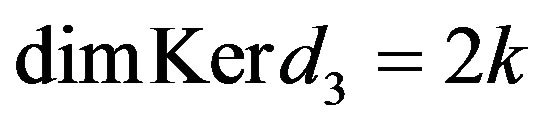 .
.
Proposition 4.8 For  and
and , we have
, we have 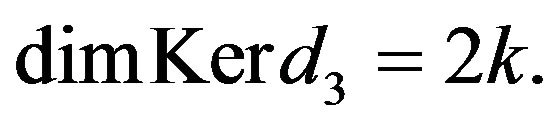
Using Propositions 4.2, 4.7, 4.8 and Theorem 4.6 we get the main result of this section.
Theorem 4.9 For 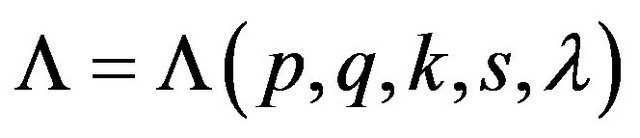 where p, q, s, k are integers such that p,
where p, q, s, k are integers such that p, 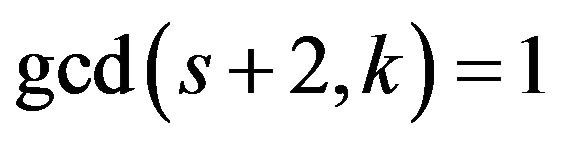
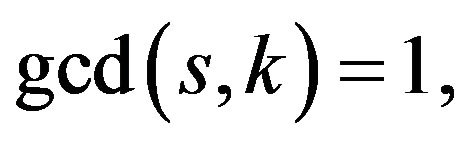
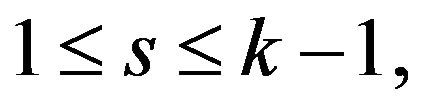
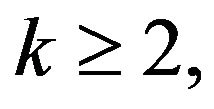
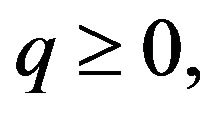 and
and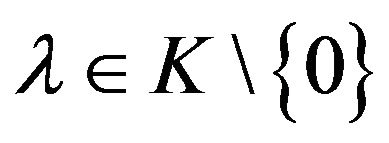 , we have
, we have 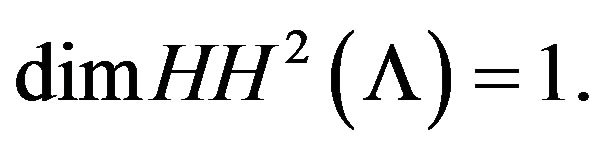
We conclude this section by giving a deformation of  which arises from the non-zero element
which arises from the non-zero element 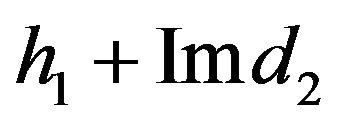 in
in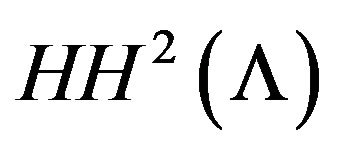 .
.
Let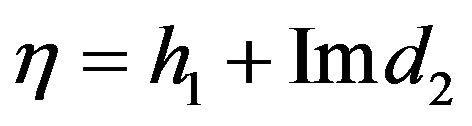 . Recall that
. Recall that
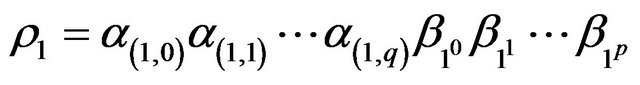 . We introduce a new parameter
. We introduce a new parameter  and define the algebra
and define the algebra 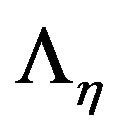 to be the algebra
to be the algebra 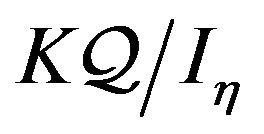 where
where 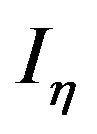 is the ideal generated by the following elements:
is the ideal generated by the following elements:
1) 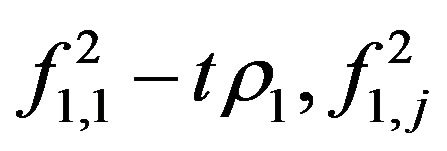 where
where 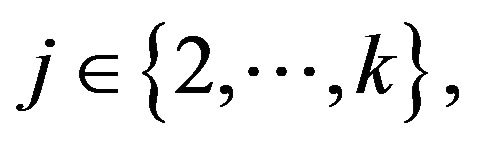
2) for all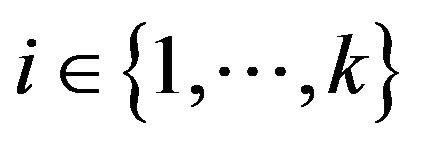 ,
, 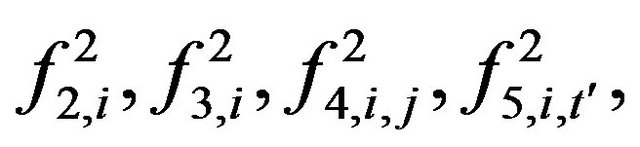 where
where 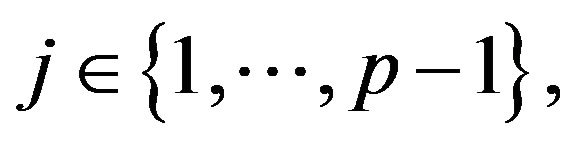
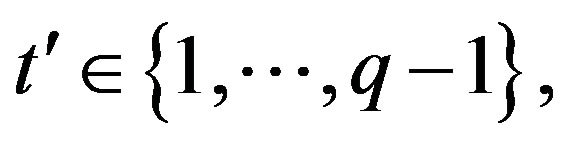
3) 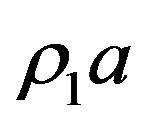 for all arrows a with
for all arrows a with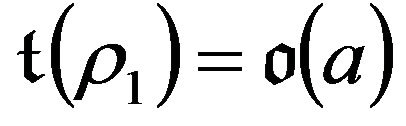 4)
4) 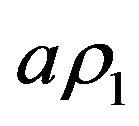 for all arrows a with
for all arrows a with 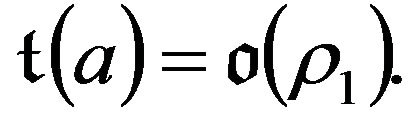
We now need to show that 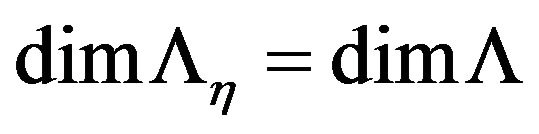 to verify that
to verify that 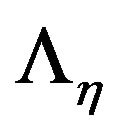 is indeed a deformation of
is indeed a deformation of . First of all, it is clear that
. First of all, it is clear that 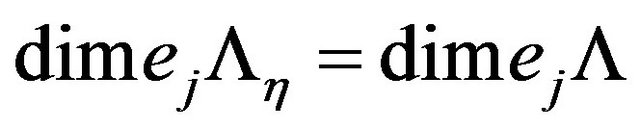 for all t and for all vertices ei with
for all t and for all vertices ei with . Now we consider
. Now we consider 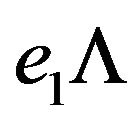 and
and 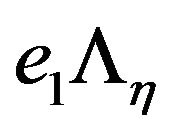 with
with![]() , and
, and 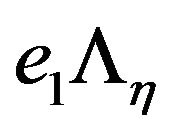 with
with![]() . These projective modules are described as follows:
. These projective modules are described as follows:
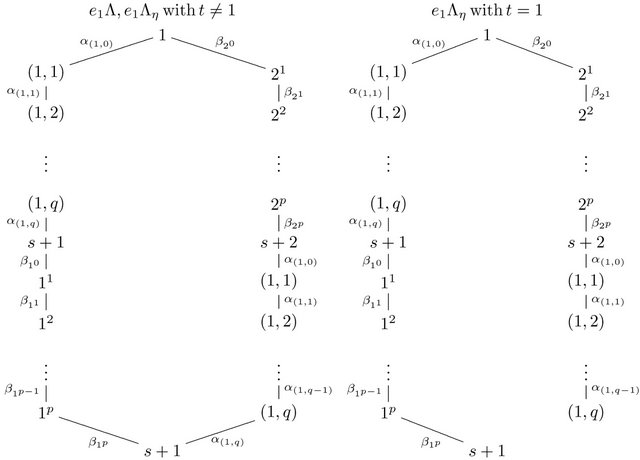
In each case we see that
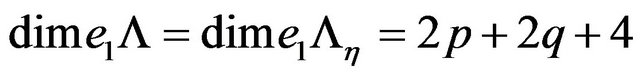
for all t. Hence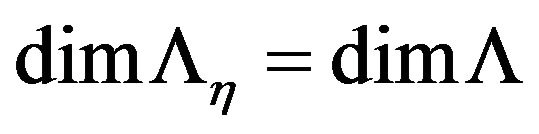 . Moreover, when
. Moreover, when ![]() the algebras
the algebras  and
and 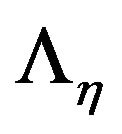 are not isomorphic since, in this case,
are not isomorphic since, in this case, 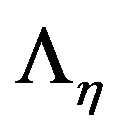 is not self-injective. Thus we have found a non-trivial deformation of
is not self-injective. Thus we have found a non-trivial deformation of .
.
Theorem 4.10 With 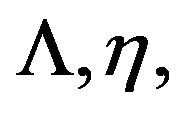 and
and 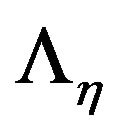 as defined above, then
as defined above, then 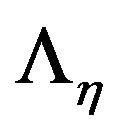 is a non-trivial deformation of
is a non-trivial deformation of . Moreover, the algebras
. Moreover, the algebras  and
and 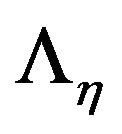 are socle equivalent.
are socle equivalent.
5. 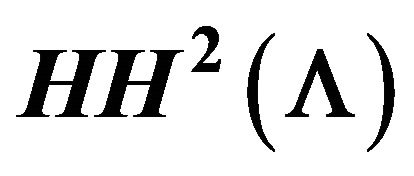 for
for 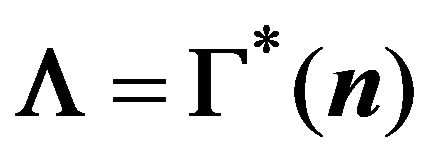
We have given the algebra 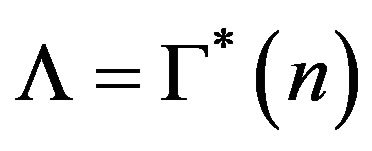 by quiver and relations in Section 2. Note that these relations are not minimal. So we will find a minimal set of relations
by quiver and relations in Section 2. Note that these relations are not minimal. So we will find a minimal set of relations 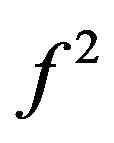 for this algebra.
for this algebra.
Let
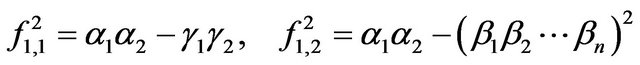
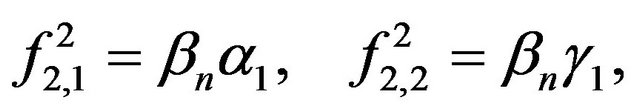
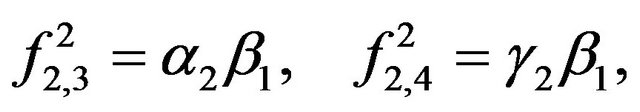
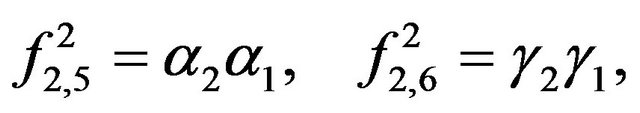
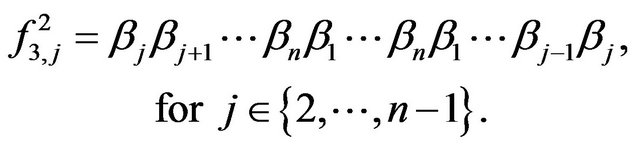
The remaining relation 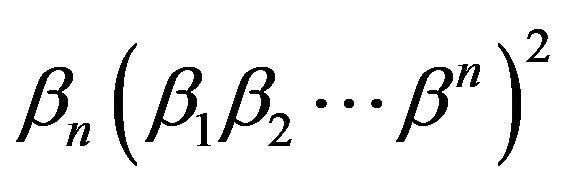 can be written as
can be written as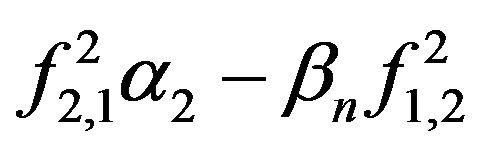 . So this relation is in I and is not in
. So this relation is in I and is not in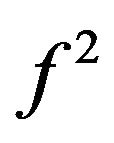 .
.
Proposition 5.1 For 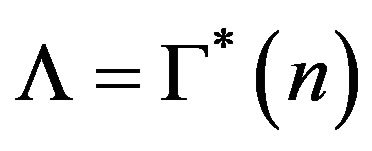 and with the above notation, the minimal set of relations is
and with the above notation, the minimal set of relations is
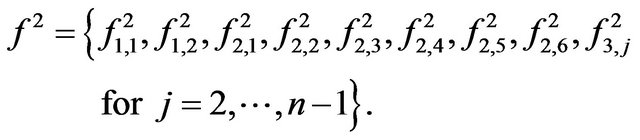
Recall that the projective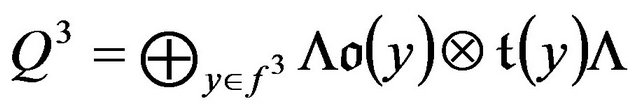 . Thus we have
. Thus we have
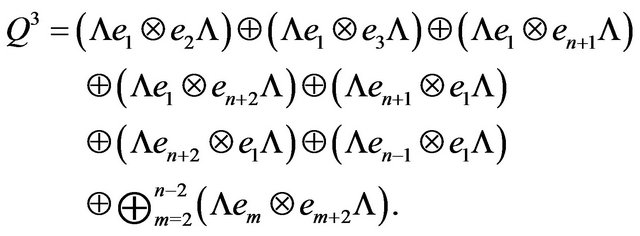
(We note that the projective  is also described in [4] although Happel gives no description of the maps in the
is also described in [4] although Happel gives no description of the maps in the  -projective resolution of
-projective resolution of .) Following [2], and with the notation introduced in Section 3, we may choose the set
.) Following [2], and with the notation introduced in Section 3, we may choose the set 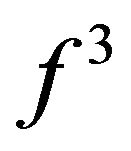 to consist of the following elements:
to consist of the following elements:
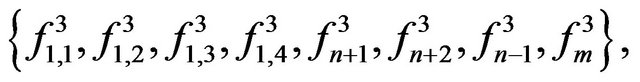
with 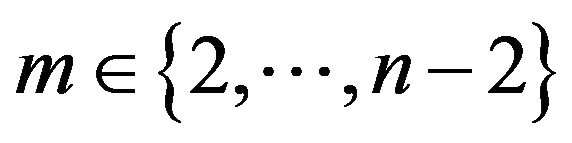 where
where
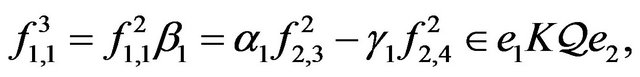
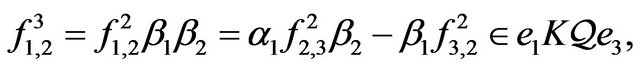

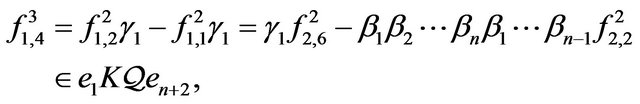
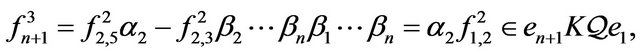
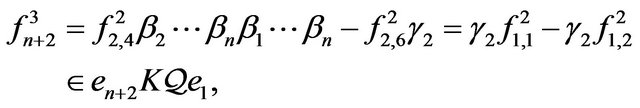
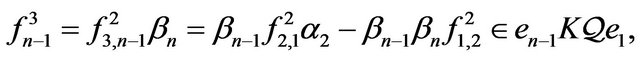
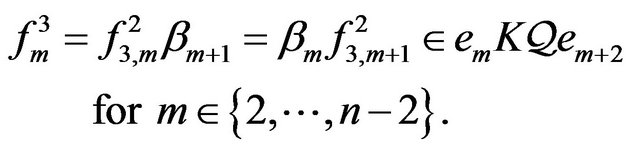
We know that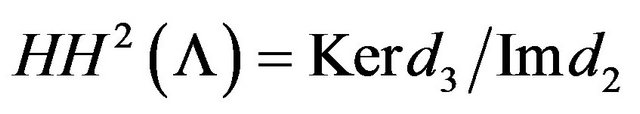 . First we will find
. First we will find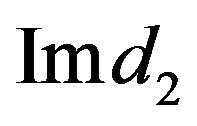 . Let
. Let 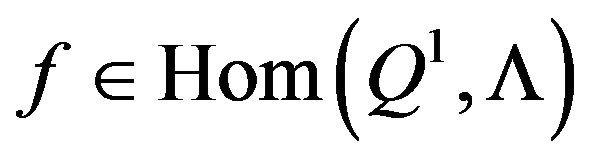 and so write
and so write
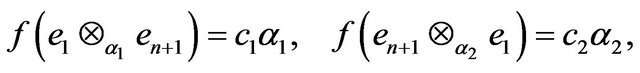
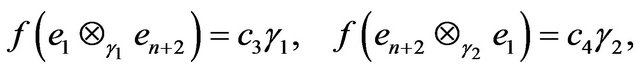
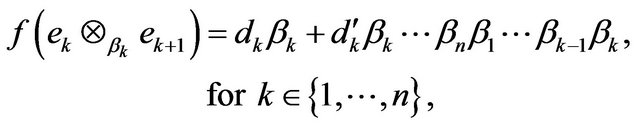
where 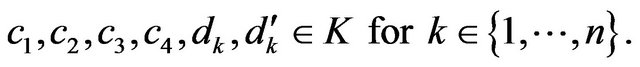
Now we find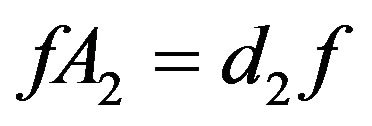 . We have
. We have
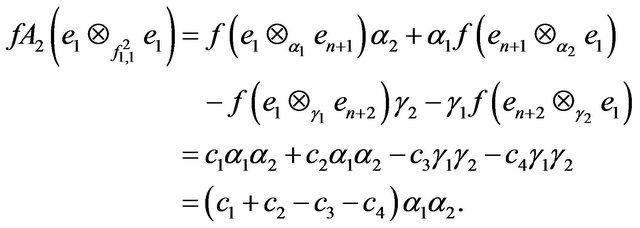
Also
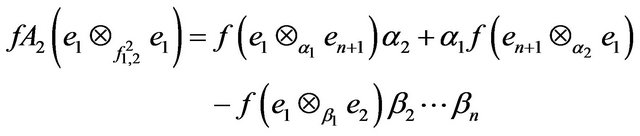
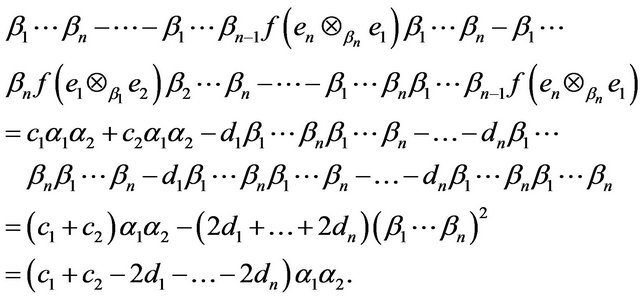
We can show by direct calculation that
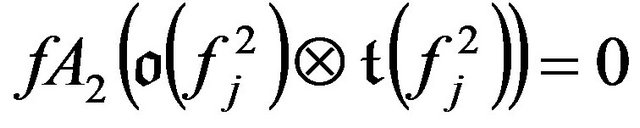 for all
for all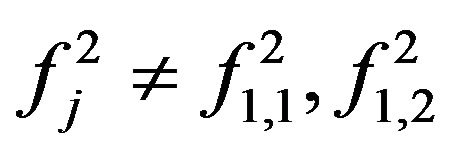 .
.
Thus 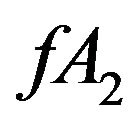 is given by
is given by
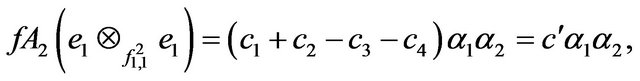
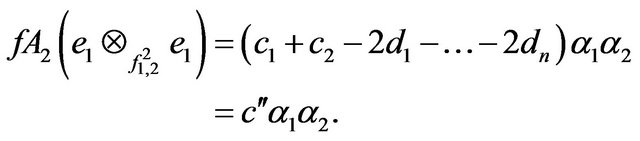
So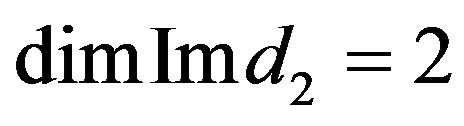 .
.
Proposition 5.2 For , we have
, we have 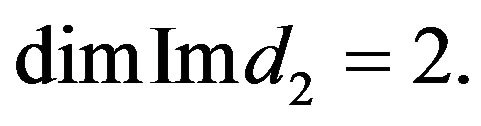
Now we determine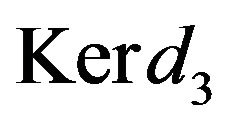 . Let
. Let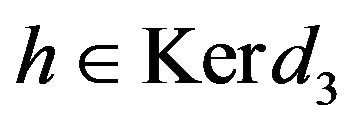 , so
, so 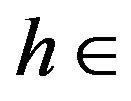
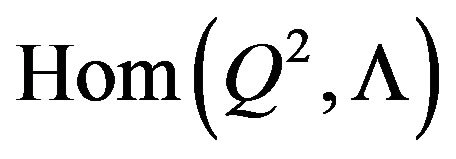 and
and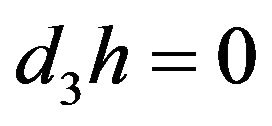 . Then
. Then 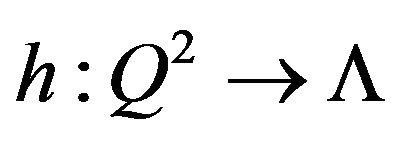 is given by
is given by
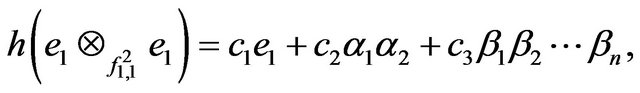
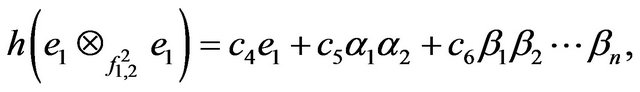
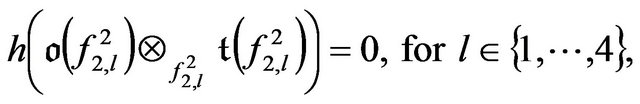
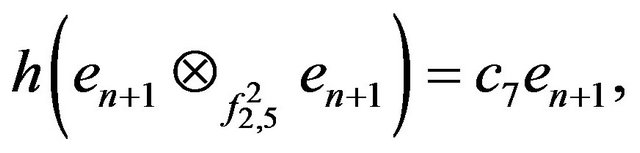
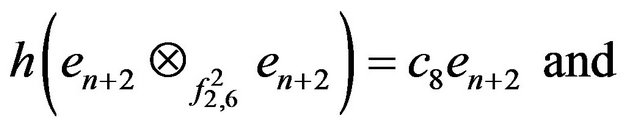
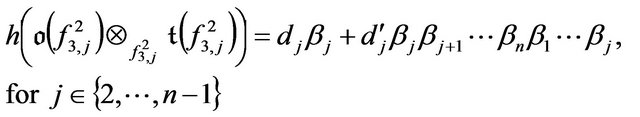
for some 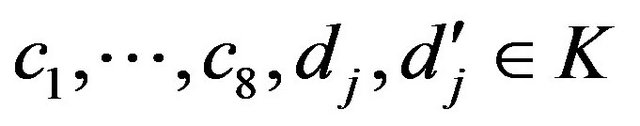 for
for 
Then
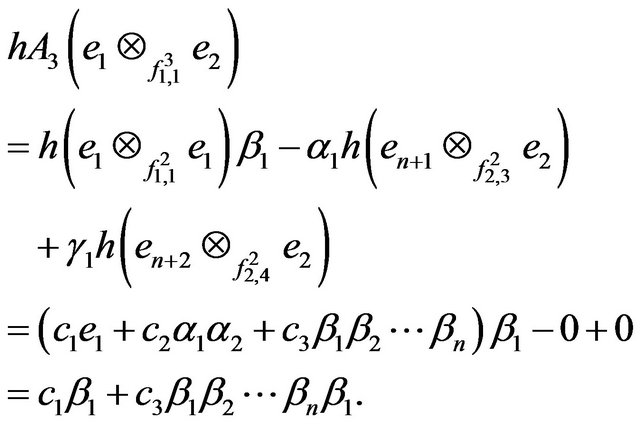
As 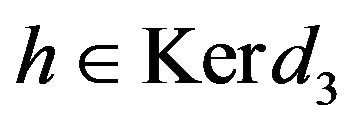 we have
we have 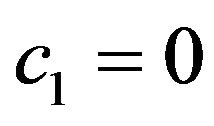 and
and 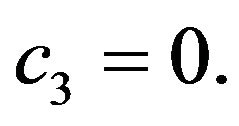
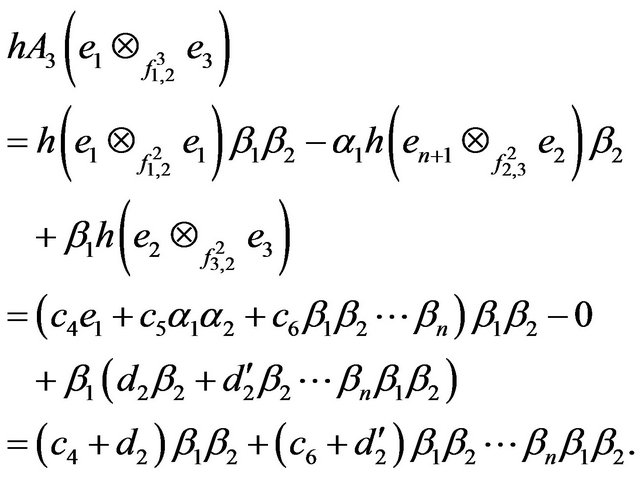
As 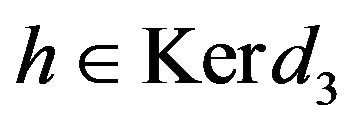 we have
we have 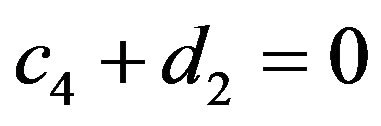 and
and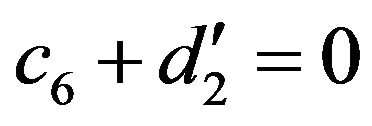 . So
. So 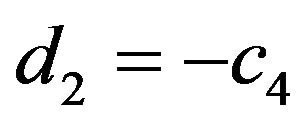 and
and 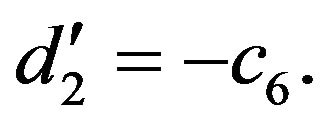
Next,
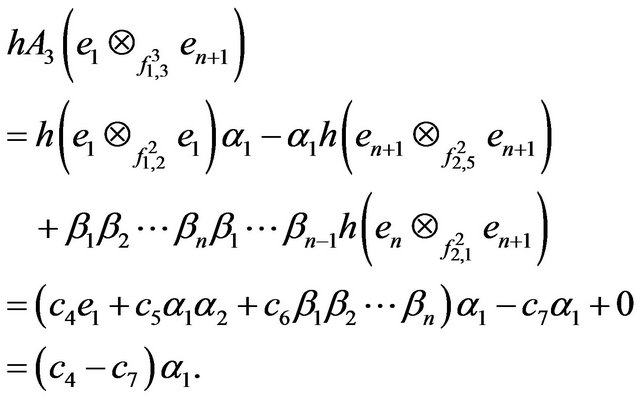
So we have 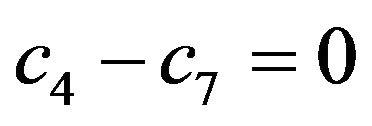 and hence
and hence 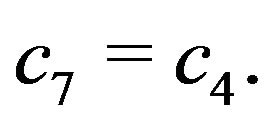
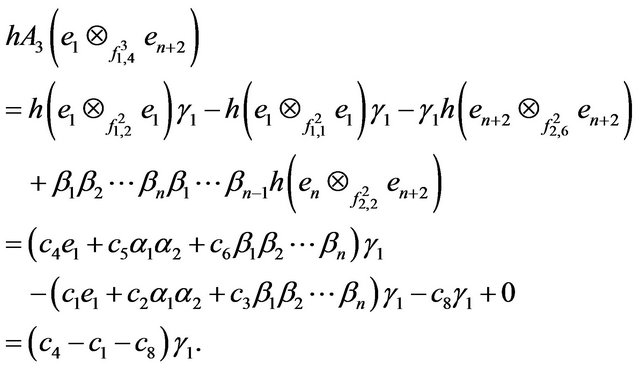
Therefore 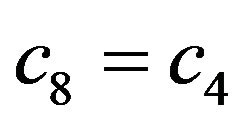 as
as 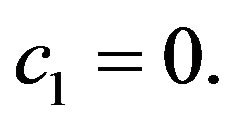
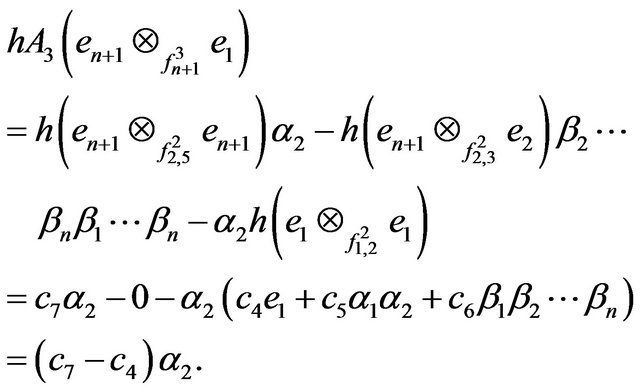
Thus again we have 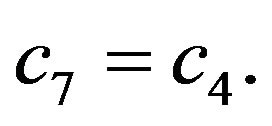
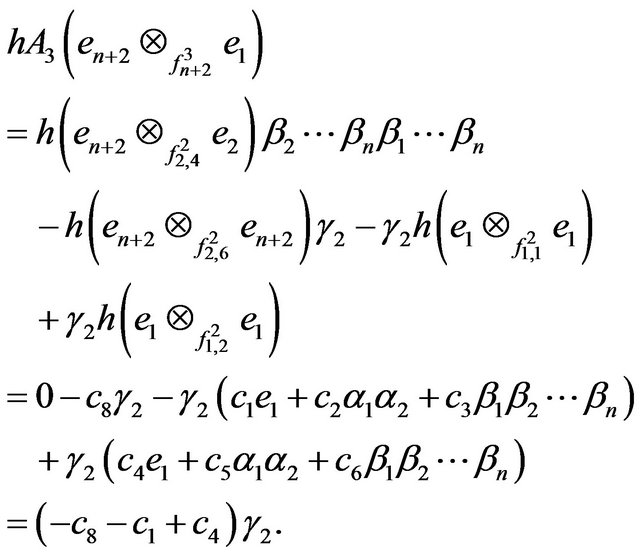
As 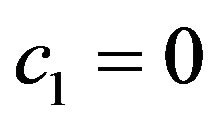 above, we have
above, we have 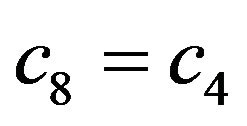 as we already know.
as we already know.
Also
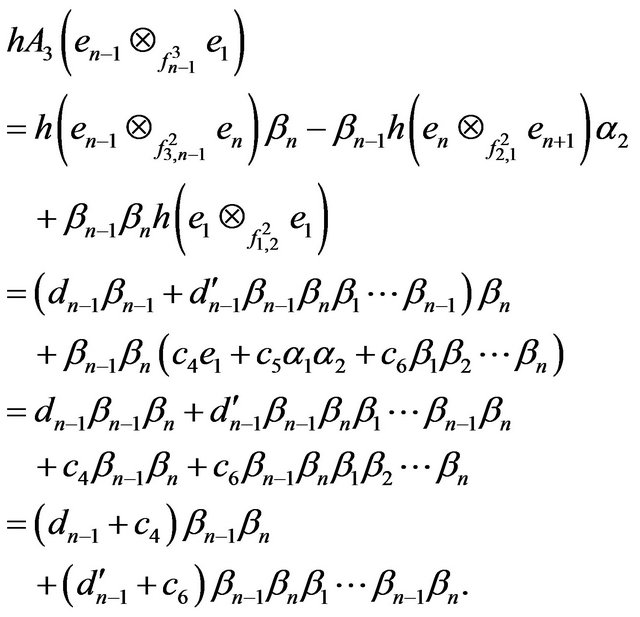
So we have 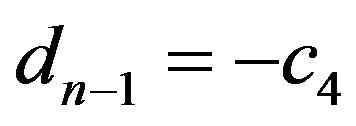 and
and 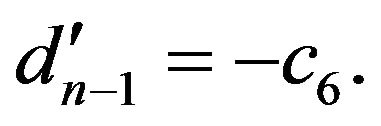
Finally, for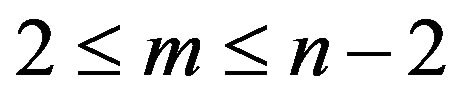 , we have
, we have
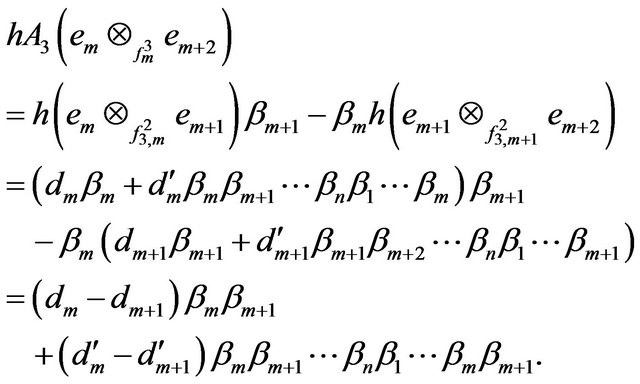
Therefore we have 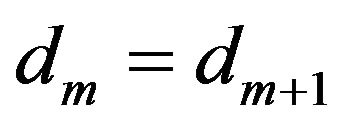 and
and . Hence
. Hence  and
and 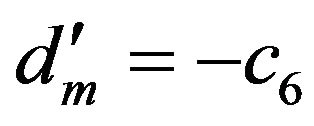 for
for 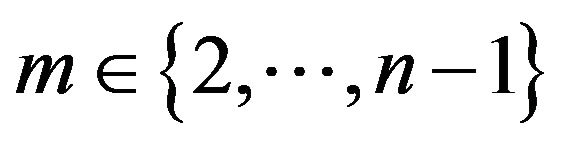 as we have above
as we have above 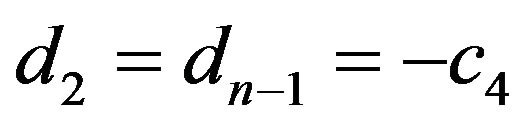 and
and 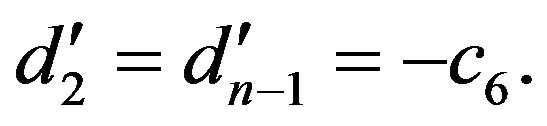
Thus  is given by
is given by
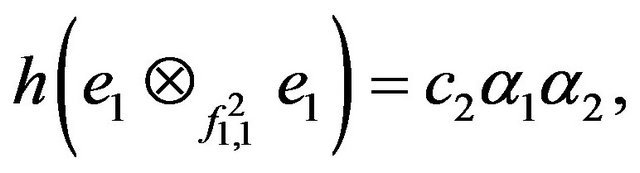
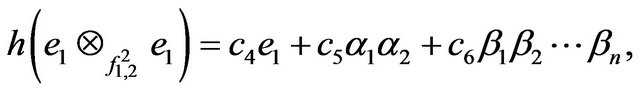

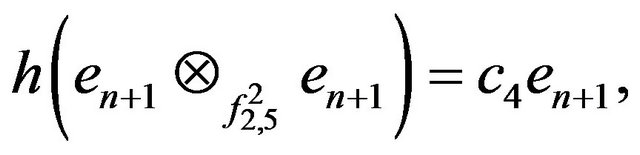

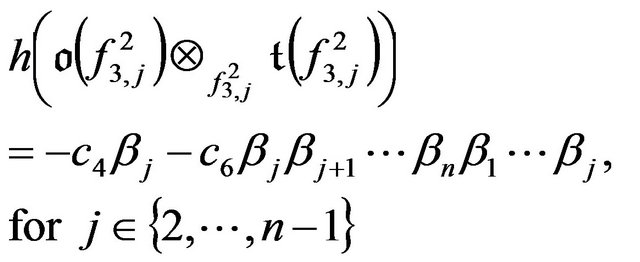
for some 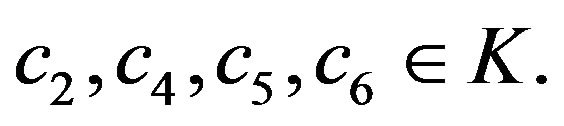
Proposition 5.3 For , we have
, we have 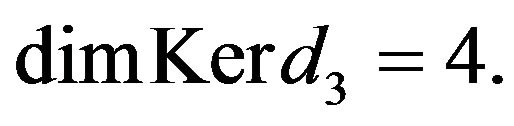
Therefore
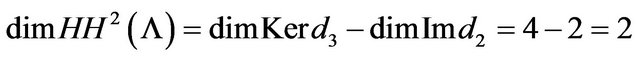
and a basis is given by the maps 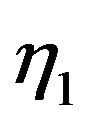 and
and 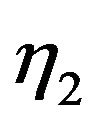 where
where 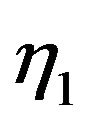 is given by
is given by
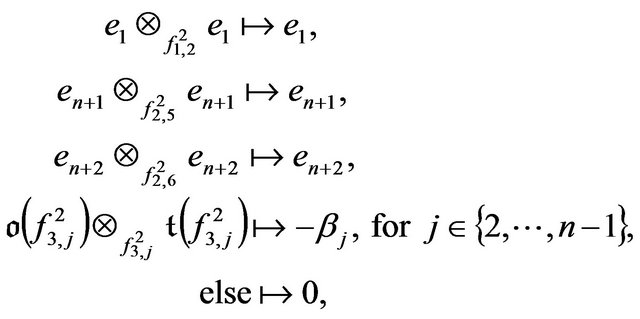
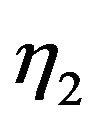 is given by
is given by
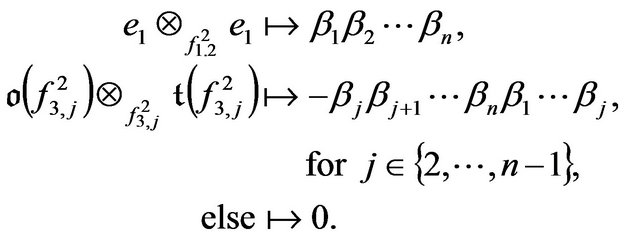
From Proposition 5.2 and Proposition 5.3 we get the main result of this section.
Theorem 5.4 For 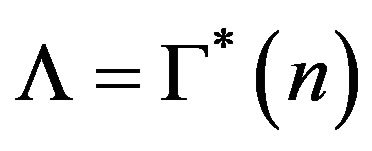 with
with 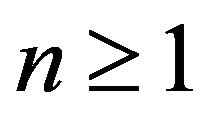 we have
we have 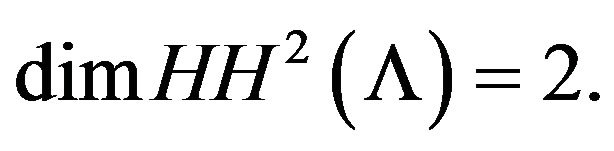
To connect this with deformations we use a similar discussion as Section 4. We introduce the parameter  and define the algebra
and define the algebra 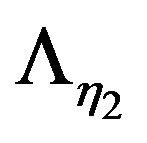 to be the algebra
to be the algebra 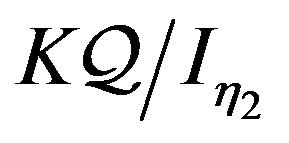 where
where  is the ideal generated by the following elements:
is the ideal generated by the following elements:
1) 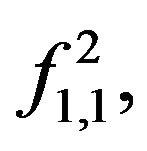
2) 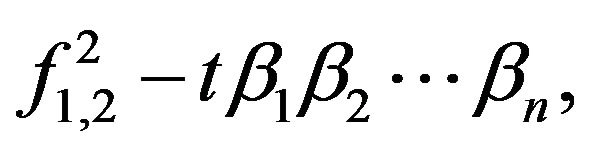
3) 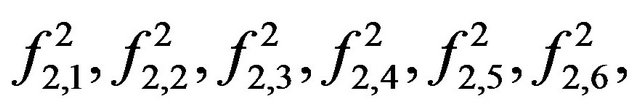
4) 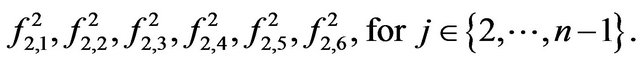
We can show that . Hence this algebra has no non-trivial deformation.
. Hence this algebra has no non-trivial deformation.
From Theorem 4.9 and Theorem 5.4 we have now found 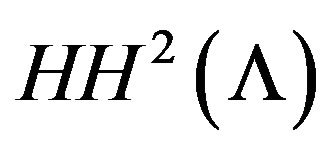 for all standard one-parametric but not weakly symmetric self-injective algebras of tame representation type.
for all standard one-parametric but not weakly symmetric self-injective algebras of tame representation type.
6. Acknowledgements
I thank Prof. Nicole Snashall for her encouragement and helpful comments.
REFERENCES
- R. Bocian, T. Holm and A. Skowroński, “Derived Equivalence Classification of One-Parametric Self-Injective Algebras,” Journal of Pure and Applied Algebra, Vol. 207, No. 3, 2006, pp. 491-536. doi:10.1016/j.jpaa.2005.10.015
- E. L. Green and N. Snashall, “Projective Bimodule Resolutions of an Algebra and Vanishing of the Second Hochschild Cohomology Group,” Forum Mathematicum, Vol. 16, No. 1, 2004, pp. 17-36. doi:10.1515/form.2004.003
- D. Al-Kadi, “Self-Injective Algebras and the Second Hochschild Cohomology Group,” Journal of Algebra, Vol. 321, No. 4, 2009, pp. 1049-1078. doi:10.1016/j.jalgebra.2008.11.019
- D. Happel, “Hochschild Cohomology of Finite-Dimensional Algebras,” Lecture Notes in Mathematics, SpringVerlag, Berlin, 1989. doi:10.1090/S0002-9947-01-02687-3
- E. L. Green, Ø. Solberg and D. Zacharia, “Minimal Projective Resolutions,” Transactions of the American Mathematical Society, Vol. 353, No. 7, 2001, pp. 2915-2939. doi:10.1007/BFb0084073

