ABSTRACT

Reconstruction property in Banach spaces introduced and studied by Casazza and Christensen in [1]. In this paper we introduce reconstruction property in Banach spaces which satisfy -property. A characterization of reconstruction property in Banach spaces which satisfy -property in terms of frames in Banach spaces is obtained. Banach frames associated with reconstruction property are discussed.

1. Introduction

Let 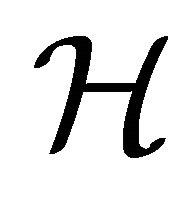be an infinite dimensional separable complex Hilbert space with inner product. A system 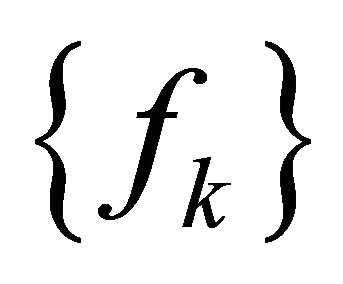called a frame (Hilbert) for 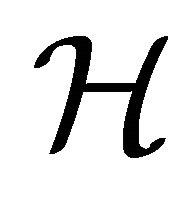if there exists positive constants A and B such that

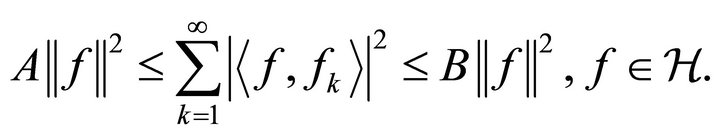
The positive constants and 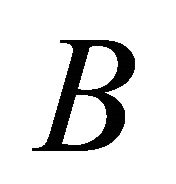are called lower and upper bounds of the frame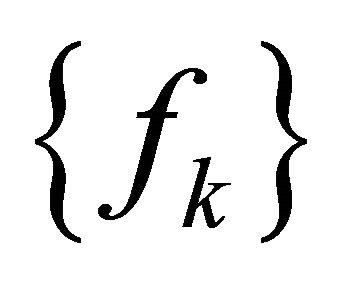, respectively. They are not unique.

The operator 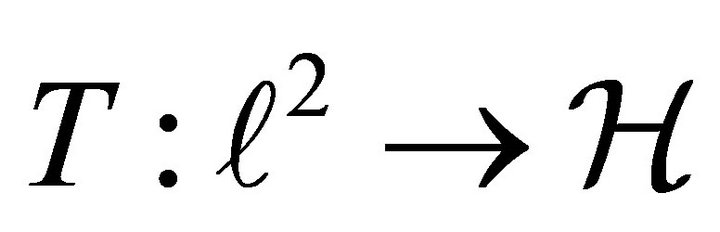given by

is called the synthesis operator or pre-frame operator. Adjoint of T is given by

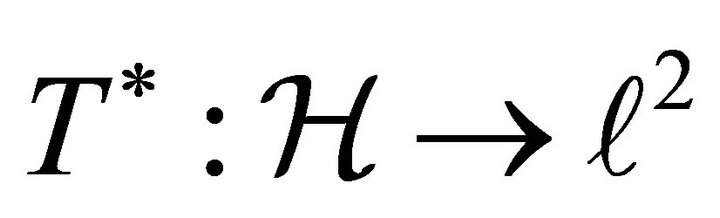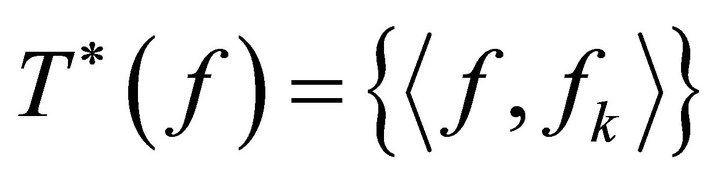, and is called the analysis operator. Composing and 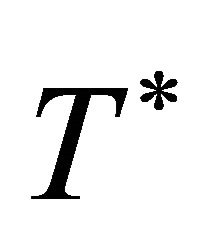we obtain the frame operator 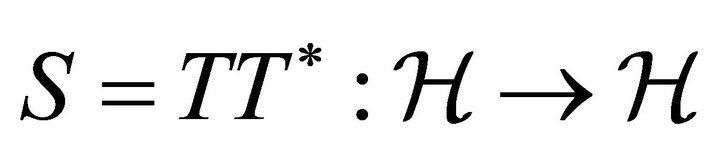given by

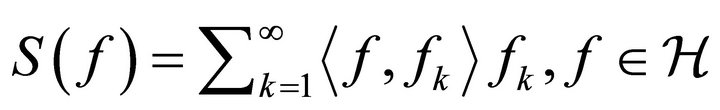. The frame operator S is a positive continuous invertible linear operator from 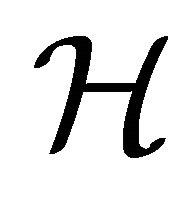onto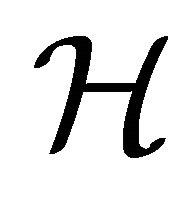. Every vector 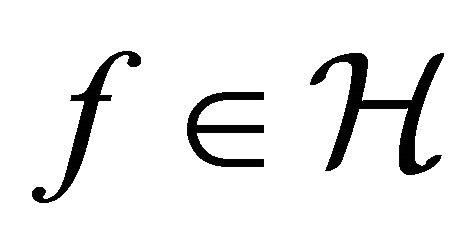can be written as:

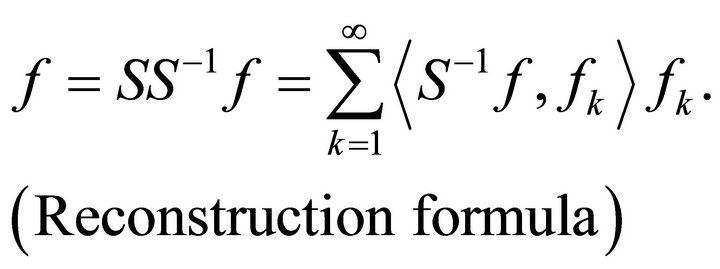
The series in the right hand side converge unconditionally and is called reconstruction formula for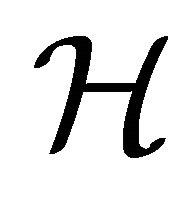. The representation of f in reconstruction formula need not be unique. Thus, frames are redundant systems in a Hilbert space which yield one natural representation for every vector in the concern Hilbert space, but which may have infinitely many different representations for a given vector.

Duffin and Schaeffer in [2] while working in nonharmonic Fourier series developed an abstract framework for the idea of time-frequency atomic decomposition by Gabor [3] and defined frames for Hilbert spaces. Due to some reason the theory of frames was not continued until 1986 when the fundamental work of Daubechies, Grossmann and Meyer published in [4]. Gröchenig in [5] generalized Hilbert frames to Banach spaces. Before the concept of Banach frames was formalized, it appeared in the foundational work of Feichtinger and Gröchenig [6,7] related to atomic decompositions. Atomic decompositions appeared in the field of applied mathematics providing many applications [8,9]. An atomic decomposition allow a representation of every vector of the space via a series expansion in terms of a fixed sequence of vectors which we call atoms. On the other hand Banach frame for a Banach space ensure reconstruction via a bounded linear operator or synthesis operator. Frames play an important role in the theory of nonuniform sampling [10], wavelet theory [11,12], signal processing [2,10], and many more. For a nice introduction of frames and their technical details one may refer to [13].

During the development of frames and expansions systems in Banach spaces Casazza and Christensen introduced reconstruction property for Banach spaces in [1]. Reconstruction property is an important tool in several areas of mathematics and engineering. In fact, it is related to bounded approximation property. Casazza and Christensen in [1] study perturbation theory related to reconstruction property. They develop more general perturbation theory that does not force equivalence of the sequences.

In this paper we introduce and study reconstruction property in Banach spaces which satisfy -property. A characterization of -reconstruction property in terms of frames in Banach spaces is obtained. Banach frames associated with reconstruction property are discussed.

2. Preliminaries

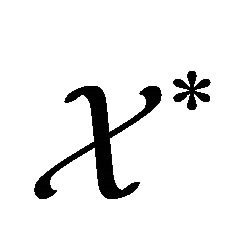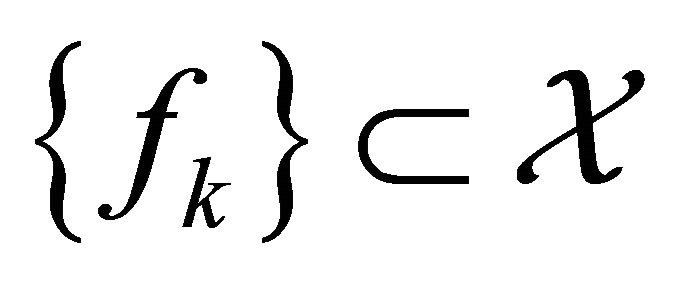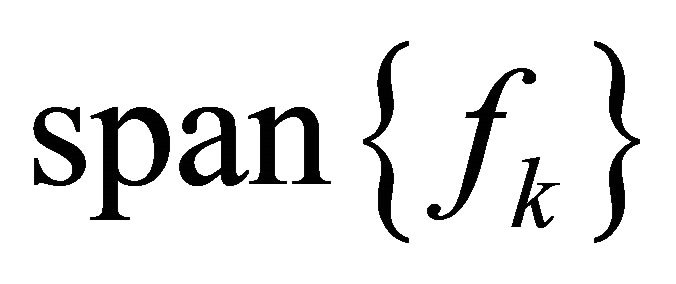Throughout this paper will denotes an infinite dimensional Banach space over a field (which can be or), be the conjugate space, and for a sequence, denotes closure of in norm topology of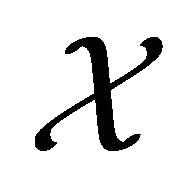. The map 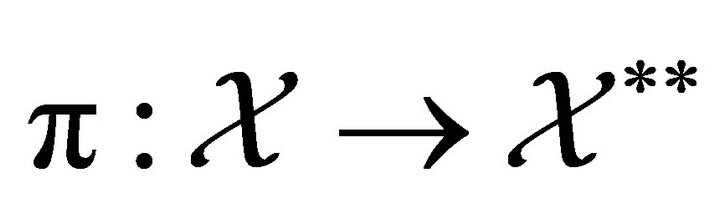denotes the canonical mapping from 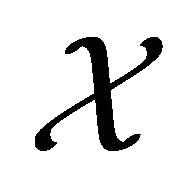into.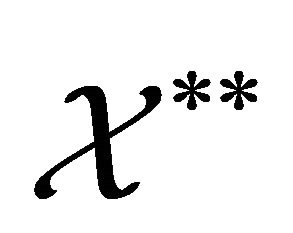

Definition 2.1 ([5]) Let 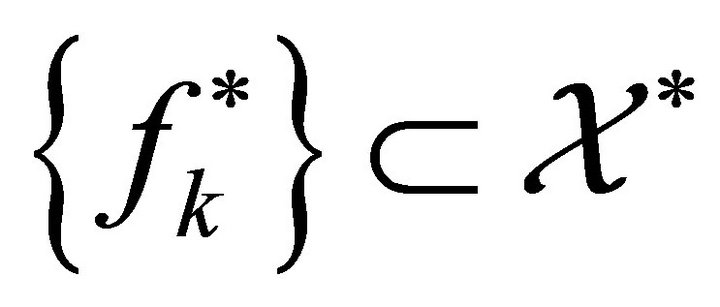and 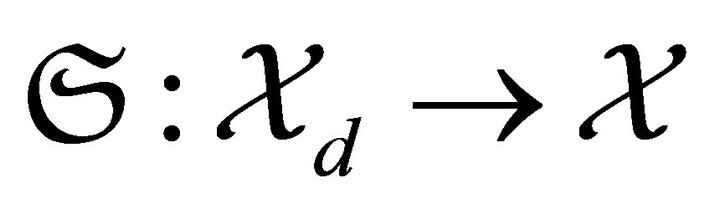be given, where 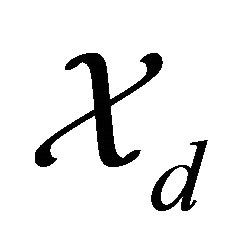is an associated Banach space of scalar valued sequences. A system 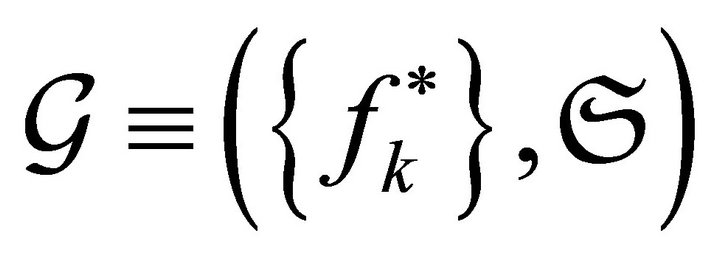is called a Banach frame for 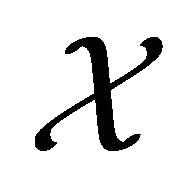with respect to 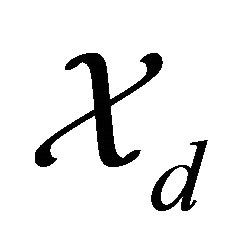if 1), for each.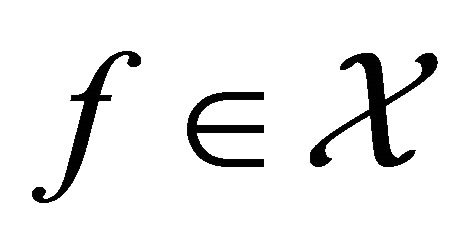

2) There exist positive constants C and D

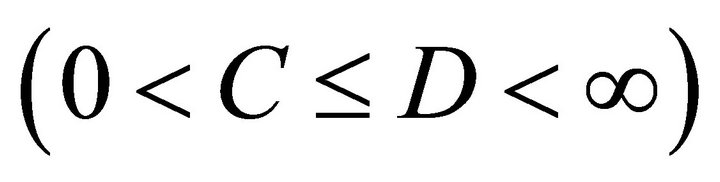such that

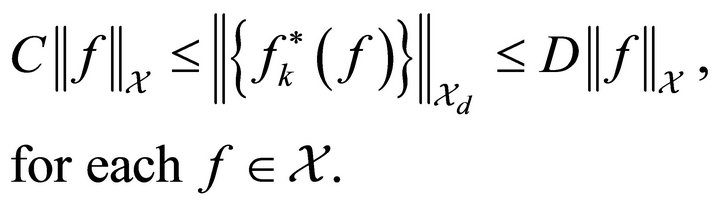(2.1)

3) is a bounded linear operator such that

As in case of frames for a Hilbert space, positive constants C and D are called lower and upper frame bounds of the Banach frame, respectively. The operator 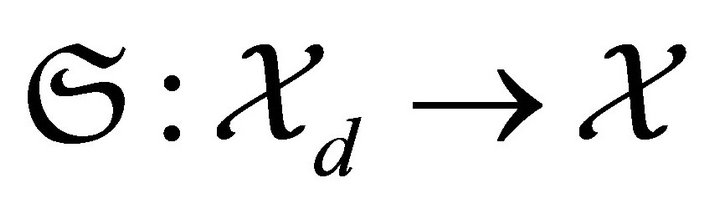is called the reconstruction operator (or the pre-frame operator). The inequality 2.1 is called the frame inequality.

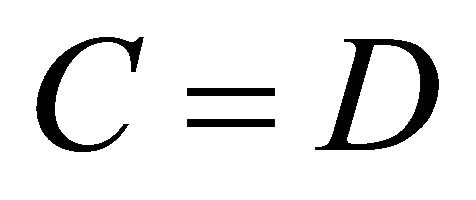The Banach frame is called  tight if and normalized tight if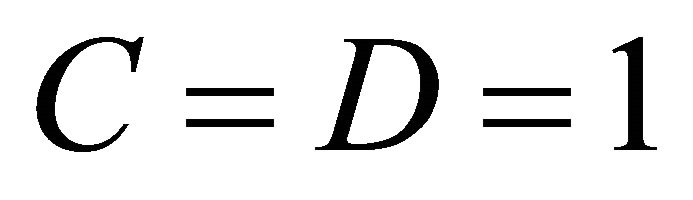. If there exists no reconstruction operator 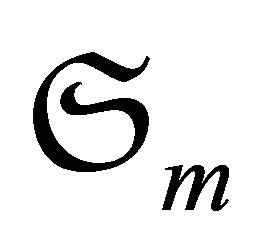such that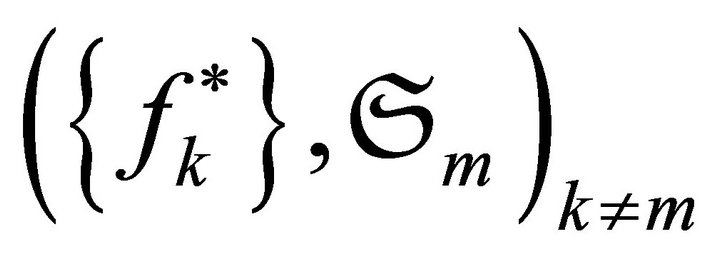

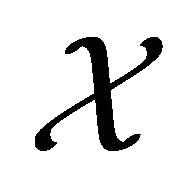is Banach frame for, then will be called an exact Banach frame.

The notion of retro Banach frames introduced and studied in [14].

Definition 2.2 ([14]) A system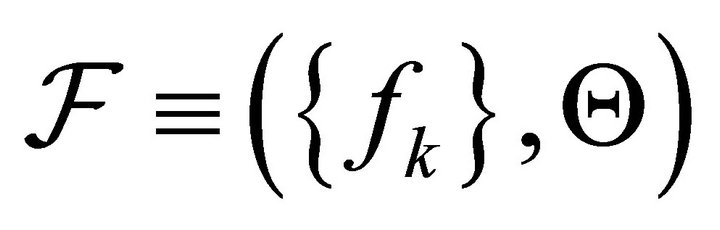

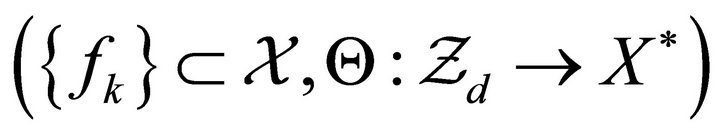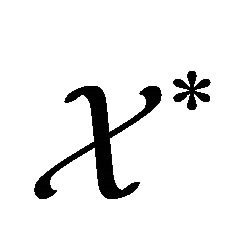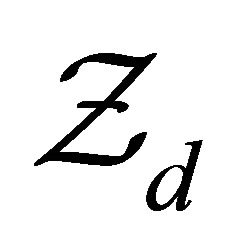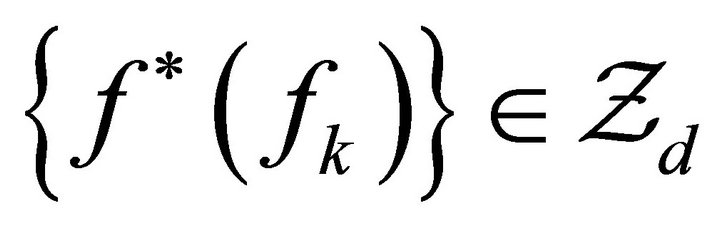is called a retro Banach frame for with respect to an associated sequence space if 1), for each.

2) There exist positive constants 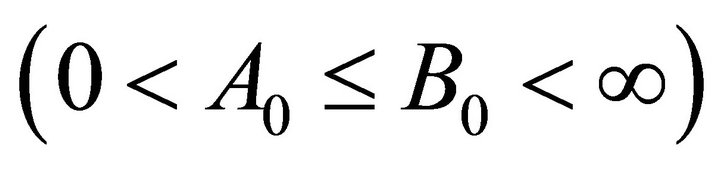such that

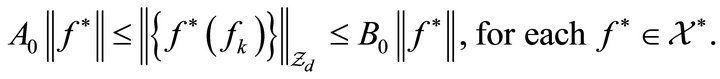
3) 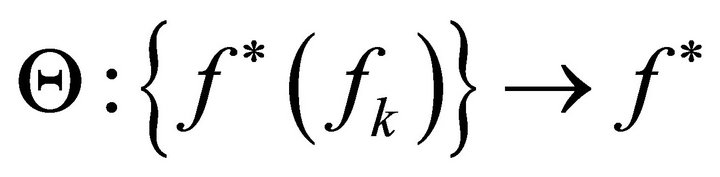is a bounded linear operator from 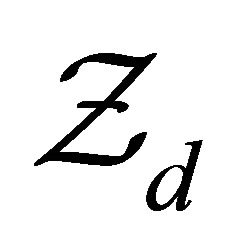onto.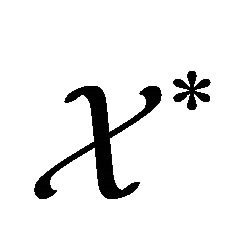

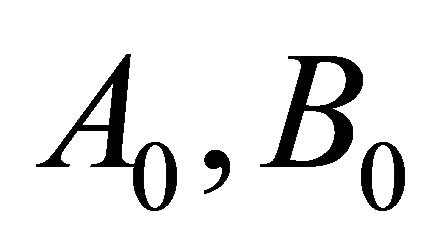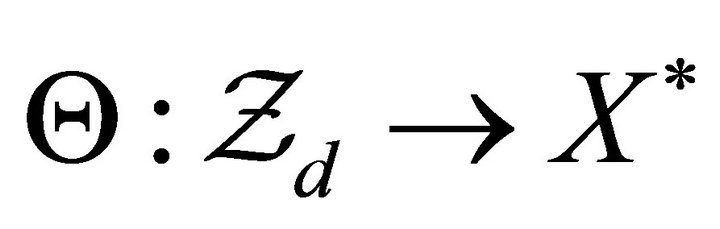The positive constant are called retro frame bounds of and operator is called retro pre-frame operator (or simply reconstruction operator) associated with.

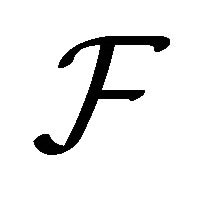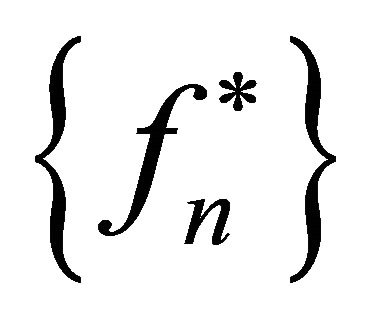
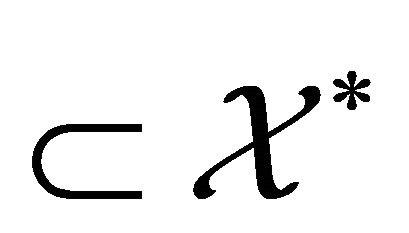Lemma 2.3. Let be a Banach space and be a sequence such that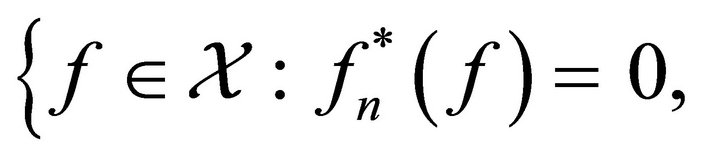

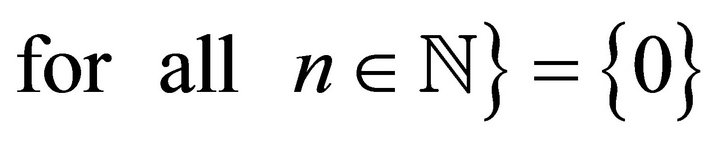. Then, 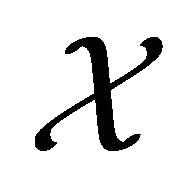is linearly isometric to the Banach space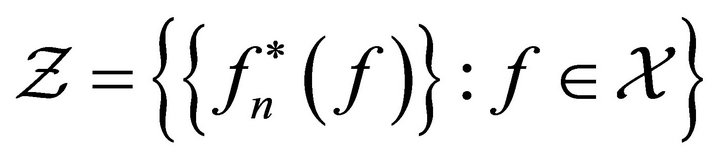, where the norm is given by.

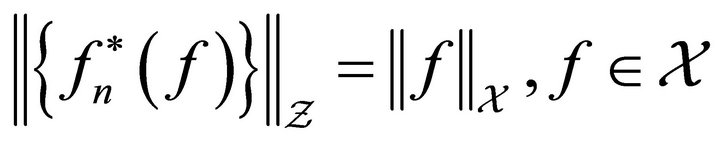Casazza and Christensen in [1] introduced reconstruction property in Banach spaces.

Definition 2.4 ([1]) Let 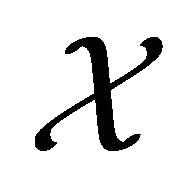be a separable Banach space. We say that a sequence 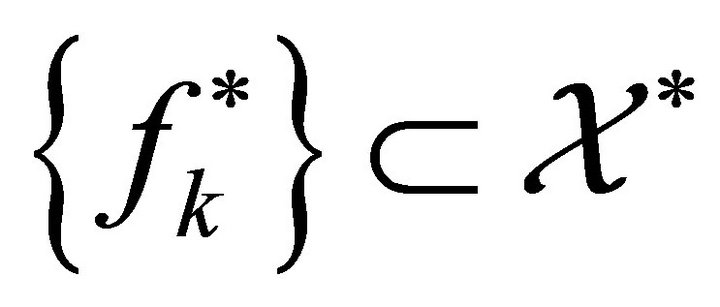has the reconstruction property for 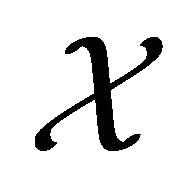with respect to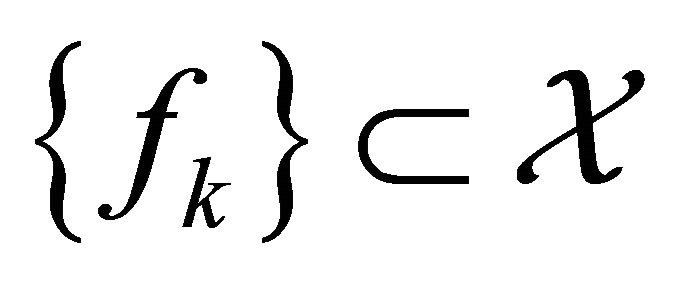, if

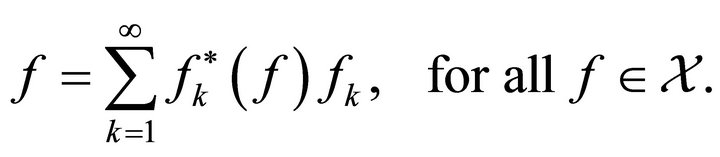
In short, we will also say 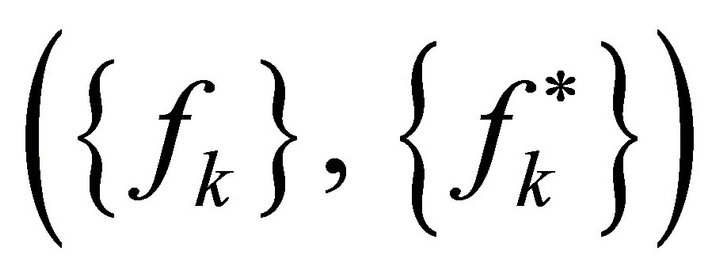has reconstruction property for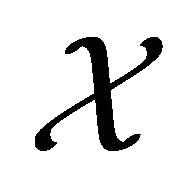. More precisely, we say that 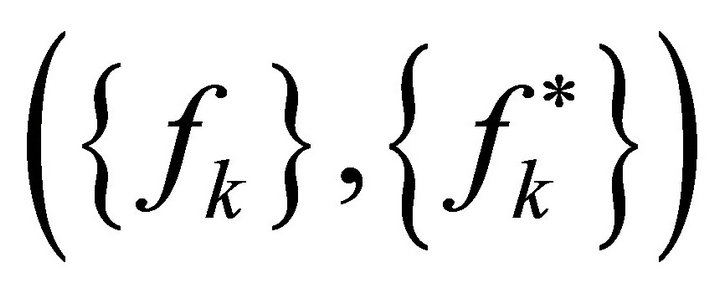is a reconstruction system for.

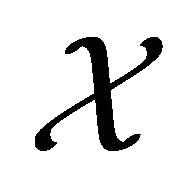Remark 2.5 An interesting example for a reconstruction property is given in [1]: Let 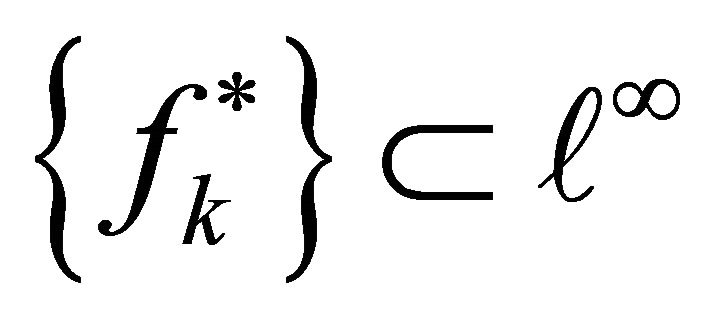and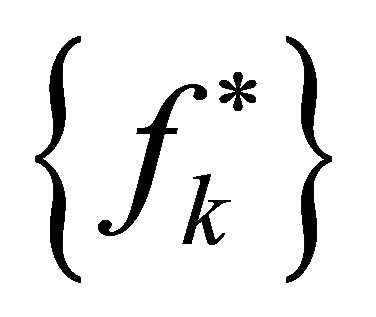

is unitarily equivalent to the unit vector basis of.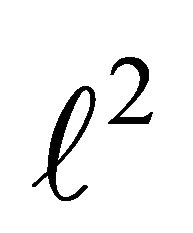

Then, 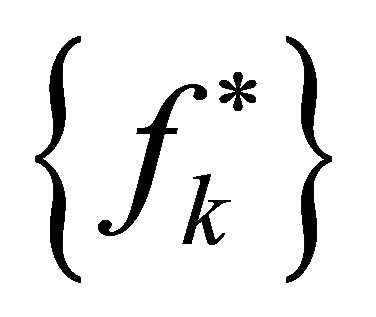has a reconstruction property with respect to its own pre-dual (that is, expansions with respect to the orthonormal basis). Further examples on reconstruction property are discussed in Example 3.4.

Definition 2.6 A reconstruction system 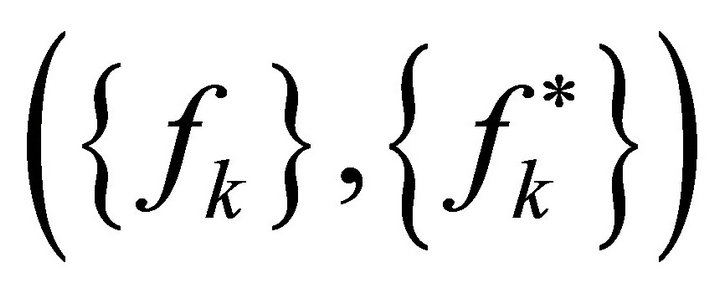for 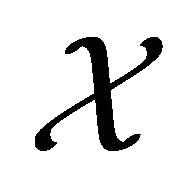is said to be 1) pre-shrinking if.

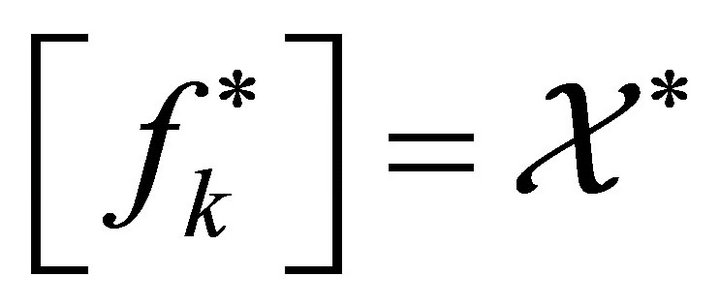2) shrinking if 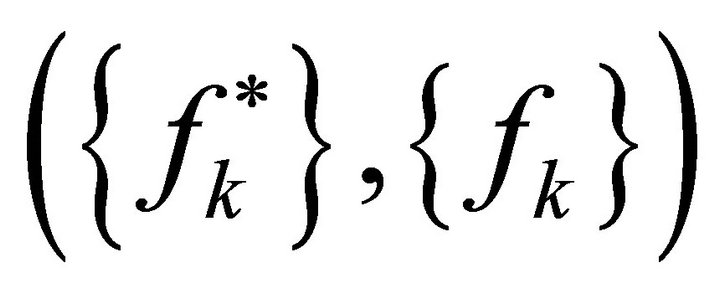is a reconstruction system for.

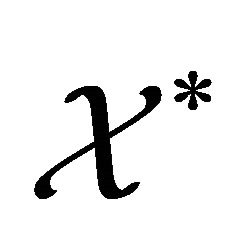Regarding existence of Banach spaces which have reconstruction system, Casazza and Christensen proved the following result.

Proposition 2.7 ([1]) There exists a Banach space 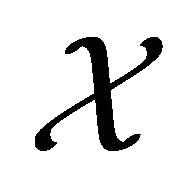with the following properties:

1) There is a sequence 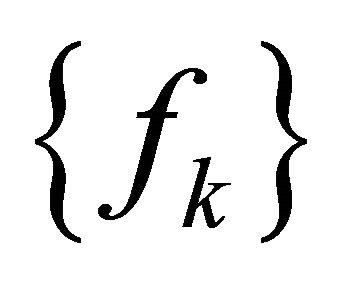such that each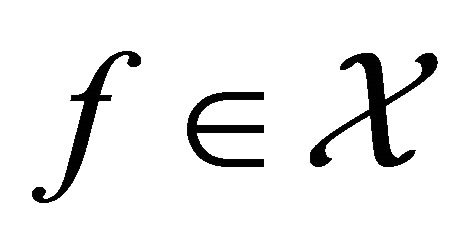

has a expansion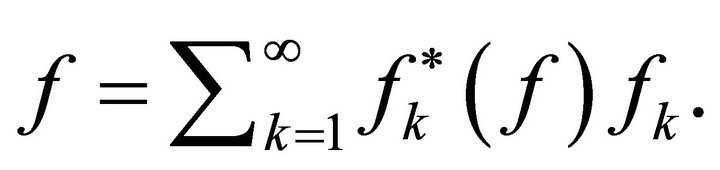

2) does not have the reconstruction property with respect to any pair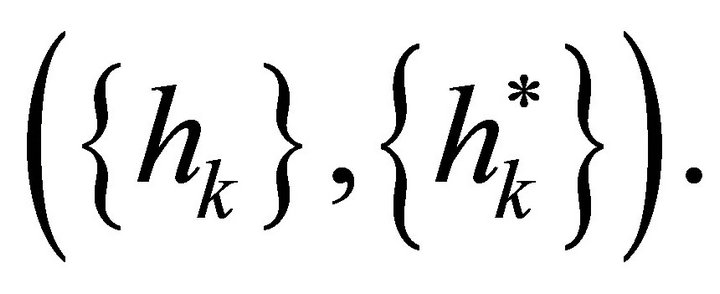

The notion of reconstruction property is related to Bounded Approximation Property (BAP). If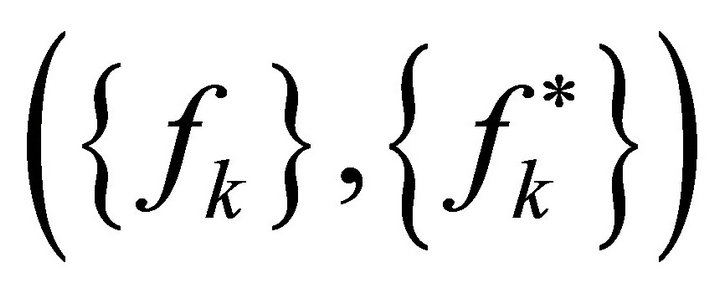

has reconstruction property for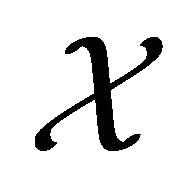, then has the bounded approximation property. So, 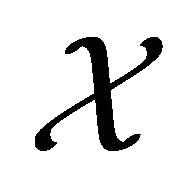is isomorphic to a complemented subspace of a Banach space with a basis. It is also used to study geometry of Banach spaces. For more results and basics on reconstruction property and bounded approximation property one may refer to [15] and references therein.

3. -Reconstruction Property

Definition 3.1 Suppose has the reconstruction property for 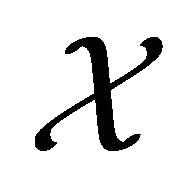with respect to.

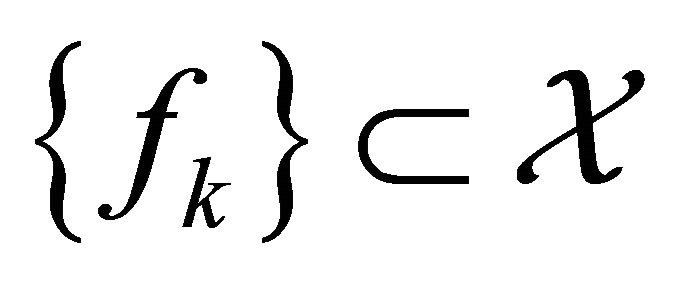Then, we say that 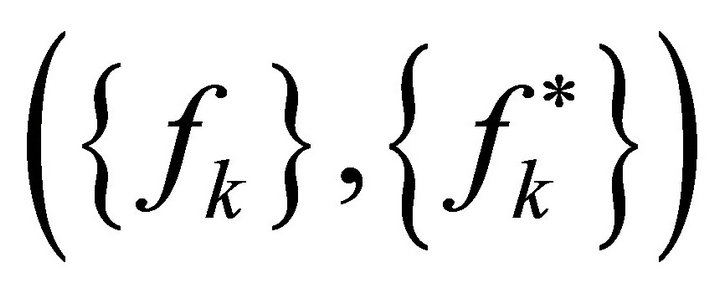satisfy property if

and there exists a functional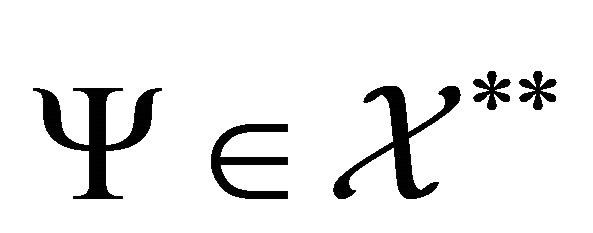

such that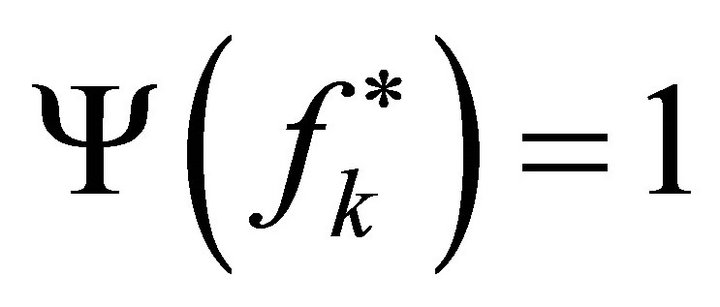, for all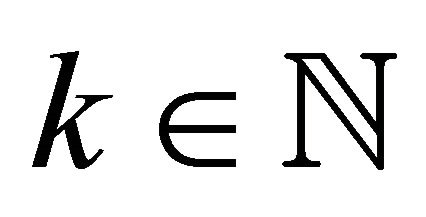. In this case we say that 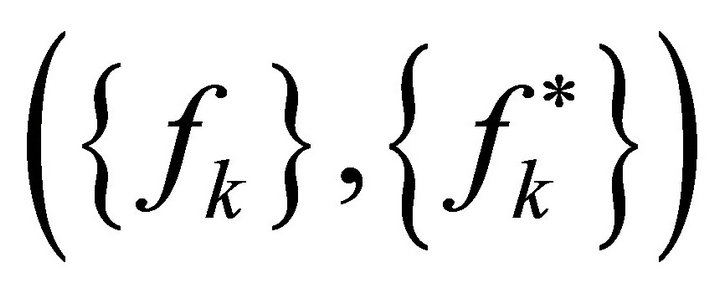is a -reconstruction system for

.

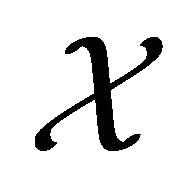Remark 3.2 If and there exists a functional 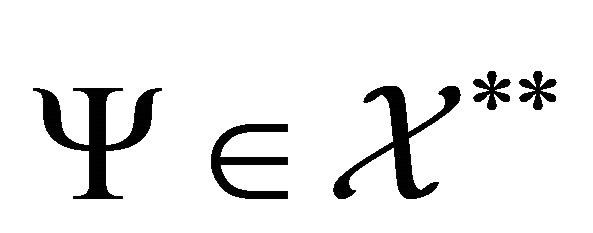such that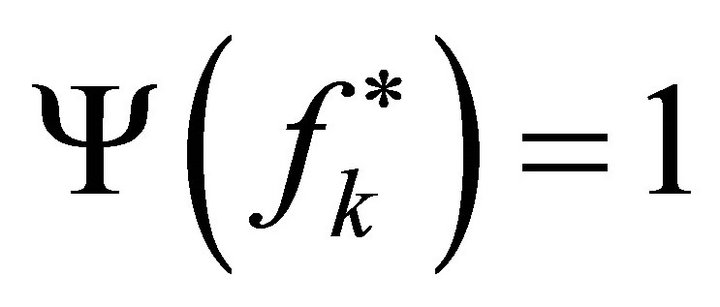, for all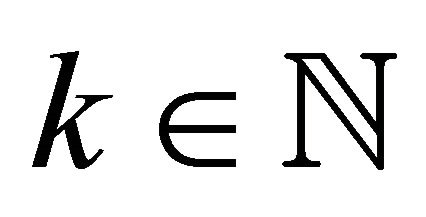then we say that 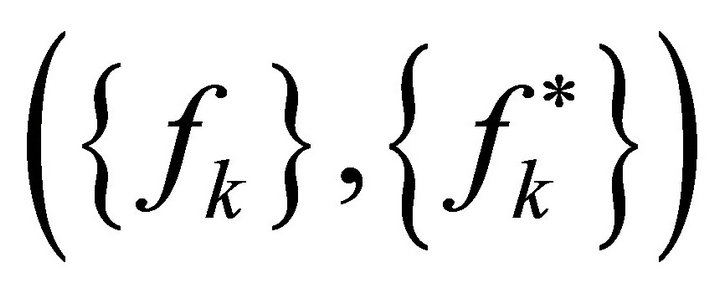is a 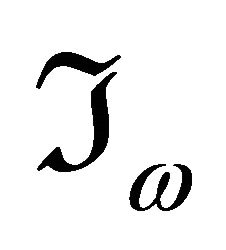-reconstruction system (or weak -reconstruction system for).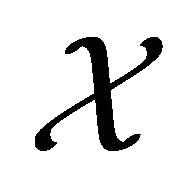

Remark 3.3 A -reconstruction system is actually a dual system of a -Schauder frame [16] in the context of reconstruction property.

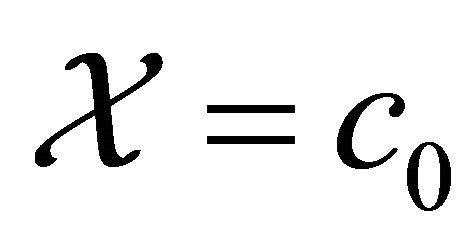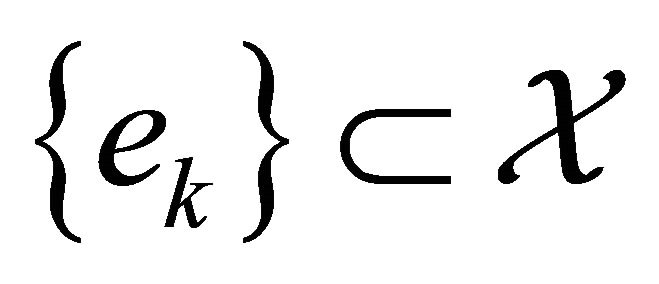Example 3.4 Let and be a sequence of canonical unit vectors. Define 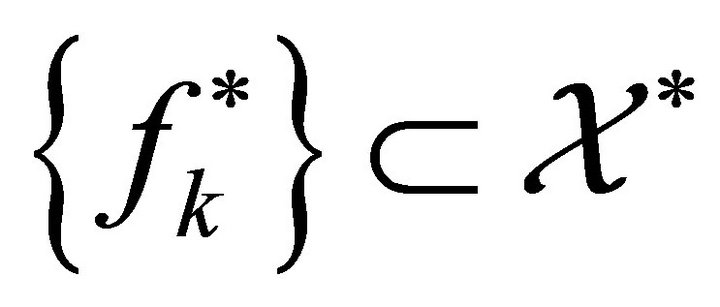by

.

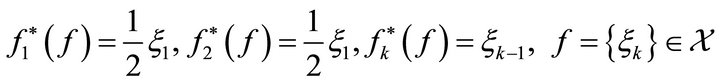Then, 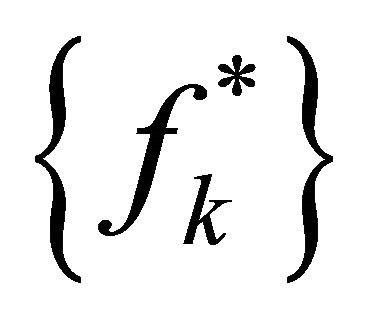has a reconstruction property with respect to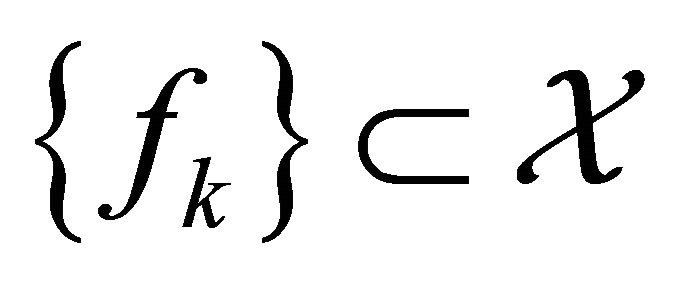, wher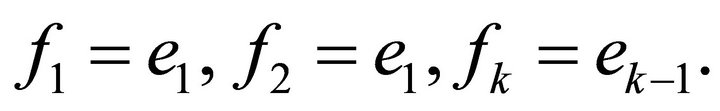e Hence

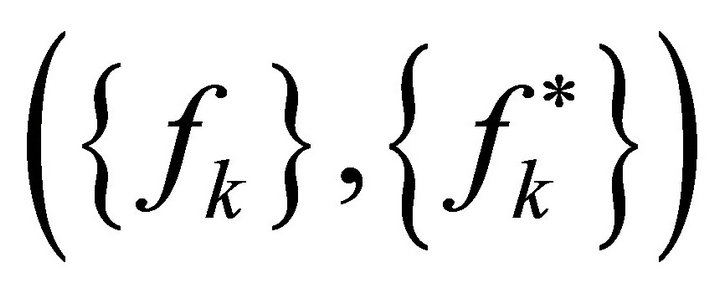is a -reconstruction system for 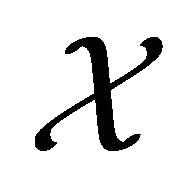[See Proposition 3.5]. Note that the reconstruction system

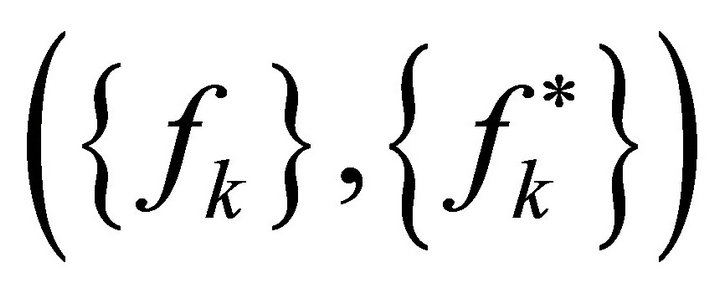is shrinking.

Now define 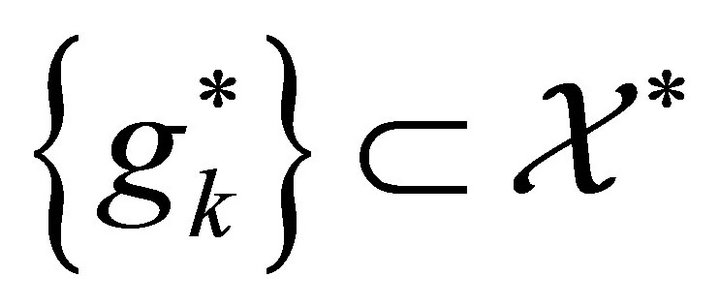by

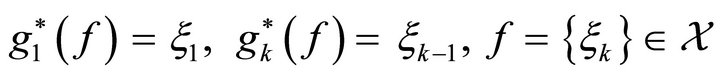. Then,

has a reconstruction property with respect to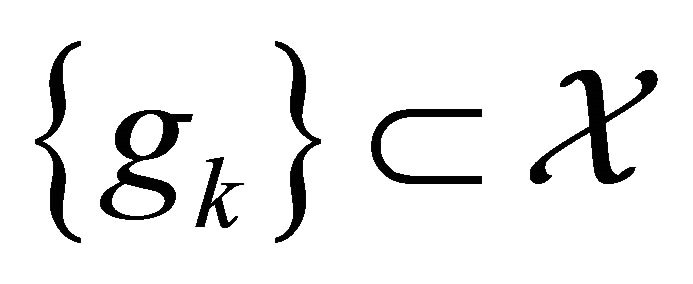, where 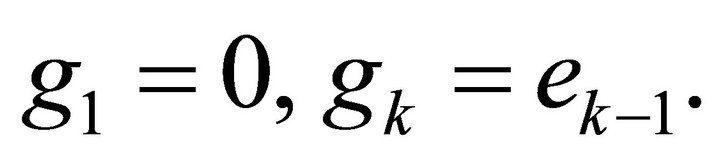By Proposition 3.5,

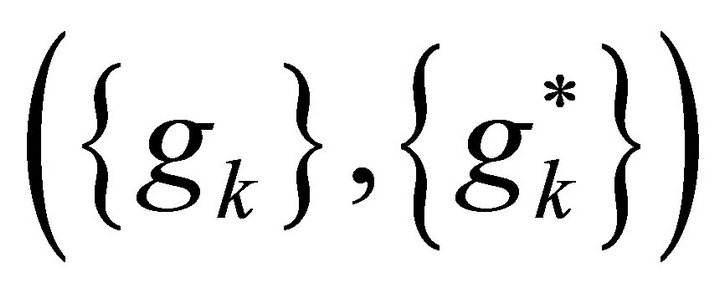is not a -reconstruction system for.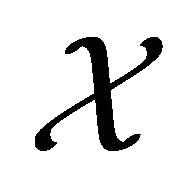

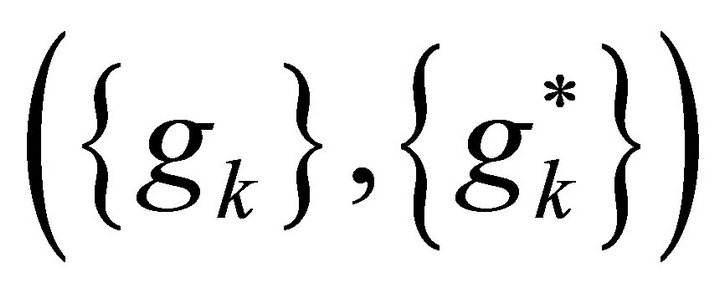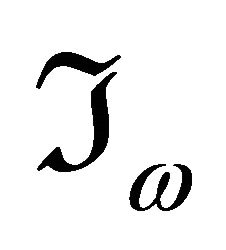Note that is -reconstruction system which is shrinking. Thus, a shrinking reconstruction system for 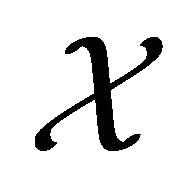need not be a -reconstruction system.

We now give a characterization of a -reconstruction system for 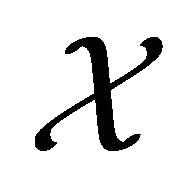as claimed in section 1, in terms of frames.

Proposition 3.5 Let 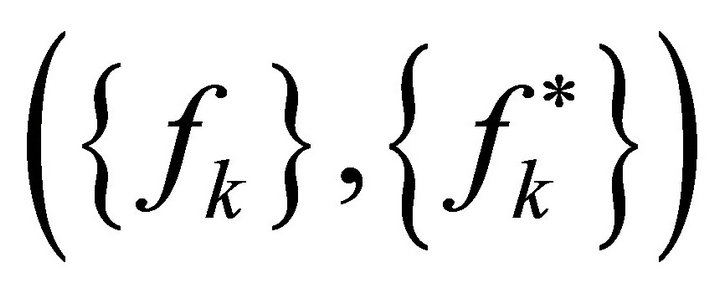be a reconstruction system for 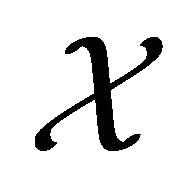with. Then,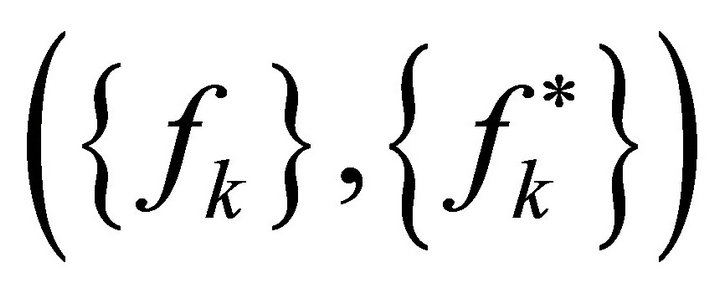

satisfy property if and only if there is no retro preframe operator such that 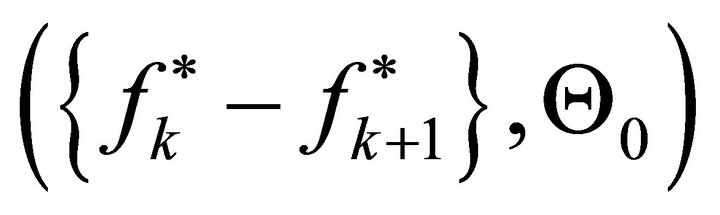is retro Banach frame for.

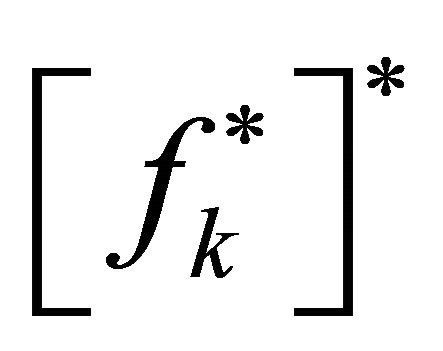This is an immediate consequence of the following lemma.

Lemma 3.6 Let 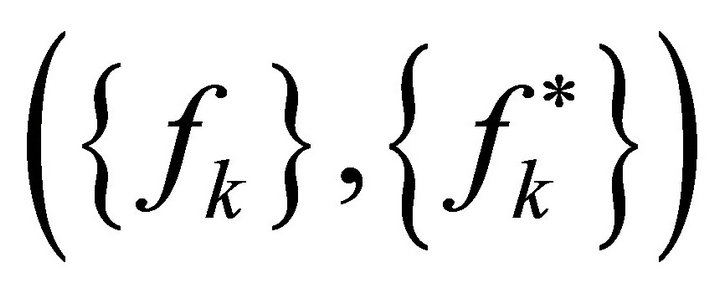be a pre-shrinking reconstruction system for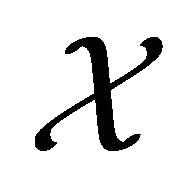. Then, 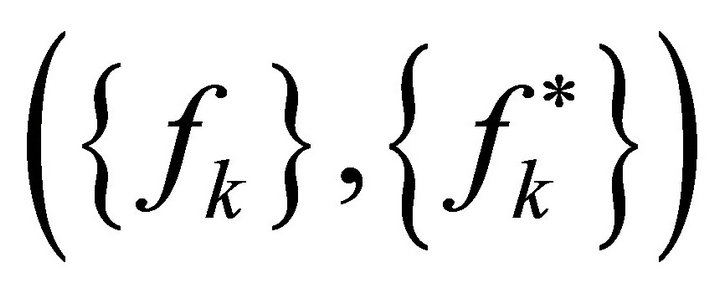is a

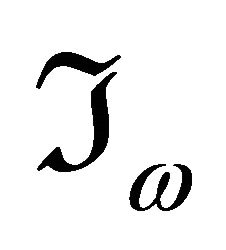-reconstruction system if and only if there exists no retro pre-frame operator such that 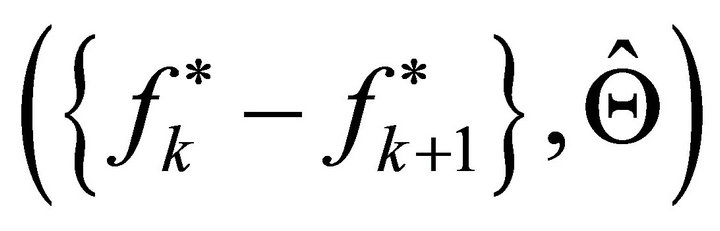is retro Banach frame for.

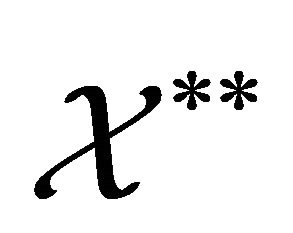Proof. Forward part is obvious. Indeed, by using lower retro frame inequality of and existence of 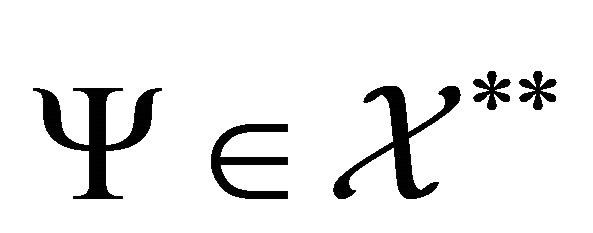such that 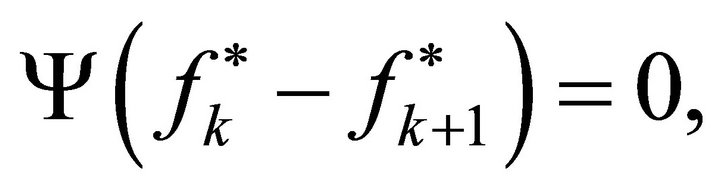for all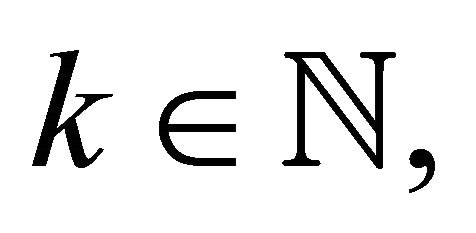

we obtain 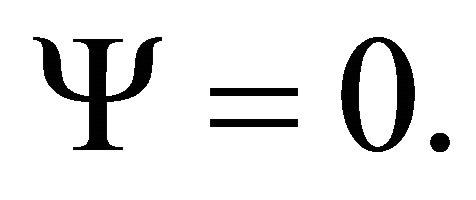This is a contradiction.

For reverse part, let if possible, there is no reconstruction operator such that 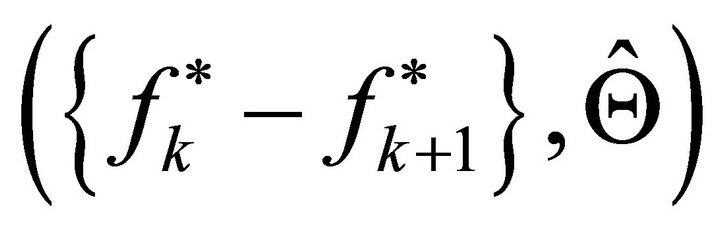is a retro Banach frame for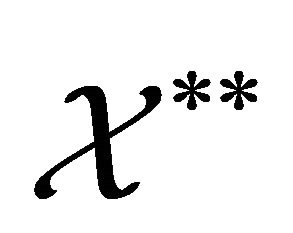. Then, Hahn Banach Theorem force to admit a non zero functional 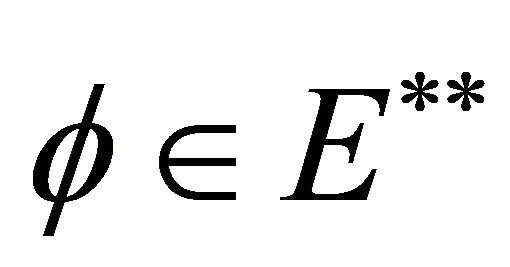such that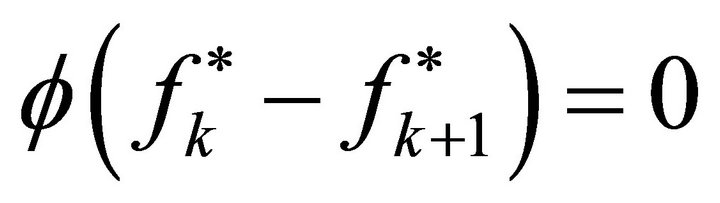, for all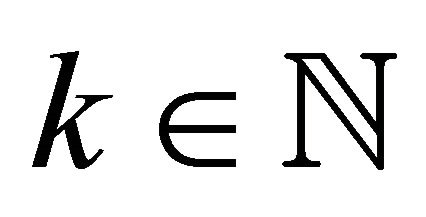. That is, 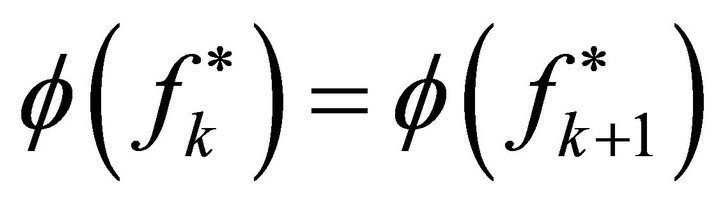, for all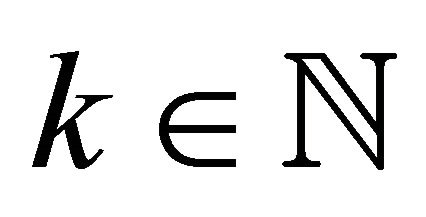. Put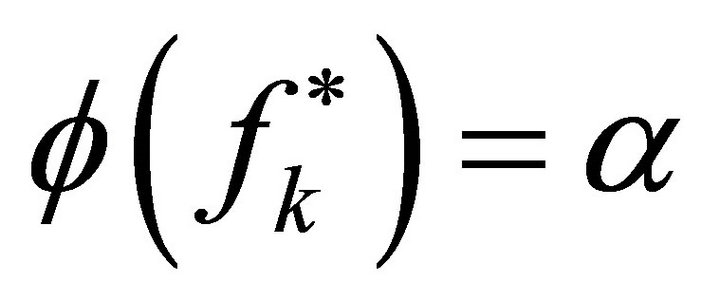, for all

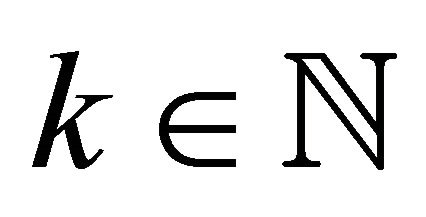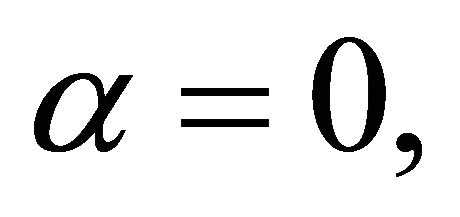. If then 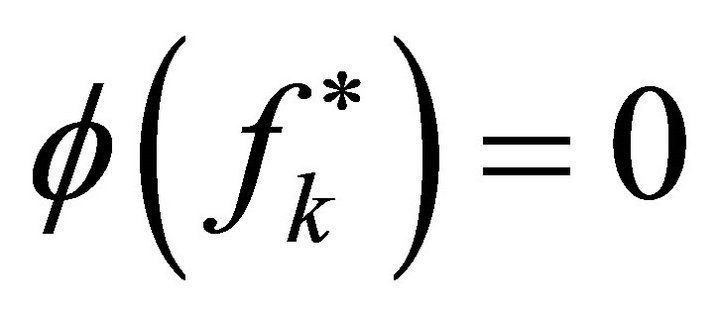for all 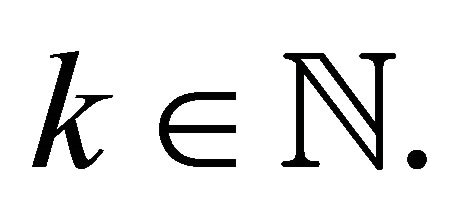But

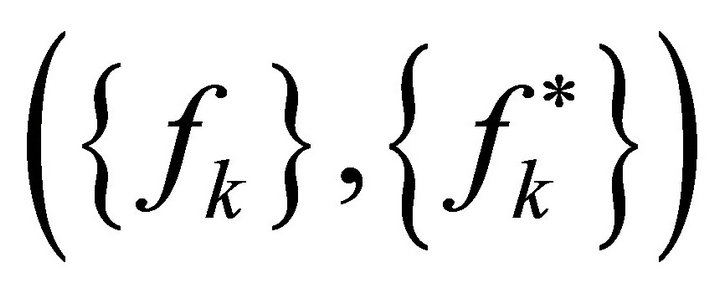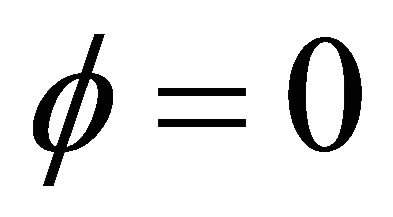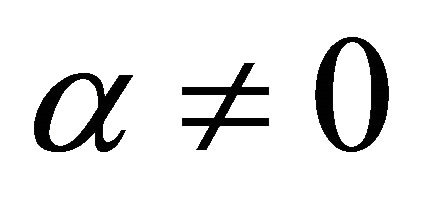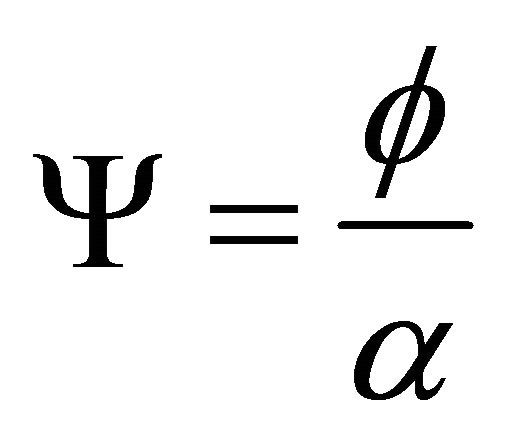is pre-shrinking, therefore, a contradiction. Thus. Put. Then, 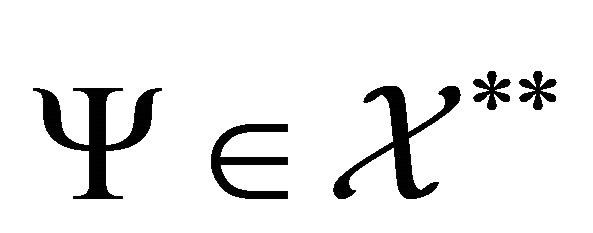is such that 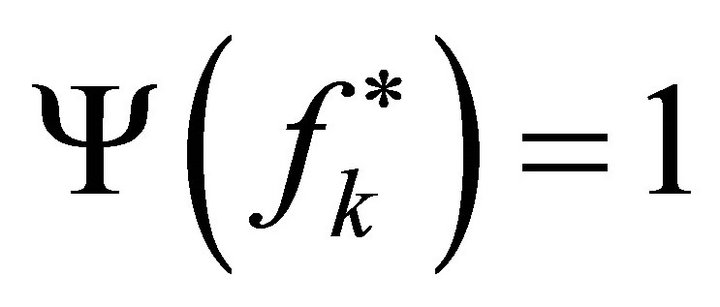for all 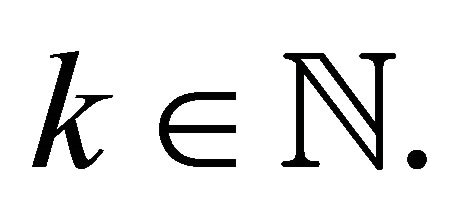Thus,

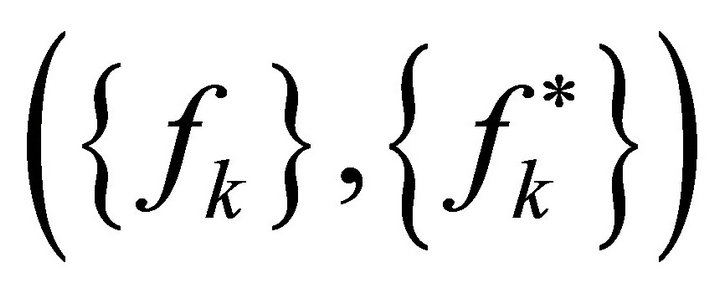is a 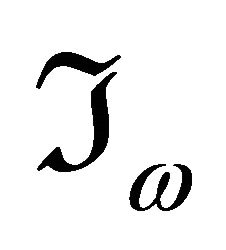-reconstruction system.

Remark 3.7 Note that Lemma 3.6 is no longer true if 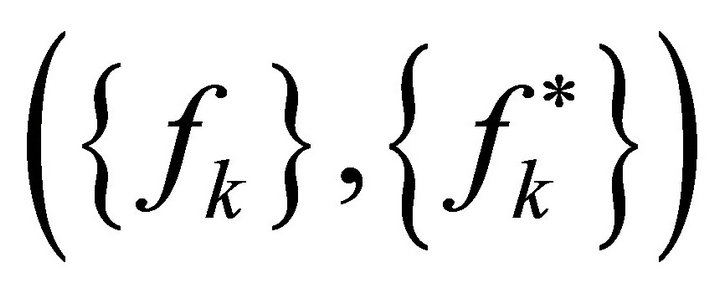is not pre-shrinking.

Application: Let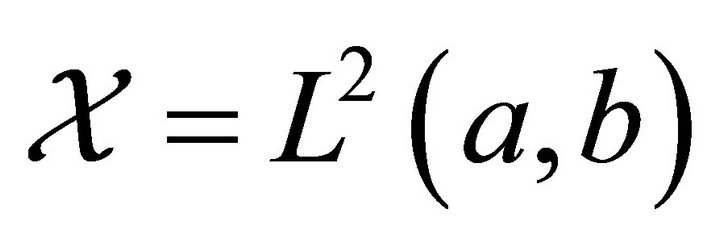. Consider a boundary value problem(BVP) with a set of n boundary conditions:

BVP: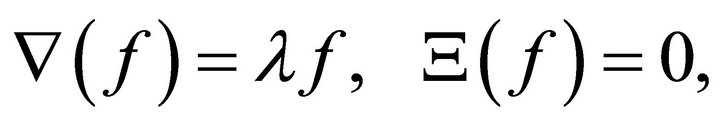

where 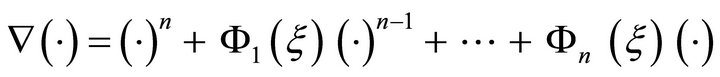is a linear differential operator with 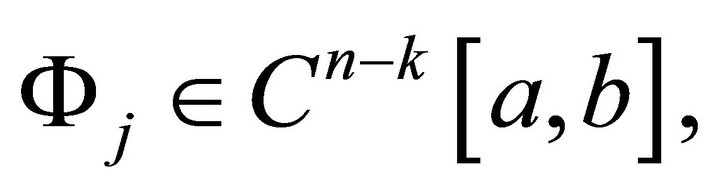and 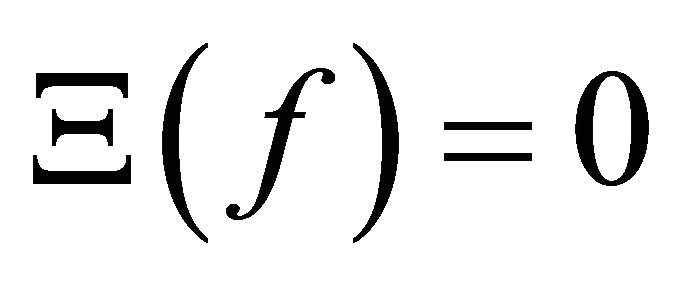denotes the set of n boundary conditions:

It is given in [17] (at page 66) that for a large class of boundary conditions (which are known as regular boundary conditions), the BVP admits a system 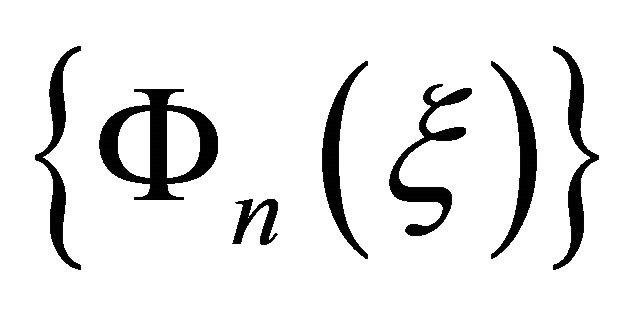and 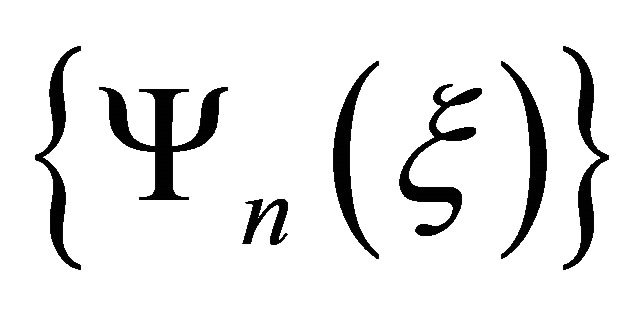consisting of eigenfunction associated with given BVP such that

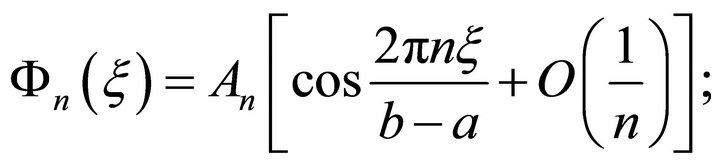
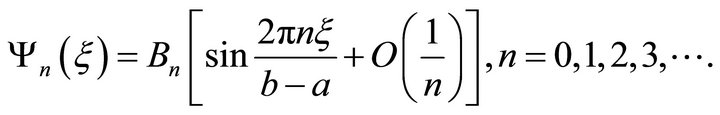
It is well known that the corresponding to

there exists a

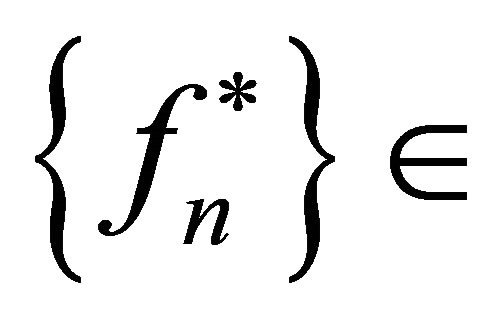
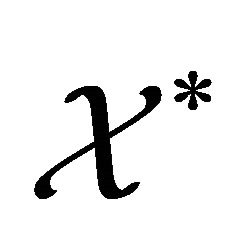such that 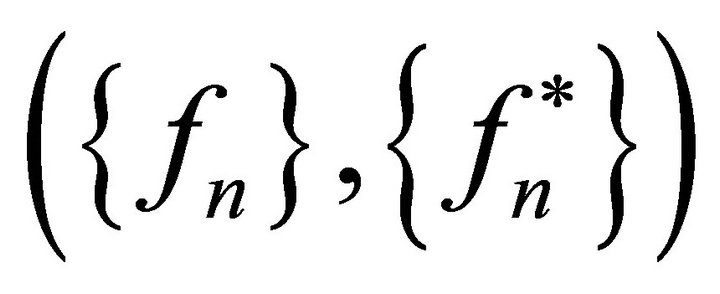is a reconstruction system for

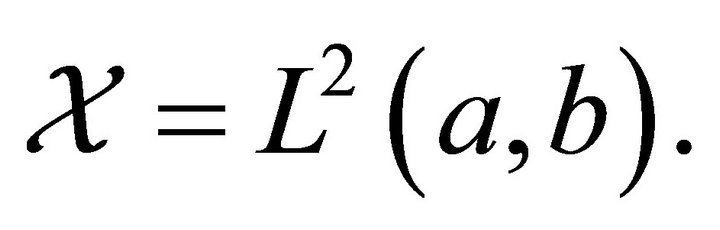Now

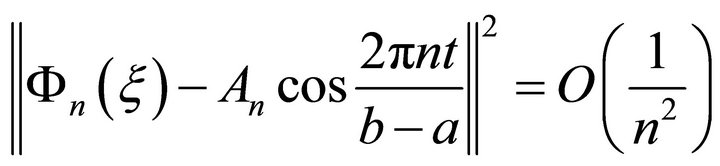and

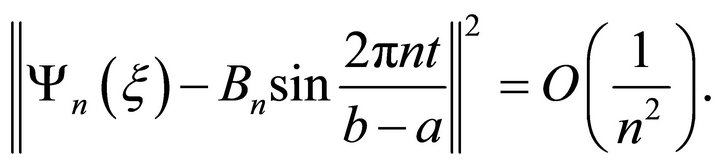
Therefore, by using Paley and Wiener theorem in [18, p. 208], there exists a sequence 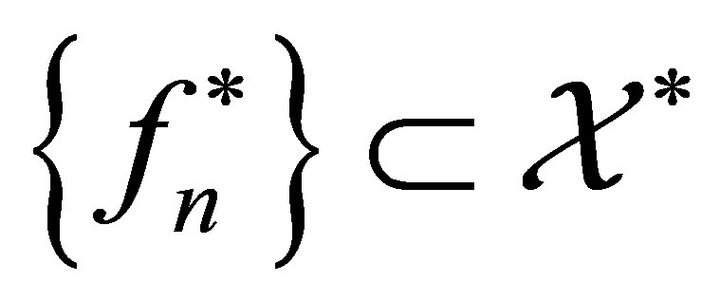such that

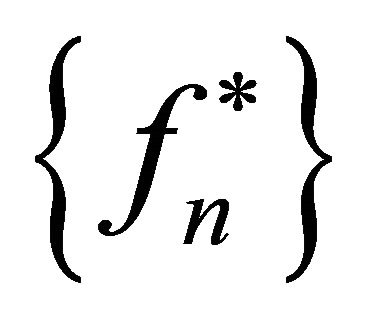admits a reconstruction system with respect to 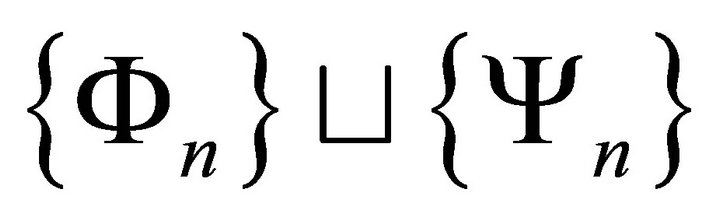. This reconstruction system is not of type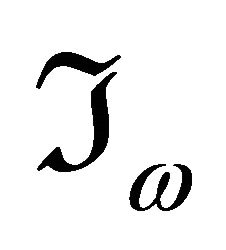. Therefore, by using Lemma 3.6, there exists a retro pre-frame operator such that 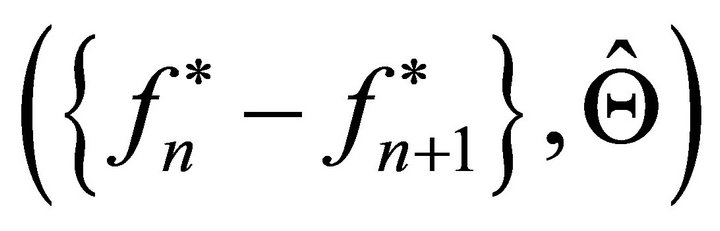is retro Banach frame for. Recall that if we write a function in terms of reconstruction system, then computation of all the coefficients is required. If calculation of coefficients which appear in the series expansion of a given reconstruction system are complicated, then we reconstruct the function by pre-frame operator of.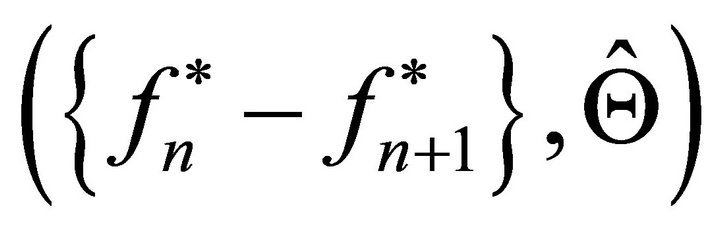

The following proposition provides a sufficient condition for a reconstruction system to satisfy property.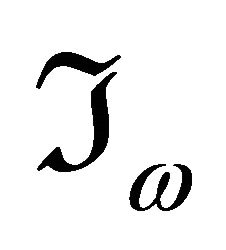

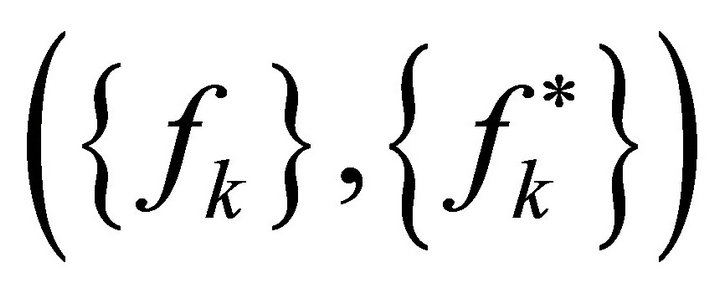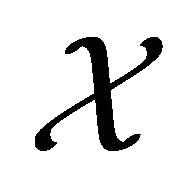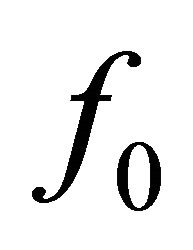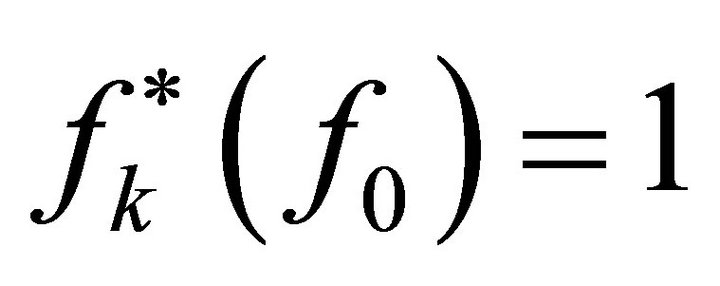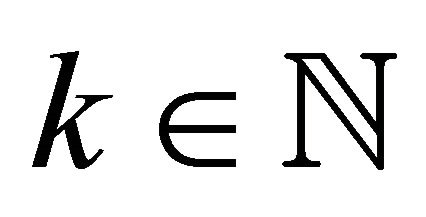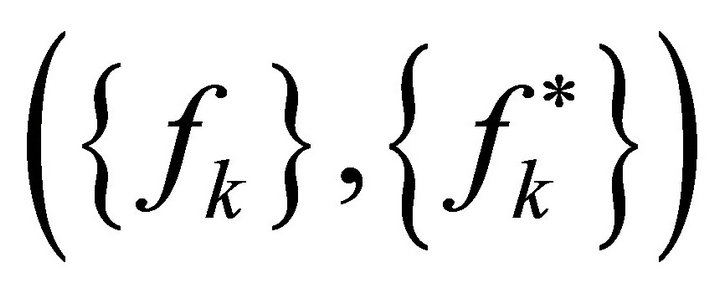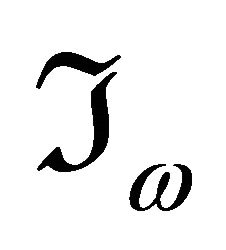Proposition 3.8 Let be a reconstruction system for. If there exists a vector in such that for all, then is a -reconstruction system.

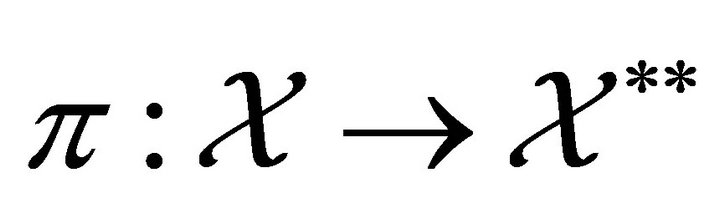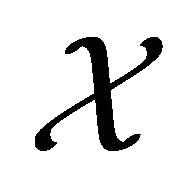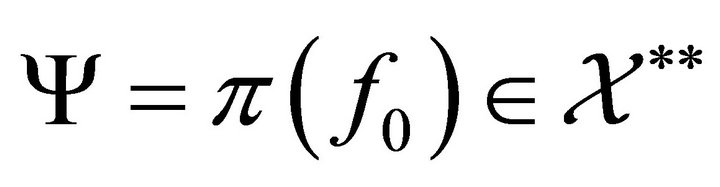Proof. Let be the canonical embedding of into. Then is such that

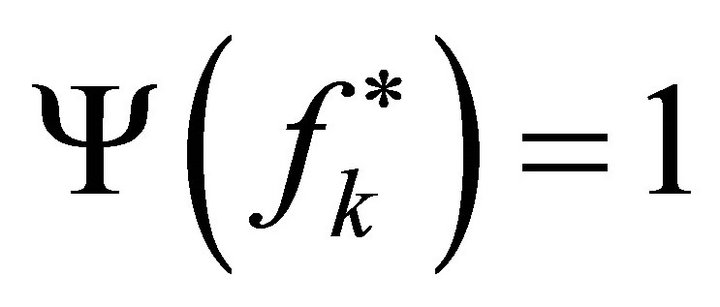, for all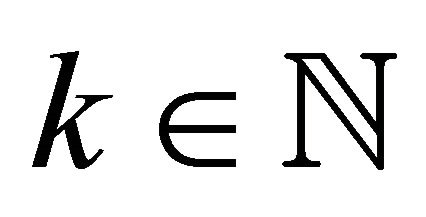. Thus, 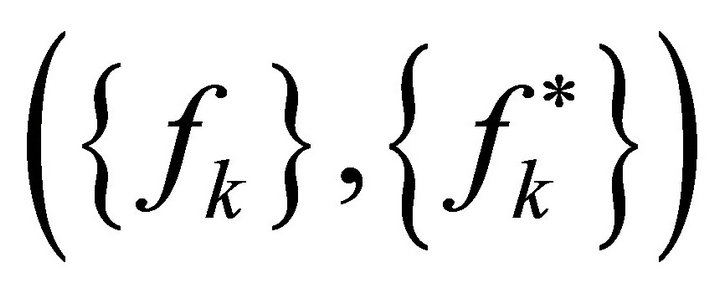is a 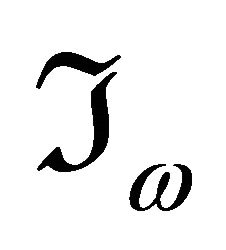reconstruction system for.

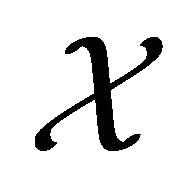Remark 3.9 The condition in Proposition 3.8 is not necessary. However, if 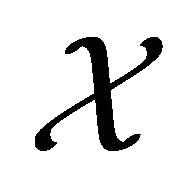is reflexive, then the condition given in Proposition 3.8 turns out to be necessary. Moreover, this is equivalent to the condition: There exists no pre-frame operator such that 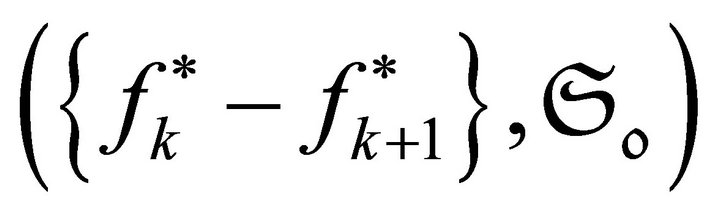is a Banach frame for.

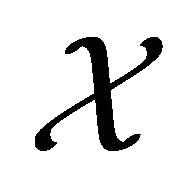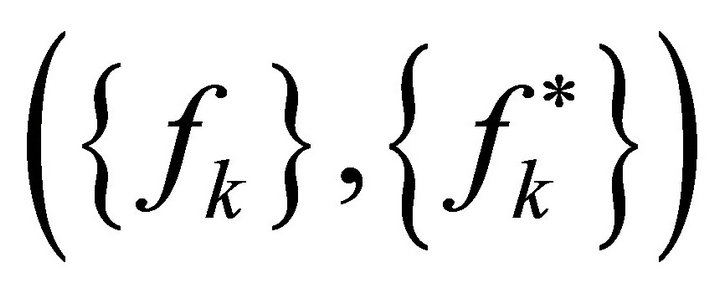To conclude the section we show that a given - reconstruction system in Banach spaces produce another -reconstruction system: Consider a -reconstruction system for.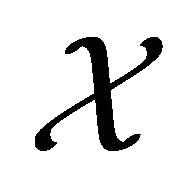

Let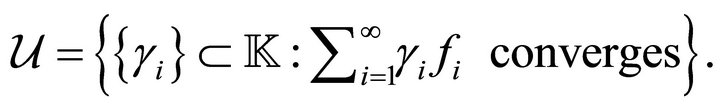

Then 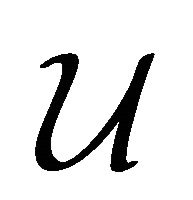is a Banach space with norm given by

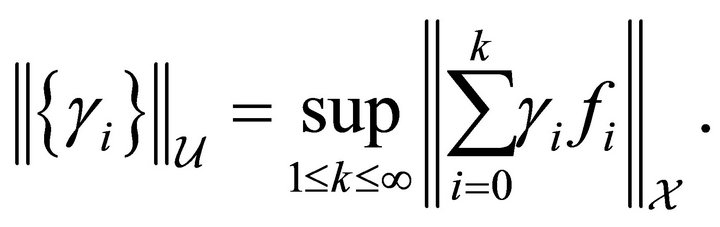
Define 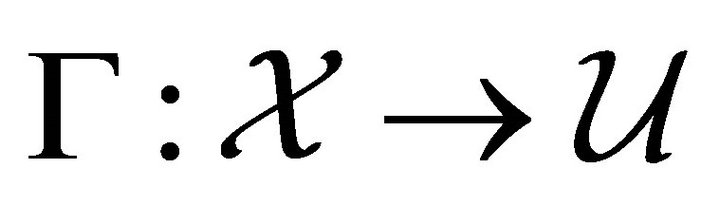by.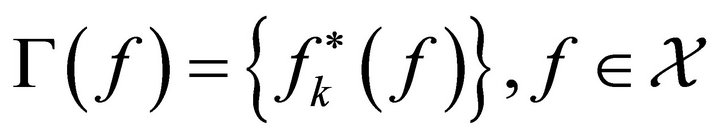

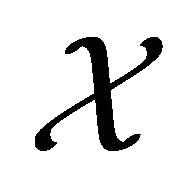Then is an isomorphism of into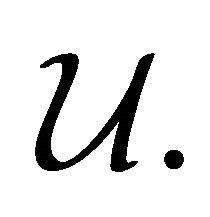

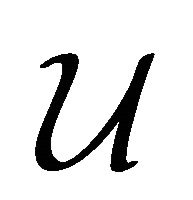Also defined by is also a bounded linear operator from onto.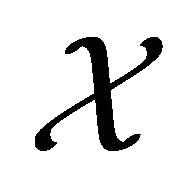

Put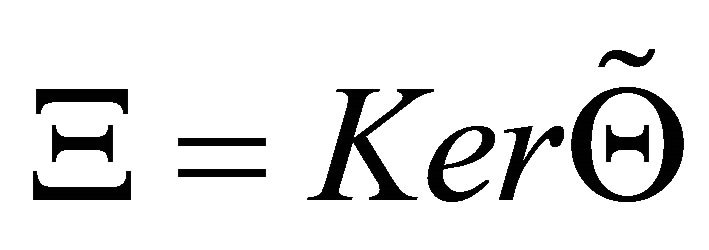. Then is a closed subspace of 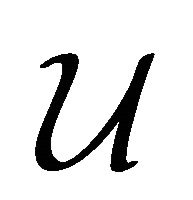such that 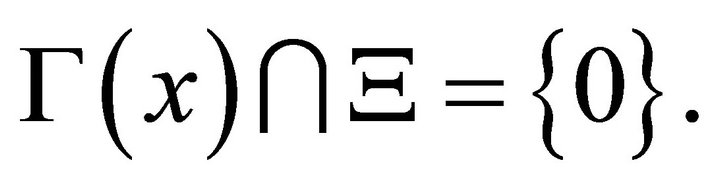Moreover, if 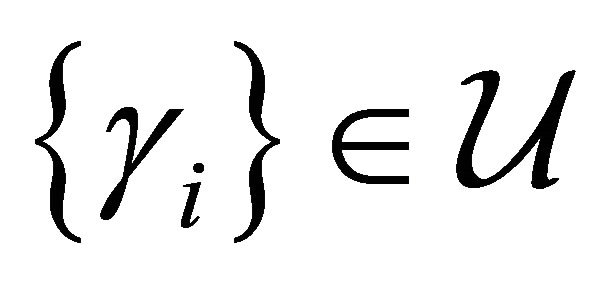is any element such that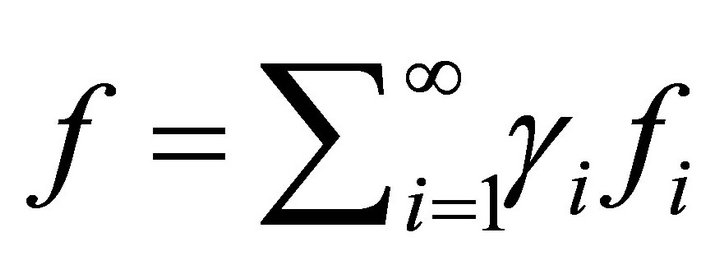, then

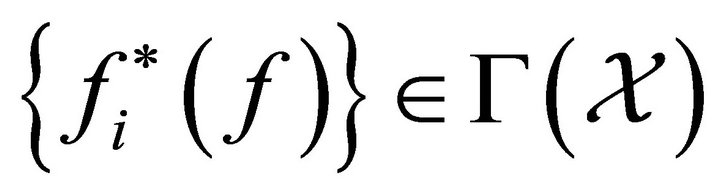and

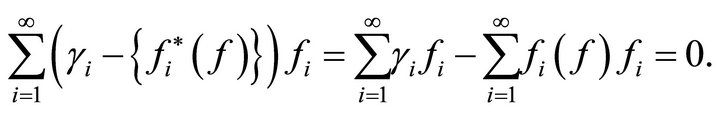
Therefore, 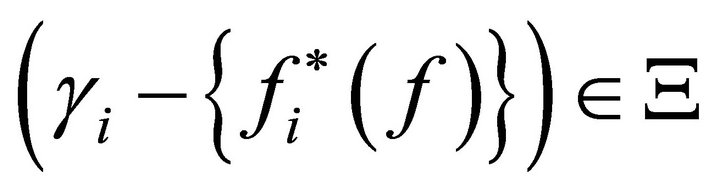is such that

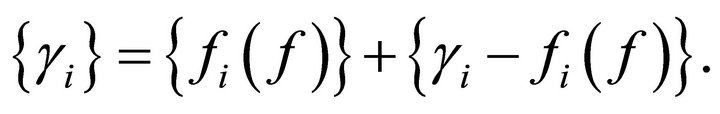
Hence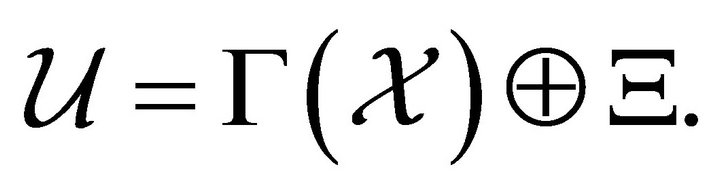

Let V be projection on 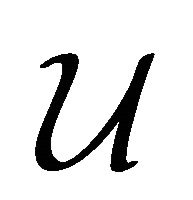onto.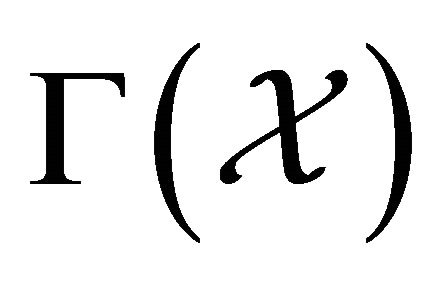

Then,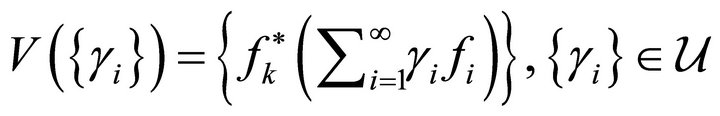. Thereforefor each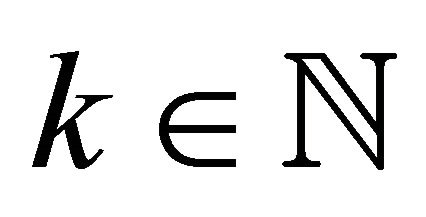, we have

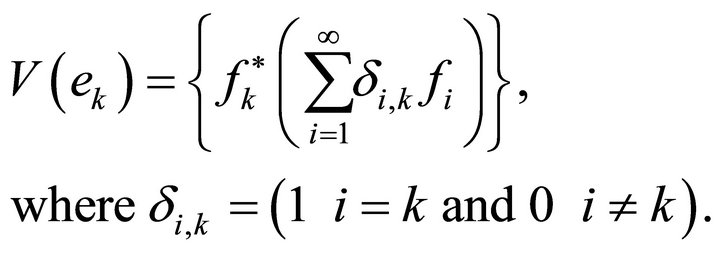
That is: 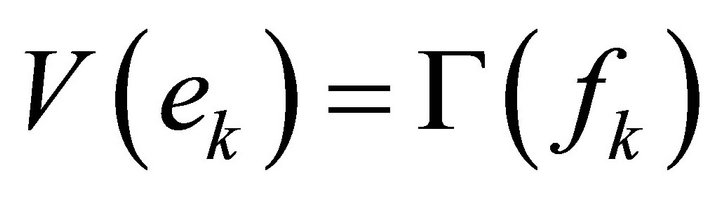for all So,

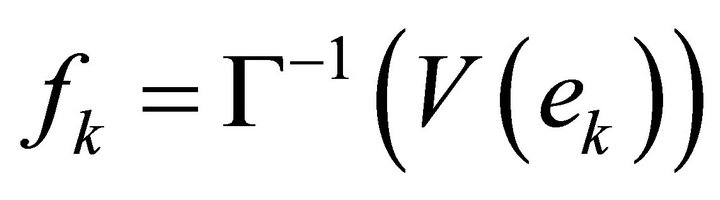for all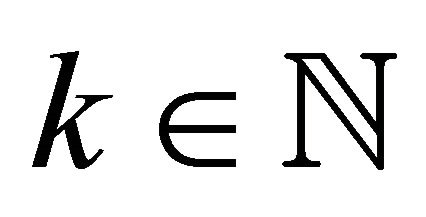, where is sequence of canonical unit vectors in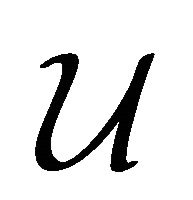. Hence

is a reconstruction system for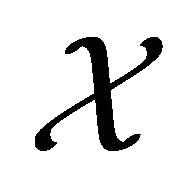

which satisfy property.

This is summarized in the following proposition.

Proposition 3.10 Let 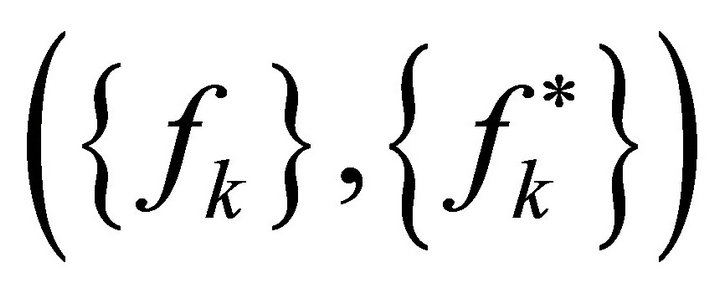be a -reconstruction system for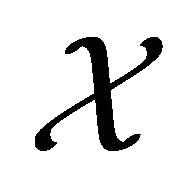. Then, there exists

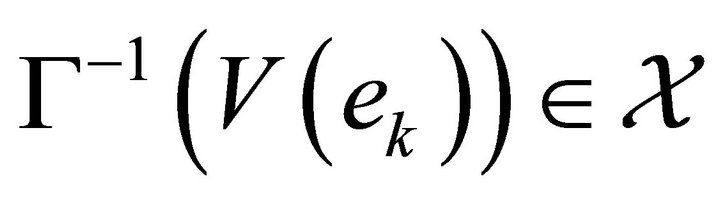such that 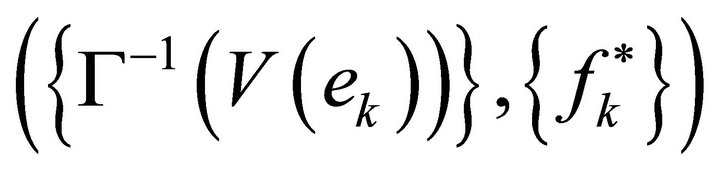is a

-reconstruction system for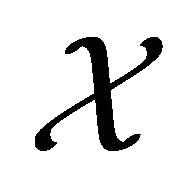, where and 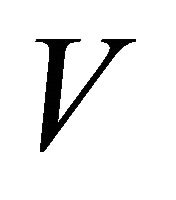are same as in above discussion.

4. Associated Banach Frames

Definition 4.1 Suppose that 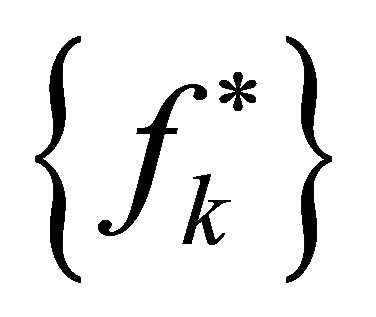has the reconstruction property for 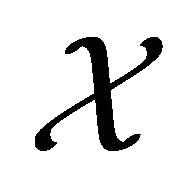with respect to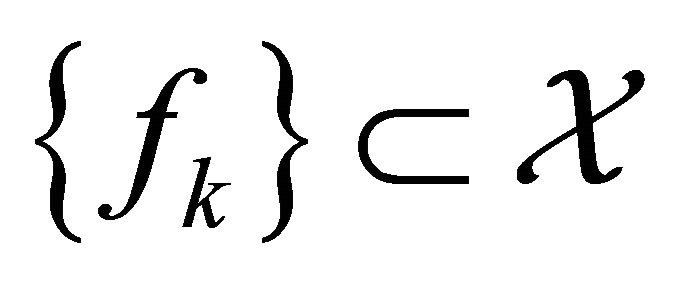. Then, there exists a reconstruction operator 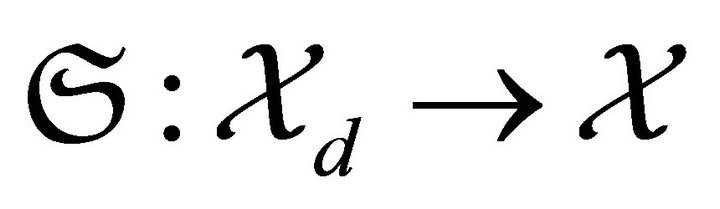such that 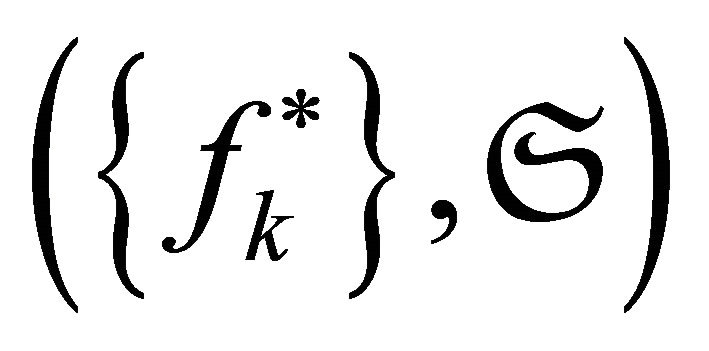is a Banach frame for 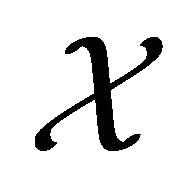with respect to some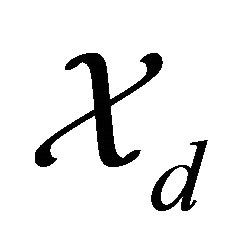. We say that 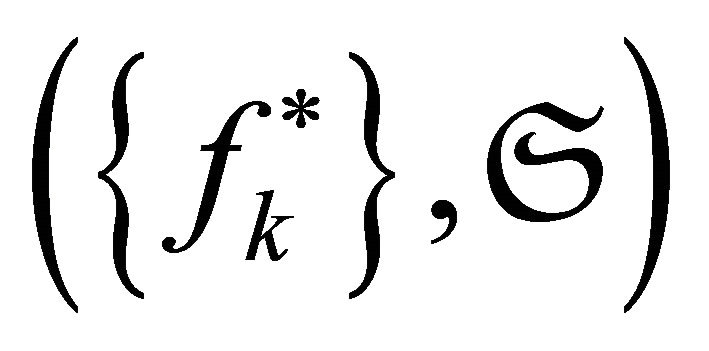is an associated Banach frame of.

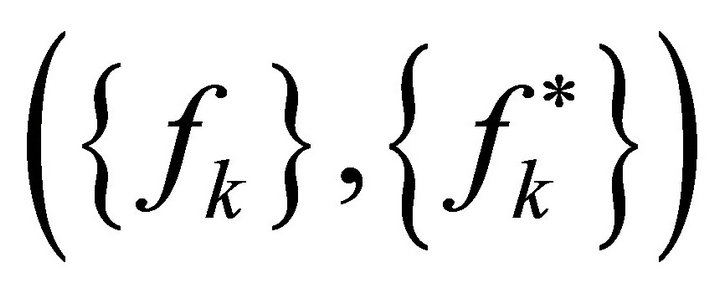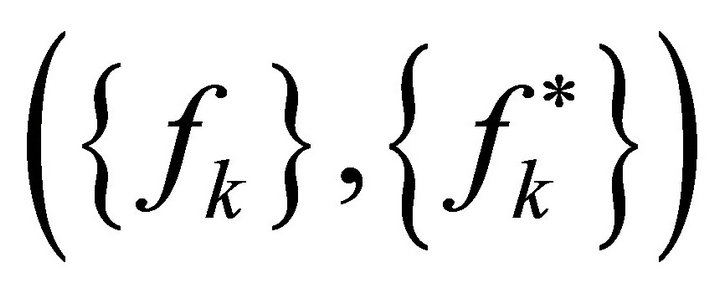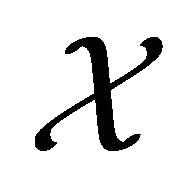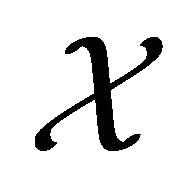Consider a reconstruction system for a Banach space. We can write each element of (we can reconstruct) by mean of an infinite series formed by 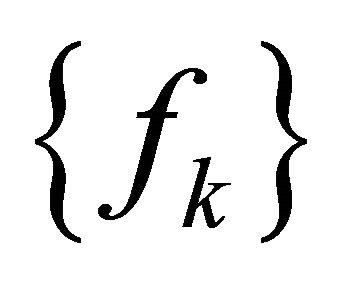over scalars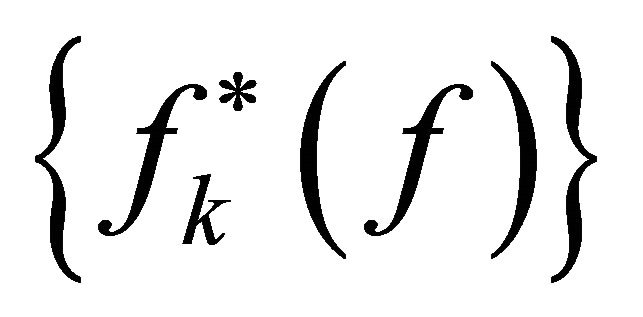. For a non zero functional 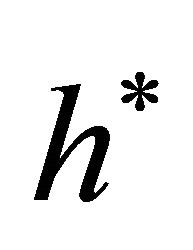(say), in general, there is

• no 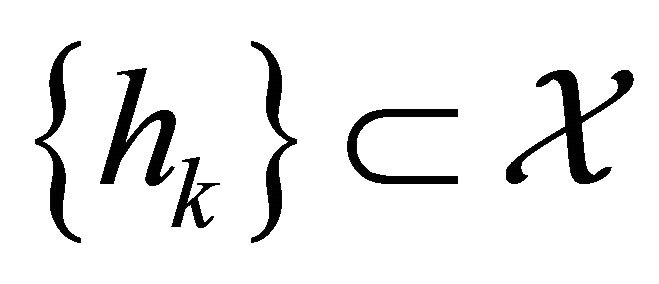such that 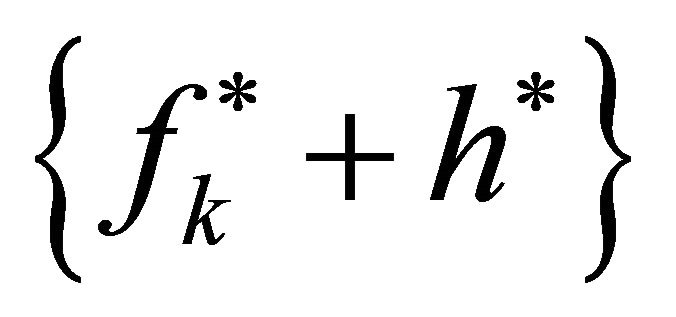has the reconstruction property for 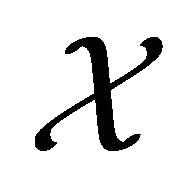with respect to.

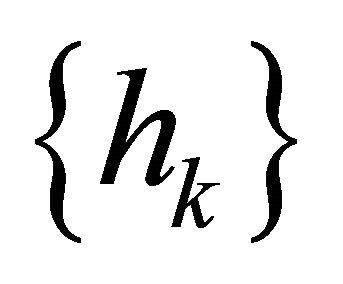• no reconstruction operator such that

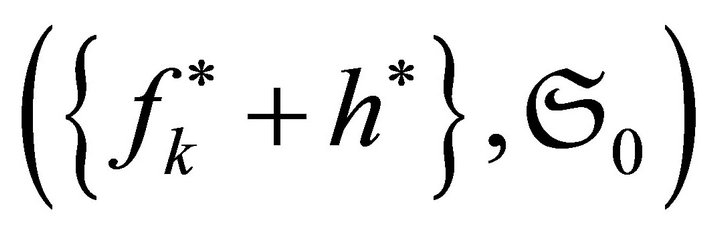is a Banach frame for.

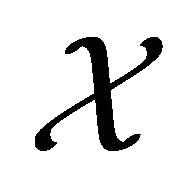More precisely, two natural and important problem arise, namely, existence of 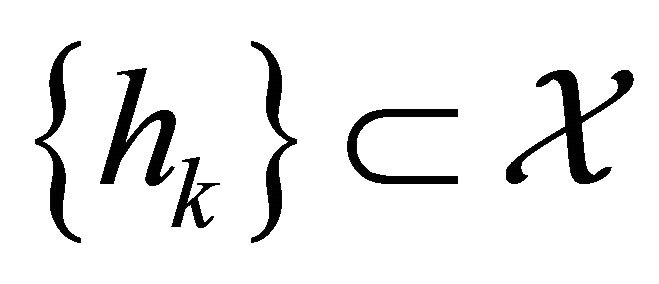such that

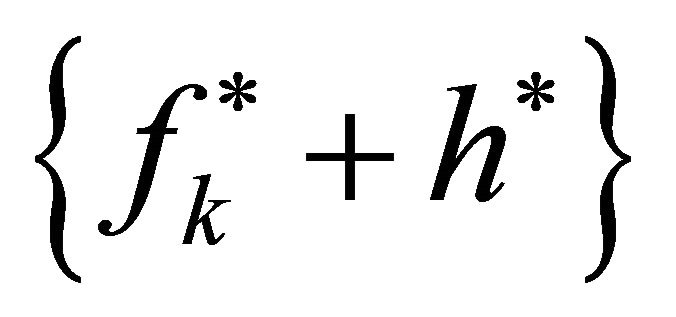has the reconstruction property for 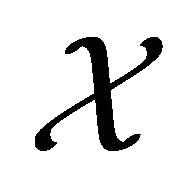with respect to 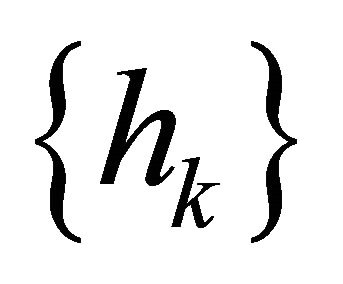and other is the existence of a reconstruction operator associated with. Cassaza and Christensen in [1] study some stability of reconstruction property in Banach spaces in terms of closeness of certain sequence to a given reconstruction system. In the present section we focus on pre-frame operator associated with.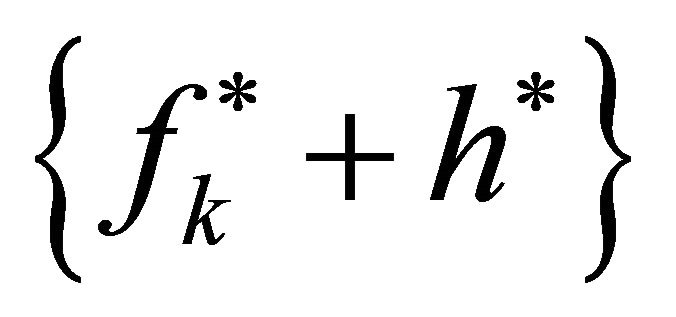

Motivation: Consider a signal space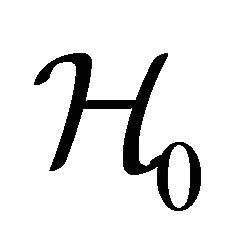. If 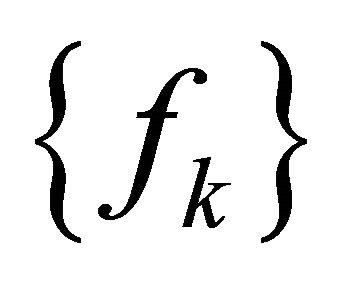is a frame (Hilbert) for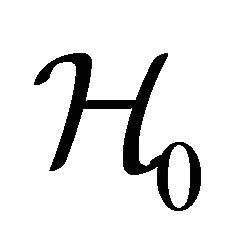, then each element of 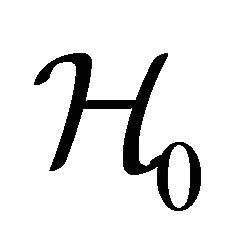can be recovered by an infinite combinations of frame elements. That is, by the reconstruction formula. If a signal f is transmitted to a receiver, then there are some kind of disturbances in the received signal. To overcome these disturbances from the receiver, frames plays an important role. Actually, a signal in the space (after its transmission) is in the form of the frame coefficients

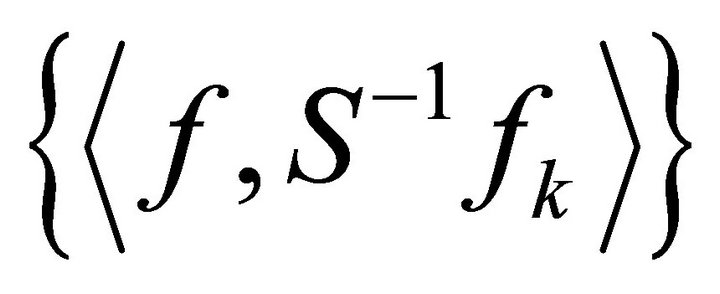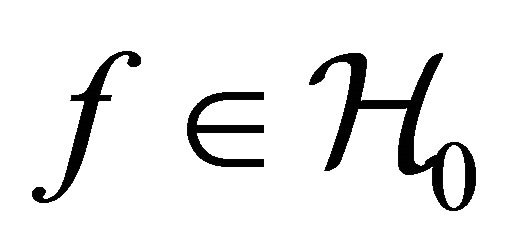,. An error 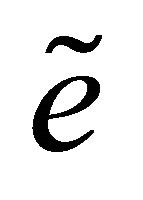is always is expected with concern signal in the space. That is, actual signal in the space is of the form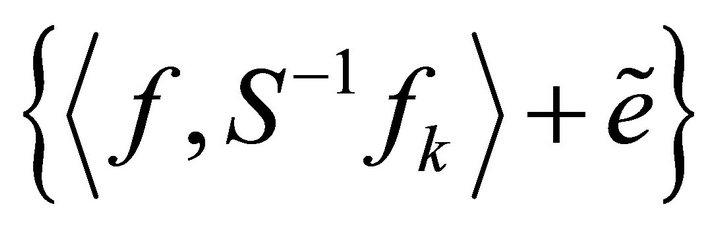, where 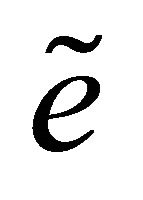is an error associated with f. An interesting discussion in this direction is given in [13]. We extend the said problem to Banach frames in general Banach spaces.

The following proposition provides sufficient condition for a reconstruction system to satisfy property 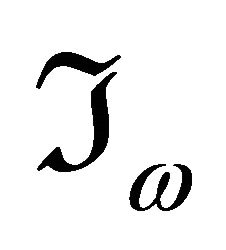in terms of non-existence of pre-frame operator associated with certain error.

Proposition 4.2 Suppose that 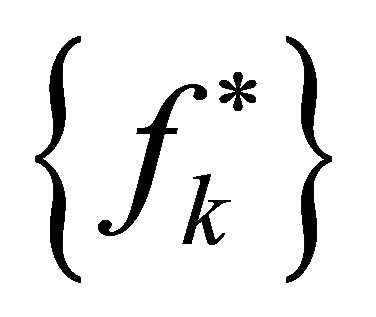has the reconstruction property for a signal space (Banach) 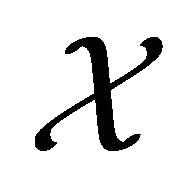with respect to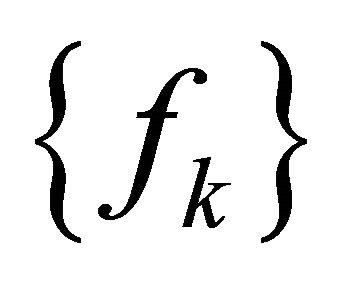. Let 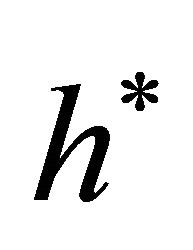(error) be in 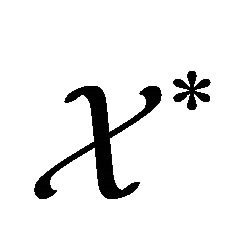for which there is no pre-frame operator such that

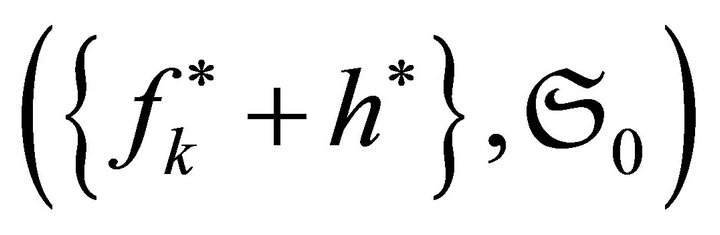is a Banach frame for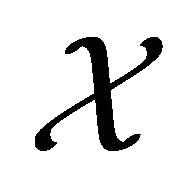, then

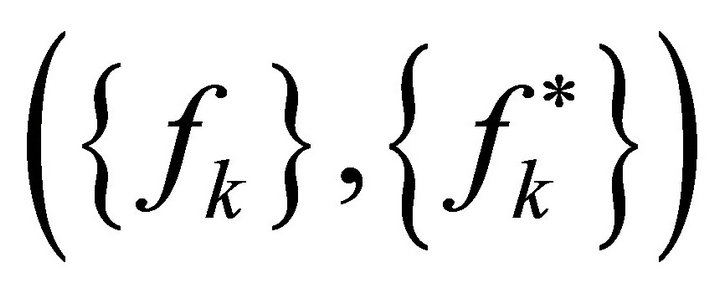is a 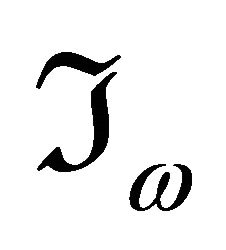-reconstruction system for.

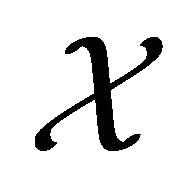Proof. Let 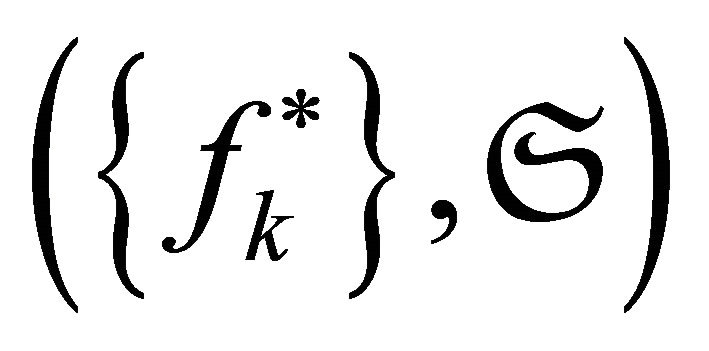be an associated Banach frame of. If there exists no pre-frame operator

such that 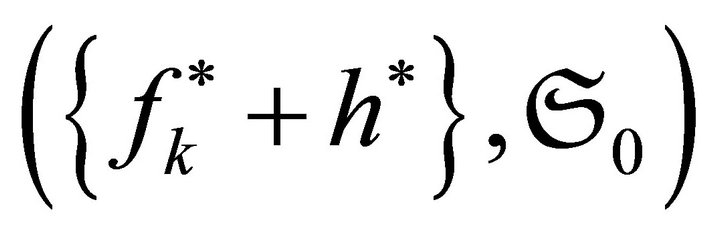is a Banach frame for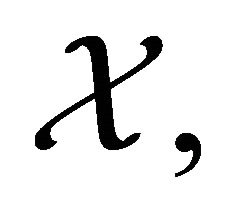

then, there is a non-zero vector 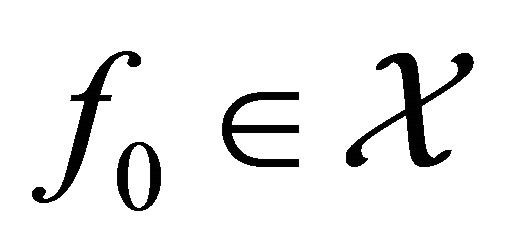such that

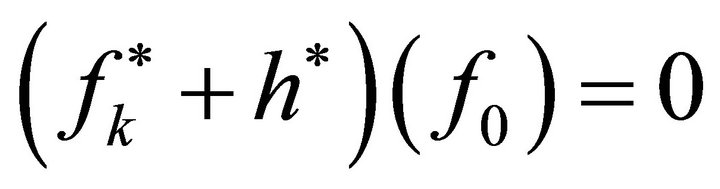, for all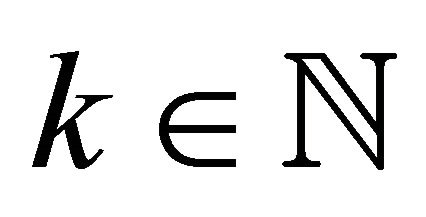. By frame inequality of

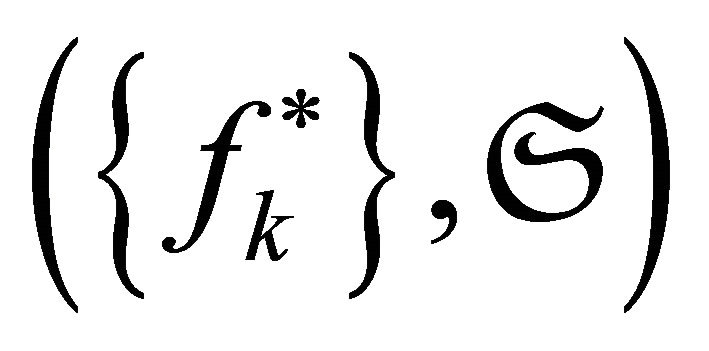, we conclude that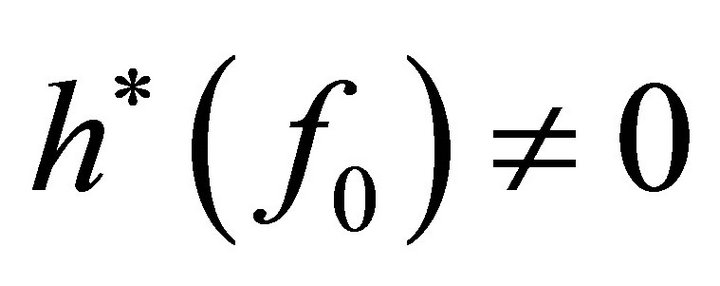. Put

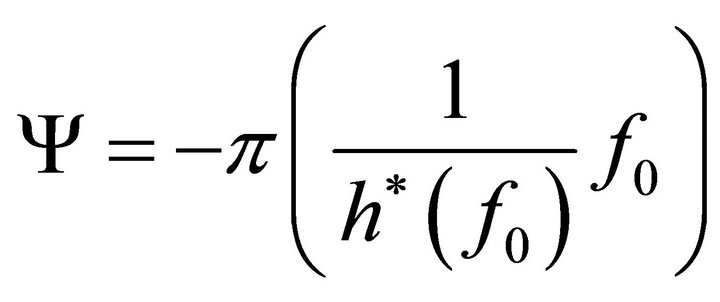. Then, 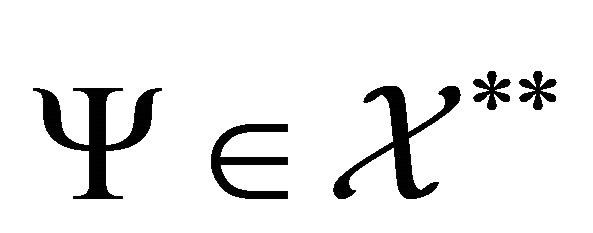is such that

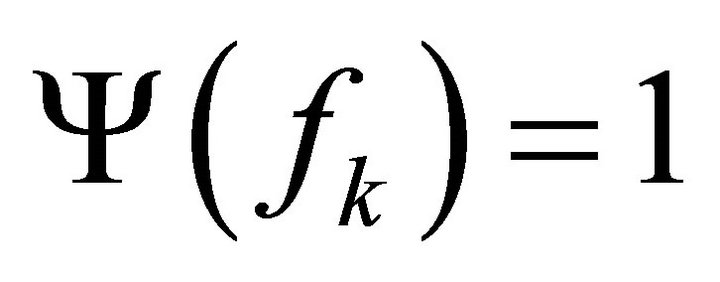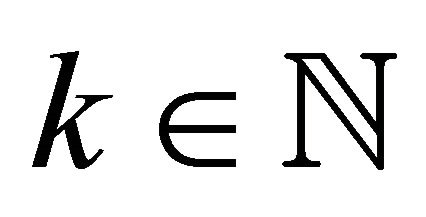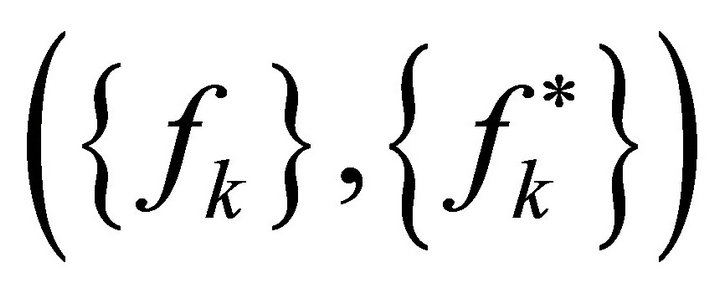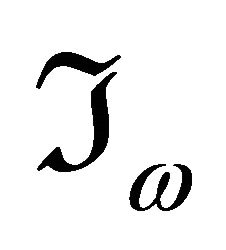, for all. Hence is a -reconstruction system for.

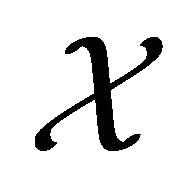Remark 4.3 The condition in Proposition 4.2 is not necessary unless correspond to a vector in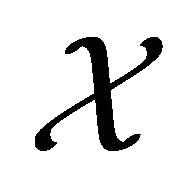. More precisely, we can find a certain error 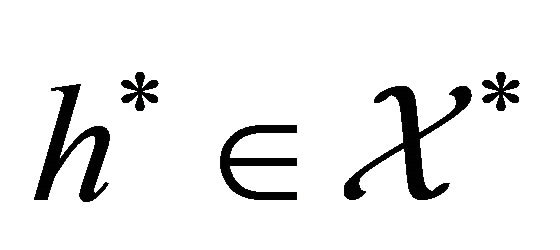such that there exists no pre-frame operator associated with 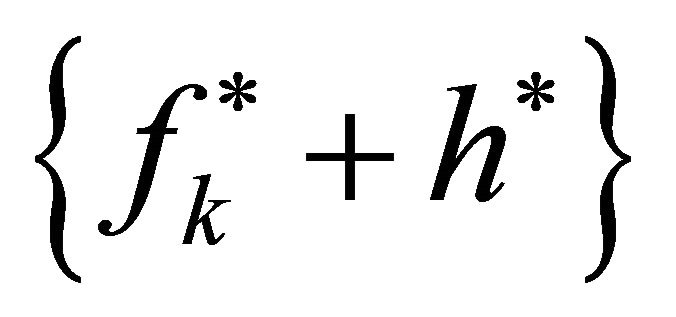provided.

Remark 4.4 Let us continue with the outcomes in Proposition 4.2, where 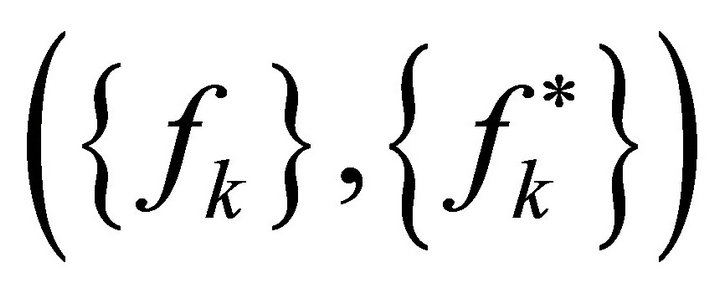is found to be a

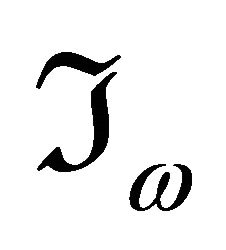-reconstruction system for 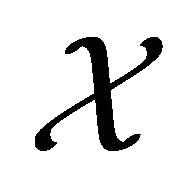provided there is no pre-frame operator such that 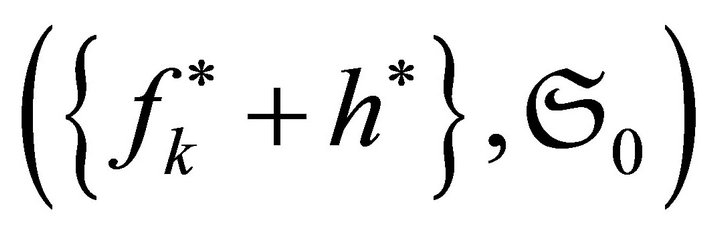is a Banach frame for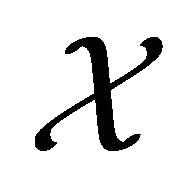, where 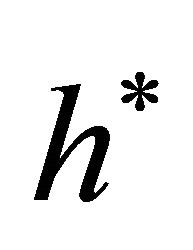is certain choice of error (functional). A natural problem arises, which is of determining a Banach space for which the system

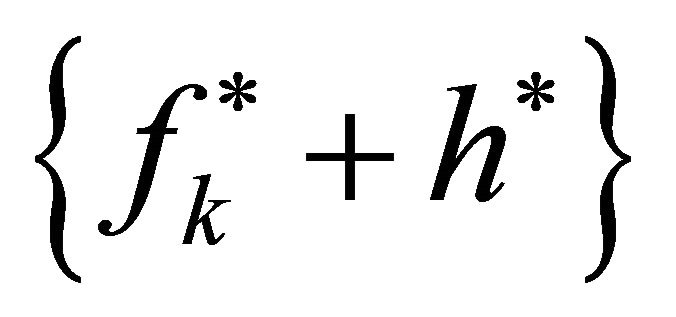admits a pre-frame operator. Answer to this problem is positive, provided 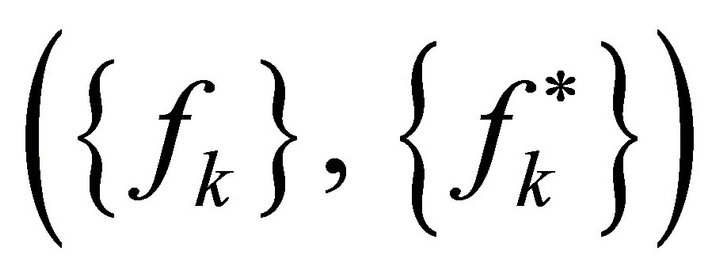is preshrinking. The outline of construction of such a Banach space can be understood as follows: Put

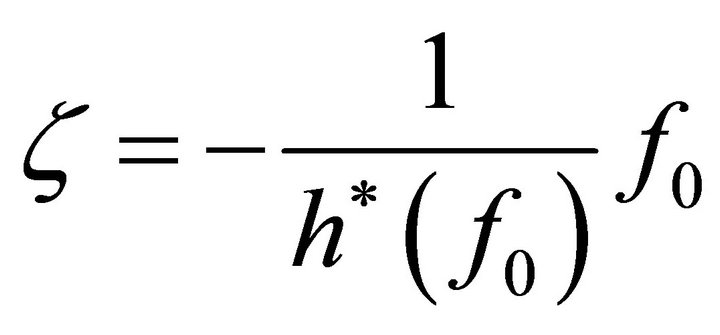(where 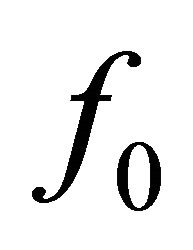is same as in the proof of Proposition 4.2). Now, there is no pre-frame operator

associated with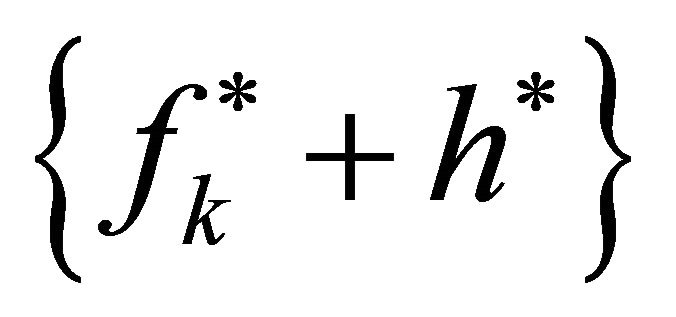, so there exists a nonzero vector such that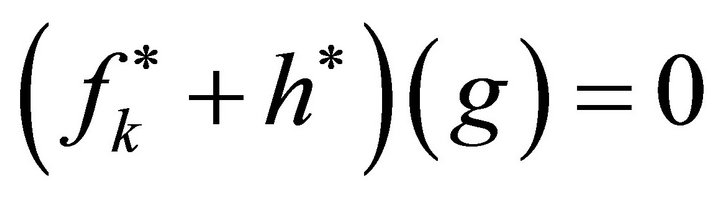, for all

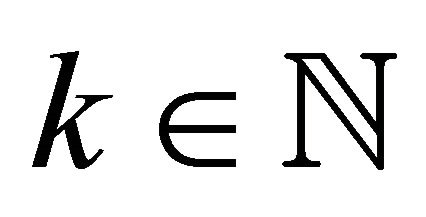. By using frame inequality of the associated Banach frame 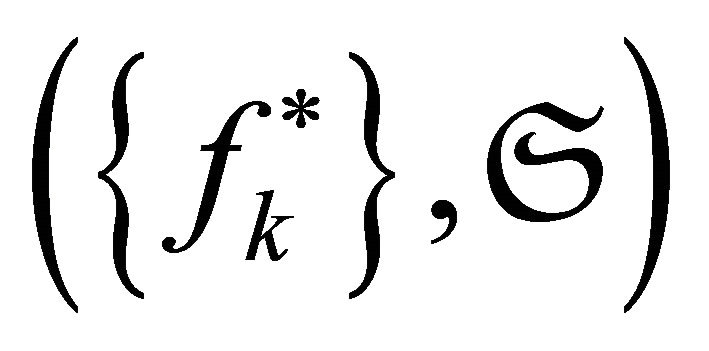we have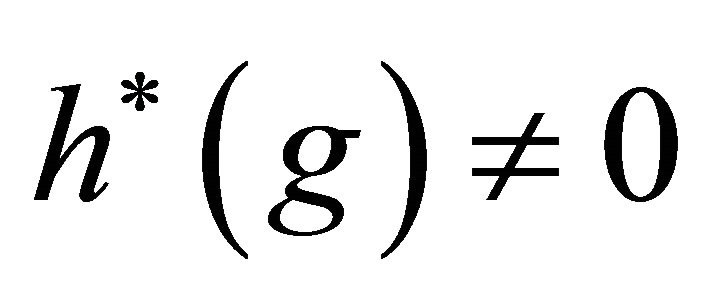. Put

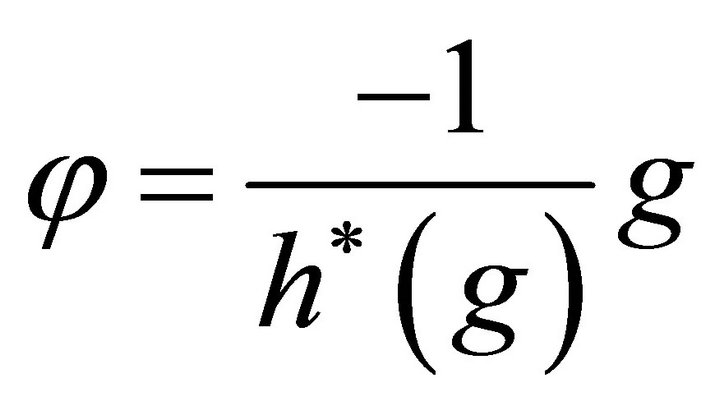. Then, is a non-zero vector in 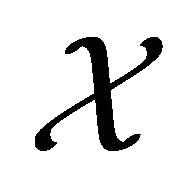such that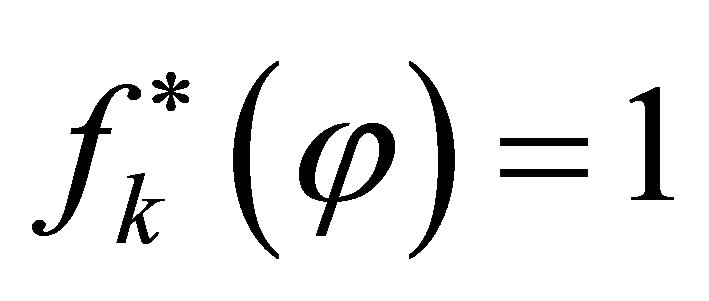, for all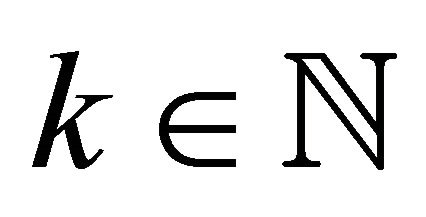. Therefore,

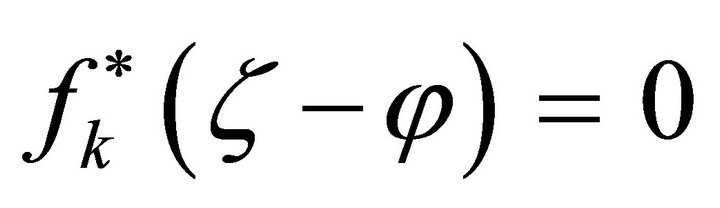for all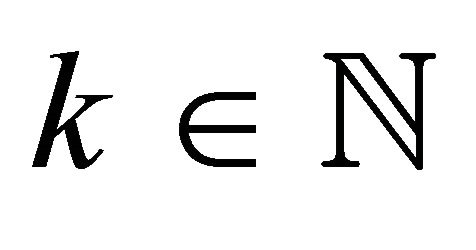. Now 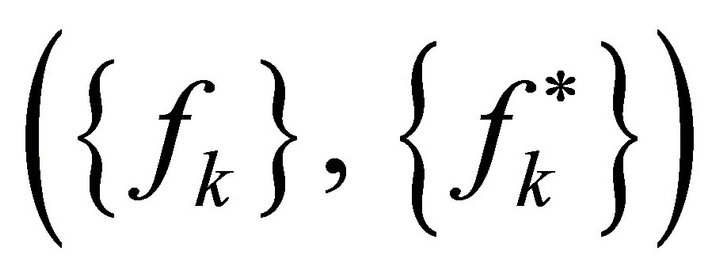is pre-shrinking, so we have. Hence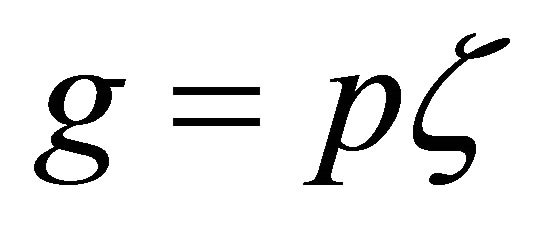, where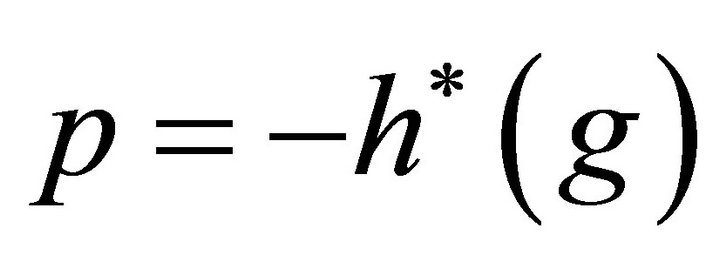. By using Lemma 2.3 there exists a pre-frame operator such that 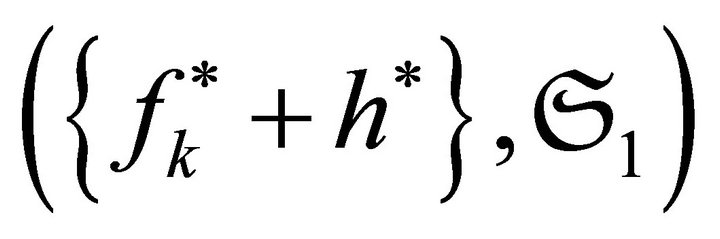is a Banach frame(normalized tight) for the Banach space , where;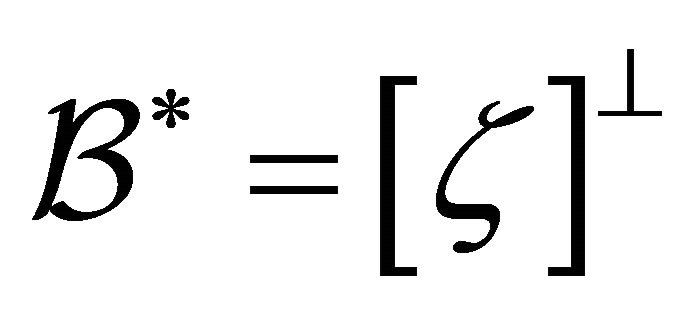

.

An application of Proposition 4.2 is given below:

Example 4.5 Let 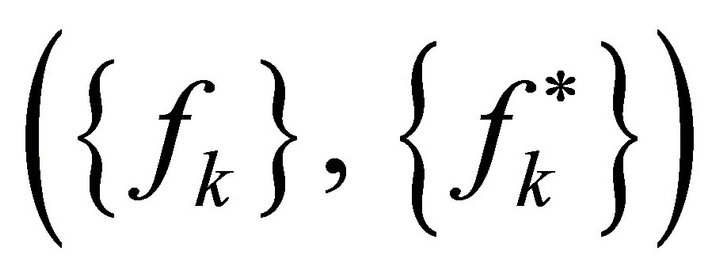be a reconstruction system given in Example 3.4 for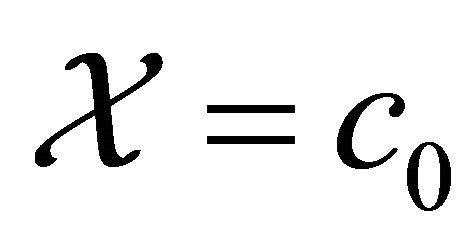. Then,

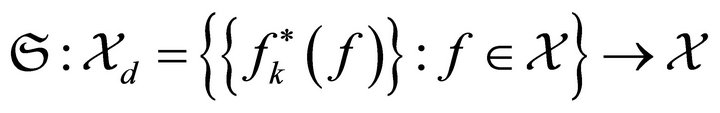is a bounded linear operator such that 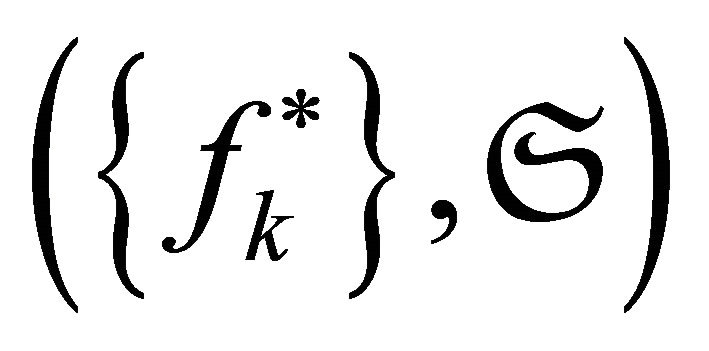is a Banach frame (associated) for 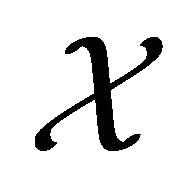with respect to 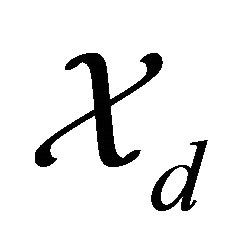and with bounds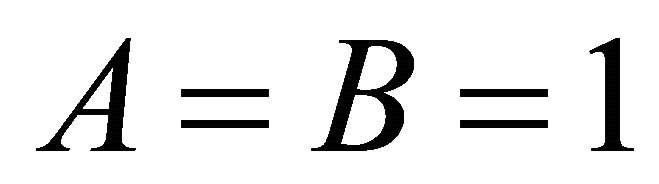. Put 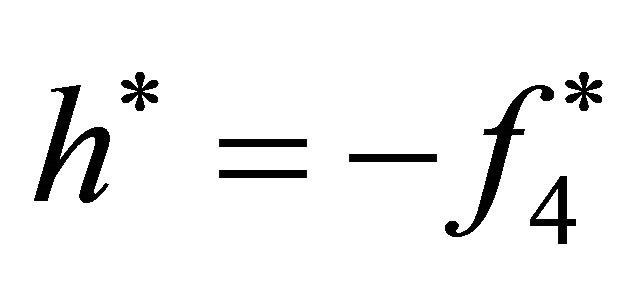(this choice makes sense, because disturbances are not constant!). Then, 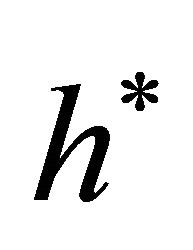is an error in for which there is no reconstruction operator such that 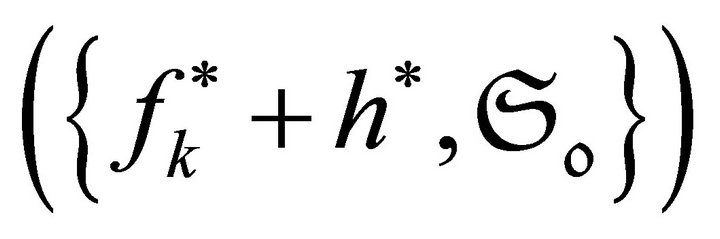is a Banach frame for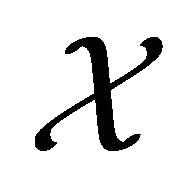. Hence by Proposition 4.2, 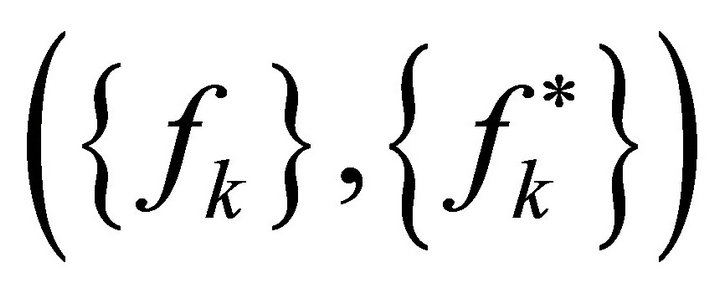is a

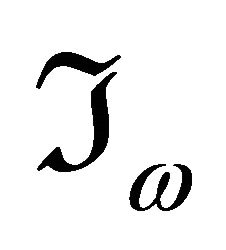-reconstruction system for.

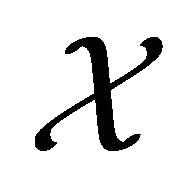Definition 4.6 Fix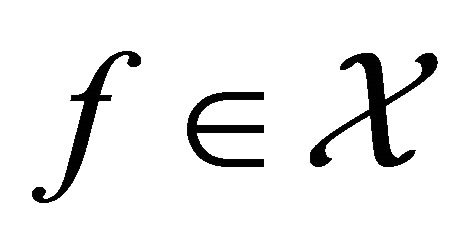. A pair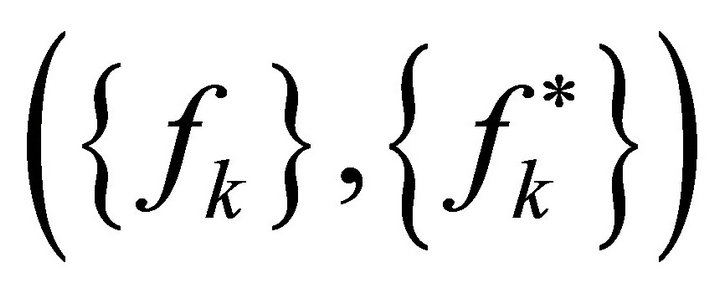, (where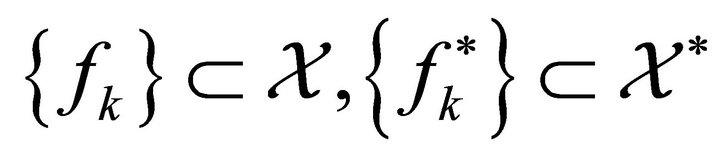) is said to be localized at, if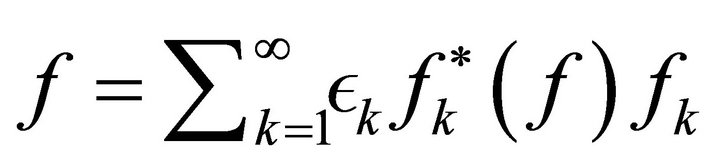, where 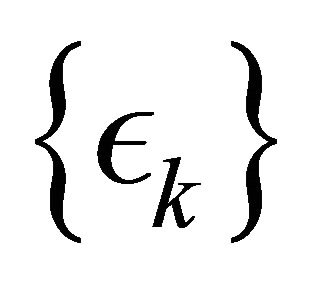is a sequence of scalars.

If 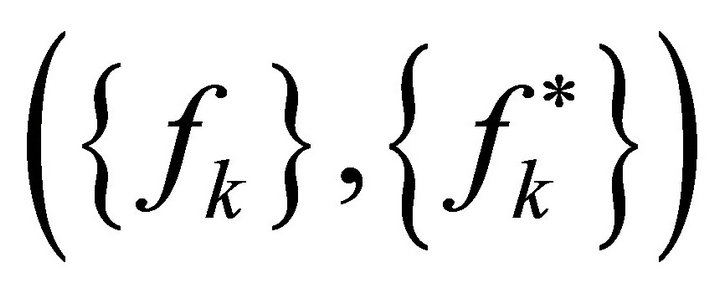is localized at every 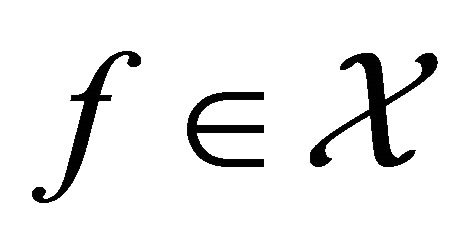with

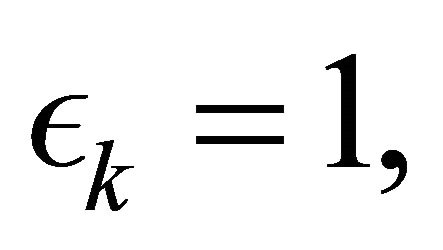for all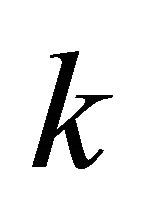, then 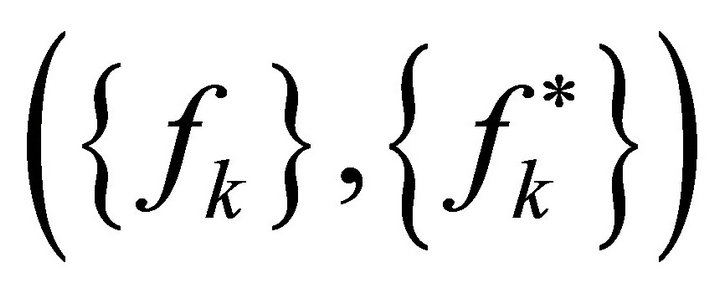turns out to be a reconstruction system for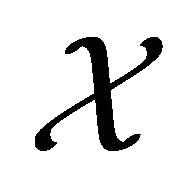. Consider a reconstruction system 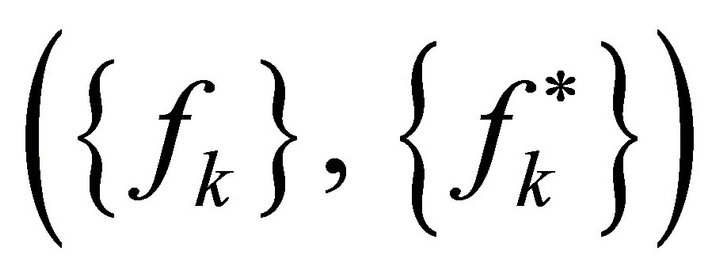for and 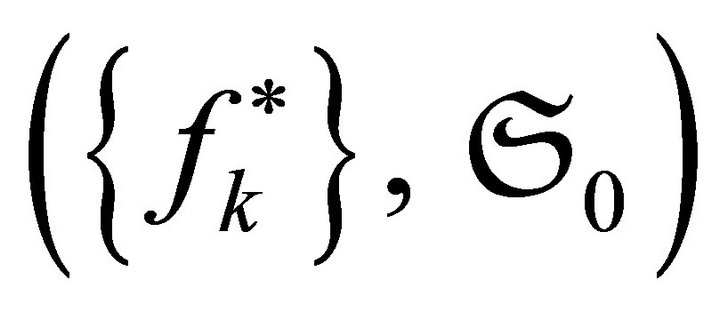be its associated Banach frame with respect to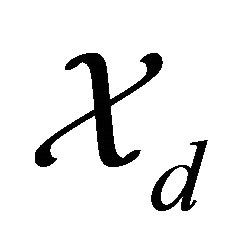. Let

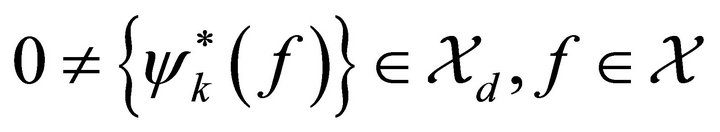. Then, in general, there is no pre-frame operator 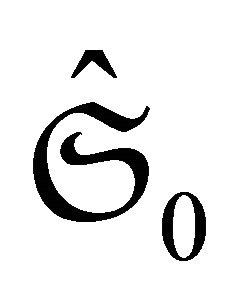associated with system

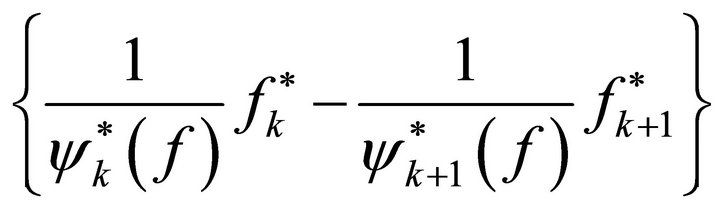. This problem is also known as stability of 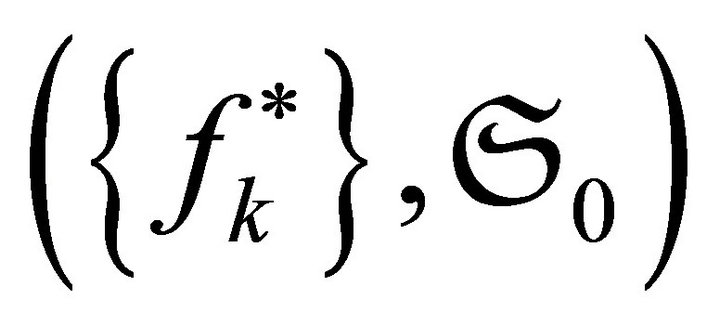with respect to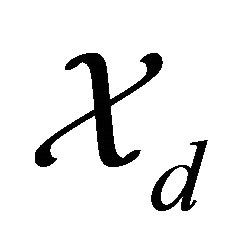. If

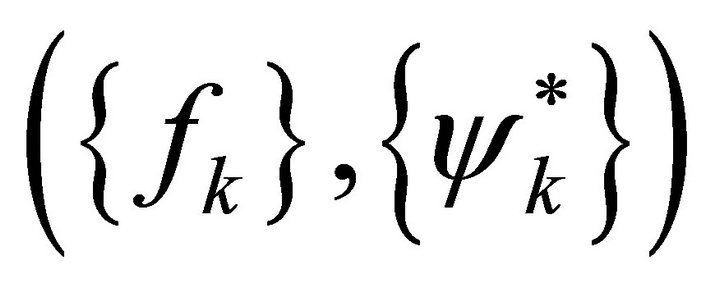is not localized at certain vectors in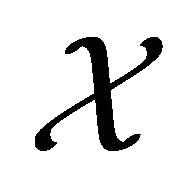then we can find such pre-frame operator associated with

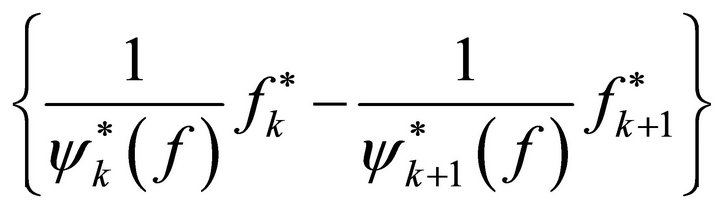. This is what concluding proposition of this paper says.

Proposition 4.7 Let 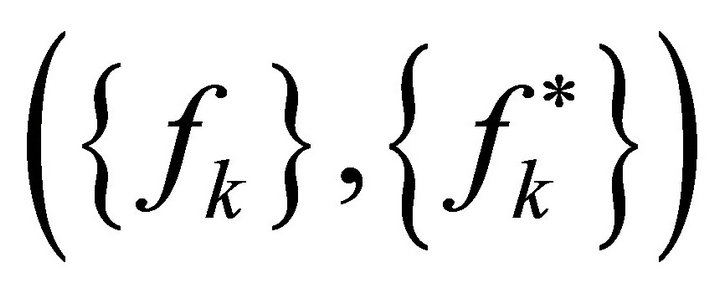be a reconstruction system for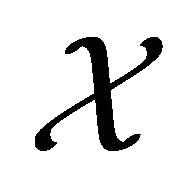. Assume that 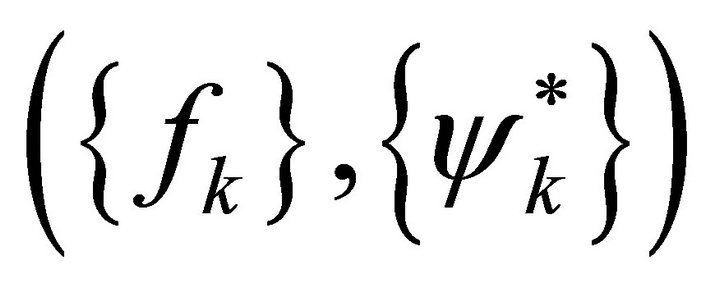is not localized at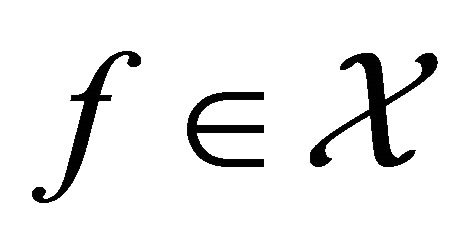, where .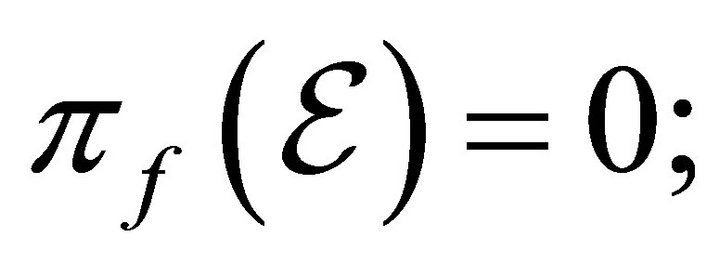

Then, there exists a pre-frame operator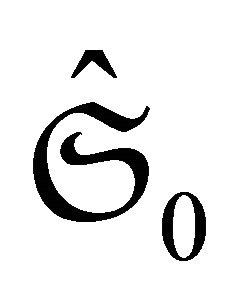, such that

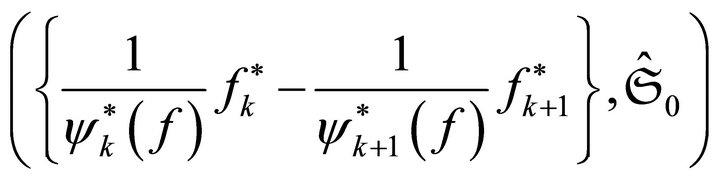is a Banach frame for.

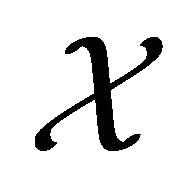Proof. Let 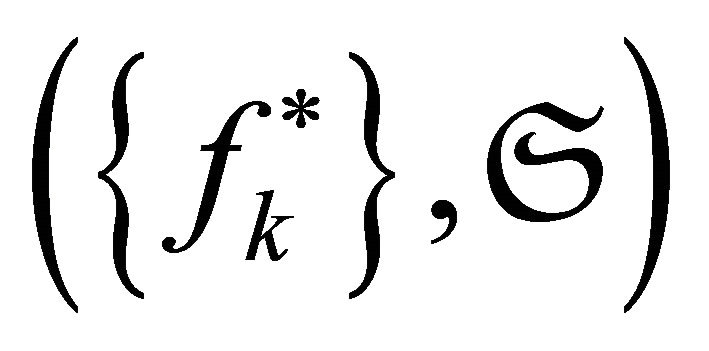be associated Banach frame of

. Let, if possible, there is no reconstruction operator, such that

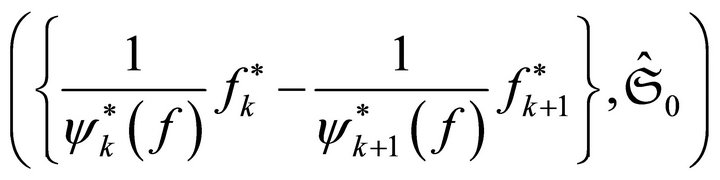is a Banach frame for. Then, there exists a non zero vector such that 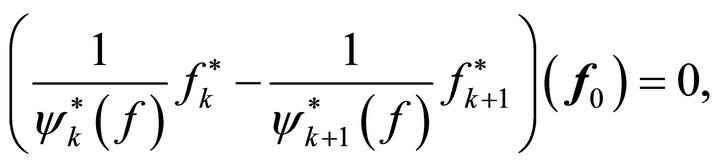for all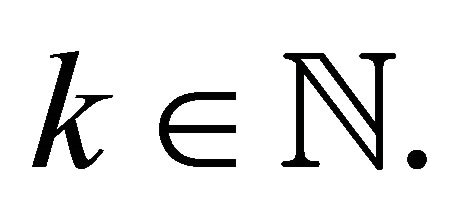

This gives

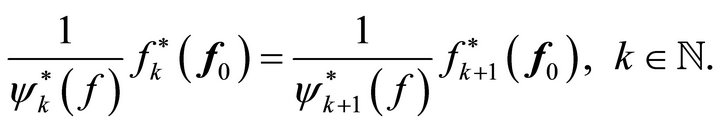
By using frame inequality of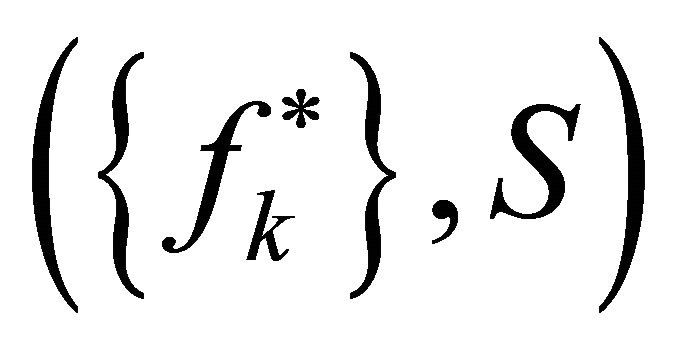, we obtain,

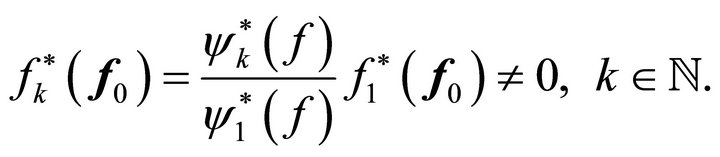
Since 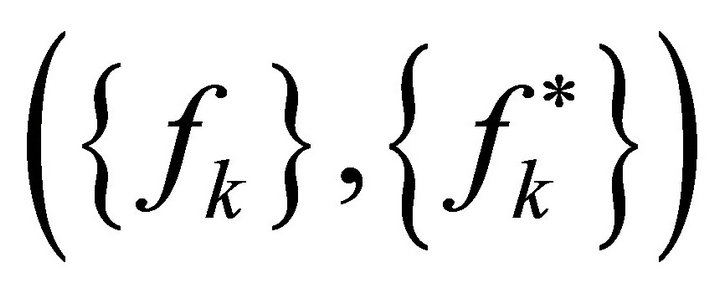is a reconstruction system for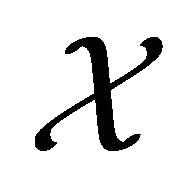, we have

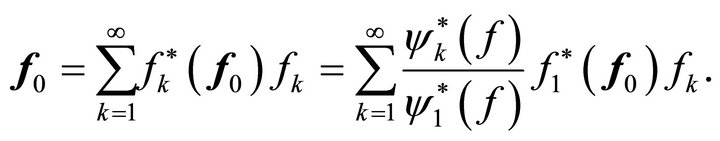
Thus, 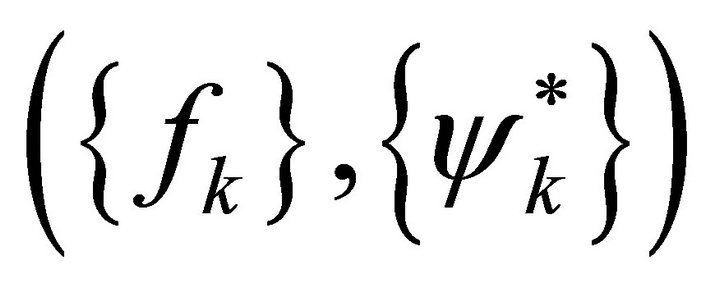is localized at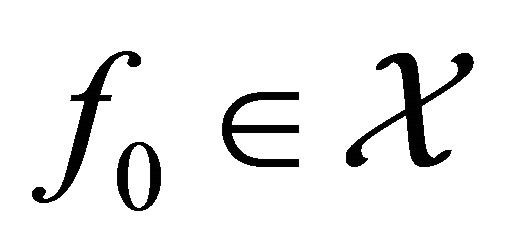, where

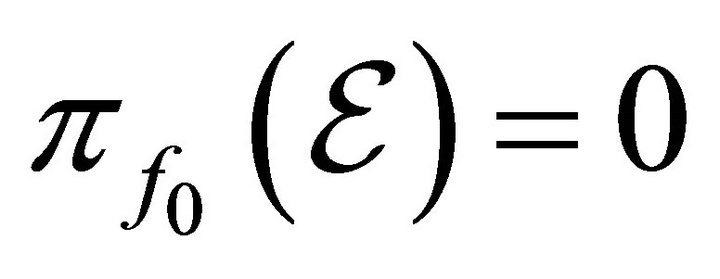, a contradiction. Hence there exists a preframe operator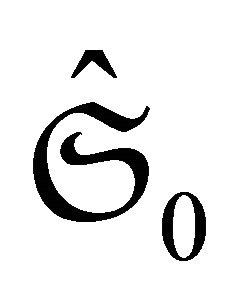, such that

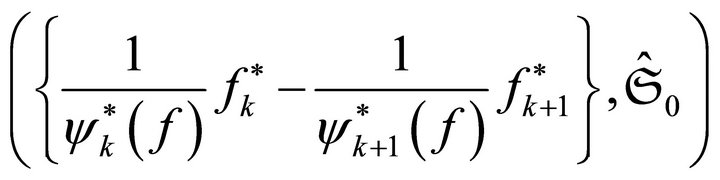is a Banach frame for.

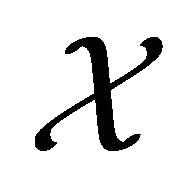5. Conclusion

The notion of -reconstruction property is proposed in section 3 and its characterization in terms of frames in Banach spaces is given. More precisely, Proposition 3.5 characterize -reconstruction property in terms of existence of pre-frame operator but in a contrapositive way. This situation is same as in electrodynamics, where there is a game of movement of electron but charge given to electron is negative! Moreover, the action of a functional from 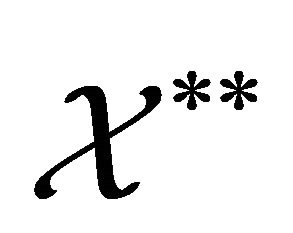on a given system from 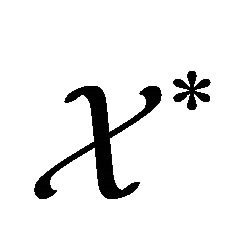decide the existence of pre-frame operator associated with certain system. This looks like dynamics of reconstruction property. By motivation from the theory of frames for Hilbert spaces which control the perturbed system associated with a signal in space(after its transmission), we extend the said situation to Banach spaces. More precisely, Proposition 4.2 control the situation in abstract setting via non-existence of pre-frame operator. Finally, the notion of local reconstruction system is proposed and its utility in complicated stability of associated Banach frames is reflected in Proposition 4.7.

REFERENCES