World Journal of Condensed Matter Physics
Vol.4 No.2(2014), Article ID:45665,8 pages DOI:10.4236/wjcmp.2014.42010
Transport Properties of Superfluid Dipolar Fermi Gas at Low Temperatures
Maryam Khademi Dehkordi
Falavarjan Branch, Islamic Azad University, Isfahan, Iran
Email: khademi@iaufala.ac.ir
Copyright © 2014 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 2 January 2014; revised 3 March 2014; accepted 21 March 2014
ABSTRACT
Transport coefficients of superfluid dipolar Fermi gas are calculated by using the Boltzmann equation approach. The interaction between Bogoliubov-Bogoliubov quasiparticles in the collision integral is considered in binary process. The shear viscosities  ;
; ; and
; and ![]() are proportional to
are proportional to ;
;![]() ; and
; and  , respectively. Also, we have found the elements of the diffusive thermal conductivities
, respectively. Also, we have found the elements of the diffusive thermal conductivities  with temperature dependency
with temperature dependency  and
and  which is proportional to
which is proportional to  and other components which are zero.
and other components which are zero.
Keywords:Shear Viscosity, Diffusive Thermal Conductivity, Superfluid Dipolar Fermi Gas

1. Introduction
Fermi gases of dipolar particles present a different physical picture. Being magnetically polarized, these particles interact via anisotropic dipole-dipole forces. The potential dipole-dipole interaction is partially repulsive and partially attractive. These features of the dipole-dipole interaction have very important consequences not only for the scattering properties of the particles in ultra cold gas, but also for the stability of the system and for the variety of its properties. The recent success in observing quantum degeneracy in ultra cold atomic Fermi gas [1] -[4] stimulates a search for gaseous Fermi systems with an achievable temperature of super fluid phase transition, which is generally very low.
Fermionic superfluidity was obtained in 2003 and allowed for the exploration of BEC-BCS crossover physics, a theoretical scenario bridging the gap between the Bardeen-Cooper-Schrieffer mean-field theory describing the behavior of weakly attracting fermions (scattering length a small and negative), and the strongly attractive regime (a small and positive) where the system behaves as a Bose-Einstein condensate of tightly bound dimers. Remarkably, although the theoretical foundations of this crossover physics had been laid in the early 1980s by the pioneering works of Nozières, Schmitt-Rink and Leggett [5] [6] , its first experimental confirmation was only made possible by the possibility of tuning interactions in ultra-cold atom vapors using Fano-Feshbach resonances [7] -[8] .
Braby et al. [9] have computed the thermal conductivity and sound attenuation length of a dilute atomic Fermi gas in the framework of kinetic theory. Above the critical temperature for superfluidity,  , the quasiparticles are fermions, whereas below
, the quasiparticles are fermions, whereas below , the dominant excitations are phonons. They have calculated the thermal conductivity in both cases. They have found that at unitarity the thermal conductivity
, the dominant excitations are phonons. They have calculated the thermal conductivity in both cases. They have found that at unitarity the thermal conductivity ![]() in the normal phase scales as
in the normal phase scales as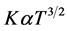 .In the superfluid phase they have found
.In the superfluid phase they have found .
.
The shear viscosity and the second viscosity of superfluid  have been formulated in the scope of Boltzmann equation approach [10] [11] . The value of the shear viscosity decreases as
have been formulated in the scope of Boltzmann equation approach [10] [11] . The value of the shear viscosity decreases as  with temperature; and the second viscosity of superfluid
with temperature; and the second viscosity of superfluid  is temperature independent in the limit of low temperatures, and in the near transition temperature behaves as inverse of the gap temperature. Theoretical study show that a gas of spin-polarized atomic
is temperature independent in the limit of low temperatures, and in the near transition temperature behaves as inverse of the gap temperature. Theoretical study show that a gas of spin-polarized atomic 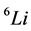 becomes superfluid at densities and temperatures comparable with those at which the Bose-Einstein experiments are performed [12] .
becomes superfluid at densities and temperatures comparable with those at which the Bose-Einstein experiments are performed [12] .
The shear viscosity tensor of the ![]() -phase of superfluid
-phase of superfluid  is calculated at low temperatures and melting pressure, by using the Boltzmann equation approach. They obtained that the elements of the shear viscosities
is calculated at low temperatures and melting pressure, by using the Boltzmann equation approach. They obtained that the elements of the shear viscosities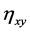 ,
,  and
and  are proportional to
are proportional to 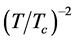 [13] [14] . Shahzamanian [15] calculated the components of the diffusive thermal conductivity of superfluid 3He-A using approximate collision integrals at low temperatures. The results are
[13] [14] . Shahzamanian [15] calculated the components of the diffusive thermal conductivity of superfluid 3He-A using approximate collision integrals at low temperatures. The results are 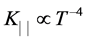 and
and .
.
Transport coefficients of the superfluid p-wave Fermi gas with weak interaction were calculated using the Boltzmann equation approach and the quasiparticle relaxation rate [16] . They have found that the superfluid Fermi gases under their considerations are similar to .
.
Diffusive thermal conductivity tensor of p-wave superfluid was calculated using the Boltzmann equation approach at low temperatures [17] . They have shown that their results are in good agreement with ![]() -phases of superfluid
-phases of superfluid  at low temperatures [18] .
at low temperatures [18] .
Here we present a calculation of the transport properties of a superfluid dipolar Fermi gas, based on the derivations of Abrikosov and Khalatnikov [19] (shear viscosity and diffusive thermal conductivity). We used a sufficiently high magnetic field so that all quasiparticles with spin up will go to superfluid state. Important collision processes are the binary scattering of quasiparticles, the decay processes in which one Bogoliubov quasi particle decays into three and coalescence processes in which three Bogoliubov quasi particles coalesce to produce one. We obtained the transition probabilities at low temperature. By using the Boltzmann equation, we calculated shear viscosity and diffusive thermal conductivity tensors.
2. Transition Probabilities
The interaction between quasiparticles in the superfluid dipolar Fermi gas was found by performing a Bogoliubov transformation on the normal state interaction:
 (1)
(1)
The Hamiltonian interaction contains the terms ,
,  ,
,  ,
,  and
and . The last two processes are not allowed because in each process the total energy should be conserved.
. The last two processes are not allowed because in each process the total energy should be conserved.
At low temperatures Bogoliubov coefficients can be written as  and
and , because more particles are gathered in the nodes of gap, that gap in direction
, because more particles are gathered in the nodes of gap, that gap in direction ![]() on the Fermi surface is
on the Fermi surface is![]() , where
, where  is the maximum gap and
is the maximum gap and  is the angle between the quasiparticle momentum and gap axis
is the angle between the quasiparticle momentum and gap axis , which is supposed to be in the direction of z-axis, in this region of temperature
, which is supposed to be in the direction of z-axis, in this region of temperature ![]() [20] . Using the bogoliubov transformation, transition probabilities in polarized Fermi gas are:
[20] . Using the bogoliubov transformation, transition probabilities in polarized Fermi gas are:
 (2)
(2)
The interparticle interaction in polarized dipolar gases that the dipoles are polarized along the z-axis reads,
 (3)
(3)
where  is magnetic dipole, and
is magnetic dipole, and ![]() is the angle between the momentum
is the angle between the momentum ![]() and the z-axis [21] . Choosing
and the z-axis [21] . Choosing ![]() along the z-axis,
along the z-axis,  is the angle between
is the angle between ![]() and
and![]() , and
, and ![]() is the angle between the plane of
is the angle between the plane of  with
with . The relations
. The relations  and
and 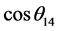 according to the momentum conservation are [22]
according to the momentum conservation are [22]
![]() (4)
(4)
![]() (5)
(5)
At low temperatures more particles are contributing in contacts about Fermi momentum [19] . By replacing Equations (3)-(5) into (2) and considering the fact that the size of momentums are equal to Fermi momentum and by neglecting the potential effects of trap in Fermi momentum, transition probability is obtained in the form below:
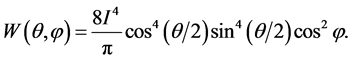 (6)
(6)
3. Collision Integral
The Boltzmann transport equation reads
 (7)
(7)
where  is the collision integral. The full distribution function is given by
is the collision integral. The full distribution function is given by . where
. where  and
and  is a small departure from equilibrium. We wrote the deviation from equilibrium as:
is a small departure from equilibrium. We wrote the deviation from equilibrium as:
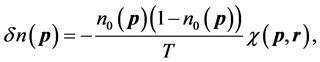 (8)
(8)
by using (8) in (7) we got [23]
 (9)
(9)
The collision integral is form [23]
 (10)
(10)
here ,
,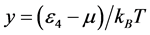 ,
,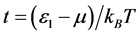 .
.
We expressed 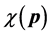 as a series of spherical harmonics in the polar angles
as a series of spherical harmonics in the polar angles ,
, . Thus
. Thus
 (11)
(11)
by inserting (11) in (10) and integrate over y we have
 (12)
(12)
where
 (13)
(13)
We divided ![]() in to even and odd functions of
in to even and odd functions of , and in the same way separated
, and in the same way separated![]() . Since
. Since , only the even part of
, only the even part of ![]() contributes to the even part of
contributes to the even part of , and similarly for the odd part [24] .
, and similarly for the odd part [24] .
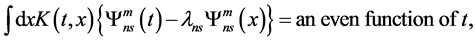 (14)
(14)
 (15)
(15)
that an even function of ![]() used for calculating of shear viscosity and odd function used for thermal conductivity [24] .
used for calculating of shear viscosity and odd function used for thermal conductivity [24] .
4. Shear Viscosity
The coefficients shear viscosity describes the response of the momentum current density to a transverse velocity field
 (16)
(16)
 is expressed in terms of the bogoliubov qusipartcle distribution function
is expressed in terms of the bogoliubov qusipartcle distribution function  by
by
 (17)
(17)
to solve the linearized Boltzmann equation, it is suitable to define 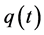 as
as
 (18)
(18)
By substituting (18) in (9) and then in (17) and compared with (16), we got [24]
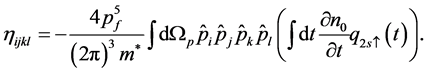 (19)
(19)
By solving the even part of  in (15) and with regard to the fact that system is polarized, we have [24] ,
in (15) and with regard to the fact that system is polarized, we have [24] ,
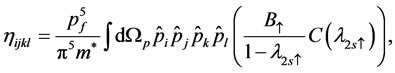 (20)
(20)
in this equation ,
,  and
and  are
are
 (21)
(21)
 (22)
(22)
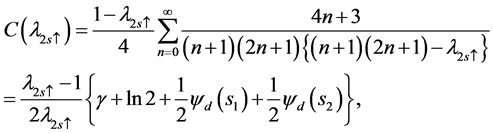 (23)
(23)
and  is Euler,s constant,
is Euler,s constant,  is a digamma function and
is a digamma function and
 ,
, (24)
(24)
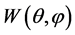 only replaces with
only replaces with , because
, because  and
and  are nearly zero.
are nearly zero.
For obtaining  and
and , we used (6) and note that
, we used (6) and note that ![]() is small for superfluid case and its maximum value is
is small for superfluid case and its maximum value is  [20] , where maximum gap,
[20] , where maximum gap,  , due to strong coupling effects is equal to
, due to strong coupling effects is equal to  [25] . Then, we obtained:
[25] . Then, we obtained:
 , (25)
, (25)
 (26)
(26)
 (27)
(27)
After substituting  ,
,  , and
, and 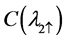 in (20) and taking the angular integrations, we have
in (20) and taking the angular integrations, we have
 (28)
(28)
 (29)
(29)
 (30)
(30)
 (31)
(31)
 (32)
(32)
5. Thermal Conductivity
The diffusive thermal conductivity tensor  is defined by
is defined by
 (33)
(33)
where ![]() is the diffusive heat current. It is noted that, in addition to heat transfer by a random diffusive process of thermal excitations described by Equations (33), there is a convective contribution to the heat current in a superfluid, even in the absence of mass flow, owing to the possibility of normal-superfluid counter flow.
is the diffusive heat current. It is noted that, in addition to heat transfer by a random diffusive process of thermal excitations described by Equations (33), there is a convective contribution to the heat current in a superfluid, even in the absence of mass flow, owing to the possibility of normal-superfluid counter flow.
At low temperatures, this contribution is negligible [24] -[26] and the measurement of the components of thermal conductivity at these temperatures is more meaningful than the measurement of the thermal conductivity components in the vicinity of .
.
The diffusive heat current may be written in terms of the polarized quasi-particle distribution functions:
 (34)
(34)
 is defined at below[26]
is defined at below[26]
 (35)
(35)
By using (35) in (34) and compared with (33) and using odd function of ![]() in (15) for polarized gas we got [24]
in (15) for polarized gas we got [24]
 (36)
(36)
By using Sykes and Brooker procedure we defined  as bellows
as bellows
 (37)
(37)
 and
and  are introduced as below
are introduced as below
 (38)
(38)
 (39)
(39)
where  and
and  are defined by (24).
are defined by (24).
By using (6) in (38), (39) we took the following explicit forms
 (40)
(40)
 (41)
(41)
By using the Sykes and Brooker procedure [24] , we calculated the diffiusive thermal conductivity tensor. After substituting ,
,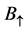 , and
, and  in (37), and taking the angular integrations, we got:
in (37), and taking the angular integrations, we got:
 (42)
(42)
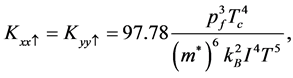 (43)
(43)
 (44)
(44)
6. Discussion and Concluding Remarks
As a result, in a superfluid dipolar Fermi gases we calculated the transition probabilities for the cases where the Bogoliubov quasiparticles are presented in coalescence, decay and binary processes and obtained that binary processes are dominated in calculation of transition probabilities, and other processes are nearly zero. Then, by using collision integral in the Boltzmann equation contained binary processes, the components of shear viscosity and thermal conductivity were calculated. We obtained ;
;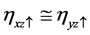 ; and
; and ![]() with temperatures dependences
with temperatures dependences ;
; ; and
; and  respectively (see Equations (28)-(32)); and we have found the components of the diffusive thermal conductivity,
respectively (see Equations (28)-(32)); and we have found the components of the diffusive thermal conductivity, 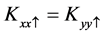 with temperature dependency
with temperature dependency  and
and  which is proportional to
which is proportional to 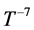 and other components which are zero. Temperature dependency of shear viscosity and thermal conductivity along the z-axis (
and other components which are zero. Temperature dependency of shear viscosity and thermal conductivity along the z-axis (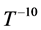 and
and ![]() respectively) were showing that the polarization is along the z-axis.
respectively) were showing that the polarization is along the z-axis.
Then, we had to compare our results in transport coefficients with [13] [14] . (They found the shear viscosities 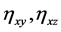 and
and  of A1-phase of superfluid 3He are proportional to (T/Tc)-2.). Two major differences were shown: a) Transition probabilities in superfluid dipolar Fermi gas are the function of temperature, but in superfluid 3He-A1 are constant. b) 3He-A1 have two kind of the quasiparticles, one for Bogoliubov quasiparticle, and another for normal quasiparticle. The wave function of the system in A1 phases of 3He is
of A1-phase of superfluid 3He are proportional to (T/Tc)-2.). Two major differences were shown: a) Transition probabilities in superfluid dipolar Fermi gas are the function of temperature, but in superfluid 3He-A1 are constant. b) 3He-A1 have two kind of the quasiparticles, one for Bogoliubov quasiparticle, and another for normal quasiparticle. The wave function of the system in A1 phases of 3He is 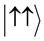 that interacts with Bogoliubov quasipaticle
that interacts with Bogoliubov quasipaticle  and normal quasiparticle
and normal quasiparticle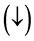 , but in superfluid dipolar Fermi gas only
, but in superfluid dipolar Fermi gas only  contributes to Bogoliubov quasiparticles
contributes to Bogoliubov quasiparticles  in interaction.
in interaction.
It is noted that, in superfluid Fermi gas in p-wave state ,
, 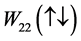 were contributed to calculations [16] [17] but in superfluid dipolar Fermi gas only
were contributed to calculations [16] [17] but in superfluid dipolar Fermi gas only  with temperature dependency contributes to calculations.
with temperature dependency contributes to calculations.
Also, in [15] the components of thermal conductivity tensor of superfluid 3He-A were calculated using approximate collision integrals at low temperatures, and the thermal coefficients 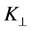 and
and ![]() have been obtained with temperature dependences
have been obtained with temperature dependences  and
and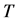 , respectively. This is due to the fact that
, respectively. This is due to the fact that 
 was taken to be a constant parameter with respect to temperatures.
was taken to be a constant parameter with respect to temperatures.
In [18] ,  ,
,  and
and  were contributed in calculations and the wave function
were contributed in calculations and the wave function  interacted with Bogoliubov and normal quasiparticles, but in superfluid dipolar gas only
interacted with Bogoliubov and normal quasiparticles, but in superfluid dipolar gas only  contributed to calculation and only
contributed to calculation and only  interacted with Bogoliubov quasiparticles. These differences in transition probabilities of
interacted with Bogoliubov quasiparticles. These differences in transition probabilities of  and superfluid Fermi gas in p-wave state with superfluid dipolar Fermi gas, have shown themselves in transport coefficients.
and superfluid Fermi gas in p-wave state with superfluid dipolar Fermi gas, have shown themselves in transport coefficients.
References
- deMarco, B. and Jin, D.S. (1999) Onset of Fermi Degeneracy in a Trapped Atomic Gas. Science, 285, 1703-1706. http://dx.doi.org/10.1126/science.285.5434.1703
- deMarco, B., Papp, S.B. and Jin, D.S. (2001) Pauli Blocking of Collisions in a Quantum Degenerate Atomic Fermi Gas. Physical Review Letters, 86, Article ID: 5409. http://dx.doi.org/10.1103/PhysRevLett.86.5409
- Truscott, A.G., Strecker, K.E., McAlexander, W.I., Partridge, G.B. and Hulet, R.G. (2001) Observation of Fermi Pressure in a Gas of Trapped Atoms. Science, 291, 2570-2572. http://dx.doi.org/10.1126/science.1059318
- Schreck, F., Khaykovich, L., Corwin, K.L., Ferrari, G., Bourdwel, T., Cubizolles, J. and Salomon, C. (2001) Quasipure Bose-Einstein Condensate Immersed in a Fermi Sea. Physical Review Letters, 87, Article ID: 080403. http://dx.doi.org/10.1103/PhysRevLett.87.080403
- Leggett, A.J. (1980) Cooper Pairing in Spin-Polarized Fermi Systems. Journal de Physique Archives, 41, 19-26. http://dx.doi.org/10.1051/jphyscol:1980704
- Nozières, P. and Schmitt-Rink, S. (1985) Bose Condensation in an Attractive Fermion Gas: From Weak to Strong Coupling Superconductivity. Journal of Low Temperature Physics, 59, 195-211. http://dx.doi.org/10.1007/BF00683774
- Jochim, S., Bartenstein, M., Altmeyer, A., Hendl, G., Riedl, S., Chin, C., Denschlag, J.H. and Grimm, R. (2003) BoseEinstein Condensation of Molecules. Science, 302, 2101-2103.
- Bourdel, T., Khaykovich, L., Cubizolles, J., Zhang, J., Chevy, F., Teichmann, M., Tarruell, L., Kokkelmans, S.J.J.M.F. and Salomon, C. (2004) Experimental Study of the BEC-BCS Crossover Region in Lithium 6. Physical Review Letters, 93, Article ID: 050401. http://dx.doi.org/10.1103/PhysRevLett.93.050401
- Braby, M., Jingyi, C. and Schäfer, T. (2010) Thermal Conductivity and Sound Attenuation in Dilute Atomic Fermi Gases. Physical Review A, 82, Article ID: 033619. http://dx.doi.org/10.1103/PhysRevA.82.033619
- Shahzamanian, M.A. and Yavary, H. (2002) The Shear Viscosity of Superfluid 6Li. Physica B, 321, 385-387. http://dx.doi.org/10.1016/S0921-4526(02)01080-3
- Shahzamanian, M.A. and Yavary, H. (2002) The Second Viscosity of Superfluid 6Li. International Journal of Modern Physics B, 16, 3797-3802. http://dx.doi.org/10.1142/S0217979202013092
- Stoof, H.T.C., Houbiers, M., Sacket, C.A. and Hulet, R.G. (1996) Superfluidity of Spin-Polarized 6Li. Physical Review Letters, 76, Article ID: 10. http://dx.doi.org/10.1103/PhysRevLett.76.10
- Shahzamanian, M.A. and Afzali, R. (2004) Shear Viscosity of Superfluid 3He-A1 at Low Temperature. Annals of Physics, 309, 281-294. http://dx.doi.org/10.1016/j.aop.2003.08.012
- Shahzamanian, M.A. and Afzali R. (2004) Quasiparticle Relaxation Rate and Shear Viscosity of Superfluid 3He-A1 at Low Temperatures. Physica B, 348, 108-114. http://dx.doi.org/10.1016/j.physb.2003.11.078
- Shahzamanian, M.A. (1989) Diffusive Thermal Conductivity of Superfluid 3He-A1 at Low Temperatures. Journal of Physics: Condensed Matter, 1, 1965-1970. http://dx.doi.org/10.1088/0953-8984/1/11/003
- Nasirimoghadam, S., Nabipoor, F., Khademi Dehkordi, M. and Shahzamanian, M.A. (2012) Transport Coefficients of the Superfluid Fermi gas in P-Wave State at Low Temperature. Physica C: Superconductivity, 483, 109-112. http://dx.doi.org/10.1016/j.physc.2012.05.020
- Khademi Dehkordi, M., Nasirimoghadam, S., Nabipoor, F. and Shahzamanian, M.A. (2012) Diffusive Thermal Conductivity of p-Wave State at Low Temperatures. Communications in Theoretical Physics, 58, 891-894. http://dx.doi.org/10.1088/0253-6102/58/6/16
- Afzali, R. and Ebrahimian, N. (2005) Diffusive Thermal Conductivity of the A1-Phase of Superfluid 3He at Low Temperatures. Journal of Physics: Condensed Matter, 17, 4441. http://dx.doi.org/10.1088/0953-8984/17/28/004
- Abrikosov, A.A. and Khalatnikov, I.M. (1959) The Theory of a Fermi Liquid. Reports on Progress in Physics, 22, 329. http://dx.doi.org/10.1088/0034-4885/22/1/310
- Takagi, S. (1975) On the NMR Properties near the 3He A-Normal Transition. Journal of Low Temperature Physics, 18, 309-314. http://dx.doi.org/10.1007/BF00118160
- Baranov, M.A. (2008) Theoretical Progress in Many-Body Physics with Ultracold Dipolar Gases. Physics Reports, 464, 71-111. http://dx.doi.org/10.1016/j.physrep.2008.04.007
- Pethick, C.J., Smith, H. and Bhattacharyya, P. (1977) Transport Processes in Superfluid 3He-B at Low Temperatures. Physical Review B, 15, 3384. http://dx.doi.org/10.1103/PhysRevB.15.3384
- Volhardt, D. and Wolfle, P. (1990) The Superfluid Phases of Helium 3. Taylor & Francis, London.
- Sykes, J. and Brooker, G.A. (1970) The Transport Coefficients of a Fermi Liquid. Annals of Physics, 56, 1-39. http://dx.doi.org/10.1016/0003-4916(70)90002-3
- Pethick, C.J. and Smith, H. (2002) Bose-Einestein Condensation in Dilute Gases. Cambridge University Press, Cambridge.
- Wolfle, P. and Einzel, D. (1978) Transport and Relaxation Properties of Superfluid 3He. Journal of Low Temperature Physics, 32, 39-56. http://dx.doi.org/10.1007/BF00116905

