International Journal of Geosciences
Vol.08 No.04(2017), Article ID:75473,19 pages
10.4236/ijg.2017.84022
Connecting Newton’s G with the Rest of Physics―Modern Newtonian Gravitation Resolving the Problem of “Big G’s” Value
Roger Ellman
The-Origin Foundation, Inc., Santa Rosa, USA

Copyright © 2017 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: February 7, 2017; Accepted: April 11, 2017; Published: April 18, 2017
ABSTRACT
As a simplified, idealized understanding of a physical system the General Relativity model has been highly successful in its gravitational role. However, it fails to address the problem of sufficiently precise measurement of “Big G”, the Newtonian Gravitation Constant, and has failed to obtain connection of “Big G” to the rest of physics. Because “Big G” arises naturally from Newton’s treatment of gravitation, this paper elaborates the Modern Newtonian Model of Gravitation and through it resolves the problems of “Big G” at which General Relativity has failed. Specifically: The causes of the problems in measuring “Big G” are resolved, “Big G” is connected to the rest of physics, and a sufficiently precise value of “Big G” is obtained by calculation from other fundamental physical constants. The companion paper The Experimental Data Validation of Modern Newtonian Gravitation over General Relativity Gravitation, which is available in this journal, publishes the results of this paper’s “Part V―Testing the Hypothesis and the Derivation”.
Keywords:
Gravitation, Newton’s “Big G”, Fundamental Constants

1. Part I―Background of the Problem
1.1. The Problem1
On October 9-10, 2014, several dozen scientists from around the world gathered at The National Institute of Standards and Technology, NIST, to consider their options.
“We’re all here because we have a problem with G- and I mean, boy, do we have a problem with G,” said Carl Williams, Chief of PML’s Quantum Measurement Division, to the assembled group on the first morning of the meeting. “This has become one of the serious issues that physics needs to address.”
Surprisingly, physicists still can’t agree on the value of the “big G” constant that features both in Isaac Newton’s law of gravitation, which dates back to the year 1687, and in Albert Einstein’s General Theory of Relativity. The Newtonian constant of gravitation, fondly known as “Big G”, used to calculate the attractive force of gravity between objects, is more than 300 years old. But although scientists have been trying to measure its value for centuries, G is still only known to about 3 significant figures as compared to most of the fundamental constants which are known to 9 significant figures.
Different experimental techniques have found contradictory values for G. And, worse yet, the more various experiments researchers conduct to pin down the gravitational constant, the more their results diverge. A plot of all the results from the past 15 years reveals a relatively wide spread in values ranging from about 6.670 to 6.676 × 10−11 m3・kg−1・s−2. CODATA, the International Council for Science Committee on Data for Science and Technology, which analyzes the results of individual experiments and provides an internationally accepted set of values for fundamental physical constants, has had to increase the uncertainty on its latest recommendation for a value of G due to the divergence of the experiments.
The problem is aggravated by that, unlike the other fundamental constants, G is described in CODATA’s “The 1986 Adjustment of the Fundamental Physical Constants” as follows:
“There is no established relationship between the gravitational constant G and other physical quantities; it stands completely uncoupled from the remainder of the adjustment.”
and that state of affairs remains unchanged through the most recent adjustment, that of 2014.
1.2. The Nature of the Solution
The solution to this set of problems consists of the following steps.
Part II―Demonstration of a fundamental problem in using the General Relativity model of gravitation in addressing the problem of “Big G” because “Big G” arises naturally from the Newtonian model of gravitation. This accounts for the wide range of measurement results that has limited the precision in the value of the constant.
Part III―Definition of what the “correct” value of the constant is a measure of and demonstration that it is practically impossible to measure it and that the correct value lies outside the entire range of the various diverse measured values and is smaller than any of them.
Part IV―Calculating the correct value of the constant by deriving a “relationship between the gravitational constant, G, and other physical quantities” [h, c, q, μ, α, π] contrary to CODATA’s report that there is no such established relationship.
Part V―Testing the hypothesis and the derivation.
2. Part II―The Fundamental Problem in the Conception of “Big G”
The fundamental problem in measuring “Big G” is the use of the General Relativity model of gravitation instead of the Newtonian model in which “Big G” arises naturally. That leads to the deeming that in effect the gravitational attraction is as if, for each gravitating body, all of its gravitating mass were concentrated at its center. The [modern] Newtonian understanding is that each of the gravitating bodies is composed of myriad individual gravitating particles. That is demonstrated as follows.
If a gravitating body were cut in half each of the halves would be a gravitating body. If the process were repeated progressively dividing each gravitating body into multiple separate bodies each would be a gravitating body. Carried out down to the atomic level each of the atomic particles of which the original undivided body is composed is an individual gravitating body and so acts whether individual and separate or as part of the original undivided body.
Every individual particle having rest mass interacts gravitationally with every other such particle. In the case of, for example, two separate spherical masses each consisting of a number of individual particles compacted together, each and every one of the individual particles in each sphere interacts gravitationally with each and every one of the particles in the other sphere. The gravitational effect that we experience is the net vector sum of those individual interactions.
Newton’s Law of Gravitation, Equation (1), states the acceleration of mass m resulting from the gravitation of mass M. According to that law that acceleration is independent of the mass of the accelerated body. That mass appears as a factor only in the application of Newton’s Second Law of Motion to the gravitational acceleration an action independent of gravitation, of the cause of the acceleration.
Picturing now the set-up for experiments to measure G in which the gravitational effect between spheres is measured, each individual particle in one of the spheres interacts in Newtonian Gravitational fashion paired with each and every of the individual particles in the other sphere or spheres. That results in two un-accounted for effects on the magnitude of the gravitational action.
1) Referring to Newton’s Law of Gravitation, Equation (1), the first effect is that the separation distance, d, for each Particle-to-Particle gravitational action varies depending on the two particles’ locations in their overall spheres. The average of those will equal the spheres’ center-to-center distance but, it is the separation distance squared that acts in the gravitational effect. In the Particle-to-Particle interaction it is each individual pair’s separation distance squared not the overall average separation that matters. Simply stated, the average separation distance squared as used in Newton’s Law of Gravitation is not the same as the average of the squared individual separation distances.
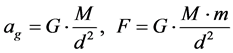 (1)
(1)
Because of the form of Newton’s Law of Gravitation and the overall gravitational concept of General Relativity, modern physics treats gravitation as depending solely on the gravitationally attracting mass and the center-to-center separation of the bodies, the characteristics of the attracted body being deemed of no significance. But, the independent gravitational action of each particle in the attracting body with each in the attracted body means that the configurations of the attracted body and the attracting body are factors in the overall effect a factor that appears in the interpretation of the d2 of Newton’s Law.
How this works out in practice is illustrated by the example of Figure 1, below. The figure compares two alternative calculations of the Newtonian Gravitational acceleration per Newton’s Law of Gravitation between two bodies: a lone single particle and a single body made up of a string of ten such particles connected together.
The two alternative calculations are:
a) Particle-to-Particle
Calculating the action between the lone single particle and each of the single particles of which the second body is composed, one at a time, ten calculations in total, and taking the total acceleration between the two bodies as being the sum of the accelerations for each of the ten particles of which body [1] is composed.
b) Center-to-Center
Calculating the action between the lone single particle and the second body overall taking the entire mass of the second body as located at its center.
The result is that the effects of the two alternatives are radically different. The Particle-to-Particle calculation result is aPtl-Ptl = 0.558 G whereas the Center-to-Center calculation result is aCtr-Ctr = 0.204 G. The concept of Newton’s Law of Gravitation is that the Center-to-Center method is used. However in actual measurement experiments the acceleration is naturally automatically determined by the Particle-to-Particle method and as a result is larger than it should be for determining Newton’s G per the Center-to-Center method. From Equation (1)
Figure 1. Alternative gravitation calculations―1st effect.
G is found by measuring ag and multiplying it by d2 divided by m. While the “d2” and “m” are correct for Newton’s Law the ag is too large and produces a G that is too large. [Adding together the individual Particle-to-Particle accelerations is valid because adding together the corresponding individual forces from F = m・a is valid.]
Furthermore, the difference between the Particle-to-Particle method and the Center-to-Center method varies with the amount of physical separation of the spheres introduced in the measurement set-up and with the diameter of each of the spheres. That, then, is a source of the spread of values obtained in various G measurement attempts.
2) The second effect is that most of the various Particle-to-Particle pairs’ lines of interaction (the line joining any pair of particles) are not parallel to the line joining the centers of the two spheres, the “centerline” (see Figure 2, below). Thus the total gravitational force of those interactions has two components: one parallel to the centerline and contributing to the overall gravitational attraction and the other at right angles to the centerline and cancelling to null when all of the Particle-to-Particle interactions are considered.
As with the first effect presented above, the various “angles” in Particle- to-Particle interactions vary with the amount of physical separation of the spheres in the set-up and with the diameters of the spheres-a variation that is a second source of the spread of values obtained in various G measurement attempts. This second effect results in a somewhat reduced gravitational action tending to off set to some extent the first effect’s enhancing of the gravitational action.
The net result of the two effect’s variation from experimental set-up to set-up causes the actual gravitational effect to vary from one experimental set-up to another. The cause of the discrepancies among the various attempts to obtain a precise value for G, the cause of the apparent variations in the value of “big G”, is not primarily measurement errors; the measurements are probably largely correct for each set-up. The problem is that in the measurement experiments the measured value for ag is slightly higher than per Newton’s Law making the calculated value of “Big G” too large. None of the measurement experiments is measuring the correct, objective “Big G” and certainly the various attempts are
Figure 2. Alternative gravitation calculations―2nd effect.
not measuring the exact same gravitational effect.
But, what about natural gravitational acceleration? All bodies near the Earth’s surface but free to fall are accelerated by gravity at the same 9.8 m/s2 regardless of the configuration of the particles making up its body. All such falling bodies experience essentially the same acceleration because the effect of the particle- on-particle gravitational interaction acts as the net average of the individual particle pair interactions. That average is dominated by the immensely greater number of particles in the attracting body, the Earth, and their immensely greater particle-to-particle separation distances compared to variation in particle locations in the attracted falling body.
3. Part III―The “Correct” Value of the Constant “Big G”
Inherent in the conception of Newtonian gravitation operating by deeming that the gravitational attraction is as if all of the mass of the gravitating bodies were concentrated at their centers is that the intended “Big G” constant correspond to that situation. That situation is approximately, and quite close to, the case for gravitating bodies separated by a distance much greater than the diameter of the bodies, such as interplanetary situations. It is not practical to obtain such conditions in a laboratory which means that it is not possible to practically measure a correct “Big G”.
Nevertheless, such a “correct Big G” is needed for space age activities and advancing inter-planetary travel and scientific probes and is highly desirable. The only solution to that dilemma is to obtain a relationship between the gravitational constant, G, and other well known physical quantities so that the correct “Big G” can be determined by calculation from the other well known physical quantities, without measurement.
Because the “correct Big G” corresponds to all of the mass of the gravitating bodies being concentrated at their centers, the “correct Big G” gravitational action experiences none of the distortions of the above effects 1 and 2. Those effects tend to produce a net increase of the associated gravitational attraction so that the gravitational attraction for the “correct Big G” is less than in the presence of the distortions. Thus, the “correct Big G” is smaller than per any of the measurements performed. It will shortly be developed that
where:
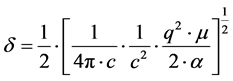 (2)
(2)
with: c = the speed of light,
q = the elementary charge,
μ = the magnetic constant,
α = the fine structure constant
the value of G is given by
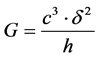 with: h = the Planck constant
with: h = the Planck constant
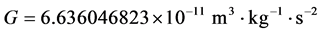
4. Part IV―The Modern Newtonian Model of Gravitation and Deriving the Value of the Constant “Big G”
The failure so far to connect “Big G” with the rest of physics is a direct consequence of attempting to do so on the basis of the General Relativity model of gravitation. The following successfully makes the connection by starting with the Newtonian model of gravitation in which “Big G” arises naturally.
The gravitational effect between two gravitating objects is, then, the net combined vector effect of myriad individual gravitating particles of one of the two objects interacting gravitationally in Particle-on-Particle pairs with myriad individual gravitating particles of the other of the two objects.
4.1. The Particle “Core” [1]
4.1.1. Gravitational Equivalent Frequency
Consider a small individual particle such as a proton or a neutron. The gravitational action of a massive body is the collective effect of the individual action, below, in each of its such particle components.
Newton’s law of gravitation expressed in terms of msource and macted-on and with both sides of the equation divided by macted-on is, of course,
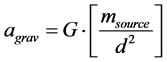 (3)
(3)
stating that gravitation is a property of a body’s mass.
However, mass and energy are equivalent, so that a mass, m, is proportional to a frequency, f, that is characteristic of that mass. That is
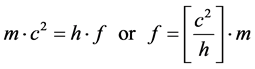 (4)
(4)
so that the msource of Equation (3) has a corresponding equivalent frequency, fsource.
That being the case, the gravitational acceleration, agrav, can be expressed in terms of that frequency as the change, Δv, in the velocity, v, of the attracted mass per time period, Tsource, of the oscillation at the corresponding frequency, fsource, as follows.
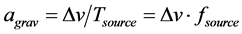 (5)
(5)
4.1.2. Gravitation and the Planck Length
It can then be reasoned setting Equation (5) = Equation (3) as follows.
 (6)
(6)
Equation (7), below, is obtained by using the fact that frequency is proportional to mass so that with fp and mp as the proton frequency and mass then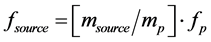 .
.
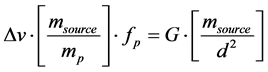 (7)
(7)
Rearranging and canceling msource on both sides of the equation,
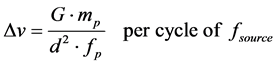 (8)
(8)
Then substituting, per Equation (4),  ,
,
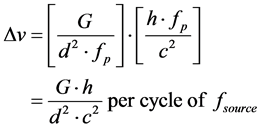 (9)
(9)
The Planck Length, lP, is defined as
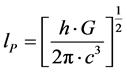 (10)
(10)
So that 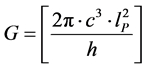
Substituting G as a function of the Planck Length from Equation (10) into G as it is in Equation (9), the following is obtained.
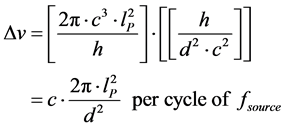 (11)
(11)
This result states that:
- The velocity change due to gravitation, Δv,
- Per cycle of the attracting mass’s equivalent frequency, fsource,
which quantity, Dv・fsource, is the gravitational acceleration, agrav,
- Is a specific fraction of the speed of light, c, namely the ratio of:
・ 2π times the Planck Length squared, 
・ the squared separation distance of the masses, d2.
That squared ratio is, of course, the usual inverse square behavior.
This also means that at distance 

The physical significance of 
That physical significance of
The fundamental distance constant, δ


Equation (11) then becomes Equation (13).

a quite pure and precise statement of gravitation, that gravitation is a function of the speed of light, c, and the inverse square law, in the context of the oscillation frequency, fsource, corresponding to the attracting, source body’s mass. It makes clear that an oscillation is an integral part of gravitation.
4.2. The Particle “Flow”
It is difficult to conceive of the immense and complicated set of interactions, i.e. each individual particle paired in gravitational interaction with each other individual particle, taking place via the individual gravitational masses each “curving space” to serve its own gravitational action. The difficulty is especially so in the absence of explanation of how “space” is subject to being curved and by what mechanism the curving is effected.
There being a need for each gravitationally acting [attracting] particle to communicate to each gravitationally acting [attracted] particle the direction from the attracted particle to the attracting one and the magnitude of the attracting particle’s gravitational attraction, there must be something flowing, continuously, carrying that information, spherically outward, from every gravitating particle to every other gravitating particle. That flow-communication is the gravitational field, an active process not a static state.
Furthermore, the necessity for gravitation that an oscillation and its frequency are closely involved in the effect [Equations (11) and (13)] and therefore in what is communicated by the flow means that the flow itself must be oscillatory.
For such a flow to persist and to have persisted the billions of years since the “Big Bang” there must be a supply of that outward flowing substance in every particle. And, that “supply” must be an extremely concentrated reservoir of that which flows outward [concentrated relative to the outward flow].
Having now just determined:
- That δ sets a limit on the minimum separation distance in gravitational interactions and therefore implies that a “core” of that radius is at the center of fundamental particles, and
- That an extremely concentrated reservoir supply of that which is flowing outward is required at the center of all particles to support the billions of years of the outward flow,
- The only reasonable conclusion is that that reservoir is a spherical “core” of radius δ at the center of all particles, and
- That its impenetrability is due to its immense concentration [billions of years worth of flow] of the flow substance [hereinafter “medium”] in the minute [δ = 4.05134 × 10−35 meters per Equation (12)] center of every particle having rest mass.
But, what “contains” that core’s supply or why doesn’t it all just quickly “slosh” out and be gone? The answer is that it is trying to do just that, to “slosh” out, as hard as it can. It cannot help propagating outward because it has no container. But it can only propagate outward at the limiting rate determined by its surface area, 4πδ2 and the fastest speed possible for it to flow, the speed of light, c.
Having established the supply of medium flow [flow substance] and its on-going outward flow serving the role of gravitational field as a property of every particle exhibiting rest mass, the question arises, “What of the electric field, much stronger than gravitation and co-present with gravitational field whenever the gravitating particle has electric charge ?”
Just as is the case for gravitation every particle having electric charge has a need to communicate its “message” to every other such particle: the direction back from the encountered particle to the transmitting one, the magnitude of the transmitting particles’ charge, and the nature of the charge, whether positive or negative. That flow-communication is the electric field, an active process not a static state.
Furthermore, for that flow to persist and to have persisted the billions of years since the “Big Bang” there must be a supply of that which is flowing outward for it at the center of every particle. And, that “supply” must be an extremely concentrated [relative to the outward flow] reservoir of that which flows outward.
Two such simultaneous flows constituting the two fields and two supporting reservoirs supplying the flows is clearly untenable. There can only be one reservoir in each particle’s “core” and one resulting flow producing both the gravitational field and the electric field if for no other reason than because two supply reservoirs would mutually interfere with comprehensive spherically outward flow of each.
The paper “A New Look at The Neutron and the Lamb Shift” [2] shows that the rest mass of the neutron is exactly equal to the rest mass of a proton plus that of an electron plus the mass equivalent of the kinetic energy of those two particles after they have electrostaticly accelerated from very far apart toward each other to a separation distance of a proton diameter. That fact is either a remarkable coincidence or evidence that the neutron is a combination of a proton and an electron.
Thus the neutron joins the other atomic particles in having both rest mass and electric charge [even though net electrically neutral]. All atomic particles have a single flow and supply reservoir producing from the same flow that which we observe and measure as electric field and gravitational field. Therefore the gravitational properties, gravitational flow and action of all particles, is closely interrelated with the particles’ electric properties, electric flow and action.
That being the case, the relating of “big G” to the rest of physics can be pursued via the physics of electric charge and electric field, which proceeds as follows.
4.3. Analytic Derivation of G in Terms of Fundamental Physics Constants
4.3.1. “Micro” vs. “Macro” Interpretation of Coulomb’s Law
Just as the gravitational effect,
- gravitational attraction, while experienced as a macro effect between bodies of significant amounts of mass, actually results from and is the accumulation of the micro interactions between pairs of single elementary particles of mass of which the bodies are composed;
so likewise the electric effect,
- electrostatic attraction or repulsion, is generally experienced as a macro effect between significant amounts of charge; however, the electric effect actually results from, and is the accumulation of, the micro interactions between pairs of single elementary charges.
Consequently it is the elementary charge, q, to which G is to be related.
Coulomb’s Law for the electric interaction demonstrates that for the case of two elementary charges the force is proportional to q1∙q2, or q2, the product of the magnitude of the two elementary charges. But, a particle cannot exert force on itself; Newton’s Third Law of Motion requires an equal magnitude opposite direction “partner” to every force, which would cancel a “self-force”. The transmitting particle, whose flow delivers force to the encountered particle experiences, in reaction to the transmitting particle’s spherically symmetrical outward flow, that Newtonian reaction as spherically symmetrical inward force with no net action because of the symmetry.
The encountered particle cannot exert such force on itself. Therefore the electric action produced by the transmitting particle, required by Coulomb’s Law to be proportional to q2 is so produced by its own charge squared, q2, which force is transmitted by, and imposed on the encountered particle by, the transmitting particle’s flow. Of course, both charges participate. Each elementary charge is simultaneously in the role of the transmitting particle and the particle encountered by that transmitted flow. But, the outward flow from the core of the transmitting particle transmits the flow effect of q2 to act as q2 on the encountered particle. The two charges in the macro statement of Coulomb’s Law are counts of the number of elementary charges in each macro charge yielding the total amount of charge involved.
Consequently it is the squared elementary charge, q2, to which G is related.
4.3.2. The Particle Core and the Core’s Outward Flow
The outward flow is flow of a highly effective substance in that it produces the effects of gravitational field and electric field; yet, it is at the same time a flow of an extremely intangible substance producing only the intangible gravitational field and electric field. But that flow is an integral component of humans’ physical world and acts in accordance with well established physical laws dealing with well known physical quantities.
But, the interior of the reservoir supply of medium is entirely foreign to us. We can not really conceive of gravitational plus electric field, stationary in place and so dense and concentrated that it supports outward flow of our world’s fields over many billions of years, any more than we can truly conceive of infinity.
The only thing known about the core, the “Core Domain” as compared to our “World Domain” is that as perceived from outside, in terms of the “World Domain” it appears to be a volume of 4/3・π・δ3 with a surface of 4・π・δ2. Therefore, volumetrically, i.e. in terms of the core volume, the outward flow through that surface is

But, that on-going flow generates potential energy field which in our “World Domain” appears to be static, not flowing. Flowing medium is static potential energy field.
Planck’s constant, h, appears as “energy per time” in the form W = h∙f. That oscillation energy, the equivalent of matter energy as in h∙f = m∙c2, cannot reside in the “f” nor in its equivalent 1/T; the energy of W = h∙f must reside in h.
Then the energy of the medium must result from the flow having the rate of “energy per flow” of h/c, which is of the fundamental dimensions [Mass × Length], which are also the fundamental dimensions of charge squared, q2.
4.3.3. The Electric Interaction of Two Elementary Charges and the Value of G
Earlier above it was stated that Equation (11) [

The interaction of two elementary charges then develops as follows for each of the two charges. The inconsistency of units here is due to the expressions in Equations (15) and (16) covering the transition from the [unknown] “Core Domain” to [our] “World Domain”. See “4.3.3 Resolution of the Issue of Units in equating Equations (15) to (16)”, below.


This means that the outward flow from particle’s “Core Domain” is the electric field of charge, [as well as the gravitational field of mass] and that the constant, k, of Equations (15) and (16) is 1/c2.
The objective here is to obtain the value of δ from which the value of lP can be calculated and from that the value of G can be calculated, all as follows.
Equating Equation (15) to Equation (16) via 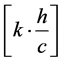
following is obtained.


Alternatively, from Equation (15) but with the constant, k = 1/c2 from Equation (16) the solution for δ is:


From Equation (12), the Planck Length is

[The failure of full coordination of lP with the third significant figure of the 2014 adjustment is due to the two errors in Part II above, which errors make their resulting G and therefore lP somewhat too large.
From Equation (10)


which is slightly below the range of the erroneous measurements, 6.670 to 6.676 × 10−11, as expected per “3. Part III-The “Correct” Value of the Constant “Big G”.
From Equation (13):
the following is develops.

4.3.4. Resolution of the Issue of Units in Equating Equation (15) to Equation (16)
The units of Equation (15), which is
but the units of Equation (16), which is
How can they be equated to each other?
The core’s outer boundary is a surface of area 4・π・δ2. It lacks the power to restrain or contain anything. However, the only way the content of the core can leave and flow outward is through the core’s surface. That flow is subject to the speed limit of light speed. That sets the flow at [4・π・δ2]・c.
That would appear to be able to fully deplete the core in the time

However, that is an extremely brief amount of time [4.504 × 10−44 seconds] whereas the universe and the flows have been in existence for billions of years. The resolution of that conflict is that the medium contained within the core is not merely the geometric core volume as we view it from our world; it is “highly concentrated volume”, the capability if freed into space outside the core for myriad core physical volumes, the volume of space.
That is the already cited difference between the “Core Domain” and the “World Domain”.
The ratio to the core’s world view geometrical volume of that “highly concentrated volume” as medium to be propagated is designated F, the medium magnifying factor, a dimensionless interpretive ratio. The Equation (22) depletion process then becomes

where F is the volume equivalent of the core medium supply relative to the core geometric volume

a pure number just as are 4/3, 4, and π of Equation (23). Saying the core is medium {M・L} vs. volume {L3} is like saying a year is {days} vs. {seconds}.
The medium magnifying factor F spans two very different regimes of material reality:
1) The natural world regime in which we exist and function;
2) The interior of the core of each particle, the supply of highly concentrated medium, minute portions of which are propagated outward in each cycle of the particle’s oscillation, gradually depleting the supply.
F spans the relationship between the “Core and World Domains”, it expresses the connection of the physical volume of the core and the concentrated-volume medium filling the core. It converts expressing the interior of the core, its substance, between units of volume, [4/3・π・δ3] {Length3}, and units of medium [h/c] {Mass・Length}, as propagated outward.
4.4. Summary of the Line of Proof
4.4.1. Step 1―The Problem: Particle-to-Particle Not Center-to-Center
The gravitational effect is not as if all of the mass of the gravitating bodies were concentrated at the center of each body. Each of the gravitating bodies is composed of myriad individual gravitating particles. Each and every one of the individual particles in each body interacts gravitationally with each and every one of the particles in the other bodies. The gravitational effect that we experience is the net vector sum of those individual interactions.
4.4.2. Step 2―The Nature of the Correct G
Two effects produce the erroneous results in experiments to measure G resulting in values for G that are too high. The correct value of G, unaffected by those errors, corresponding to measuring G with a separation distance, d, very much greater than the size of the gravitating bodies, is thus somewhat smaller than any of the erroneous values.
4.4.3. Step 3―The Radius and “Core” in Each Particle
Just as inertial mass, m, has an equivalent frequency m∙c2/h, so also does gravitational mass. Following out the implications of that, the result is Equation (13), repeated below.

The physical significance of this is that it sets a limit on the minimum separation distance in gravitational interactions and thus implies that a “core” of radius δ is at the center of every fundamental particle.
4.4.4. Step 4―Each Particle Must Transmit Outward Flow
The Particle-to-Particle nature of gravitation creates a need for each gravitationally acting [attracting] particle to communicate to each gravitationally acting [attracted] particle the direction from the attracted particle to the attracting one and the magnitude of the attracting particle’s gravitational attraction. Thus, there must be something flowing, continuously, carrying that information, spherically outward, from every gravitating particle.
4.4.5. Step 5―Each Particle’s “Core”―Is a Reservoir Supply for the Flow
For the flow of Step 4 to have persisted the billions of years since the “Big Bang” there must be a supply of that flowing substance in every particle. And, that supply must be an extremely concentrated reservoir relative to the outward flow. The only possible such reservoir is the “core” of Step 3.
4.4.6. Step 6―Definition of δ
The radius of that core relates to the Planck Length as Equation (12), repeated below.

The value of G can be calculated from δ or from the Planck Length, Equation (10), repeated below.



Thus, if δ can be related to and calculated from the rest of physics, then likewise can G.
4.4.7. Step 7―Electric Charge Flow Is Like Flow from Mass = Same Sole Flow
Just as in the case of gravitation, every particle having electric charge must communicate its “message” to every other such particle. That flow-communica- tion is the electric field.
Two simultaneous flows, one gravitational and one electric constituting the two fields and with two supporting reservoirs supplying the flows is clearly untenable. There can only be one reservoir in each particle’s “core” and one resulting flow producing both the gravitational field and the electric field.
4.4.8. Step 8―Elementary Charge Coulomb Action Is q2, Not Merely q
Between two elementary charges, the particle encountered by the transmitting particle’s electric field flow cannot exert force on itself because of Newton’s Third Law. Therefore the electric action produced by the transmitting particle, required by Coulomb’s Law to be of magnitude q2 is so produced by its own elementary charge squared, q2.
4.4.9. Step 9―“Core Domain” vs. “World Domain”
The only thing known about the “core”, the “Core Domain” as compared to our “World Domain” is that as perceived from outside, in terms of the “World Domain” the “Core Domain” appears to be a volume of 4/3∙π∙δ3 with a surface of 4∙π・δ2. Therefore, in terms of the “World Domain”, the outward flow through that surface would appear to be 4・π∙δ2・c.
However, Equation (13), shortly above, was interpreted to limit to δ the closet possible approach distance to a particle. But, when two such particles, for example two elementary charges, are involved, the minimum approach distance is 2∙δ because each of the two particles cannot individually be approached closer than δ.
4.4.10. Step 10―The Outward Flow Appears in the “World Domain” Proportional to h/c
The on-going outward flow generates potential energy field, which in our “World Domain” appears to be static, not flowing. Flowing medium appears to us as static potential energy field.
Planck’s constant, h, appears as “energy per time” in the form W = h∙f. That oscillation energy, the equivalent of matter energy as in h∙f = m∙c2, cannot reside in “f” nor its equivalent 1/T. The energy of W = h∙f must reside in h.
The energy of the flowing medium, the electric potential energy field, results from the flow having the rate of “energy per flow” proportional to h/c, which is of the fundamental dimensions [Mass × Length], which are also the fundamental dimensions of charge squared, q2, charge being that which we associate with electric field.
4.4.11. Step 11―The Electric Interaction of Two Elementary Charges
The interaction of two elementary charges then develops as follows for each of the two charges. The “Core Domain” flow,

causes and is proportional to the “World Domain” flow,

Further, per the Fine Structure Constant,
which rearranged is
and using k = 1/c2 [to convert “natural” statcoulombs to coulombs], results in
from which

Or, alternatively, from Equation (15) but with the constant, k = 1/c2 from Equation (16)

4.4.12. Final Step―G Calculated from the Planck Length or δ


5. Part V―Testing the Hypothesis and the Derivation
Testing the hypothesis and the derivation requires having available at hand the specific details and data of one of the numerous “Big G” measurement experiments conducted in recent years. The selected experiment can be any one of that group so long as the confidence that it contains negligible measurement errors is high. Preferably the testing should be conducted independently on several of the recent measurement experiments.
The procedure is to calculate the force, F [the force not the medium magnifying factor], per Equation (1) by two different methods using the parameters of the selected experiment to obtain a comparison ratio that of Calc1 to Calc2, below. For these calculations G = 1 can be used because only a ratio is sought.
Calc1―That of the General Relativity model with the objects’ masses concentrated at the objects’ centers. This calculation is simple and direct because there is only one value of d, the center to center spacing of the masses, and only one calculation.
Calc2―That of the Modern Newtonian Gravitation model with each individual particle in each object reacting, one-on-one, with each individual particle of the other object (s), a procedure analogous to the calculation of Figure 1 but including the additional effect illustrated in Figure 2. This calculation is quite complicated in that there are many values of d, a different one for each particle pair, and the sought value of F is the vector sum of all the individual particle to particle forces.
Because only the ratio of Calc1 to Calc2 is sought, the values of M and m can be ignored; the “lump amount” of each in Calc1 being the same as the sum of all the individual particles of those “lumps” in Calc2. However, the dimensions of the physical bodies of M and m are fundamental to the individual particle-on particle interactions for Calc2. The calculations deal only with the alternative treatments of d in Calc1 and Calc2 plus that in most cases only a partial component of the particle to particle force enters into the overall value of the force, F, in Calc2 as indicated in Figure 2.
The result will be that the F of Calc2 will be greater than the F of Calc1 implying a greater G as discussed in Part II.
The ratio, R, of the F of Calc1 divided by the F of Calc2 applied to the value of G that the original selected experiment obtained [R × G] will then yield a G corrected for the problems analyzed in Part II. Subject only to the experimental accuracy of the selected experiment the corrected G will be that of Equation (21),
Comprehensive Gravitation
This paper is an essential integral part of a set of papers treating Comprehensive Gravitation. The papers of the set are listed in references [3] [4] [5] [6] and [7] .
References*
[1] Ellman, R. (2001) Gravitational Equivalent Frequency and the Planck Length. Eprint Archive at https://arxiv.org/find/physics/1/au:+Ellman/0/1/0/all/0/1, physics/0101074.
[2] Ellman, R. (1998) A New Look at The Neutron and the Lamb Shift. Eprint Archive at https://arxiv.org/find/physics/1/au:+Ellman/0/1/0/all/0/1, physics/9808045.
[3] Ellman, R. (2016) Comprehensive Gravitation. ResearchGate. https://www.researchgate.net/profile/Roger_Ellman/info
[4] Ellman, R. (2016) Connecting Newton’s G with the Rest of Physics―Modern Newtonian Gravitation Resolving the Problem of “Big G’s” Value. ResearchGate. https://www.researchgate.net/profile/Roger_Ellman/info
[5] Ellman, R. (2016) The Experimental Data Validation of Modern Newtonian Gravitation over General Relativity Gravitation. ResearchGate. https://www.researchgate.net/profile/Roger_Ellman/info
[6] Ellman, R. (2016) The Mechanics of Gravitation―What It Is; How It Operates. ResearchGate. https://www.researchgate.net/profile/Roger_Ellman/info
[7] Ellman, R. (2016) Gravitational and Anti-Gravitational Applications. ResearchGate. https://www.researchgate.net/profile/Roger_Ellman/info
Submit or recommend next manuscript to SCIRP and we will provide best service for you:
Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.
A wide selection of journals (inclusive of 9 subjects, more than 200 journals)
Providing 24-hour high-quality service
User-friendly online submission system
Fair and swift peer-review system
Efficient typesetting and proofreading procedure
Display of the result of downloads and visits, as well as the number of cited articles
Maximum dissemination of your research work
Submit your manuscript at: http://papersubmission.scirp.org/
Or contact ijg@scirp.org
NOTES
1Descriptions of the Problem Derived From: 1) The website of the National Institute of Standards and Technology (NIST). 2) The website of The Committee on Data for Science and Technology (CODATA). 3) The website of “Nature, International Weekly Journal of Science”.
*References [3] - [7] are a complete set to be taken together.

















