Open Journal of Applied Sciences
Vol.06 No.05(2016), Article ID:66650,4 pages
10.4236/ojapps.2016.65031
The First Eccentric Zagreb Index of Linear Polycene Parallelogram of Benzenoid
Mehdi Alaeiyan1, Mohammad Reza Farahani1, Muhammad Kamran Jamil2, M. R. Rajesh Kanna3
1Department of Mathematics of Iran University of Science and Technology (IUST), Tehran, Iran
2Department of Mathematics, Riphah Institute of Computing and Applied Sciences (RICAS), Riphah International University, Lahore, Pakistan
3Department of Mathematics, Maharani’s Science College for Women, Mysore, India

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 21 March 2016; accepted 20 May 2016; published 23 May 2016
ABSTRACT
Let G = (V,E) be a graph, where V(G) is a non-empty set of vertices and E(G) is a set of edges, e = uvÎE(G), d(u) is degree of vertex u. Then the first Zagreb polynomial and the first Zagreb index Zg1(G,x) and Zg1(G) of the graph G are defined as 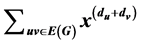 and
and 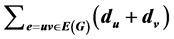 respec- tively. Recently Ghorbani and Hosseinzadeh introduced the first Eccentric Zagreb index as
respec- tively. Recently Ghorbani and Hosseinzadeh introduced the first Eccentric Zagreb index as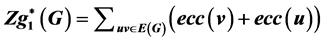 , that ecc(u) is the largest distance between u and any other vertex v of G. In this paper, we compute this new index (the first Eccentric Zagreb index or third Zagreb index) of an infinite family of linear Polycene parallelogram of benzenoid.
, that ecc(u) is the largest distance between u and any other vertex v of G. In this paper, we compute this new index (the first Eccentric Zagreb index or third Zagreb index) of an infinite family of linear Polycene parallelogram of benzenoid.
Keywords:
Molecular Graph, Linear Polycene Parallelogram of Benzenoid, Zagreb Topological Index, Eccentricity Connectivity Index, Cut Method

1. Introduction
By a graph, we mean a finite, undirected, simple graph. We denote the vertex set and the edge set of a graph G by V(G) and E(G), respectively. And the number of first neighbors of vertex u in G (the degree of u) is denoted by d(u). For notation and graph theory terminology not presented here, we follow [1] - [3] . All of the graphs in this paper are simple and a topological index of a graph is a number related to a graph which is invariant under graph automorphisms and is a numeric quantity from the structural graph of a molecule.
One of the best known and widely used is the Zagreb topological index Zg1 introduced by I. Gutman and N. Trinajstić in 1972 as [1] [2]
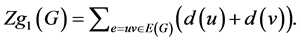
Also, we know another definition of the first Zagreb index as the sum of the squares of the degrees of all vertices of G.
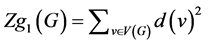
where du denotes the degree of u. Mathematical properties of the first Zagreb index for general graphs can be found in [4] - [8] .
Let x,yÎV(G), then the distance d(x,y) between x and y is defined as the length of any shortest path in G connecting x and y [9] - [11] .
In other words,
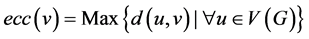 .
.
The radius and diameter of a graph G are defined as the minimum and maximum eccentricity among vertices of G, respectively. In other words,
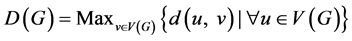 ,
,
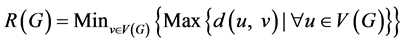 .
.
Recently in 2012, M. Ghorbani and M. A. Hosseinzadeh introduced a new version of first Zagreb index (the Eccentric version and ecc(v) denotes the eccentricity of vertex v) as follows [12] :
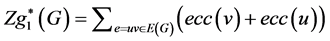 .
.
In this study, we call this eccentric version of the first Zagreb index by the third Zagreb index and denote by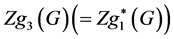 . And in continue, a formula of the third Zagreb index for an infinite family of linear Polycene parallelogram of benzenoid by using the Cut Method is obtained.
. And in continue, a formula of the third Zagreb index for an infinite family of linear Polycene parallelogram of benzenoid by using the Cut Method is obtained.
2. Results and Discussion
In this sections, we compute the third Zagreb index M3(G) for linear Polycene parallelogram of benzenoid P(n,n) ("n ≥ 1). This family of benzenoid graph has 2n(n+2) vertices/atoms and
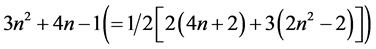 edges (bonds) [13] - [23] . The general representation of linear Po-
edges (bonds) [13] - [23] . The general representation of linear Po-
lycene parallelogram of benzenoid P(n,n) is shown in Figure 1.
Now, we can exhibit the closed formula of the third Zagreb index M3(Hk) in the following theorem.
Theorem 1. Considering the linear Polycene parallelogram of benzenoid P(n,n) ("nÎℕ), then its third Zagreb index is equal to
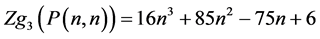 .
.
Proof. "nÎℕ, let P(n,n) be the linear Polycene parallelogram of benzenoid, as shown in Figure 1. To achieve our aims, we use of the Cut Method. Definition of the Cut Method and some of its properties are presented in [24] . Thus, we encourage readers to look at Figure 1 and see all cuts of the linear Polycene parallelogram of benzenoid P(n,n).
So according to Figure 1, one can see that the eccentric vertices with degree two are between 2n+1, 2n+2, 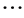 , 4n−6, 4n−4, 4n−2, 4n−1 or the number set
, 4n−6, 4n−4, 4n−2, 4n−1 or the number set
And also, the eccentric vertices with degree two are between 2n, 2n+1 to 4n−4, 4n−3 or in the number set
Figure 1. The eccentric of vertices of linear polycene parallelogram of benzenoid P(n,n) [14] .

Therefore, by using above results and [14] - [23] , we have the following computations for the third Zagreb index of the linear Polycene parallelogram of benzenoid P(n,n) as:
Cite this paper
Mehdi Alaeiyan,Mohammad Reza Farahani,Muhammad Kamran Jamil,M. R. Rajesh Kanna, (2016) The First Eccentric Zagreb Index of Linear Polycene Parallelogram of Benzenoid. Open Journal of Applied Sciences,06,315-318. doi: 10.4236/ojapps.2016.65031
References
- 1. Gutman, I. and Trinajstic, N. (1972) Graph Theory and Molecular Orbitals. III. Total π-Electron Energy of Alternant Hydrocarbons. Chemical Physics Letters, 17, 535-538. http://dx.doi.org/10.1016/0009-2614(72)85099-1
- 2. Gutman, I. and Das, K.C. (2004) The First Zagreb Index 30 Years after. MATCH Communications in Mathematical and in Computer Chemistry, 50, 83-92.
- 3. Todeschini, R. and Consonni, V. (2000) Handbook of Molecular Descriptors. Wiley, Weinheim. http://dx.doi.org/10.1002/9783527613106
- 4. Nikolic, S., Kovacevic, G., Milicevic, A. and Trinajstic, N. (2003) The Zagreb Indices 30 Years after. Croatica Chemica Acta, 76, 113-124.
- 5. DeCaen, D. (1988) An Upper Bound on the Sum of Degrees in a Graph. Discrete Mathematics, 185, 245-248. http://dx.doi.org/10.1016/S0012-365X(97)00213-6
- 6. Das, K.C. (2003) Sharp Bounds for the Sum of the Squares of the Degrees of a Graph. Kragujevac Journal of Mathematics, 25, 31-49.
- 7. Peled, U.N., Petreschi, R. and Sterbini, A. (1999) (n,e)-Graphs with Maximum Sum of Squares of Degrees. Journal of Graph Theory, 31, 283-295. http://dx.doi.org/10.1002/(SICI)1097-0118(199908)31:4<283::AID-JGT3>3.0.CO;2-H
- 8. Szekely, L.A., Clark, L.H. and Entringer, R.C. (1992) An Inequality for Degree Sequences. Discrete Mathematics, 103, 293-300. http://dx.doi.org/10.1016/0012-365X(92)90321-6
- 9. Ghorbani, M. and Hosseinzadeh, M.A. (2012) A New Version of Zagreb Indices. Filomat, 26, 93-100. http://dx.doi.org/10.2298/FIL1201093G
- 10. Sharma, V., Goswami, R. and Madan, A.K. (1997) Eccentric Connectivity Index: A Novel Highly of Descriptor for Structure-Property and Structure-Activity Studies. Journal of Chemical Information and Computer Sciences, 37, 273- 282. http://dx.doi.org/10.1021/ci960049h
- 11. Gupta, S., Singh, M. and Madan, A.K. (2000) Connective Eccentricity Index: A Novel Topological Descriptor for Predicting biological Activity. Journal of Molecular Graphics & Modelling, 18, 18-25. http://dx.doi.org/10.1016/S1093-3263(00)00027-9
- 12. Gupta, S., Singh, M. and Madan, A.K. (2002) Application of Graph Theory: Relationship of Eccentric Connectivity Index and Wiener’s Index with Anti-Inammatory Activity. Journal of Mathematical Analysis and Applications, 266, 259-268. http://dx.doi.org/10.1006/jmaa.2000.7243
- 13. Khadikar, P.V. (2010) Padmakar-Ivan Index in Nanotechnology. Iranian Journal of Mathematical Chemistry, 1, 7-42.
- 14. Alaeiyan, M., Mojarad, R. and Asadpour, J. (2011) A New Method for Computing Eccentric Connectivity Polynomial of an Infinite Family of Linear Polycene Parallelogram of Benzenoid. Optoelectronics and Advanced Materials-Rapid Communications, 5, 761-763.
- 15. Alaeiyan, M. and Asadpour, J. (2012) Computing the MEC Polynomial of an Infinite Family of the Linear Parallelogram P(n,n). Optoelectronics and Advanced Materials-Rapid Communications, 6, 191-193.
- 16. Farahani, M.R. (2014) Connective Eccentric Index of Linear Parallelogram P(n,m). International Letters of Chemistry, Physics and Astronomy, 18, 57-62.
- 17. Farahani, M.R. (2015) On Connectivity Indices of an Infinite Family of the Linear Parallelogram of Benzenoid Graph. International Letters of Chemistry, Physics and Astronomy, 54, 131-134.
- 18. Farahani, M.R. (2014) Two Types of Connectivity Indices of the Linear Parallelogram Benzenoid. New Frontiers in Chemistry, 23, 73-77.
- 19. Farahani, M.R. (2015) Zagreb Indices and Their Polynomials of the Linear Parallelogram of Benzenoid Graph. Global Journal of Chemistry, 1, 16-19.
- 20. Farahani, M.R., Rajesh Kanna, M.R., Rehman, H.M. and Jamil, M.K. (2016) About the Augmented Eccentric Connectivity Index of Linear Polycene Parallelogram Benzenoid P(n,n). Journal of Environmental Science, Computer Science, Engineering & Technology, in Press.
- 21. Farahani, M.R., Jamil, M.K. and Rajesh Kanna, M.R. (2016) About the Ediz Eccentric Connectivity Index of Linear Polycene Parallelogram Benzenoid. International Journal of Scientific & Engineering Research, 7, 1469-1475.
- 22. Farahani, M.R., Asadpour, J. and Rajesh Kanna, M.R. (2016) Computing the Geometric-Arithmetic Eccentricity Index of an Infinite Family of Benzenoid. Asian Academic Research Journal of Multidisciplinary, 3, 23-29.
- 23. Farahani, M.R., Rajesh Kanna, M.R. and Hosamani, S.M. (2016) On the Second Zagreb Eccentricity Index of Linear Polycene Parallelogram of Benzenoid P(N,N). Journal of Environmental Science, Computer Science, Engineering & Technology, in Press.
- 24. Klavzar, S. (2008) A Bird’s Eye View of the Cut Method and a Survey of Its Applications in Chemical Graph Theory. MATCH Communications in Mathematical and in Computer Chemistry, 60, 255-274.




