Open Journal of Discrete Mathematics
Vol.3 No.3(2013), Article ID:34497,4 pages DOI:10.4236/ojdm.2013.33022
Delay-Dependent Robust Passive Control for Uncertain Discrete-Time Systems with Time Delays*
1College of Sciences, Hebei University of Science and Technology, Shijiazhuang, China
2Nanjing University of Aeronautics and Astronautics, Nanjing, China
Email: wangjufang1981@126.com, changlongyu@126.com
Copyright © 2013 Jufang Wang et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.
Received April 19, 2013; revised May 20, 2013; accepted June 15, 2013
Keywords: Robust Passive Control; Discrete Systems; Time Delay
ABSTRACT
This paper considers the problem of robust passive control for uncertain discrete systems with time-varying delays. We pay attention to designing a state feedback controller which guarantees the passivity of the closed-loop system for all admissible uncertainties. In terms of a linear matrix inequality, a sufficient condition for the solvability of this problem is presented and the explicit expression of the desired state feedback controller is given.
1. Introduction
In the past several years, much attention has been paid to the study of stability of systems with control input delay. Much of them is focused on the passivity analysis for classes of time-delay systems. Using classical definitions of passivity and positive realness, the conditions for a nonlinear system can be rendered passive via smooth state feedback, see [1,2]. The robust passive control problem for time-delay systems was dealt with in [3,4] via various approaches. The robust passivity synthesis problem for discrete-time-delay systems is investigated in [5,6], but all these time delays are constant. To the best knowledge of authors, the problem of robust passive control for discrete-time systems with time-varying delays has not been fully investigated, which is more complex.
In this paper, we deal with the problem of robust passive feedback control for discrete systems with parameter uncertainties and time-varying delays. The parameter uncertainties are assumed to be time-varying but normbounded. The purpose is to construct a state feedback controller such that the closed-loop system is strictly passive and obtain a delay-dependent condition for the solvability of the problem.
2. Statement of the Problem
Consider the following uncertain discrete-time system with time-varying delays:
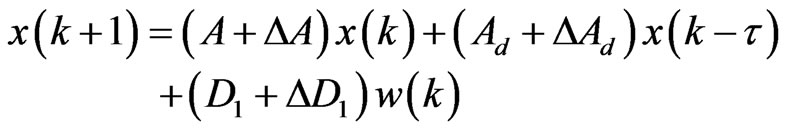 (2.1)
(2.1)
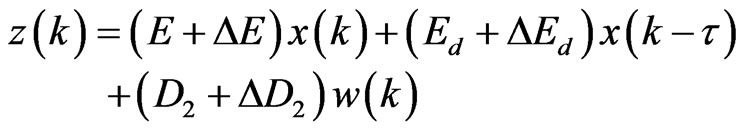 (2.2)
(2.2)
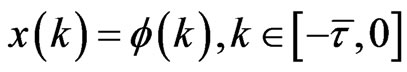 (2.3)
(2.3)
where 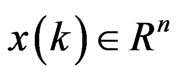 is the state,
is the state, 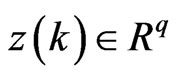 is the controlled output,
is the controlled output, 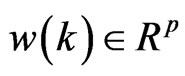 the disturbance input which is assumed to belong to
the disturbance input which is assumed to belong to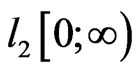 ;
; 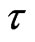 is a positive integer representing the time-varying delay of the system, which satisfies the following assumption:
is a positive integer representing the time-varying delay of the system, which satisfies the following assumption: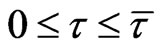 .
. 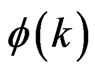 is a real-valued initial function on
is a real-valued initial function on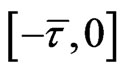 ;
; 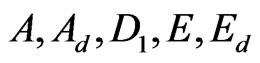 and
and 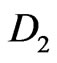 are known real constant matrices;
are known real constant matrices; 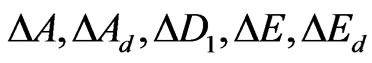 and
and 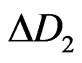 are unknown matrices representing time-varying parameter uncertainties, and are assumed to be of the form
are unknown matrices representing time-varying parameter uncertainties, and are assumed to be of the form
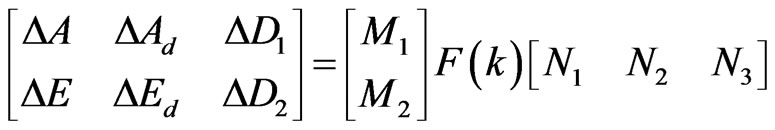 (2.4)
(2.4)
where 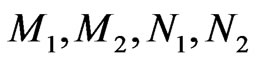 and
and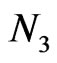 , are known real constant matrices and
, are known real constant matrices and 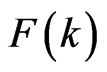 satisfies:
satisfies:
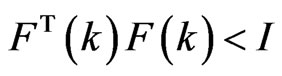 . (2.5)
. (2.5)
Our problem is to establish the passive control for systems (2.1)-(2.3) to determine the conditions. To this end, we introduce the following fact and related definition of passivity.
Lemma 2.1 Given constant symmetric matrices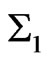 ,
, 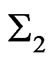 ,
, 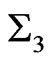 , where
, where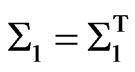 , and
, and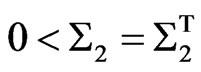 , then
, then
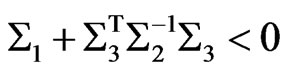 if and only if
if and only if
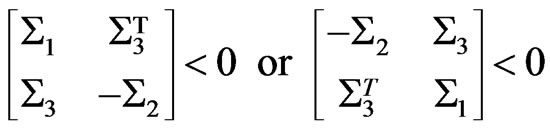 .
.
Lemma 2.2 Given constant matrices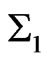 ,
, 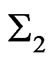 ,
, 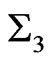 of appropriate dimensions with
of appropriate dimensions with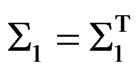 . Then
. Then
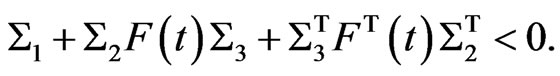
where 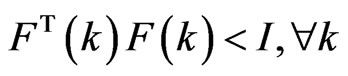 if and only if for some scalar
if and only if for some scalar 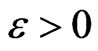
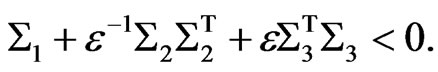
Lemma 2.3 Let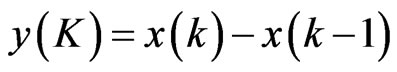 , then the following inequality holds for any matrices R, S1,
, then the following inequality holds for any matrices R, S1, 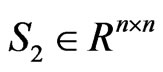 and positive scalar
and positive scalar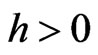 :
:
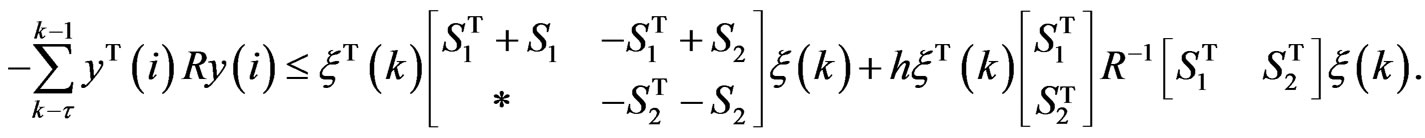
Definition 2.1 The dynamical systems (2.1) - (2.3) is called passive if there exists a scalar 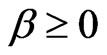 such that
such that
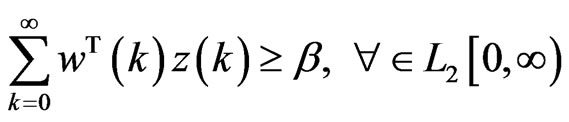
where 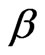 is some constant which depends on the initial condition of the system.
is some constant which depends on the initial condition of the system.
In addition, the systems (2.1)-(2.3) is said to be strictly passive if it is passive and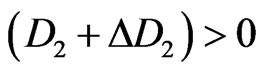 . In the sequel, we provide conditions under which a class of discretetime linear dynamical systems with time-varying parameter uncertainties can be guaranteed to be strictly passive. First, we have the following result pertaining to the system (2.1)-(2.3).
. In the sequel, we provide conditions under which a class of discretetime linear dynamical systems with time-varying parameter uncertainties can be guaranteed to be strictly passive. First, we have the following result pertaining to the system (2.1)-(2.3).
3. Proof of Main Results
Theorem 3.1 The discrete-time systems with time delay (2.3) is strictly passive if there exist symmetric positive definite matrices P, R, Q and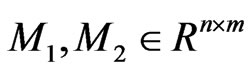 , such that the following LMI holds:
, such that the following LMI holds:
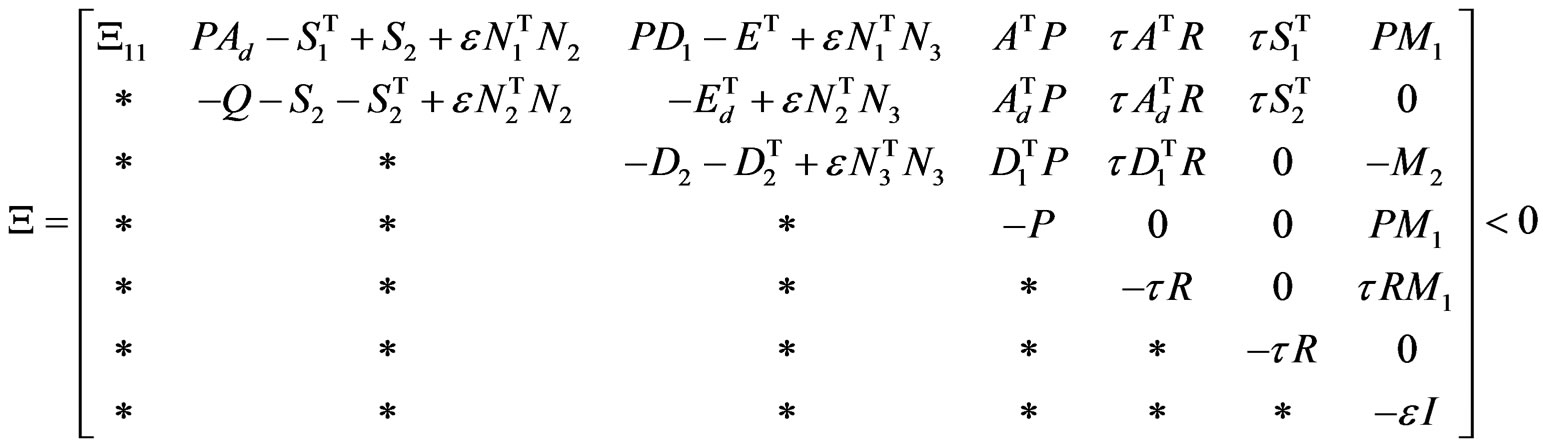 (3.1)
(3.1)
where 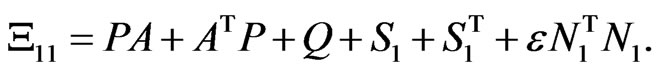
Proof. Choose a Lyapunov function candidate for the system (2.1) - (2.3) as follows:
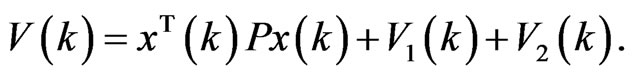 (3.2)
(3.2)
where
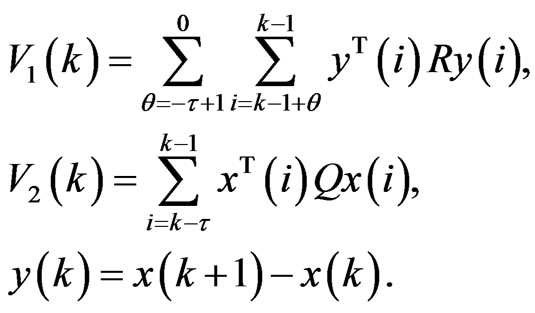
Now, by some calculations, we can get that
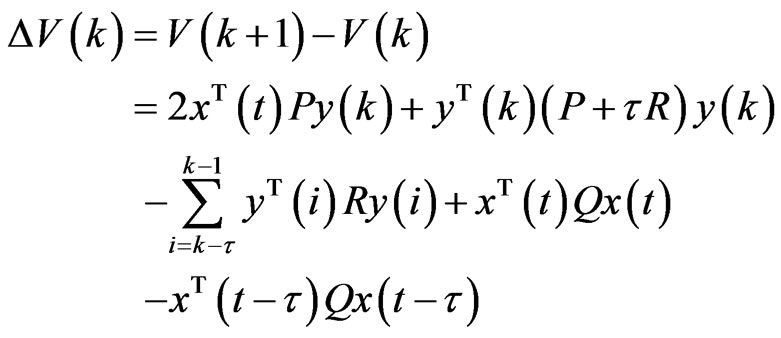 (3.3)
(3.3)
We define that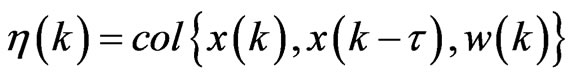 , then have
, then have
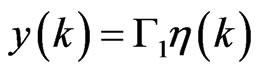 . (3.4)
. (3.4)
From the Lemma 2.3, for , we can have that
, we can have that
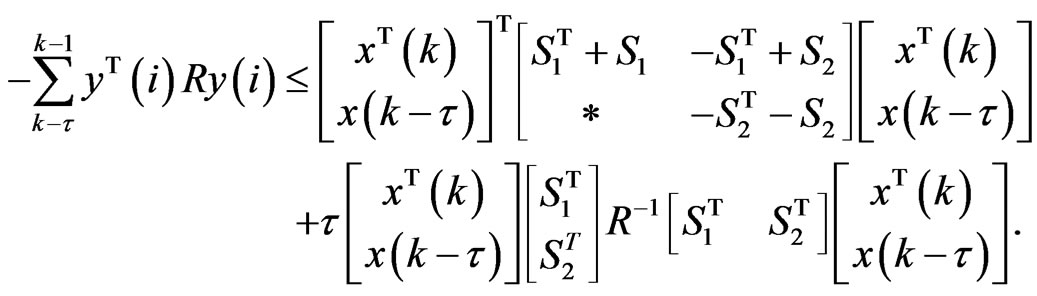 (3.5)
(3.5)
We have (3.3) and (3.4) into (3.2), after some manipulation, then obtain the following inequality:
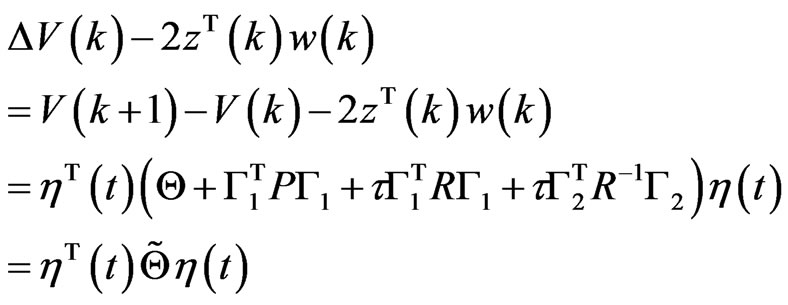 (3.6)
(3.6)
where
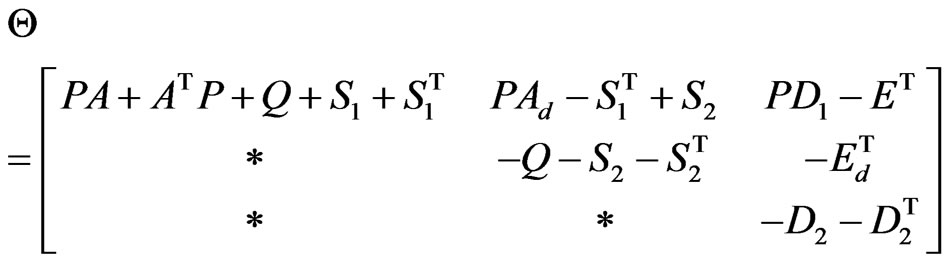
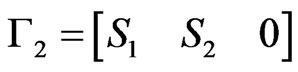
 .
.
If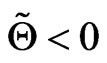 , then
, then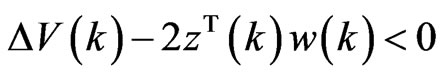 , and from which it follows that
, and from which it follows that
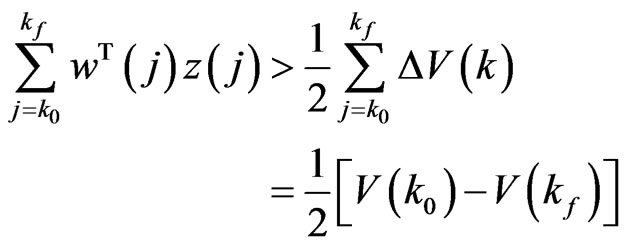 . (3.7)
. (3.7)
Since 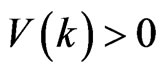 for
for 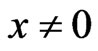 and
and 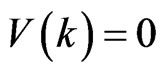 for x = 0, it follow as
for x = 0, it follow as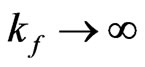 , that systems (2.1) - (2.3) is strictly passive. In view of Definition 2.1, the strictly passive condition is guaranteed if
, that systems (2.1) - (2.3) is strictly passive. In view of Definition 2.1, the strictly passive condition is guaranteed if 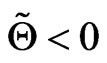 and it can be expressed conveniently as
and it can be expressed conveniently as
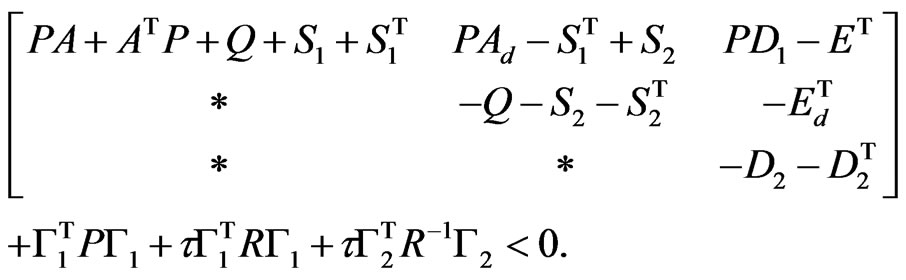
(3.8)
where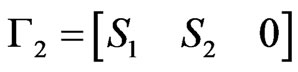
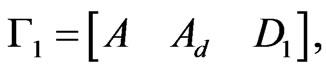 .
.
Application of Lemma 2.1 to the above inequality, it puts into the following form:
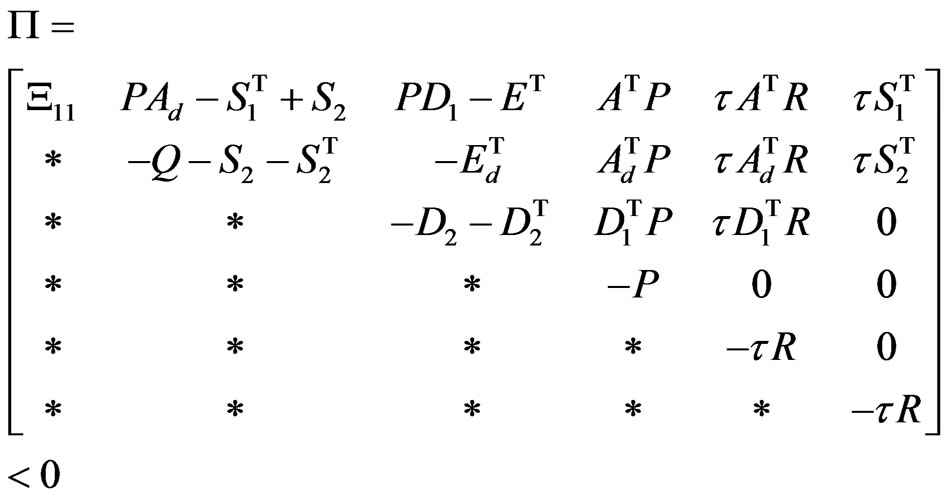
(3.9)
Substituting the uncertainty structure (2.5) into (3.9) and rearranging, we get the following inequality
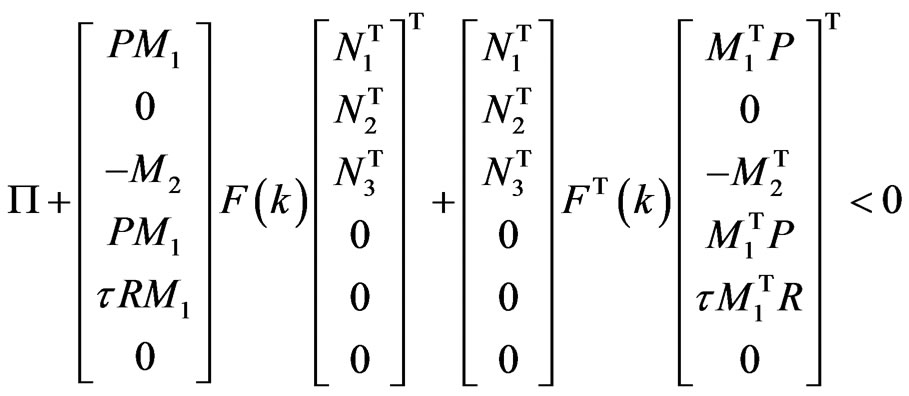
(3.10)
Then by Lemma 2.2, the inequality (3.10) holds if and only if for some 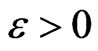
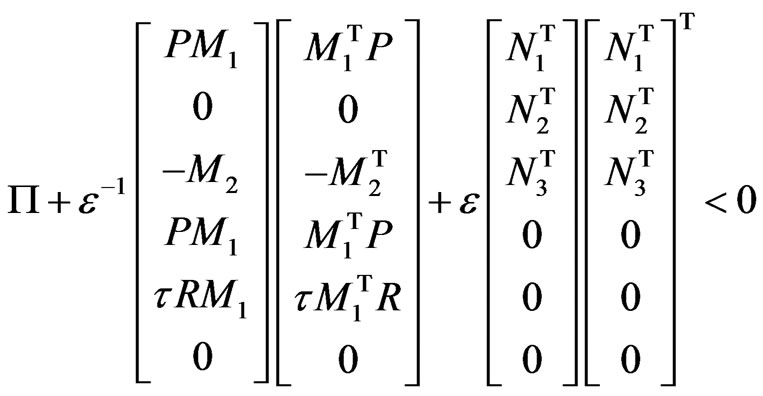 (3.11)
(3.11)
for all admissible uncertainties satisfying (2.4). On using Lemma 2.1 in (3.11), it becomes that 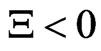 in (3.1). This completes the proof.
in (3.1). This completes the proof.
4. Robust Passive State Feedback Controller
We now build on the foregoing results by considering the passive control problem, that is, designing a state feedback controller to render the closed-loop time-delay system passive. Extending the system (2.1)-(2.3), we consider a class of time-delay systems of the form:
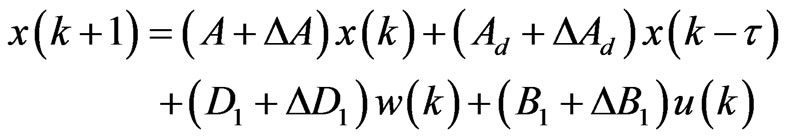 (4.1)
(4.1)
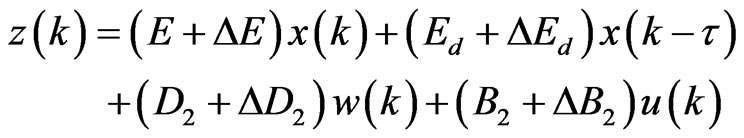 (4.2)
(4.2)
where 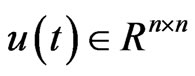 is the control input,
is the control input, 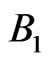 ,
, 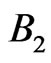 , are known real constant matrices;
, are known real constant matrices; 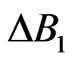 and
and 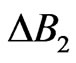 are unknown matrices representing time-varying parametre uncertainties, and are assumed to be of the form:
are unknown matrices representing time-varying parametre uncertainties, and are assumed to be of the form:
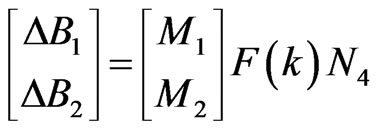 . (4.3)
. (4.3)
Then the transformed system becomes
 (4.4)
(4.4)
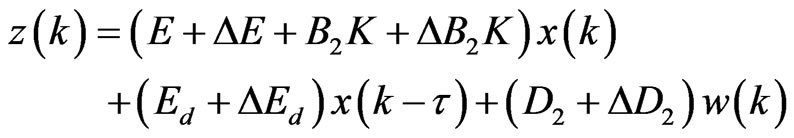 (4.5)
(4.5)
then we observe that
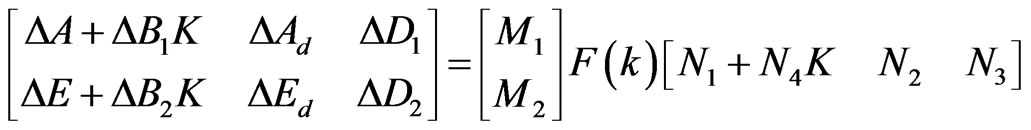 . (4.6)
. (4.6)
The following theorem establishes the main result.
Theorem 4.1 Consider the uncertain discrete-time delay system (4.4), (4.5). If there exists a positive scalar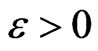 , a real matrix Y, three symmetric positive definite matrices
, a real matrix Y, three symmetric positive definite matrices ,
, 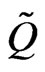 ,
, 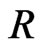 such that the following inequality holds:
such that the following inequality holds:
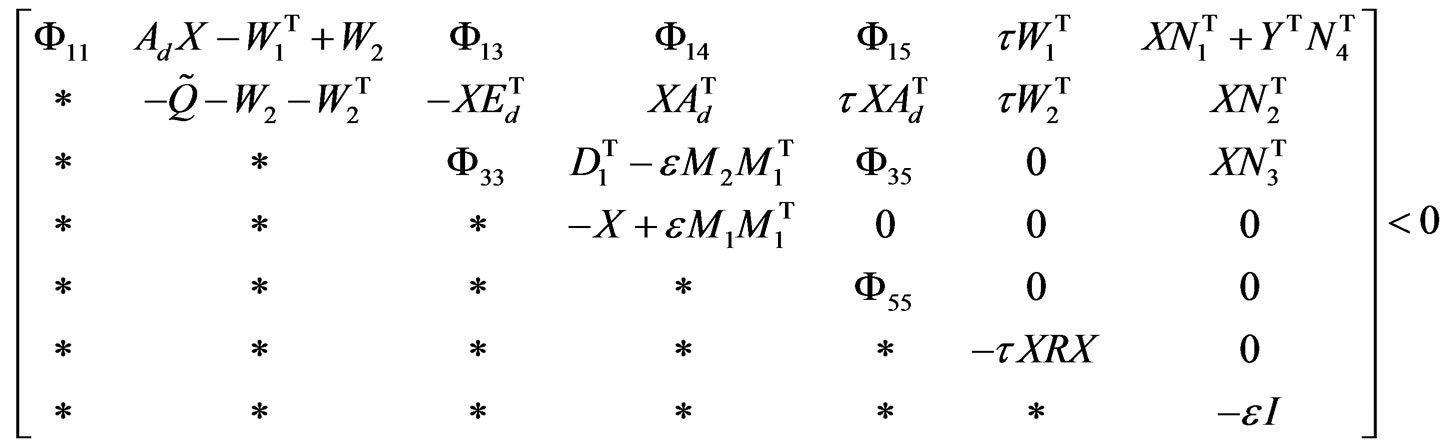 (4.7)
(4.7)
where
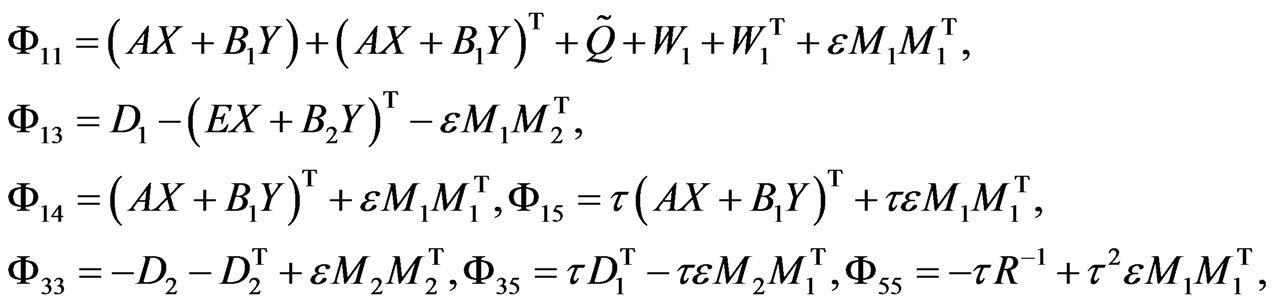
then the systems (4.4), (4.5) are strictly passive, and the state-feedback gain matrix is given by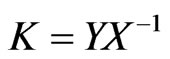 .
.
Proof. Similar to Theorem 3.1.
Remark 4.1 It is noted that the matrix inequalities conditions in Theorem 4.1 are not LMIs. In order to solve the matrix inequalities conditions in Theorem 4.1, we can follow a similar line as in Lee et al. (2004) and Moon et al. (2001) to provide a nonlinear minimization problem subject to LMIs.
REFERENCES
- W. Lin, “Global Asymptotic Stabilization of General Nonlinear Systems with Stable Free Dynamics via Passivity and Bounded Feedback,” Automatica, Vol. 32, No. 6, 1996, pp. 915-924. doi:10.1016/0005-1098(96)00013-1
- W. Z. Su and L. H. Xie, “Robust Control of Nonlinear Feedback Passive Systems,” Automatica, Vol. 28, No. 2, 1996, pp. 5-93.
- B. T. Cui and M. G. Hua, “Robust Passive Control for Uncertain Discrete-Time Systems with Time-Varying Delays,” Chaos, Solitons & Fractals, Vol. 29, No. 2, 2006, pp. 331-341. doi:10.1016/j.chaos.2005.08.039
- Z. H. Guan, H. Zhang and S. H. Yang, “Robust Passive Control for Internet-Based Switching Systems with TimeDelay,” Chaos, Solitons & Fractals, Vol. 36, No. 2, 2008, pp. 479-486. doi:10.1016/j.chaos.2006.06.039
- W. Lin and C. I. Byrnes, “Passivity and Absolute Stabilization of a Class of Discrete-Time Nonlinear Systems,” Automatica, Vol. 31, No. 2, 1995, pp. 263-267. doi:10.1016/0005-1098(94)00075-T
- Q. K. Song, J. L. Liang and Z. D. Wang, “Passivity Analysis of Discrete-Time Stochastic Neural Networks with Time-Varying Delays,” Neurocomputing, Vol. 72, No. 7-9, 2009, pp. 1782-1788. doi:10.1016/j.neucom.2008.05.006
NOTES
*The project is supported by the Natural Science Foundation of China (10901045) and (11201112), and Natural Science Foundation of Hebei Province (A2013208147) and (A2011208012) and the Foundation of Hebei University of Science and Technology (XL200757).

