ABSTRACT

By analyzing the susceptible-infected model, susceptible-infected-recovered-susceptible model and susceptible infectedrecovered model, we get the improved Kermachk-Mckendrick model. And by applying the controlled threshold value, we get the conditions of isolated rate for infectious disease eventually disappeared.

1. Introduction

With the acceleration of global economy, the contact and communication among people are more and more frequent. In addition, as the environmental pollution is increasingly serious, more and more infectious diseases have brought huge impact and harm to human beings. Infectious diseases prediction is an important scientific controlled means for infectious diseases, so how to forecast infectious diseases accurately is an important issue in the field of current medical research [1]. At present, the forecasting methods for infectious diseases are mainly qualitative prediction [2], quantitative prediction and comprehensive prediction [3]. The quantitative prediction has many methods, including regression analysis [4], gray system [5], markov chain [6] and neural network [7] forecasting method. Using mathematical models to forecast the popular trend of infectious diseases has become a consensus and further can help find the spread mechanism of infectious disease. In this paper, mathematical models for several kinds of common infectious diseases were reviewed, and by analysing the controlled threshold value of Kermachk-Mckendrick model, we obtain the isolation rate of infectious diseases terminated. It is conducive for government departments to take effective measures to prevent the further spread of infectious diseases, and control the spread of infectious diseases effectively.

2. The Model of Infectious Diseases

2.1. Susceptible Infected Model

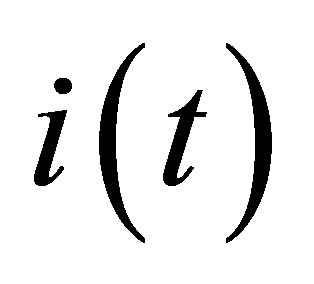Supposed the total number in examined area remains the same at the spread of disease period, regardless of people's life and death and migration, population are divided into healthy subjects and patients, the two in proportion of the total number are and at 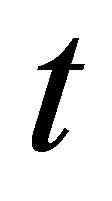moments respectively, 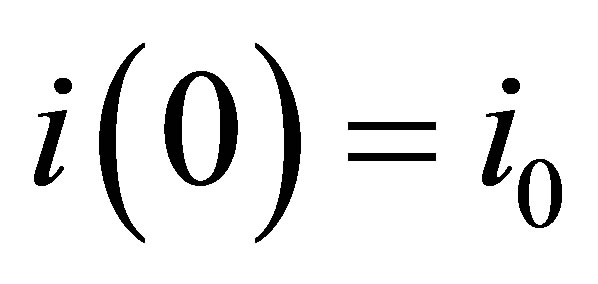is the proportion of patients at the initial time, and

.

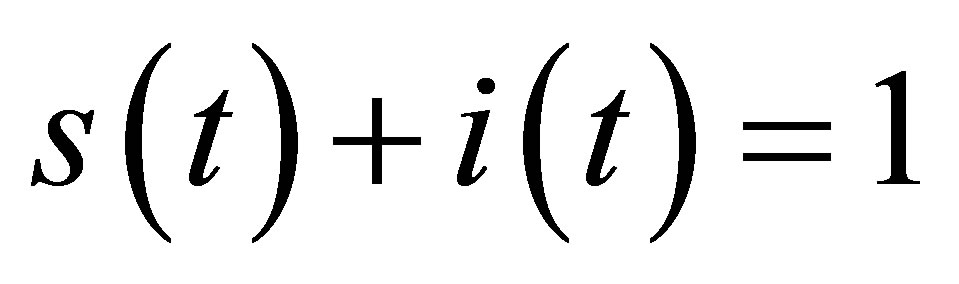Assume that the average number of each patient contact effectively at every day is a constant. That is to say, turn the healthy subjects into infected patients when the patients contact with healthy subjects effectively. Model is as follows:

i.e.,

(1).

The model is called Logistic model. We can find

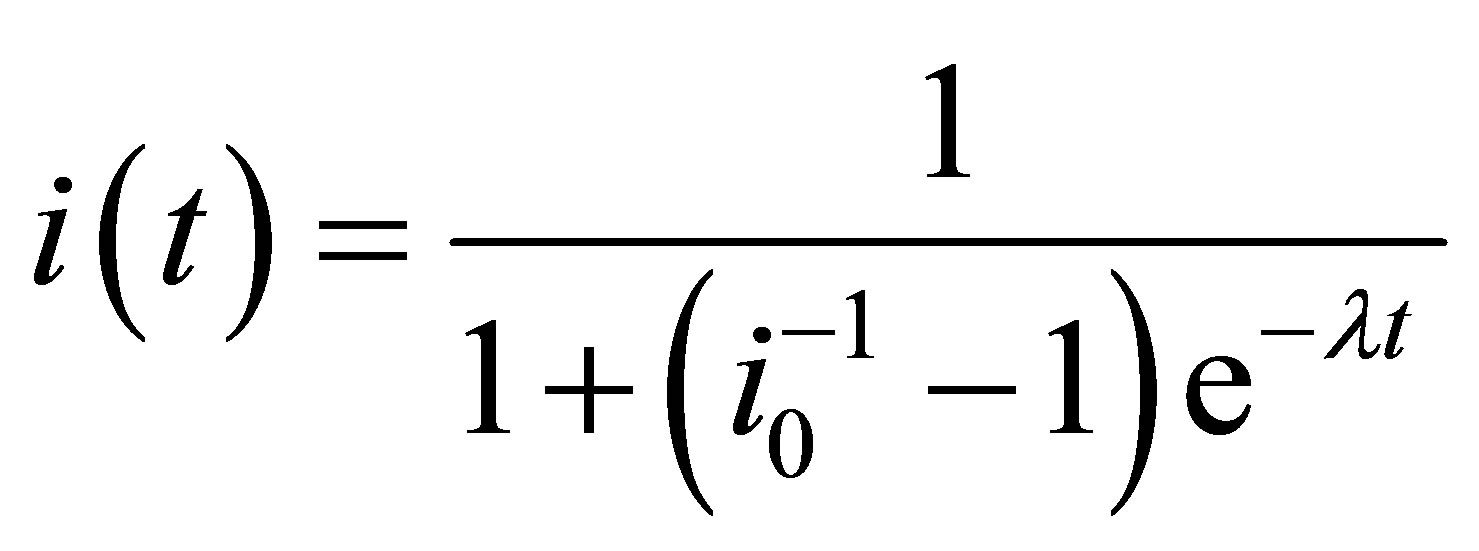
When, , That is all people will be infected and become the patient, this is obviously not conform to the truth. So we consider the condition that patient can be cured or immune, the improved models are following.

2.2. Susceptible Infected Recovered Susceptible Model

Let infectious diseases be immunity that patients can be cured to be healthy people, on the other hand, healthy people can be infected again, assuming that the proportion of patients healed every day is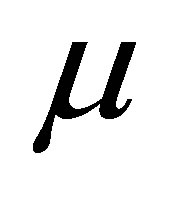, is cure rate for days. The model is as follows:

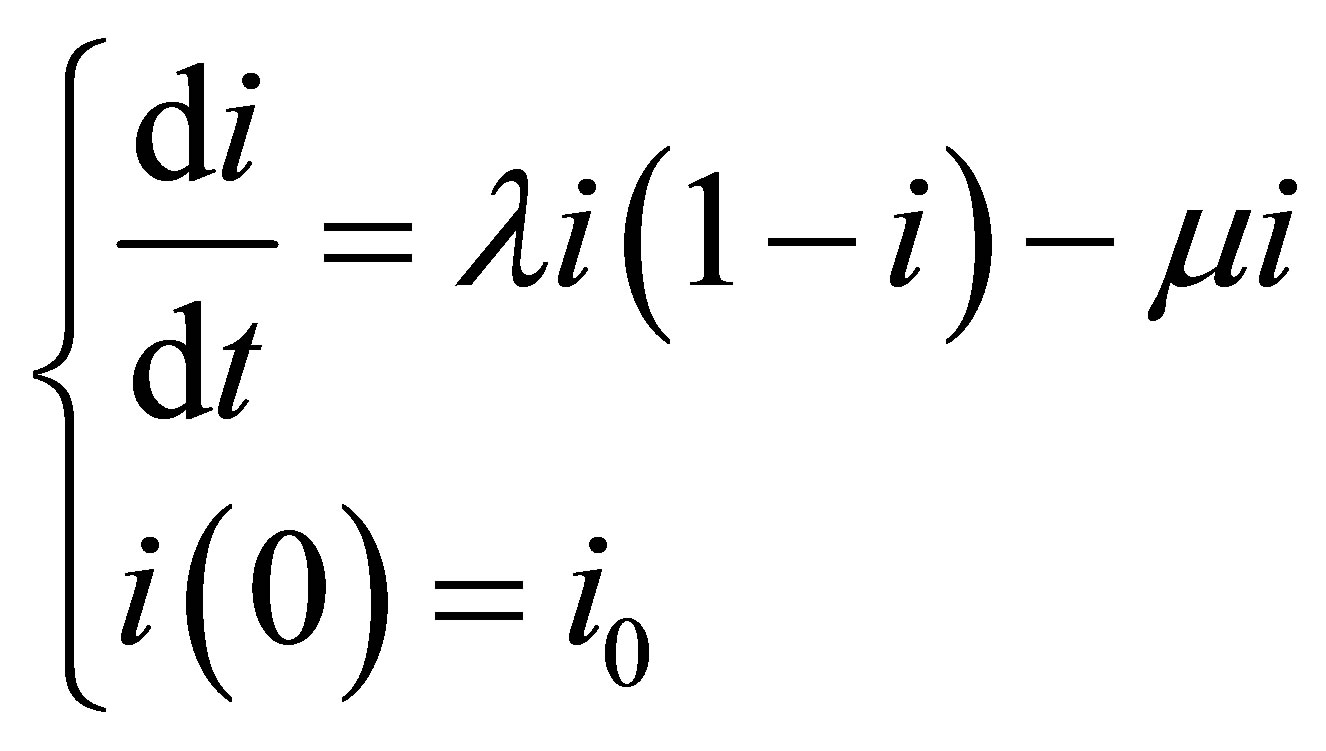(2)

Supposed the rate of patients daily contact is.

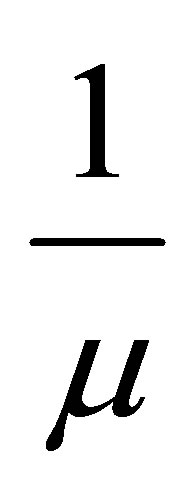
is the period of infection,

,

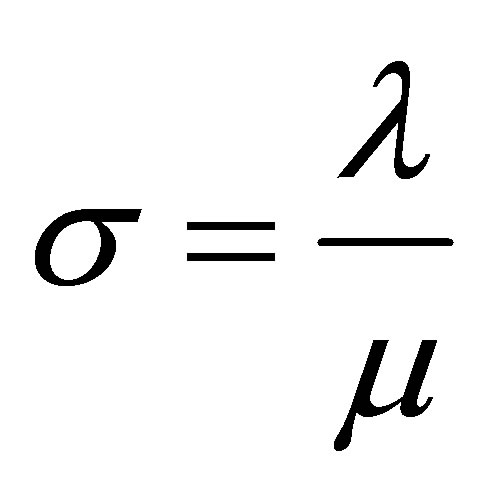is called the contact number and is the number of each patient has contacted effectively during the period of infection.

2.3. Susceptible Infected Recovered Model

Assumed that the total number of people is constant N, the proportion of patients, health, and out of proportion is 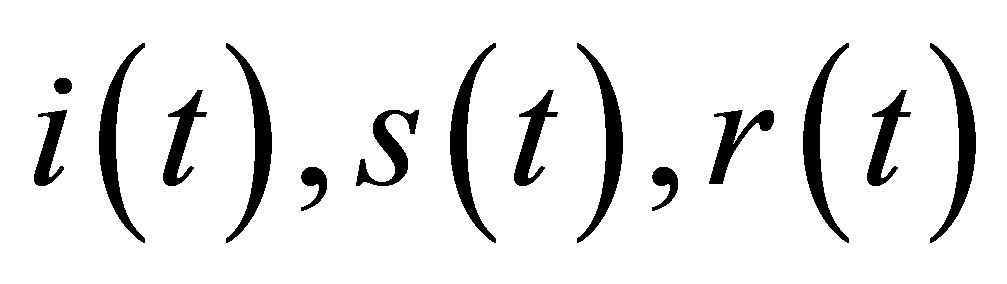respectively. Let the patient’s contact rate be, cured rate be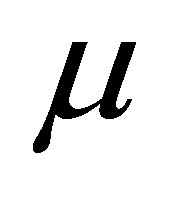, contacted number be

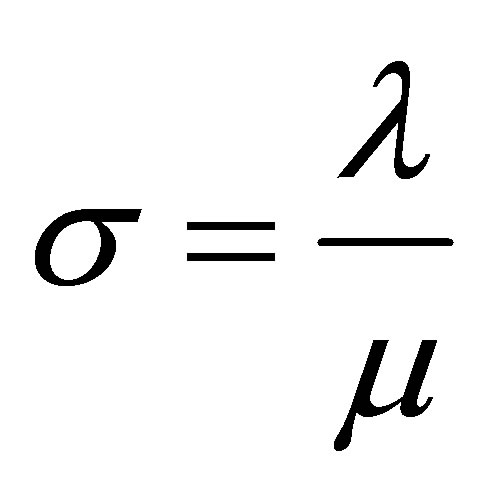, and.

The model is as follows:

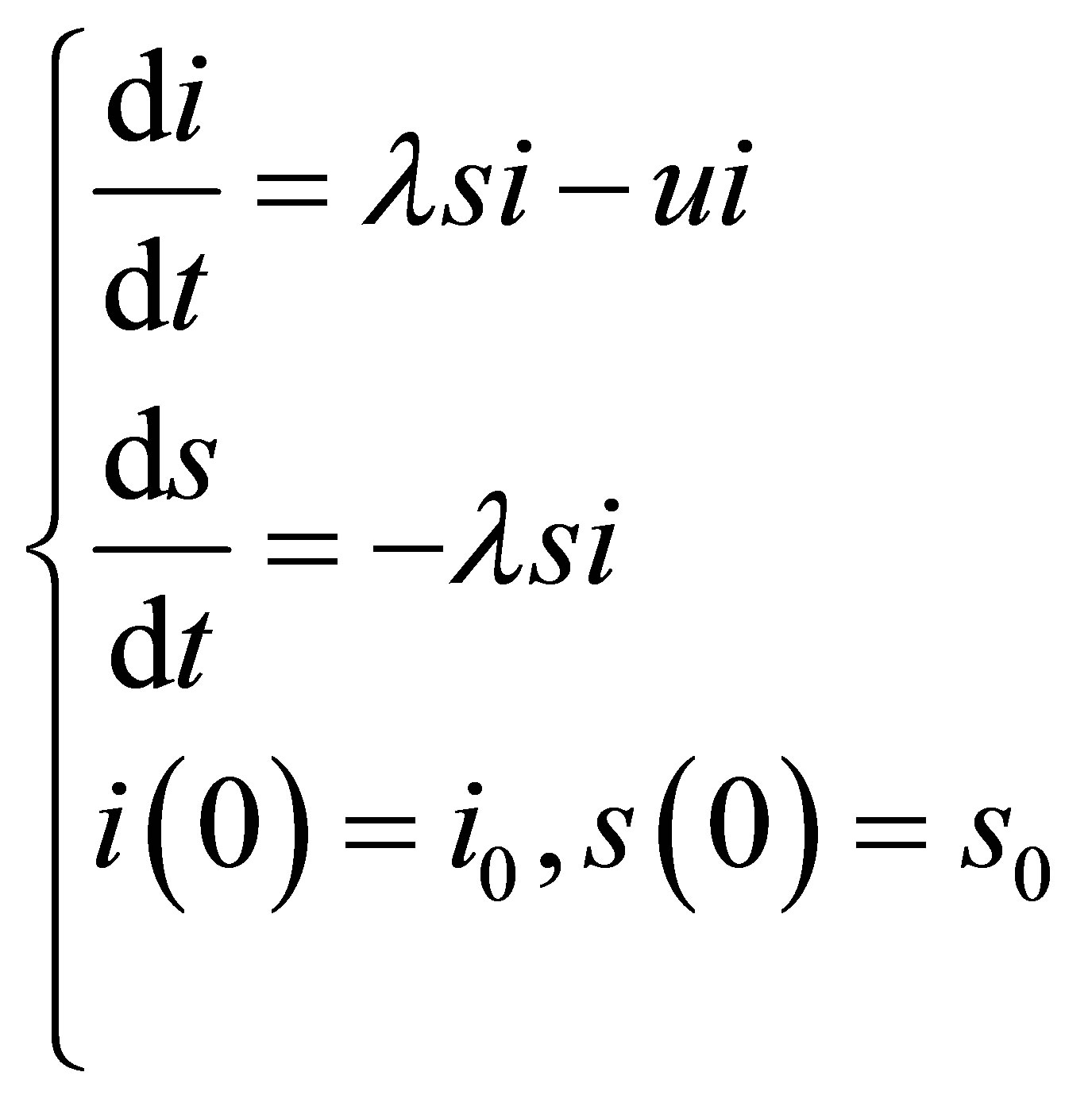(3)

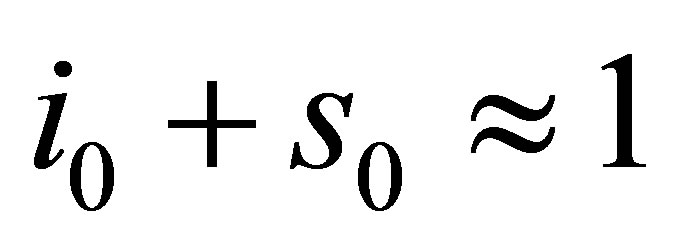(usually 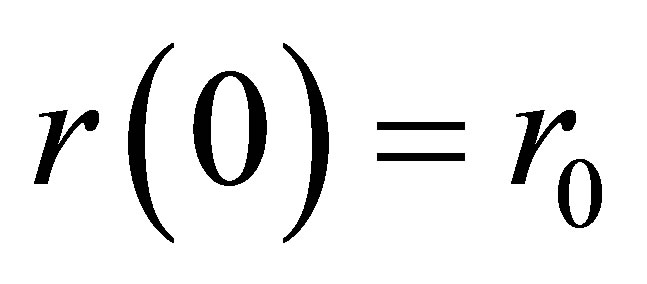is very small).

2.4. Kermachk-Mckendrick Model for Epidemics

Assumption 1: the total number of people in the area we study is constant, not changed along with time.

Let susceptible class be, infected class be, removed class be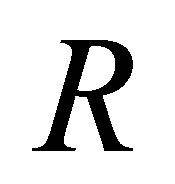. The number of classes be

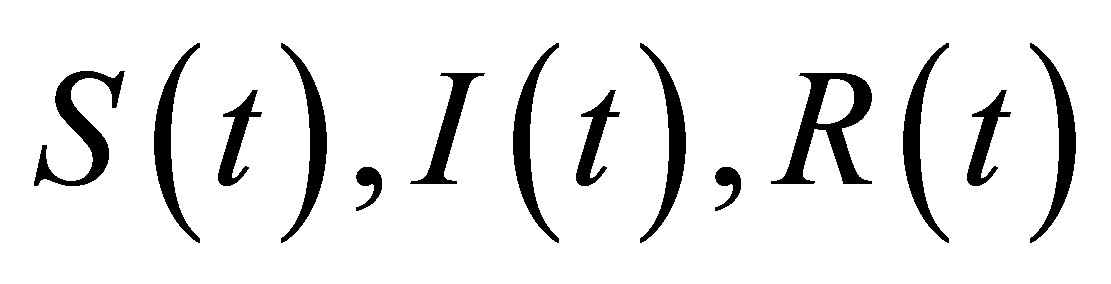represented the class of, , 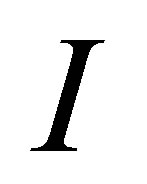at the moment respectively. i.e.,

.

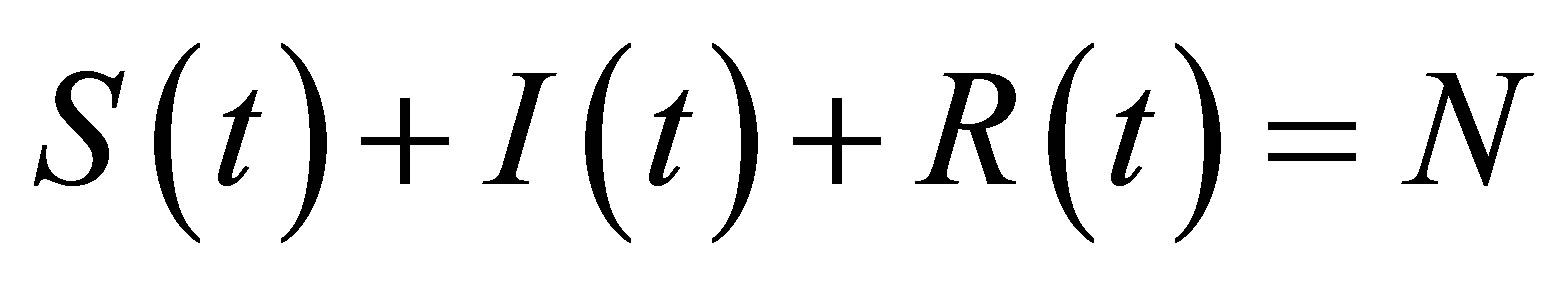Assumption 2: Due to the effects by infectious diseases, the rate of the number of susceptible persons changed along with time is directly proportional to the product of the number of susceptible and infected person.

Assumption 3: Let the speed of the class from the infected person to move out is proportional to the number of infected person at that time.

Assumption 4: Regardless of the natural birth rate and death rate of the population at the area in research time.

The mathematical model of infectious diseases is as follows:

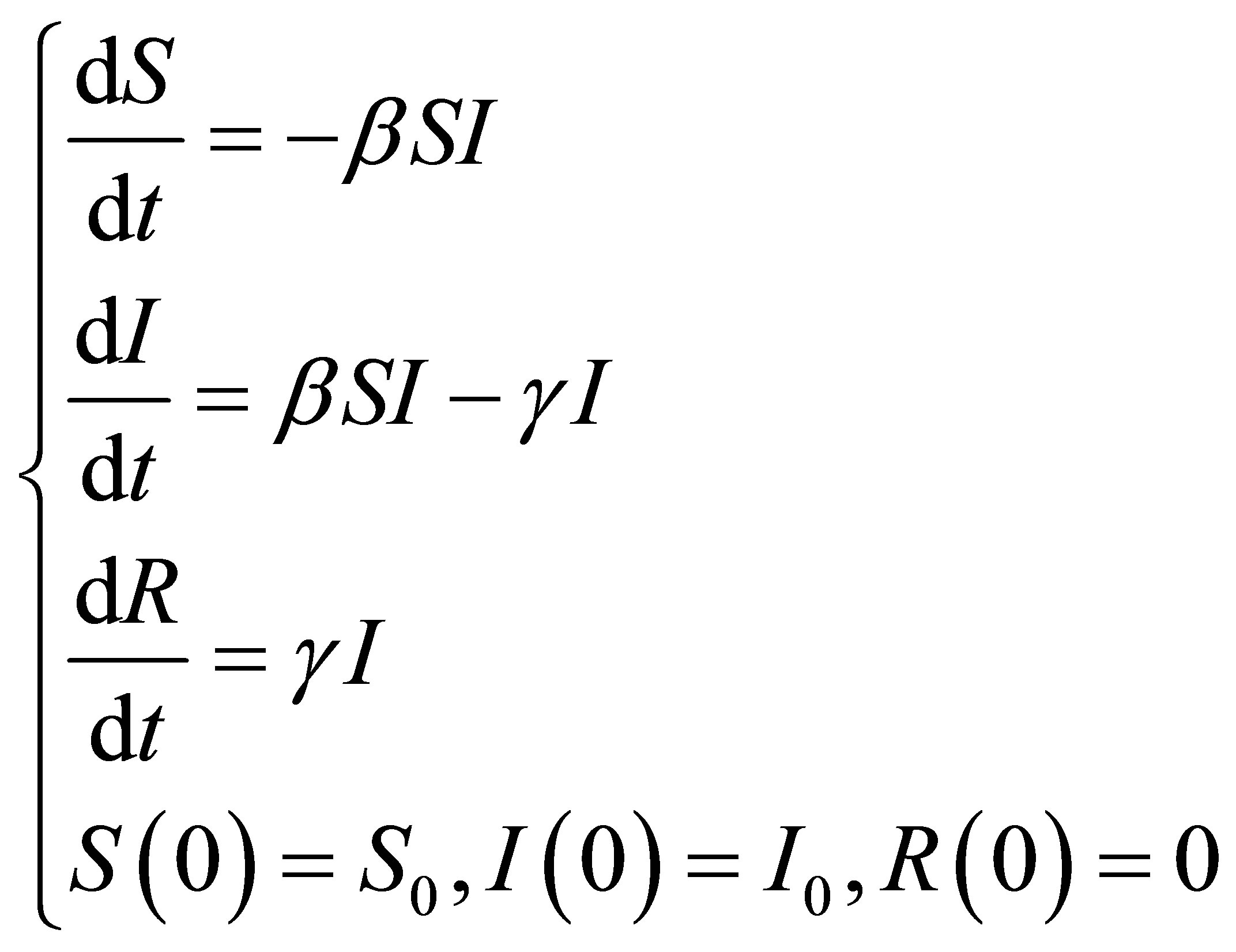(4)

For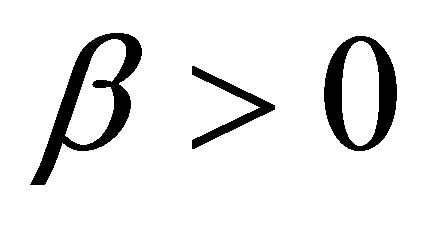,is infectious rate .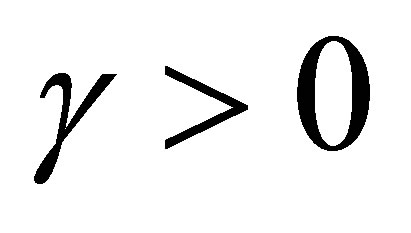, is removal rate,

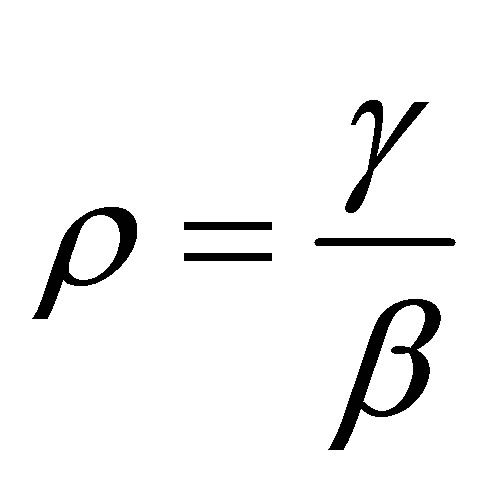
is relative removal rate. Let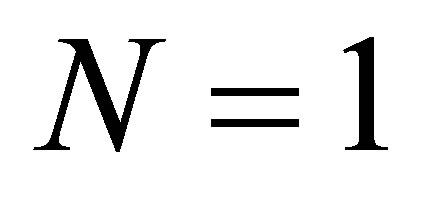, then

.

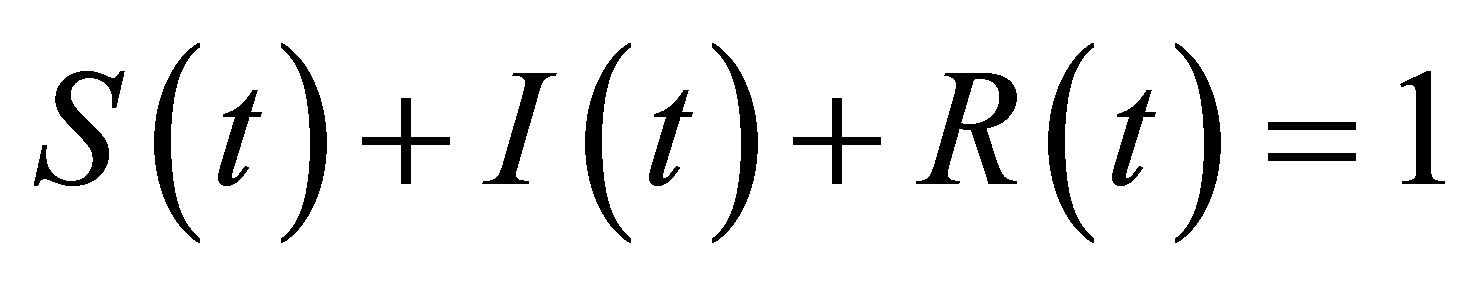By solving the model, we have

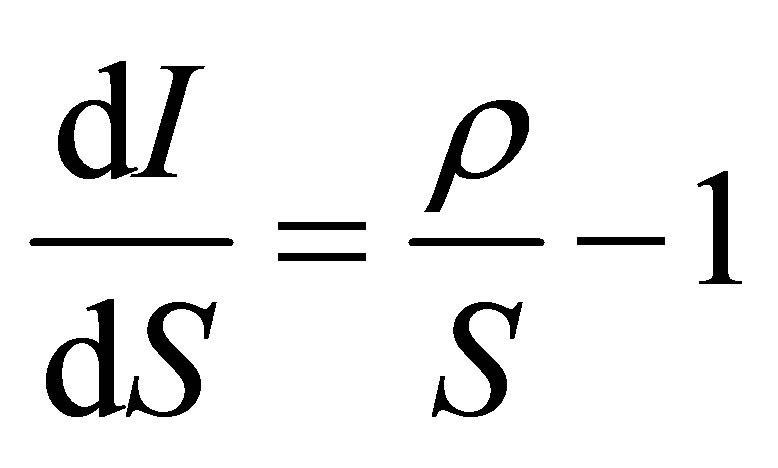in the initial conditions, we have

,

.

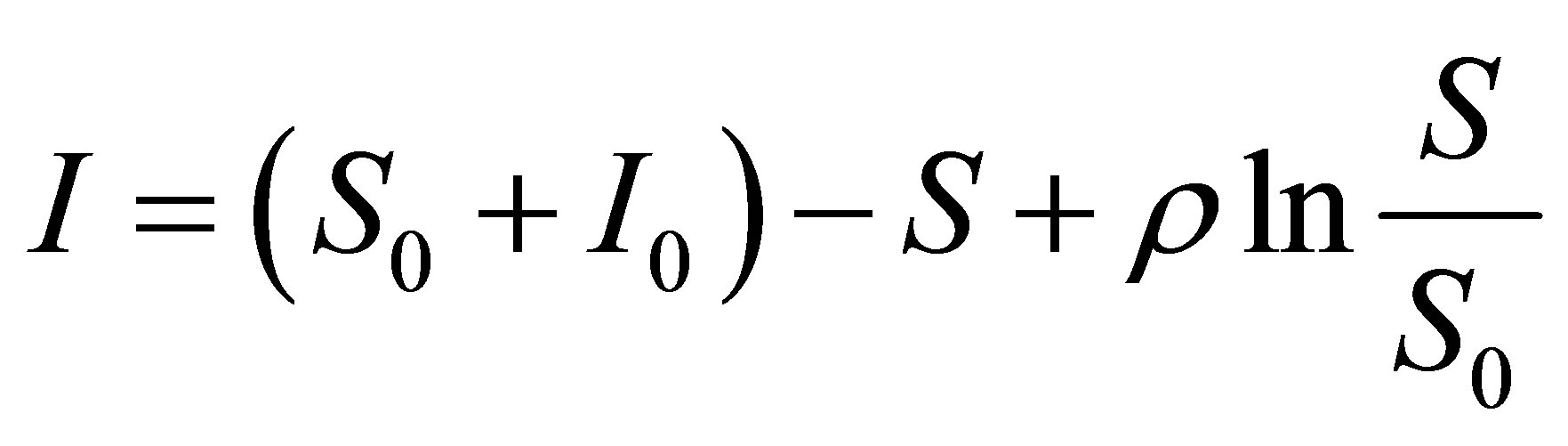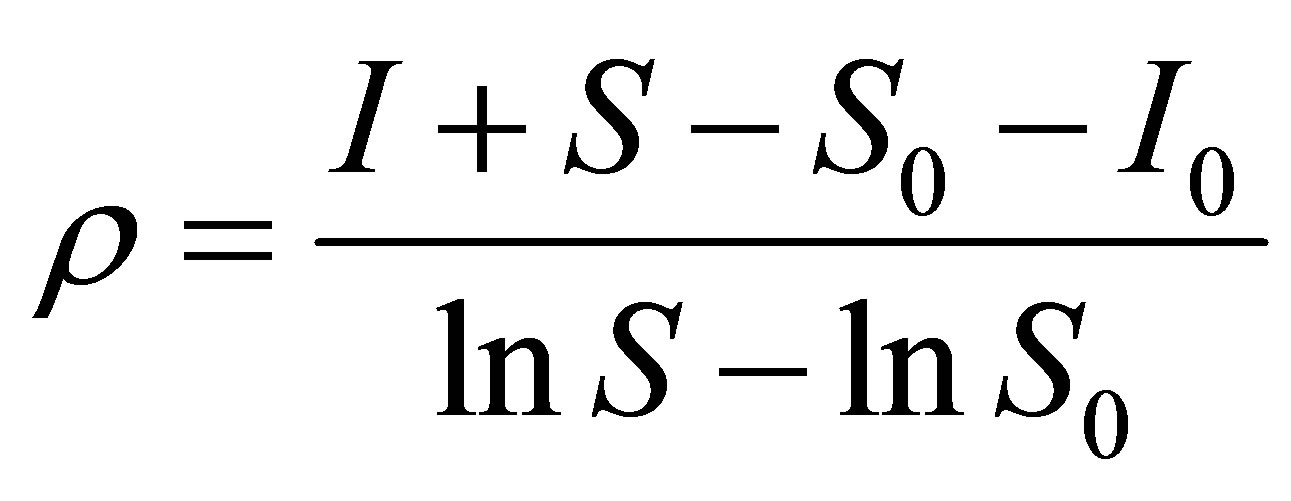when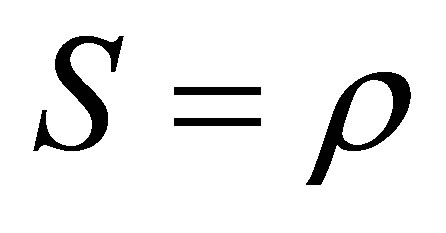, 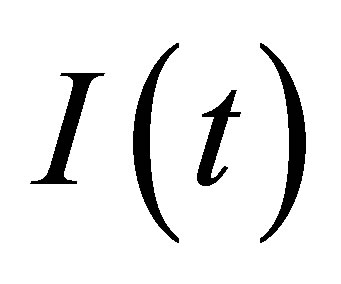can achieve the maximum, and

.

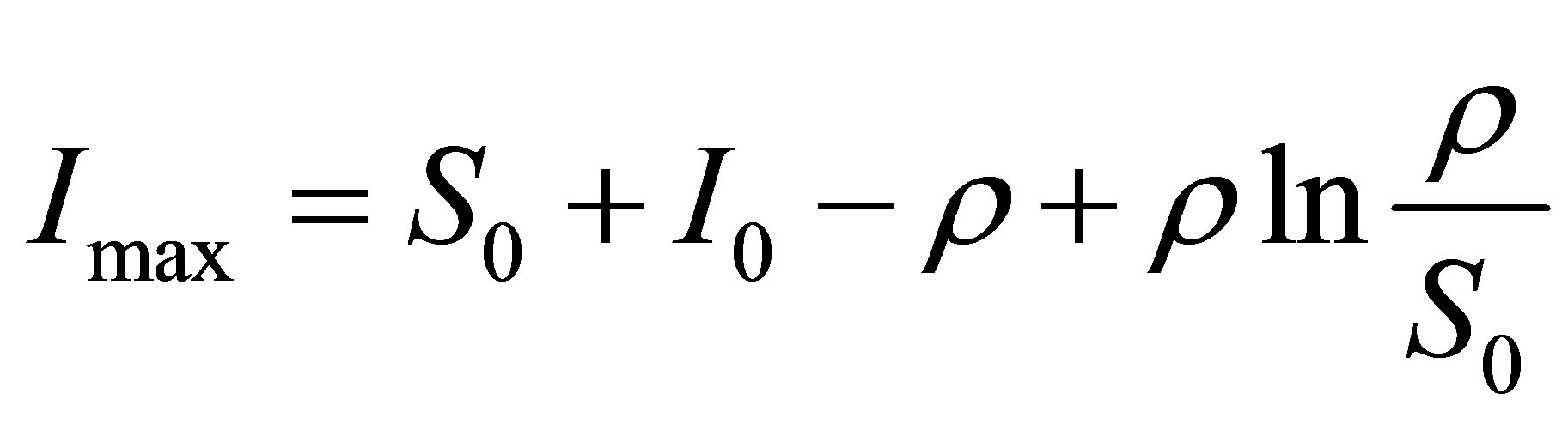Therefore

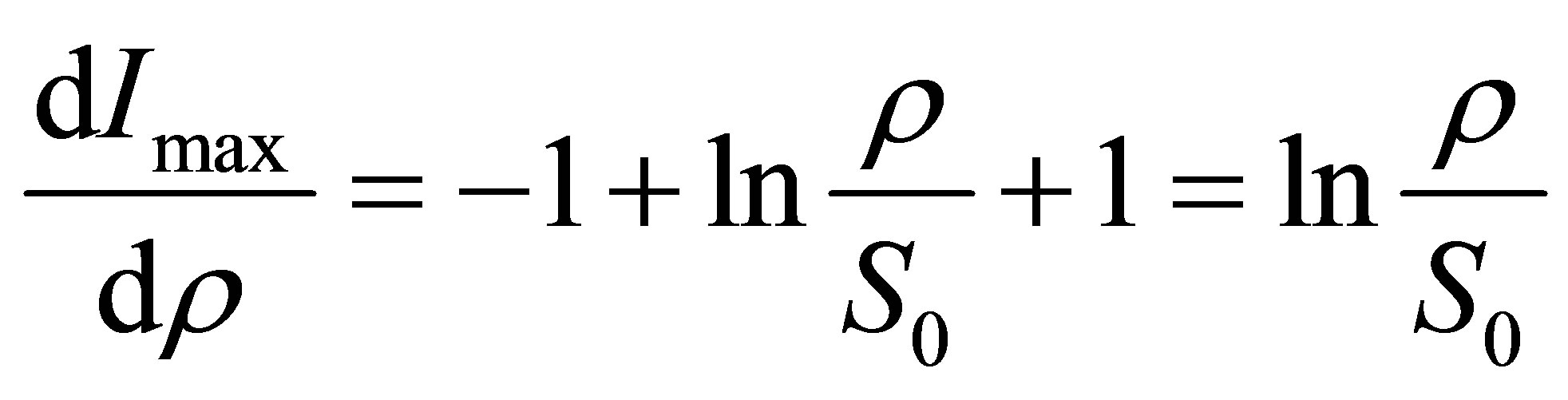
when

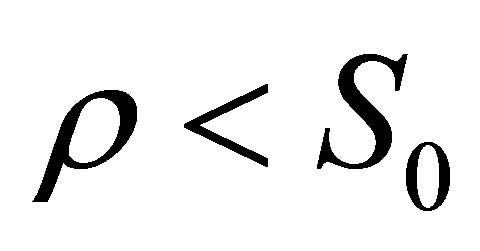, therefore 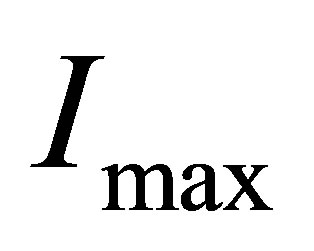is reduced function by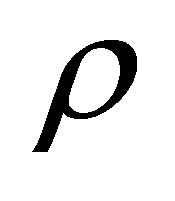. we get the following theorem:

Theorem 1: Let 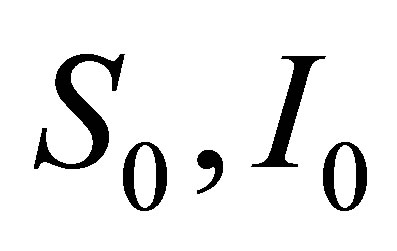be defined, when, the number of infected person 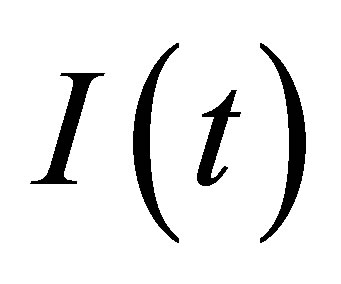can maximize, the maximum is 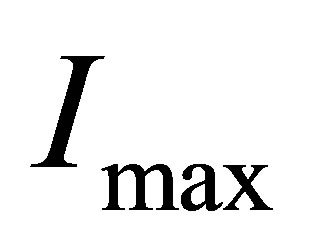and is the reduced function by.

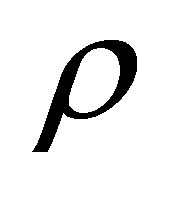From this theorem we know, if we want to reduce, we can complete it by improving 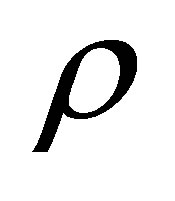, that is to say, we can adopt isolation control to the class. Let the isolation rate be, and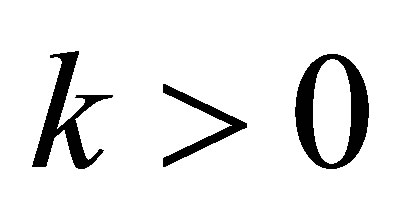, then we obtain the improved Kermachk-Mckendrick model.

3. Improved Kermachk-Mckendrick Model

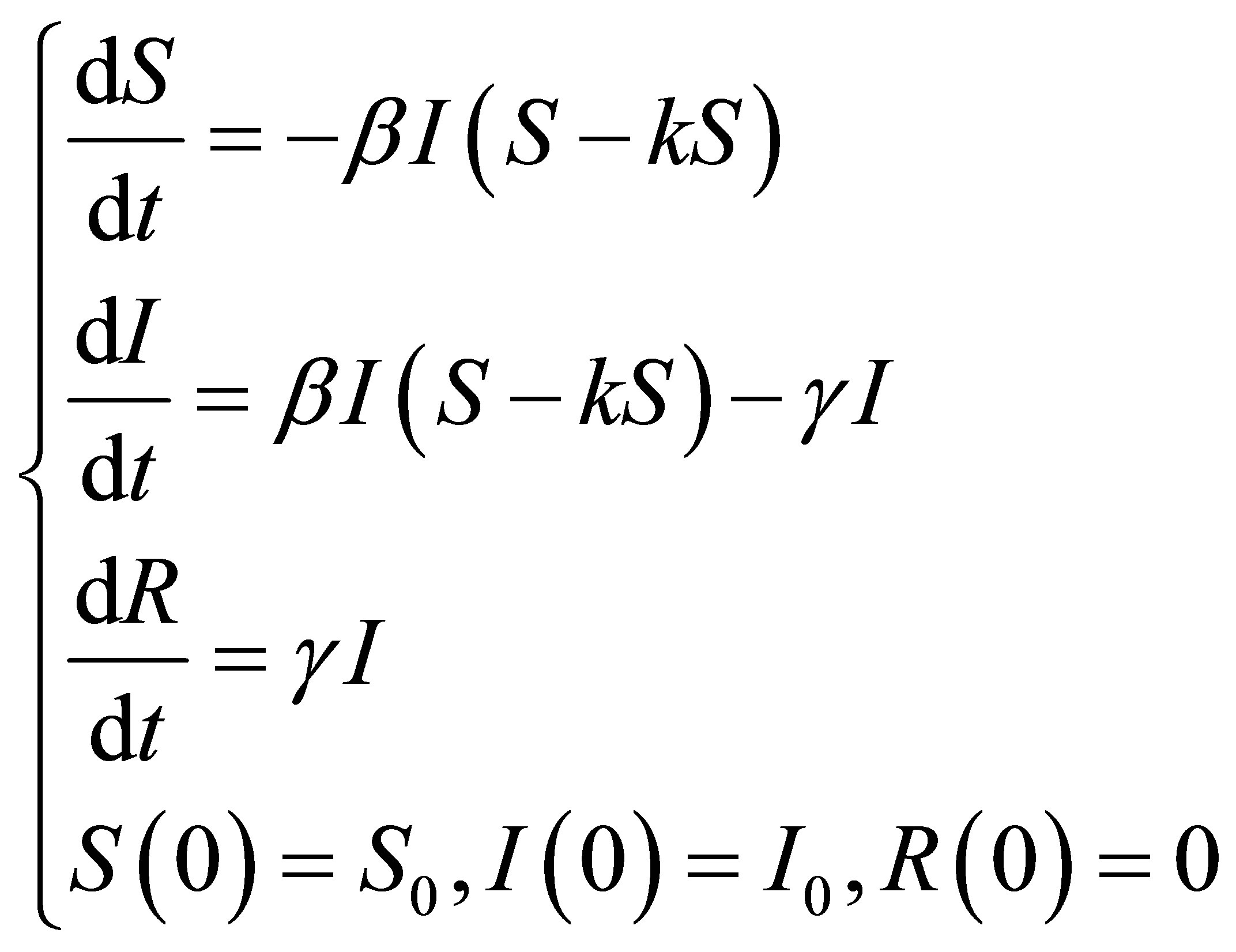(5)

i.e.

Let

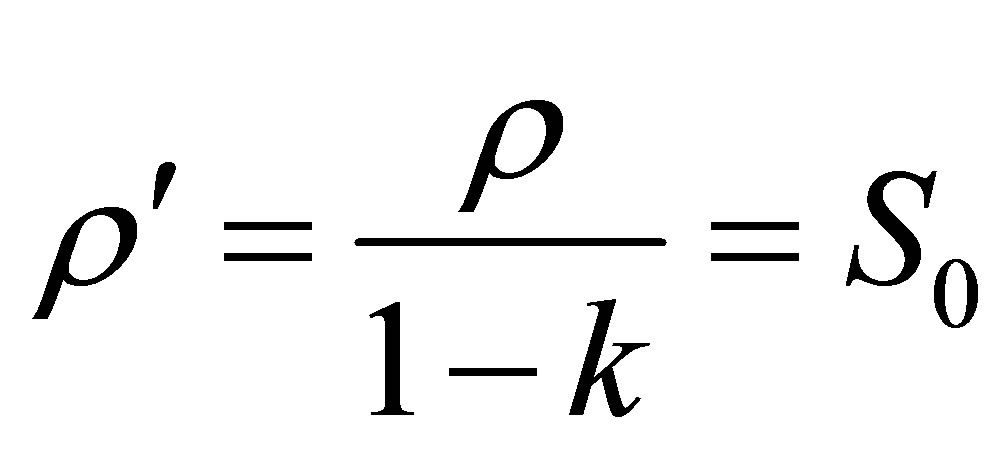then

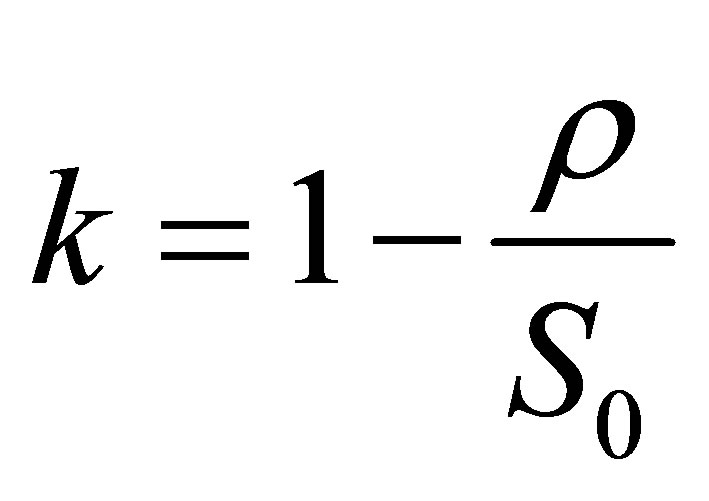
is the isolated rate. By theorem 1, we know

when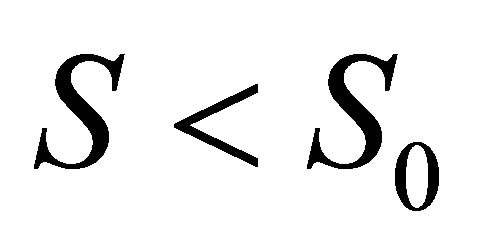. then we obtain the following conclusion:

Theorem 2: For model (5), when

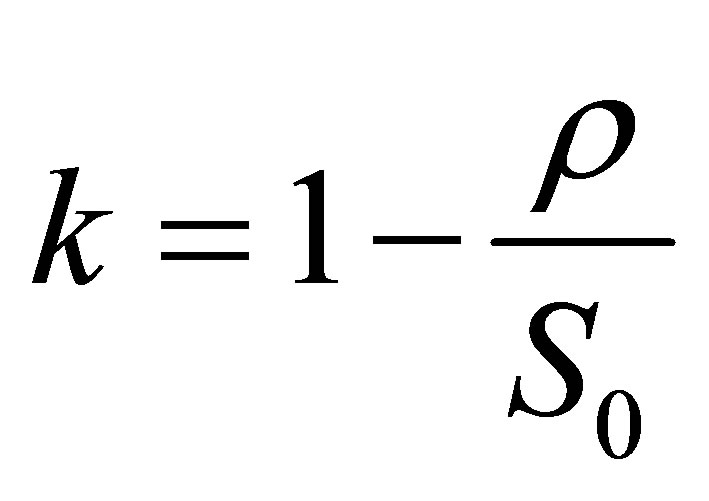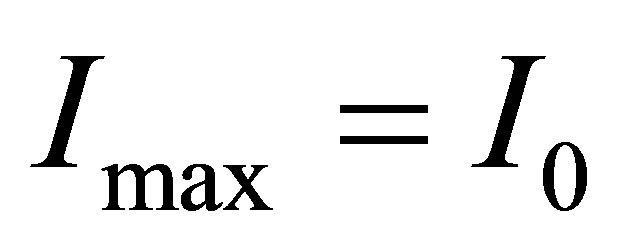then, the number of infected person is decreased along with time, when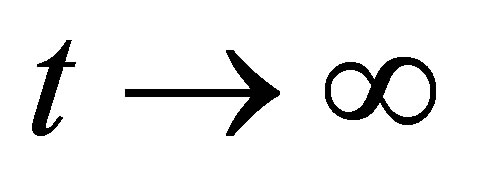, the susceptible infectious disease is eventually disappear. When

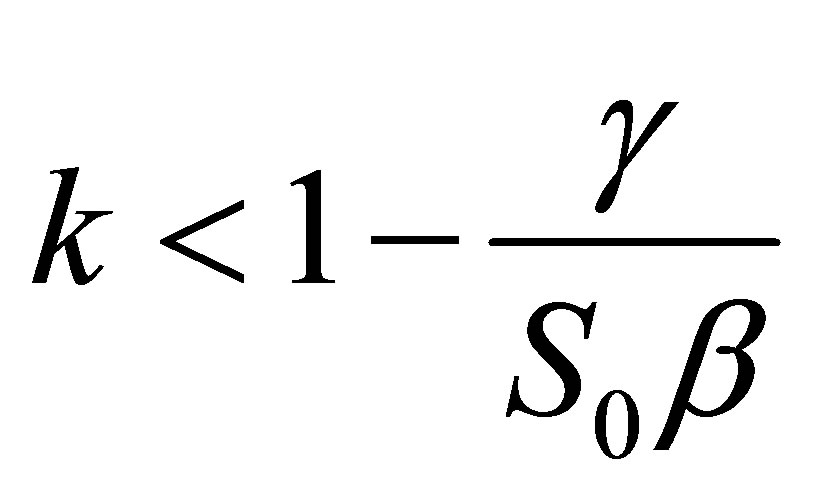the number of 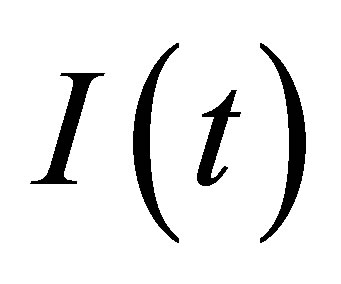still is a peak value.

In a word, applying isolation control of susceptible infectious disease model is the key to prevent the spread of susceptible infectious diseases. The isolated rate must meet some conditions, then we can control.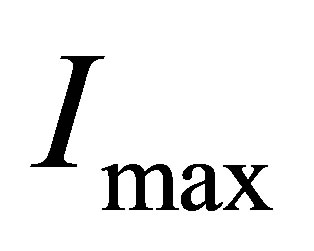

REFERENCES

NOTES

^*Corresponding author.