Journal of Applied Mathematics and Physics
Vol.04 No.03(2016), Article ID:64903,8 pages
10.4236/jamp.2016.43057
Comparative Phenomenological Description of Even-Even Isotopes at Mass Region A ≈ 70
Samir U. El-Kameesy1, Hesham Shahbunder1,2, Karima E. Abdelmageed3, Heba Elwany1*
1Department of Physics, Faculty of Science, Ain Shams University, Cairo, Egypt
2Department of Physics, Collage of Sciences and Humanities, Sattam Bin Abdulaziz University, Kharj, Saudi Arabia
3Department of Physics, Faculty of Science, Benha University, Benha, Egypt

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 25 January 2016; accepted 20 March 2016; published 23 March 2016
ABSTRACT
In the present work the nuclear structure properties and the backbending phenomena of even- even isotopes at A ≈ 70 mass region are analyzed using two simultaneous theoretical models based on a simple modified version of the collective model predictions besides an improved version of exponential model with the inclusion of pairing correlation. In general, both models successfully describe the backbending phenomena in that region. From the comparison between the predictions of the two proposed models a firm conclusion is obtained concerning the superiority of the simple improved version of the exponential model in describing the forward and down- bending region of the φ-ω2 plots.
Keywords:
Energy Levels, Moment of Inertia, Yrast Bands, Backbending, Cr, Ge, Se and Kr Even Mass Isotopes, Collective Model, Exponential Model

1. Introduction
Lately even-even nuclei at mass region A ≈ 70 have recently become important testing ground for most of the advanced theories, where the calculated predictions may be compared with the corresponding experimental data. Previous works showed that there is a clear evidence for a major change in the nature of the ground state levels below I = 18 ћ in even-even nuclei in that region [1] - [8] . Furthermore, at higher spin values a very regular structure develops. It is simply called the backbending phenomenon which occurs as one plots the moment of inertia versus the square of the rotational frequency. These nuclei have several interesting features such as oblate and prolate deformations as well as rapid variations in shape as a function of both spin and mass number. The sudden disappearance of E2 strength at certain spins indicates a shape change that requires the considerations of upper pf configuration [9] .
A crossing of any two bands [in the (E, I) plane] means that at certain critical angular momentum I = IC the energies of the corresponding two states belonging to different bands are approximately equal. In particular, a crossing of any two bands which form a portion of the yrast line leads apparently to a rearrangement in the intrinsic structure in the de-exciting nucleus. Such a rearrangement is sometimes very abrupt.
The band crossing effect looks much more dramatic if the vibrational frequency ω instead of I is used as an independent variable. In such a representation all the important physical quantities as energy, angular momentum, aligned angular momentum and moment of inertia, etc. were discovered experimentally for the first time by Johnson et al., [10] and are often called a backbending effect.
Johnson et al., [10] chose to represent the excitation energies E(I) of the ground-state levels in terms of a plot between the nuclear moment of inertia φ and the squared rotational frequencies ω2. Such plots have revealed that in some cases, φ increases so rapidly with I that ω2 actually decreases as higher spin states are reached, resulting in the appearance of backbending in these plots. That is because the experimental level spacing starts falling below that given by the I(I + 1) rule for E(I).
Well deformed nuclei in their ground states have a moment of inertia which is typically about half of the value expected for rigid rotors. This is interpreted as due to the presence of strong pairing force between the nucleons in the nucleus. With increasing frequency of rotation, the correlations due to the pairing force are reduced as a result of Coriolies anti-pairing effect (the CAP effect) until these correlations disappear at a critical angular momentum. As a result, inertia increases with the rotational angular velocity and is expected to adopt the rigid rotor value at the critical angular momentum. This situation was first predicted by Mottelson and Valatin [11] .
The investigation of Lieder et al., [12] and Fassler et al., [13] indicates that at spin I ≈ 10 - 14, the ground- state rotational band is crossed and mixed with a second (super) band. After the crossing, the members of the superband become the yarst levels. If the interaction between both bands is strong, the mixing of the wave functions in the crossing region is also strong and the phase transition occurs smoothly; no dramatic irregularities in the behavior of φ and the quadrupole moment Q are observed. If the band interaction V is weak, a sudden change in the intrinsic structure occurs which causes a marked increase of the moment of inertia φ (backbending) and a certain decrease of the transition probabilities B(E) (and also the quadrupole moment Q).
Several works have confirmed that backbending could be influenced by the ground state band energy spacing and the pairing gap [14] - [17] . Also, the fact that the moment of inertia is almost doubled and is approaching the value of a rigid rotation suggests that the transition is associated with pair correlation [18] . A large amount of works have been done in studying the antialignment effect of pair correlation on the moment of inertia [17] [19] .
The backbending mechanism of 48Cr has been studied by Hara et al. [5] , making use of the projected shell model [20] . The obtained results proved that the backbending in 48Cr is based on band crossing. This result differs from that of Tanaka et al. interpretation based on the Cranked Hartee-Fock-Bogoliubov (CHFB), which claims that the backbending in the area under investigation is not due to level crossing mechanism [21] .
Furthermore, the pairing force has been considered to have an important role in backbending phenomena but it is not sufficiently outlined [22] . Cranking model analysis of 80Br energy levels reveals the possible existence of neutron alignment at ω = 0.7 MeV [23] .
Many attempts have been performed to provide theoretical description of the backbending phenomena. The variable moment of inertia (VMI) gives a very good description of the ground state bands of even-even nuclei up to the point where backbending occurs [24] [25] . Also, several works utilized the band mixing calculations to describe backbending [26] [27] .
The lack of clear description concerning the backbending phenomena in the A ≈ 70 mass region led us to reinvestigate the phenomena applying a simple five parameter formula based on a dynamic version of the unified collective model. Additionally, an improved version of the exponential model with pairing attenuation has been also applied in the present work [28] . It is hoped by such work to have a good description of the backbending regions besides those of low-lying states.
2. The Modified Version of Collective Model Description
Zvonov and Mitroshin [29] have applied a dynamic version of the unified collective model of nuclei as a universal mechanism forming quasirotational bands in spherical, transitional and deformed nuclei. It holds well for the ground state bands in even-even nuclei 40 ≤ A ≤ 180.
In this model, the energy spectrum of vibrational states with  is given by
is given by
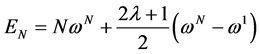 (1)
(1)
where λ is a constant depends on the number of phonons “N” and the spin I, for the yrast bands λ = 2,

where  is a universal constant = 5.5 ´ 10−2 for (40 ≤ A ≤ 190) and
is a universal constant = 5.5 ´ 10−2 for (40 ≤ A ≤ 190) and 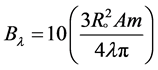
A further improvement of this model is given taking into consideration the possibility that the energy levels of even-even nuclei can be treated as dynamic modes too where the energy EN can be obtained by the following formula:
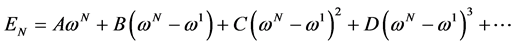 (2)
(2)
where A, B, C and D are constants.
The even power terms in the previous expression are comparable to the so-called Harris expansion for rotational spectra [30] [31] . The odd power terms in Equation (2) could be described as the residual interaction coming from band crossing. Furthermore, Equation (2) is equivalent to the extended variable moment of inertia model to high spin given by Anagnostatos [32] based on the article given by Das and Banerjee [33] .
In that work the energy of states of an even-even nucleus is in the form:
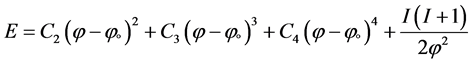 (3)
(3)
where C2, C3, C4 and φₒ are the four parameters of the model; φₒ is the moment of inertia of the first excited state (2+).
In a very pronounced description A. Bohr and B. Mottelson [34] have stated a familiar expression obtained by quantizing the classical Hamiltonian for a symmetric top in the following form:
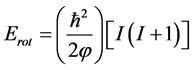 (4)
(4)
where φ is the effective moment of inertia. For sufficiently small values of I, one can employ an expression in powers of 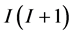 for purely rotational motion as follows:
for purely rotational motion as follows:
 (5)
(5)
where A is the inertial parameter, while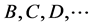 . Are corresponding higher-order inertial parameters. In many cases, the precision of the energy measurements makes possible a determination of higher order terms in the expansion in powers
. Are corresponding higher-order inertial parameters. In many cases, the precision of the energy measurements makes possible a determination of higher order terms in the expansion in powers . If the energy is expressed as power series in the rotational frequencies (ω) rather than in the angular momentum (I), it is found that a greater simplicity and improvement in the rate of convergence could be obtained [34] . Furthermore, the dependence of the moment of inertia on the collective parameters also gives rise to a coupling between the rotational motion and the vibrational excitation associated with the oscillations in these parameters. As a consequence, there is mainly a competition between combinations of rotational and vibrational motions inside the nucleus.
. If the energy is expressed as power series in the rotational frequencies (ω) rather than in the angular momentum (I), it is found that a greater simplicity and improvement in the rate of convergence could be obtained [34] . Furthermore, the dependence of the moment of inertia on the collective parameters also gives rise to a coupling between the rotational motion and the vibrational excitation associated with the oscillations in these parameters. As a consequence, there is mainly a competition between combinations of rotational and vibrational motions inside the nucleus.
Based on the present proposed dynamic version (Equation (2)), the aforementioned discussion concerning the rotational-vibrational motion and the previously predicted model given by Anangnostatos [32] (Equation (3)), an improved relation could be stated by adding a term ( ) representing the rotational contribution to the nuclear motion as follows:
) representing the rotational contribution to the nuclear motion as follows:
 (6)
(6)
where F is the inertial parameter and in the same time measures the weighted magnitude of the rotational contribution.
3. The Improved Exponential Model Description
Sood and Jain [35] have previously developed an exponential model based on the exponential dependence of the nuclear moment of inertia on pairing correlation [18] . They gave the following relation:
 (7)
(7)
For medium light nuclei, Ic can take values smaller than 18 ћ because the backbending phenomenon in that region (A ≈ 70) lies at spin I ≈ 10 ћ [4] . These works led us to use a suitable Ic values to represent both the variation of the moment of inertia and the pairing correlation and to give the model the ability to describe well the φ-ω2 plot region, in particular the forward and down-bending regions.
The modified version of the exponential model with pairing attenuation has the following form [7] [28] :

where φₒ, ∆ₒ and υ are the free parameters of the model, which are adjusted to give a least-square fit to the experimental data. This approach is supported by Ma and Rasmussen suggestion that there is an exponential dependence of the moment of inertia of the parameter υ for a wide range of υ values [36] .
4. Investigation of Backbending via the Applied Models Predictions
The anomalous behavior, i.e. backbending of several medium light even-even nuclei (Cr, Ge, Se and Kr), has been studied using the modified version of the collective model and the improved exponential model. The predictions of the applied models compared with the corresponding experimental results [37] are given in Table 1.
The plots of the calculated data of 2φ/ћ2 versus (ћω)2 for these isotopes are given in Figure 1, where the experimental data are also presented. The excitation energy E(I) of the yrast bands, the moment of inertia and the squared rotational frequency ω2 are deduced by using the well-known relation [28] :


The calculated parameters are given in Table 2 where the root mean square deviation (σ) values of fitting procedure are also included. The mean square deviation is given by:

In Figure 1, the experimental data show a clear evidence of backbending phenomenon in all the presented nuclei at I = 8 − 12 ћ. It is clear from the same figure that the predictions of both the improved exponential and the dynamic collective models describe very well the ground-state levels in Cr, Ge, Se and Kr even mass isotopes up to high spins. Furthermore, the predictions of the applied improved exponential model reproduce very well the backbending phenomenon in those nuclei and its application improves as the atomic mass number increases. This result may give an indication that the pairing force contribution to the backbending phenomenon increases as the atomic mass number increases. Another noticeable success of the model (IEM) is shown in the same figure concerning 68Ge, 72Se and 78Kr and 80Kr where the forward and down-bending regions are very well described by its predictions.
Table 1. Experimental and calculated level energies (keV) of ground-state bands in Cr, Ge, Se and Kr even-even nuclei using a dynamic version (DVM), an improved dynamic version (IDVM) of the collective model and a rather improved exponential model (IEM).
Table 2. The fitting parameters of the dynamic version (DVM), the improved dynamic version (IDVM) of the collective model and the improved exponential model (IEM).
Figure 1. Calculated and experimental moment of inertia 2φ/ћ² vs. (ћω)2 for yrast band level of some light nuclei at A ≈ 70.
5. Conclusions
In the persent study, the application of an improved dynamic version of the collective model along with an improved exponential model based on the pairing correlation gives a fairly accurate description of the high spin states in Cr, Ge, Se and Kr. Furthermore, the applied models give overall satisfactory results concering the description of backbending phenomena. The forward and down-bending regions of φ-ω2 plots are well described by means of the improved exponentional model predictions. In contrary, in acute backbending cases, the im- proved dynamic version model roughly holds so that further microscopic calculations are needed.
As aconsequance, the appearance of the backbending phenomena in medium light nuclei at low spins (I = 8 − 12 ћ) can be interpreted on the framework of the pairing force which supports the band crossing mechansim in analogy with the earlier calculations [5] based on the projected shell model.
Cite this paper
Samir U.El-Kameesy,HeshamShahbunder,Karima E.Abdelmageed,HebaElwany, (2016) Comparative Phenomenological Description of Even-Even Isotopes at Mass Region A ≈ 70. Journal of Applied Mathematics and Physics,04,511-518. doi: 10.4236/jamp.2016.43057
References
- 1. Cameron, J.A., Bentley, M.A., Bruce, A.M., Cunningham, R.A., Gelletly, W., Price, H.G., Simpson, J., Warner, D.D. and James, A.N. (1990) High-Spin States in the Mirror Nuclei 49Cr and 49Mn. Physics Letters B, 235, 239-244.
http://dx.doi.org/10.1016/0370-2693(90)91957-D - 2. Brandolini, F., Lenzi, S.M., Napoli, D.R., Ribas, R.V., Somacal, H., Ur, C.A., Bazzacco, D., Cameron, J.A., de Angelis, G., De Poli, M., Fahlander, C., Gadea, A., Lunardi, S., Martinez-Pinedo, G., Medina, N.H., Rossi Alvarez, C., Sànchez Solano, J. and Svensson, C.E. (1998) Precise DSAM Lifetime Measurements in 48Cr and 50Cr as a Test of Large Scale Shell Model Calculations. Nuclear Physics A, 642, 387-406.
http://dx.doi.org/10.1016/S0375-9474(98)00543-0 - 3. Caurier, E., Zuker, A.P., Poves, A. and Martinez-Piendo, G. (1994) Full PF Shell Model Study of A = 48 Nuclei. Physical Review C, 50, 225-236.
http://dx.doi.org/10.1103/PhysRevC.50.225 - 4. Velázquez, V., Hirsch, J.G. and Sun, Y. (2001) Band Crossing and Signature Splitting in Odd Mass of Shell Nuclei. Nuclear Physics A, 686, 129-140.
http://dx.doi.org/10.1016/S0375-9474(00)00507-8 - 5. Hara, K., Sun, Y. and Mizusaki, T. (1999) Backbending Mechanism of 48Cr. Physical Review Letters, 83, 1922-1925.
http://dx.doi.org/10.1103/PhysRevLett.83.1922 - 6. Abd El-Ghany, S., El-Kameesy, S.U., Khazbk, A. and El-Khateeb, S.A. (2014) Extension of a Dynamic Version of the Collective Model to High Energy States in Even-Mass Ce and Nd Isotopes. Arab Journal of Nuclear Science and Applications, 47, 88-93.
- 7. El-Kameesy, S.U., Alharbi, H.H. and Alhendi, H.A. (2005) Backbending Phenomena in Light Nuclei at A = 60. Frontiers in Nuclear Structure Astrophysics and Reactions, 831, 448-450.
http://dx.doi.org/10.1063/1.2200978 - 8. Raduta, A.A. and Budaca, R. (2011) Semimicroscopic Description of Backbending Phenomena in Some Deformed Even-Even Nuclei. Physical Review C, 84, Article ID: 044323.
http://dx.doi.org/10.1103/physrevc.84.044323 - 9. Cameron, J.A., Rodriguez, J.L., Jonkman, J., Hackman, G., Mullins, S.M., Svensson, C.E., Waddington, J.C., Yao, L.H., Drake, T.E., Cromaz, M., De Graaf, J.H., Zwartz, G., Andrews, H.R., Ball, G., Galindo-Uribarri, A., Janzen, V.P., Radford, D.C. and Ward, D. (1998) Spectroscopy of Cross-Conjugate Nuclei 46Ti-50Cr and 47V-49Cr near f 7/2-Shell Band Termination. Physical Review C, 58, 808-820.
http://dx.doi.org/10.1103/PhysRevC.58.808 - 10. Johnson, A., Ryde, H. and Hjorth, S.A. (1972) Nuclear Moment of Inertia at High Rotational Frequencies. Nuclear Physics A, 179, 753-768.
http://dx.doi.org/10.1016/0375-9474(72)90617-3 - 11. Mottelson, B.R. and Valatin, J.G. (1960) Effect of Nuclear Rotation on the Pairing Correlation. Physical Review Letters, 5, 511-512.
http://dx.doi.org/10.1103/PhysRevLett.5.511 - 12. Lieder, R.M. and Ryde, H. (1978) Phenomena in Fast Rotating Heavy Nuclei. Advances in Nuclear Physics, 10, 1-128.
http://dx.doi.org/10.1007/978-1-4757-4401-9_1 - 13. Faessler, A., Ploszajezak, M. and Schmid, K.W. (1981) Description of High Spin State. Progress Particle and Nuclear Physic, 5, 79-142.
http://dx.doi.org/10.1016/0146-6410(81)90048-X - 14. Hagemann, G.B. and Hamamoto, I. (1992) Interaction Strength of Two Crossing Band. Physical Review C, 46, 838-844.
http://dx.doi.org/10.1103/PhysRevC.46.838 - 15. Wu, X., Gu, J. and Iwamoto, A. (1999) Statistical Properties of Quasiparticle Spectra in Deformed Nuclei. Physical Review C, 59, 215-220.
http://dx.doi.org/10.1103/PhysRevC.59.215 - 16. Zeng, J.Y., Jin, T.H. and Zhao, Z.J. (1994) Reduction of Nuclear Moment of Inertia Due to Pairing Interaction. Physical Review C, 50, 1388-1397.
http://dx.doi.org/10.1103/PhysRevC.50.1388 - 17. Alaseri, S.M., Bakeer, J.H. and Nafie, H.O. (2015) Backbending Phenomena for Some Even-Even Nuclei in Medium Light Region. International Journal of Sciences: Basic and Applied Research, 24, 149-162.
- 18. Bohr, A. and Mottelson, B.R. (1975) Chapter 5-Nuclear Structure Physics. Vol. Π, Benjamin, New York, 212-283.
- 19. Liu, S.X., Zeng, J.Y. and Yu, L. (2004) Particle-Number-Conserving Treatment for the Backbending in Yb Isotopes. Nuclear Physics A, 735, 77-85.
http://dx.doi.org/10.1016/j.nuclphysa.2004.02.007 - 20. Hara, K. and Sun, Y. (1995) Projected Shell Model and High-Spin Spectroscopy. International Journal of Modern Physics E, 4, 637-785.
http://dx.doi.org/10.1142/S0218301395000250 - 21. Sheikh, J.A. and Hara, K. (1999) Triaxial Projected Shell Model Approach. Physical Review Letters, 82, 3968-3971.
http://dx.doi.org/10.1103/PhysRevLett.82.3968 - 22. Sorensen, R.A. (1976) Treatment of the Pairing Force by Cranked HFB: A Model of Back-Bending. Nuclear Physics A, 269, 301-311.
http://dx.doi.org/10.1016/0375-9474(76)90682-5 - 23. Ray, I., Banerjee, P., Bhattacharya, S., Saha-Sarkar, M., Muralithar, S., Singh, R.P. and Bhowmik, R.K. (2000) Structure of Positive-Parity Yrast Band in 80Br. Nuclear Physics A, 678, 258-274.
http://dx.doi.org/10.1016/S0375-9474(00)00335-3 - 24. Gupta, R.K. (1971) Nuclear-Softness Model of Ground-State Bands in Even-Even Nuclei. Physics Letters B, 36, 173-178.
http://dx.doi.org/10.1016/0370-2693(71)90061-X - 25. Mariscotti, M.A.J., Scharff-Goldhaber, G. and Buck, B. (1969) Phenomenological Analysis of Ground State Bands in Even-Even Nuclei. Physical Review, 178, 1864-1866.
http://dx.doi.org/10.1103/PhysRev.178.1864 - 26. Reinecke, M. and Ruder, H. (1976) Ein Teilchen-Rotor-Modell mit VMI-Rumpf für Backbending-Kerne. Zeitschrift für Physik A Atoms and Nuclei, 279, 93-97.
- 27. Goodman, A.L. and Goswami, A. (1974) Extension of the Variable Moment of Inertia Model to Large Values of Angular Momentum and Explanation of the S Shape of the g vs ω2 Curve. Physical Review C, 9, 1948-1953.
http://dx.doi.org/10.1103/PhysRevC.9.1948 - 28. Alhendi, H.A., Alharbi, H.H. and El-Kameesy, S.U. (2006) Application of Improved Exponential Model to Some Nuclei in the Region A = 100-192. Journal of Physics G: Nuclear and Particle Physics, 32, 93-99.
http://dx.doi.org/10.1088/0954-3899/32/2/003 - 29. Zvonov, V.S. and Mitroshin, V.E. (1977) Structure of Quasirotational Bands of 72Se and 74Se. Annual Conference on Nuclear Spectroscopy and Nuclear Structure, Tashkent, 10, 468-472.
- 30. Hu, Z.X. and Zeng, J.Y. (1997) Comparison of the Harris and ab Expressions for the Description of Nuclear Superdeformed Rotational Bands. Physical Review C, 56, 2523-2527.
http://dx.doi.org/10.1103/PhysRevC.56.2523 - 31. Harris, S.M. (1965) Higher Order Corrections to the Cranking Model. Physical Review, 138, 509-513.
http://dx.doi.org/10.1103/PhysRev.138.B509 - 32. Anagnostatos, G.S. (1980) Comments on Extension of the Variable Moment of Inertia Model to High Spins. Physical Review C, 22, 305-308.
http://dx.doi.org/10.1103/PhysRevC.22.305 - 33. Das, T.K. and Banerjee, B. (1973) Extension of the Variable Moment of Inertia Model to High Spins. Physical Review C, 7, 2590-2592.
http://dx.doi.org/10.1103/PhysRevC.7.2590 - 34. Bohr, A. and Mottelson, B.R. (1975) Chap 4. Nuclear Structure Physics. Vol. 2, Benjamin, New York, 23-24.
- 35. Sood, P.C. and Jain, A.K. (1978) Exponential Model with Pairing Attenuation and the Backbending Phenomena. Physical Review C, 18, 1906-1916.
http://dx.doi.org/10.1103/PhysRevC.18.1906 - 36. Ma, C.W. and Rasmussen, J.O. (1974) Exponential Dependence of the Nuclear Moment of Inertia on Pairing Correlation and the Pairing Stretch Model for Nuclear Rotation. Physical Review C, 9, 1083-1090.
http://dx.doi.org/10.1103/PhysRevC.9.1083 - 37. Tuli, J.K. and Sonzogni, A. (2011) NNDC Data Service. Journal of the Korean Physical Society, 59, 1325-1328.
NOTES
*Corresponding author.




