ABSTRACT

This study introduces an alternative through two phases of goal programming to overcome the existing membership model problem that does not have a specific mathematical method to examine whether the receipt number of members is compatible with the criteria or characteristics that apply for membership through the lexicographic goal programming (LGP) and multi-choice goal programming with utility function (MCGP-U). It is applied for membership artificial data. The results indicate that both goal programming methods could meet the retail loyalty program membership modus operandi.

Keywords:

Lexicographic Goal Programming, Multi-Choice Goal Programming with Utility Function, Retail Loyalty Program Membership

1. Introduction

The rising cost of living in Malaysia is not a foreign thing. Customer’s action on spending regularly at the retail businesses to get the reward offered (as the reward could help in reducing cost of living) through the membership is regarded as less intelligent and only beneficial the trader. Therefore, a new membership model that allows members to spend or take back the value of the money spent by points accumulated at the other outlet is expected to help customers cover the cost of living and increasing their purchasing power. Therefore, membership which involves three categories of members, namely customers, employees and program management (3P) where they can re-enjoy paid fees through profit sharing by redemption services outside retail chains are formed [1] . Effectiveness of the existing membership model [2] [3] were specialized on the suitability of the membership model in economic field. Utility functions applied by existing membership were in the form of multi-attribute function and there are still no specific methods used by researchers to measure or calculate the utility function empirically. Although some researchers [4] - [6] already formed some method to realize this, their methods are not suitable with the membership concept formed. The study found LGP application can meet the priority criteria required in the membership program and combination of LGP [7] and MCGP-U [8] may helpful to solve the problem through membership model. However, their processes were not narrated broadly in the study.

As a whole, this study contributes for a new retail membership or new business cycle, which, despite of easing members’ cost of living, it also could fulfil the members’ need outside the program provider’s outlet based on their preferences (such as buying by using accumulated points at the program providers’ outlet) since nowadays, retailer tend to “trap” their customers intheir business environment (i.e. customers accumulate points from buying goods at their store and have to redeem their rewards also at the store). However, the integrated goal programing used for the membership model could be a new alternative for the decision makers, marketing expert and loyalty program provider to measure the effectiveness of retail membership loyalty program developed by their institution based on members’ preferences (using utility function) and benefit sharing with the members (such as business profit and reward provider accessibility). Integrated goal programming also could be a simple way (compared to previous re- search) in “computing” the utility function.Thus, this study shows how the new membership model was formed based on the existing membership model in Section 2. In Section 3, we describe a detailed introduction to the theoretical of LGP and MCGP-U, the practicality of both method and its application through membership model developed and current membership features. The study concludes with a summary in Section 4.

2. Membership Model

Fundamental of existing membership [3] [7] [9] were based on formation of utility function in order to measure member’s satisfaction through several factors such as membership size (number of members sharing same benefit), intensity consumption (frequency of facility consumption assumed to bring satisfaction) and type of facility offered. In order to form a membership model which emphasized on the heterogeneity of the member’s demand through diversity of rewards offered, we choose a mixed club membership by Konishi, [2] as a reference which could be formulated as follows:

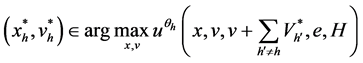(1)

where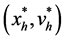, private good vector and 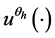are based on utility function that could be depicted as follows:

(2)

where x, consumption of private good, v, intensity consumption vector (in hours), V, aggregate intensity consumption vector by all members in club, e, profile facility and H, number of members in club.

Even though mixed club emphasized on heterogenous feature, the model is not considering lifetime membership. So, Konishi’s mixed club feature were modified and applied to no lifetime membership as an intergenerational club membership concept by Sandler [3] which could be formulated as follows:

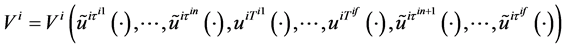(3)

where multi-period utility function for member and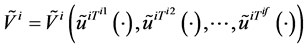, multi-period utility function for non-mem- bers.

Integration of both membership models was applied to modified existing retail membership concept. Therefore, members could redeem their reward outside membership program provider’s outlets. The new membership model was formulated as follows:

(4)

subject to, where, membership profit sharing, 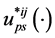, utility function for i-th member, , membership period at the early of membership card ownership, 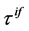, lifetime until member’s end of life.

Equation (4) were subject to a utility function, 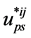, which could be written as:

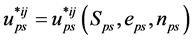(5)

where, membership size, , membership profile for i-th member, 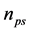, reward redemption service provider profile.

In order to examine the effectiveness of membership model formed empirically, two phase goal programming which involved LGP and MCGP-U were conducted. Its theoretical and practical significance of the goal programming integration will be discussed thoroughly in the next section.

3. Membership Model Examination

3.1. Goal Programming

Goal programming (GP) is a method that often used by the decision makers to solve their problem since introduced by Charnes and Cooper, [10] . Before that GP was extended through multiple objective goal programming (MOGP) [11] , followed by Ignizio [12] by GP method based on priority through lexicographic GP (LGP), and Chang [8] through multi-choice goal programming with utility function (MCGP-U). Since it was developed, GP is widely used as a technique to solve multiple objective problems. Some studies also applied this method for their case study with various issues such as education, library system and transportation problem [13] - [16] .

In this study, in order to fit in the membership model developed in Section 2, firstly, we apply LGP, which are based on the idea that the decision makers (DM) are interested in minimizing the value of unachieved goals for the interest goals lexicographically [17] . Hence, LGP is based on priority according to its level of achievement that are not dependent on each other. Through this technique, the achievement (in the form of excessive achievements and unachieved goals) for each goal can be identified. The classic LGP model was introduced by Ignizio [12] are defined as follows:

Definition (Tamiz, [18] ): A lexicographic minimization defined as a sequential minimization of each priority whilst maintaining the minimal values reached by all higher priority level minimizations.

The algebraic representation of LGP is given as:

(6)

subject to

(7)

where, j-th decision making variable, , coefficients in i-th goals or rigid constraint, and are respectively the negative and positive deviation for goal i, is the right hand side rigid constraint for i oraspiration goal for i, a is the achievement vector for the LGP and is where is usually a linear function of the weighted, unwanted deviation variables at priority level k and K is the lowest priority level.

At the second stage of membership effectiveness examination, LGP was integrated with MCGP-U [8] . Development of the MCGP-U theory for this study are based on previous researches [8] [16] .

Definition 1: The utility function can be viewed as which assign a real number to every outcomein order to show that it captures 3P’s preferences based on the desired goals of the objectives, where is the feasible points and is the set of real numbers.

Right linear utility function (RLUF) used in this study [8] could be depicted as follows:

Proposition 1: P1 and the level of utility achieved in the RLUF (Figure 1) are equivalent or have same optimal solutions.

Proof: approaches to the highest value = 1 (i.e.,) for the utility function (Equation (11)) because should be maximized in the objective function. This forces to approach (from Equation (10)) because the deviations (and) should also be maximized in the objective function. It is obvious that P1’s behaviour and the level of utility achieved, which is as high as possible in the RLUF have the same optimal solutions.

RLUF case: The program provider would like to increase the utility value as much as possible in the RLUF (Figure 1). In order to achieve this goal, value should be as close to as possible. This case can be formulated as follows:

(P1)

(8)

subject to

(9)

(10)

(11)

(12)

(13)

, (F is a feasible set, x is unrestricted in sign).

where and are weights attached to the positive and negative deviations, respectively, and, and. □

However, MCGP-U only based on the utility function that involves a continuous variable with a range of interval values. Therefore, to optimize the utility functions that involve more than one variable (i.e. Equation (4)-(5)), the lexicographical goal programming (LGP) is applied first to the problems created (based on Figure 2) through membership model.

These features of LGP and MCGP-U makes both two methods in goal programming be seen as an appropriate method to be applied to the membership model. This is

consistent with the objective of achieving the maximum satisfaction through the concept of membership. Besides, the membership model consists of utility functions that involve a number of criteria based on the goals by priority (see previous mem- bership model [2] [3] [9] ).

3.2. Goal Programming Integration to Examine Membership Effectiveness

In the first phase (LGP application), Equation (5) in the previous section were considered. LGP problem formed as follows:

(14)

subject to

(15)

(16)

(17)

where, and, respectively i-th member in the membership program in shopper category, , employees, , and membership program management,., and, respectively, where number of reward provider profile whom could be reached by 3P members., and, respectively, were weighted to represent the fulfillment of these 3P categories., the amount of maximum membership fixed by retailer M and, profit distribution for all members.

The second phase (MCGP-U application) act as a solution for Equation (4) after applying the result from phase one. Value of 60% serve as an aspiration value in order to know the members’ satisfaction based on the number of members who remain loyal to a certain period. The mathematical model established as follows:

(18)

subject to

(19)

(20)

(21)

(22)

where j, membership lifetime, and, respectively, positive and negative deviation from retail M membership program, and, respectively, positive and negative deviation for the number of members at period j, , the number of members at period j, , dissatisfaction for goal which assigned to members at period j, , objective value which assigned to the members at period j, , utility assigned to members at period j, , i-th member in the membership program for retail M at period j, and, respectively, upper and lower limit for the number of members at period j.

Equations (6)-(14) are followed closely to the new retail business membership modus operandi (itsmembership heterogeneity and non-lifetime features are modeled mathe- matically as Equation (4) and Equation (5)) as Figure 2.

Profit sharing, shared by the retail business (PS) and number of reward provider profile serves as aspiration level. Results obtained by LINGO software for existing membership (EM) and new membership (NM) shown as follows Table 1:

a. Result of LGP and MCGP-U.

result indicates number of dissatisfied members. However, among, nobody dissatisfied for benefit gained from membership that could be seen as in. and value has zero values, as number of optimum members (number of members who could join the membership through phase one results) are equal to the number of members who remain loyal as members after having some benefits of the membership. Utility value 1 obtained from the results shows that both memberships successfully meet the constraints and conditions.

4. Conclusion

The results coincide with Buchanan [9] argument, which member’s satisfaction relies on number of members whose sharing the benefit. PS value shared also could affect their satisfaction, which supported the formation of a new membership model that was based on profit sharing. Solution based on LGP and MCGP-U could give systematic derived information (loyalty program membership modus operandi followed closely) which may help policy making based on results obtained. Hence, this method allows the measurement of the optimal number of acceptable members when the model is sheltered by some constraints based on the characteristics of the membership model. However, there is some limitation of this study. Each step involved in the GP inte- gration or its algorithm is not described in detail since the authors believe every deci- sion makers has their own retail membership features (that could be applied as LGP and MCGP-U constraints). It is synchronized with one of the authorsʼ aim (instead improving for new retail membership business cycle) for this study, which is to show an alternative to test the membership function in efficient way based on membership mo- dus operandi. The tested data also were small and involve artificial data. We believe that the effectiveness of the membership model developed may be proved convincingly if the data is larger, involves membership program real data or tested by using another programming language software (that could bear for complex constraints and larger data).

Acknowledgements

Authors expressing gratitude and thanks to Ministry of Higher Education Malaysia for MyBrain15 and School of Informatics and Applied Mathematics and Research Management Centre, University Malaysia Terengganu for their sponsorship.

Cite this paper

Safiih, L.M. and Mezral, A.G.A. (2016) Integration of Two- Phase Goal Programming to Examine the Effectiveness of Membership Model. Theoretical Economics Letters, 6, 868-877. http://dx.doi.org/10.4236/tel.2016.65090

References