ABSTRACT

The purpose of this paper is to establish, paralleling a well-known result for definite integrals, the conditional convergence of a family of trigonometric sine series. The fundamental idea is to group appropriately the terms of the series in order to show absolute divergence of the series, given the well established result that the series as it stands is convergent.

1. Introduction

It is well-known that the family of improper trigonometric sine integrals whose coefficients converge steadily to zero form a set of conditionally convergent integrals whenever the integral of the coefficients themselves divergences (see [1]). However, it is also interesting to observe that there is a parallel result for infinite series. The discrete problem requires an entirely different and novel approach, which is presented in this paper. The novelty resides in a detailed understanding of the properties of the greatest integer function as it relates to convergence and in the use of Euler’s function to ascertain a proper uniform lower bound. There is also a well-known number theory result which turns out to be useful.

The following theorem gives the appropriate generalization:

Theorem. Suppose that one has a steadily decreasing sequence of numbers f(n), 0 ≤ n < ∞ ( where n is a nonnegative integer ), such that f(n) tends to 0 as n tends to infinity. Suppose also that the sum of the f(n)’s is infinite. Then

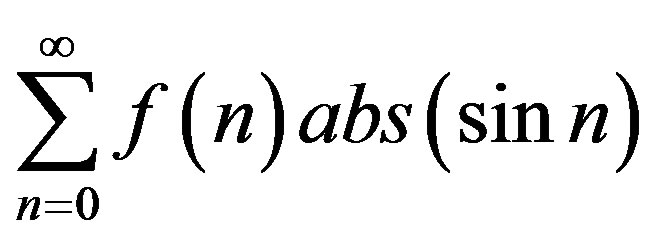(1)

is likewise infinite, where abs stands for “absolute value”. In other words the series

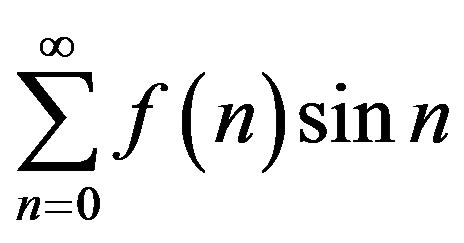(2)

is conditionally convergent.

Proof. First of all it is well known that the series (2) is convergent (see [2]). Next let us observe that sin1, sin2, and sin3 (angles being expressed in radians) are all positive; then sin4, sin5, and sin6 are all negative, etc., the signs alternating essentially in groups of 3 (or perhaps 4 at times). In fact we shall show that we have sequences, , 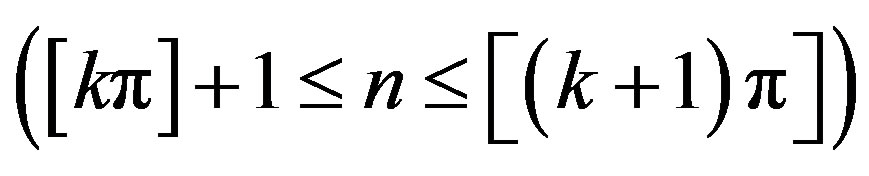0 ≤ k < ∞, with sinn being of constant sign in each sequence and with the brackets denoting the greatest integer function. Indeed we see that

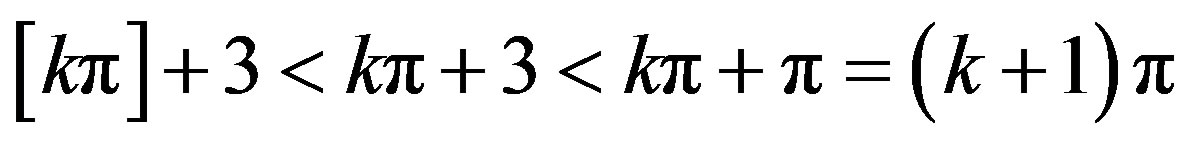so that

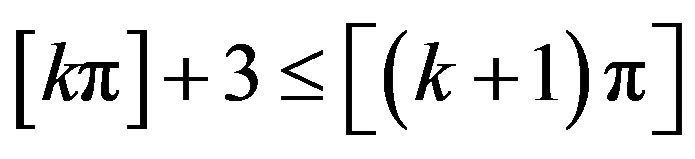. (3)

It follows that both [kπ] + 1 and [kπ] + 2 are values of n whose sines are within the kth sequence. Also, [kπ] + 4 may or may not be a value of n whose sine is within that sequence, but such an event will obtain for an infinite number of values of k (see [3]). On the other hand, we can show that [kπ] + 5 is not a value of n whose sine is in the kth sequence. In fact

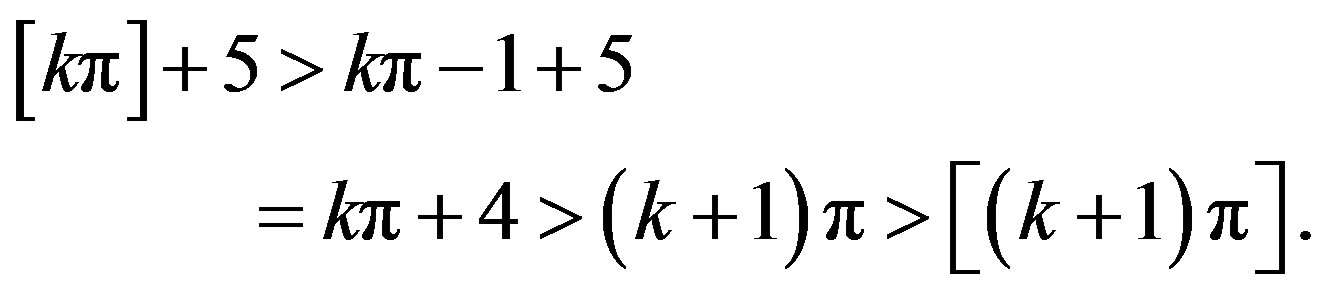(4)

So the kth sequence definitely has either three or four members. In any event it is clear that

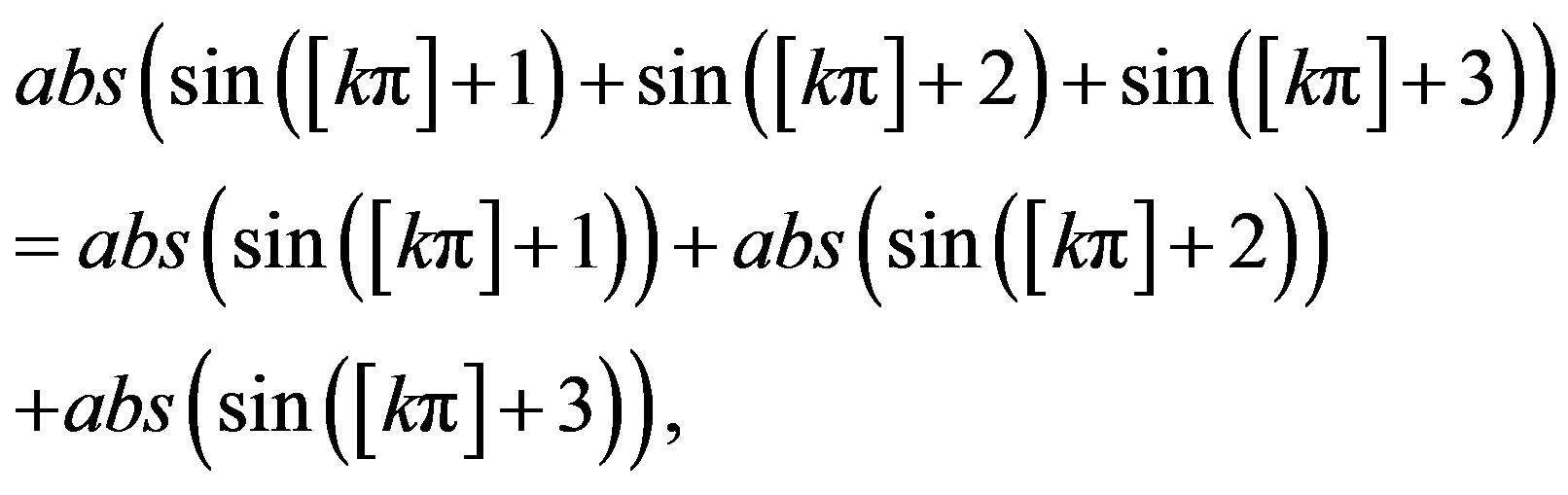(5)

where, as before, abs means “absolute value”.

Observe now that, just in case sin ([kπ] + 4) would appear in a grouping, Equation (5) would certainly provide a lower bound on the sum of the absolute values within that grouping.

Our next step is to use Euler’s formula to obtain a closed form expression for Equation (5). Indeed we have

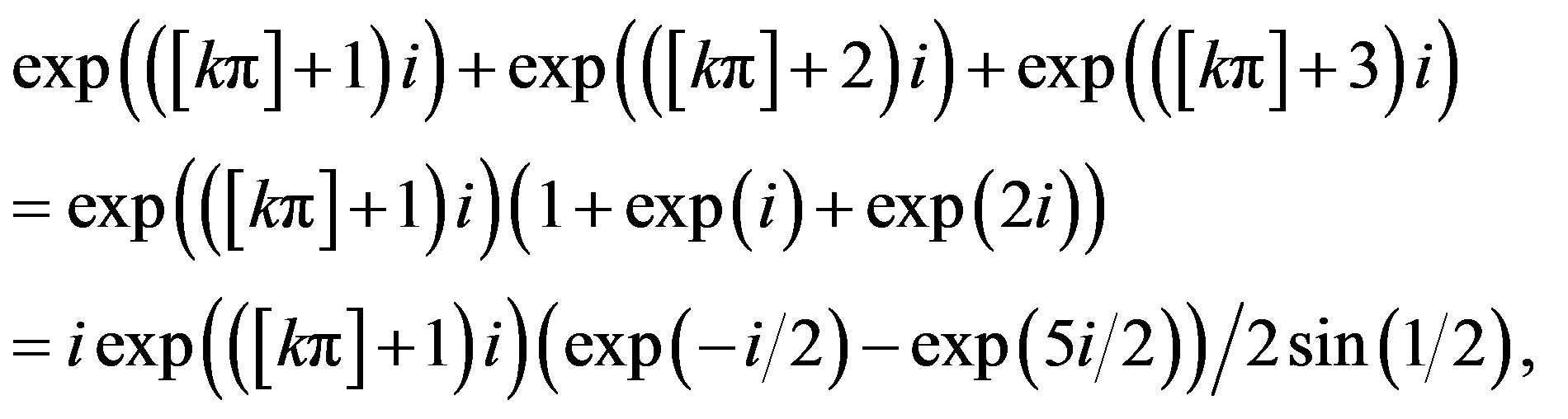(6)

where exp stands for the exponential function. So, in order to determine Equation (5), we need the imaginary part of the right member of Equation (6), which is found to be

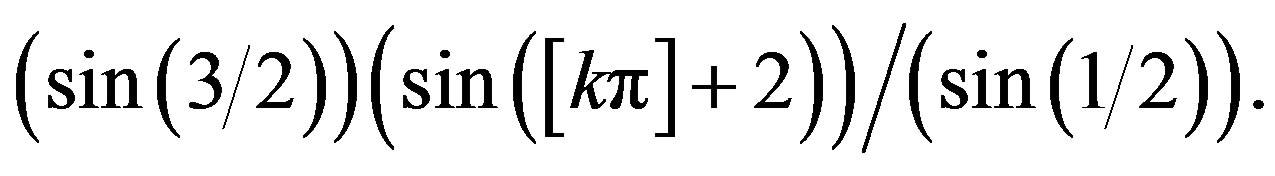(7)

We see that our closed form expression for Equation (5) is the absolute value of Expression (7), which is just

(8)

Next let us determine a uniform positive lower bound for Expression (8), i.e., for all k. Observe that

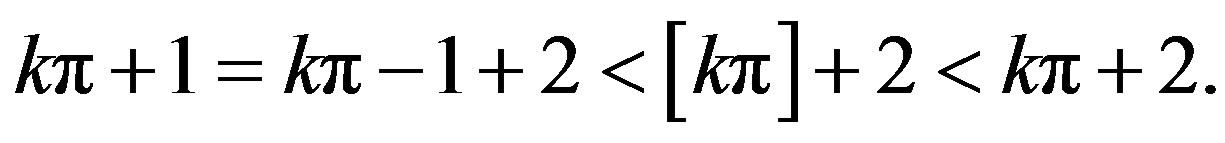(9)

From Expression (9) it follows that abs(sin([kπ] + 2)) lies between sin1 and sin2, sin1 being the smaller of the two. Thus our positive lower bound for Quantity (8) (for all k) is

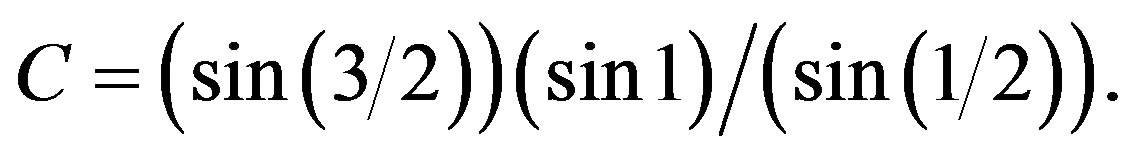(10)

Therefore, since {f(n)} is a steadily decreasing sequence, we assert that

(11)

Our last task is to show that the sum on the right side of Inequality (11) is infinite. On the contrary assume that the sum is finite. Let us examine the sums

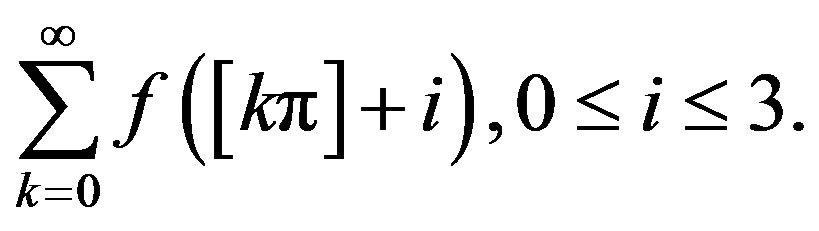(12)

For example suppose that i = 2 or 3. Now

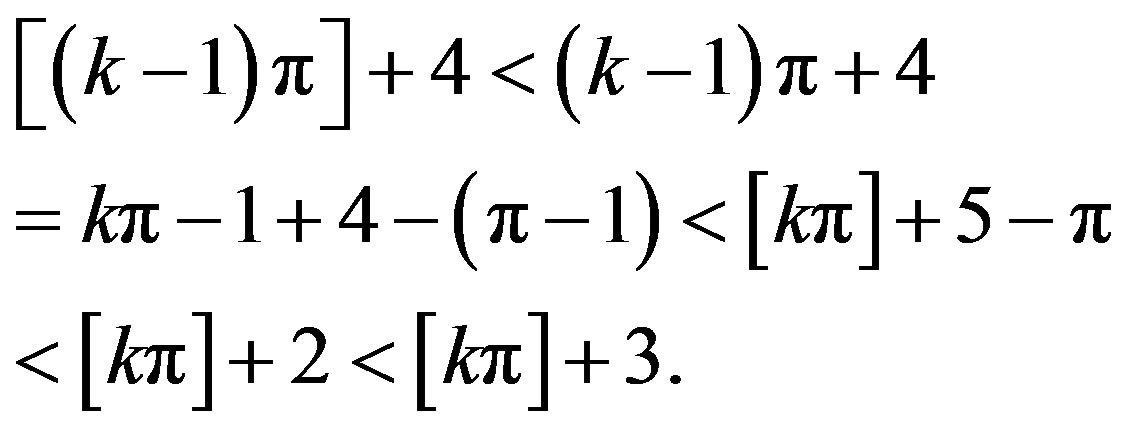(13)

However, then

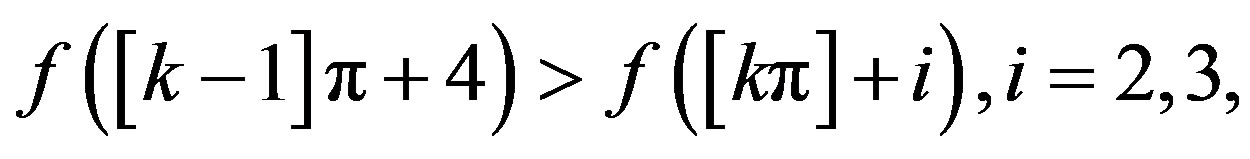(14)

so that, by dominance, (12) converges for i = 2 and 3. Suppose next that i = 0 or 1. In a fashion similar to the development of Expression (13), one has

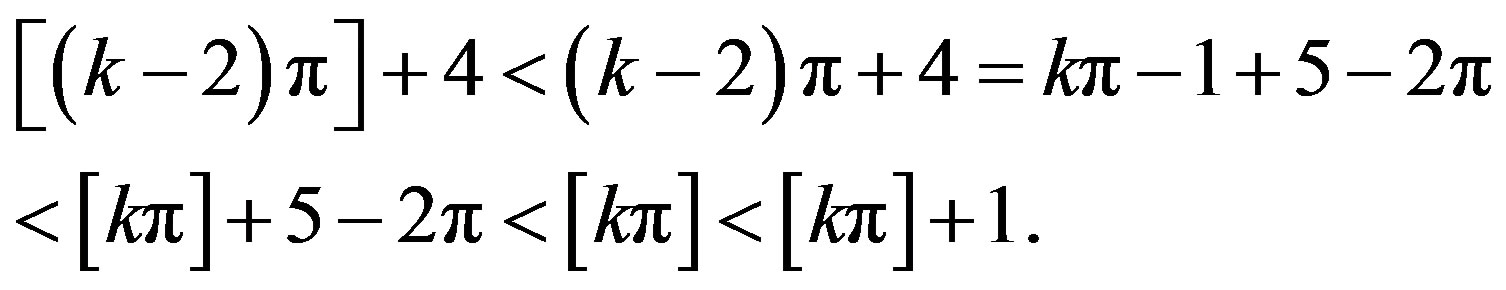(15)

Thus

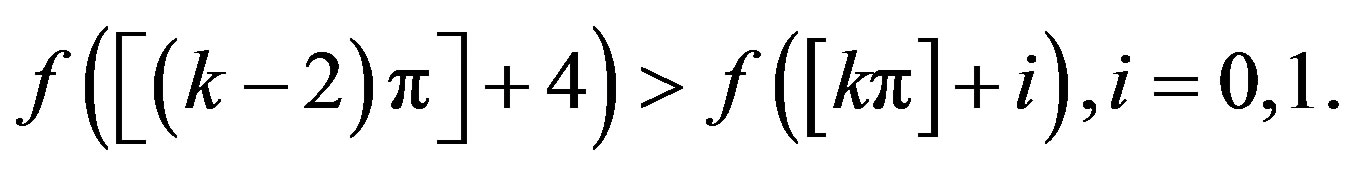(16)

It follows that Quantities (12) converge, and therefore

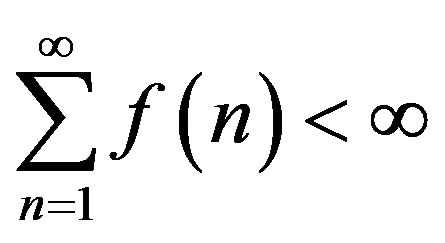
, (17)

in contradiction to the hypothesis of the theorem. Therefore, the series on the right side of Inequality (11) diverges, and the theorem is proved.

Example. Consider

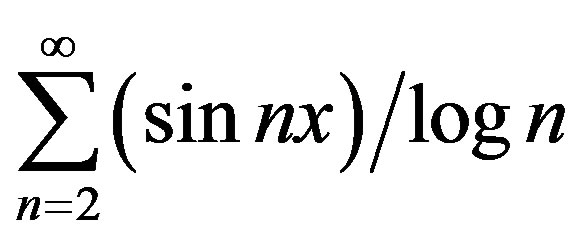(18)

when x = 1. It is clear that 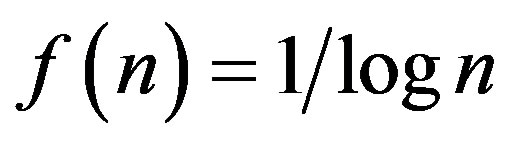is a strictly decreasing function of n and tends to 0 as n tends to ∞. Also, since 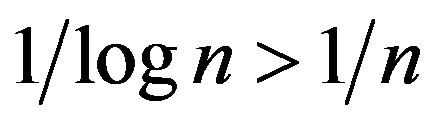and the harmonic series is divergent, so is the sum of the f(n)’s. According to our theorem, this infinite series for x = 1 is conditionally convergent. This also is a classic example of a trigonometric series which is not a Fourier series (see [4]). The underlying reason for that conclusion is that

is divergent, a fact which follows from the well-known integral test since

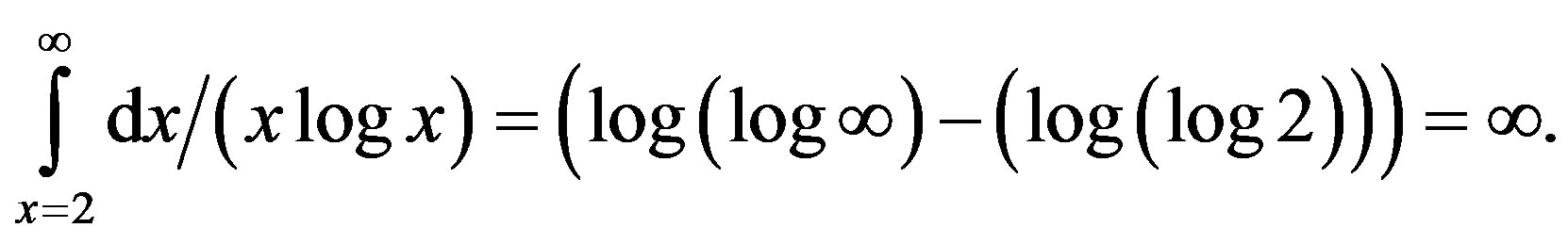
2. Conclusion

Using a novel approach in the discrete case, which employs a well-known result in number theory together with Euler’s formula, we have proved a convergence theorem for infinite series which is a logical parallel to the corresponding integral case involving an oscillating integrand.

REFERENCES