Optics and Photonics Journal
Vol.4 No.8(2014), Article
ID:49278,9
pages
DOI:10.4236/opj.2014.48022
New Approach to Modeling a Solar Cell in Relation to Its Efficiency—Laplace Transform Technique
Mohamed Abdelhady Kamel El-Adawi1, Ibtissam Abdelrahman Al-Nuaim2
1Physics Department, Faculty of Education, Ain Shams University Heliopolis, Cairo, Egypt
2Physics Department, Faculty of Science for Girls, Dammam University, Saudi Arabia
Email: adawish1@hotmail.com
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 13 May 2014; revised 12 June 2014; accepted 11 July 2014
ABSTRACT
Heating a solar cell subjected to incident solar irradiance is studied. Laplace Integral Transform technique is applied to get the temperature field within the cell. The efficiency as a function of the cell temperature is estimated, and its variation with the local day time is thus clarified. Different cooling levels are considered. An illustrative example is given. The results show that the diurnal temperature variation of the cell is significant, while the efficiency is revealed to be a slowly varying function of temperature along the day time. It is revealed that as the temperature of the cell increases the efficiency decreases. Thus shading and cooling conditions may be useful to increase its efficiency.
Keywords:Solar Cell, Efficiency, Temperature Field within a Solar Cell, Laplace Transform Technique

1. Introduction
Manufacturing of photovoltaic energy convertors with higher efficiencies is an important challenge. This topic has aroused the interest of many investigators [1] -[23] .
Heating a solar cell subjected to the incident global solar radiation affects its photovoltaic performance [17] - [23] . The solar p-n cell is a semiconductor photovoltaic device. The main parameters that characterize the solar cell outputs are [24] : the short circuit current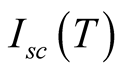 , the open-circuit voltage
, the open-circuit voltage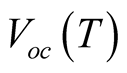 , and the diode saturation current
, and the diode saturation current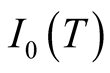 .
.
Such parameters depend principally on the cell temperature [24] .
The objective of the present trial is to find analytically the temperature of a solar cell subjected to incident global solar radiation and to reveal the variation of its efficiency along the local day time.
Indeed few theoretical trials [15] [16] are made to predict theoretically the cell temperature. These attempts are basically numerically oriented.
In the present work, we aim to find an analytical expression for cell temperature and its variation with the local day time by applying the integral Laplace transform technique as a well established method to solve the heating problem considering the cell as composed of two layers system, as a thin active film in perfect thermal contact with a bulk substrate. This problem is not easy to be solved due to the presence of a boundary condition of fourth kind at the interface between the two layers.
Then we have to correlate this function with the solar cell efficiency through the cell temperature-dependent parameters to reveal its efficiency variation along the solar day length.
2. Derivation of the Basic Equations
To set up the heating problems in a general form, it is assumed that the target solar cell consists of two layers (Figure 1).
The front layer (the film layer) is of finite thickness “ ”, deposited on a substrate layer of semi-infinite dimensions as shown. The temperature field within the film layer is
”, deposited on a substrate layer of semi-infinite dimensions as shown. The temperature field within the film layer is 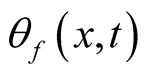 and that in the substrate layer in
and that in the substrate layer in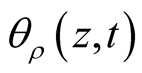 . The solar insulation
. The solar insulation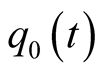 , W/m2 is assumed to fall in x-direction perpendicular to the front surface. It is partly absorbed and partly reflected. The absorbed part is
, W/m2 is assumed to fall in x-direction perpendicular to the front surface. It is partly absorbed and partly reflected. The absorbed part is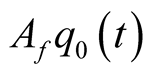 .
.
Where, 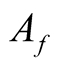 is the absorption coefficient at the surface of the front layer subjected to the incident solar radiation.
is the absorption coefficient at the surface of the front layer subjected to the incident solar radiation.
Two coincident coordinate axes, 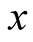 and
and 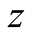 are considered. The substrate layer starts at
are considered. The substrate layer starts at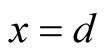 , or
, or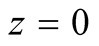 . To find
. To find 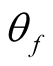 and
and 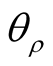 the heat diffusion equations for both layers are written as follows:
the heat diffusion equations for both layers are written as follows:
1) For the front layer:
 (1)
(1)
2) In the substrate:
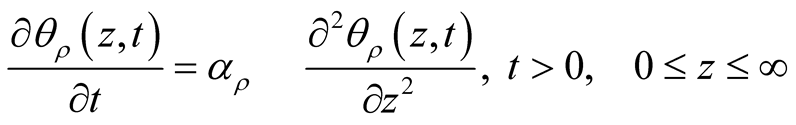 (2)
(2)
where, 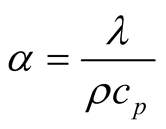 is the thermal diffusivity written in terms of the thermal conductivity
is the thermal diffusivity written in terms of the thermal conductivity 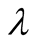 (= W/mK) and the heat capacity per unit volume
(= W/mK) and the heat capacity per unit volume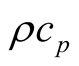 ,
,  is the density (kg/m3) and
is the density (kg/m3) and 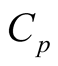 is the specific heat (J/kg·K).
is the specific heat (J/kg·K).
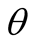 , represents the excess temperature
, represents the excess temperature 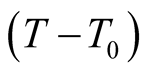 relative to the ambient temperature
relative to the ambient temperature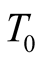 . The system of Equations (1), and (2) is subjected to the following initial and boundary conditions:
. The system of Equations (1), and (2) is subjected to the following initial and boundary conditions:
3)  (3)
(3)
4) 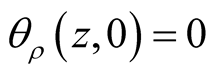 (4)
(4)
At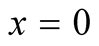 ,
,

Figure 1. A schematic diagram for the suggested system.
5) 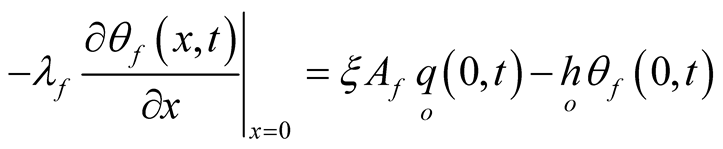 (5)
(5)
At the interface between both layers, the boundary condition of the forth kind is:
6) 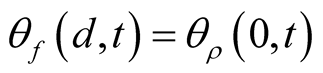 (6)
(6)
7) 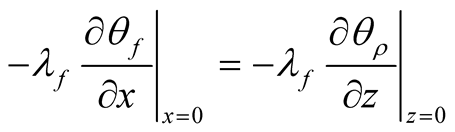 (7)
(7)
Moreover, the following condition is accepted:
8) 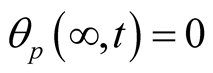 (8)
(8)
In addition, one has to consider the heat balance condition written in the form:
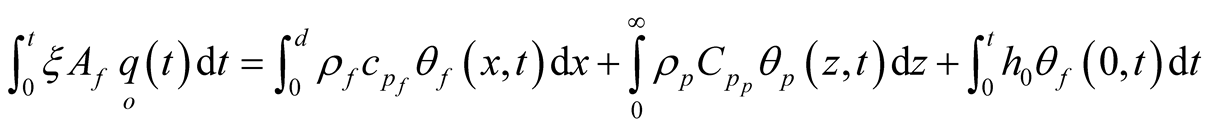 (9)
(9)
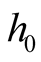 (W/m2 K) is the heat transfer coefficient at the front surface.
(W/m2 K) is the heat transfer coefficient at the front surface.
And  is the fraction of the solar energy absorbed at the front surface that is consumed in heating the cell and is not converted into photovoltaic power.
is the fraction of the solar energy absorbed at the front surface that is consumed in heating the cell and is not converted into photovoltaic power.
To solve the system of Equations (1), and (2), one has to apply the integral Laplace transform rules to the system and also to the initial and boundary conditions.
Reference [25] solved a laser heating problem similar to the problem considered in the present work. The fundamental difference between both works is that the laser irradiance is considered constant. Moreover cooling conditions are neglected in [25] . These factors are taken into consideration for the present problem.
The incident solar irradiance function 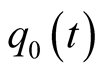 received on a horizontal surface is taken in the form published elsewhere by the authors of the present work [26] .
received on a horizontal surface is taken in the form published elsewhere by the authors of the present work [26] .
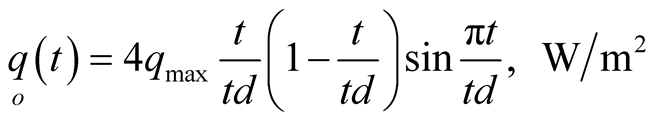 (10)
(10)
where 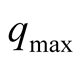 is the maximum value occurring at a local midday time
is the maximum value occurring at a local midday time .
.
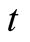 is the local day time starting from sunrise
is the local day time starting from sunrise 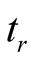 up to sunset
up to sunset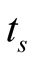 .
.
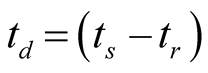 is the length of the solar day given as [27] .
is the length of the solar day given as [27] .
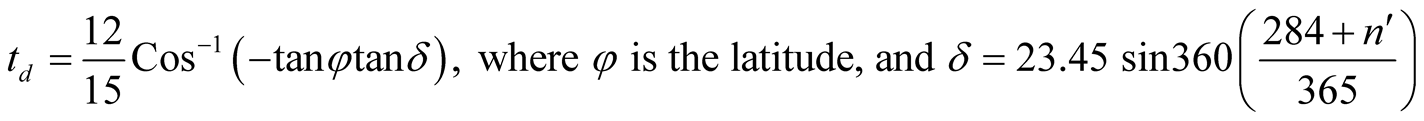 (11)
(11)
is the solar declination [27] .
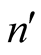 is the day of the year
is the day of the year 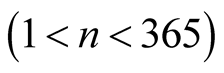 starting from 1 January. Taking Laplace transform with respect to time and following the same steps as in [25] , one can get finally the temperature fields in the form:
starting from 1 January. Taking Laplace transform with respect to time and following the same steps as in [25] , one can get finally the temperature fields in the form:
In the front layer:
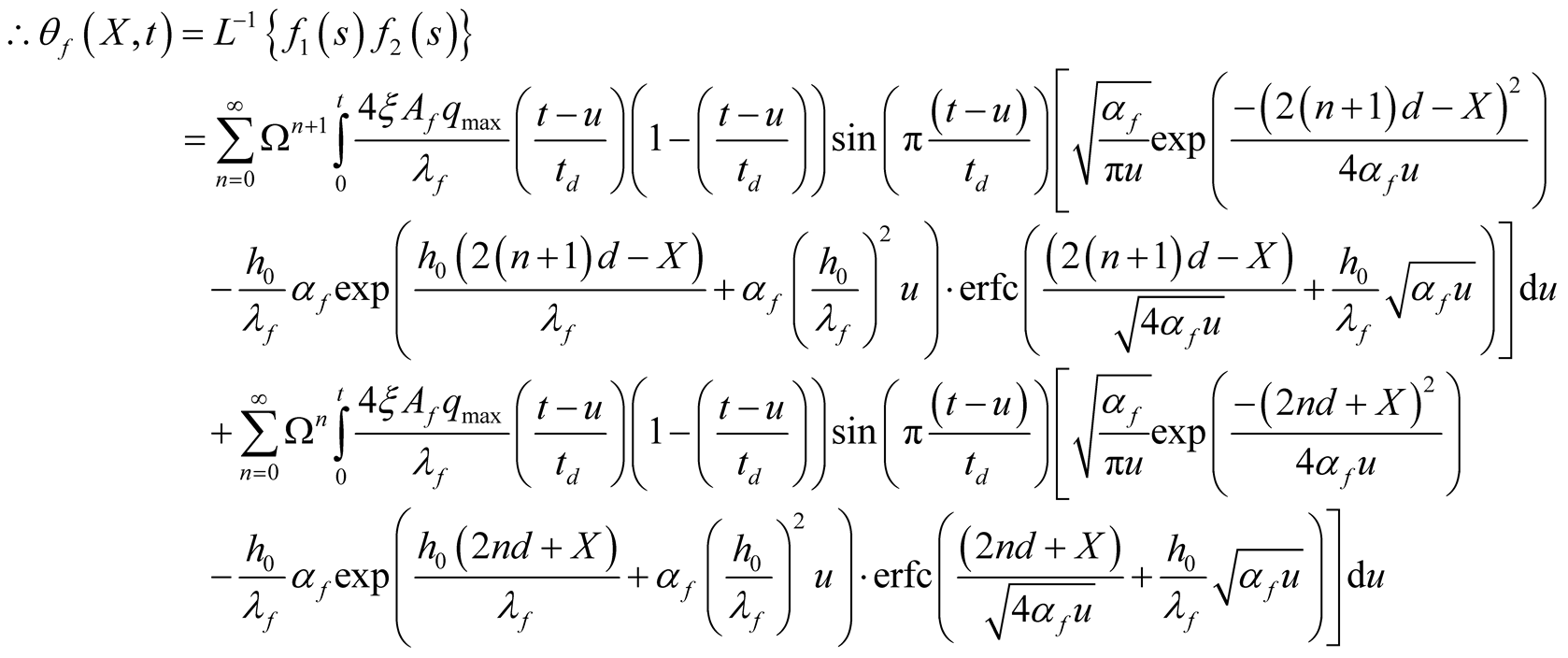 (12)
(12)
In the substrate:
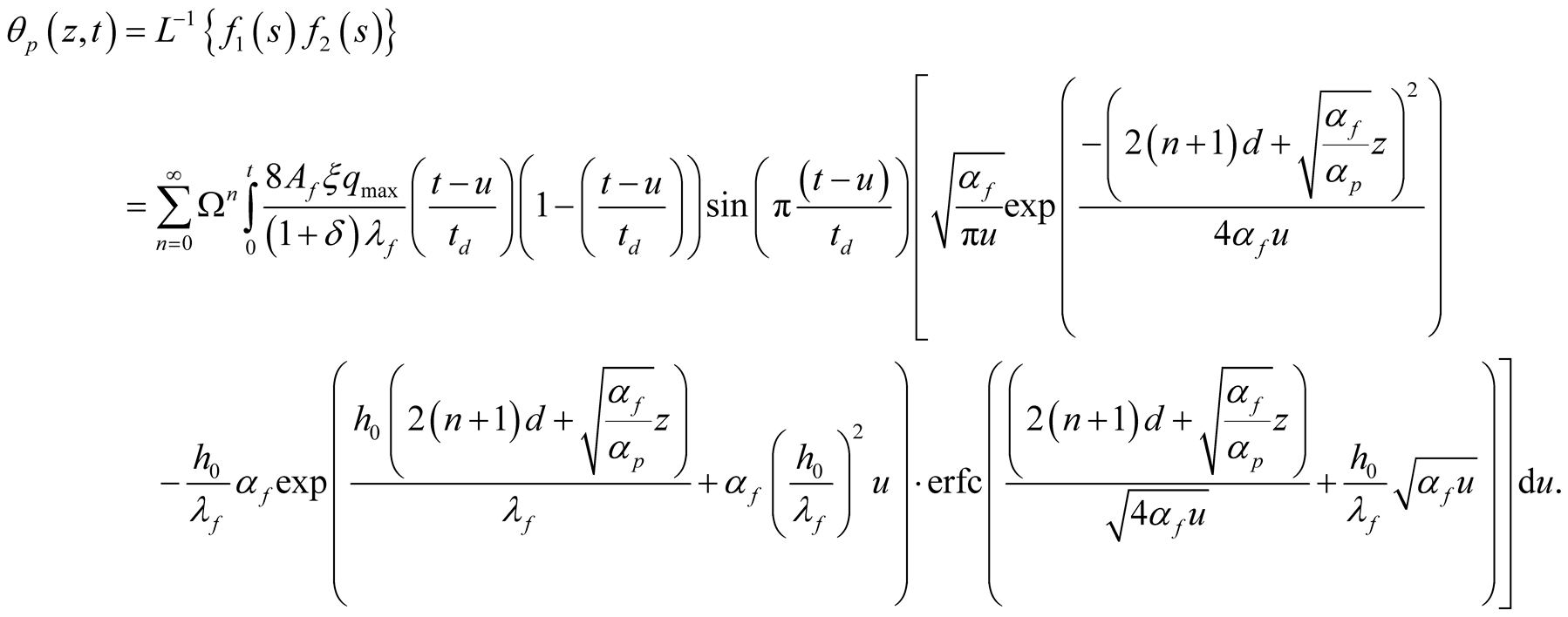 (13)
(13)
where,
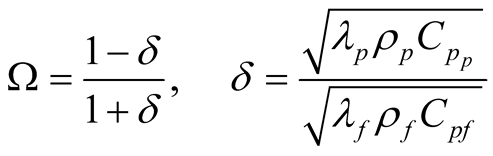 (14)
(14)
In the present work, data for 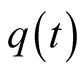 published in Jeddah [28] , is considered.
published in Jeddah [28] , is considered.
where , and
, and 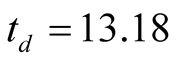 hours.
hours.
For a single layer system, one has to substitute  and
and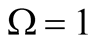 .
.
The following relations are also considered:
1) 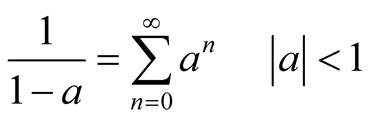 (15)
(15)
2) The convolution theory [29] :
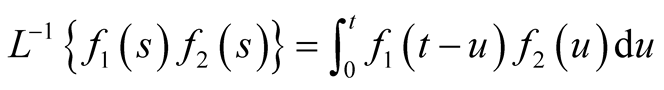 (16)
(16)
where 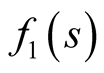 is the Laplace transform of the function
is the Laplace transform of the function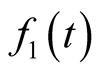 .
.
And 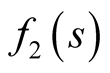 is the Laplace transform of the function
is the Laplace transform of the function .
.
3) The following inverse Laplace transforms [30] is considered:
 (17)
(17)
where, erfc º is the complementary error function.
In the following the variation of the cell efficiency as a function of its temperature is studied where:
The efficiency 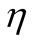 is defined as [23] :
is defined as [23] :
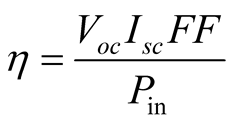 (18)
(18)
The physical quantities that are function of the temperature are indicated as:
 is the open-circuit voltage with an ideal value [23] :
is the open-circuit voltage with an ideal value [23] :
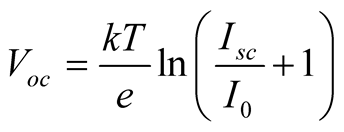 (19)
(19)
 —Boltzmann’s constant (
—Boltzmann’s constant (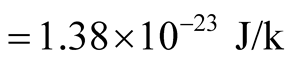 ).
).
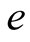 —is the electron charge (
—is the electron charge (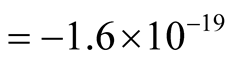 coulomb).
coulomb).
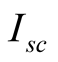 —is the short-circuit current, ideally this is equal to the light-generated current.
—is the short-circuit current, ideally this is equal to the light-generated current.
 is given as [31] .
is given as [31] .
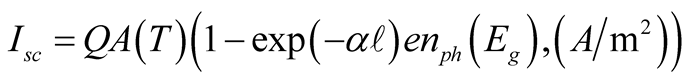 (20)
(20)
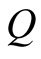 —is the collection efficiency (is taken as 0.8),
—is the collection efficiency (is taken as 0.8), 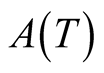 is the absorption coefficient for solid silicon at its surface and is given for the range T = 300˚K - 1685 ˚K by the relation [31] :
is the absorption coefficient for solid silicon at its surface and is given for the range T = 300˚K - 1685 ˚K by the relation [31] :
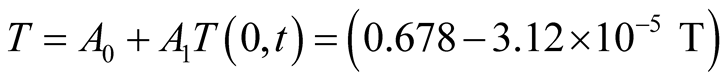 (21)
(21)
For an ionized donor impurity concentration of value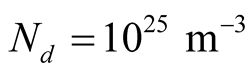 , the temperature variation of the attenuation coefficient a is given by the relation [31] :
, the temperature variation of the attenuation coefficient a is given by the relation [31] :
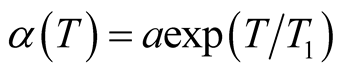 (22)
(22)
where, 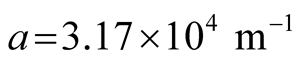 and T1 =346 ˚K.
and T1 =346 ˚K.
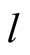 ,
, 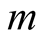 is the thickness of the absorbing semiconductor bulk.
is the thickness of the absorbing semiconductor bulk.
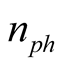 ,
, 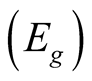 , is the number of photons with energy greater than the band gap
, is the number of photons with energy greater than the band gap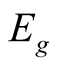 .
.
Moreover,  , the diode saturation current is given as in [23] [32] .
, the diode saturation current is given as in [23] [32] .
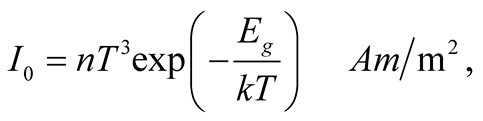 (23)
(23)
where,  is the non-ideality factor, this factor is taken as unity.
is the non-ideality factor, this factor is taken as unity.
Besides, one has to consider the dependence of the band gap on the temperature expressed as in [32] :
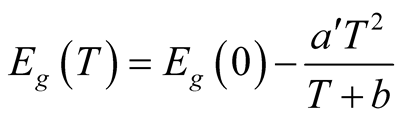 (24)
(24)
The values of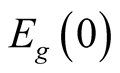 ,
, 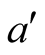 and
and 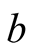 for silicon [32] are:
for silicon [32] are:
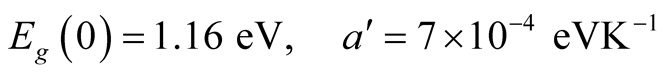 , b = 1100 ˚K.
, b = 1100 ˚K.
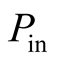 is the received incident solar irradiance W/m2.
is the received incident solar irradiance W/m2.
3. Computations
As an illustrative example, a single layer  solar silicon cell of thickness 0.02 m is considered. Its temperature is computed, at different values of the local day time,
solar silicon cell of thickness 0.02 m is considered. Its temperature is computed, at different values of the local day time, 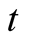 , the temperature at
, the temperature at 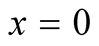 for simplicity is accepted as the cell temperature, rather than taken an average value along its thickness.
for simplicity is accepted as the cell temperature, rather than taken an average value along its thickness.
Corresponding to each value 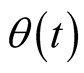 of the cell, one has to compute all the physical quantities required to estimate its efficiency
of the cell, one has to compute all the physical quantities required to estimate its efficiency 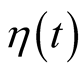 namely,
namely, 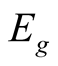 ,
, 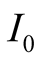 ,
, 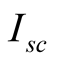 ,
,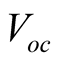 . These functions are given in Table 1 the efficiency
. These functions are given in Table 1 the efficiency 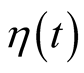 as a function of the local day time is illustrated as graphically in Figure 2.
as a function of the local day time is illustrated as graphically in Figure 2.
Table 1. The efficiency h as a function of local day time.
The cell temperature as a function of the local day time for three different values of the heat transfer coefficients 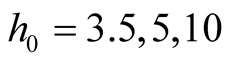 W/m2K, limiting the cooling conditions, are given in Table 2, Table 3, and Table4
W/m2K, limiting the cooling conditions, are given in Table 2, Table 3, and Table4
The effect of the different cooling levels on 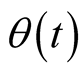 is shown graphically in Figure 3.
is shown graphically in Figure 3.
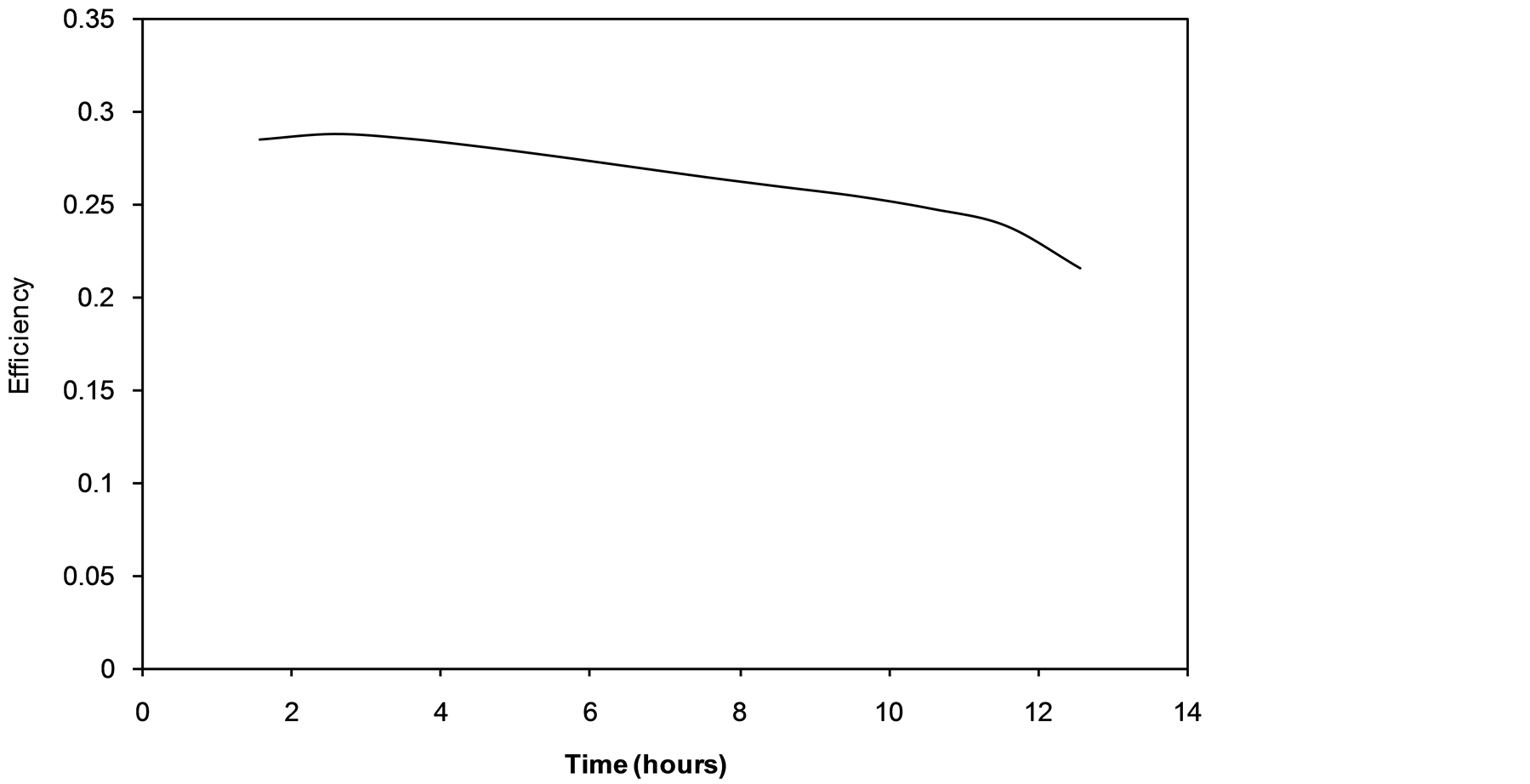
Figure 2. Efficiency as a function of local day time.
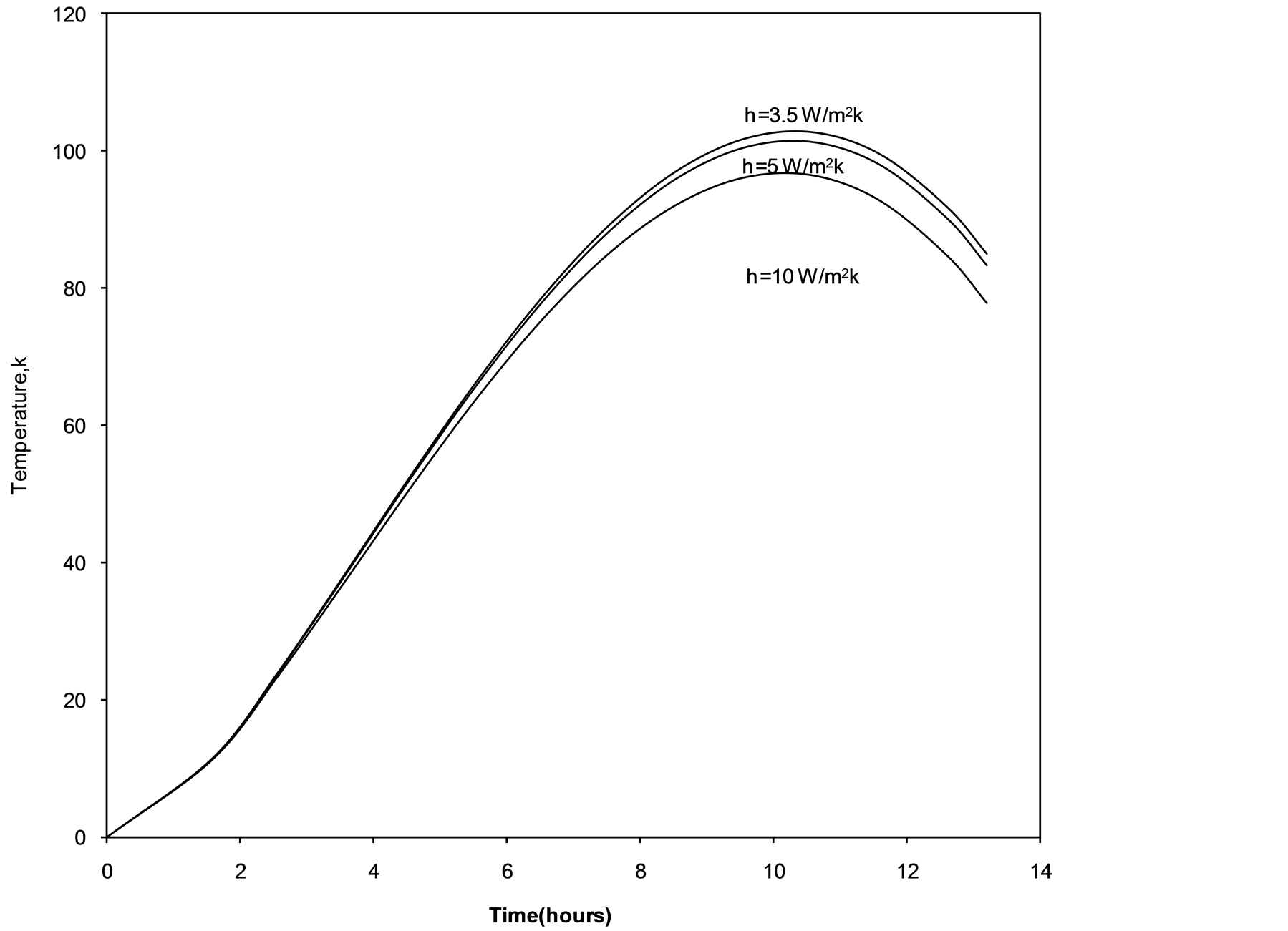
Figure 3. The cell difference temperature as a function of local day time with the coefficient of heat transfer (h), and the thickness (=0.02 m) as parameters.
Table 3. The cell difference temperature as a function of local day time with the coefficient of heat transfer (h0 = 5 W/m2 K) and the thickness d = 0.02 m, taken as parameters.
4. Conclusions
The obtained results reveal that:
1) The temperature variation of the solar cell with the local day time is significant within the considered operating conditions.
2) The cooling conditions slightly affect the temperature of the cell.
3) The efficiency of the cell varies slowly with the local day time.
4) Computations reveal that the efficiency 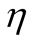 ranges between 21% - 28% for the considered silicon cell.
ranges between 21% - 28% for the considered silicon cell.
Table 4. The cell difference temperature as a function of local day time with the coefficient of heat transfer (h0 = 10 W/m2K) and the thickness d = 0.02 m, taken as parameters.
5) As the solar cell temperature increases its efficiency decreases. Thus shading and cooling conditions may be useful to increase its efficiency.
References
- Joseph, J.W. and Paul, R. (1960) Effect of Temperature on Photovoltaic Solar Energy Conversion. Journal of Applied Physics, 31, 571-578. http://dx.doi.org/10.1063/1.1735630
- Blakers, A.W. and Green, M.A. (1986) 20% Efficiency Silicon Solar Cells. Applied Physics Letters, 48, 20. http://dx.doi.org/10.1063/1.96799
- Olsen, L.C., Huber D.A., Dunham, G. and Addis, F. (1990) PC-ID Modeling of Depletion Layer Recombination in GaAs Solar Cells, IEEE, 1,415-419.
- Verlinden, P.J., Swanson, R.M., Sinton, R.A., Crane R.A., Tilford, C., Perkins, J. and Garrison, K. (1993) High-Efficiency, Point-Contact Silicon Solar Cells for Fresnel Lens concentrator Modules, IEEE, 58-64.
- Ray, K.P., Mullen, E.G. and Trumble, T.M. (1993) Results from the High Efficiency Solar Panel Experiment Flown on CREES. IEEE, Transactions on Nuclear Science, 40, 1505-1511.
- Zhao, J., Wang, A., Altematt, P.P., Wenham, S.R. and Green, M.A. (1994) 24% Efficient Silicon Solar Cells. IEEE, 2, 1477-1480.
- Verlinden, P.J., Swanson, R.M. and Crane, R.A. (1994) 7000 High-Efficiency Cells for a Dream. Progress in Photo Voltaics: Research and Applications, 2, 143-152. http://dx.doi.org/10.1002/pip.4670020209
- Venkatasubramanian, R., Malta, D.P., Timmons, M.L., Posthill, J.B., Hutchby, J.A., Ahrekiet, A., Keyes, B. and Wangensteen, T. (1994) IEEE, 1692-1696.
- Wettling, W. (1995) High Efficiency Silicon Solar Cells; State of the Art and Trends. Solar Energy Materials and Solar Cells, 38, 487-500. http://dx.doi.org/10.1016/0927-0248(94)00240-1
- Bouazzi, A.S., Abaab, M. and Rezig, B. (1997) A New Model of Very High Efficiency Buried Emitter Silicon Solar Cell. Solar Energy Materials and Solar Cells, 46, 29-41. http://dx.doi.org/10.1016/S0927-0248(96)00091-8
- Yerokhov, V.Y., Melnyk, I.I. and Korovin, A.V. (1999) External Bias as the Factor of Efficiency Increase of Silicon MIS/IL Solar Cells. Solar Energy Materials and Solar Cells, 58, 225-236. http://dx.doi.org/10.1016/S0927-0248(98)00206-2
- Luque, V.D. and del Valle, C.A. (1999) On the Effects of Tilted Light in a Global Prediction of AlGaAs/GaAs Solar Cell Performance. Solar Energy Materials and Solar Cells, 57, 313-322. http://dx.doi.org/10.1016/S0927-0248(98)00188-3
- Luque, A. and Marti, A. (1999) Limiting Efficiency of Coupled Thermal and Photovoltaic Converters. Solar Energy Materials and Solar Cells, 58, 147-165. http://dx.doi.org/10.1016/S0927-0248(98)00199-8
- Ferekides, C.S., Marinskiy, D., Viswanathan, V., Teltali, B., Palekis, V., Selvaraj, P. and Morel, D.L. (2000) High Efficiency CSS CdTe Solar Cells. Thin Solid Films, 361-362, 520-526. http://dx.doi.org/10.1016/S0040-6090(99)00824-X
- Laugier, A. and Roger, J.A. (1994) Les Photopiles Solaires. Part 2, Charpter 8, Technique and Documentation, Paris.
- Bouzek, K. and Kavan, L. (1999) Heat Losses in Gratzel Solar Cells. Solar Energy Materials and Solar Cells, 57, 359-371. http://dx.doi.org/10.1016/S0927-0248(98)00189-5
- El-Adawi, M.K. and Al-Nuaim, I.A. (2007) The Temperature Functional Dependence of Voc for a Solar Cell in Relation to Its Efficiency-New Approach. Desalination, 209, 91-96. http://dx.doi.org/10.1016/j.desal.2007.04.014
- El-Adawi, M.K. and Al-Shameri, N.S. (2012) The Efficiency of a p-n Solar Diode as a Function of the Recombination Velocity within the Depletion Layer. Optics and Photonics Journal, 2, 326-331. http://dx.doi.org/10.4236/opj.2012.24040
- El-Adawi, M.K. and Al-Shameri, N.S. (2012) The Efficiency of the Solar Converter as a Function of the Doping Degrees and the Incident Solar Spectral Photon Flux. Canadian Journal on Scientific and Industrial Research, 3, 112-122.
- Markvart, T. (1994) Solar Electricity. Unesco Energy Series, Charpter 7, John Wiley & Sons Ltd., UK.
- Stutenaumer, U., Negash, T. and Abdi, A. (1999) Performance of Small Scale Photovoltaic Systems and Their Potentials for Rural Electrification in Ethiopia. Renewable Energy, 18, 35-48. http://dx.doi.org/10.1016/S0960-1481(98)00784-8
- El-Adawi, M.K. and Al-Nuaim, I.A. (2010) The Temperature Variation of a Solar Cell in Relation to Its Performance. Journal of Environmental Science & Engineering, 4, 56-59.
- Green, M.A. (1982) Solar Cells: Operating Principles Technology and System Applications. Prentice-Hall, Englewood Cliffs.
- Glunz, S.W. (2006) New Concepts for High-Efficiency Silicon Solar Cells. Solar Energy Materials & Solar Cells, 90, 3276-3284. http://dx.doi.org/10.1016/j.solmat.2005.09.019
- El-Adawi, M.K., Abdelnaby, M.A. and Shalaby, S.A. (1995) Laser Heating of a Two-Layer System with Constant Surface Absorption: An Exact Solution. International Journal of Heat and Mass Transfer, 38, 947-952. http://dx.doi.org/10.1016/0017-9310(94)00162-O
- El-Adawi, M.K. and Al-Nuaim, I.A. (2001) Prediction of Diurnal Global Solar Irradiance on a Horizontal Surface General Approach. Proceedings of the 1st Saudi Science Conference, KFUPM, Saudi Arabia, 9-11 April 2001, 16, 63-78.
- Duffiee, J.A. and Beckman, W.A. (1974) Solar Energy Thermal Processes. John Wiley& Sons, New York.
- El-Bar, O. (1983) Energy Balance over Slant Surfaces. M.Sc. Thesis, Faculty of Meteorology and Environmental Science, King Abdul Aziz University, Jeddah.
- Spiegel, M.R. (1965) Laplace Transforms. Schaum’s Outline Series, McGraw-Hill, New York.
- Exkert, E.R.G. and Drake, R.M. (1972) Analysis of Heat and Mars Transfer. International Student Edition, Ch. 4, McGraw-Hill, Tokyo, 179.
- Bhattacharyya, A. and Streetman, B.G. (1981) Dynamics of Pulsed CO2 Laser Annealing of Silicon. Journal of Physics D: Applied Physics, 14, L67-L72. http://dx.doi.org/10.1088/0022-3727/14/5/002
- Tiwari, G.N. and Suneja, S. (1997) Solar Thermal Engineering Systems. Narosa Publishing House, London.



 ,
,  ,
, 