Optics and Photonics Journal
Vol.3 No.1(2013), Article ID:28877,5 pages DOI:10.4236/opj.2013.31003
Optical Characterization of Erbium Doped KY3F10 Fluoride Single Crystals
1University of El-Tarf, El-Tarf, Algeria
2Laboratory of Laser Physics, Optical Spectroscopy and Optoelectronics (LAPLASO), University of Annaba, Annaba, Algeria
Email: diafma@yahoo.fr
Received July 3, 2012; revised November 3, 2012; accepted November 10, 2012
Keywords: Solid State Laser; Laser Spectroscopy; Laser Materials; Fluoride; Rare Earth; KY3F10 Crystals; Judd-Ofelt Theory
ABSTRACT
Preparation and optical characterisation of Erbium doped KY3F10 single crystals are reported. The crystals were grown using the Czochralski pulling technique. The single phase was controlled by X-ray diffraction analysis. Room temperature optical spectra were recorded between 200 and 1600 nm. They were used to fit the three phenomenological Judd-Ofelt (JO) parameters Ωt (t = 2, 4, 6). The emission transition probabilities, branching ratios and radiative lifetimes are then deduced. The possibility to use such material for laser emissions is discussed.
1. Introduction
The fluoride single crystals were intensively studied during the last decade [1-8] in order to develop efficient and reliable all solid state lasers emitting in both visible and near infrared domain. The crystals studied are doped with rare earth ions which constitute the optically active centres by considering the 4f-4f transitions. Among all solidstate laser materials, the fluorides have the advantageous to be transparent in a large electromagnetic domain and they have low-maximum phonon frequency leading to a large number of potential emitting levels.
In this study, we present the optical absorption properties of KY3F10 laser material doped with trivalent Erbium ions. The Judd-Ofelt (JO) analysis was applied to this crystal in order to determine the optical transition probabilities, the branching ratio and the radiation lifetimes of the Erbium main emitting levels. The study seems to have not been done previously as far as we know. It enriches the literature and shows that the investigated crystal offers the possibility to generate a lot of emissions in the near infrared spectral range and especially the visible range.
2. Experimental Procedure
2.1. Material
The KY3F10 compound belongs to KF-YF3 pseudo-binary system. It melts congruently with a not very high melting point [9]. It crystallises in a face centred cubic structure having eight molecules per unit cell. The lattice is made up of two ionic groups (KY3F8)2+ and (KY3F12)2− which are alterned along orthogonal crystallographic directions [10,11]. All atoms occupy special crystallographic positions. Potassium and Yttrium ions are placed at 8c and 24e Wyckoff sites respectively. Fluorine ions occupy two different sites [12]. The Yttrium ions, for which are partially substituted the Er3+ ions, occupy a C4v tetragonal symmetry site. KY3F10 is an isotropic crystal.
2.2. Growth and Optical Techniques
Crystals of KY3F10 doped with different concentrations of Erbium ions were grown by Czochralski technique. We use three fluoride compounds: KF, YF3 and ErF3. These powder compounds with 4N (99.99%) purity are from Merck an Aldrich. They are finely crushed and weighed in the stoichiometric proportions and placed in a vitreous carbon crucible. We, first, heat the mixture under vacuum for 4 h with progressive temperature increase until 500˚C. Czochralski pulling takes place with a molybdenum rod under continuous argon circulation highly purified. The pulled crystals have more than 1 cm in diameter and 3 cm in length. Checked in polarized light, they are exempted of makles, crackles. They could be easily cut into laser bulk single crystals with high optical quality. The sample used for optical absorption measurements was polished to flat and parallel faces.
X-ray diffraction spectra confirm that KY3F10 single crystals have a cubic structure with a lattice parameter a = 11.55 Ǻ in good agreement with literature data [9,12, 13]. The Erbium ions which substitute Yttrium ions occupy C4v symmetry sites. The pulled crystals are of good optical quality. For spectroscopic measurements, the crystals were cleaved and polished in order to obtain parallel face samples with 2.65 mm thickness. The room temperature optical spectra were recorded with a PerkinElmer Lambda 9 double beam spectrophotometer. As KY3F10 is an isotropic crystal, the absorption spectra were obtained without using the birefringent polarizer of the used spectrophotometer.
3. Optical Spectroscopy
3.1. Judd-Ofelt Theory
In Judd-Ofelt approach [14,15], the measured electric dipole transition strengths  of the chosen transition
of the chosen transition 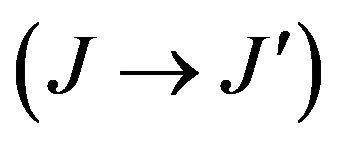 are determined using the following expression:
are determined using the following expression:
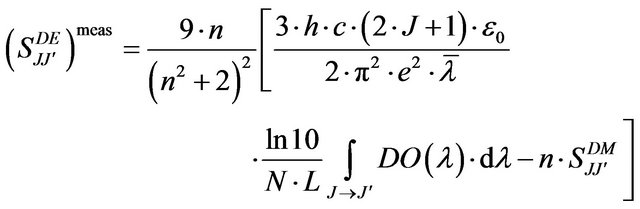 (1)
(1)
Where J and J' represent the total angular momentum quantum numbers of the initial and final levels, respectively, n the refractive index of the material, h the Planck constant, c the vacuum light celerity, ε0 the vacuum permittivity, e the electron charge, λ is the average wavelength of the 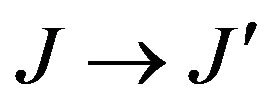 absorption transition, N the Er3+ concentration, L the thickness of the sample, DO(λ) the measured optical density and
absorption transition, N the Er3+ concentration, L the thickness of the sample, DO(λ) the measured optical density and  is the magnetic dipolar line strengths.
is the magnetic dipolar line strengths.
In the case of Er3+, many transitions have magnetic dipolar components [16].
The three J-O intensity parameters W2, W4 and W6 can be then calculated by solving the over determined set of equation given by the following equation:
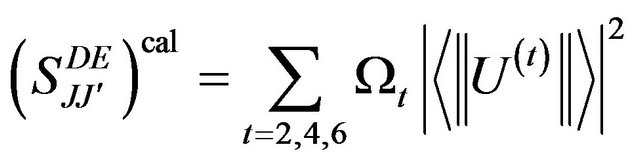 (2)
(2)
Where 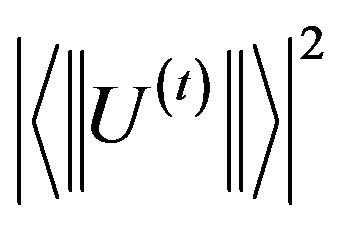 are the squared reduced matrix elements of rank t (t = 2, 4, 6) between the two multiplets characterized by the quantum number (S, L, J) and (S', L', J'). The matrix elements U(t) used in the present work were tabulated by Kaminski [17] for the Er3+ ions. However, when two absorption transitions are overlapped, the squared matrix element was taken to be the sum of the corresponding squared matrix elements.
are the squared reduced matrix elements of rank t (t = 2, 4, 6) between the two multiplets characterized by the quantum number (S, L, J) and (S', L', J'). The matrix elements U(t) used in the present work were tabulated by Kaminski [17] for the Er3+ ions. However, when two absorption transitions are overlapped, the squared matrix element was taken to be the sum of the corresponding squared matrix elements.
Thus, a series of calculation was carried out while varying the number of transitions used in the fitting procedure. The fitting accuracy of each calculation was evaluated from the root mean-square (rms) deviation between measured and calculated line strengths of the transitions.
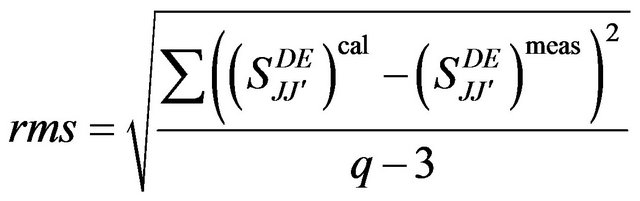 (3)
(3)
Where q is the number of spectral bands analyzed and 3 reflects the number of fitting parameters.
The J-O parameters are determined from the fitting procedure and they are used to calculate other transitions properties between ground and excited states of the Er3+ ion.
The radiative transition probabilities AJJ' for emissions from emitting levels [LS(J)] are given by [16]:
 (4)
(4)
Where the electric dipole line strengths (SDE) are calculated from Equation (2) while the magnetic dipole line strengths used in this work are reported in [16,18]. Then, the radiative lifetime t for an excited state (J) is calculated by:
 (5)
(5)
Where the summation of AJJ' terms is over all lower energy levels. The branching ratio bR is given by the equation:
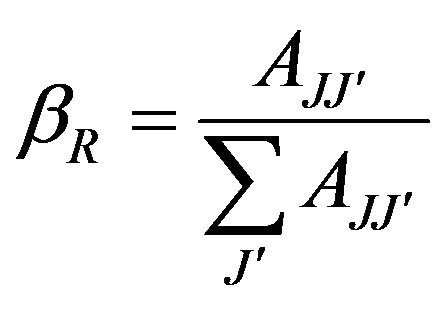 (6)
(6)
3.2. Data Analysis and Results
Room temperature optical spectra were recorded between 200 and 1600 nm. The observed spectra were independent of doping concentration in the range 0.1% - 1% molar concentration. Figure 1 shows the erbium energy levels.
The Judd-Ofelt theory, widely used to analyse the forced electric dipole transitions within the 4fn configuration of the rare earth ions and extensively introduced in the literature [19-23] is performed on Er3+ doped KY3F10. We have identified seven absorption bands around 518.85 nm, 553.86 nm, 574.35 nm, 685.28 nm, 829.31 nm, 973.26 nm and 1521.65 nm (Figure 2).
The observed bands are attributed, respectively, to the multiplets 4F7/2, 2H11/2, 4S3/2, 4F9/2, 4I9/2, 4I11/2 and 4I13/2 [5]. They are used in the fitting procedure. The three phenomenological obtained parameters, by the method of least-square fitting, are W2 = 1.932, W4 = 0.729 and W6 =
1.953 with rms = 0.345 (in 10−20 cm2∙units). These parameters are in accordance with those calculated for other fluoride hosts [24-28]. The spectroscopic quality factor χ = W4/W6 is approximately 0.37 which is in the range of

Figure 1. Energy level scheme of Erbium.
0.11 - 1.25. The calculated 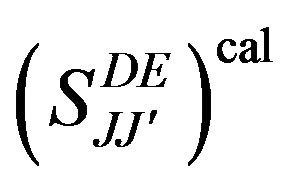 line strengths for all measured transitions were then deduced (Table 1). The computed transition probabilities and corresponding radiative lifetimes were then deduced and are given in Table 2.
line strengths for all measured transitions were then deduced (Table 1). The computed transition probabilities and corresponding radiative lifetimes were then deduced and are given in Table 2.
It is obvious that the branching ratio and the radiative lifetime are two critical parameters that can predict the possibility of laser emission. In the case of the infrared transition whose emission wavelength is centered around 1521 nm, the branching ratio is maximum and the radiative lifetime is nearly equal to 7 ms. Such a transition is investigated for eye safe laser. The level 4I13/2 is metastable level which may serve as reservoir level. The KY3F10 compound is serious contenders for eye-safe near infrared laser systems that can be efficiently pumped at 980 nm emitted by commercialized diode laser. Also, the fluorescence branching ratios for 2H11/2 → 4I15/2 and 4S3/2 → 4I15/2 transitions are 92% and 67% respectively which is large enough to be available to generate green light up-conversion emission. Therefore, Erbium doped KY3F10 crystal may be regarded as a potential solid-state Er3+ laser host material.

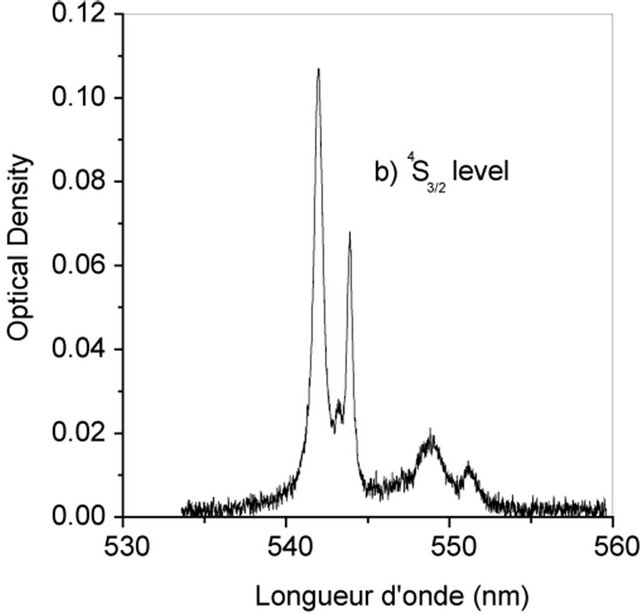
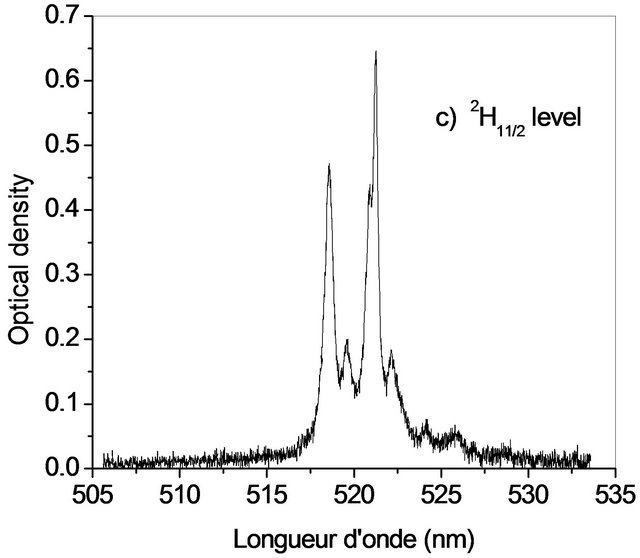
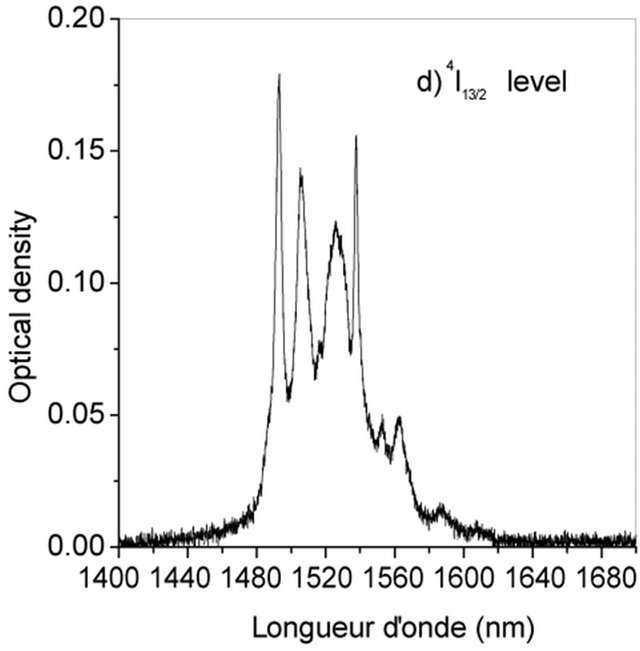
Figure 2. Room temperature absorption spectra of Er3+ doped KY3F10.
Table 1. Measured and calculated transition strengths for Er3+ ions in KY3F10 single crystal.
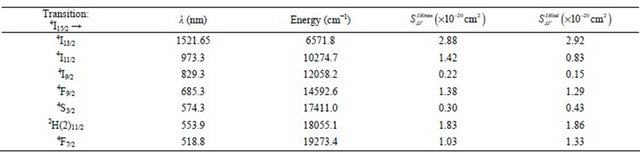
Table 2. Calculated electric and magnetic dipole emission probabilities, life times and branching ratios in KY3F10 doped with Erbium ions.

4. Conclusion
Er3+ doped single crystals were grown by Czochralski technique. The measured absorption line strengths at room temperature were used for a standard Judd-Ofelt analysis. The JO parameters were determined and they are in accordance with those calculated for other fluorid hosts. The radiative transition probabilities, radiative lifetimes and branching ratios of the main intermanifold transitions of Er3+ were calculated. Because of his low phonon energies, this crystal could permit up-conversion laser emissions. This study shows that Er3+ doped KY3F10 crystal possesses several competitive spectroscopic properties suggesting that this material can give rise to laser emissions in the near infrared spectral range (in the case of infrared transition 4I13/2 → 4I15/2) as well as in the visible (for the green transition 2H11/2, 4S3/2 → 4I15/2).
REFERENCES
- K. Heyde, K. Binnemans and C. Görller-Walrand, “Spectoscopic Properties of KY3F10:Er3+,” Journal of the Chemical Society, Faraday Transactions, Vol. 94, No. 12, 1998, pp. 1671-1674. doi:10.1039/a800610e
- J. P. R. Wells, A. Sugiyama, T. P. J. Han and H. G. Gallagher, “Laser Site Selective Excitation of KY3F10 Doped with Samarium,” Journal of Luminescence, Vol. 85, No. 1-3, 1999, pp. 91-102. doi:10.1016/S0022-2313(99)00150-7
- C. Borel and B. Viana, “Laser Hosts Emitting in the Range 1.5 - 2 µm,” Annales de Chimie Françaises, Vol. 20, 1995, pp. 227-232.
- M. Diaf, A. Braud, C. Labbé, J. L. Doualan, S. Girard, J. Margerie, R. Moncorgé and M. Thuau, “Synthesis and Spectroscopic Studies of Tm3+-doped KY3F10 Single Crystals,” Canadian Journal of Physics, Vol. 77, No. 9, 1999, pp. 693-697. doi:10.1139/cjp-77-9-693
- C. Wyss, W. Lüthy, H. P. Weber, P. Rogin and J. Hulliger, “Emission Properties of an Optimised 2.8 µm Er3+:YLF Laser,” Optics Communications, Vol. 139, No. 4-6, 1997, pp. 215-218. doi:10.1016/S0030-4018(97)00130-2
- M. Mujaji and J. P. R. Wells, “Spectroscopy and Crystal-Field Analysis of KY3F10:Ho3+,” Journal of Physics: Condensed Matter, Vol. 21, No. 25, 2009, pp. 255402- 255408 doi:10.1088/0953-8984/21/25/255402
- A. Braud, S. Girard, J. L. Doualan, M. Thuau and R. Moncorgé, “Energy Transfer Processes in Yb:Tm-Doped KY3F10, LiYF4 and BaY2F8 Single Crystals for Laser Operation at 1.5 and 2.3 µm,” Physical Review B, Vol. 61, No. 8, 2000, pp. 5280-5292. doi:10.1103/PhysRevB.61.5280
- A. Grzechnik, W. A. Crichton and J. Y. Gesland, “Sheelite CaWO4 at High Pressures,” Solid State Sciences, Vol. 5, No. 5, 2003, pp. 757-764. doi:10.1016/S1293-2558(03)00094-3
- B. Chai, J. Lefaucher, A. Pham, G. Lutts and J. Nichols, “Growth of High Quality Single Crystals of KYF4 by TSSG Method,” SPIE Proceedings, Vol. 131, 1993, p. 1863.
- M. Mortier, J. Y. Gesland, M. Rousseau, M. A. Pimenta, L. O. Laderia, J. C. Machado, D. Silva and G. A. Barbosa, “Raman Scattering Investigations of KY3F10,” Journal of Raman Spectroscopy, Vol. 22, No. 7, 1991, pp. 393-396. doi:10.1002/jrs.1250220706
- A. P. Ayala, M. A. S. Olivera, J. Y. Gesland and R. L. Moreira, “Electrical and Dielectric Investigations of the Conductions Processes in Crystals,” Journal of Physics: Condensed Matter, Vol. 10, No. 23, 1998, p. 5161. doi:10.1088/0953-8984/10/23/016
- J. W. Pierce and H. Y. P. Hong, “Laser Focus,” Proceedings of the Tenth Rare Earth Research Conference, Vol. 1, 1976, p. 527.
- R. Y. Abdulsabirov, M. A. Dubiniski, N. M. Kazakov, N. I. Silkin and S. I. Yagudin, “New Fluoride Laser Host,” Soviet Physics-Crystallography, Vol. 32, No. 4, 1987, p. 559.
- B. R. Judd, “Optical Absorption Intensities of Rare-Earth Ions,” Physical Review, Vol. 127, No. 3, 1962, pp. 750- 761. doi:10.1103/PhysRev.127.750
- G. S. Ofelt, “Intensities of Crystal Spectra of Rare-Earth Ions,” Journal of Chemical Physics, Vol. 37, No. 3, 1962, pp. 511-520. doi:10.1063/1.1701366
- W. T. Carnall, P. R. Fields and K. Rajnak, “Electronic Energy Levels in the Trivalent Lanthanide Aquo Ions, Pr, Nd, Sm, Dy, Ho, Er and Tm,” Journal of Chemical Physics, Vol. 49, No. 10, 1968, pp. 4412-4423. doi:10.1063/1.1669892
- A. A. Kaminskii, “Laser Crystals: Their Physics and Properties,” 2nd Edition, Springer-Verlag, Berlin, 1989.
- M. J. Weber, “Probabilities of Radiative and Non Radiaive Decay of Er3+ in LaF3,” Physical Review, Vol. 157, No. 2, 1967, pp. 262-272. doi:10.1103/PhysRev.157.262
- W. F. Krupke, “Radiative Transition Probabilities within the 4f3 Ground Configuration of Nd:YAG,” IEEE Journal of Quantum Electronics, Vol. 7, No. 4, 1971, pp. 153-159. doi:10.1109/JQE.1971.1076623
- W. F. Krupke, “Induced Emission Cross Sections in Neodymium Laser Glasses,” IEEE Journal of Quantum Electronics, Vol. 10, No. 4, 1974, pp. 450-457. doi:10.1109/JQE.1974.1068162
- M. J. Weber and T. E. Varitimos, “Optical Spectra and Intensities of Nd3+ in YAlO3,” Journal of Applied Physics, Vol. 42, No. 12, 1971, pp. 4996-5005. doi:10.1063/1.1659885
- R. Beach, M. D. Shinn, L. Davis, R. W. Solarz and W. F. Krupke, “Optical Absorption and Stimulated Emission of Neodymium in Yttrium Orthosilicate,” IEEE Journal of Quantum Electronics, Vol. 26, No. 8, 1990, pp. 1405- 1412. doi:10.1109/3.59689
- D. K. Sardar, R. C. Velarde-Montecinos, and S. Vizcarra, “Spectroscopic Properties of Nd3+ in CaF2,” Physica Status Solidi A, Vol. 136, 1993, pp. 555-560. doi:10.1002/pssa.2211360228
- K. Labbaci and M. Diaf, “Crystal Growth and Spectroscopic Investigations of Tm3+ Ions doped 5Na-9YF3 Fluoride Single Crystals,” Physica Scripta, Vol. 75, No. 3, 2007, pp. 27-330. doi:10.1088/0031-8949/75/3/018
- X. Li, Z. Lin, L. Zhang and G. Wang, “Growth and Spectroscopic Characterization of Er3+:NaY (MoO4)2 Crystal,” Journal of Crystal Growth, Vol. 293, No. 1, 2006, pp. 157-161. doi:10.1016/j.jcrysgro.2006.04.100
- A. M. Tkachuk, S. I. Klokishner, A. V. Poletimova and L. M. Petrove, “Probabilities of Intracenter Transitions and Self-Quenching of Luminescence in BaEr2F8 and BaHo2F8 Crystals,” Optics and Spectroscopy, Vol. 60, No. 6, 1986, pp. 745-751.
- D. K. Sardar, J. B. Gruber, B. Zandi, J. A. Hutchinson and C. W. Trussel, “Judd-Ofelt Analysis of Er3+ (4f11) Absorption Intensities in Phosphate Glass: Er3+, Yb3+,” Journal of Applied Physics, Vol. 93, No 4, 2003, pp. 2041-2046. doi:10.1063/1.1536738
- S. Hubert, D. Meichenin, B. W. Zhou and F. Auzel, “Emission Properties, Oscillator Strengths and Laser Parameters of Er3+ in LiYF4 at 2.7 μm,” Journal of Luminescence, Vol. 50, No. 1, 1991, pp. 7-15. doi:10.1016/0022-2313(91)90004-F

