New Journal of Glass and Ceramics
Vol.4 No.2(2014), Article ID:45416,4 pages DOI:10.4236/njgc.2014.42005
Analysis of Activation Energies & Experimental Evidence for Energetic Phase Separation in GexSe1−x Glassy System
Deepak Sharma1, Anand Mohan Awasthi2
1Applied Science Department, SCRIET, CCS University, Meerut, India
2UGC-DAE Consortium for Scientific Research, Indore, India
Email: deepak22phys@gmail.com
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 12 March 2014; revised 13 April 2014; accepted 19 April 2014
ABSTRACT
Glass science reveals peculiar properties due to the lack of long range order and presence of heterogeneity in Chalcogenide glasses. In thermal studies, structural relaxation at the glass transition region is governed by the activation energy of the cooperative unit (zU). In the cooperative molecular dynamics, we are considering the analysis of three activation energies, namely activation energy per BMS (U), activation energy of the cooperative unit (zU) and the apparent activation energy (z2U). From the energetic dynamics of activation energy analysis across the GexSe1−x glass series, data represent three-phase segregation. From our data, we also observed that the value of UCRR/RTg across the GexSe1−x glass series is nominally changed from 34.343 to 36.19.
Keywords:Activation Energies, Chalcogenide Glasses, Glass Transition, Heterogeneity, Molecular Dynamics, Structural Relaxation

1. Introduction
Structural relaxation is an atomic level rearrangement observed in most amorphous materials and Chalcogenide glasses below the crystallization temperature. Structural relaxation has an impact on optical [1] , mechanical [2] , and electronic transport properties [3] -[6] , and has been also reported for metallic glasses [7] . Amorphous semiconductor such as Si [8] , Ge [9] , and Chalcogenide glasses, their various elemental combinations are reported for application in electronic nonvolatile resistive memories based on phase change [5] [6] and in thermal transport properties. Nowadays the development of phase change memory technology led to the appearance of new materials. Chalcogenide glass semiconductors are one of these families due to their interesting properties. Structural relaxations in Chalcogenide glasses occur through cooperative rearranging region, which grow with decreasing temperature. Earlier studies on thermal annealing have shown the presence of significant defect annihilation during the structural relaxation process where point defects such as vacancies, dangling bond, and distorted bonds disappear at traps and recombine in pairs [8] . Our recent work has demonstrated unexpected nano-metric signals reported first time by Raman scattering in present GexSe1−x glasses [10] in place of Boson peak feature in Chalcogenide glasses [11] . The general nature of these excess vibration acoustic vs. optic is quite debated [12] -[16] . The purpose of the current work is to report experimental observations of three-phase separation from activation energies data analysis and nominally change in the values of UCRR/RTg in thermal studies by modulating differential scanning calorimetry.
2. Experimental Details
Chalcogenide glasses containing S/Se or Te constitute a rich family in combination with group IV elements. Intense research activities based on these glasses are due to technological applications in resistive phase change memory devices. GexSe1−x glasses were prepared by melt quenching technique, preparation details of glasses are mentioned in the article [10] [17] . Amorphous nature of the sample was verified by XRD, which shows no crystalline Bragg peaks. Glassy nature of the samples was confirmed by modulated differential scanning calorimetry MDSC (2910, TA Instruments). Glass transition temperature Tg was determined from the inflexion point of baseline shift in their heat capacity data, obtained at heating scan rate of 5˚C/min, and modulation of ±1˚C at 80 sec period [18] [19] .
3. Results & Discussion
Structural relaxation at the liquid glass transition has been studied for many years. It is commonly believed that glass transition is just to avoid the crystallization process. Glassy state is an extension of liquid state in which viscosity increases above ~1013 poise; it means that solid does not flow. Glass transition would then be kinetic in nature, a gradual freezing out of equivalent configurations. The value of glass transition (Tg) and the shape of specific heat depend on heating and cooling rates of the measurements and also on the thermal history of the sample [20] [21] . In general, it has observed with the data, position of glass transition temperature shift towards at high temperature, with high heating rates employed.
We have made numerous efforts to understand the nature of glass transition and relate Tg easily to some quantitative quantity which is UCRR/RTg. In the usual liquid regime above the melting point the isochoric activation energies are very small but the apparent activation energies grow dramatically in the supercooled regime. UCRR is the activation energy per basic molecular structural unit at cooperative rearranging region. The concept of cooperative molecular motion is useful to rationalize dramatic changes in the transport properties of liquids as they are cooled towards their glass transition. Mode coupling theory (MCT) [22] attributes slowing down particle motion at low temperature which eventually causes a structural arrest of the liquid dynamics. However, there has been no direct experimental observation of this kind of cooperative motion. It is natural to suppose that cooperative motion might be associated with dynamical heterogeneity. The intermolecular spatial correlation in the glassy state is determined by the collective rearrangement inside the glass transition zone. Correlation length ζ considered by Adam Gibb’s theory [23] characterizes this cooperative rearranging region in under cooled liquids. The “Arrhenius” activation energy of the basic molecular species is defined as
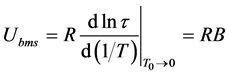
Ubms is identified with the potential barrier formed by the interaction of the molecule with its neighbors [24] [25] . Microscopically glass former is cooled; the identity and nature of dynamical entity undergo significant changes―from being atomic and fast tunneling, to dipolar/rigid polyhedral slowly liberating molecules to loosely connect molecular-clusters, sluggishly diffusing because of free volume [26] . This implies a successive collectivization of the basic atomic motion. Therefore, the assumption of single activation energy and time-scale oversimplifies the hierarchically evolving nature of microscopic glass dynamics.
Non-Arrhenicity ensues from the imperfect de-mixing of a-and β-relaxation modes, implying that the mole-
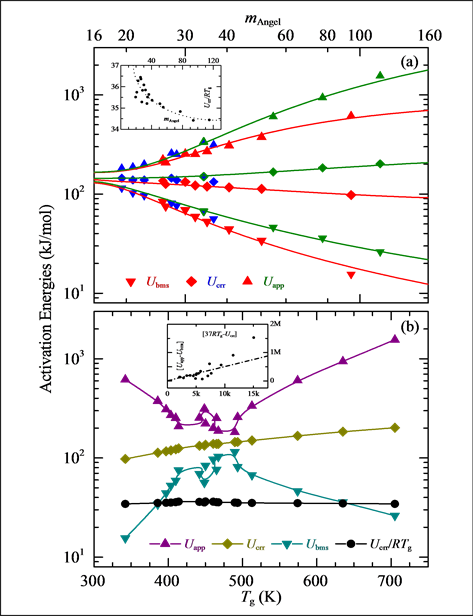
Figure 1. Behavior of three activation energies and energetic phase segregation.
cular dynamical units in the glass transition region are not independent (i.e., imprecisely defined). This imprecision gets embodied in the form of Vogel-Fulcher temperature dependence of the (atomic) relaxation time. The Vogel-Fulcher form is sometimes interpreted as super-Arrhenius in following manner 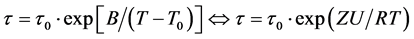 .
.
To describe the cooperative molecular dynamics one thus uses three activation energies viz., that of a basic molecular species Ubms = RB, cooperative unit Ucrr = ZRB, and the apparent one Uapp = Z2RB. Activation energies determined for the energetic characterization of GexSe1−x system are shown in Figure 1 against Tg and the glass fragility mAngel. The A-G factor Ucrr/RTg seen nominally constant, varies within 34.41 and 36.44! The concurrences  when
when 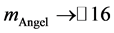 and
and ![]() (Figure 1(b) inset) provide (37-Ucrr/RTg) as the “energetic measure” of non-Arrhenicity/spatial uniformity.
(Figure 1(b) inset) provide (37-Ucrr/RTg) as the “energetic measure” of non-Arrhenicity/spatial uniformity.
References
- Kryshenik, V.M., Ivanitsky, V.P. and Kovtunenko, V.S. (2005) Irreversible Relaxations Transformations in Amorphous Chalcogenide. Journal of Optoelectronics and Advanced Materials, 7, 2953-2962.
- Kalb, J., Spaepen, F., Leervad Pedersen, T.P. and Wuttig, M. (2003) Viscosity and Elastic Constants of Amorphous Thin Films of Te Alloys Used for Optical Data Storage. Journal of Applied Physics, 94, 4908-4912. http://dx.doi.org/10.1063/1.1610775
- Kasap, S.O., Polischuk, B., Aiyah, V. and Yannacopoulos, S. (1990) Drift Mobility Relaxations in a-Se. Journal of Applied Physics, 67, 1918-1922. http://dx.doi.org/10.1063/1.345592
- Koughia, K., Shakoor, Z., Kasap, S.O. and Marshall, J.M. (2005) Density of Localized Electronic States in a-Se from Electron Time of Flight Photocurrent Measurements. Journal of Applied Physics, 97, 33706-33717. http://dx.doi.org/10.1063/1.1835560
- Pirovano, A., Lacaita, A.L., Pellizzer, F., Kostylev, S.A., Benvenuti, A. and Bez, R. (2004) Low Field Amorphous State Resistance and Thershold Voltage Drift in Chalcogenide Materials. Transactions on Electron Devices, 51, 714- 719. http://dx.doi.org/10.1109/TED.2004.825805
- Ielmini, D., Lacaita, A.L. and Mantegazza, D. (2007) Recovery and Drift Dynamics of Resistance and Thershold Voltages in Phase Change Memories. Transactions on Electron Devices, 54, 308-315. http://dx.doi.org/10.1109/TED.2006.888752
- Tiwari, G.P., Ramanujan, R.V., Gonalb, M.R., Prasad, R., Raj, P., Badguzar, B.P. and Goswami, G.L. (2001) Structural Relaxation in Metallic Glasses. Materials Science and Engineering A, 499, 304-306.
- Roorda, S., Sinke, W.C., Poate, J.M., Jacobson, D.C., Dierker, S., Dennis, B.S., Eaglesham, D.J., Spaepen, F. and Fuoss, P. (1991) Structural Relaxation and Defect Annihilation in Pure Amorphous Silicon. Physical Review B, 44, 3702-3725.
- Donovan, E.P., Spaepen, F., Turnbull, D., Poate, J.M. and Jacobson, D.C. (1985) Calorimetric Studies of Crystallization and Relaxation in Amorphous Silicon and Germanium Prepared by Ion Implantation. Journal of Applied Physics, 57, 1795-1804. http://dx.doi.org/10.1063/1.334406
- Sharma, D., Ingale, A. and Awasthi, A.M. (2005) Confined Acoustic Modes and Spectral Determination of Network Connectivity: Raman Signatures Of Nanometric Structure in g-GexSe1-x. Solid State Communications, 134, 653-658.
- Foret, M., Vacher, R., Courtens, E. and Monaco, G. (2002) Merging of the Acoustic Branch with the Boson Peak in Densified Silica Glasses. Physical Review B, 66, 024204-024210. http://dx.doi.org/10.1103/PhysRevB.66.024204
- Sokolov, A.P., Kisliuk, A., Soltwisch, M. and Quitmann, D. (1992) Medium Range Order in Glasses: Comparison of Raman and Diffraction Measurements. Physical Review Letters, 69, 1540-1543. http://dx.doi.org/10.1103/PhysRevLett.69.1540
- Masciovecchio, C., Mazzacurati, V., Monaco, G., Ruocco, G., Scopigno, T., Sette, F., Benassi, P., Consulo, A., Fontana, A., Krisch, M., Mermet, A., Montagna, M., Rossi, F., Sampoli, M., Signorelli, G. and Verbeni, R. (1999) Acoustic Nature of Boson Peak in Vitreous Silica. Philosophical Magazine Part B, 79, 2013-2020. http://dx.doi.org/10.1080/13642819908223089
- Fontana, A., Dell’Anna, R., Montagna, M., Rossi, F., Viliani, G., Ruocco, G., Sampoli, M., Buchenau, U. and Wischnewski, A. (1992) The Raman Coupling Function in Amorphous Silica and the Nature of the Long Wavelength Excitations in Disordered Systems. Europhysics Letters, 47, 56-62. http://dx.doi.org/10.1209/epl/i1999-00351-x
- Hehlen, B., Courtens, E., Vacher, R., Yamanaka, A., Kataoka, M. and Inoue, K. (2000) Hyper-Raman Scattering Observation of the Boson Peak in Vitreous Silica. Physical Review Letters, 84, 5355-5358. http://dx.doi.org/10.1103/PhysRevLett.84.5355
- Matic, A., Engberg, D., Masciovecchio, C. and Borjesson, L. (2001) Sound Wave Scattering in Network Glasses. Physical Review Letters, 86, 3803-3806. http://dx.doi.org/10.1103/PhysRevLett.86.3803
- Sharma, D., Sampath, S., Lalla, N.P. and Awasthi, A.M. (2005) Mesoscopic Organization and Structural Phases in Network Forming GexSe1-x Glasses. Physica B, 357, 290-298. http://dx.doi.org/10.1016/j.physb.2004.11.078
- Awasthi, A.M. and Sampath, S. (2001) Template Compositional Behavior of Relaxation Time Divergence in GexSe1-x. Materials Science and Engineering: A, 304-306, 476-479. http://dx.doi.org/10.1016/S0921-5093(00)01498-2
- Awasthi, A.M. and Sampath, S. (2002) Thermo Kinetic Anomalies across Rigidity Threshold in GexSe1-x. Materials Transactions, 43, 2046-2049. http://dx.doi.org/10.2320/matertrans.43.2046
- Moynihan, C.T., Macedo, P.B., Montrose, C.J., Gupta, P.K., Debolt, M.A., Dill, J.F., Dom, B.E., Drake, P.W., Esteal, A.J., Elterman, P.B., Moeller, R.P., Sasabae, H. and Wilder, J.A. (1976) Structural Relaxation in Vitreous Materials. Annals of the New York Academy of Sciences, 279, 15-35. http://dx.doi.org/10.1111/j.1749-6632.1976.tb39688.x
- Moynihan, C.T., Esteal, A.J., Wilder, J. and Tucker, J. (1974) Dependence of the Glass Transition Temperature on Heating and Cooling Rate. Journal of Physical Chemistry, 78, 2673-2677. http://dx.doi.org/10.1021/j100619a008
- Götze, W. and Sjogren, L. (1996) Comments on the Mode Coupling Theory for Structural Relaxation. Chemical Physics, 212, 47-59. http://dx.doi.org/10.1016/S0301-0104(96)00094-8
- Adam, G. and Gibbs, J.H. (1965) On the Temperature Dependence of Cooperative Relaxation Properties in Glass Forming Liquids. Journal of Chemical Physics, 43, 139-146. http://dx.doi.org/10.1063/1.1696442
- McCrum, N.G., Read, B.E. and Williams, G. (1987) Anelastic and Dielectric Effects in Polymeric Solids. Wiley, New York.
- Ferry, J.D. (1980) Visco-Elastic Properties of Polymers. Wiley, New York.
- Doliwa, B. and Heuer, A. (2002) How Do Dynamic Heterogeneities Evolve in Time? Journal of Non-Crystalline Solids, 307-310, 32-39. http://dx.doi.org/10.1016/S0022-3093(02)01437-0

